A New Voltage-Multiplier-Based Power Converter Configuration Suitable for Renewable Energy Sources and Sustainability Applications
Abstract
:1. Research Gap and Introduction
- This study presents a novel switched-capacitor- and switched-inductor-based DC-DC boost converter.
- The proposed converter is suitable for high-gain PV-based grid applications, with two SL cells at the input side and a voltage-doubler CP cell at the output side in its topology.
- At the same time, the SL blocks make the converter capable of decreasing the input current ripples and increasing the input voltage.
- The SC-cell simply doubles the generated voltage by the boost converter. At the same time, this cell mitigates the voltage stress across the switches in boost configuration.
- A simple control technique is applied to converter switches. On the input side, both switches are derived synchronously, and the boost switch is activated for the time intervals that the input switches are deactivated.
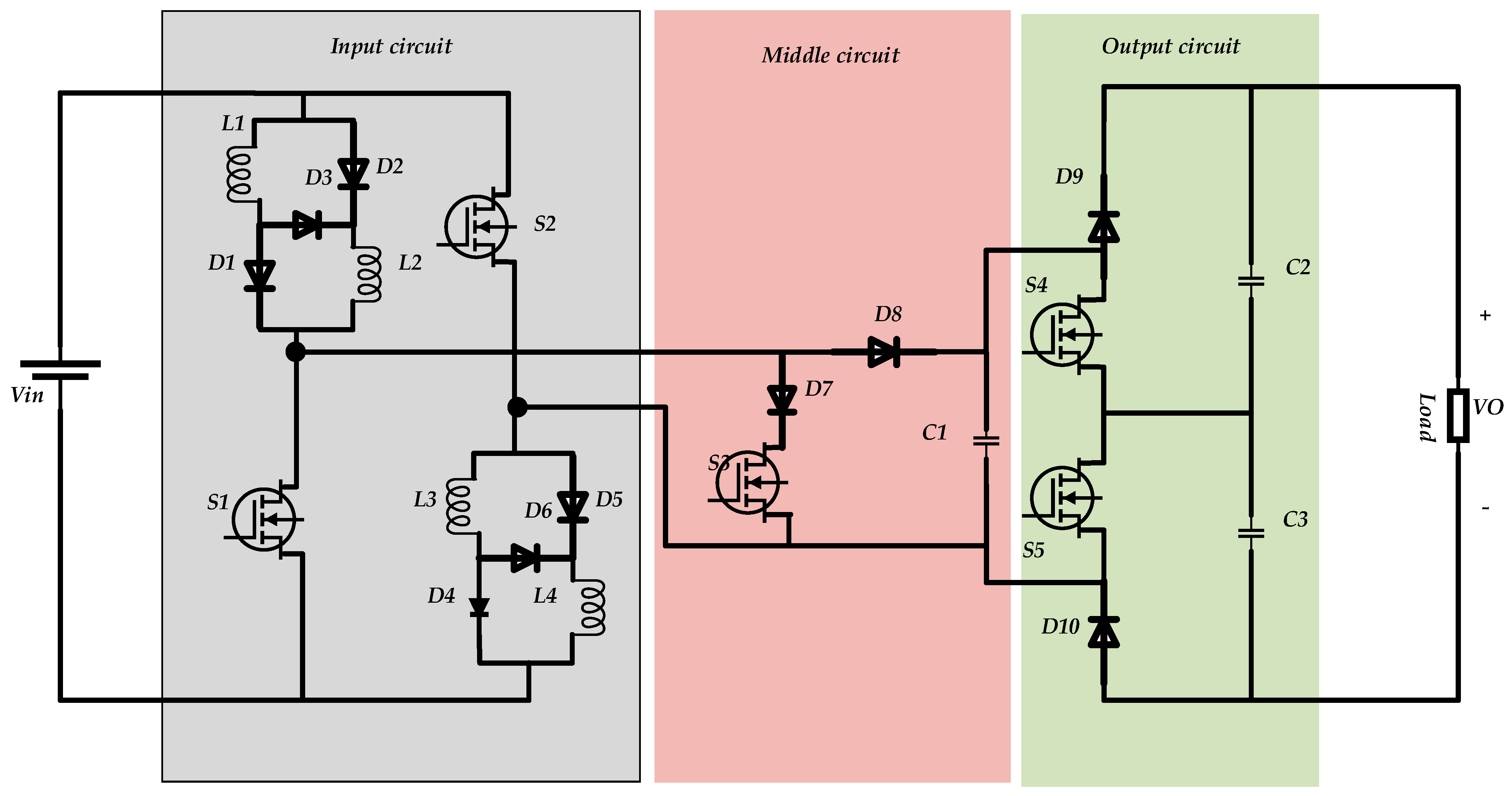
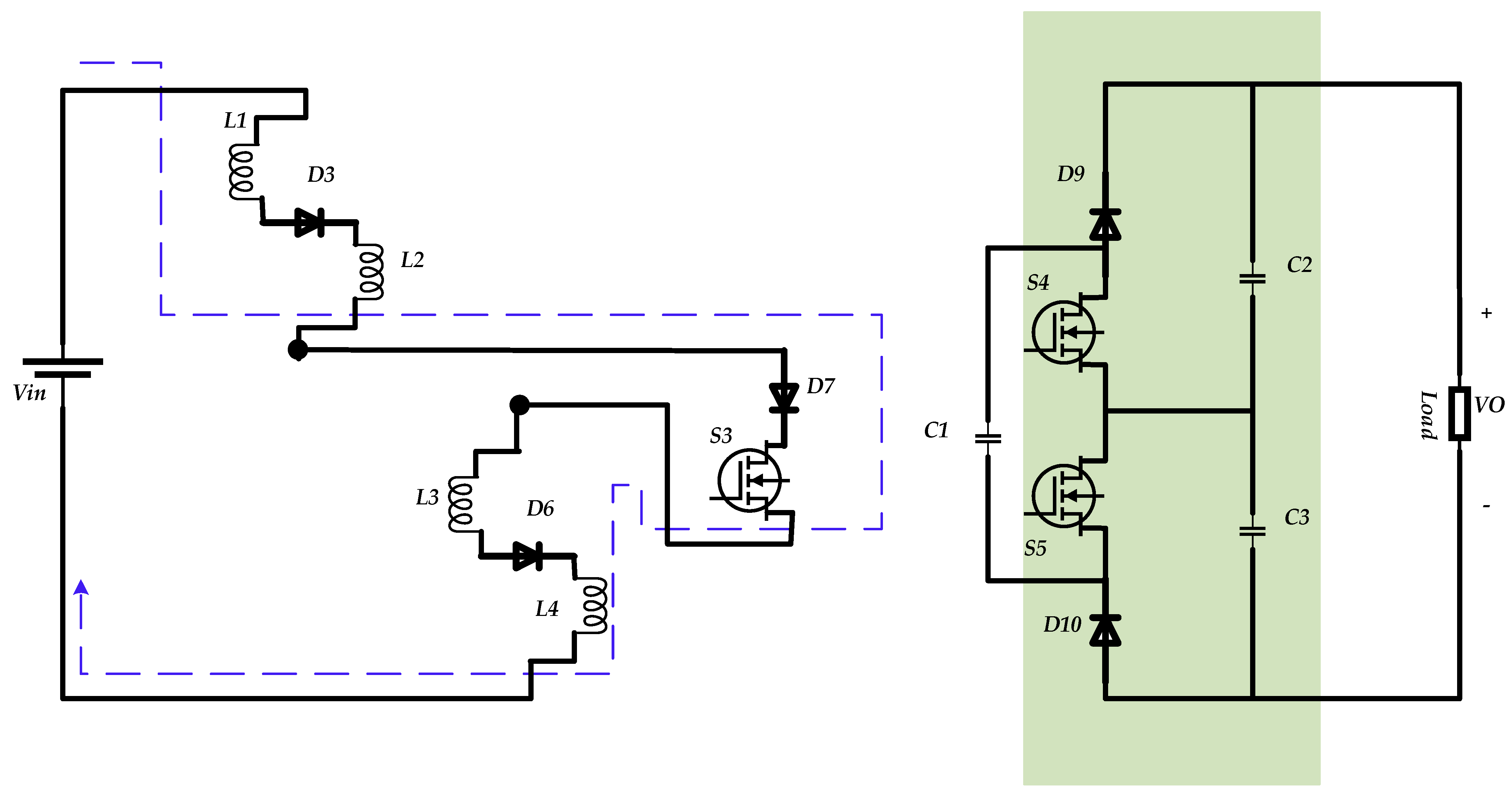
2. Description of the Proposed Circuit
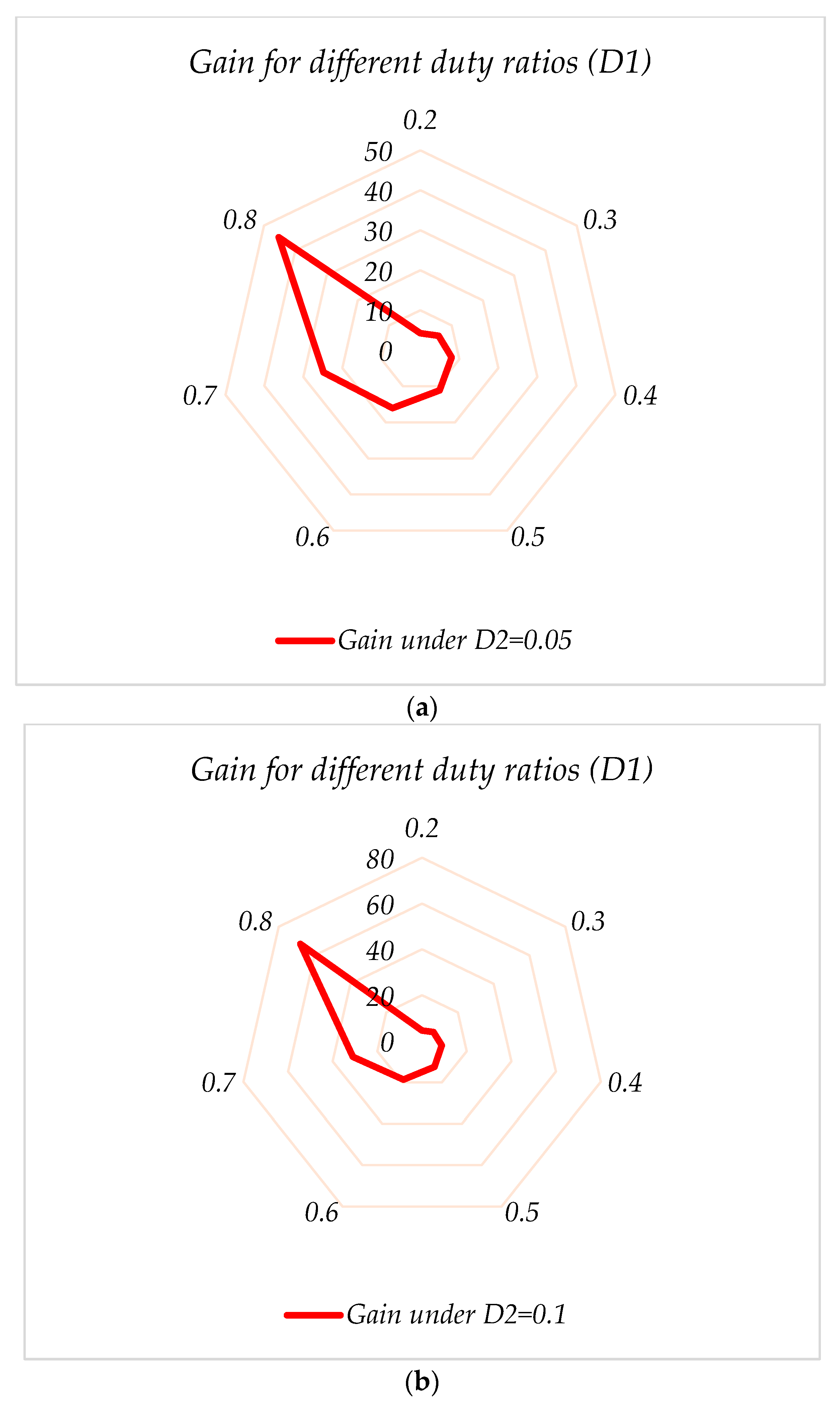
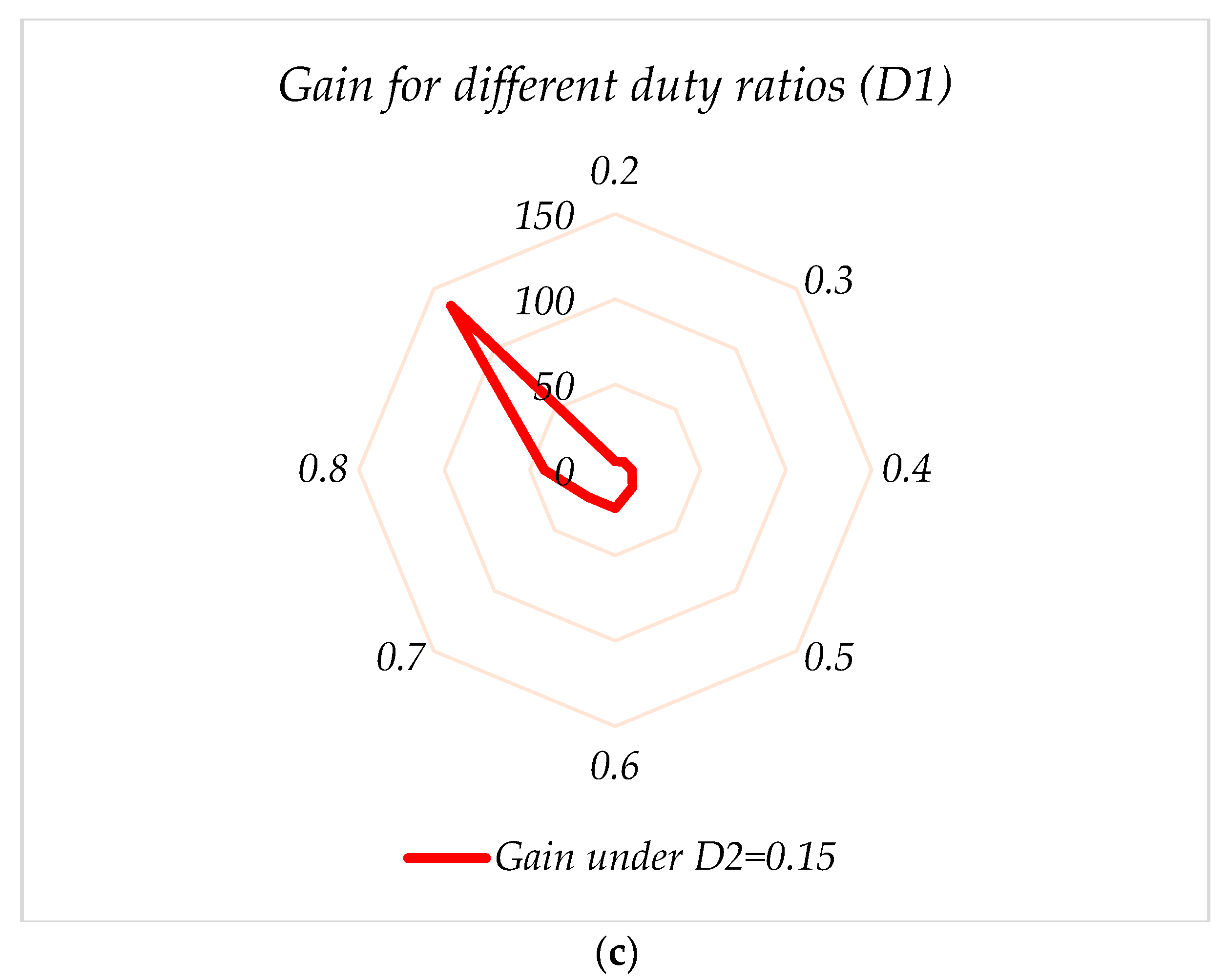
2.1. Working Modes of the Converter and Gain Calculations
2.2. Voltage Stresses across the Semiconductor Devices
2.3. Current Stresses of the Inductors
2.4. Capacitor Design
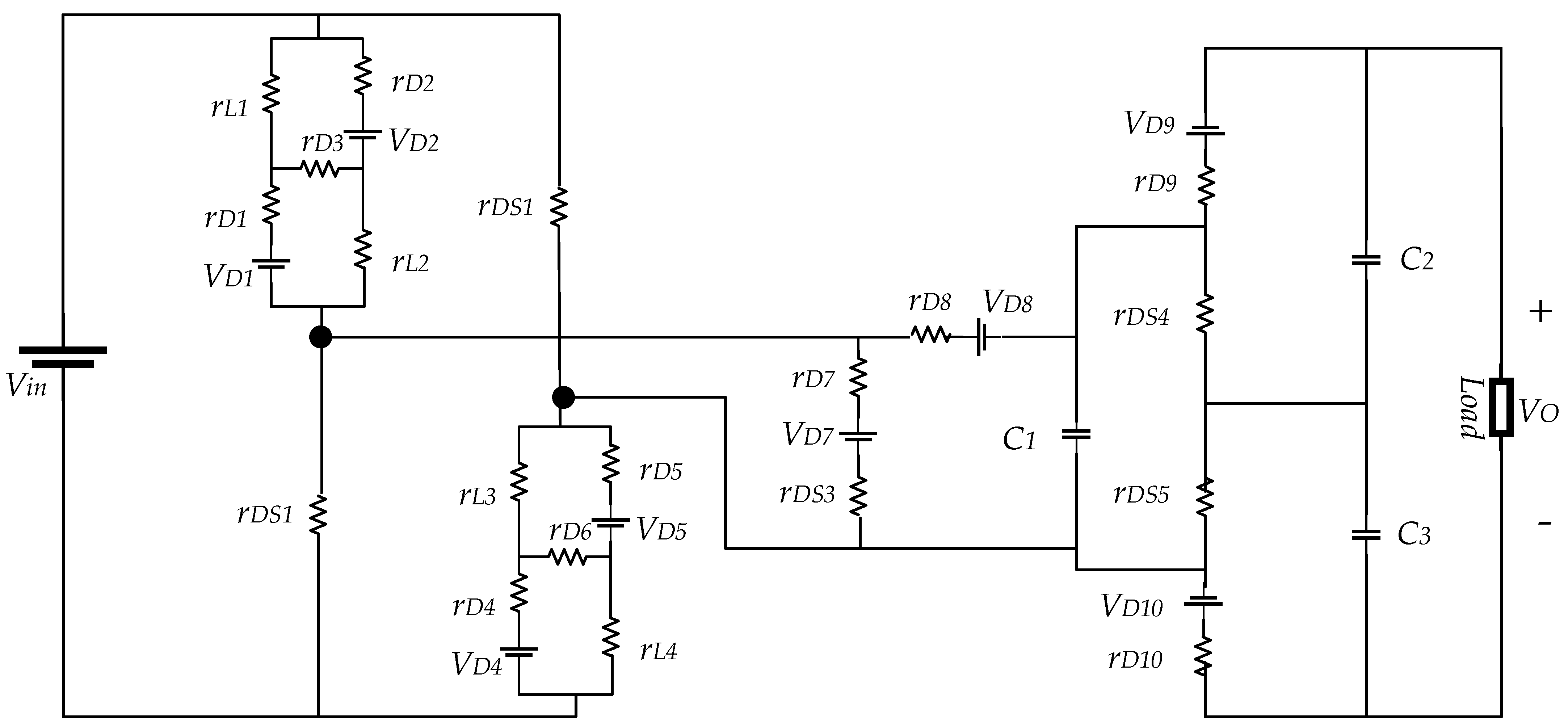
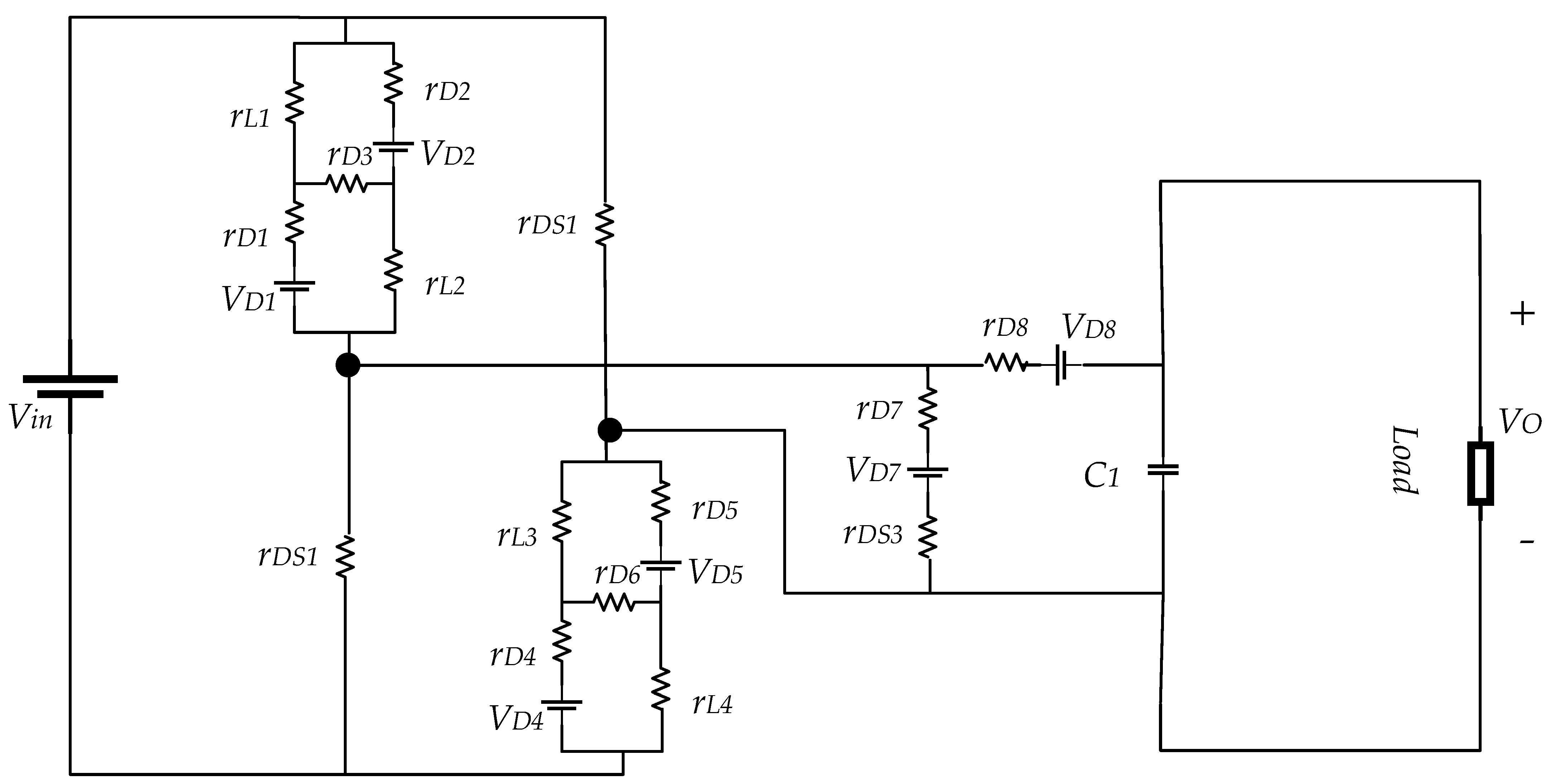
3. Working with Non-Ideal Components (Real Condition)
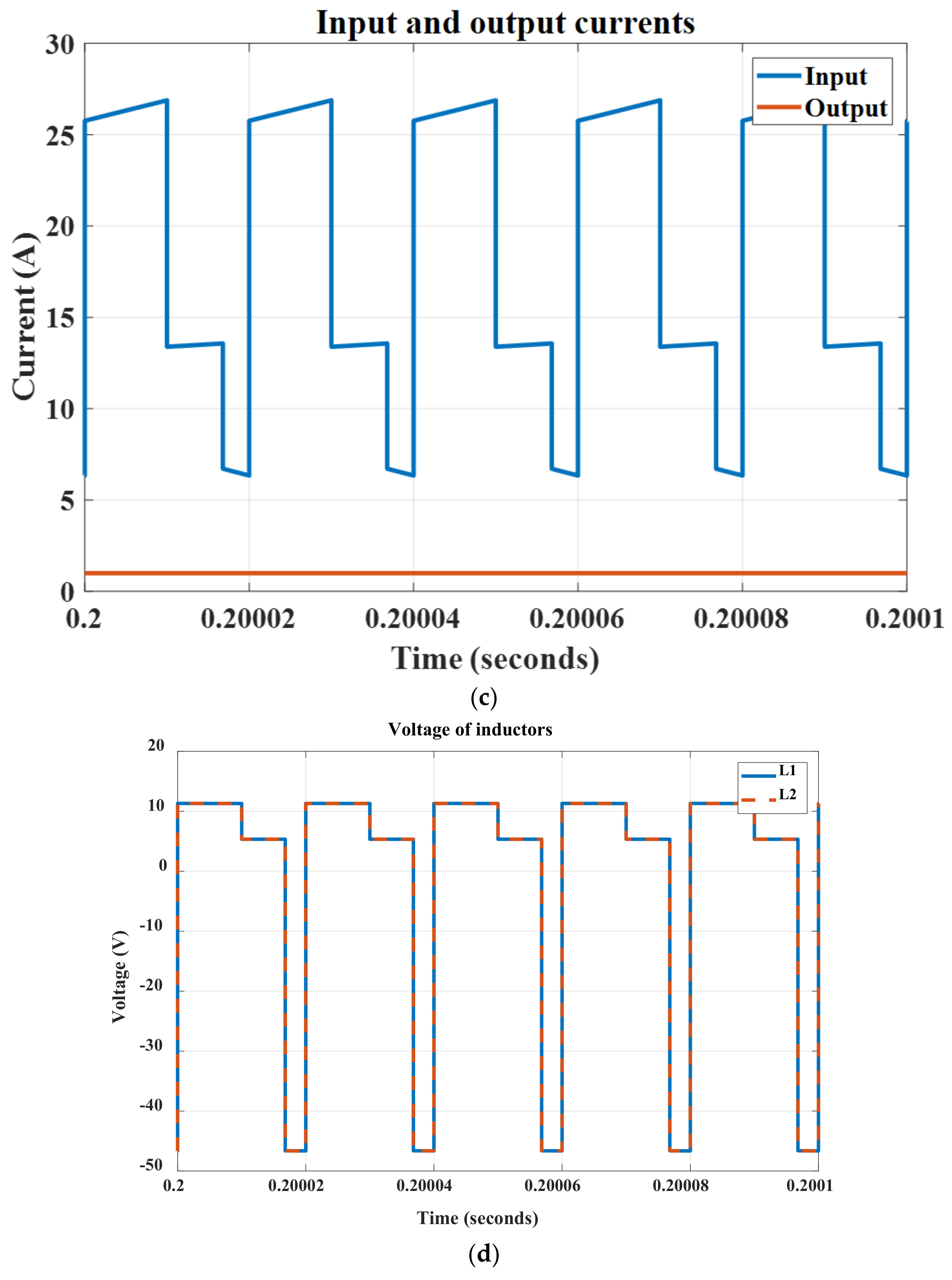
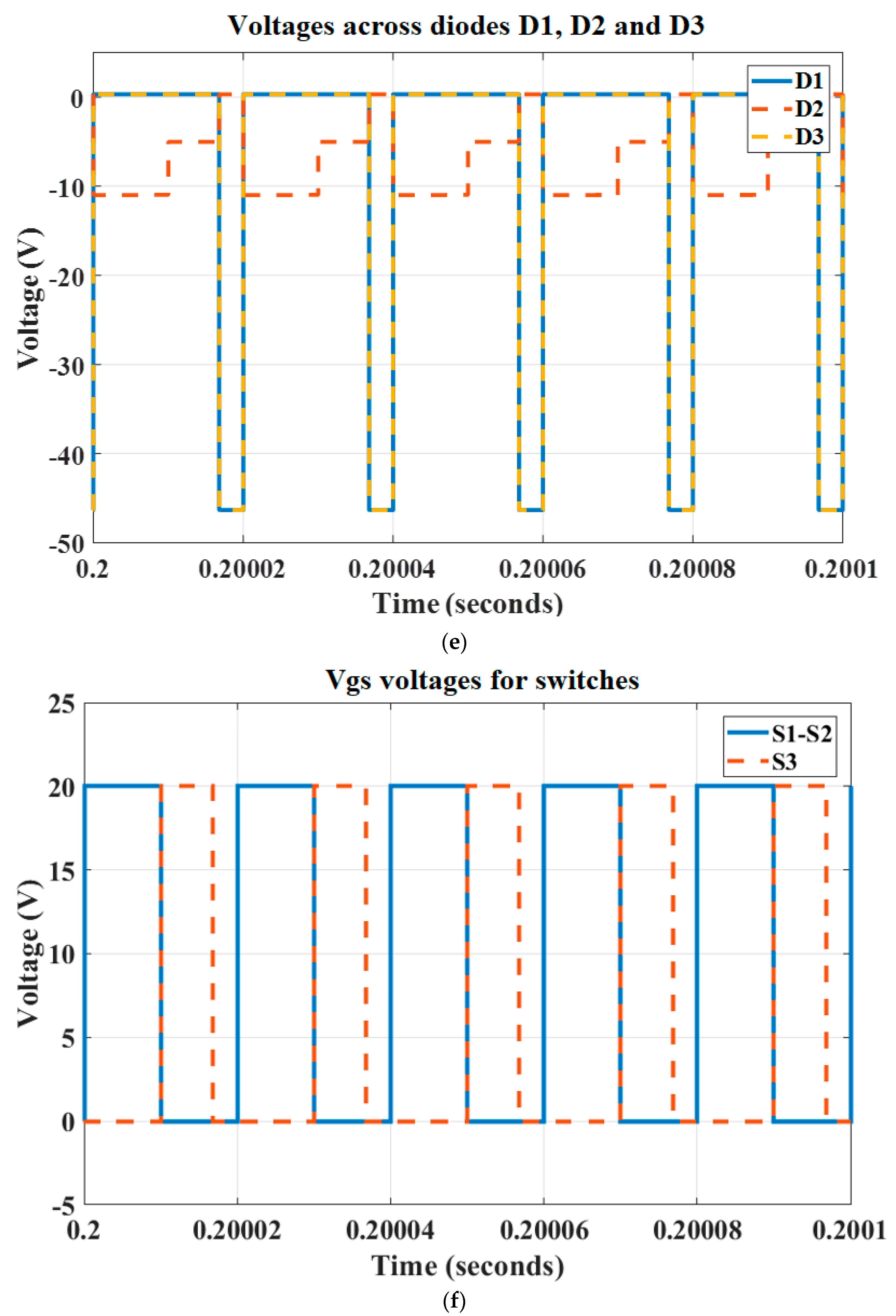
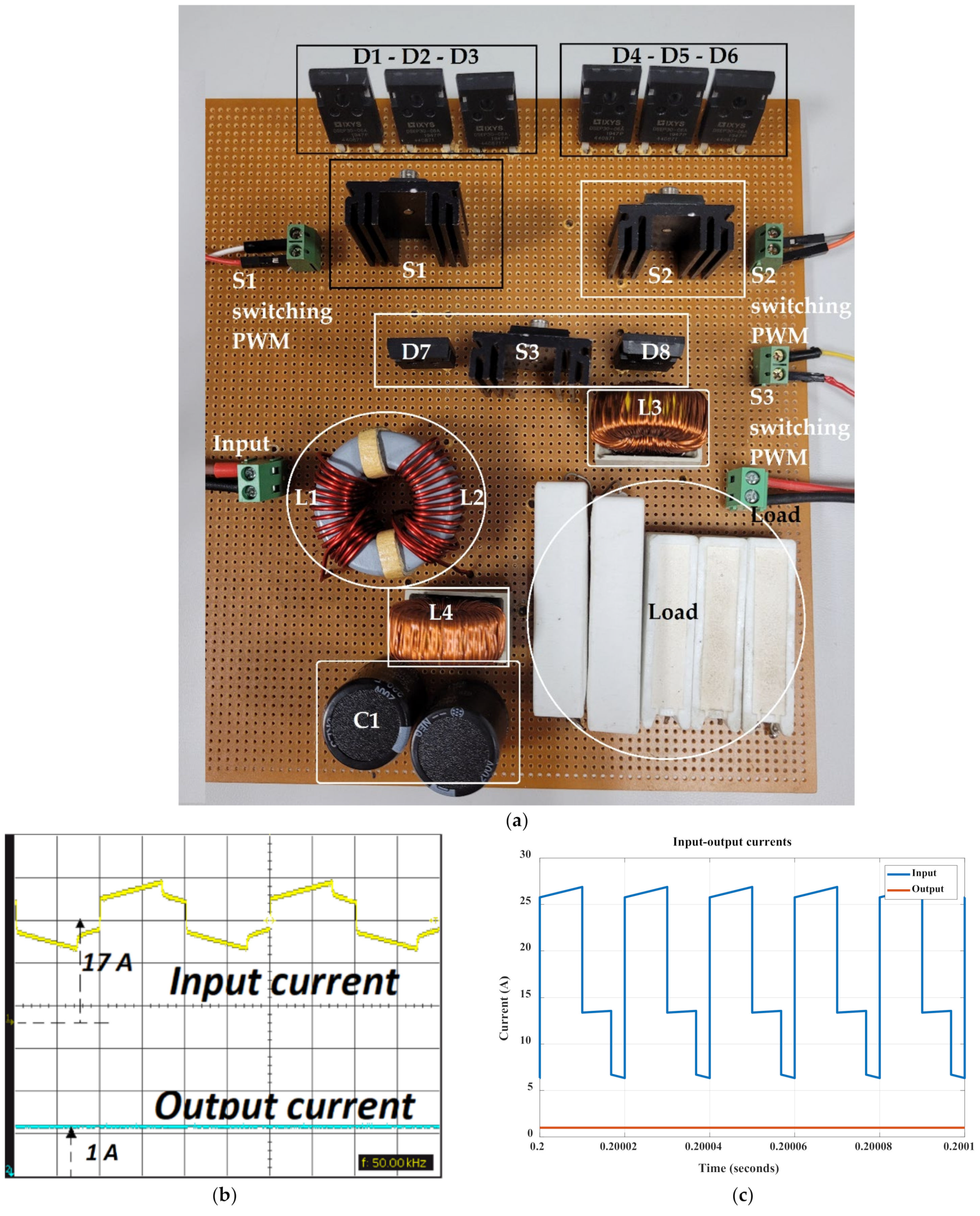
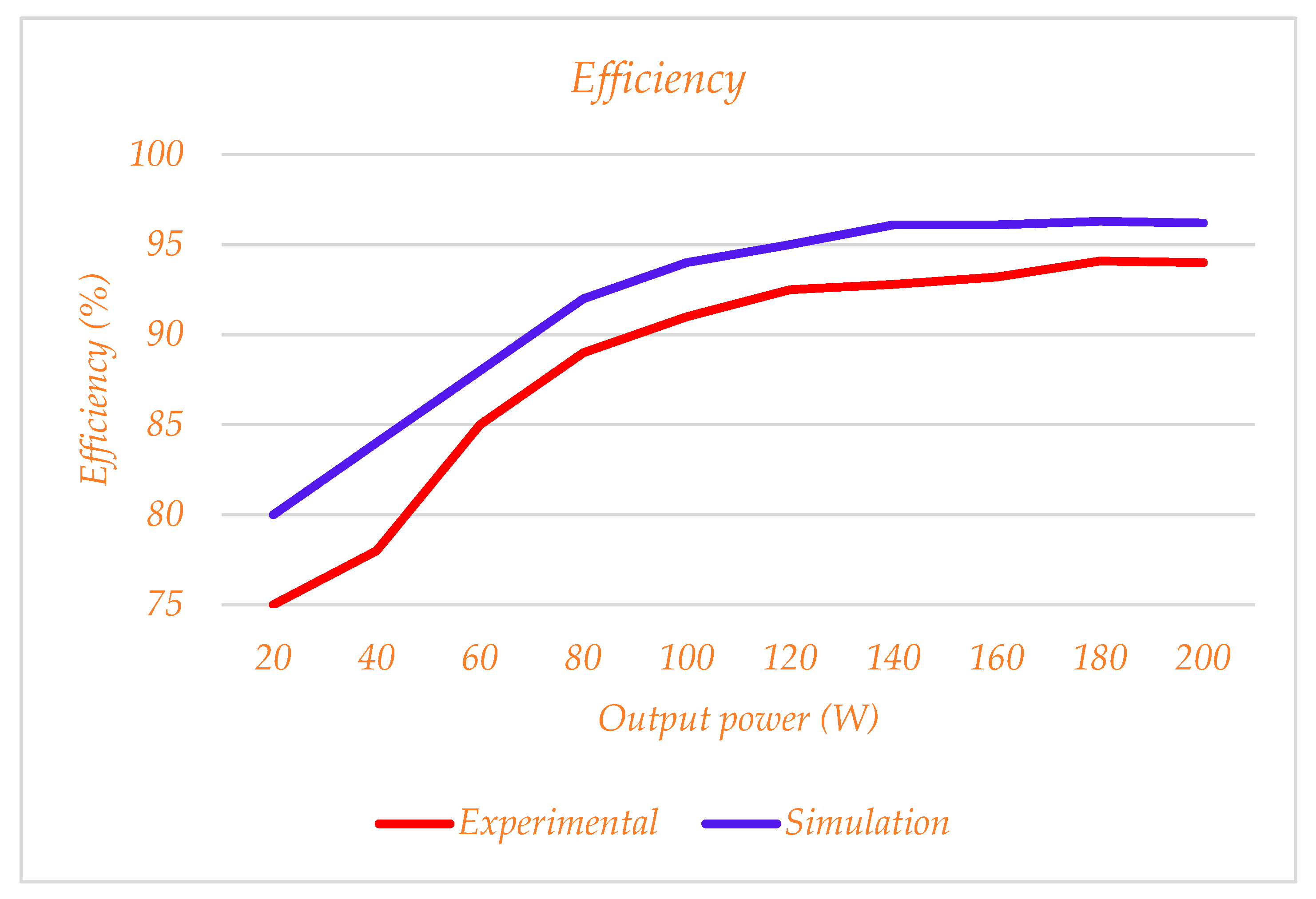
4. Analysis, Simulation, and Experimental Results
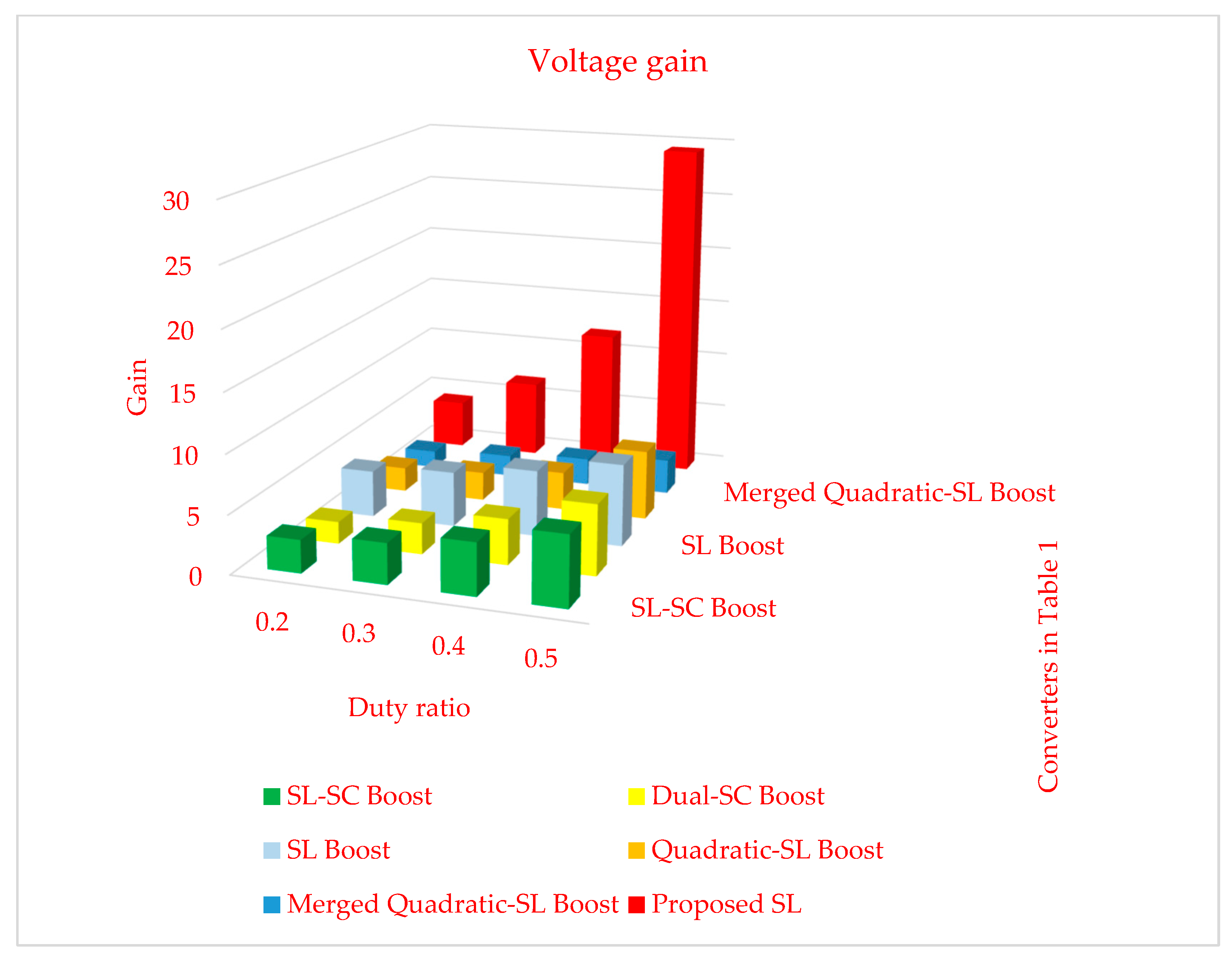
4.1. Comparison with Similar Converters and Performance Investigation
| Converter | No. of Switches | No. of Inductors | No. of Diodes | No. of Capacitors | Voltage Stress across the Switch | Voltage Gain | Gain at D = 0.5 |
|---|---|---|---|---|---|---|---|
| [29] | 1 | 2 | 5 | 4 | 7 | ||
| [32] | 3 | 2 | 2 | 1 | 5 | ||
| [33] | 3 | 2 | 0 | 1 | 3 | ||
| [34] n = 1 | 1 | 1 + 1 coupled inductor | 5 | 3 | 6 | ||
| [35] | 1 | 3 | 4 | 4 | 6 | ||
| Proposed | 3 | 4 or 2 coupled inductor | 8 | 1 | 16.66 |
4.2. Simulation Results
4.3. Experimental Results
5. Conclusions
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| RESs | Renewable energy sources |
| PV | Photovoltaic |
| FCs | Fuel cells |
| CCM | Continuous conduction mode |
| DCM | Discontinuous conduction mode |
| BCM | Boundary conduction mode |
| V2G | Vehicle to grid |
| G2V | Grid to vehicle |
| V2V | Vehicle to vehicle |
| PWM | Pulse width modulation |
| ZVS | Zero voltage switching |
| ZCS | Zero current switching |
| CP | Charge pump |
| SL | Switched-inductor |
| SC | Switched-capacitor |
| Inductance of all inductors to | |
| Current for the inductors to | |
| Duty cycle for the switches S1-S2 | |
| Duty cycle for the switch S3 | |
| voltage across the capacitor | |
| Voltage across the drain-source pins of MOSFETs | |
| Voltage across the diodes | |
| Minimum value of the inductors to work at the BCM | |
| Internal resistance of the inductors | |
| Internal resistance of the diodes | |
| Input power | |
| Switching losses | |
| Switching losses of the switches | |
| Switching losses of the diodes | |
| Switching frequency | |
| Parasitic capacitor for the drain-source pins of the switches | |
| Forward voltage for the diodes | |
| Electrical charge across the parasitic capacitor of the diodes |
References
- Lee, H.-S.; Yun, J.-J. High-efficiency bidirectional buck–boost converter for photovoltaic and energy storage systems in a smart grid. IEEE Trans. Power Electron. 2018, 34, 4316–4328. [Google Scholar] [CrossRef]
- Jongudomkarn, J.; Liu, J.; Yanagisawa, Y.; Bevrani, H.; Ise, T. Model predictive control for indirect boost matrix converter based on virtual synchronous generator. IEEE Access 2020, 8, 60364–60381. [Google Scholar] [CrossRef]
- Chen, S.; Zhou, L.; Luo, Q.; Gao, W.; Wei, Y.; Sun, P.; Du, X. Research on topology of the high step-up boost converter with coupled inductor. IEEE Trans. Power Electron. 2019, 34, 10733–10745. [Google Scholar] [CrossRef]
- Janabi, A.; Wang, B. Switched-capacitor voltage boost converter for electric and hybrid electric vehicle drives. IEEE Trans. Power Electron. 2019, 35, 5615–5624. [Google Scholar] [CrossRef]
- Wang, R.; Sun, Q.; Sun, C.; Zhang, H.; Gui, Y.; Wang, P. Vehicle-vehicle energy interaction converter of electric vehicles: A disturbance observer based sliding mode control algorithm. IEEE Trans. Veh. Technol. 2021, 70, 9910–9921. [Google Scholar] [CrossRef]
- George, L.; Gargiulo, G.D.; Lehmann, T.; Hamilton, T.J. A 0.04 mm2 Buck-Boost DC-DC Converter for Biomedical Implants Using Adaptive Gain and Discrete Frequency Scaling Control. IEEE Trans. Biomed. Circuits Syst. 2015, 10, 668–678. [Google Scholar] [CrossRef]
- Bi, K.; Liu, Y.; Zhu, Y.; Fan, Q. An impedance source modular DC/DC converter for energy storage system: Analysis and design. Int. J. Electr. Power Energy Syst. 2021, 133, 107261. [Google Scholar] [CrossRef]
- Srinivasan, M.; Kwasinski, A. Control analysis of parallel DC-DC converters in a DC microgrid with constant power loads. Int. J. Electr. Power Energy Syst. 2020, 122, 106207. [Google Scholar] [CrossRef]
- Apablaza, D.; Muñoz, J. Laboratory implementation of a boost interleaved converter for PV applications. IEEE Lat. Am. Trans. 2016, 14, 2738–2743. [Google Scholar] [CrossRef]
- Das, D.; Madichetty, S.; Singh, B.; Mishra, S. Luenberger observer based current estimated boost converter for PV maximum power extraction—A current sensorless approach. IEEE J. Photovolt. 2018, 9, 278–286. [Google Scholar] [CrossRef]
- Wu, H.; Mu, T.; Ge, H.; Xing, Y. Full-range soft-switching-isolated buck-boost converters with integrated interleaved boost converter and phase-shifted control. IEEE Trans. Power Electron. 2015, 31, 987–999. [Google Scholar] [CrossRef]
- Liu, Q.; Qian, Q.; Ren, B.; Xu, S.; Sun, W.; Yang, L. A Two-Stage Buck–Boost Integrated LLC Converter with Extended ZVS Range and Reduced Conduction Loss for High-Frequency and High-Efficiency Applications. IEEE J. Emerg. Sel. Top. Power Electron. 2019, 9, 727–743. [Google Scholar] [CrossRef]
- Folmer, S.; Stala, R. DC-DC High Voltage Gain Switched Capacitor Converter with Multilevel Output Voltage and Zero-Voltage Switching. IEEE Access 2021, 9, 129692–129705. [Google Scholar] [CrossRef]
- Stala, R.; Chojowski, M.; Waradzyn, Z.; Mondzik, A.; Folmer, S.; Penczek, A.; Skała, A.; Piróg, S. High-gain switched-capacitor DC-DC converter with low count of switches and low voltage stress of switches. IEEE Access 2021, 9, 114267–114281. [Google Scholar] [CrossRef]
- Dashtaki, M.A.; Nafisi, H.; Pouresmaeil, E.; Khorsandi, A. Virtual Inertia Implementation in Dual Two-Level Voltage Source Inverters. In Proceedings of the 2020 IEEE 11th Power Electronics, Drive Systems, and Technologies Conference (PEDSTC), Tehran, Iran, 4–6 February 2020. [Google Scholar]
- Liu, J.; Wang, K.; Zheng, Z.; Li, C.; Li, Y. A dual-active-clamp quasi-resonant isolated boost converter for PV integration to medium-voltage DC grids. IEEE J. Emerg. Sel. Top. Power Electron. 2019, 8, 3444–3456. [Google Scholar] [CrossRef]
- Yang, L.; Yu, W.; Zhang, J. High voltage gain ratio isolated resonant switched-capacitor converter for sustainable energy. IEEE Access 2019, 7, 23055–23067. [Google Scholar] [CrossRef]
- Xue, J.; Lee, H. Enabling high-frequency high-efficiency non-isolated boost converters with quasi-square-wave zero-voltage switching and on-chip dynamic dead-time-controlled synchronous gate drive. IEEE Trans. Power Electron. 2015, 30, 6817–6828. [Google Scholar] [CrossRef]
- Wu, Y.-E.; Ke, Y.-T. A novel bidirectional isolated DC-DC converter with high voltage gain and wide input voltage. IEEE Trans. Power Electron. 2020, 36, 7973–7985. [Google Scholar] [CrossRef]
- Bai, C.; Han, B.; Kwon, B.H.; Kim, M. Highly efficient bidirectional series-resonant DC/DC converter over wide range of battery voltages. IEEE Trans. Power Electron. 2019, 35, 3636–3650. [Google Scholar] [CrossRef]
- Yang, D.; Duan, B.; Zhang, C.; Shang, Y.; Song, J.; Bai, H.; Su, Q. High-Efficiency Bidirectional Three-Level Series-Resonant Converter with Buck-Boost Capacity for High-Output Voltage Applications. IEEE Trans. Transp. Electr. 2021, 7, 969–982. [Google Scholar] [CrossRef]
- Chen, C.; Liu, J.; Lee, H. A 2-MHz 9–45-V input high-efficiency three-switch ZVS step-up/-down hybrid converter. IEEE J. Solid-State Circuits 2020, 56, 855–865. [Google Scholar] [CrossRef]
- Liang, Y.; Liu, X.; Xu, G.; Yue, S. A Dual-Transformer-Based Bidirectional DC–DC Converter of Using Blocking Capacitor for Wide ZVS Range. IEEE Access 2020, 8, 170568–170578. [Google Scholar] [CrossRef]
- Zhang, Y.; Huang, Y.; Fang, P.; Gao, X.; Yang, Y.; Liu, J. Capacitors voltage ripple complementary control on three-level boost fed single-phase VSI with enhanced power decoupling capability. IEEE Trans. Power Electron. 2021, 36, 14220–14236. [Google Scholar] [CrossRef]
- Wu, F.; Wang, K.; Luo, S. Hybrid-Three-Level Current-Fed Series-Resonant Isolated DC-DC Converter and Its Optimization Modulation Strategy. IEEE Trans. Power Electron. 2021, 37, 196–205. [Google Scholar] [CrossRef]
- Wang, Y.; Zhao, C.; Zhang, L. Adaptive High-Power Laser-Based Simultaneous Wireless Information and Power Transfer System with Current-Fed Boost MPPT Converter. IEEE Photon. J. 2021, 13, 8400111. [Google Scholar] [CrossRef]
- Kumar, P.; Veerachary, M. Z-network plus switched-capacitor boost DC–DC converter. IEEE J. Emerg. Sel. Top. Power Electron. 2019, 9, 791–803. [Google Scholar] [CrossRef]
- Panda, K.P.; Bana, P.R.; Kiselychnyk, O.; Wang, J.; Panda, G. A single-source switched-capacitor-based step-up multilevel inverter with reduced components. IEEE Trans. Ind. Appl. 2021, 57, 3801–3811. [Google Scholar] [CrossRef]
- Fardoun, A.A.; Ismail, E.H. Ultra step-up DC–DC converter with reduced switch stress. IEEE Trans. Ind. Appl. 2010, 46, 2025–2034. [Google Scholar] [CrossRef]
- Li, G.; Jin, X.; Chen, X.; Mu, X. A novel quadratic boost converter with low inductor currents. CPSS Trans. Power Electron. Appl. 2020, 5, 1–10. [Google Scholar] [CrossRef]
- Axelrod, B.; Berkovich, Y.; Ioinovici, A. Switched-capacitor/switched-inductor structures for getting transformerless hybrid DC–DC PWM converters. IEEE Trans. Circuits Syst. I Regul. Pap. 2008, 55, 687–696. [Google Scholar] [CrossRef]
- Lakshmi, M.; Hemamalini, S. Nonisolated high gain DC–DC converter for DC microgrids. IEEE Trans. Ind. Electron. 2017, 65, 1205–1212. [Google Scholar] [CrossRef]
- Yang, L.-S.; Liang, T.-J. Analysis and implementation of a novel bidirectional DC–DC converter. IEEE Trans. Ind. Electron. 2011, 59, 422–434. [Google Scholar] [CrossRef]
- Lee, S.-W.; Do, H.-L. Quadratic boost DC–DC converter with high voltage gain and reduced voltage stresses. IEEE Trans. Power Electron. 2018, 34, 2397–2404. [Google Scholar] [CrossRef]
- Pires, V.F.; Cordeiro, A.; Foito, D.; Silva, J.F. High step-up DC–DC converter for fuel cell vehicles based on merged quadratic boost—Ćuk. IEEE Trans. Veh. Technol. 2019, 68, 7521–7530. [Google Scholar] [CrossRef]







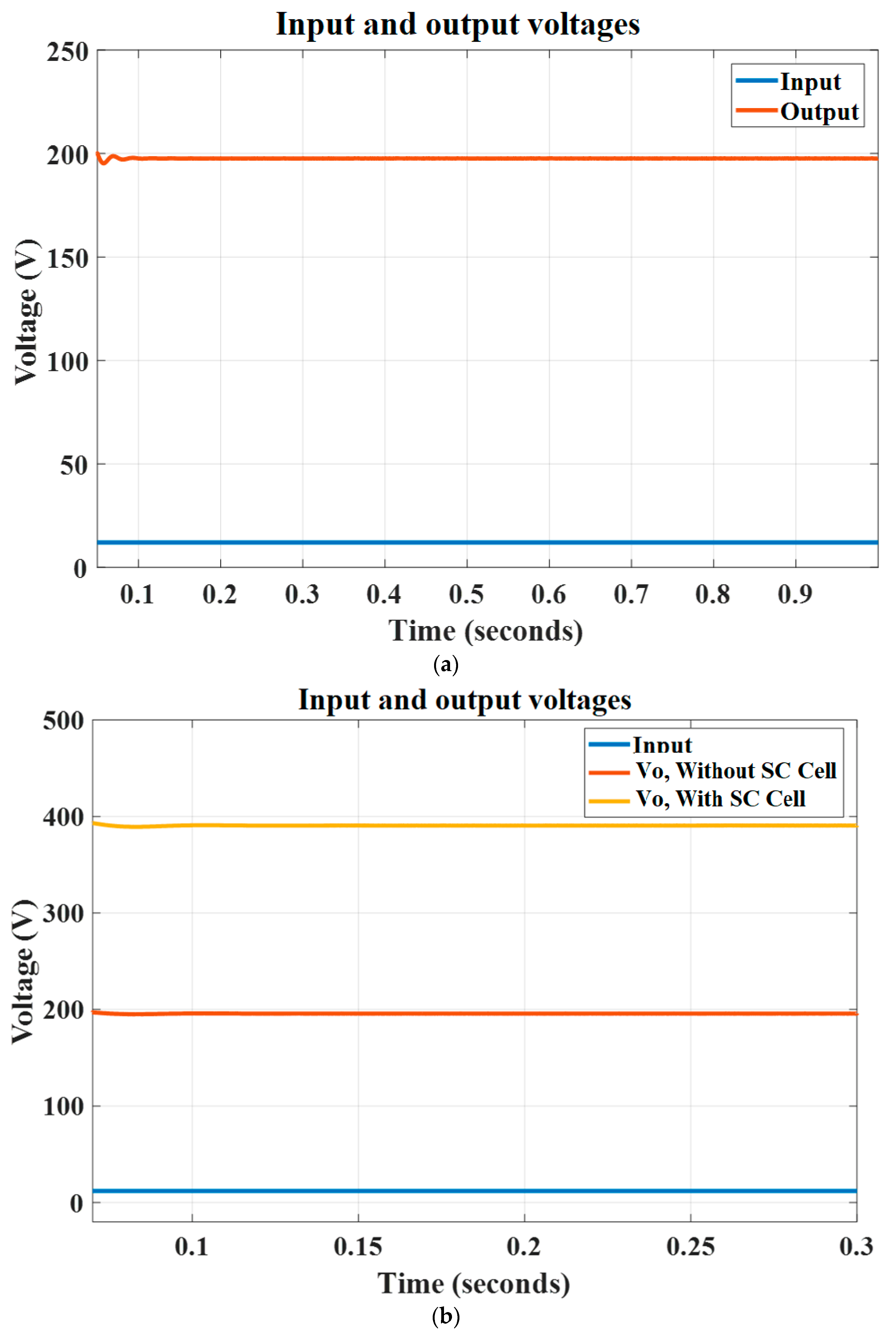



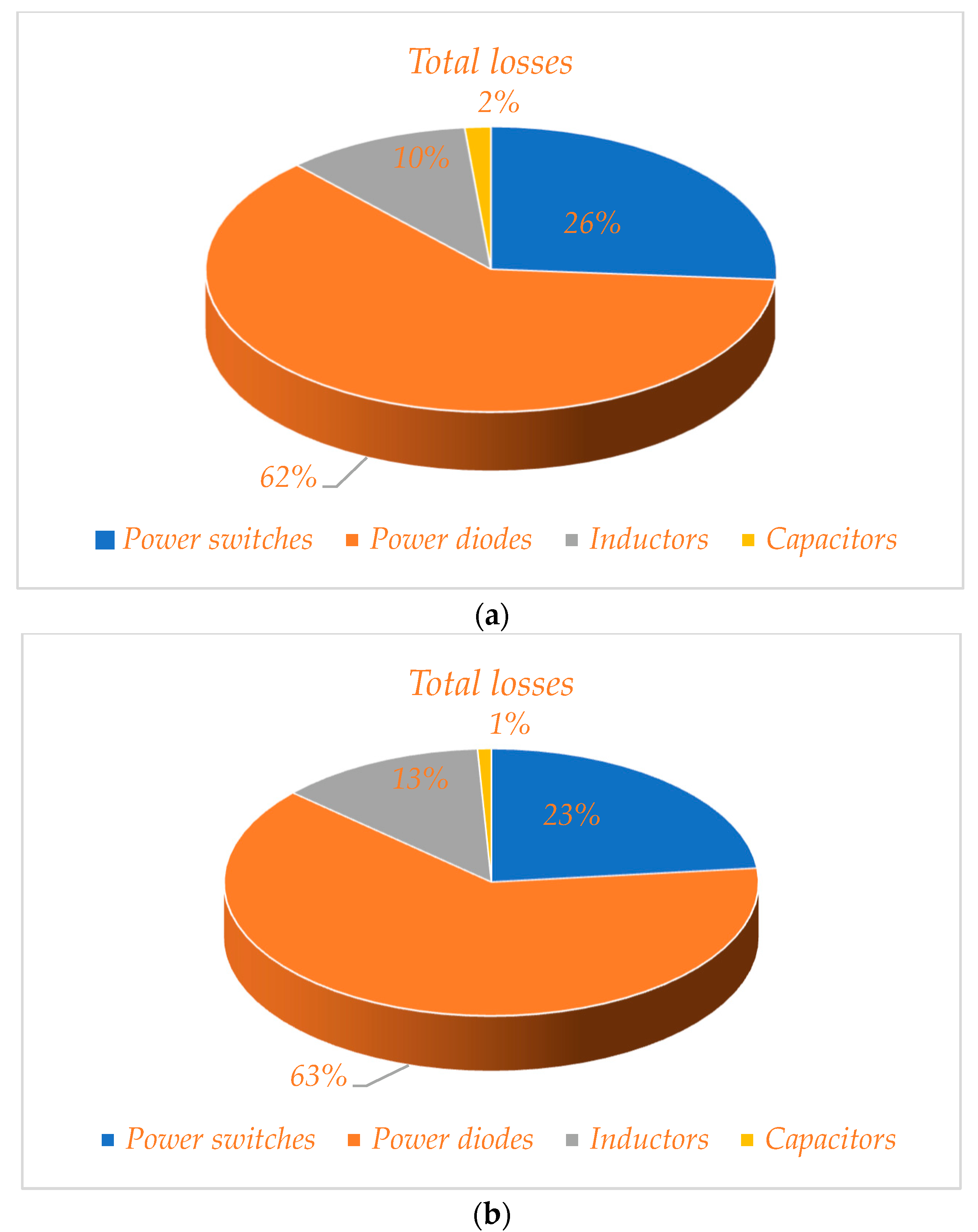
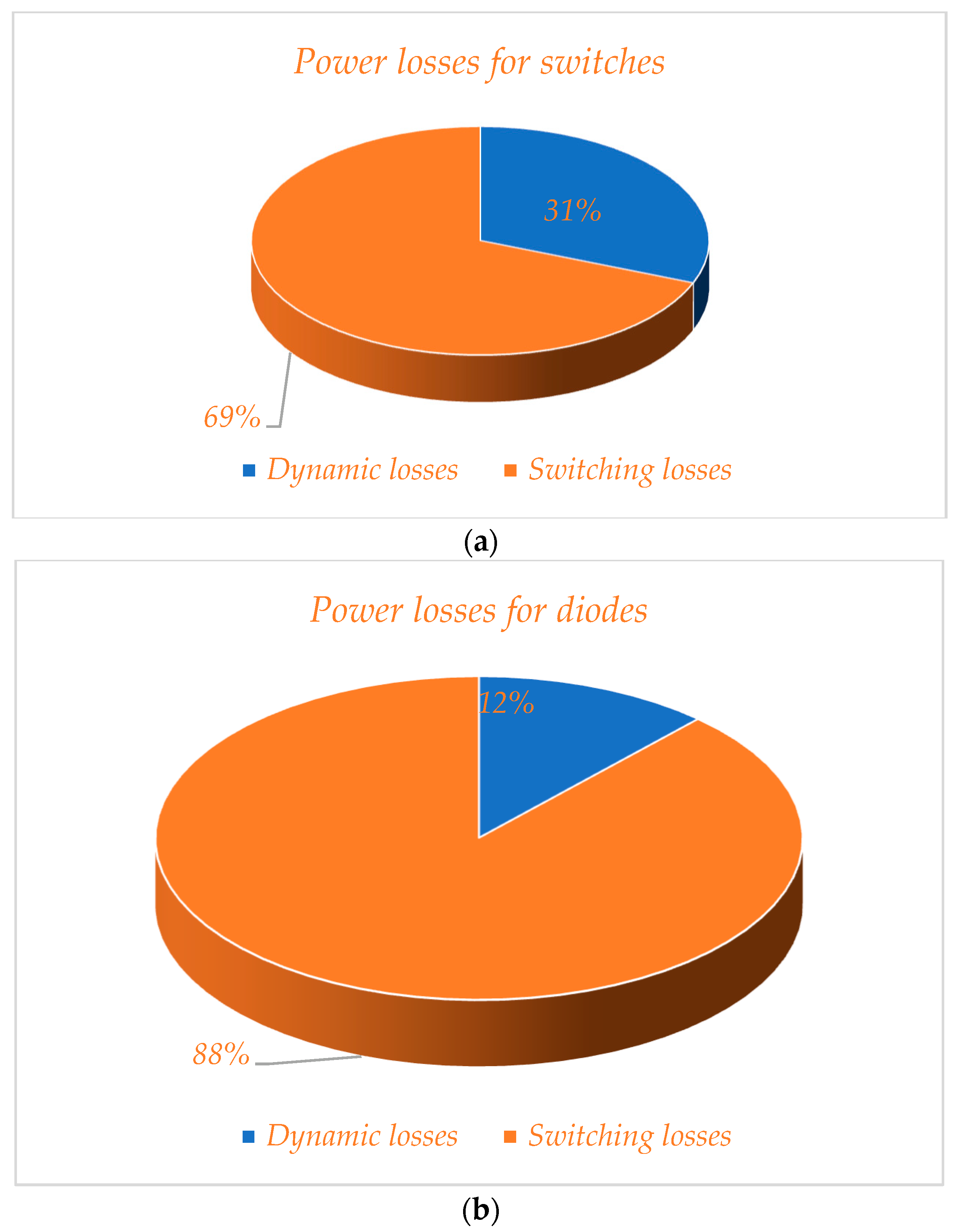
| Parameter Name | Value |
|---|---|
| Rated power () | 200 W |
| Input voltage () | 12 V |
| Output voltage () | 200 V (without SC-cell), 400 V (with SC-cell) |
| 0.5 | |
| 0.35 | |
| Switching frequency () | 50 kHz |
| Inductors ( | 400 µH |
| Capacitors () | 100 µF |
| Power MOSFETs () | TP65H035G4WS |
| Power Diodes () | DSEP30-06 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
N, K.; Mollajafari, M.; Mohammadiazar, F.; Akbari, E.; Sheykhi, E.; Chaoui, H. A New Voltage-Multiplier-Based Power Converter Configuration Suitable for Renewable Energy Sources and Sustainability Applications. Sustainability 2022, 14, 16698. https://doi.org/10.3390/su142416698
N K, Mollajafari M, Mohammadiazar F, Akbari E, Sheykhi E, Chaoui H. A New Voltage-Multiplier-Based Power Converter Configuration Suitable for Renewable Energy Sources and Sustainability Applications. Sustainability. 2022; 14(24):16698. https://doi.org/10.3390/su142416698
Chicago/Turabian StyleN, Kanagaraj, Morteza Mollajafari, Farzam Mohammadiazar, Ehsan Akbari, Ebrahim Sheykhi, and Hicham Chaoui. 2022. "A New Voltage-Multiplier-Based Power Converter Configuration Suitable for Renewable Energy Sources and Sustainability Applications" Sustainability 14, no. 24: 16698. https://doi.org/10.3390/su142416698
APA StyleN, K., Mollajafari, M., Mohammadiazar, F., Akbari, E., Sheykhi, E., & Chaoui, H. (2022). A New Voltage-Multiplier-Based Power Converter Configuration Suitable for Renewable Energy Sources and Sustainability Applications. Sustainability, 14(24), 16698. https://doi.org/10.3390/su142416698







