Study on Mechanical Calculation Model of Arch Ring in Freestanding Stone Cave-Dwelling
Abstract
:1. Introduction
2. Research Methodology
2.1. Basic Hypotheses Adopted in Calculation Model of Freestanding Stone Cave-Dwelling
- 1.
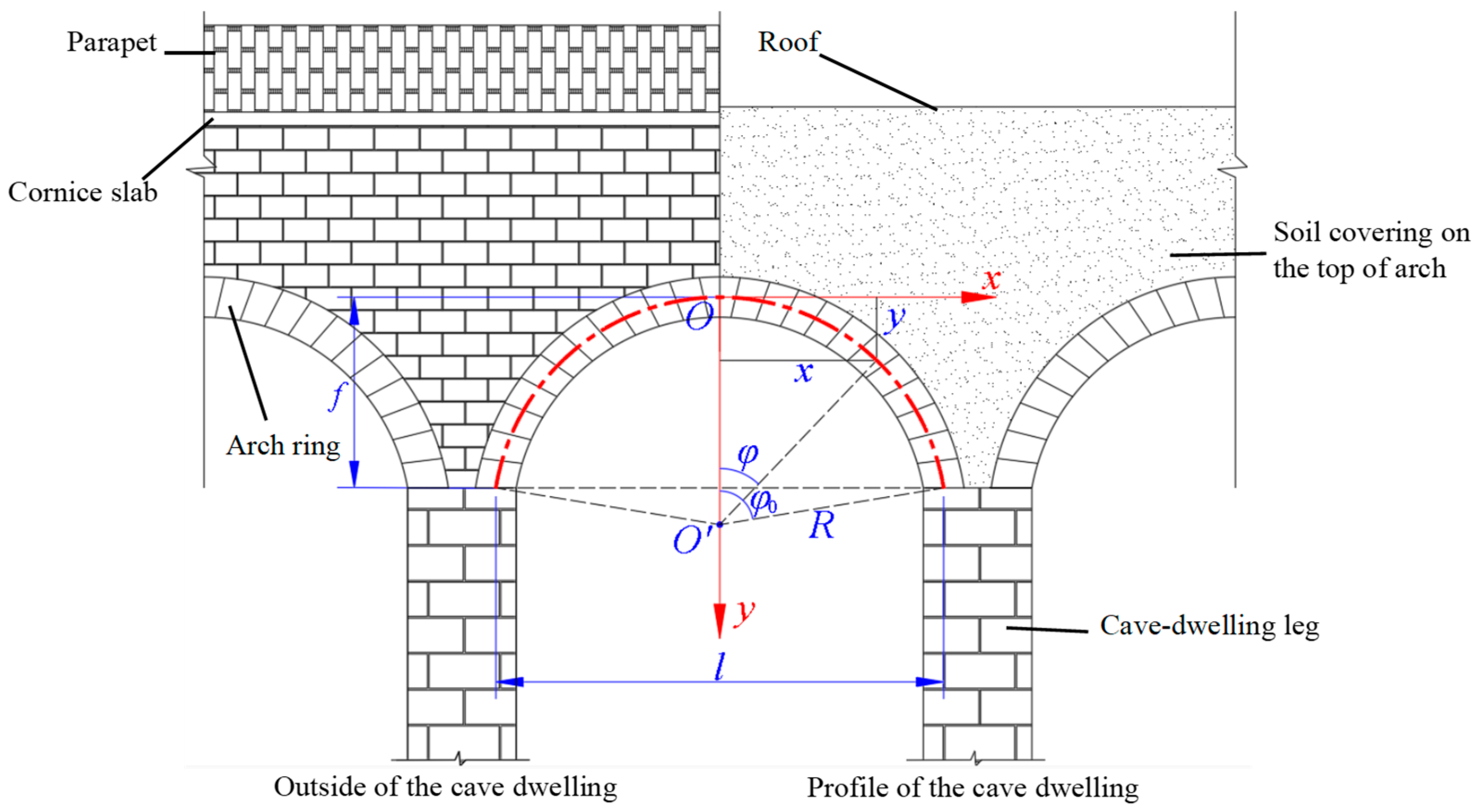
- Freestanding cave dwellings are generally connected by multiple spans. As the arch rings are subjected to basically the same forces per span, a single-span arch ring is taken for the sake of simplicity of analysis.
- 2.
- The arch axis curve of the arch ring has multiple forms, such as parabola, catenary and circular arc, etc. Here, the axis of the arch ring of the stone cave-dwelling is simplified as an equivalent cross-sectional circular arc. If the coordinate origin O is set at the vault of the arch, to the right as the x-axis, down as the y-axis, see Figure 2. Then the axis equation of the circular arc arch ring is expressed as:
- 3.
- Normally, when f/l > 0.2, only the effect of bending deformation is considered, and the effects of shear deformation and axial deformation are not taken into account [42].
- 4.
- It can be seen from Figure 2 that the loads on the freestanding stone cave-dwelling are mainly the self-weight of the arch ring, the self-weight of the fill, and the live load on the roof. The filling roof is regarded as a horizontal surface; the live load is considered to be uniformly distributed; the arch ring is assumed to be equally thick; and bending stiffness EI is constant. Then the load concentration P at any section of the arch ring is [39]:
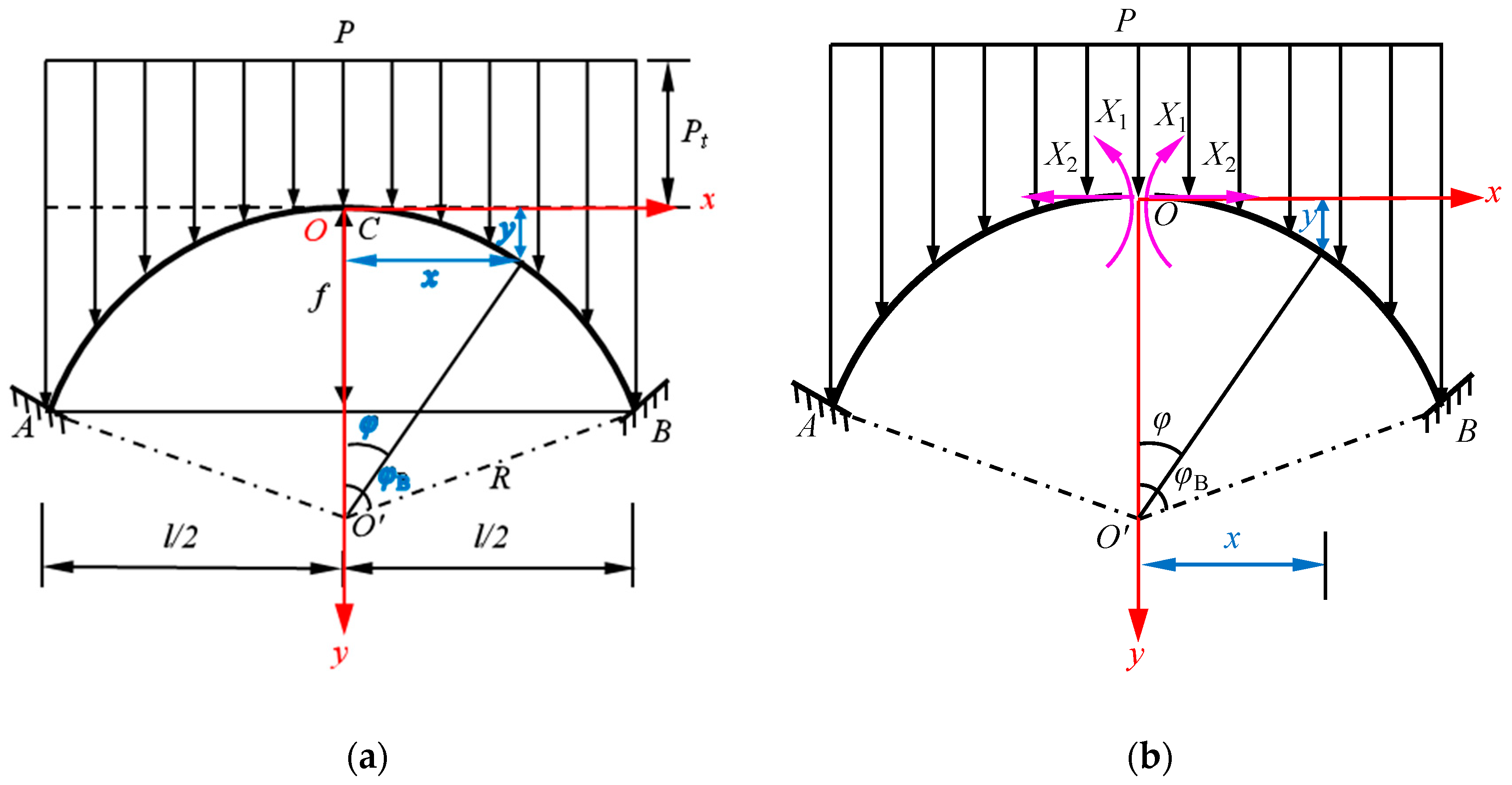
2.2. Derivation of Force Calculation Formula for Circular Arch Ring under the Calculation Model of Hingeless Arch
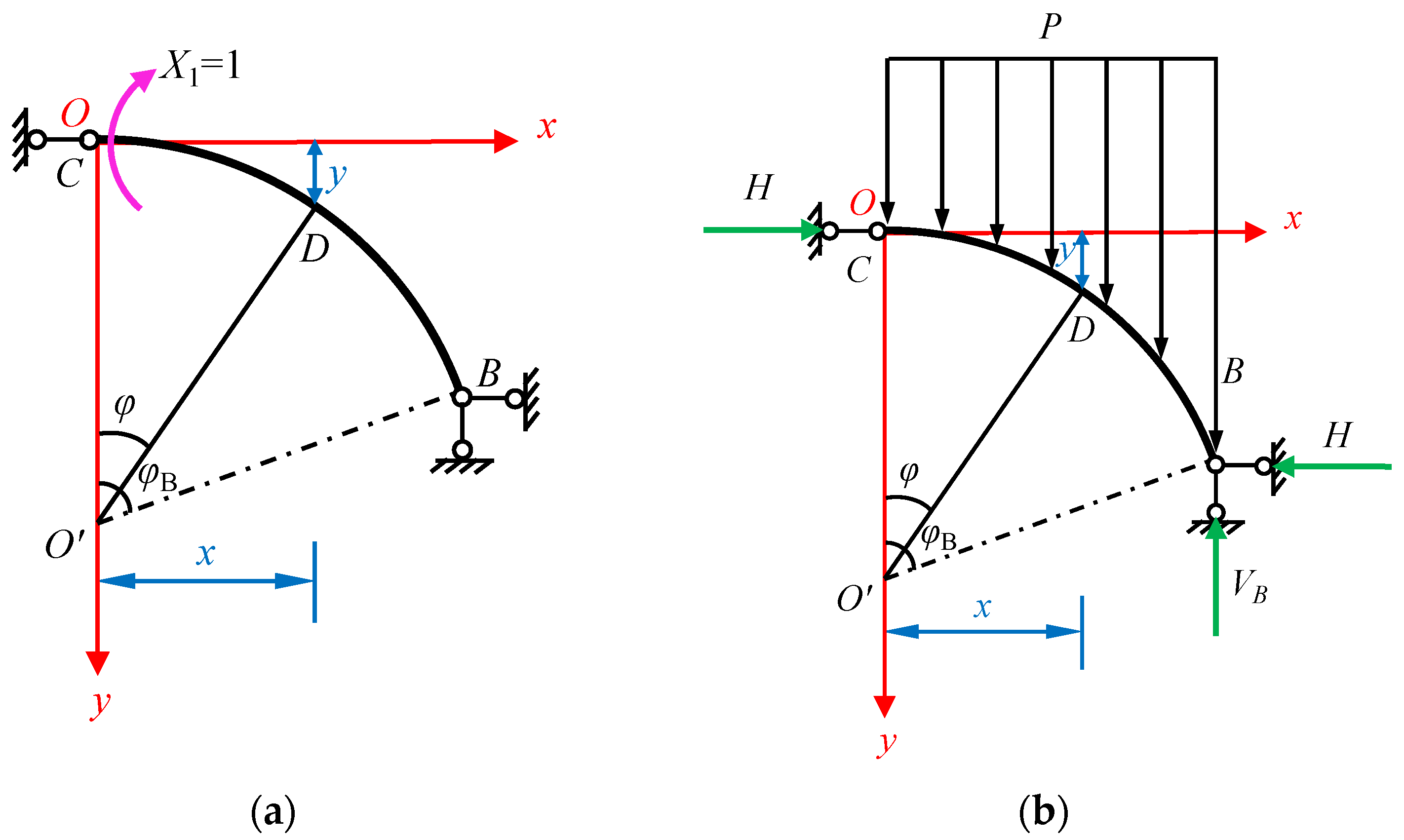
2.2.1. Selection of Basic System and Equation of Force Method
2.2.2. Calculation of Force Method Coefficient and Free Term
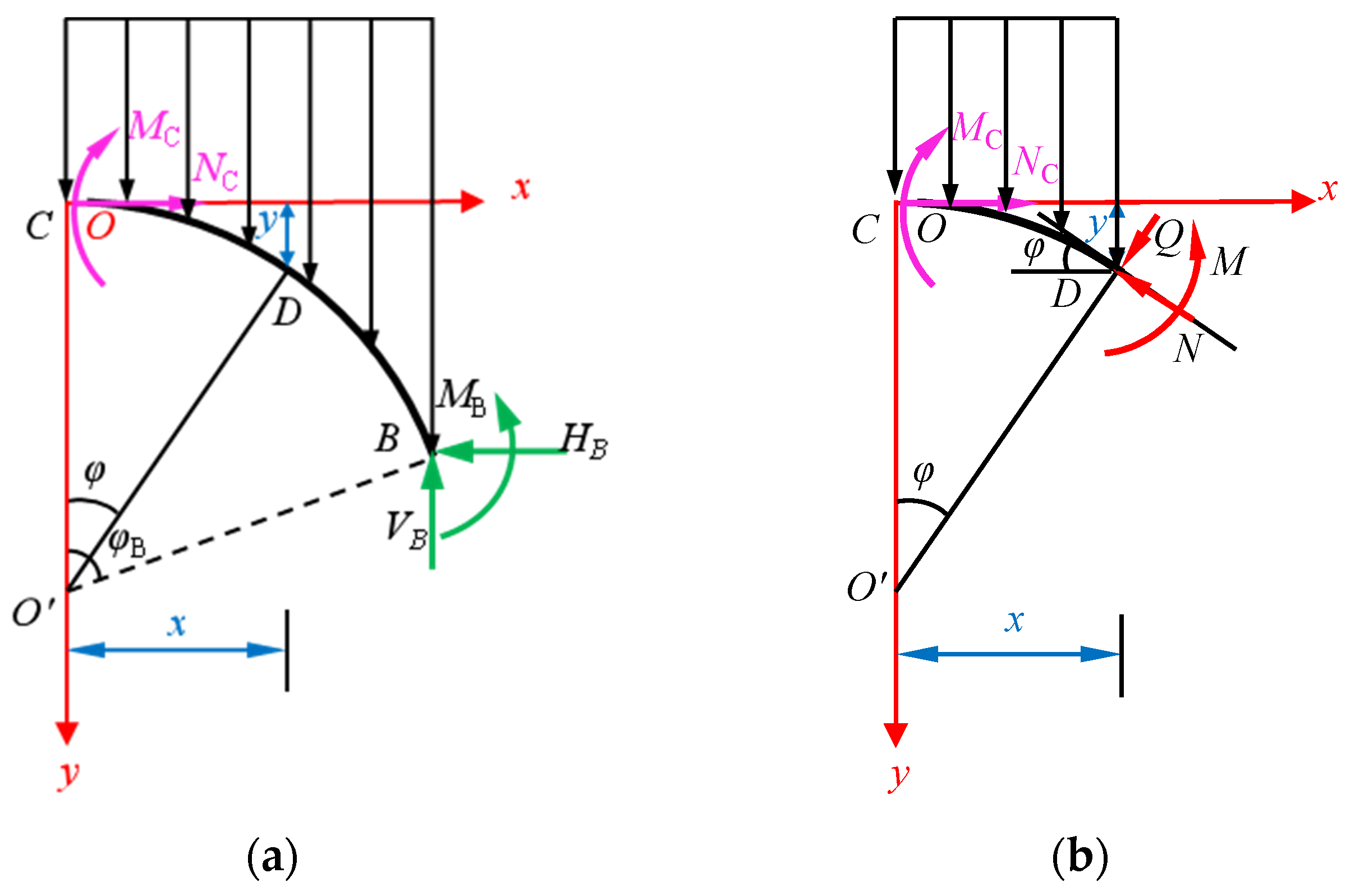
2.2.3. Calculation of Redundant Unknown Force
2.2.4. Calculation of Internal Forces in the Arch Ring Section
- bending moment:
- bending moment:
2.3. Derivation of force Calculation Formula for Circular Arch Ring under the Calculation Model of Two-Hinged Arch
2.3.1. Selection of Basic System and Equation of Force Method
2.3.2. Calculation of Force Method Coefficient and Free Term
2.3.3. Calculation of Redundant Unknown Force
2.3.4. Calculation of Internal Forces in the Arch Ring Section
- bending moment:
- bending moment:
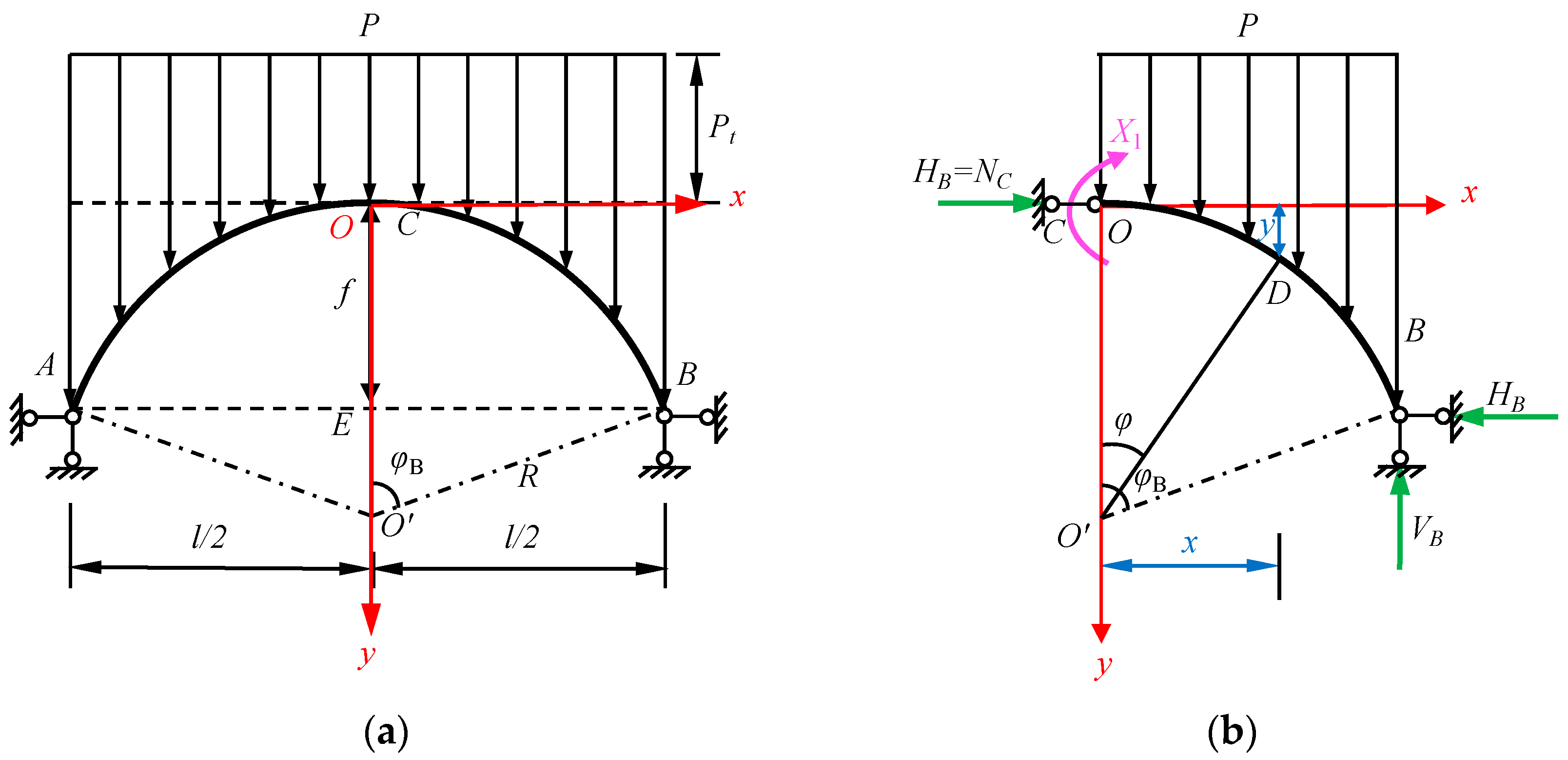
2.4. Derivation of Force Calculation Formula for Circular Arch Ring under the Calculation Model of Three-Hinged Arch
2.4.1. Simplicity of Calculation Model and Determination of the Support Reaction Force
2.4.2. Internal Force Formula of Any Section of Arch Ring
3. Results and Analysis
3.1. Example Parameter
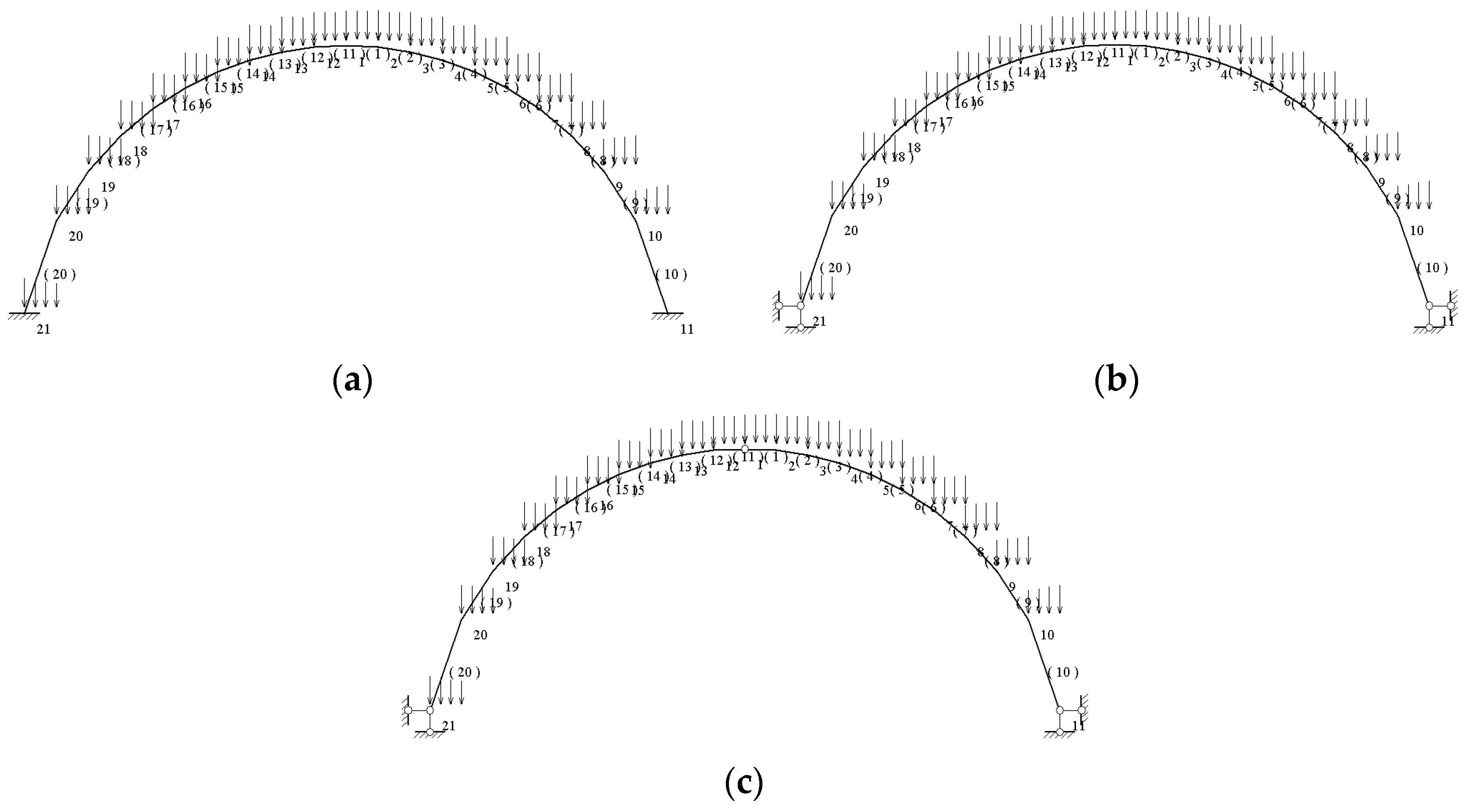
3.2. Simulation Verification
3.3. Comparison and Analysis of Calculation Results under the Three Mechanical Models
3.3.1. Comparison of Support’s Reaction Force
3.3.2. Comparison of Cross-Section Internal Forces in the Arch Ring
3.4. Section Design and Recheck of the Arch Ring
4. Conclusions
- Under the aforementioned three mechanical calculation models, the vertical reactions of the support are equivalent, the horizontal thrust is basically the same, and the relative difference is less than 10%.
- Under the aforementioned three mechanical calculation models, the maximum shear force occurs at the support of the arch foot; The maximum axial force occurs near the support of the arch foot, and the axial force of the arch vault section is roughly half of that of the arch foot. The distribution trend of the shear force diagram and axial force diagram is basically the same, and the values of axial force and shear force are similar, but the bending moment diagram is different. The bending moment diagrams of the hingeless arch and the two-hinged arch are more evenly distributed than those of the three-hinged arch. In addition, under these two mechanical calculation models, there is a certain bending moment at the top of the arch ring, which is close to the actual situation.
- The theoretical calculation results under the hingeless arch and two-hinged arch models are more consistent with the actual failure characteristics. The two-hinged arch calculation model is safer, more accurate, and more reliable than the hingeless arch calculation model when it is used in the force analysis of the circular arc arch ring. But the three-hinged arch can be used for a rough estimation.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wang, Z.; Wei, Q.; He, Y. Study on Constructive System of Green Cave Dwelling in Loess Plateau—Interpretation with the “Regional Gene” Theory. J. Zhejiang Univ.-Sci. A 2007, 8, 1754–1761. [Google Scholar] [CrossRef]
- Hu, X.F.; Zhang, F.L.; Xue, J.Y.; Zhu, W.W.; Liu, S.; Dai, M.X. Disease Analysis and Reinforcement Measures of Loess Caves. Ind. Constr. 2019, 49, 6–13. [Google Scholar]
- Zhang, F.L.; Tian, P.G.; Zhu, W.W.; Yang, K.; Hu, X.F.; Fan, D.G. Analysis of Mechanical Properties of Cave Dwelling Structure and Research on Reinforcement and Protection Technology; China Construction Industry Press: Beijing, China, 2020. [Google Scholar]
- Akubue, J. Earth Sheltered Housing; Design Concepts for Urban Ground-Scrapers. Int. J. Archit. Arts Appl. 2021, 7, 7. [Google Scholar] [CrossRef]
- Korsavi, S.; Mehdizade, S. The Earth and Ice-Pits Sustainable Architecture in Iran. In Proceedings of the European Conference on Sustainability, Energy and the Environment, Brighton, UK, 4–7 July 2013. [Google Scholar]
- Zeng, X.D.; Song, D.M. Green Design Explore of Earth-Sheltered Architecture: A Case Study of Chongqing Empirical Study Center of Architecture Energy-Saving Technology. J. Civ. Archit. Environ. Eng. 2011, 33, 104–108. [Google Scholar]
- Lee, J.H.; Choi, W.K.; Suh, S.J.; Cho, D.W. A Study on the Energy Needs Patterns for the House According to the Earth Sheltering Method. J. Archit. Inst. Korea Plan. Des. 2008, 24, 233–240. [Google Scholar]
- Tundrea, H.; Maxineasa, S.G.; Simion, I.M.; Taranu, N.; Budescu, M.; Gavrilescu, M. Environmental Impact Assessment and Thermal Performances of Modern Earth Sheltered Houses. Environ. Eng. Manag. J. 2014, 13, 2363–2369. [Google Scholar]
- Atkison, L.J. Earth Sheltered Housing/Cold Climate Design. In The Potential of Earth-Sheltered and Underground Space, 1st ed.; Holthusen, T.L., Ed.; Pergamon Press: Oxford, UK, 1981; pp. 187–190. [Google Scholar]
- Krundyshev, B.L. Engineering Composition Factors Optimization & Classification in the Earth Sheltered Housing Design. Int. J. Appl. Eng. Res. 2017, 12, 10848–10855. [Google Scholar]
- Hayashi, Y. The Future of Earth-Sheltered Architecture in China’s Farming Villages. Tunn. Undergr. Space Technol. 1986, 1, 167–169. [Google Scholar] [CrossRef]
- Staff, G.B. Earth-Sheltered Houses: How to Build an Affordable Underground Home; New Society Publishers: Gabriola, BC, Canada, 2006. [Google Scholar]
- Tong, L.P.; Han, C.P. Earth-Arched Structural Systems of Primitive Earth-Sheltered Dwelling. Constr. Technol. 2008, 37, 113–115. [Google Scholar]
- Tong, L.P.; Han, C.P. Study on the Structural Properties of Self-Support Systems for Earth-Sheltered Dwelling. Sichuan Build. Sci. 2009, 35, 71–73. [Google Scholar]
- Chen, R.F. Scientific Research on the Traditional Construction of Dikeng Cave Dwellings. Master’s Thesis, Zhengzhou University, Zhengzhou, China, 2011. [Google Scholar]
- Cao, Y.; Zhang, Y.X.; Tong, L.P. Study on Size Design and Its Influence on Mechanical Properties of Sunken Cave Dwelling. Build. Sci. 2012, 28, 103–107. [Google Scholar]
- Hao, Y.e.; Liang, X.W.; Yang, H.X. Static Behavior and Strengthening Methods of Single-Hole Loess Cave Dwelling by Cliff in Yan’an of Northern Shaanxi. Henan Sci. 2016, 34, 1852–1857. [Google Scholar]
- Liu, B.T.; Liu, X.L.; Zhang, S. Analysis of Pressure Arch Effect and Failure Mode of Cliff-Type Cave Dwellings with Different Covering Soil Thickness. China Non-Met. Miner. Ind. 2018, 132, 36–40+44. [Google Scholar]
- Guo, P.G. Stability Analysis of Loess Cave Dwellings under Longitudinal Earthquake. Earthq. Resist. Eng. Retrofit. 2016, 38, 133–138. [Google Scholar]
- Guo, P.G.; Tong, L.P. Influence of Crosswise Earthquake on Loess Cave Dwellings’ Stability. Earthq. Eng. Eng. Dyn. 2015, 35, 56–63. [Google Scholar]
- Hao, Y.e.; Liang, X.W.; Lan, Y.Q. Numerical Simulation and Dynamic Analysis of Single-Hole Cliff-Side Loess Cave Dwelling under Seismic Actions. Geofluids 2021, 2021, 6890445. [Google Scholar] [CrossRef]
- Xue, J.Y.; Zhao, X.B.; Zhang, F.L.; Hu, X.F.; Liu, X.H. Shaking Tabletest on Model Structure of Underground Loess Cave. J. Build. Struct. 2021, 42, 14–23. [Google Scholar]
- Tong, L.P.; Xu, C.X. Testing and Evaluation of Indoor and Outdoor Thermal Environment of Sunken Cave Dwelling in Summer. Build. Sci. 2015, 31, 9–14. [Google Scholar]
- Zhu, J.Y.; Nie, P.; Tong, L.P.; Zhao, X.; Xing, C.J. Thermal Responsive Characteristics of Cliff-Side Cave Dwellings in West Area of He’ Nan under Extreme Climate Conditions. Build. Sci. 2017, 33, 76–81+89. [Google Scholar]
- Zhu, J.Y.; Tong, L.P. Study on Thermal Performance of Underground Cave Dwelling in West Area of He’ Nan in Winter. Build. Sci. 2016, 32, 99–105+126. [Google Scholar]
- Han, J.M.; Su, S.Q. Stability Analysis of Weibei Pitted Loess Cave Dwellings in Rainfall Infiltration. J. Archit. Civ. Eng. 2012, 29, 104–110. [Google Scholar]
- Wang, N.Q.; Zhang, S. Sensitivity Analysis of Stability of Cave Dwellings Based on Orthogonal Design. Sci. Technol. Eng. 2017, 17, 119–124. [Google Scholar]
- Wang, N.Q.; Zhang, S. Quantitative Evaluation of Stability on Loess Cave Dwellings Based on Blind Data Theory. Chin. J. Undergr. Space Eng. 2017, 13, 822–827. [Google Scholar]
- Wu, C.J.; Gan, Z.M.; Meng, C.P. Stability of Cave Dwelling of Loess Hills in North Part of Shaanxi Province. J. Shaanxi Norm. Univ. (Nat. Sci. Ed.) 2005, 33, 119–122. [Google Scholar]
- Wu, W.; Ji, D. Using Flac3D to Simulation and Analysis of Cave Dwelling Stability. Int. J. Digit. Content Technol. Its Appl. 2012. [Google Scholar] [CrossRef]
- Wu, W.L.; Yang, H.X. Stability Analysis of the Loess Cave Dwelling in Shanbei Region Using Comsol. Ind. Constr. 2017, 47, 119–122. [Google Scholar]
- Yang, K.; Zhang, F.L.; Zhu, W.W.; Xue, J.Y.; Zhou, H.L.; Dai, M.X.; Liu, J. Stability Analysis of Loess Cave Dwelling by Cliff Based on Strength Reduction Method. Ind. Constr. 2019, 49, 25–30. [Google Scholar]
- Tong, L.P.; Zhang, M. Application of Brick -Arch Technology on Reinforcement of Earth Sheltered Dwelling. Constr. Technol. 2016, 45, 82–85. [Google Scholar]
- Xue, J.Y.; Ling, H.Q.; Zhou, H.L.; Zhang, F.L.; Zhao, X.B. Shaking Table Tests for Independent Stone Hoop Cave Reinforcement Model. J. Vib. Shock 2022, 41, 211–221. [Google Scholar]
- Zhang, F.L.; Tian, P.G.; Bian, Z.W.; Lei, B.; Yun, Z.Y.; Shi, J.C. The Strengthening Method of Loess Cave Based on the Stress Mechanism of Arch Structure and New Brick Arch. In Proceedings of the 12th Academic Conference on Building Construction Renovation and Disease Treatment, Zhengzhou, China, 25–26 November 2018. [Google Scholar]
- Zhang, F.L.; Zhou, G.M.; Xue, J.Y.; Zhao, X.B.; Hu, X.F.; Liu, Z.Q. Shaking Table Test on Damaged Brick Hoop Caves Strengthened by Cement Mortar Coating with Polypropylene Packing Belt Meshes. J. Build. Struct. 2021, 42, 113–124. [Google Scholar]
- Wang, F.J.; Liu, R.S.; Ma, Z.H. Damage Measures Analysis of Independent Cave Dwelling under Earthquake. Sci. Technol. Eng. 2018, 18, 281–286. [Google Scholar]
- Yan, Y.M. Force Analysis and Section Calculation of Arch Ring of Stone-Built Cave. Proc. Fifth Natl. Conf. Struct. Eng. 1996, 1, 672–675. [Google Scholar]
- Yan, Y.M.; Guo, B.S. Research on the Reasonable Arch Ring of Stone-Built Cave. Proc. Fourth Natl. Conf. Struct. Eng. 1995, 3, 655–658. [Google Scholar]
- Zhang, Y.X.; Tian, X.Y. Destroy Causes of Cave Dwelling and Its Cure Measure. J. Northwest Univ. Agric. For. Sci. Technol. (Nat. Sci. Ed.) 2004, 32, 145–149. [Google Scholar]
- Huang, C.H.; Wang, Z.J. Structure Analysis on the Cave Dwelling. J. Chang. Inst. Technol. (Nat. Sci. Ed.) 2009, 10, 46–48. [Google Scholar]
- Bao, S.H. Structural Mechanics, 3rd ed.; Wuhan University of Technology Press: Wuhan, China, 2008. [Google Scholar]
- Chen, Z.H.; Wen, G.Z.; Wang, D.Q.; Liu, H.J.; Chen, M.D. Structural Mechanics, 1st ed.; Higher Education Press: Beijing, China, 2020. [Google Scholar]





| Parameters Name | Value |
|---|---|
| span-length l (m) | 3.6 |
| arch height f (m) | 1.5 |
| arch ring thickness h1 (mm) | 250 |
| covering soil thickness h2 (m) | 1.0 |
| arch ring self-weight γ1 (kN/m3) | 24.8 |
| filling soil self-weight γ2 (kN/m3) uniformly distributed live load on the roof q (kN/m2) item coefficient of normal permanent load γG | 20 |
| 2.0 | |
| 1.3 | |
| item coefficient of active load γQ | 1.5 |
| combined value coefficient ψ | 0.7 |
| Bearing Reactions | Hingeless Arch | Two-Hinged Arch | Three-Hinged Arch |
|---|---|---|---|
| horizontal thrust (kN) | 48.54 (46.62) | 40.96 (41.02) | 44.76 (44.83) |
| vertical reaction (kN) | 84.14 (84.47) | 84.14 (84.47) | 84.14 (84.47) |
| Calculation Model Type | Section Position | Bending Moment M (kN·m) | Shearing Force Q (kN) | Axial Force N (kN) |
|---|---|---|---|---|
| Hingeless arch | Arch vault | 3.29 (3.95) | 0 (2.30) | 48.54 (46.56) |
| Arch foot | 8.97 (6.64) | 32.57 (16.49) | 91.51 (95.06) | |
| Maximum absolute values | 8.97 (6.64) | 32.57 (20.56) | 91.67 (95.06) | |
| Two-hinged arch | Arch vault | 5.70 (5.71) | 0 (2.02) | 40.96 (40.97) |
| Arch foot | 0 (0) | 25.12 (11.20) | 90.15 (93.23) | |
| Maximum absolute values | 7.47 (7.33) | 25.12 (15.27) | 90.15 (93.23) | |
| Three-hinged arch | Arch vault | 0 (0) | 0 (2.21) | 44.76 (44.77) |
| Arch foot | 0 (0) | 28.85 (14.80) | 90.83 (94.48) | |
| Maximum absolute values | 9.96 (9.80) | 28.85 (18.87) | 90.87 (94.48) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hao, Y.; Lan, Y. Study on Mechanical Calculation Model of Arch Ring in Freestanding Stone Cave-Dwelling. Sustainability 2023, 15, 9545. https://doi.org/10.3390/su15129545
Hao Y, Lan Y. Study on Mechanical Calculation Model of Arch Ring in Freestanding Stone Cave-Dwelling. Sustainability. 2023; 15(12):9545. https://doi.org/10.3390/su15129545
Chicago/Turabian StyleHao, Yan’e, and Yongqiang Lan. 2023. "Study on Mechanical Calculation Model of Arch Ring in Freestanding Stone Cave-Dwelling" Sustainability 15, no. 12: 9545. https://doi.org/10.3390/su15129545
APA StyleHao, Y., & Lan, Y. (2023). Study on Mechanical Calculation Model of Arch Ring in Freestanding Stone Cave-Dwelling. Sustainability, 15(12), 9545. https://doi.org/10.3390/su15129545





