Numerical Simulation and Application of an Oxygen-Enriched Side-Blown Smelting Furnace for the Treatment of Electroplating Sludge
Abstract
:1. Introduction
2. Model Establishment and Calculation
2.1. Geometry Model with Simplification
2.2. Grid Division and Independence Test
2.3. Mathematic Model and Calculation Method
2.3.1. Basic Governing Equations
- (1)
- Continuity Equation
- (2)
- Volume Fraction Equation
- (3)
- Momentum Equation
2.3.2. Turbulence Model
2.3.3. Boundary Conditions and Property Parameters of Material
- (1)
- Inlet conditions
- (2)
- Outlet conditions
- (3)
- Wall boundary conditions
- (4)
- Material
2.3.4. Solver Settings
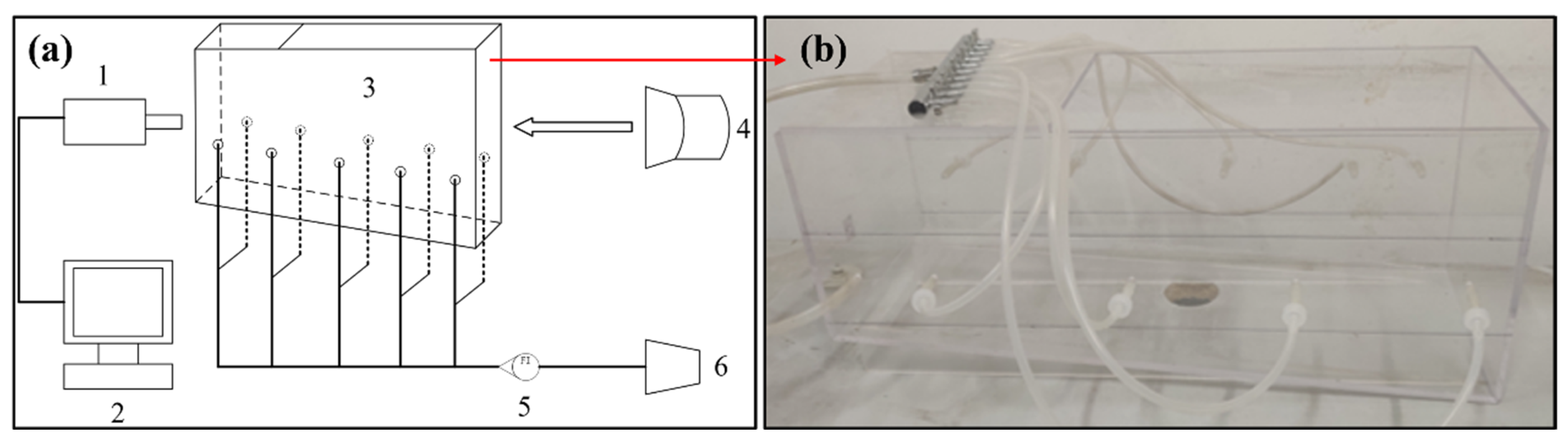
2.4. Establishment of the Water Model
2.5. Numerical Simulation
2.5.1. Basic Working Conditions
2.5.2. Single-Factor Numerical Experiment
2.5.3. Multifactor Comprehensive Optimization
- (1)
- Definition I: Indicator layer matrix M
- (2)
- Definition II: Factor layer matrix
- (3)
- Definition III: Level layer matrix
- (4)
- Definition IV: Weight matrix
3. Results and Discussion
3.1. Grid Independence Test
3.2. Model Verification
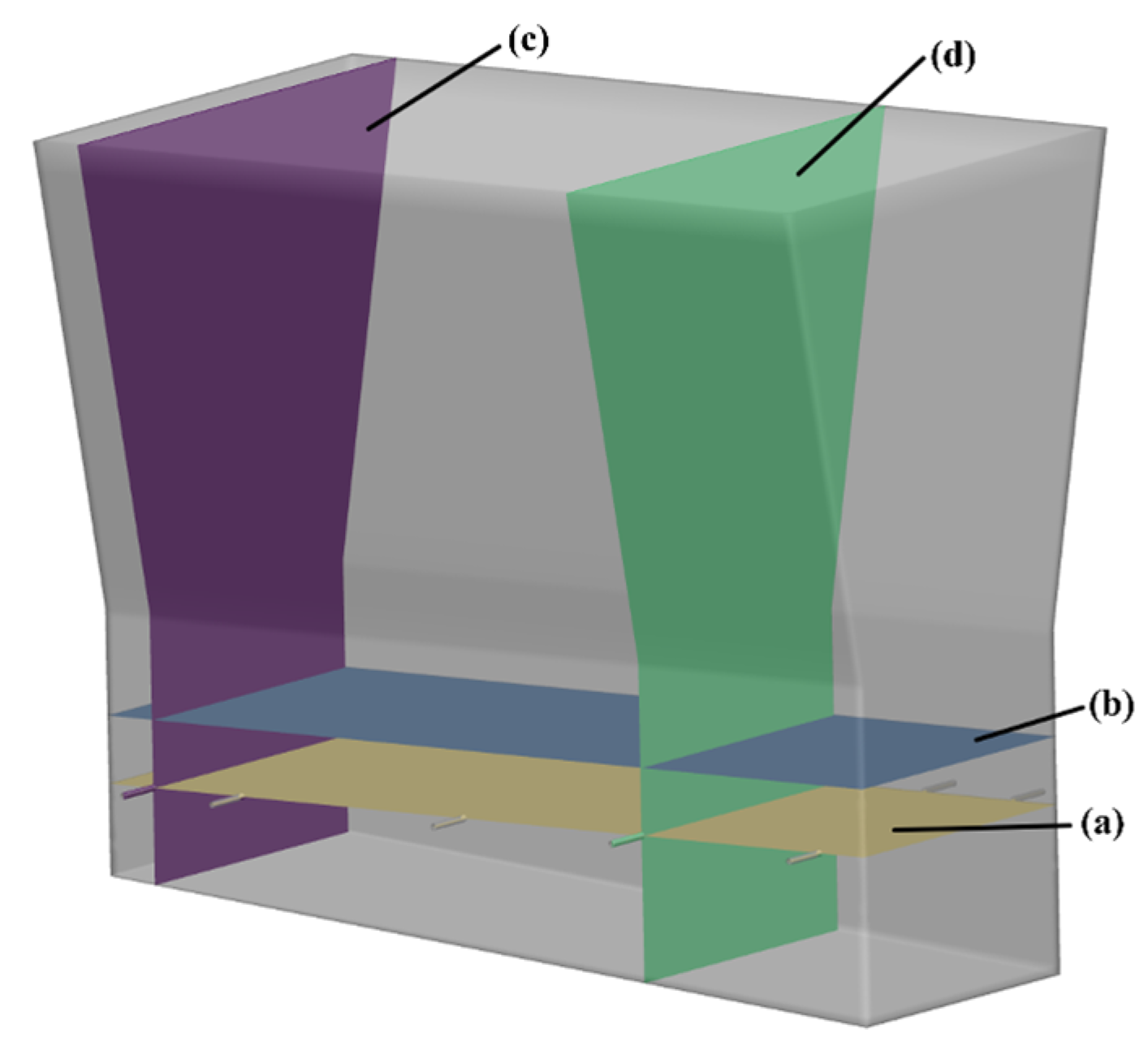
3.3. Flow Field and Temperature Distribution under Basic Working Conditions
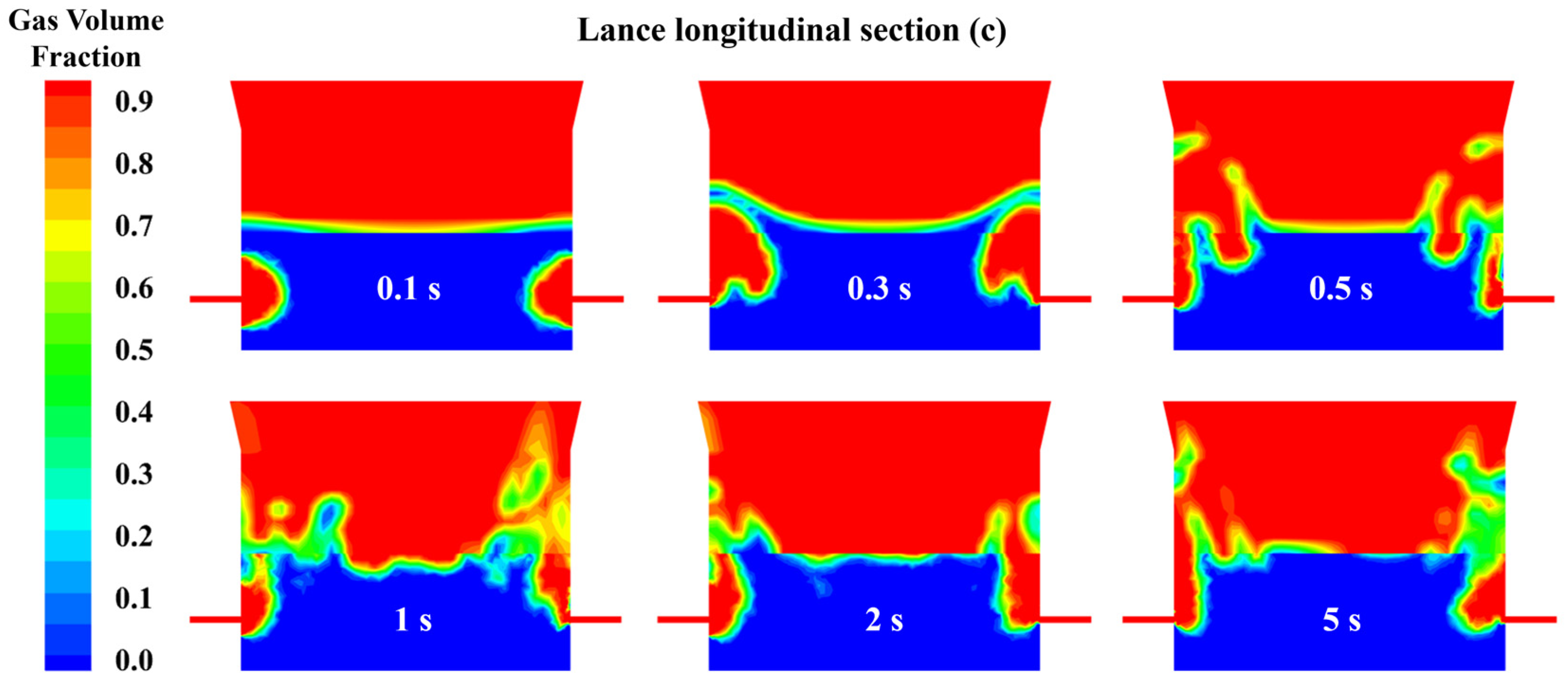
3.3.1. Variation in Gas Volume Fraction Distribution with Time
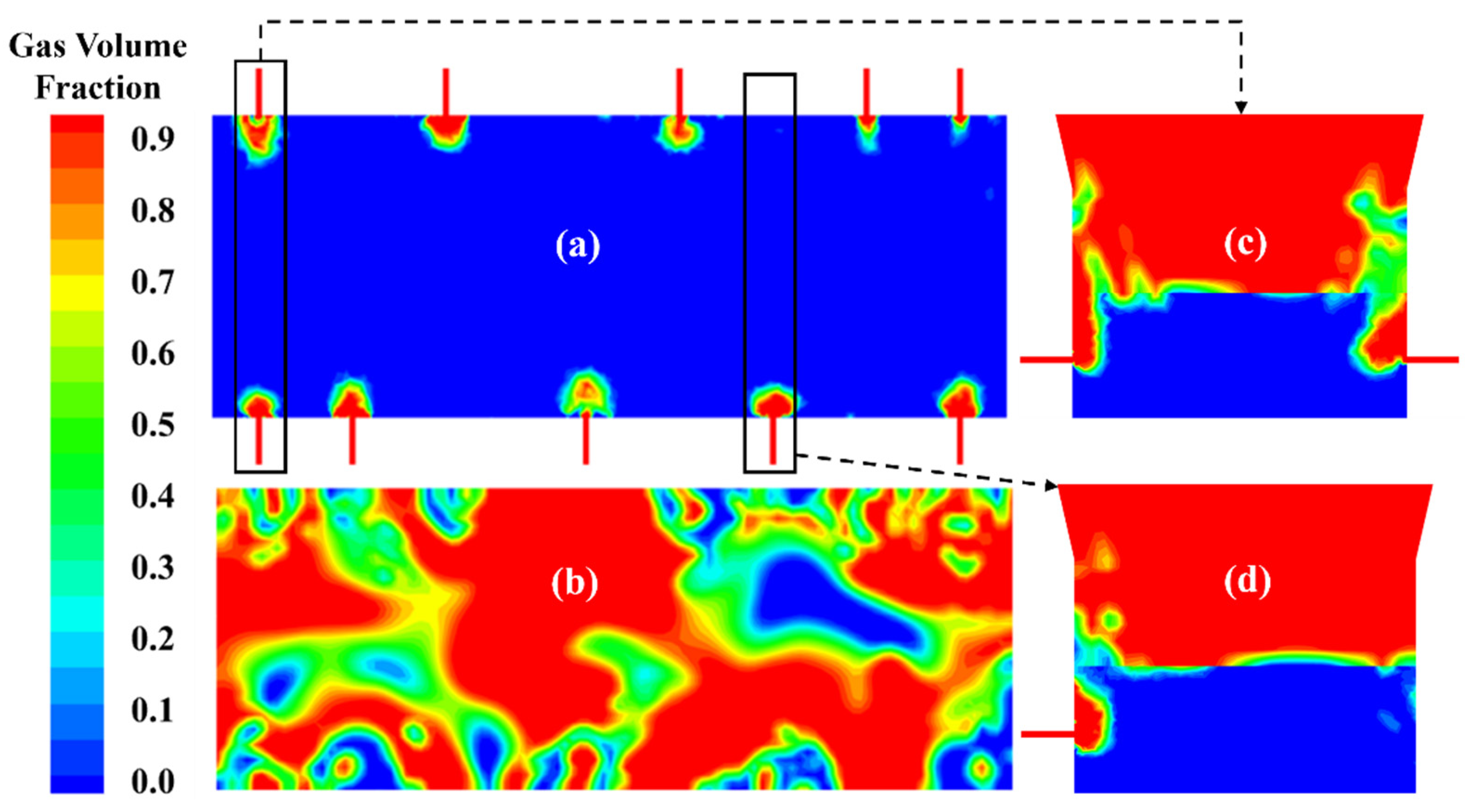
3.3.2. Gas Volume Fraction Distribution
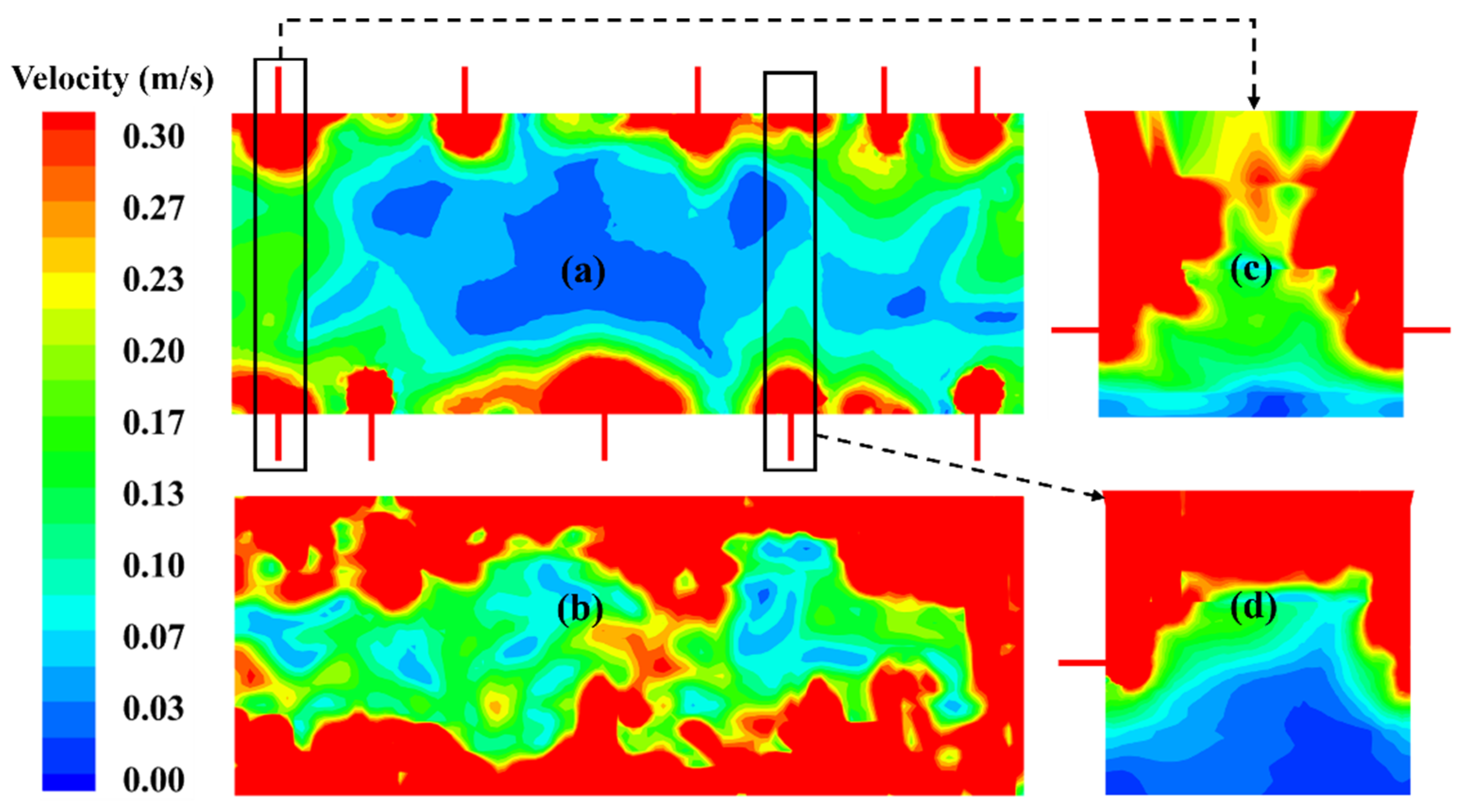
3.3.3. Velocity Distribution
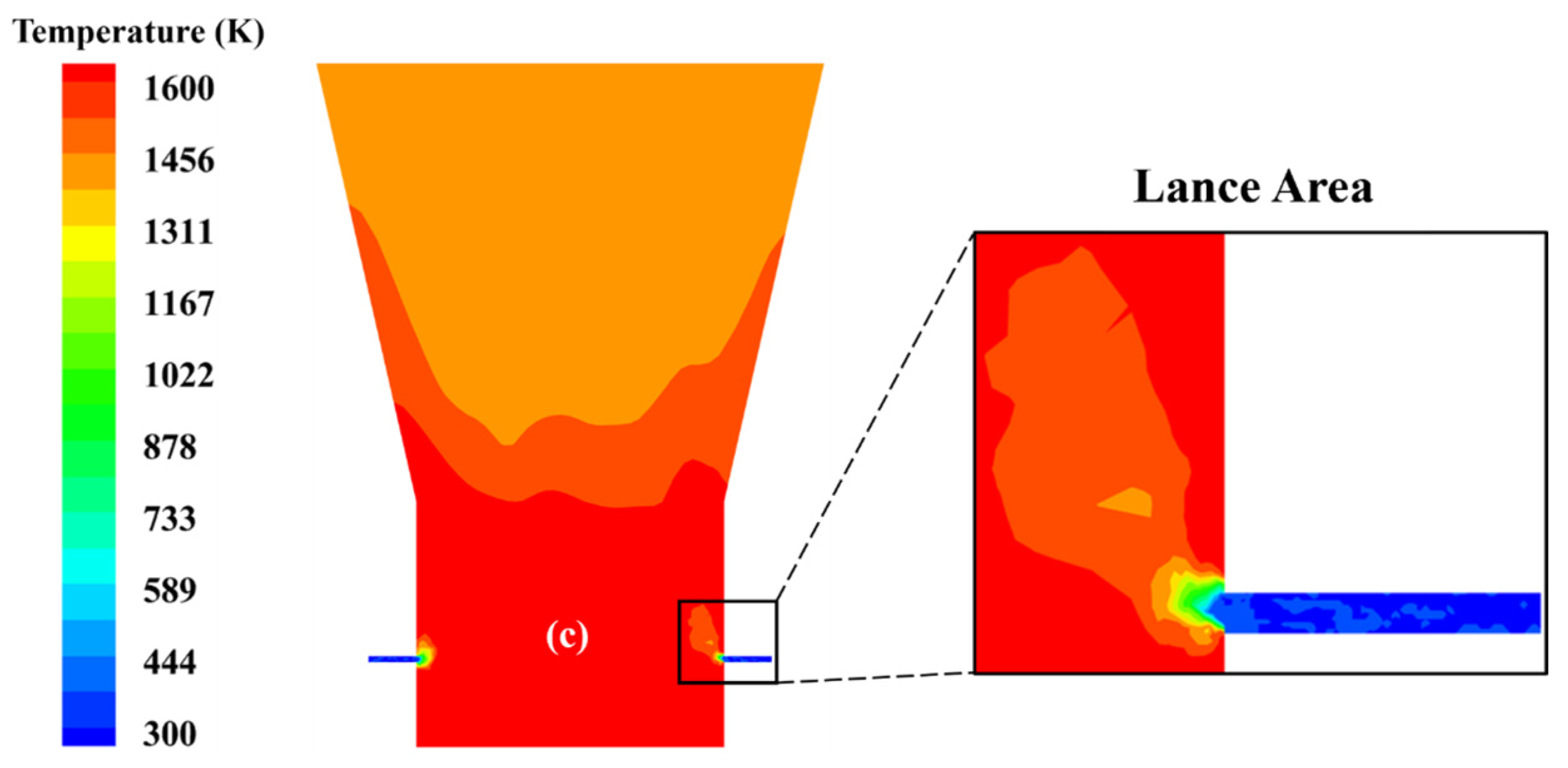
3.3.4. Temperature Distribution
3.4. Analysis and Optimization of Key Parameters
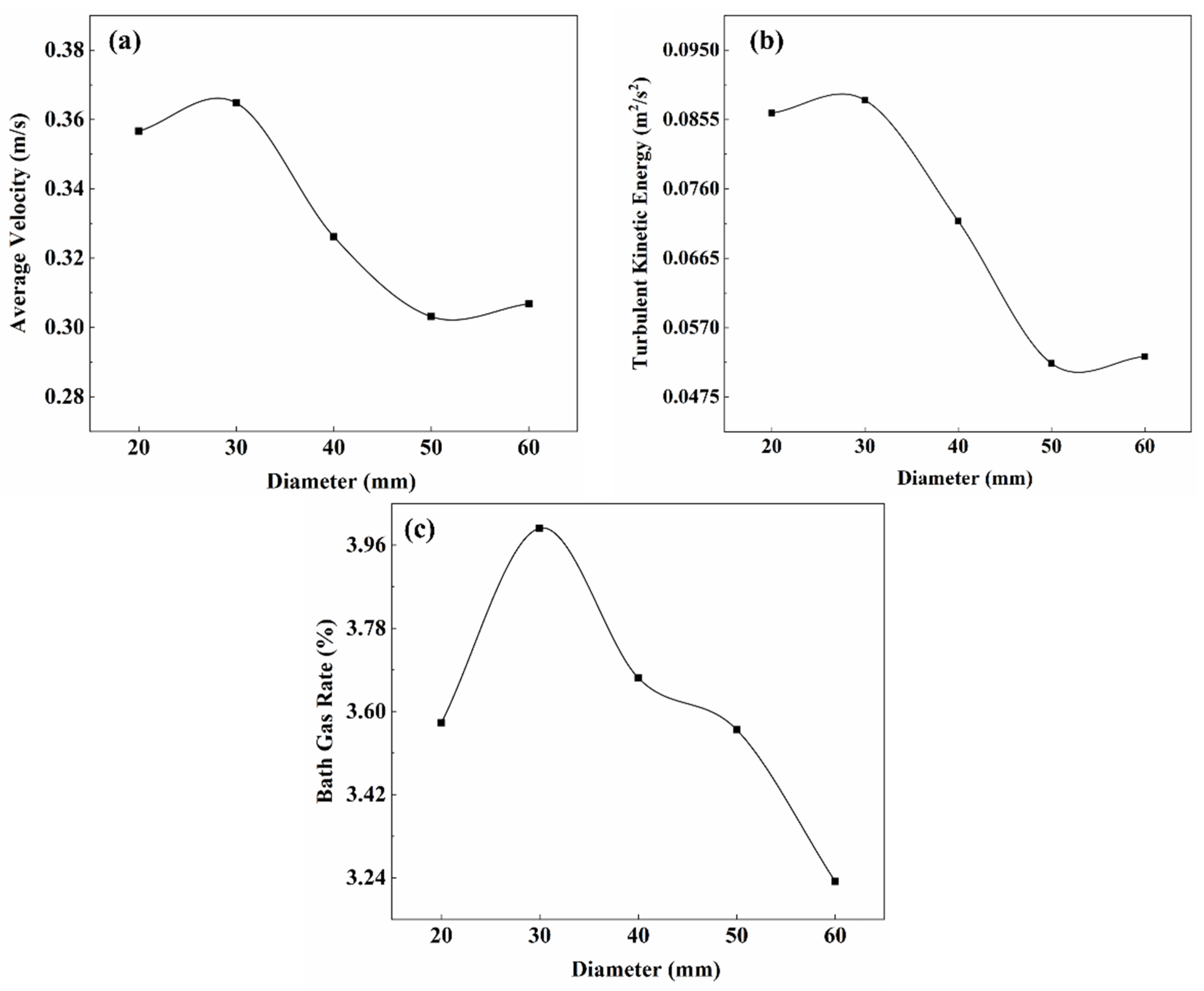
3.4.1. Lance Diameter
3.4.2. Lance Inclination
3.4.3. Bath Depth
3.4.4. Lance Spacing
3.5. Matrix Analysis of the Orthogonal Experimental Results
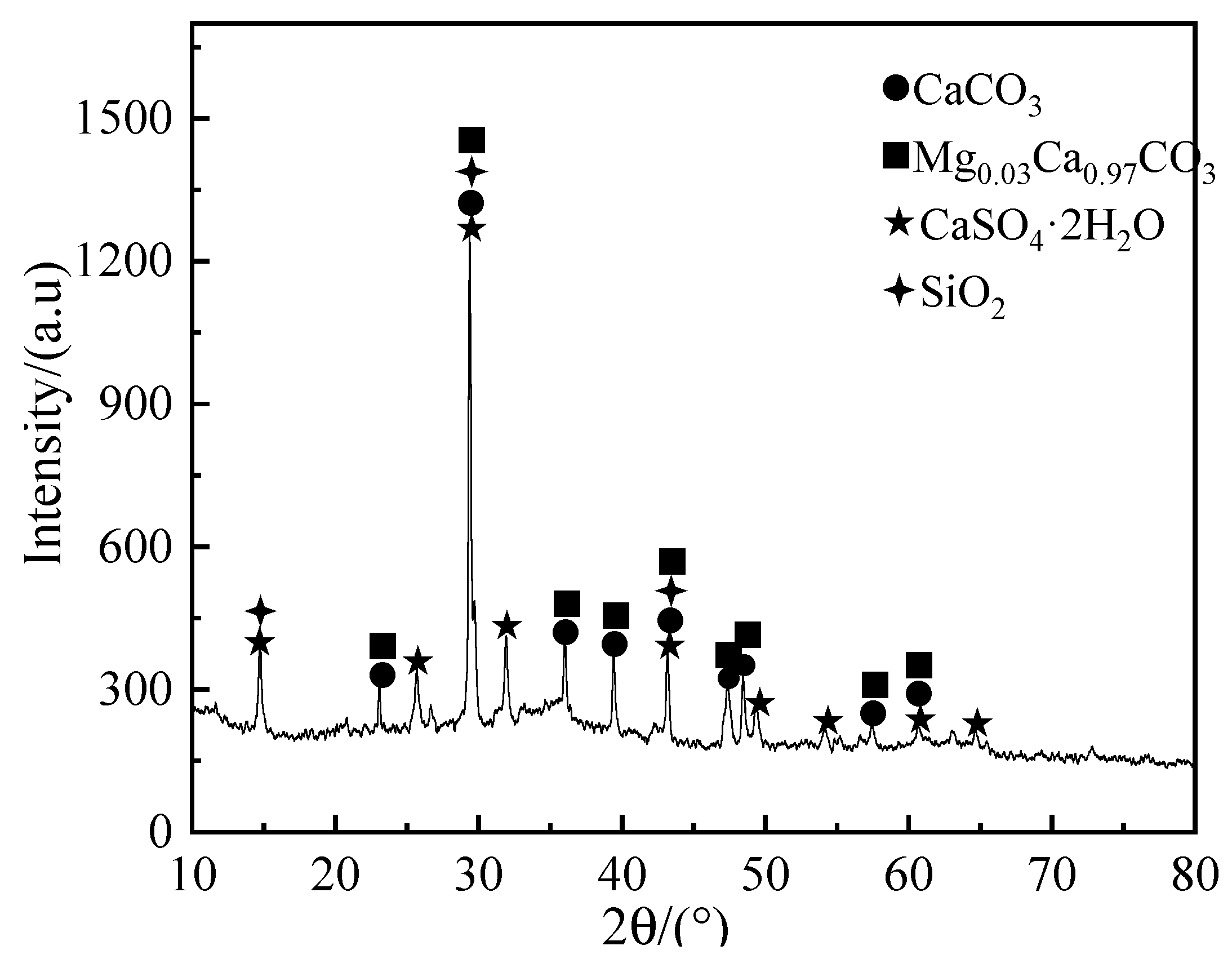
3.6. Industrial Application Verification
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhou, J.Z.; Wu, Y.Y.; Liu, C.; Orpe, A.; Liu, Q.; Xu, Z.P.; Qian, G.R.; Qiao, S.Z. Effective Self-Purification of Polynary Metal Electroplating Wastewaters through Formation of Layered Double Hydroxides. Environ. Sci. Technol. 2010, 44, 8884–8890. [Google Scholar] [CrossRef] [PubMed]
- Li, C.; Xie, F.; Ma, Y.; Cai, T.; Li, H.; Huang, Z.; Yuan, G. Multiple heavy metals extraction and recovery from hazardous electroplating sludge waste via ultrasonically enhanced two-stage acid leaching. J. Hazard. Mater. 2010, 178, 823–833. [Google Scholar] [CrossRef] [PubMed]
- Magalhaes, J.M.; Silva, J.E.; Castro, F.P.; Labrincha, J.A. Physical and chemical characterisation of metal finishing industrial wastes. J. Environ. Manag. 2005, 75, 157–166. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kuchar, D.; Fukuta, T.; Onyango, M.S.; Matsuda, H. Sulfidation treatment of copper-containing plating sludge towards copper resource recovery. J. Hazard. Mater. 2006, 138, 86–94. [Google Scholar] [CrossRef] [PubMed]
- Jordan, M.M.; Almendro-Candel, M.B.; Romero, M.; Rincón, J. Application of sewage sludge in the manufacturing of ceramic tile bodies. Appl. Clay Sci. 2005, 30, 219–224. [Google Scholar] [CrossRef] [Green Version]
- Karlovic, E.S.; Dalmacija, B.D.; Tamas, Z.S.; Prica, M.D.; Ranogajec, J.G. Preliminary evaluation of galvanic sludge immobilization in clay-based matrix as an environmentally safe process. J. Environ. Sci. Health Part A Toxic/Hazard. Subst. Environ. Eng. 2008, 43, 528–537. [Google Scholar] [CrossRef] [PubMed]
- Li, P.; Yao, X.; Guo, T.Y.; Li, D.B. Numerical simulation of gas-liquid mixing characteristics in side-blown bath. Nonferrous Met. Equip. 2019, 2, 16–24. [Google Scholar]
- Huda, N.; Naser, J.; Brooks, G.; Reuter, M.A.; Matusewicz, R.W.; Iaeng, A. Computational Fluid Dynamic Modeling Study of Slag Fuming in Top Submerged Lance Smelting Furnace. In Proceedings of the World Congress on Engineering (WCE 2010), London, UK, 30 June–2 July 2010; International Association of Engineers-IAENG: Hong Kong, China, 2010; pp. 1069–1074. [Google Scholar]
- Lei, M.; Wang, Y.Z.; Zhang, J.Y.; Cheng, W.L.; Zheng, S.B.; Wang, B.; Hong, X. The multiphase flow model simulates fluid flow in a smelting reduction furnace. Chin. J. Proc. Eng. 2009, 9, 420–425. [Google Scholar]
- Yan, H.J.; Liu, F.K.; Zhang, Z.Y.; Gao, Q.; Liu, L.; Cui, Z.X.; Shen, D.B. Influence of lance arrangement on bottom-blowing bath smelting process. Chin. J. Nonferrous Met. 2012, 22, 2393–2400. [Google Scholar]
- Davis, M.; Dry, R.; Schwarz, P.; Symbols, G. Flow simulation of the hismelt process. In Proceedings of the Third International Conference on CFD in the Minerals and Process Industries CSIRO, Melbourne, Australia, 10–12 December 2003. [Google Scholar]
- Yan, H.J.; Xia, T.; Liu, L.; He, Q.A.; He, Z.J.; Li, Q.Q. Numerical simulation and structural optimization of gas-liquid two-phase flow in reduction furnace of lead-rich slag. Chin. J. Nonferrous Met. 2014, 24, 2642–2651. [Google Scholar]
- Li, Y.; Ma, F. The Numerical Simulation and Optimal Design of Nozzle Jet for Gas-fluid Two Phase Flow. In Proceedings of the International Conference on Advanced Engineering Materials and Technology (AEMT2011), Sanya, China, 29–31 July 2011; Volume 291–294, pp. 2403–2406. [Google Scholar] [CrossRef]
- Zhan, S.H.; Lai, Z.B.; Xiao, Z.Q. Agitation in a side-blown metal bath. J. Cent. South Univ. Soc. Sci. 2003, 2, 148–151. [Google Scholar]
- Li, X.L.; Liu, Y.; Wang, D.X.; Zhang, T.A. Physical Simulation of Emulsion Phenomena in Copper Sideblown Smelting Process. J. North Univ. Nat. Sci. 2018, 39, 649–653. [Google Scholar]
- Zhu, Z.Y.; Zhou, P.; Chen, Z.; Long, P.; Zhang, L. Numerical simulation on effect of air injection on two-phase flow in oxygen-enriched side-blown furnace. J. Cent. South Univ. Sci. Technol. 2022, 53, 398–408. [Google Scholar]
- Liu, F.K. Numerical Simulation and Optimization of Mulfiphase Flow in Bottom Blowing Lead Smelting Furnace. Ph.D. Thesis, Central South University, Changsha, China, 2013. [Google Scholar]
- Hamdia, K.M.; Ghasemi, H. Quantifying the uncertainties in modeling soft composites via a multiscale approach. Int. J. Solids Struct. 2022, 256, 111959. [Google Scholar] [CrossRef]
- Bian, Z.; Chen, D.; Sun, L.; Wang, L.; Zhao, H. Numerical Simulation and Experimental Investigation of Multiphase Flow in an Oxygen-Rich Side-Blown Bath Smelting Furnace. SSRN 2022, 4119238, preprint. [Google Scholar] [CrossRef]
- Rabha, S.S.; Buwa, V.V. Volume-of-fluid (VOF) simulations of rise of single/multiple bubbles in sheared liquids. Chem. Eng. Sci. 2010, 65, 527–537. [Google Scholar] [CrossRef]
- Cong, D.; Jian-Zhong, L.; Zhen-Yu, H.; Jun-Hu, Z.; Ke-Fa, C. VOF Method for Impinging-Jet Atomizers. Energy Fuels 2010, 24, 451–455. [Google Scholar] [CrossRef]
- Feng, K.F.; Zhang, J.Y.; Wang, B.; Xu, J.; Xie, J.Y.; Cheng, W.L.; Yin, D.Y.; Zheng, S.B. Numerical Simulation Study on Immersed Side-Blowing in C-H-2 Smelting Reduction Furnace. In Proceedings of the 5th International Symposium on High-Temperature Metallurgical Processing held during 143rd TMS Annual Meeting and Exhibition, San Diego, CA, USA, 16–20 February 2014. [Google Scholar]
- ANSYS. ANSYS Fluent Theory Guide; Fluent Inc.: Canonsburg, PA, USA, 2021; p. 2021. [Google Scholar]
- Zhang, L.; Zhang, L.; He, Y. The Process and Application of Oxygen-Enriched Air Side Blown Smelting of Lead-Zinc Materials. In Proceedings of the 9th International Symposium on Lead and Zinc Processing (PbZn), San Diego, CA, USA, 23–27 February 2020; Springer International Publishing: Cham, Switzerland, 2020; pp. 291–299. [Google Scholar] [CrossRef]
- Sundström, A.W.; Eksteen, J.J.; Georgalli, G.A. A review of the physical properties of base metal mattes. J. South Afr. Inst. Min. Met. 2008, 108, 431–448. [Google Scholar]
- Cai, Z.P.; Liang, Y.; Zhang, C.X. Experimental Study and Measurement Technology of Chemical Metallurgy Model; Metallurgical Industry Press: Beijing, China, 2001. [Google Scholar]
- Xia, T. Numerical Simulation and Optimization of Multiphase Flow in Reduction Furnace of Liquid High Lead Slag. Postgraduate Thesis, Central South University, Changsha, China, 2014. [Google Scholar]
- Cai, Z.P.; Liang, Y.; Qian, Z.M.; Ma, E.X.; Wei, W.S.; He, Y.L.; Wang, L.S. The model experiment study of continuous lead smelting with bottom blowing oxygen (I) the reasonable arrangement of the distance between the bottom blowing gun and the partition wall. Chin. J. Proc. Eng. 1985, 4, 113–122. [Google Scholar]
- Peng, Q.R. Copper Metallurgy; Central South University Press: Changsha, China, 2004. [Google Scholar]
Disclaimer/Publisher’s Note: The statements, opinions, and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions, or products referred to in the content. |
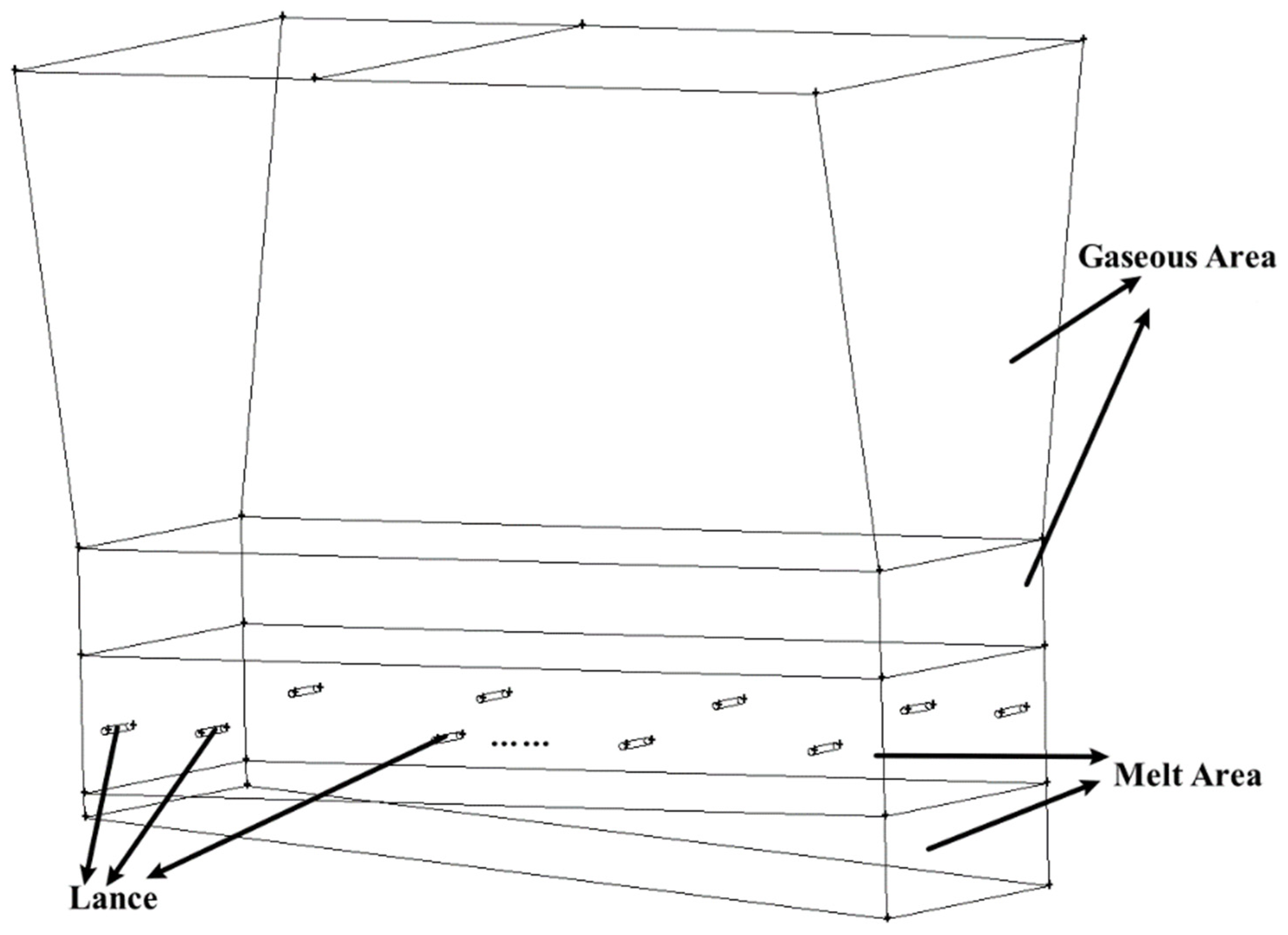


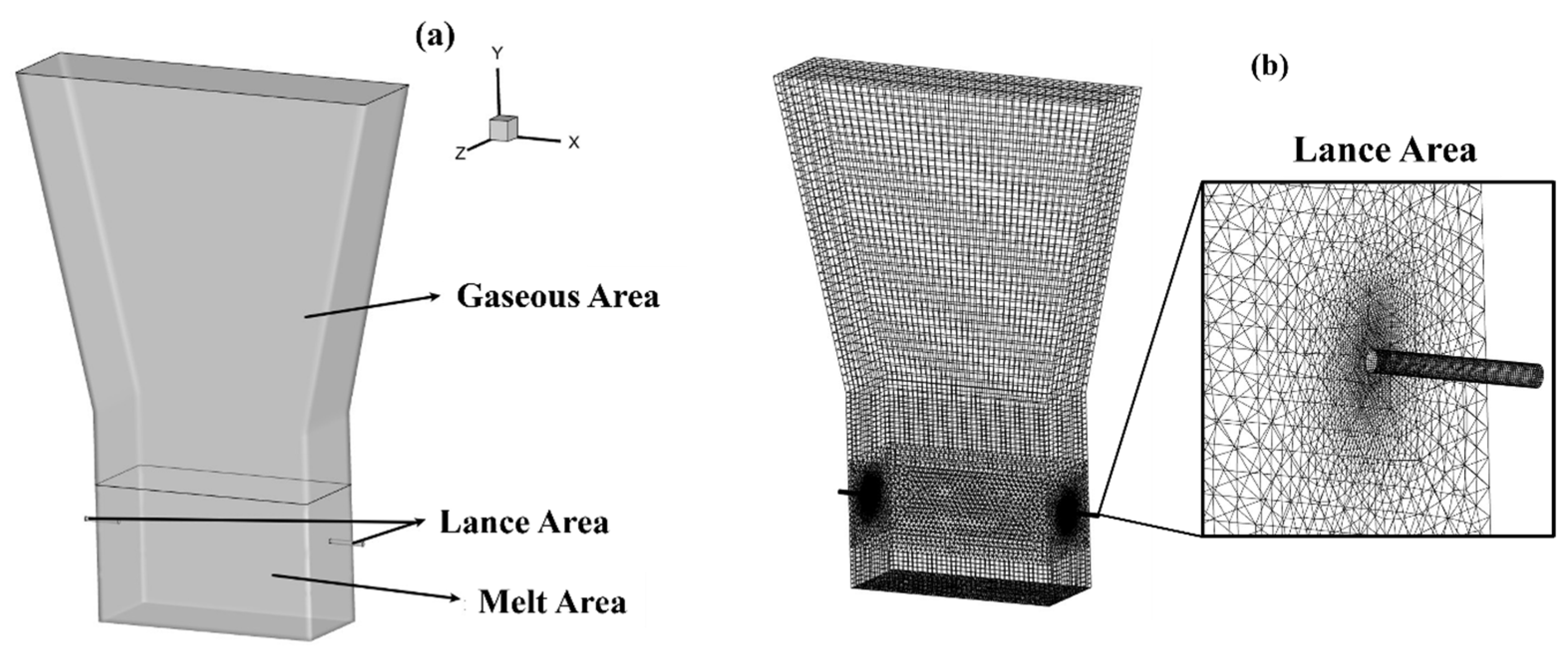






| Zone | Scheme Elements | Scheme Type | Interval Size/Count | Quantity of Elements |
|---|---|---|---|---|
| Gaseous area | hexahedral | Map | Size: 20 | 117,600 |
| Melt area | Tet/Hybrid | TGird | Size: 6 | 570,629 |
| Lance area (Face mesh) | Quadrilateral | Pave | Count: 30 | \ |
| Lance area | Hex/Wedge | Cooper | Size: 4 | 6068 × 10 |
| Fluid | Density/ (kg·m−3) | Temperature/ K | Viscosity/ (kg·m−1·s−1) | Specific Heat Capacity/ (J·kg−1·K−1) | Thermal Conductivity/ (W·m−1·K−1) |
|---|---|---|---|---|---|
| Copper Matte | 4600 | 1573.15 | 0.0022 | 607 | 0.04381 |
| Oxygen-enriched Air | 1.235 | 298.15 | 1.817 × 10−5 | 967.854 | 0.02444 |
| Factors | Level 1 | Level 2 | Level 3 |
|---|---|---|---|
| A Lance Diameter/mm | 25 | 30 | 35 |
| B Lance Inclination/° | 12 | 15 | 18 |
| C Lance Spacing/mm | 850 | 950 | 1050 |
| D Bath Depth/mm | 1450 | 1500 | 1550 |
| Experiment Number | Level of Lance Diameter | Level of Lance Inclination | Level of Lance Spacing | Level of Bath Depth |
|---|---|---|---|---|
| 1 | 1 | 1 | 1 | 1 |
| 2 | 1 | 2 | 2 | 2 |
| 3 | 1 | 3 | 3 | 3 |
| 4 | 2 | 1 | 2 | 3 |
| 5 | 2 | 2 | 3 | 1 |
| 6 | 2 | 3 | 1 | 2 |
| 7 | 3 | 1 | 3 | 2 |
| 8 | 3 | 2 | 1 | 3 |
| 9 | 3 | 3 | 2 | 1 |
| Element Number | 322,445 | 748,909 | 1,044,726 |
|---|---|---|---|
| Average Velocity (m/s) | 0.37 | 0.319 | 0.32 |
| Result | Water Model Experimental | Numerical Simulation | Error/% |
|---|---|---|---|
| db/dn 1) | 6.93 | 7.1 | 2.39 |
| Experiment Number | Lance Diameter /mm | Lance Inclination /° | Lance Spacing /mm | Bath Depth /mm | Average Velocity /ms−1 | Turbulent Kinetic Energy /m2 s2 | Bath Gas Rate /% |
|---|---|---|---|---|---|---|---|
| 1 | 1 | 1 | 1 | 1 | 0.248 | 0.0275 | 6.87 |
| 2 | 1 | 2 | 2 | 2 | 0.272 | 0.0333 | 7.91 |
| 3 | 1 | 3 | 3 | 3 | 0.280 | 0.0316 | 8.02 |
| 4 | 2 | 1 | 2 | 3 | 0.249 | 0.0218 | 7.61 |
| 5 | 2 | 2 | 3 | 1 | 0.233 | 0.0251 | 6.68 |
| 6 | 2 | 3 | 1 | 2 | 0.270 | 0.0258 | 7.64 |
| 7 | 3 | 1 | 3 | 2 | 0.314 | 0.0162 | 7.48 |
| 8 | 3 | 2 | 1 | 3 | 0.291 | 0.02 | 8.21 |
| 9 | 3 | 3 | 2 | 1 | 0.241 | 0.0178 | 7.23 |
| K1 | 0.2667 | 0.2703 | 0.2697 | 0.2407 | Intuitive analysis of average velocity | ||
| K2 | 0.2507 | 0.2653 | 0.254 | 0.2853 | |||
| K3 | 0.282 | 0.2637 | 0.2757 | 0.2733 | |||
| R | 0.0313 | 0.0066 | 0.0217 | 0.0446 | |||
| K1 | 0.0308 | 0.0218 | 0.0244 | 0.0235 | Intuitive analysis of turbulent kinetic energy | ||
| K2 | 0.0242 | 0.0261 | 0.0243 | 0.0251 | |||
| K3 | 0.018 | 0.0251 | 0.0243 | 0.0245 | |||
| R | 0.0128 | 0.0043 | 0.0001 | 0.0016 | |||
| K1 | 7.6 | 7.32 | 7.5733 | 6.9267 | Intuitive analysis of bath gas rate | ||
| K2 | 7.31 | 7.6 | 7.5833 | 7.6767 | |||
| K3 | 7.64 | 7.63 | 7.3933 | 7.9467 | |||
| R | 0.33 | 0.31 | 0.19 | 1.02 | |||
| Elements | Fe | Ca | Cu | Al | Si | Sn | Mg | Ni | Mn | C | S |
|---|---|---|---|---|---|---|---|---|---|---|---|
| Wt% | 10.34 | 10.95 | 6.64~7.50 | 2.51 | 1.61 | 0.86 | 0.91 | 0.50 | 0.37 | 7.58 | 5.89 |
| Smelting Method | Chemical Composition/wt% | |||||
|---|---|---|---|---|---|---|
| Cu | Fe | S | Ni | Zn | Pb | |
| ES | 46.56 | 21.21 | 21.98 | 3.11 | 0.07 | 0.06 |
| Closed blast furnace (Oxygen-enriched air) | 41.57 | 28.66 | 23.79 | - | - | - |
| Otokunp | 52.46 | 19.81 | 22.37 | 0.23 | ||
| Vanukov | 41–55 | 25–14 | 22–24 | 4.5–5.2 | - | - |
| Ausmelt | 44.50 | 23.60 | 23.80 | - | 3.20 | - |
| Mitsubishi | 65.70 | 9.20 | 21.90 | - | - | - |
| Smelting Method | Chemical Composition/wt% | |||||||
|---|---|---|---|---|---|---|---|---|
| Cu | Fe | Fe3O4 | SiO2 | S | Al2O3 | CaO | MgO | |
| ES | 0.27 | 25.86 | 36.5 | 0.3 | 5.9 | 19.5 | 0.95 | |
| Closed blast furnace (Oxygen-enriched air) | 0.42 | 29.00 | - | 38.0 | - | 7.5 | 11.0 | 0.74 |
| Vanukov | 0.45 | 35.00 | 3.15 | 35.0 | 0.7 | 3.8 | 8.0 | 1.40 |
| Otokunp | 0.78 | 44.06 | - | 29.7 | 1.4 | 7.8 | 0.6 | - |
| Mitsubishi | 0.60 | 38.20 | - | 32.2 | 0.6 | 2.9 | 5.9 | - |
| Ausmelt | 0.65 | 34.00 | 7.50 | 31.0 | 2.8 | 7.5 | 5.0 | - |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, B.; Liu, W.; Jiao, F.; Zhang, L.; Qin, W.; Jiang, S. Numerical Simulation and Application of an Oxygen-Enriched Side-Blown Smelting Furnace for the Treatment of Electroplating Sludge. Sustainability 2023, 15, 10721. https://doi.org/10.3390/su151310721
Yang B, Liu W, Jiao F, Zhang L, Qin W, Jiang S. Numerical Simulation and Application of an Oxygen-Enriched Side-Blown Smelting Furnace for the Treatment of Electroplating Sludge. Sustainability. 2023; 15(13):10721. https://doi.org/10.3390/su151310721
Chicago/Turabian StyleYang, Biwei, Wei Liu, Fen Jiao, Lin Zhang, Wenqing Qin, and Shanqin Jiang. 2023. "Numerical Simulation and Application of an Oxygen-Enriched Side-Blown Smelting Furnace for the Treatment of Electroplating Sludge" Sustainability 15, no. 13: 10721. https://doi.org/10.3390/su151310721
APA StyleYang, B., Liu, W., Jiao, F., Zhang, L., Qin, W., & Jiang, S. (2023). Numerical Simulation and Application of an Oxygen-Enriched Side-Blown Smelting Furnace for the Treatment of Electroplating Sludge. Sustainability, 15(13), 10721. https://doi.org/10.3390/su151310721







