DSM Formula for Local-Global Interaction Buckling of Cold-Formed Stainless Steel I-Beams
Abstract
:1. Introduction
2. Concept of DSM Design Method
2.1. General Format of a DSM Formula
2.2. Accounting for Interaction Buckling Effect with DSM
3. DSM Formula for Stainless Steel I-Beams
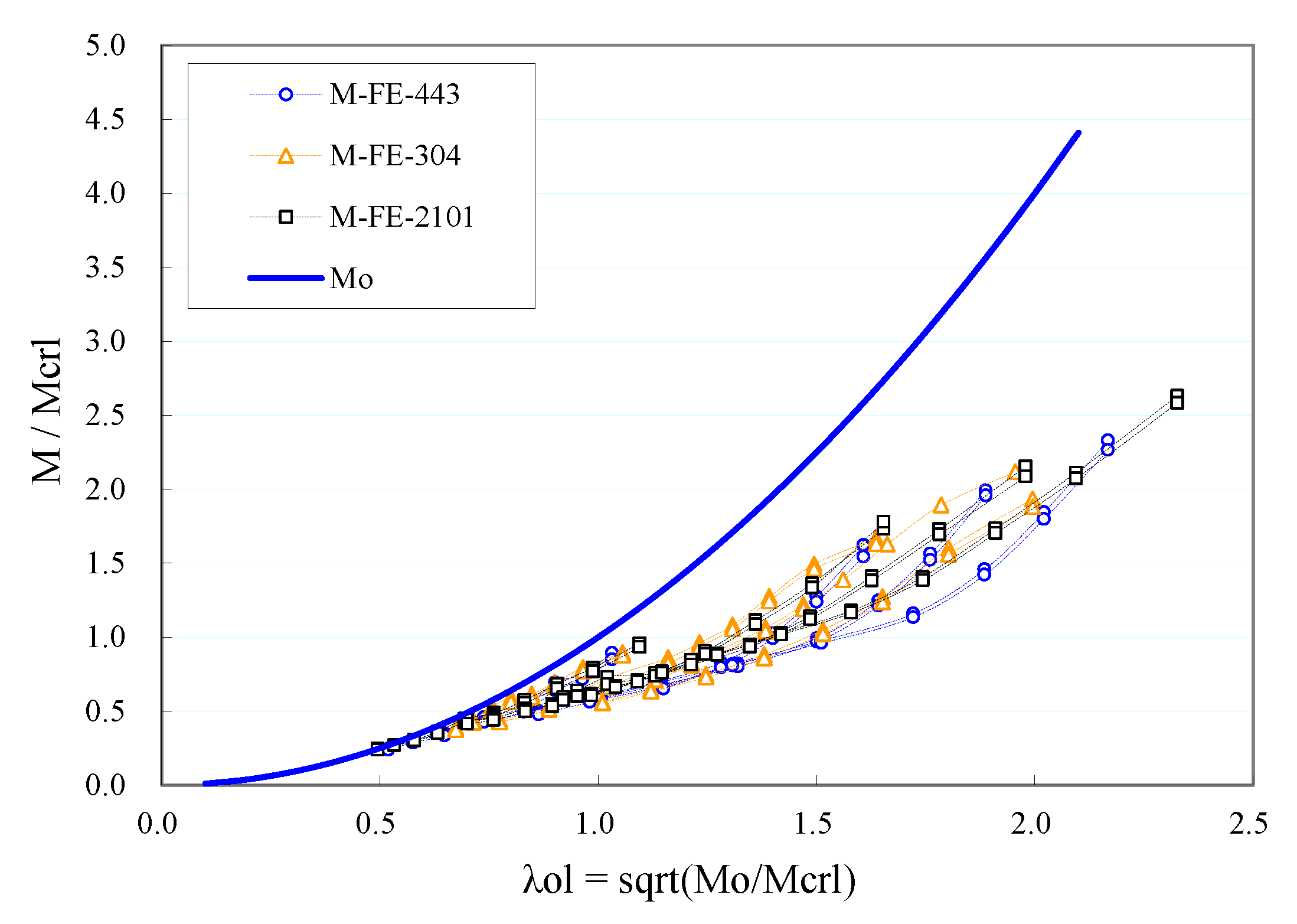
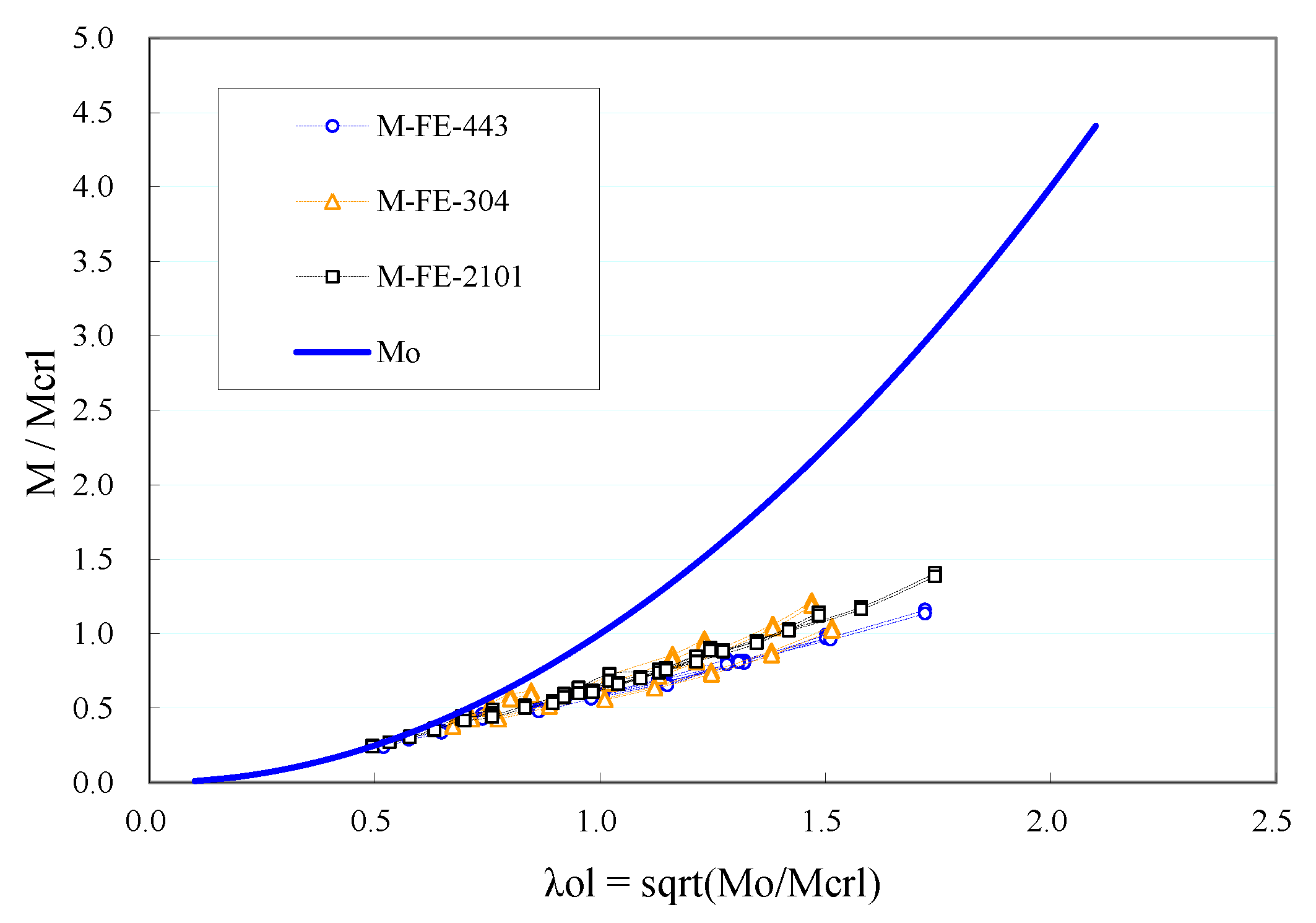
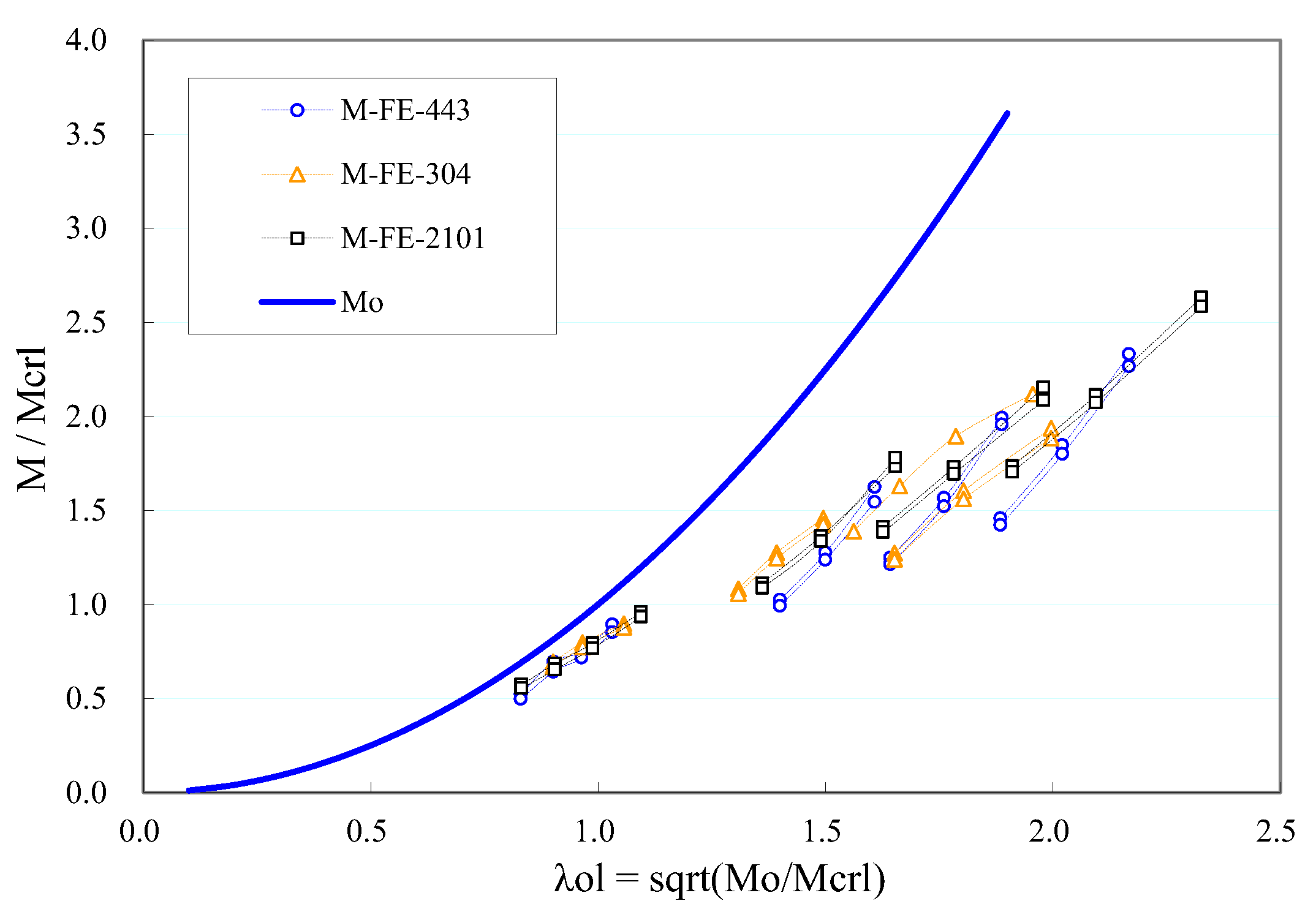
3.1. A Database for Local-Global Interaction Buckling of Stainless Steel Beams
3.2. Current DSM Formula of the Best Performance
3.3. Proposed DSM for Stainless Steel I-Section Beams
3.4. A Novel Two-Phase DSM Formula
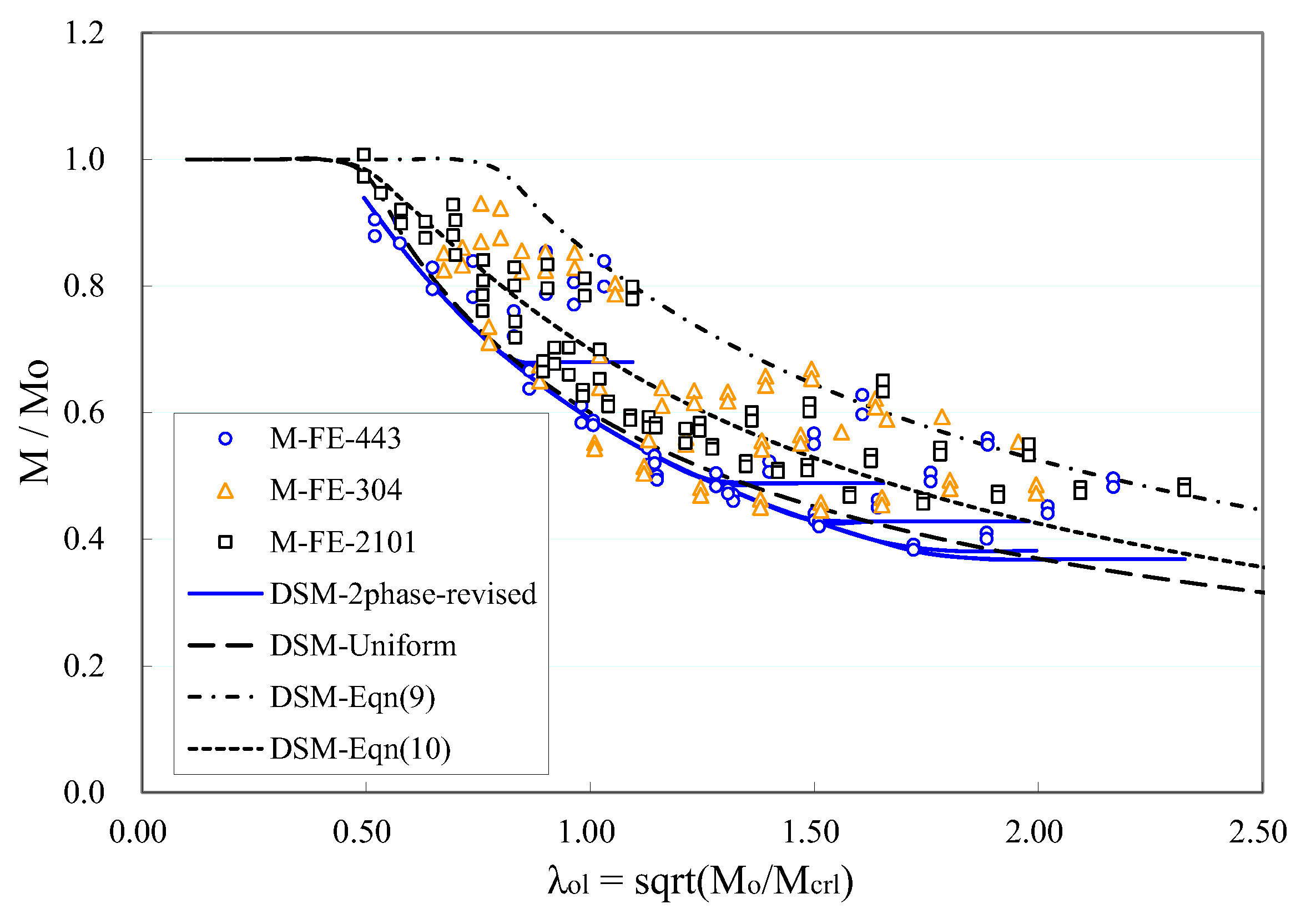
3.5. Revised Two-Phase DSM Formula
4. Conclusions Remarks
- Existing DSM formulae in literature were found incapable of accurately predicting the strength of cold-formed stainless steel beams and lacking a due level of reliability. A traditional-form DSM equation was regressed from the collected strength database, offering improved accuracy and adequate reliability level.
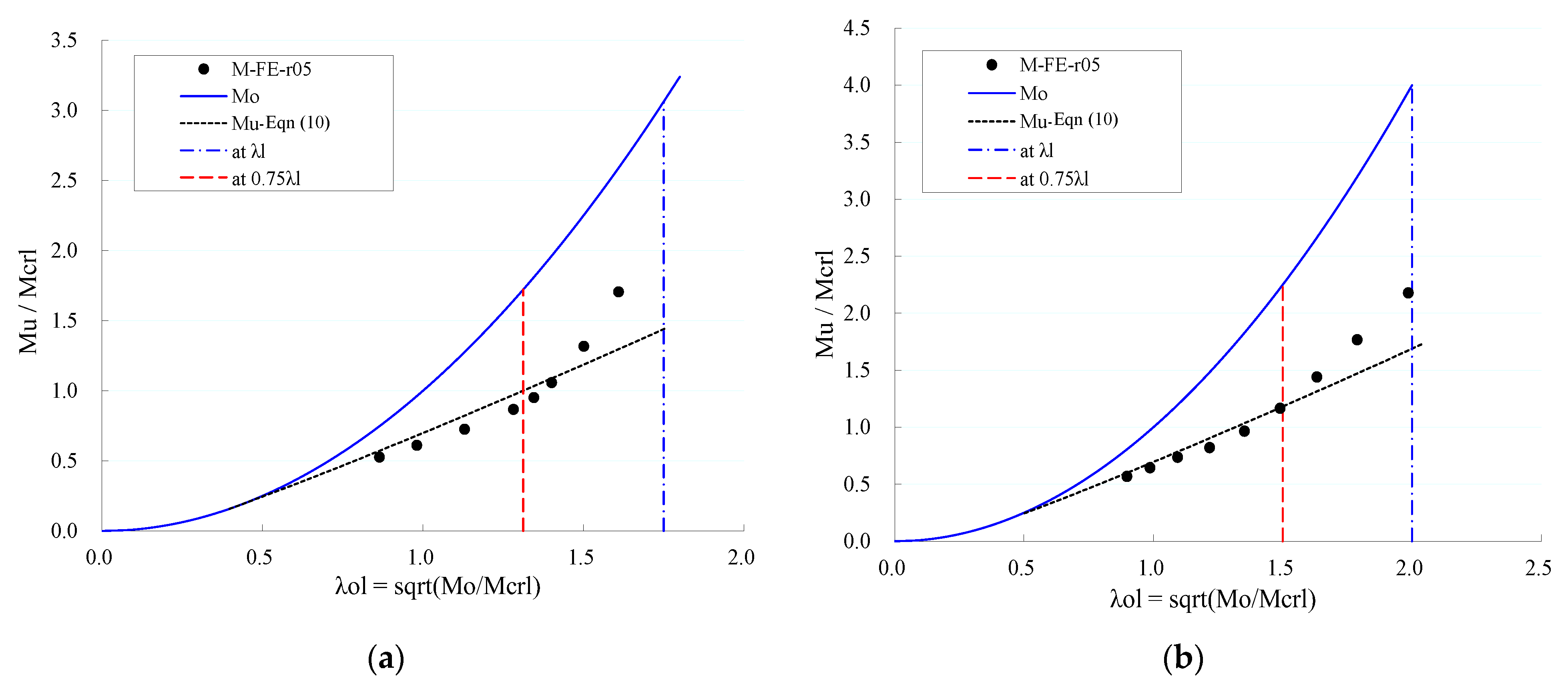
- Cold-formed stainless steel I-beams could be distinguished as “long” or “short” members with criteria (i) short members λol > 0.75λl and (ii) long members λol < 0.75λl, where λl and λol are “section slenderness” and “relative slenderness” defined in Equation (7) and Equation (8) of the paper.
- Varying trend of the member strengths differs considerably between the ‘long’ and ‘short’ members. A fundamental drawback of the traditional-form DSM formula was observed, viz. the mathematical expression hindered it from accurately following the strength varying trend of stainless steel I-beams and therefore resulting in generally high deviation in predictions and undue conservatism for intermediate and short members.
- Novel two-phase DSM was therefore proposed with a prescribed level of reliability. The novel two-phase DSM formula was found to predict member strengths more accurately over the whole slenderness range, resulting in strength predictions with much lower deviation from actual strengths. Enhanced design strengths by up to 39% could be obtained from the two-phase DSM formula for members with intermediate and short spans.
- A revised version of the two-phase DSM was further proposed, which simplified the formula expression at some cost of the member strength of short members.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Hancock, G.J.; Kwon, Y.B.; Stefan Bernard, E. Strength design curves for thin-walled sections undergoing distortional buckling. J. Constr. Steel Res. 1994, 31, 169–186. [Google Scholar] [CrossRef]
- Schafer, B.W.; Peköz, T. Direct Strength Prediction of Cold-Formed Members Using Numerical Elastic Buckling Solutions. In Proceedings of the Fourteenth International Specialty Conference on Cold-Formed Steel Structures, St. Louis, MO, USA, 15 October 1998. [Google Scholar]
- Papangelis, J.P.; Hancock, G.J. Computer analysis of thin-walled structural members. Comput. Struct. 1995, 56, 157–176. [Google Scholar] [CrossRef]
- Schafer, B.W.; Adany, S. Buckling analysis of cold-formed steel members using CUFSM: Conventional and constrained finite strip methods. In Proceedings of the 18th International Specialty Conference on Cold-Formed Steel Structures: Recent Research and Developments in Cold-Formed Steel Design and Construction, University of Missouri-Rolla, Orlando, FL, USA, 26–27 October 2006. [Google Scholar]
- Bebiano, R.; Camotim, D.; Goncalves, R. GBTUL 2.0 A second-generation code for the GBT-based buckling and vibration analysis of thin-walled members. Thin-Walled Struct. 2018, 124, 235–257. [Google Scholar] [CrossRef]
- Schafer, B.W. Local, Distortional, and Euler Buckling of Thin-Walled Columns. J. Struct. Eng. ASCE 2002, 128, 289–299. [Google Scholar] [CrossRef] [Green Version]
- Schafer, B.W. Review: The Direct Strength Method of cold-formed steel member design. J. Constr. Steel Res. 2008, 64, 766–778. [Google Scholar] [CrossRef]
- Pham, C.H.; Hancock, G.J. Direct Strength Design of Cold-Formed Purlins; Research Report; Department of Civil Engineering, University of Sydney: Sydney, Australia, 2007; pp. 1–37. [Google Scholar]
- Papangelis, J.; Trahair, N.; Hancock, G. Direct strength method for shear capacity of beams with corrugated webs. J. Constr. Steel Res. 2017, 137, 152–160. [Google Scholar] [CrossRef]
- Tovar, J.; Sputo, T. Application of direct strength method to axially loaded perforated cold-formed steel studs: Distortional and local buckling. Thin-Walled Struct. 2005, 43, 1882–1912. [Google Scholar] [CrossRef]
- Lecce, M. Distortional Buckling of Stainless Steel Sections; University of Sydney: Sydney, Australia, 2006. [Google Scholar]
- Becque, J.; Lecce, M.; Rasmussen, K.J.R. The direct strength method for stainless steel compression members. J. Constr. Steel Res. 2008, 64, 1231–1238. [Google Scholar] [CrossRef]
- Rossi, B.; Jaspart, J.P.; Rasmussen, K.J.R. Combined Distortional and Overall Flexural-Torsional Buckling of Cold-Formed Stainless Steel Sections: Design. J. Struct. Eng. 2010, 136, 354–360. [Google Scholar] [CrossRef]
- Chen, M.; Fan, S.; Li, C.; Zeng, S. Direct strength method for stainless steel lipped channel columns undergoing local buckling. Int. J. Steel Struct. 2020, 20, 1822–1830. [Google Scholar] [CrossRef]
- Zhu, J.H.; Young, B. Aluminum alloy tubular columns—Part II: Parametric study and design using direct strength method. Thin-Walled Struct. 2006, 44, 969–985. [Google Scholar] [CrossRef]
- AISI S100; North American Cold-formed Steel Specification for the Design of Cold-formed Steel Structural Members. American Iron and Steel Institute: Washington, DC, USA, 2012.
- AS/NZS4600; Australian/New Zealand Standard: Cold-Formed Steel Structures. Standards Australia: Sydney, Australia; Standards New Zealand: Wellington, New Zealand, 2018.
- Arrayago, I.; Rasmussen, K.J.R.; Real, E. Full slenderness range DSM approach for stainless steel hollow cross-sections. J. Constr. Steel Res. 2017, 133, 156–166. [Google Scholar] [CrossRef]
- Arrayago, I.; Real, E.; Mirambell, E. Design of stainless steel continuous beams with tubular cross-sections. Eng. Struct. 2017, 151, 422–431. [Google Scholar] [CrossRef]
- Huang, Y.; Young, B. Experimental and numerical investigation of cold-formed lean duplex stainless steel flexural members. Thin-Walled Struct. 2013, 73, 216–228. [Google Scholar] [CrossRef] [Green Version]
- Zhou, F.; Young, B. Tests of cold-formed stainless steel tubular flexural members. Thin-Walled Struct. 2005, 43, 1325–1337. [Google Scholar] [CrossRef]
- Li, H.-T.; Young, B. Web crippling of cold-formed ferritic stainless steel square and rectangular hollow sections. Eng. Struct. 2018, 176, 968–980. [Google Scholar] [CrossRef]
- Theofanous, M.; Gardner, L. Experimental and numerical studies of lean duplex stainless steel beams. J. Constr. Steel Res. 2010, 66, 816–825.24. [Google Scholar] [CrossRef]
- Lepcha, K.H.; Patton, M.L. A numerical study on structural behaviour of lean duplex stainless steel tubular beams with rectangular web openings. Structures 2021, 32, 1233–1249. [Google Scholar] [CrossRef]
- Saliba, N.; Gardner, L. Cross-section stability of lean duplex stainless steel welded I-sections. J. Constr. Steel Res. 2013, 80, 1–14. [Google Scholar] [CrossRef]
- Sun, Y.; Zhao, O. Material response and local stability of high-chromium stainless steel welded I-sections. Eng. Struct. 2019, 178, 212–226. [Google Scholar] [CrossRef]
- Lalthazuala, R.; Singh, K.D. Investigations on structural performance of hybrid stainless steel I-beams based on slenderness. Thin-Walled Struct. 2019, 137, 197–212. [Google Scholar] [CrossRef]
- Zhou, Y.; Uy, B.; Wang, J.; Li, D. Behaviour and design of welded stainless steel beams with compact sections under flexure and shear. J. Constr. Steel Res. 2021, 187, 106996. [Google Scholar] [CrossRef]
- Hassanein, M.F.; Silvestre, N. Flexural behavior of lean duplex stainless steel girders with slender unstiffened webs. J. Constr. Steel Res. 2013, 85, 12–23. [Google Scholar] [CrossRef]
- Chen, X.W.; Yuan, H.X.; Real, E.; Du, X.X.; Schafer, B.W. Experimental behaviour of stainless steel plate girders under combined bending and shear. J. Constr. Steel Res. 2020, 166, 105900. [Google Scholar] [CrossRef]
- Niu, S.; Rasmussen, K.J.R.; Fan, F. Distortional-Global Interaction Buckling of Stainless Steel C-beams: Part I—Experimental Investigation. J. Constr. Steel Res. 2014, 96, 127–139. [Google Scholar] [CrossRef]
- Fan, S.; Chen, M.; Li, S.; Ding, Z.; Shu, G.; Zheng, B. Stainless steel lipped C-section beams: Numerical modelling and development of design rules. J. Constr. Steel Res. 2019, 152, 29–41. [Google Scholar] [CrossRef]
- Karthik, C.; Anbarasu, M.; Dar, M.A. Cold-formed ferritic stainless steel closed-section built-up beams: Tests and flexural response. Thin-Walled Struct. 2022, 180, 109820. [Google Scholar] [CrossRef]
- Niu, S.; Rasmussen, K.J.R.; Fan, F. Local-Global Interaction Buckling of Stainless Steel I-Beams. I: Experimental Investigation. J. Struct. Eng. 2015, 141, 04014194. [Google Scholar] [CrossRef]
- Niu, S.; Rasmussen, K.J.; Fan, F. Local–global interaction buckling of stainless steel I-beams. II: Numerical study and design. J. Struct. Eng. 2015, 141, 04014195. [Google Scholar] [CrossRef]
- Niu, S.; Rasmussen, K.J.R. Numerical Study of the Sectional-Global Interaction Buckling of Stainless Steel Beams; Research Report no. R945; School of Civil Engineering, University of Sydney: Sydney, Australia, 2013. [Google Scholar]
- AS/NZS4673; Australian/New Zealand Standard: Cold-Formed Stainless Steel Structures. Standards Australia: Sydney, Australia; Standards New Zealand: Wellington, New Zealand, 2001.
- ASTM E527; Standard Practice for Numbering Metals and Alloys in the Unified Numbering System (UNS). American Society for Testing and Materials: West Conshohocken, PA, USA, 2012.
- Lin, S.H.; Yu, W.W.; Galambos, T.V. Load and Resistance Factor Design of Cold-Formed Stainless Steel: Statistical Analysis of Material Properties and Development of the LRFD Provision; Fourth Progress Report; Univ. of Missouri-Rolla: Rolla, MO, USA, 1988. [Google Scholar]





| Section | H (mm) | B (mm) | T (mm) | R (mm) | fcrl (MPa) | E0 (GPa) | f0.2 (MPa) | n |
|---|---|---|---|---|---|---|---|---|
| 304-I-L11 | 180 | 130 | 2.30 | 3.5 | 216 | 210 | 246 | 5.1 |
| 304-I-L17 | 180 | 130 | 1.48 | 3.5 | 89 | |||
| 304-I-L20 | 180 | 130 | 1.25 | 3.5 | 63 | |||
| 304-I-L23 | 180 | 97 | 0.85 | 3.5 | 50 | |||
| 443-I-L11 | 180 | 100 | 1.90 | 3.0 | 227 | 197 | 286 | 8.2 |
| 443-I-L17 | 180 | 100 | 1.22 | 3.0 | 93 | |||
| 443-I-L20 | 180 | 100 | 1.05 | 3.0 | 69 | |||
| 443-I-L24 | 180 | 100 | 0.90 | 3.0 | 51 | |||
| 2101-I-L11 | 176.5 | 130 | 3.00 | 2.6 | 350 | 200 | 436 | 5.0 |
| 2101-I-L17 | 176.5 | 130 | 1.98 | 2.6 | 152 | |||
| 2101-I-L20 | 176.5 | 130 | 1.65 | 2.6 | 105 | |||
| 2101-I-L24 | 176.5 | 130 | 1.40 | 2.6 | 76 |
| Section | Parametric Study Results |
|---|---|
| 443-I-L11 (λl = 1.1) | L = 1.0, 1.5, 2.0, 2.5, 3.0, 3.5, 4.0, 4.5 m M-FE05 = 10.81, 9.06, 8.4, 6.33, 5.54, 4.21, 3.62, 2.96 kNm M-FE10 = 10.29, 8.66, 7.74, 6.0, 5.17, 4.04, 3.48, 2.87 kNm |
| 443-I-L17 (λl = 1.7) | L= 1.0, 1.5, 2.0, 2.5, 3.0, 3.5, 4.0 m M-FE05 = 5.19, 4.08, 3.28, 2.65, 2.22, 1.88, 1.59 kNm M-FE10 = 4.94, 3.96, 3.17, 2.54, 2.11, 1.80, 1.52 kNm |
| 443-I-L20 (λl = 2.0) | L = 1.0, 1.5, 2.0, 2.5, 3.0, 3.5, 4.0 m M-FE05 = 5.19, 4.08, 3.28, 2.65, 2.22, 1.88, 1.59 kNm M-FE10 = 4.94, 3.96, 3.17, 2.54, 2.11, 1.80, 1.52 kNm |
| 443-I-L24 (λl = 2.4) | L = 1.0, 1.5, 2.0, 2.5, 3.0, 3.5, 4.0 m M-FE05 = 3.03, 2.40, 1.89, 1.51, 1.27, 1.06, 0.86 kNm M-FE10 = 2.94, 2.34, 1.85, 1.48, 1.25, 1.05, 0.85 kNm |
| 304-I-L11 (λl = 1.1) | L = 1.0, 1.5, 2.0, 2.5, 3.0, 3.5, 4.0, 4.5 m M-FE05 = 14.78, 13.12, 11.42, 10.14, 9.75, 8.79, 7.26, 6.38 kNm M-FE10 = 14.47, 12.74, 11.03, 9.75, 9.26, 8.22, 7.02, 6.18 kNm |
| 304-I-L17 (λl = 1.7) | L= 1.0, 1.5, 2.0, 2.5, 3.0, 3.5, 4.5, 5.5, 6.5 m M-FE05 = 7.34, 6.57, 5.60, 4.76, 4.24, 3.79, 3.17, 2.34, 1.94 kNm M-FE10 = 7.18, 6.42, 5.48, 4.65, 4.11, 3.62, 2.93, 2.25, 1.87 kNm |
| 304-I-L20 (λl = 2.0) | L= 1.0, 1.5, 2.0, 2.5, 3.0, 3.5, 4.5, 5.0 m M-FE05 = 5.5, 4.92, 4.23, 3.61, 3.17, 2.76, 2.15, \ kNm M-FE10 = \, \, \, \, 3.10, 2.70, 2.11, 1.85 kNm |
| 304-I-L23 (λl = 2.3) | L = 1.0, 1.5, 2.0, 2.5, 3.0, 3.5, 4.0, 4.5 m M-FE05 = 2.32, 1.92, 1.53, 1.26, 1.06, 0.9, 0.78, 0.68 kNm M-FE10 = 2.26, 1.87, 1.49, 1.23, 1.03, 0.87, 0.76, 0.67 kNm |
| 2101-I-L11 (λl = 1.1) | L = 1.0, 1.5, 2.0, 2.5, 3.0, 3.5, 4.0, 4.5, 5.0, 5.5 m M-FE05 = 32.55, 26.95, 23.23, 19.51, 16.58, 15.2, 12.26, 10.47, 9.14, 8.39 kNm M-FE10 = 31.72, 26.03, 22.19, 18.83, 15.94, 14.42, 11.90, 10.22, \, 8.10 kNm |
| 2101-I-L17 (λl = 1.7) | L = 1.0, 1.5, 2.0, 2.5, 3.0, 3.5, 4.0, 4.5, 5.0, 5.5 m M-FE05 = 16.97, 13.35, 10.9, 8.86, 7.43, 7.14, 5.83, 5.06, 4.45, 4.33 kNm M-FE10 = 17.43, 13.08, 10.65, 8.66, 7.23, 6.68, 5.61, 4.89, 4.31, 4.07 kNm |
| 2101-I-L20 (λl = 2.0) | L = 1.0, 1.5, 2.0, 2.5, 3.0, 3.5, 4.0, 4.5, 5.0 m M-FE05 = 12.27, 9.85, 8.04, 6.51, 5.41, 4.82, 4.03, 3.51, 3.11 kNm M-FE10 = 11.88, 9.65, 7.88, 6.39, 5.33, 4.63, 3.98, 3.45, 3.03 kNm |
| 2101-I-L24 (λl = 2.4) | L = 1.0, 1.5, 2.0, 2.5, 3.0, 3.5, 4.0, 4.5, 5.0, 5.5 m M-FE05 = 9.21, 7.39, 6.07, 4.92, 4.13, 3.6, 3.11, 2.68, 2.34, 2.23 kNm M-FE10 = 9.04, 7.25, 5.96, 4.84, 4.07, 3.56, 3.08, 2.65, 2.31, 2.09 kNm |
| Curve | a | b | c | Pm | VP | β | φ |
|---|---|---|---|---|---|---|---|
| DSM-uniform | 0.60 | 0.0 | 0.70 | 1.15 | 0.14 | 2.5 | 0.90 |
| Section | λl | λTrans | λTrans/λl |
|---|---|---|---|
| 443-I-L11 | 1.10 | 0.85 | 0.77 |
| 443-I-L17 | 1.70 | 1.35 | 0.79 |
| 443-I-L20 | 2.00 | 1.56 | 0.78 |
| 443-I-L24 | 2.40 | 1.85 | 0.77 |
| 304-I-L11 | 1.10 | 0.83 | 0.75 |
| 304-I-L17 | 1.70 | 1.23 | 0.72 |
| 304-I-L20 | 2.00 | 1.40 | 0.70 |
| 304-I-L23 | 2.30 | 1.53 | 0.67 |
| 2101-I-L11 | 1.10 | 0.86 | 0.78 |
| 2101-I-L17 | 1.70 | 1.31 | 0.77 |
| 2101-I-L20 | 2.00 | 1.50 | 0.75 |
| 2101-I-L24 | 2.40 | 1.85 | 0.77 |
| Average | / | / | 0.75 |
| Curve | a | b | c | Pm | VP | β | φ |
|---|---|---|---|---|---|---|---|
| Long members | 0.70 | 0.11 | 0.93 | 1.08 | 0.08 | 2.5 | 0.90 |
| Short members | 1.74 | 0.47 + 0.47λl | 0.43 | 1.08 | 0.05 | 2.5 | 0.90 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, Y.; Niu, S.; Zhi, X. DSM Formula for Local-Global Interaction Buckling of Cold-Formed Stainless Steel I-Beams. Sustainability 2023, 15, 1333. https://doi.org/10.3390/su15021333
Yang Y, Niu S, Zhi X. DSM Formula for Local-Global Interaction Buckling of Cold-Formed Stainless Steel I-Beams. Sustainability. 2023; 15(2):1333. https://doi.org/10.3390/su15021333
Chicago/Turabian StyleYang, Yueming, Shuang Niu, and Xudong Zhi. 2023. "DSM Formula for Local-Global Interaction Buckling of Cold-Formed Stainless Steel I-Beams" Sustainability 15, no. 2: 1333. https://doi.org/10.3390/su15021333
APA StyleYang, Y., Niu, S., & Zhi, X. (2023). DSM Formula for Local-Global Interaction Buckling of Cold-Formed Stainless Steel I-Beams. Sustainability, 15(2), 1333. https://doi.org/10.3390/su15021333









