How Is Educational Gamification Represented in School Curriculum? An Investigation of Chinese Secondary Mathematics Textbooks

Abstract

Textbooks, as potentially implemented curriculum, play an important role in school education. Earlier studies in mathematics education revealed that teaching using mathematics games had a positive effect on students’ learning. However, how mathematics games are presented in mathematics textbooks has rarely been systematically examined. In this study, we aimed to investigate how mathematics games are presented in school mathematics textbooks in China. We selected three series of Chinese secondary mathematics textbooks (Grades 7–9) and identified 112 mathematics games in total; then, we coded and analyzed the games according to an analysis framework we established for the study. The results showed that, across the three series of textbooks, the distribution of games was inconsistent and, within the same series of textbooks, it was irregular across the different grade levels; in terms of locations and cognitive objectives, most games were presented as exercise questions and the main purpose was to improve students’ problem solving ability. Moreover, most of the games were single player games and there were slightly more competitive games than non-competitive games. The implications of the findings for the design and research of mathematics games in mathematics textbooks are discussed at the end of the study.

1. Introduction

Education researchers, policy makers and practitioners have increasingly recognized that textbooks, as potentially implemented curriculum [1], play an important role in school teaching and learning, and that is particularly the case in mathematics teaching and learning. Accordingly, mathematics textbook research has attracted growing attention from researchers in mathematics education over the last two decades, as revealed in a survey study conducted by Fan et al. [2]. This fact can also be seen from a series of international conferences on mathematics textbook research and development (ICMT) held since 2014 and three special issues focusing on mathematics textbook research published by ZDM-Mathematics Education since 2013 [3].

According to the results of the Programme for International Student Assessment (PISA), students in China, as in some other east Asian countries or regions, are top performers [4]; however, research has also shown that Chinese students had often more negative attitude towards learning mathematics [5]. In this connection, over the last three decades, Chinese mathematics curriculum reform has paid much attention to promoting students’ interest in mathematics, which is also emphasized in the development of school mathematics textbooks in China [6].

This study focuses on how gamification is represented in school mathematics textbooks in China. Gamification was defined by Deterding et al. as “the use of game design elements in non-game contexts” [7], and in this study, we defined educational gamification as the use of games and/or game design elements in the educational context, mainly for the purpose of teaching and learning. Researchers have argued that game-based learning is a good way to increase students’ interest in learning mathematics. For example, it can make students more engaged in learning mathematics, construct an interactive learning environment for students, and promote communication skills if it is conducted in groups [8,9,10]. In fact, the ideas of teaching and learning with entertainment, or more broadly, educational gamification, can also be traced back to ancient Chinese ways of teaching and learning, and this also occurs in mathematics teaching and learning. This can be seen from the fact that there are many interesting mathematics questions with daily life contexts in the ancient Chinese mathematics masterpiece, Nine Chapters on the Mathematical Art [11]. In addition, by looking back into the Chinese mathematics curriculum standards and syllabuses in the different periods of the 20th Century, it can also be found that gaining experience in numbers through daily games and homework was one of the aims of the Chinese primary school arithmetic syllabus published in 1923 and the use of mathematics games is a way of teaching and learning mathematics in primary schools [12]. In a sense, this also implies that the use of mathematics games is a sustainable or lasting strategy of mathematics teaching and learning.

Although mathematics educators have realized the importance of mathematics textbooks and the benefits of using mathematics games in mathematics teaching and learning, there have been few studies concerning the presentation of mathematics games in mathematics textbooks. In this study, we aim to investigate how mathematics games are presented in school mathematics textbooks in China. More specifically, by analyzing three series of Chinese junior secondary school mathematics textbooks, one published by People’s Education Press, one by Beijing Normal University Press, and the other published by Shanghai Educational Press, we intend to address the following two research questions.

(1)

How are mathematics games presented in three selected series of Chinese secondary mathematics textbooks?

(2)

What are the similarities and differences in the presentation of mathematics games among the three series of Chinese mathematics textbooks?

2. Literature Review

2.1. Game and Mathematics Game

In the largest Chinese comprehensive dictionary Cihai (7th edition), the word game is defined as follows [13]:

A kind of cultural entertainment. There are games to develop intelligence and games to develop physical strength. The former includes word games, while the latter includes active games (such as hide and seek, carrying relay, etc.) and non-competitive sports (such as recreational ball, etc.). In addition, there are video games and online games.

(p. 5530, translated from Chinese)

There are classic definitions in the studies related to games. It is believed that games are: (1) governed by rules; (2) competition or strife to achieve specified, discrete outcomes or goals; moreover, the participants of the games are human [7].

Mathematics games are a type of games. Oldfield [8] defined mathematical games by specifying the following aspects:

• It is an activity involving:
  EITHER a challenge against a task or one or more opponents.
  Or a common task to be tackled either individually or (more normally) in conjunction with others.
• The activity is governed by a set of rules, and has a clear underlying structure to it.
• The activity normally has a distinct finishing point.

4.

The activity has specific mathematical cognitive objectives.

(p. 41)

According to Bright, Harvey and Wheeler [14], an instructional game should be freely engaged. Mousoulides and Sriraman [15] defined a task or activity as a pedagogical appropriate mathematical game when it meets seven criteria. Most of these seven criteria are similar to those described above, but they also think a mathematical game should be enjoyable and winning a game is a purpose. This means that a mathematics game should give students entertainment and, to some extent, be competitive.

2.2. Mathematics Game and Mathematics Education

Many studies have investigated the implementation of mathematics games in mathematics teaching and learning, and most of them have shown that game-based intervention plays a positive role in students’ mathematics learning. Nevertheless, in those studies, mathematics games in a game-based learning environment often refer to digital mathematics games, video games and so on, and few studies have looked into the effect of non-digital mathematics games on mathematics teaching and learning.

Some meta-analyses have been conducted to analyze the effect of mathematics games on students’ mathematics learning. For example, Ran et al. conducted a meta-analysis on the effects of computer technology intervention on low-performing students’ mathematics achievement in K−12 classrooms, which included 45 independent effect sizes extracted from 34 empirical studies, where there were 2044 students in total [16]. In the meta-analysis, the authors categorized computer technology into four types, and the results showed that computer technology had, overall, a statistically positive effect, and game-based intervention had the third largest effect among the four types [16]. Tokac et al. explored the effects of learning mathematics video games on K−12 students’ mathematics achievement by conducting a meta-analysis and found that it has a slightly positive effect on students’ learning gains [17].

Some studies directly examined the effect of mathematics games on mathematics teaching and learning. Denham conducted a case study to explore the effect of the Play Curricular-activity Reflection and Discussion (PCaRD) model of instruction with digital games on mathematics teaching and learning in the middle school classroom via semi-structured interviews with three mathematics teachers, and the results showed that this model was a sound digital game-based learning model, but teachers had difficulty in understanding its usefulness and in implementing it [18]. Gök and İnan didactically described and analyzed 16 sixth-grade students’ experience process of digital game-based learning in Turkey. In the study, the game Race with Numbers was a variation of the game Race to 20 and was designed as a warm-up activity in a mathematics classroom for students to learn mathematics knowledge according to the Theory of Didactical Situations. The study reported that the Theory of Didactical Situations had significant potential in designing mathematics games for mathematics teaching and learning and that mathematics games can help students understand what they had learned and encourage them to use process skills such as problem solving [19]. Ferro et al. investigated the effect of using Gea 2: A New Earth, a digital “serious game” (SG) designed for both entertainment and learning purposes, by simulating real-world scenarios in science, technology, engineering and mathematics (STEM) education. The researchers used the SG as a complementary activity to traditional classroom activities for high school teachers and students, where approximately 100 participants were involved, and concluded that the game was attractive and enjoyable for students and could promote students’ engagement in lessons [20].

In addition, some studies have explored the process of teaching and learning with mathematics games. For example, Cheng et al. examined the characteristics of students’ interactional transition that would potentially lead to better learning during the process of their co-constructing knowledge in an augmented reality-based mathematics game, and the results show that task types, modalities, participant characteristics and learning goals all played a crucial role in determining how learners co-constructed knowledge [21]. Anis et al. used the so-called nominal group technique approach to develop and validate a framework for designing the components and elements of non-digital games based on problem-solving skills for preschool early mathematics on the basis of experts’ views and agreement [22].

As mentioned above, most of the earlier studies addressed the effect of digital mathematics games on mathematics teaching and learning. Due to the importance of mathematics games, it is worth looking further into the presentation of mathematics games in mathematics textbooks and the effect of mathematics games on mathematics teaching and learning.

Only two Chinese Master’s theses have investigated the distribution and features of the presentation of mathematics games in the Chinese primary mathematics textbooks published by Peoples’ Education Press (PEP). Both analyzed the distribution of mathematics games in different mathematics domains and in terms of the types of mathematics games. It should be noted that while Zhang [23] identified 232 mathematics games and classified the mathematics games into competitive games, role-playing games, chess and card playing games, hands-on operation games and electronic games, Zhang [24] identified 534 mathematics games in the textbooks; he presented two ways of classification, one in terms of competition, intelligence, guessing, operation, role and language, and the other in terms of how they are embedded (containing warm-up, exploration, exercise) and completed in mathematics lessons. It can be found that the total number of mathematics games identified by the two authors are quite different, indicating that, to some extent, the definition of mathematics games has not been well-established.

There are two Chinese Master’s theses comparing the presentation of mathematics content, as well as its sequence, locations and other aspects, in Chinese PEP primary mathematics textbooks with those in Singapore Marshall Cavendish mathematics textbooks [25] and those in the Korean national approved mathematics textbooks [26], respectively. They mentioned that all the three series of the mathematics textbooks introduced mathematics games under a special rubric. In addition, some Chinese mathematics educators introduced the features of games in some foreign mathematics textbooks for primary and secondary schools in some professional journals [27,28,29], but the articles are discussion papers rather than research papers.

To summarize, while there are a considerable number of studies exploring the impacts of employing mathematics games, particularly digital games, on mathematics teaching and learning and the results are generally positive, there have been few studies investigating how mathematics games are presented in mathematics textbooks, particularly at the secondary level. As textbooks play an important role in mathematics teaching and learning, this is also the main reason that we conducted this study.

3. Materials and Methods

This section will introduce the selection of the textbooks (i.e., the materials of the study), the definition of mathematics games, the analysis framework and the coding procedure used in this study.

3.1. Textbook Selection

In the Chinese mainland, compulsory education consists of nine years of primary and junior secondary education. The latest national mathematics curriculum standards for compulsory education was published by the Ministry of Education in China in 2022, and the new editions of mathematics textbooks published by different presses are being developed following the latest mathematics curriculum standards and will not be in use until the autumn semester of 2024.

Currently, there are two mathematics curriculum standards for compulsory education in effect in the Chinese mainland, one is the Shanghai Mathematics Curriculum Standards for Primary and Secondary Schools (in short, Shanghai curriculum standards) coming into effect in 2004, and the other is the national Mathematics Curriculum Standards for Compulsory Education (2011 Edition) (in short, national curriculum standards), which came into effect in 2011. The former was approved by the Shanghai Education Commission and implemented in primary and secondary schools in Shanghai, and the latter was approved by the Chinese Ministry of Education and implemented in the Chinese mainland, with the exception of Shanghai. In Shanghai, the primary education stage consists of five years, following by four years of junior secondary education, compared with six years of primary schools and three years of junior secondary nationwide.

There are, in total, 10 series of mathematics textbooks for compulsory education at the secondary stage (Grades 7–9) nationwide in China, except in Shanghai, where there is only one series at the secondary stage (Grades 6–9). As aforementioned, in this study, we selected three series of Chinese mathematics textbooks for junior secondary schools, and to keep consistent in grade levels, we analyzed the textbooks for Grades 7 to 9.

Series 1: The mathematics textbooks published by Shanghai Educational Press (in short, SEP series), following the Shanghai curriculum standards;

Series 2: The mathematics textbooks published by People’s Education Press (in short, PEP series), following the national curriculum standards;

Series 3: The mathematics textbooks published by Beijing Normal University Press (in short, BNUP series), following the national curriculum standards.

The main reason for us selecting those three series is the popularity of the textbooks in terms of use. The first series has been used in almost all the secondary schools in Shanghai, while the second series and the third series have been considered the two most widely used in all the other parts of the Chinese mainland [30].

There are two textbooks for each grade level, one per semester, in each series of mathematics textbooks, except for the SEP series, in which there is a third textbook for Grade 9, which is an enrichment of learning and the mathematics contents covered in this textbook is not required for the Senior High School Entrance Examination. For completeness, we also include this book in our analysis.

3.2. The Definition of Mathematics Games

In this study, by taking into account the definitions of mathematics games, as reviewed above, and the characteristics of mathematics games presented in the Chinese mathematics textbooks, we define “mathematics game” as a task or a learning activity that meets the following criteria in terms of its objective, process, entertainment, challenge, rule and finishing point, as given in

Table 1. The criteria of mathematics games defined in this study.

Criterion	Description
Objective	Having specific mathematical cognitive objectives or learning experience.
Process	Students use mathematical knowledge and/or thinking skills in playing the game.
Entertainment	Enjoyable, motivating, interesting and/or engaging.
Challenge	Involving a challenge against either a task or an opponent(s).
Rule	Governed by a set of rules.
Finishing point	Students need to finish the task or win the game.

.

3.3. Analysis Procedure

The first step for coding is to identify the “analysis unit”, and we regard a lesson as an analysis unit. It should be noted that the homework (or after-class exercise) section in each lesson indicates the end of the lesson. Moreover, there is a chapter summary (and exercise) in the end of each chapter in the three series of textbooks; an “integration and practice” module (section) in the end of each textbook of the BNUP series; “inquiry activity” modules in some chapters of the SEP series; and “project learning” modules, as well as “mathematics activities” modules, in some chapters of the PEP series. As all of them are separated from, or in a sense parallel to, normal lessons, we also regard each of them as a lesson. The number of chapters and lessons in each book is shown in

Table 2. The total numbers of chapters (and lessons) in the three series of textbooks.

	Grade 7		Grade 8		Grade 9		Total
	1st	2nd	1st	2nd	1st	2nd
BNUP	6 (63)	6 (57)	7 (59)	6 (56)	6 (54)	3 (36)	34 (325)
PEP	4 (33)	6 (38)	5 (40)	5 (30)	5 (39)	4 (22)	29 (202)
SEP	3 (40)	4 (49)	4 (55)	4 (56)	3 (43)	2 (23) +2 (26) 1	22 (292)

¹ The figure refers to the third textbook for enrichment in the SEP series as aforementioned.

.

Then, the second step for coding is to establish our analysis framework, which is shown in

Table 3. An analysis framework of mathematics games in textbooks.

Dimension	Description
Location	Worked example, exercise, main text (excluding worked examples and exercises), others
Mathematics domain	Number and algebra, shape and geometry, statistics and probability
Cognitive objective	Concept understanding, skill training, problem solving
Engagement	Direct game, indirect game, background game
Participant	Single player game, double player game, multi-player game
Competitiveness	Competitive game, non-competitive game

. Considering the national curriculum standards and the characteristics of the mathematics games in the three series of mathematics textbooks, as mentioned earlier, we have included six dimensions in the analysis framework, including locations in a lesson, mathematics domains, cognitive objectives, engagement and number of participants and, finally, competitiveness of the games.

In relation to the analysis framework, we first identified the location of each mathematics game, if any, in each lesson according to the characteristics of the locations of the mathematics games presented in the three series of the textbooks, which include worked examples, exercises, main texts (excluding worked examples and exercises) and others (or other locations). It should be noted that the “integration and practice” modules in the BNUP series and “project learning” modules in the PEP series have main texts and exercises, so we also looked at the main texts or exercises in terms of the location, if there are mathematics games presented in these lessons (modules); in contrast, there are only mathematics activities in the “inquiry activity” modules in the SEP series and “mathematics activities” modules in the PEP series, so we coded them as “others” (or other locations) if there are games in these modules.

Secondly, the second and the third dimensions are in line with the mathematics contents and cognitive objectives. The national curriculum standards classified the mathematics domains into “number and algebra”, “shape and geometry”, “statistics and probability”, and “integration and practice” [31]. In fact, all the mathematics games we identified were in the first three domains, so the last domain was excluded in the mathematics domains, for convenience. In terms of the cognitive objectives, they comprise concept understanding, skill training and problem solving, which is a common classification of cognitive objectives of learning mathematic in primary and secondary education stage around the world [32], so we follow it to code this dimension.

Lastly, the last three dimensions focus on the features of the games themselves. Engagement is a key element in participating in a mathematics game in a digital environment, so we classified this dimension into one of three categories: first, direct game–students can directly play the game in learning mathematics; second, indirect game–students watch the participants play the game or experience the game in their minds, and justify whether the game is fair or modify the rules of the game; and third, background game–students use the games as a background or context in learning mathematics. Two examples of games are given in Section 4.5. In terms of the participants, as opponents are commonly regarded as part of the game, we also considered the number of participants in the games as an indicator. In addition, some definitions of the mathematics game regard it as a competition or regard winning a mathematics game as the purpose, particularly in video games and digital games [9,15].

The third step for coding is to actually code the games. In this phase, two of the authors first identified mathematics games in the lessons in the BNUP series, according to the definition mentioned earlier, and coded them independently, then discussed together to reach an agreement. Secondly, the two authors identified mathematics games in the lessons in the PEP series and the SEP series, and then discussed to reach an agreement. Thirdly, the two authors coded the mathematics games in the PEP series and the SEP series and checked the inter-rater reliability by calculating the Kappa values, which were 0.92 for location, 1 for mathematics domain, 0.87 for cognitive objective, 0.85 for engagement, 0.96 for the number of participants and 0.84 for competitiveness. As all the Kappa values range between 0.8 to 1, they all indicate that a high reliability is maintained.

4. Results

In this section, we first report the results regarding the distribution of the games presented in the three series of mathematics textbooks in terms of different grade levels, locations, mathematics domains and cognitive objectives. Then, we present the results concerning the distribution of the games in the three series of textbooks according to the form of engagement, the number of participants and the competitiveness of the games presented.

4.1. An Overall Picture

The distribution of the total number of games presented in the different series of mathematics textbooks is shown in

Table 4. Distribution of games in different grade levels in the three series of textbooks.

	Grade 7	Grade 8	Grade 9	Total
BNUP	51	1	23	75
PEP	7	2	11	20
SEP	5	11	1	17
Total	63	14	35	112

. In total, there are 112 games presented in all three series of mathematics textbooks, and the number of games in the BNUP, PEP and SEP series is 75, 20 and 17, respectively, which means that the BNUP series provided many more games than the other two series of textbooks. In addition, there are slightly more games presented in the PEP series than in the SEP series.

Comparing Grade 7 to Grade 9, it appears that the number of games in Grade 7 is about twice that in Grade 9, and Grade 9 is more than twice that in Grade 8. To our surprise, although in the comparison between the three series of mathematics textbooks and the three grades, the number of games presented in the SEP series and that in all the textbooks of the three series at Grade 8 is the least, the games in Grade 8 are overwhelmingly found in the SEP series, which seems to be very different from the other two series. In contrast, in the BNUP series, the largest number of games was found in Grade 7, followed by Grade 9 and Grade 8, while in the PEP series, the number of games in Grade 9 is the largest, followed by Grade 7 and Grade 8. These results show that there is no consistency in the distribution of games across the three series of mathematics textbooks. Similarly, no consistent pattern is found in the distribution of games across the grade levels within each series of textbooks. We think it appears very clear that there is a lack of consensus in the design and presentation of games in the textbooks, implying more attention and research are needed in this line.

By taking into account the total number of lessons in each series, we can see that, as shown in

Table 5. Average number of games-per-lesson in the different grades and three series of mathematics textbooks.

	Grade 7		Grade 8		Grade 9		Average
	1st	2nd	1st	2nd	1st	2nd
BNUP	0.27	0.60	0	0.02	0.31	0.17	0.23
PEP	0.12	0.08	0.03	0.03	0.28	0	0.10
SEP	0.05	0.06	0	0.20	0	0.02	0.06
Average	0.17	0.28	0.01	0.09	0.21	0.07	NA

, the average number of games-per-lesson in the BNUP series is the highest, followed by the PEP and SEP series. In the BNUP series, the average number of games-per-lesson in the second semester of Grade 7 is the highest, with more than half of the lessons containing games, and that in the first semester of Grade 8 is the lowest, with no lessons containing games. In the PEP series, the average number of games-per-lesson in the first semester of Grade 9 is the highest, with more than one quarter of the lessons containing games, and that in the second semester is the lowest, with no games in any lesson. In the SEP series, the average number of games-per-lesson in the second semester of Grade 8 is the highest, with one-fifth of the lessons containing games, whereas the textbooks for the first semester of both Grades 8 and 9 contain no games.

Taking a wholistic review of all three series, the results show that across the different grades, the average number of games-per-lesson in Grade 7 is the highest (0.23), followed by Grade 9 (0.14) and Grade 8 (0.05). Moreover, the average number of games-per-lesson in the second semester of Grades 7 and 8 is higher than that in the first semester. In contrast, the average number of games-per-lesson in the first semester of Grade 9 is higher than that in the second semester. The reason for this may be related to the mathematical domains covered in the different semesters. In China, the content of mathematics in textbooks is mainly divided into three domains; namely, “number and algebra”, “shape and geometry” and “statistics and probability”. As is well known, compared with the first two mathematics domains, the domain of “statistics and probability” contains contents for more games, such as playing dice, coin toss and turntable games. After further analysis, we found that the topics in the “statistics and probability” domain were all introduced in the second semesters of Grades 7–8 and the first semester of Grade 9 (see more details below).

To summarize, the results show that there is no consistency and regularity in the distribution of games across the three different series of mathematics textbooks and the different grade levels. The BNUP series present a significantly higher total and average number of games-per-lesson than the PEP series, which is slightly higher than the SEP series. Furthermore, Grade 7 contains the largest number of games, as well as the average number of games-per-lesson, followed by Grade 9 and then Grade 8, which has the lowest. It was also noticeable that, overall, the average number of games-per-lesson in the second semester of Grades 7–8 and the first semester of Grade 9 is higher than that in the other semester of the same grade, which is related to the fact that the “statistics and probability” domain is introduced in these three semesters of Grades 7–9 and contains more opportunities for students to learn through mathematics games.

4.2. How Games Are Distributed in Different Locations

Table 6. Distribution of games in different locations.

	Main Texts	Worked Examples	Exercises	Others
BNUP	7	3	65	0
PEP	2	1	6	11
SEP	2	5	5	5
Total	11	9	76	16

shows the distribution of games in the different locations of the three series of mathematics textbooks. It can be seen that most of the games are located in the exercises. In Chinese mathematics textbooks, exercises are generally divided into in-class exercises that students are expected to complete in the class and after-class exercises that students complete independently after the class. Moreover, it was found that the after-class exercises contain more games (45) than the in-class exercises (31), which contain more than the other three places of the textbooks, namely, main texts, worked examples, and the other locations. This result suggests that the textbooks attach greater importance to the integration of games in exercises, whether in class or after class.

A further look at each series of mathematics textbooks reveals that the games in the BNUP series are mainly distributed in exercises; the games in the PEP textbooks are mainly distributed in other locations, followed by the exercises; the distribution in the SEP series is relatively uniform in the worked examples, exercises and other locations.

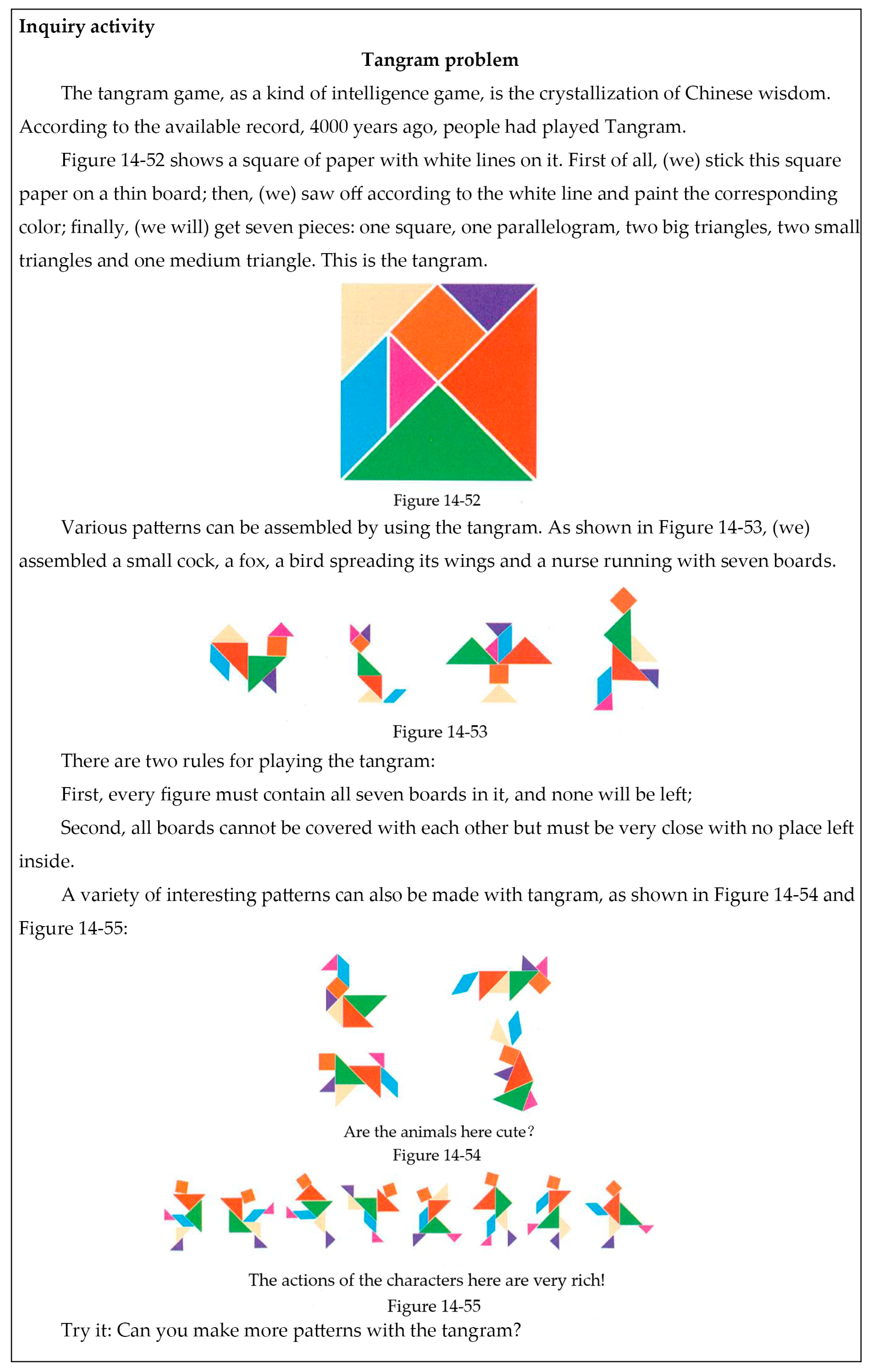
It is worth mentioning that the contents of the other locations, in both the PEP and SEP series, focus on activities, which can explain why there are many games in those locations in the two series. As we know, games are a kind of activity [33], so it is not surprising to see that they are often provided as activities in mathematics textbooks. Figure 1 shows an example of games in the other locations in the SEP series.

Example 1: Inquiry activity—Tangram problem

This is a traditional Chinese mathematical game, called “Tangram” in English (Figure 1). A tangram consists of seven geometric figures (five triangles, one square and one parallelogram). When students play this game, they need to use these seven boards and put them together to form a pattern. Partners can conjecture each other’s action, or they can work together as a team. From the various patterns shown in Figure 1, we can see that this is a challenging “shape and geometry” game, which provides an opportunity for students to give full play to their imagination.

4.3. How Games Are Distributed in Mathematics Domains

Consistent with the overall results, we can see in

Table 7. Distribution of the proportion of games in different mathematical domains and grade levels.

	Grade 7	Grade 8	Grade 9	Average
Number and algebra	34.9% (22) 1	0% (0)	0% (0)	0.060 2
Shape and geometry	33.3% (21)	21.4% (3)	2.9% (1)	0.065
Statistics and probability	31.8% (20)	78.6% (11)	97.1% (34)	0.975

¹ The figure refers to the number of games; ² the figure refers to the average number of games-per-lesson in each mathematics domain.

that the domain of “statistics and probability” has the highest average number of games-per-lesson, followed by “shape and geometry” and, finally, “number and algebra”. Specifically, in the “statistics and probability” domain, the largest number of games were presented in Grade 9. While in the “shape and geometry” domain, the largest were in Grade 7, and in the “number and algebra” domain, they were also in Grade 7.

In the same grade, by comparing the distribution of games in different mathematics domains, we can see that the percentage of games in Grade 7 was almost evenly distributed across the three mathematical domains. However, this is not the case in Grade 8 and Grade 9. The percentage of games in the “statistics and probability” domain in Grade 8 and Grade 9 is 78.6% and 97.1%, respectively, while the proportion of games in the “shape and geometry” domain is very small, and somewhat surprisingly, there is no game in the “number and algebra” domain in these two grade levels.

By further examining the distribution of games in the “number and algebra” domain, we can see that, as shown in

Table 8. Distribution of games in different series of mathematics textbooks, grades, and domains.

		Grade 7	Grade 8	Grade 9	Total
Number and algebra	BNUP	17	0	0	17
	PEP	5	0	0	5
	SEP	0	0	0	0
Shape and geometry	BNUP	15	1	0	16
	PEP	1	2	1	4
	SEP	5	0	0	5
Statistics and probability	BNUP	19	0	23	42
	PEP	1	0	10	11
	SEP	0	11	1	12

, they come from two series of mathematics textbooks, namely the BNUP and PEP series, particularly the former; similarly, the games in the “shape and geometry” and “statistics and probability” domains are mainly from the BNUP series, while in the domain of “probability and statistics”, games are found in all three series. This indicates, to some degree, the close connection between the idea of educational gamification and the learning of probability and statistics, due to the nature of this mathematics domain, as aforementioned.

4.4. How the Games Are Distributed in Cognitive Objectives

Table 9. Distribution of the games in different cognitive objectives in the textbooks.

	Concept Understanding	Skill Training	Problem Solving
BNUP	7 (9.3%)	10 (13.3%)	58 (77.3%)
PEP	2 (10%)	0 (0%)	18 (90%)
SEP	2 (11.8%)	1 (5.9%)	14 (82.4%)
Total	11 (9.8%)	11 (9.8%)	90 (80.4%)

depicts the distribution of the textbook games across the different cognitive objectives, as mentioned above. It can be seen that in all three series, a great majority of the games, approximately 80% in total, were focused on problem solving as the cognitive objective. This is understandable as most games are provided as exercise questions, the main aims of which are to improve students’ ability in problem solving. We further examined the games targeted at the cognitive objective of problem solving in the exercises; the result shows that in all 76 exercise games, 59 (77.6%) were designed to solve mathematics problems.

In addition to problem solving, there are as many games for concept understanding as for skill training. It appears that games are more or less considered equally important for these two cognitive objectives.

Comparing the three series of textbooks, we can see that significantly more problem-solving games were found in the BNUP series than in the other two series. In addition, the distribution of games in these three cognitive objectives is somehow similar between the PEP and SEP mathematics textbooks. However, it was noticeable that there is no game aiming at skill training in the PEP mathematics textbooks. In addition, no clear pattern is found across the three series of the textbooks.

4.5. How Students Are Engaged in the Games

Table 10. Comparison of different participation of games in the three series of mathematics textbooks.

		Direct Game	Indirect Game	Background Game
Number and algebra	BNUP	10	4	3
	PEP	3	1	1
	SEP	0	0	0
Shape and geometry	BNUP	14	0	2
	PEP	4	0	0
	SEP	3	2	0
Statistics and probability	BNUP	21	20	1
	PEP	7	4	0
	SEP	2	10	0

shows the result concerning the distribution of the games in different ways of engagement, as described above, across different mathematics domains in each series of mathematics textbooks.

It can be seen from Table 10 that among the 112 games presented in the three series, 64 are direct games, 41 are indirect games and 7 are background games. Overall, it is clear that different types of games, in terms of the forms of engagement, received more or less attention from all the three series of textbooks, which we think is a positive sign.

To take a closer look at textbooks, below shows two examples, one being a direct game and the other an indirect game.

Example 2: Direct game—“24 points” (students can directly participate in the game)

Figure 2 shows a traditional Chinese mathematical game, “24 points”, presented in the “number and algebra” domain in the BNUP series, and its purpose is to improve students’ ability in arithmetic operations. Students can play the game alone, in pairs or in groups.

Example 3: Indirect game—“Minesweeper” (students can indirectly participate in the game)

Figure 3 is a digital game called “Minesweeper”, presented in the PEP series. Although students cannot play “Minesweeper” in a mathematics class, they can play it in their leisure time. When solving the problems in Figure 3, students’ previous experience in playing this game can help them simulate it in their minds and determine which areas are safer to click.

It should be noted that out of the 112 games in all the three series of mathematics textbooks, there are only two digital games, and both of them are “Minesweeping” games. One is distributed in the first semester of Grade 9 of the PEP textbooks and the other is distributed in the second semester of Grade 7 of the BNUP textbooks. In addition, both are “statistics and probability” games. Given the rapid integration of ICT in mathematics teaching and learning, as reviewed earlier, it appears clear that there is large room for the enhancement of providing digital games in these Chinese mathematics textbooks.

A further examination of the data in Table 10 reveals that a majority of the games presented in the BNUP and PEP series are direct games, 60.0% and 70.0%, respectively, while in the SEP series, the figure is 29.4% and all of the others are indirect games (70.6%). Given that the SEP series presents the least number of games, this result indicates that, compared with the SEP textbooks, students using the other two series of textbooks have considerably more opportunities to directly play games in their learning of mathematics.

Looking into the three mathematics domains, we can also see that in the “number and algebra” and “shape and geometry” domains, most of the games presented in the three series are direct games, while in the “statistics and probability” domain, the numbers of direct games and indirect games are, overall, almost equivalent. Furthermore, the “statistics and probability” domain contains the largest number of direct games, followed by the “shape and geometry” domain, and finally the “number and algebra” domain, which is understandable. In fact, most games presented in the “statistics and probability” domain come from peoples’ daily life, and they were originally intended to solve real life problems, such as deciding which team to play first by flipping a coin. Games that evolved from these daily life activities are relatively easy, not only to use in mathematics classes, but also to design in textbook development.

Similarly, most games found in the “shape and geometry” domain are related to hands-on manipulations, such as jigsaw puzzles, origami and pattern design (see the example in Figure 1). They can be used to enhance students’ interest and to improve their ability in spatial imagination, so both textbooks and teachers would more likely present them to students.

4.6. How Many Players There Are in the Games and How Competitive They Are

Table 11. Distribution of the games in terms of the number of players.

	Single	Double	Multiple
BNUP (n = 75) 1	41 (54.7%)	25 (33.3%)	9 (12%)
PEP (n = 20)	12 (60%)	4 (20%)	4 (20%)
SEP (n = 17)	6 (35.3%)	8 (47.1%)	3 (17.6%)
Total (n = 112)	59 (53.6%)	37 (33.0%)	16 (14.3%)

¹ The figure in each bracket refers to the number of the mathematics games in each series or all the three series.

shows the distribution of the games in terms of the number of players in each series. From the table, we can see that, in total, a great majority of the games presented in the three series are single player games (n = 59), followed by double player games (n = 37) and then multi-player games (n = 16). A closer look at the data reveals that while most (64.7%) of the games presented in the SEP series are double or multiple player games, the figures in the BNUP and PEP are both less than half, i.e., 45.3% and 40%, respectively. In that sense, and considering the importance of cooperative learning in mathematics learning and problem solving, it appears that it merits more attention in the BNUP and PEP series to provide a higher percentage of double and multiple player games.

Finally, let us look at the competitiveness of the games presented in those mathematics textbooks.

Table 12. Distribution of the games in terms of competitiveness.

	Competitive	Non-Competitive
BNUP (n = 75) 1	40 (53.3%)	35 (46.7%)
PEP (n = 20)	6 (30%)	14 (70%)
SEP (n = 17)	12 (70.6%)	5 (29.4%)
Total (n = 112)	58 (51.8%)	54 (48.2)

¹ The figure in each bracket refers to the number of the mathematics games in each series or all the three series.

presents the coding results based on the analysis framework described above. It can be seen that the BNUP series presented nearly an equal number of competitive and non-competitive games, with a ratio of 8:7; the PEP and SEP series presented a rather different distribution and in the opposite directions, i.e., the ratio of the PEP series is 3:7, while in the SEP series, it is almost 7:3. Again, no consistency is found across the three series, indicating that consensus is far from being established.

Furthermore, we found that the single player games presented in the textbooks are mainly non-competitive, while the double player games are mainly competitive, and the multi-player games are equally competitive and non-competitive. The result is understandable as there is no opponent in a single player game, and in many cases, students need to complete the task by themselves. However, to a large extent, a double player game requires competition to decide who is the winner. In addition, multi-player games are evenly distributed between competitive games and non-competitive games.

5. Discussion and Conclusions

This study is concerned with how educational gamification is represented in the school curriculum. More specifically, the study examined how mathematics games are presented in the three series of mathematics textbooks, as potentially implemented curriculum, in China at the secondary level.

The results show that, first, there is largely no consistency and regularity in the distribution of games across the three series of mathematics textbooks and across the grade levels within each series. Specifically, the number of games in the BNUP series is the largest, followed by the PEP series and finally the SEP series. Comparing these three series of mathematics textbooks, the results suggest that the BNUP series pays more attention to games than the other two series. Furthermore, overall, the consistency between the BNUP and PEP series is higher than that between the BNUP and SEP series. According to the results, we can see that there is a lack of consensus regarding the presentation of games in the textbooks, which appears to be more experienced-based rather than research-based; therefore, we think that more research about how games should be presented in mathematics textbooks is worth conducting in order to establish a consensus or common ground in this area.

Second, it should be noted that, in China, the BNUP and PEP series are developed based on the national mathematics curriculum standards, while the SEP series is based on the Shanghai mathematics curriculum standards. This can, to some extent, explain why the SEP series is different from the other two series of mathematics textbooks. From 2022, China has begun to implement the unified national mathematics curriculum standards and the new textbooks will be in use in 2024. It would be interesting to see how the new national curriculum would impact textbook development in terms of the presentation of games.

Third, it is found that the presentation of games is largely content-related. The results reveal that most games are provided in Grade 7. The main reason is that Grade 7 has more lessons. Furthermore, the average number of games-per-lesson in the second semester of Grades 7–8 and the first semester of Grade 9 is higher than that in the other semester of the same grade. This is because much of the mathematical content in these three semesters are closely related to games, such as the “statistics and probability” domain. Combined with the other two mathematics domains, it appears that, overall, the distribution of games mainly depends on where the “statistics and probability” domain is covered.

Earlier research has shown that “statistics and probability” contents in Vietnamese and German high school mathematics textbooks also include mathematics games (e.g., playing dice) to present concepts or rules [37]. These results are in a sense expected, as probability was originally invented to solve fairness problems, and most of them are competitive. This means that many problems in probability have the characteristics of games, so it is reasonable that more games are presented in the domain of “statistics and probability” compared with other mathematics domains.

Fourth, considering the different locations of mathematics textbooks, the results show that most games are presented as exercise questions. This is understandable as contextualized questions may promote students’ interest and, hence, help students learn mathematics. In fact, in the PISA framework, context is one of the dimensions of the mathematics tasks, which is further divided into four categories: personal, occupational, social and scientific, and the game is one of the elements of the personal contexts [38].

Fifth, in terms of cognitive objectives, the study reveals that the main purpose of the games in the mathematics textbooks is to solve problems, which is not only consistent with the features of educational games [39], but also echoes the previous conclusion. As mentioned earlier, most (67.9%) of the games are exercises, and among the exercises, most games (77.6%) are aimed at problem solving. In this connection, we think further study should focus on how games-related questions, particularly those presented as exercises questions, have an impact on students’ problem solving ability.

Furthermore, concerning the engagement of games, the results show that a great majority of games are designed for direct or indirect participation, which indicates that the textbooks attach greater importance to the engagement of games than to the use of them only as background or context in students’ learning of mathematics. In addition, it was noticeable that there are only two digital games, both “Minesweeping” games, in these three series of mathematics textbooks. On the other hand, as mentioned in the literature review, most educational games in the current research are digital games, e.g., computer games [40]. Given the fast development of the integration of ICT into mathematics teaching and learning, we think more attention should be paid to the integration of more digital games into mathematics textbooks in China in the future.

Finally, regarding the number of participants in the games, the study found that most of the games in the mathematics textbooks are single player games, followed by double player games and finally multi-player games. In terms of competitiveness, it is found that, in the three series of Chinese mathematics textbooks, there are slightly more competitive games than non-competitive games; moreover, the single player games presented are mainly non-competitive, whilst the double player games presented are mainly competitive, and the number of competitive and non-competitive games is nearly equal in multi-player games. Researchers have argued that competition is an important element in games [41], and an issue that remains to be further studied is what is a reasonable distribution of different types of games in mathematics textbooks.

To end this paper, we should remind the readers that the three series of mathematics textbooks in our study are not randomly selected, and the results we obtained from those three series cannot be generalized without further research evidence to other mathematics textbook series in China, let alone in other countries. In the future, it is also worth comparing the presentation of mathematics games in secondary mathematics textbooks in different countries to explore possible ways of enhancing the provision of games in mathematics textbooks. Moreover, as our study focused on mathematics textbooks at the secondary level, we think it would be equally, if not more, interesting to see how educational gamification is represented in primary school mathematics textbooks, given the importance of developing young children’s interest in mathematics learning at the early education stage.