An Experimental and Theoretical Study on the Tailings Dam with Geotextile Bags
Abstract
1. Introduction
2. Experiments
2.1. The Slope Slip Test of the Geotextile Bag
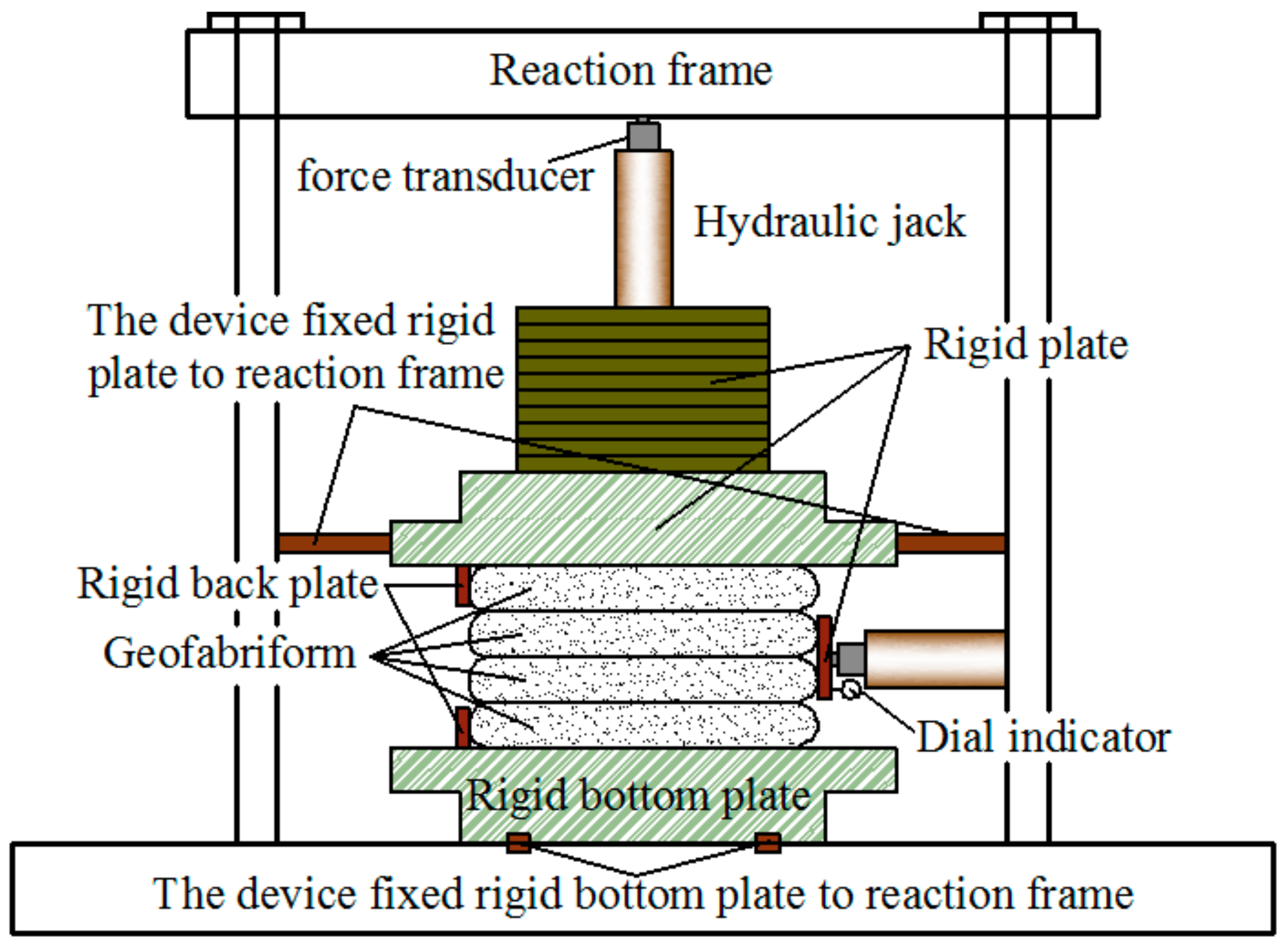
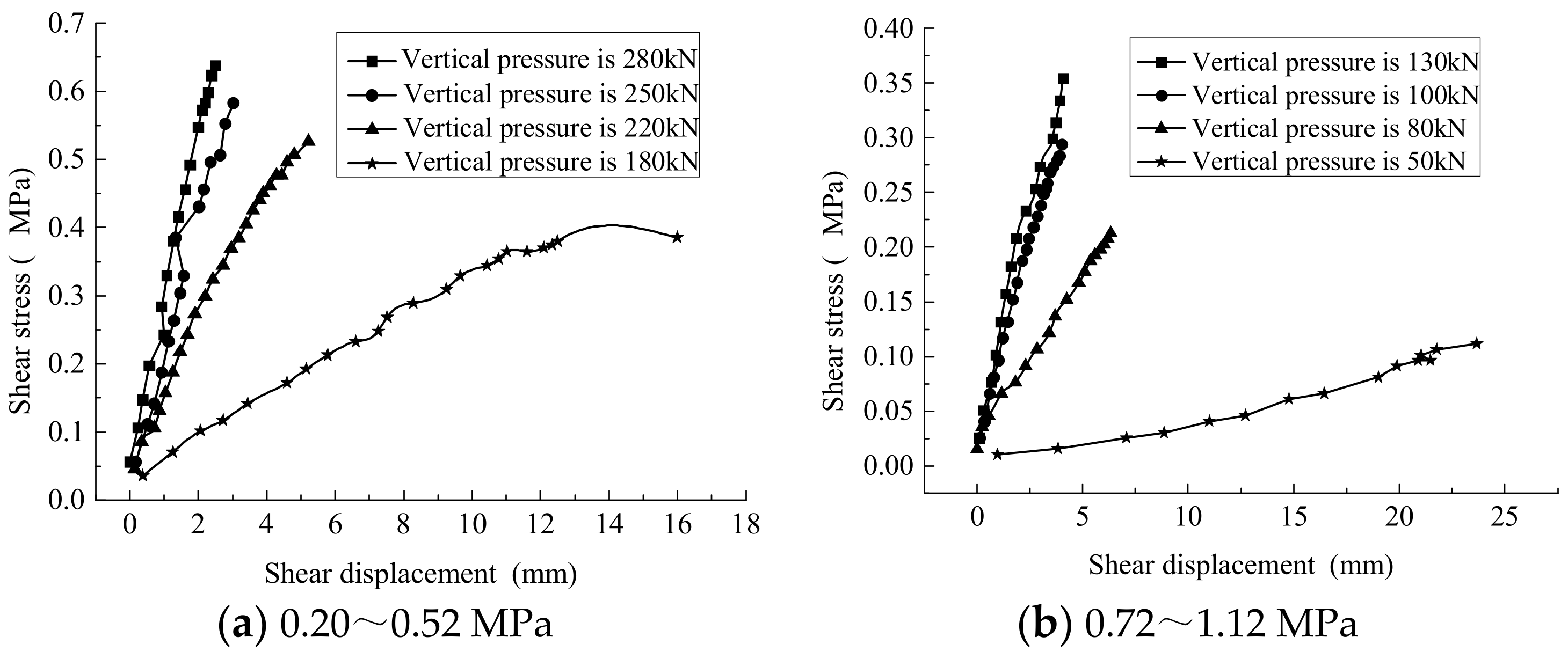
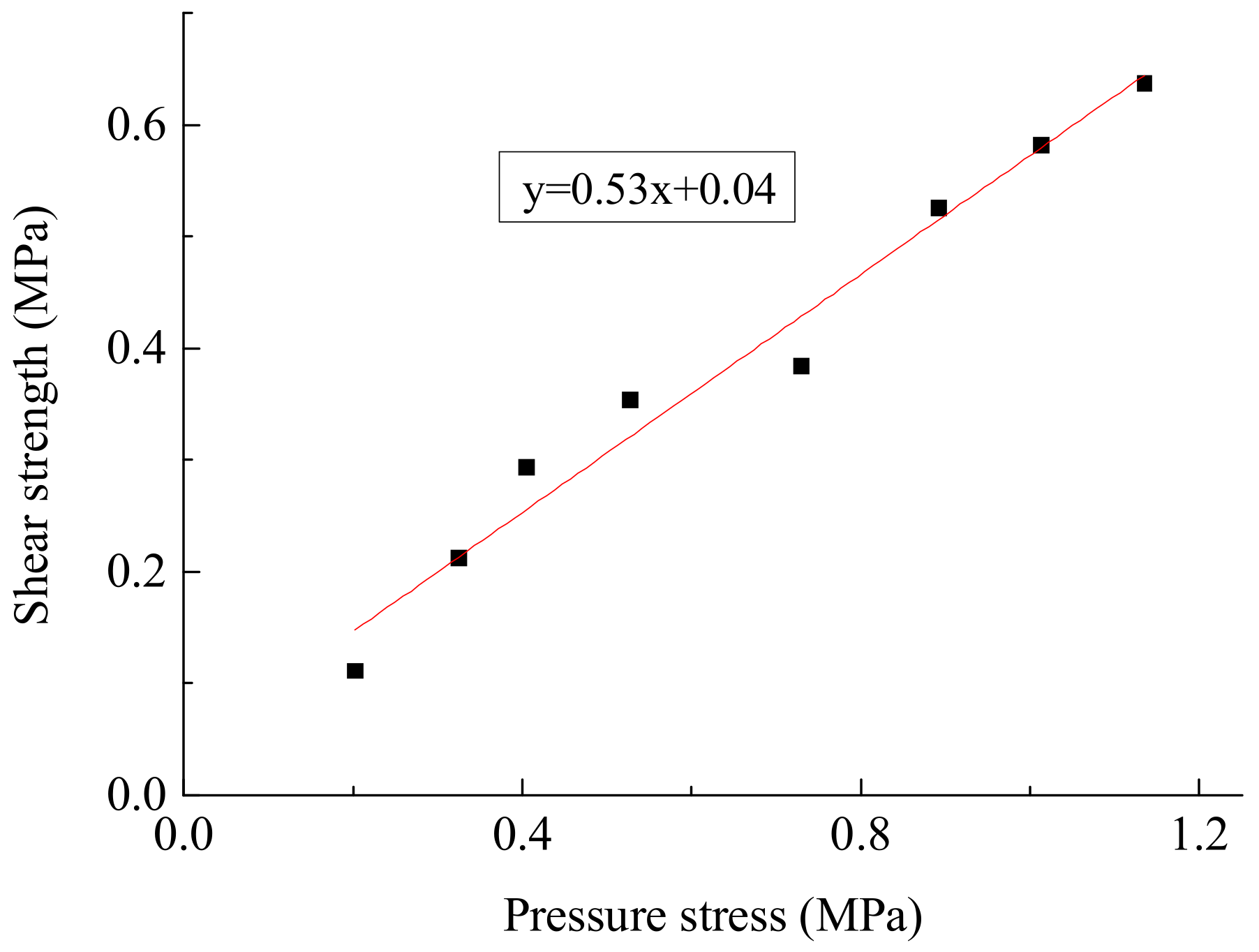
2.2. The Shear Tests of the Geotextile Bags
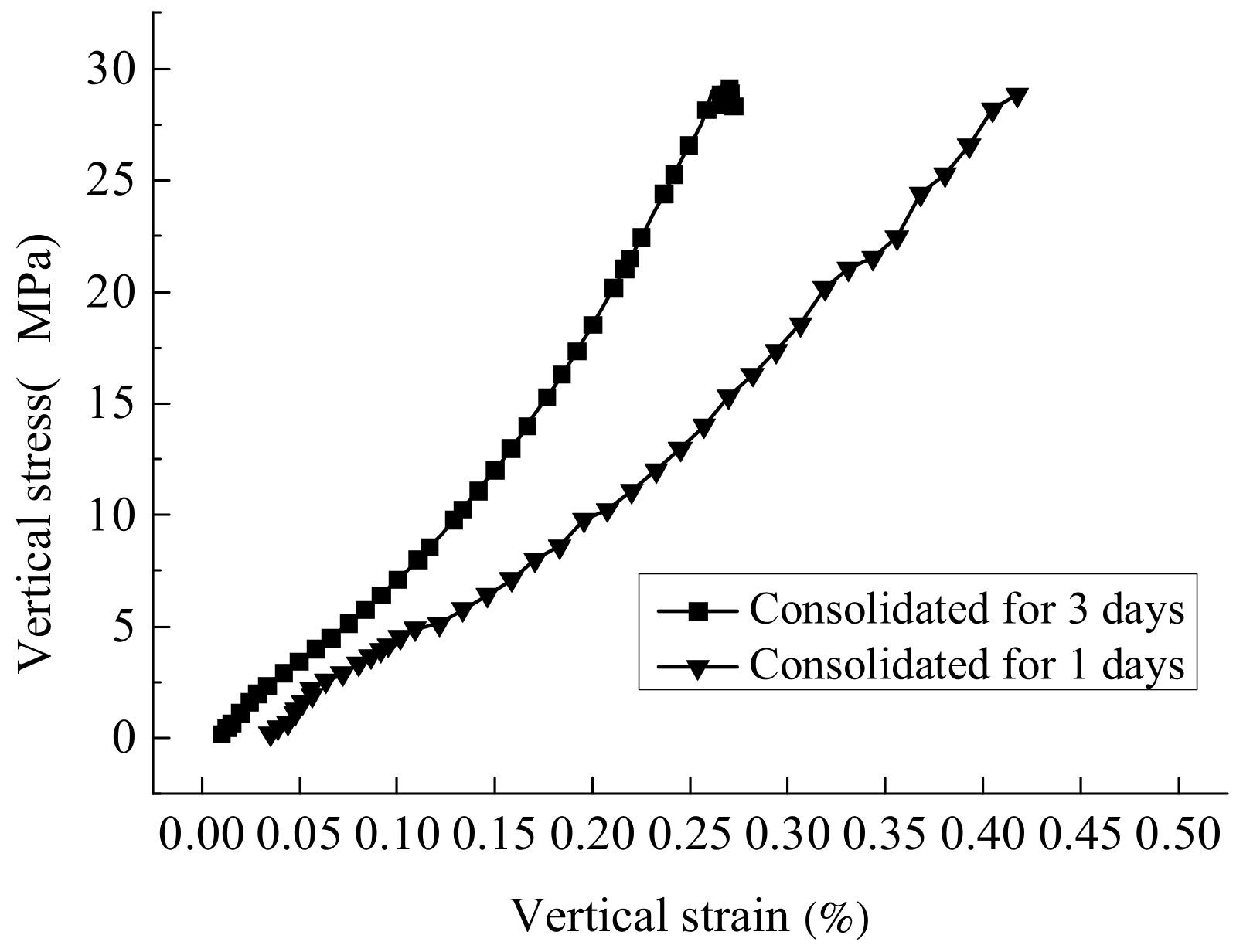
2.3. The Compression Tests of Geotextile Bags
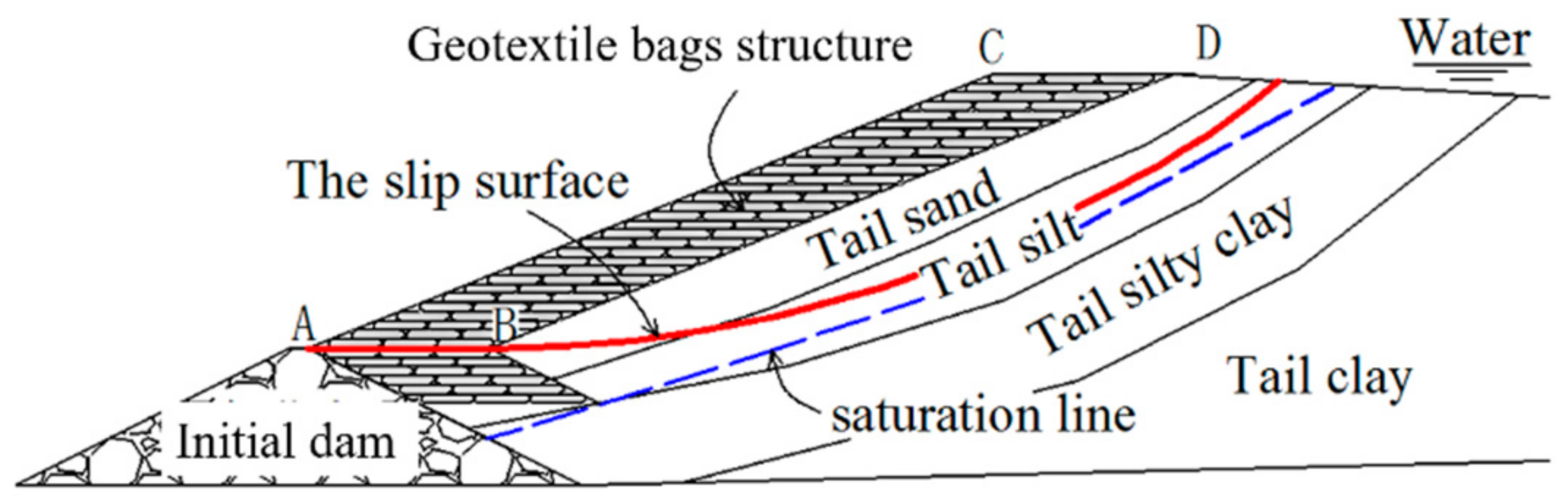
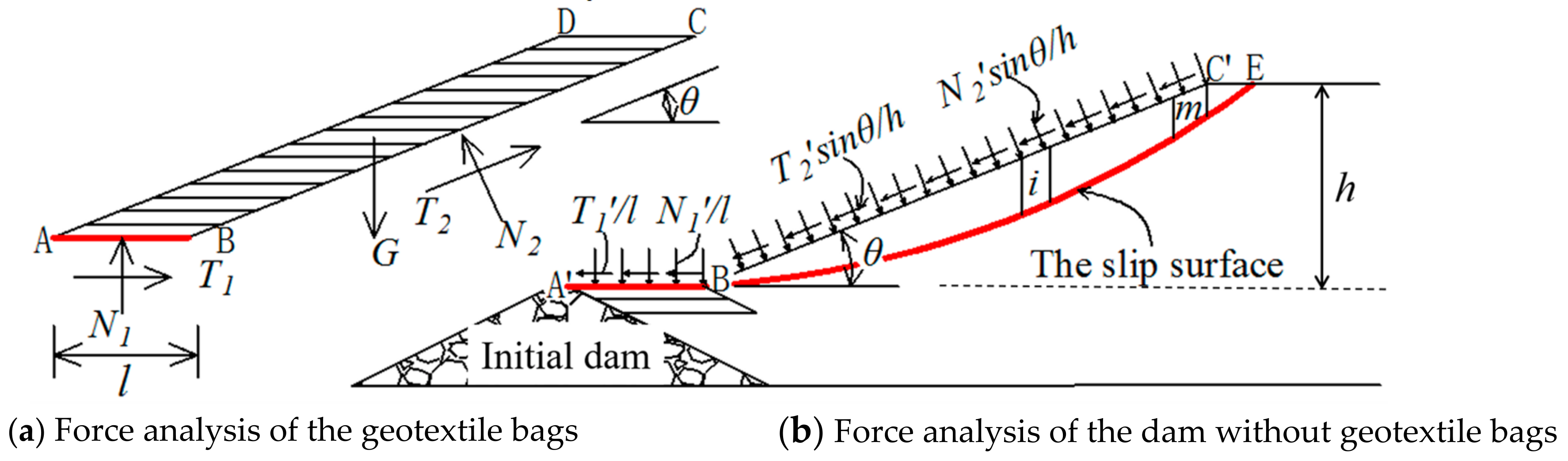
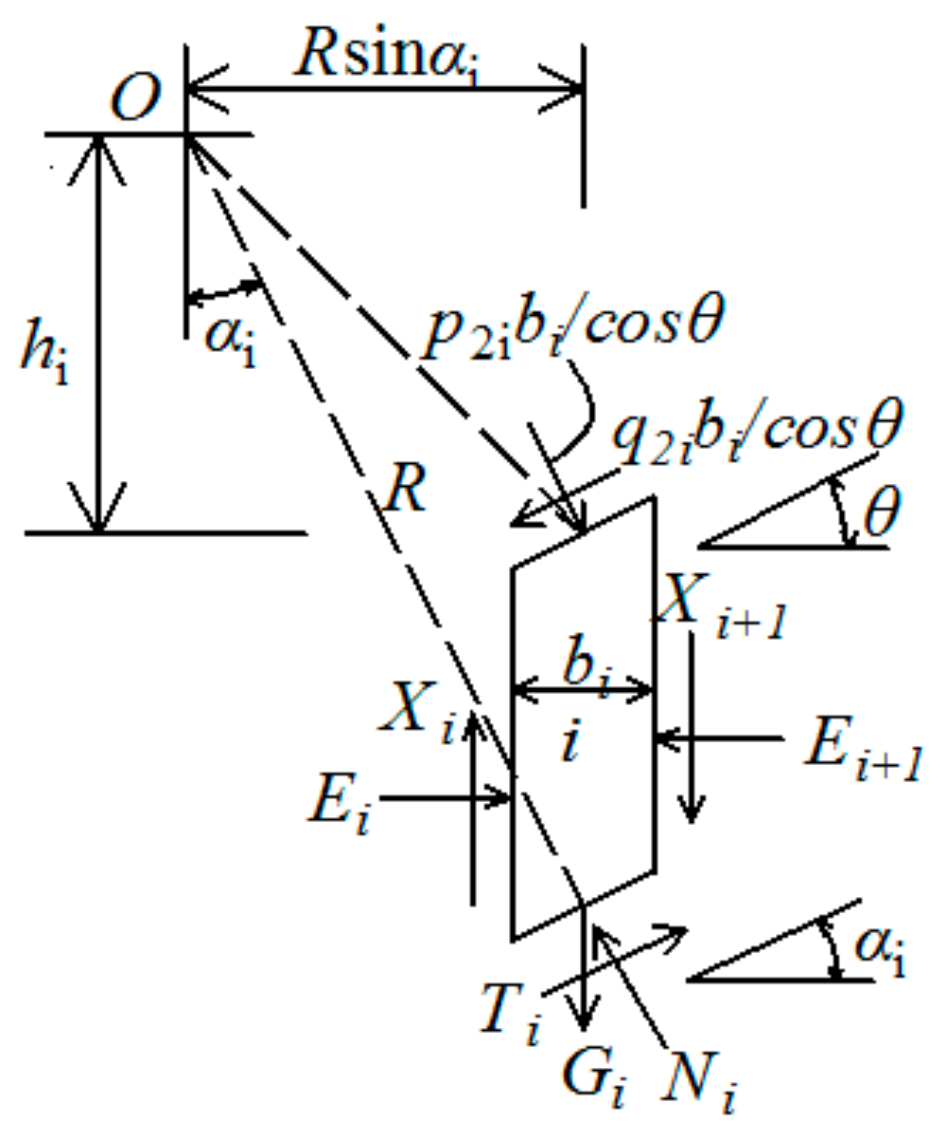
3. The Stability Calculation of the Tailings Dam Using Geotextile Bags (TDGB)
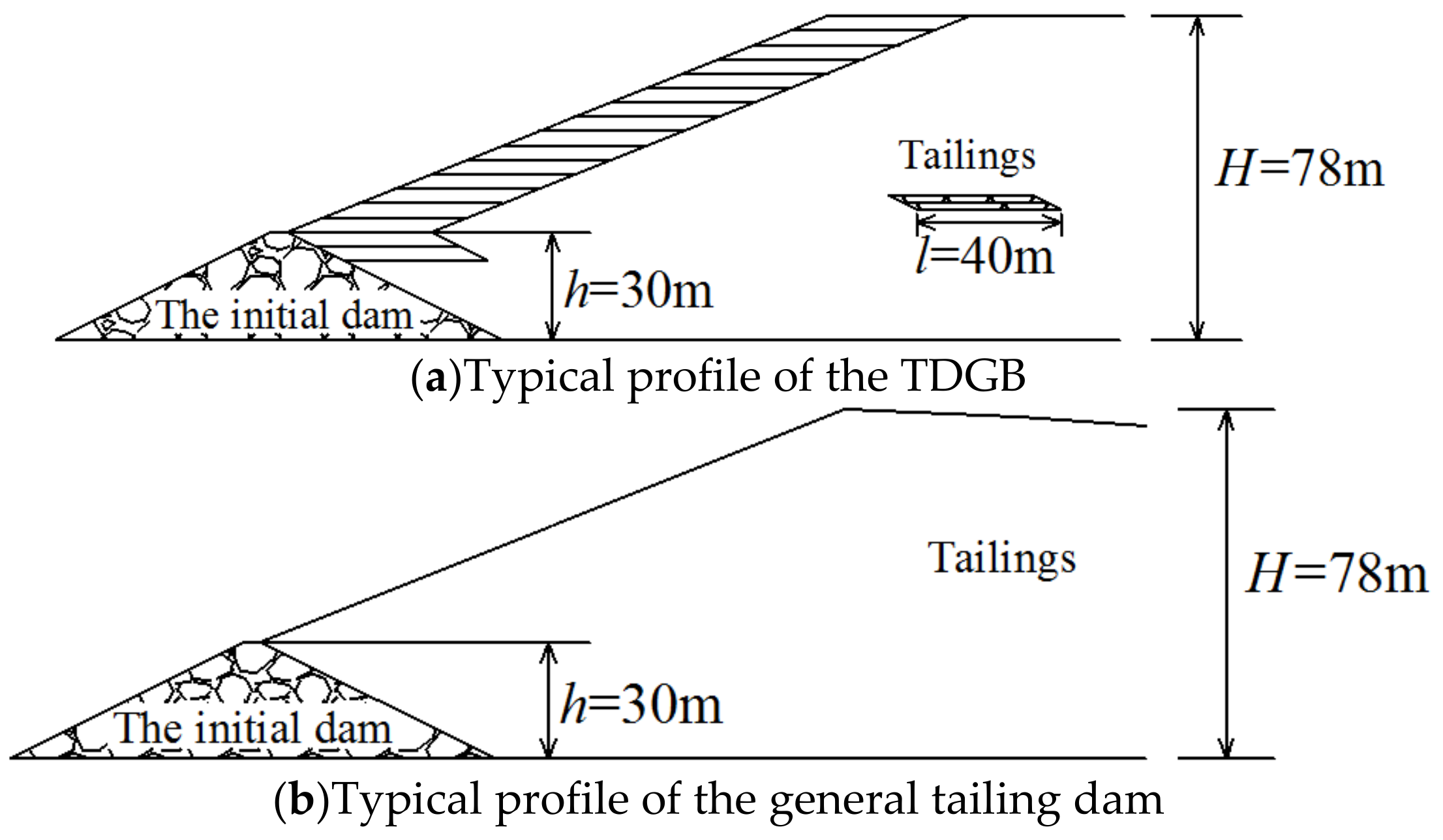
4. Some Calculation Examples
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Glotov, V.E.; Chlachula, J.; Glotova, L.P.; Little, E. Causes and environmental impact of the gold-tailings dam failure at Karamken, the Russian Far East. Eng. Geol. 2018, 245, 236–247. [Google Scholar] [CrossRef]
- USGS. United States Geological Survey (Mineral Commodity Summaries): Phosphate Rock; US Government Printing Office: Washington, DC, USA, 2020. [Google Scholar]
- China Mineral Resources; Ministry of Natural Resources PRC: Beijing, China, 2020.
- Kossoff, D.; Dubbin, W.E.; Alfredsson, M.; Edwards, S.J.; Macklin, M.G.; Hudson-Edwards, K.A. Mine tailings dams: Characteristics, failure, environmental impacts, and remediation. Appl. Geochem. 2014, 51, 229–245. [Google Scholar] [CrossRef]
- Dong, T.; Cao, P.; Gui, R.; Lin, Q.B.; Liu, Z.Z. Experimental study on permeability coefficient in layered fine tailings under seepage condition. Geofluids 2021, 2021, 8850138. [Google Scholar] [CrossRef]
- Yin, G.Z.; Li, G.Z.; Wei, Z.A.; Wan, L.; Shui, G.H.; Jing, X.F. Stability analysis of a copper tailings dam via laboratory model tests: A Chinese case study. Miner. Eng. 2011, 24, 122–130. [Google Scholar] [CrossRef]
- Zhang, C.; Li, B.; Wu, F.H.; Yu, Y.; Zhang, Y.; Xu, H. Research on modification of mechanical properties of recycled aggregate concrete by replacing sand with graphite tailings. Rev. Adv. Mater. Sci. 2022, 61, 493–512. [Google Scholar] [CrossRef]
- Hegde, A.; Das, T. Finite element-based probabilistic stability analysis of rock-fill tailing dam considering regional seismicity. Innov. Infrastruct. Solut. 2019, 4, 37. [Google Scholar] [CrossRef]
- Yin, G.; Wei, Z.; Wang, J.G.; Wan, L.; Shen, L. Interaction characteristics of geosynthetics with fine tailings in pullout test. Geosynth. Int. 2008, 15, 428–436. [Google Scholar] [CrossRef]
- Azam, S.; Li, Q.R. Tailings dam failures: A review of the last one hundred years. Geotech. News 2010, 28, 50–53. [Google Scholar]
- Yang, Y.H.; Wei, Z.A.; Cao, G.S.; Yang, Y.; Wang, H.; Zhuang, S.N. A case study on utilizing geotextile tubes for tailings dams construction in China. Geotext. Geomembr. 2019, 47, 187–192. [Google Scholar] [CrossRef]
- Jiang, F.L.; Wu, H.N.; Liu, Y.; Chen, G.; Guo, J.J.; Wang, Z. Comprehensive evaluation system for stability of multiple dams in a uranium tailings reservoir: Based on the TOPSIS model and bow tie model. R. Soc. Open Sci. 2020, 7, 191566. [Google Scholar] [CrossRef]
- Du, Y.Q.; Xie, B.; Mullarney, B.; Zhang, C. Deposition of fine tailing particles and profile zoning of tailings dams. Soil Mech. Found. Eng. 2019, 56, 359–365. [Google Scholar] [CrossRef]
- Wang, W.S.; Yin, G.Z.; Wei, Z.A.; Jing, X.F.; Chen, Y.L. Analysis of the dynamic response and stability of fine-grained tailings dam by upstream embankment method in the area of high intensity earthquake. Chin. J. Rock Mech. Eng. 2017, 36, 1201–1214. [Google Scholar]
- Wu, S.C.; Zhao, Z.Q.; Zhang, X.Q.; Cheng, H.Y.; He, P.B.; He, F.Y. Stability analysis and reinforcement design of tailings dam expansion engineering. J. Kunming Univ. Sci. Technol. (Nat. Sci.) 2021, 46, 36–44. [Google Scholar]
- Yang, C.H.; Zhang, C.; Li, Q.M.; Yu, Y.Z.; Ma, C.K.; Duan, Z.J. Disaster mechanism and prevention methods of large-scale high tailings dam. Rock Soil Mech. 2021, 42, 1–17. [Google Scholar]
- Li, Q.Y.; Ma, G.W.; Li, P.; Su, Z.D. Contrastive analysis of dynamic response of tailings dam with and without geofabriform by shaking table model test. E3S Web Conf. 2020, 198, 01041. [Google Scholar] [CrossRef]
- Ye, E.J.; Duo, L.D.; Zhang, F.; Wang, X.Y. Application of geofabriform method damming technology in a tailings dam. Nonferrous Met. (Min. Sect.) 2020, 72, 47–50. [Google Scholar]
- Zhang, W.X.; Wang, M.L. Stability analysis based on geofabriform method and raising and expanding volume of a tailings dam. China Tungsten Ind. 2020, 35, 13–17. [Google Scholar]
- Xue, Z.Y. Feasibility analysis of Xipiao tailings dam with wide top constructed by mold bag method. Mod. Min. 2021, 37, 95–97, 101. [Google Scholar]
- Wang, P. Geofabriform Method; Water Resources and Hydropower Engineering Press: Beijing, China, 2006; pp. 20–21. [Google Scholar]
- Chu, J.; Yan, S.W.; Li, W. Innovative methods for dike construction: An overview. Geotext. Geomembr. 2012, 30, 35–42. [Google Scholar] [CrossRef]
- Yan, S.W.; Chu, J. Construction of an offshore dike using slurry filled geotextile mats. Geotext. Geomembr. 2010, 22, 422–433. [Google Scholar] [CrossRef]
- Oberhagemann, K.; Hossain, M.M. Geotextile bag revetments for large rivers in Bangladesh. Geotext. Geomembr. 2011, 29, 402–414. [Google Scholar] [CrossRef]
- Matsushima, K.; Mohri, Y.; Yamazaki, S.; Hori, T.; Ariyoshi, M.; Tatsuoka, F. Design of earth dams allowing temporary overtopping based on Hydraulic failure experiments and flood analysis. In Proceedings of the 4th Asian Regional Conference on Geosynthetics, Shanghai, China, 18 June 2008. [Google Scholar]
- Xu, Y.F.; Huang, J.; Du, Y.J. Earth reinforcement using soilbags. Geotext. Geomembr. 2008, 26, 279–289. [Google Scholar] [CrossRef]
- Liu, S.H.; Wang, Y.S. Reinforcement mechanism of soilbags and its applications. Geotech. Eng. Tech. 2007, 21, 221–225. [Google Scholar]
- Yang, J.X.; Hu, J.; Wu, Y.W.; Zhang, B.Y. Numerical Simulation of Seepage and Stability of Tailing Dams: A Case Study in Ledong, China. Sustainability 2022, 14, 12393. [Google Scholar] [CrossRef]
- Bai, F.Q.; Liu, S.H.; Wang, Y.Q. Research on reinforcement mechanism and failure strength of soilbags. Rock Soil Mech. 2010, 31, 172–176. [Google Scholar]
- Qiu, C.L.; Yan, S.W. Mechanical behavior analysis of large scale geofabriform. Eng. Mech. 2009, 26, 92–97. [Google Scholar]
- Wang, M. Study on deformation and mechanical properties of geotextile tube. Master’s Thesis, Zhejiang University, Hangzhou, China, 2013. [Google Scholar]
- Lohani, T.N.; Matsushima, K.; Aqil, U.; Mohri, Y.; Tatsuoka, F. Evaluating the strength and deformation characteristiccs of a soil bag pile from full scale Laboratory tests. Geosynth. Int. 2006, 6, 246–264. [Google Scholar] [CrossRef]
- Liu, S.H.; Matsuoka, H. A new earth reinforcement method by soilbags. Rock Soil Mech. 2007, 28, 1665–1670. [Google Scholar]
- Wang, Y.Q.; Liu, S.H.; Yang, J.J. Model test of sandy soil slope reinforced by soilbags and upper-bound solution. Chin. J. Rock Mech. Eng. 2009, 29, 4006–4013. [Google Scholar]
- Liu, S.H.; Bai, F.Q.; Wang, Y.S.; Wang, S.; Li, Z. Treatment for expansive eoil channel slope with soilbags. J. Aerosp. Eng. 2013, 26, 657–666. [Google Scholar] [CrossRef]
- Matsushima, K.; Aqil, U.; Mohri, Y.; Tatsuoka, F. Shear strength and deformation characteristics of geosynthetic soilbags stacked horizontal and inclined. Geosynth. Int. 2008, 2, 119–135. [Google Scholar] [CrossRef]
- Huang, C.-C.; Matsushima, K.; Mohri, Y.; Tatsuoka, F. Analysis of sand slopes stabilized with facing of soilbags with extended reinforcement strips. Geosynth. Int. 2008, 4, 232–245. [Google Scholar] [CrossRef]
- Chen, Z.Y.; Zhou, J.X.; Wang, H.J. Soil Mechanics; Tsinghua University Press: Beijing, China, 1994. [Google Scholar]






| Name of Dam | Tailings Types | Construction Method | Year of Failure | Fatalities |
|---|---|---|---|---|
| Huogudu, Yunnan Tin Group Co., Yunnan province | Tin | Upstream | 1962 | 171 |
| Niujiaolong, Shizhuyuan Non-ferrous Metals Co., Hunan province | copper | Upstream | 1985 | 49 |
| Longjiaoshan, Daye Iron Ore mine, Hubei province | Iron | Upstream | 1994 | 31 |
| Dachang, Nandan Tin mine, Guangxi province | Tin | Upstream | 2000 | 28 |
| Zhenan Gold mine, Shanxi province | Gold | Upstream | 2006 | 17 |
| Xiangfen tailings pond, Shanxi province | Iron | Upstream | 2008 | 277 |
| Xinyi Zijin Tin mine, Guangdong province | Tin | Upstream | 2010 | 28 |
| Specimen Size (m) | Consolidation Days (Day) | Moisture Content (%) | Critical Angle (°) | Average Critical Angle (°) | Friction Coefficient |
|---|---|---|---|---|---|
| 0.5 × 0.5 × 0.1 | 4 | 17.8 | 25.43 | 24.09 | 0.45 |
| 24.10 | |||||
| 23.19 | |||||
| 23.63 | |||||
| 0.5 × 0.5 × 0.1 | 8 | 16.9 | 28.62 | 28.01 | 0.53 |
| 26.23 | |||||
| 27.89 | |||||
| 29.30 | |||||
| 0.5 × 0.5 × 0.1 | 12 | 15.5 | 30.65 | 30.93 | 0.60 |
| 29.25 | |||||
| 31.64 | |||||
| 32.19 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Q.; Ma, G.; Lu, Y. An Experimental and Theoretical Study on the Tailings Dam with Geotextile Bags. Sustainability 2023, 15, 4768. https://doi.org/10.3390/su15064768
Li Q, Ma G, Lu Y. An Experimental and Theoretical Study on the Tailings Dam with Geotextile Bags. Sustainability. 2023; 15(6):4768. https://doi.org/10.3390/su15064768
Chicago/Turabian StyleLi, Qiaoyan, Guowei Ma, and Yulin Lu. 2023. "An Experimental and Theoretical Study on the Tailings Dam with Geotextile Bags" Sustainability 15, no. 6: 4768. https://doi.org/10.3390/su15064768
APA StyleLi, Q., Ma, G., & Lu, Y. (2023). An Experimental and Theoretical Study on the Tailings Dam with Geotextile Bags. Sustainability, 15(6), 4768. https://doi.org/10.3390/su15064768







