Investigating the Relationship between the Manning Coefficients (n) of a Perforated Subsurface Stormwater Drainage Pipe and the Hydraulic Parameters
Abstract
:1. Introduction
2. Materials and Methods
2.1. The Perforation Characteristics of a Six-Row Regular-Perforated Sand-Slot Pipe Sample
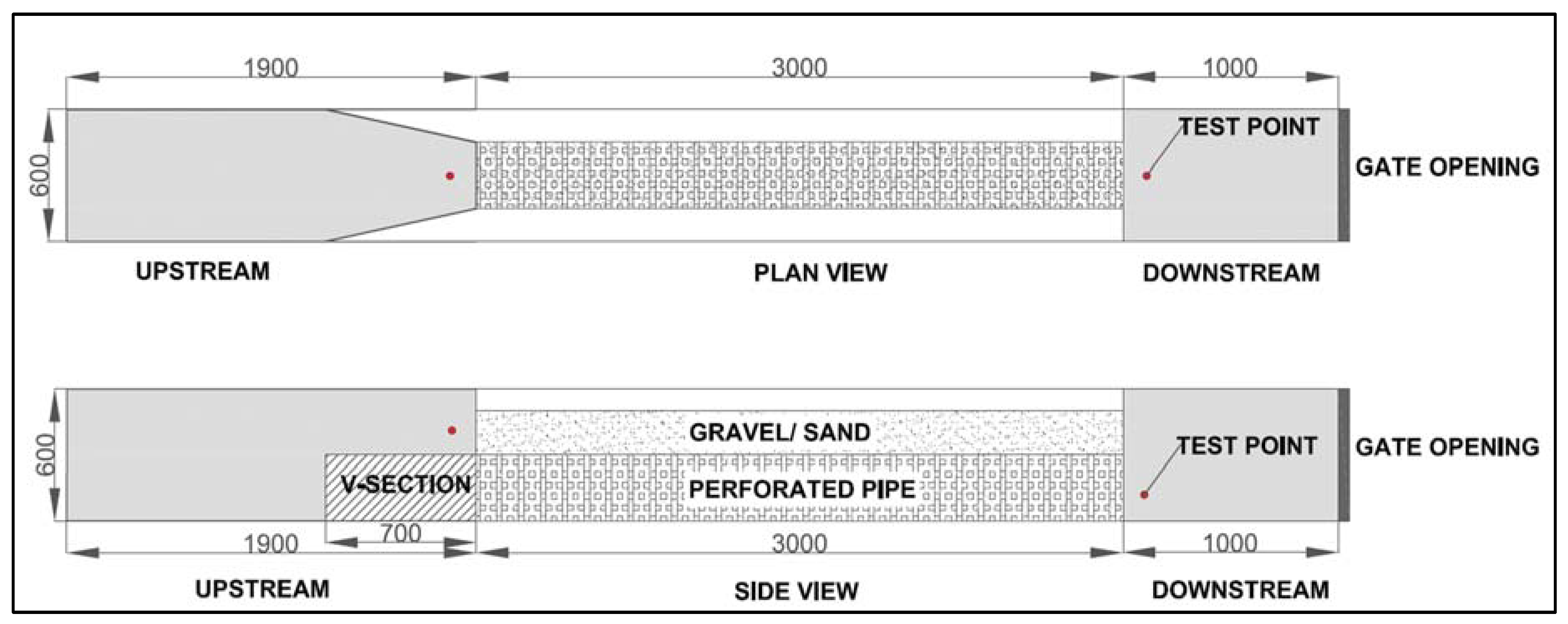
2.2. Experimental Set-Up
2.3. Calculations of Roughness/Manning (n) of the Pipe and Froude Number (Fr) of the Flow
- n: Manning
- v: Velocity of flow (m/s)
- R: Hydraulic Radius
- S: Longitudinal Slope
- Fr: Froude number
- V: Velocity of flow (m/s)
- G: Acceleration of gravity (m2/s)
- Y: Depth of the flow section (m)
| Froude Number, Fr | State of Flow | Description |
|---|---|---|
| Fr = 1 | Critical | Flow celerity equal to flow velocity |
| Fr < 1 | Subcritical | Slow flow—tranquil and streaming |
| Fr > 1 | Supercritical | High velocity—rapid, shooting, and torrential |
3. Results
Effects of Hydraulic Performance of Manning Coefficient (n) versus Flow Velocity, Flow Depth, and Froude Number for Gate Fully Open (GFO) and Gate Partially Open (GPO) Scenarios with Longitudinal Slopes of 1:750 and 1:1000
4. Discussion
5. Conclusions
- (a)
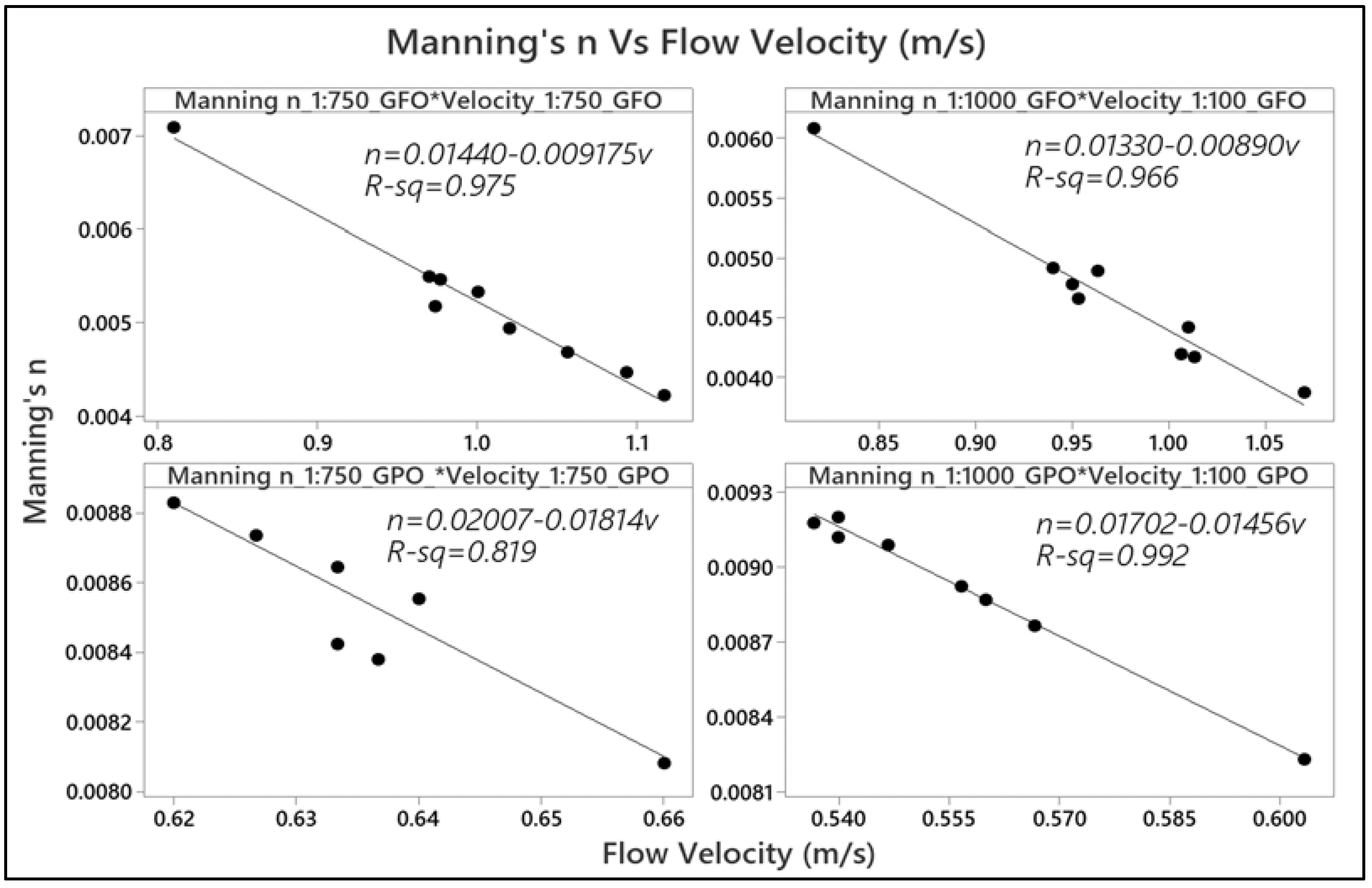
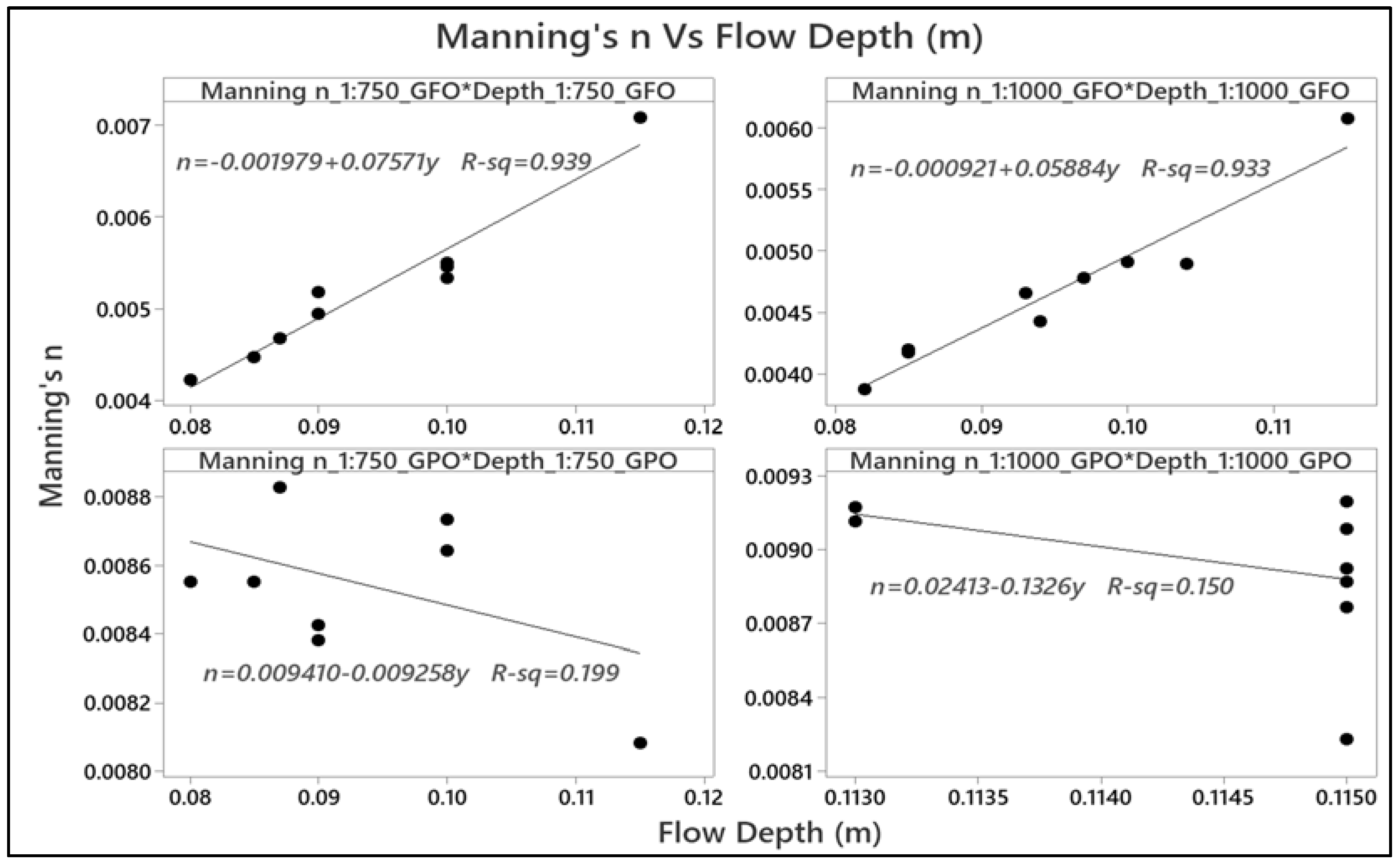
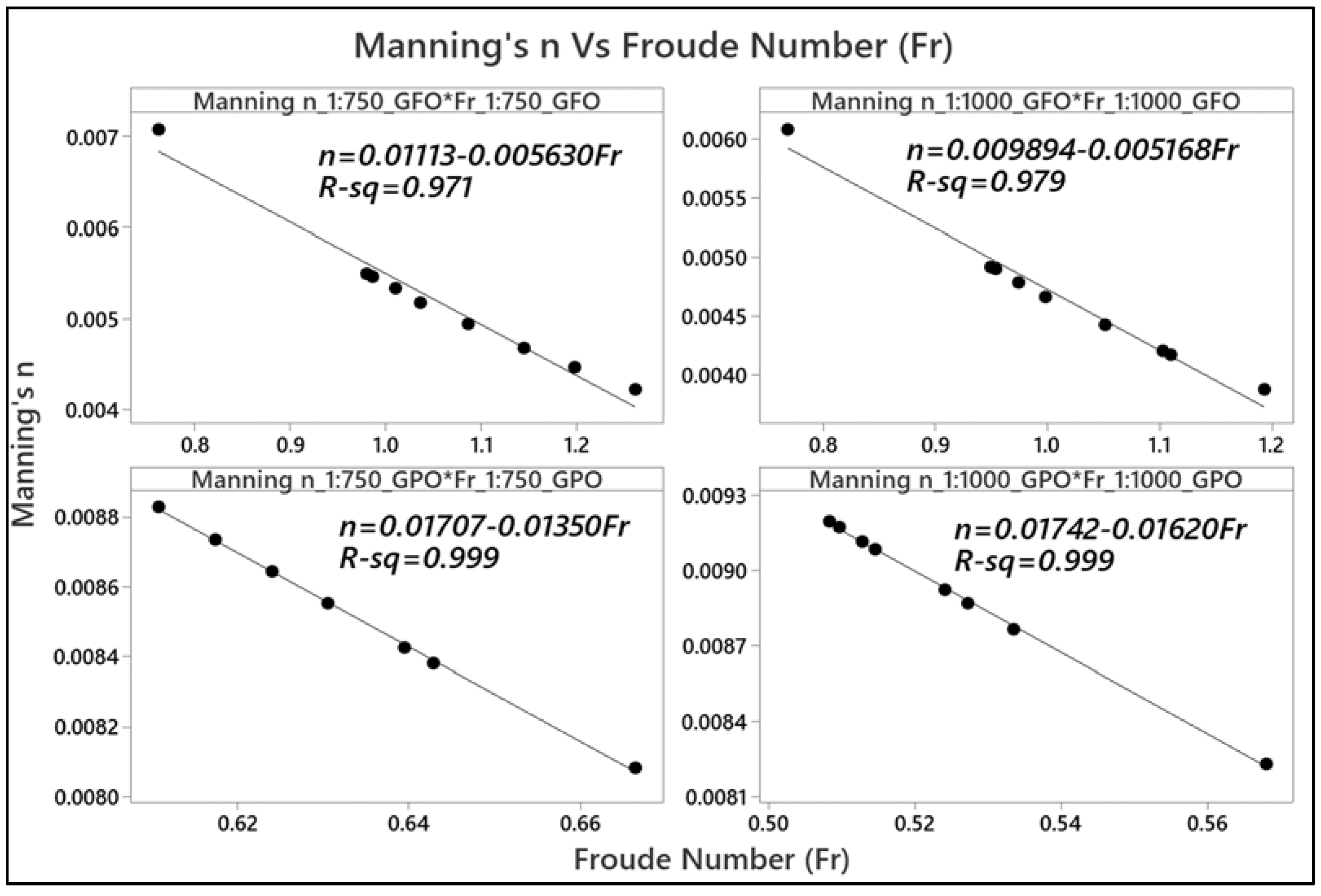
- The results of a flow condition in the above perforated pipe under GFO showed a significant relationship between the Manning’s n and other hydraulic parameters of flow velocity, depth, and Froude number, and the R2 value was close to 1 with both pipe slopes of 1:750 and 1:1000. Since the roughness coefficient (Manning’s n) of the pipe increases, it is able to reduce the flow velocity in the pipe, resulting in a lower peak of flow and the ability to control the quantity of the storm water in the subsurface urban drainages. An inverse linear relationship between the Manning coefficients and the flow velocity was also achieved with the coefficients of determination (R2) of 0.975 and 0.966, which resulted in predicting the values of flow velocities based on the calculated Manning’s n that fell within the range of 0.004 to 0.009 using the equations v = ((0.01440 − n)/0.009175) and v = ((0.01330 − n)/0.00890), with the various pipe slopes of 1:750 and 1:1000, respectively. However, as the Manning’s n increased, the depth of flow in the perforated pipe also increased linearly, and the equations y = ((n + 0.001979)/0.07571) and y = ((n + 0.000921)/0.05884) were attained when the ranges of Manning’s n were within 0.004 to 0.009 for the pipe with slopes of 1:750 and 1:1000, respectively. On top of that, the calculated Froude numbers showed an inverse linear relationship with the calculated Manning’s n of the pipe, and the equations Fr = ((0.01113 − n)/0.005630) and Fr = ((0.009894 − n)/0.005168) can be found with the Manning’s n ranging between 0.004 and 0.009 with pipe slopes of 1:750 and 1:1000, respectively.
- (b)
- In addition, in a flow condition in the perforated pipe under GPO, the R2 of 0.819 and 0.992 were achieved because of the converse linear relationship between the Manning coefficients and the flow velocity, which resulted in predicting the values of flow velocities based on the calculated Manning’s n that fell within the range of 0.004 to 0.009 using the equations v = ((0.02007 − n)/0.01814) and v = ((0.01702 − n)/0.01456), with the various pipe slopes of 1:750 and 1:1000, respectively. However, it was perceived that increasing and decreasing the Manning coefficients did not significantly affect the depths of flow in the pipe with both pipe slopes. Even so, an inverse linear relationship was obtained between the Froude number and the Manning’s n, yielding the equations Fr = ((0.01707 − n)/0.01350) and Fr = ((0.01742 − n)/0.01620) with R2 approximately close to 1.00 to find the Froude numbers with the Manning’s n ranging between 0.004 and 0.009, respectively. Thus, from the findings of this work, it was concluded that by using a perforated pipe with a high Manning coefficient (n) along the pipe under both GFO and GPO conditions with two slopes of 1:750 and 1:1000, the velocity of runoff inflow will be decreased, thereby reducing the peak runoff volume and flash flooding. Therefore, the subsurface perforated pipe under the GFO and GPO conditions can be recommended to be used for the best management practices (BMP) in sustainable stormwater management.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Barnett, T.P.; Pierce, D.W.; Hidalgo, H.G.; Bonfils, C.; Santer, B.D.; Das, T.; Bala, G.; Wood, A.W.; Nozawa, T.; Mirin, A.A.; et al. Human-Induced Changes in the Hydrology of the Western United States; DigitalCommons, University of Nebraska-Lincoln: Lincoln, NE, USA, 2008. [Google Scholar]
- Milly, P.C.D.; Betancourt, J.; Falkenmark, M.; Hirsch, R.M.; Kundzewicz, Z.W.; Lettenmaier, D.P.; Stouffer, R.J. Stationarity is dead: Whither water management? Science 2008, 319, 573–574. [Google Scholar] [CrossRef]
- Karl, T.R.; Knight, R.W.; Plummer, N. Trends in high-frequency climate variability in the twentieth century. Nature 1995, 377, 217–220. [Google Scholar] [CrossRef]
- Karl, T.R.; Knight, R.W. Secular trends of precipitation amount, frequency, and intensity in the United States. Bull. Am. Meteorol. Soc. 1998, 79, 231–241. [Google Scholar] [CrossRef]
- Wang, D.; Hagen, S.C.; Alizad, K. Climate change impact and uncertainty analysis of extreme rainfall events in the Apalachicola River basin, Florida. J. Hydrol. 2013, 480, 125–135. [Google Scholar] [CrossRef]
- FAO; IFAD; UNICEF; WFP; WHO. The State of Food Security and Nutrition in the World 2018. Building Climate Resilience for Food Security and Nutrition; FAO: Rome, Italy, 2018. [Google Scholar]
- Abida, H.; Sabourin, J.F. Grass swale-perforated pipe system for stormwater management. J. Irrig. Drain. Eng. 2006, 132, 55–63. [Google Scholar] [CrossRef]
- Livingston, E.H. State perspectives on water quality criteria, design of urban runoff quality controls. In Proceedings of the Engineering Foundation Conference, Palm Coast, FL, USA, 10–15 January 1988; pp. 15–218. [Google Scholar]
- Goddard, J.B. Storm Water Detention/Retention: A New Solution. Public Works Magazine. 1 June 1984. [Google Scholar]
- Schueler, T.R. Controlling Urban Runoff: A Practical Manual for Planning and Designing Urban BMP’S; Department of Environmental Programs, Metropolitan Washington Council of Governments: Washington, DC, USA, 1987. [Google Scholar]
- Stahre, P.; Urbonas, B. Swedish approach to infiltration and percolation design. In Design of Urban Runoff Quality Controls; ASCE: New York, NY, USA, 1989; pp. 307–322. [Google Scholar]
- Tu, M.C.; Traver, R.G. Optimal configuration of an underdrain delivery system for a stormwater infiltration trench. J. Irrig. Drain. Eng. 2019, 145, 05019007. [Google Scholar] [CrossRef]
- Murphy, P.; Kaye, N.B.; Khan, A.A. Hydraulic Performance of Aggregate Beds with Perforated Pipe Underdrains Flowing Full. J. Irrig. Drain. Eng. 2014, 140, 04014023. [Google Scholar] [CrossRef]
- Afrin, T.; Khan, A.A.; Kaye, N.B.; Testik, F.Y. Numerical Model for the Hydraulic Performance of Perforated Pipe Underdrains Surrounded by Loose Aggregate. J. Hydraul. Eng. 2016, 142, 04016018. [Google Scholar] [CrossRef]
- Li, Y.; Buchberger, S.G.; Sansalone, J.J. Variably saturated flow in stormwater partial exfiltration trench. J. Environ. Eng. 1999, 125, 556–565. [Google Scholar] [CrossRef]
- Guo, J.C.Y.; Kocman, S.M.; Ramaswami, A. Design of two layered porous landscaping detention basin. J. Environ. Eng. 2009, 135, 1268–1274. [Google Scholar] [CrossRef]
- Schwartz, S.S. Effective curve number and hydrologic design of pervious concrete storm-water systems. J. Hydrol. Eng. 2010, 15, 465–474. [Google Scholar] [CrossRef]
- Guo, J.C.Y. Cap-orifice as a flow regulator for rain garden design. J. Irrig. Drain. Eng. 2012, 138, 198–202. [Google Scholar] [CrossRef]
- Akan, A.O. Preliminary design aid for bioretention filters. J. Hydrol. Eng. 2013, 18, 318–323. [Google Scholar] [CrossRef]
- Zakaria, N.A.; Ab Ghani, A.; Abdullah, R.; Mohd Sidek, L.; Ainan, A. Bio-ecological drainage system (BIOECODS) for water quantity and quality control. Int. J. River Basin Manag. 2003, 1, 237–251. [Google Scholar] [CrossRef]
- Ab Ghani, A.; Zakaria, N.A.; Abdullah, R.; Yusof, M.F.; Sidek, L.M.; Kassim, A.H.; Ainan, A. Bio-Ecological Drainage System (BIOECODS): Concept, Design and Construction. In Proceedings of the International Conference on HydroScience and Engineering, Brisbane, Australia, 31 May–3 June 2004. [Google Scholar]
- Lai, S.H.; Kee, L.C.; Zakaria, N.A.; Ab Ghani, A.; Chang, C.K.; Leow, C.S. Flow Pattern and Hydraulic Characteristic for Subsurface Drainage Module. In Proceedings of the International Conference on Water Resources (ICWR 2009), Langkawi, Kedah, Malaysia, 26–27 May 2009. [Google Scholar]
- Kee, L.C.; Zakaria, N.A.; Lau, T.L.; Chang, C.K.; Ab Ghani, A. Determination of manning’s for subsurface modular channel. In Proceedings of the 3rd International Conference on Managing Rivers in 21st Century: Sustainable Solutions for Global Crisis of Flooding, Pollution and Water Scarcity (Rivers 2011), Penang, Malaysia, 6–9 December 2011. [Google Scholar]
- Muhammad, M.M.; Yusof, K.W.; Mustafa, M.R.U.; Zakaria, N.A.; Ab Ghani, A. Prediction models for flow resistance in flexible vegetated channels. Int. J. River Basin Manag. 2018, 16, 427–437. [Google Scholar] [CrossRef]
- Mohammadpour, R.; Kashfy, M.; Zakaria, N.A.; Ab Ghani, A. Manning’s Roughness Coefficient for Ecological Subsurface Channel with Modules. Int. J. River. Basin. Manag. 2020, 18, 349–361. [Google Scholar] [CrossRef]
- Gaj, N.; Madramootoo, C.A. Effects of Perforation Geometry on Pipe Drainage in Agricultural Lands. J. Irrig. Drain. Eng. 2020, 146, 04020015. [Google Scholar] [CrossRef]
- Ghane, E. Agricultural Drainage; Extension Bulletin E3370; Michigan State University (MSU): East Lansing, MI, USA, 2018. [Google Scholar]
- Ghane, E. Choice of pipe material influences drain spacing and system cost in subsurface drainage design. Appl. Eng. Agric. 2022, 38, 685–695. [Google Scholar] [CrossRef]
- Oyarce, P.; Gurovich, L.; Duarte, V. Experimental evaluation of agricultural drains. J. Irrig. Drain. Eng. 2016, 143, 04016082. [Google Scholar] [CrossRef]
- Gurovich, L.; Oyarce, P. Modeling Agricultural Drainage Hydraulic Nets. Irrig. Drain. Sys. Eng. 2015, 4, 1000149. [Google Scholar] [CrossRef]
- DID (JPS) Malaysia. Urban Stormwater Management Manual for Malaysia (MSMA), 1st ed.; DID (JPS) Malaysia: Kuala Lumpur, Malaysia, 2000. [Google Scholar]
- Ab Ghani, A.; Zakaria, N.A.; Kiat, C.C.; Ariffin, J.; Hasan, Z.A.; Abdul Ghaffar, A.B. Revised equations for Manning’s coefficient for Sand-Bed Rivers. Int. J. River Basin Manag. 2007, 5, 329–346. [Google Scholar] [CrossRef]
- Pradhan, A.; Khatua, K.K. Assessment of roughness coefficient for meandering compound channels. KSCE J. Civ. Eng. 2018, 22, 2010–2022. [Google Scholar] [CrossRef]
- Zainalfikry, M.K.; Ab Ghani, A.; Zakaria, N.A.; Chan, N.W. Flow Resistance in Ecological Subdrainage Channel. Lect. Notes Civ. Eng. 2020, 53, 1117–1127. [Google Scholar]
- Bakry, M.F. Effect of submerged weeds on the design: Procedure of Earthen Egyptian Canals. Irrig. Drain. Sys. 1992, 6, 179–188. [Google Scholar] [CrossRef]
- Trout, T.J. Furrow flow velocity effect on hydraulic roughness. J. Irrig. Drain. Eng. 1992, 118, 981–987. [Google Scholar] [CrossRef]
- Chang, T.H.; Huang, S.T.; Chen, S.; Lai, J.C. Estimation of Manning roughness coefficient on precast ecological concrete blocks. J. Mar. Sci. Technol. 2010, 18, 308–316. [Google Scholar] [CrossRef]
- Chen, Y.C.; Kao, S.P.; Lin, J.Y.; Yang, H.C. Retardance coefficient of vegetated channels estimated by the Froude Number. Ecol. Eng. 2009, 35, 1027–1035. [Google Scholar] [CrossRef]
- Fathi-Moghadam, M.; Drikvandi, K. Manning roughness coefficient for rivers and flood plains with non-submerged vegetation. Int. J. Hydraul. Eng. 2012, 1, 1–4. [Google Scholar]
- Conesa-García, C.; Sanchez-Tudela, J.L.; Perez-Cutillas, P.; Martinez Capel, F. Spatial variation of the vegetative roughness in Mediterranean torrential streams affected by check dams. Hydrol. Sci. J. 2018, 63, 114–135. [Google Scholar] [CrossRef]

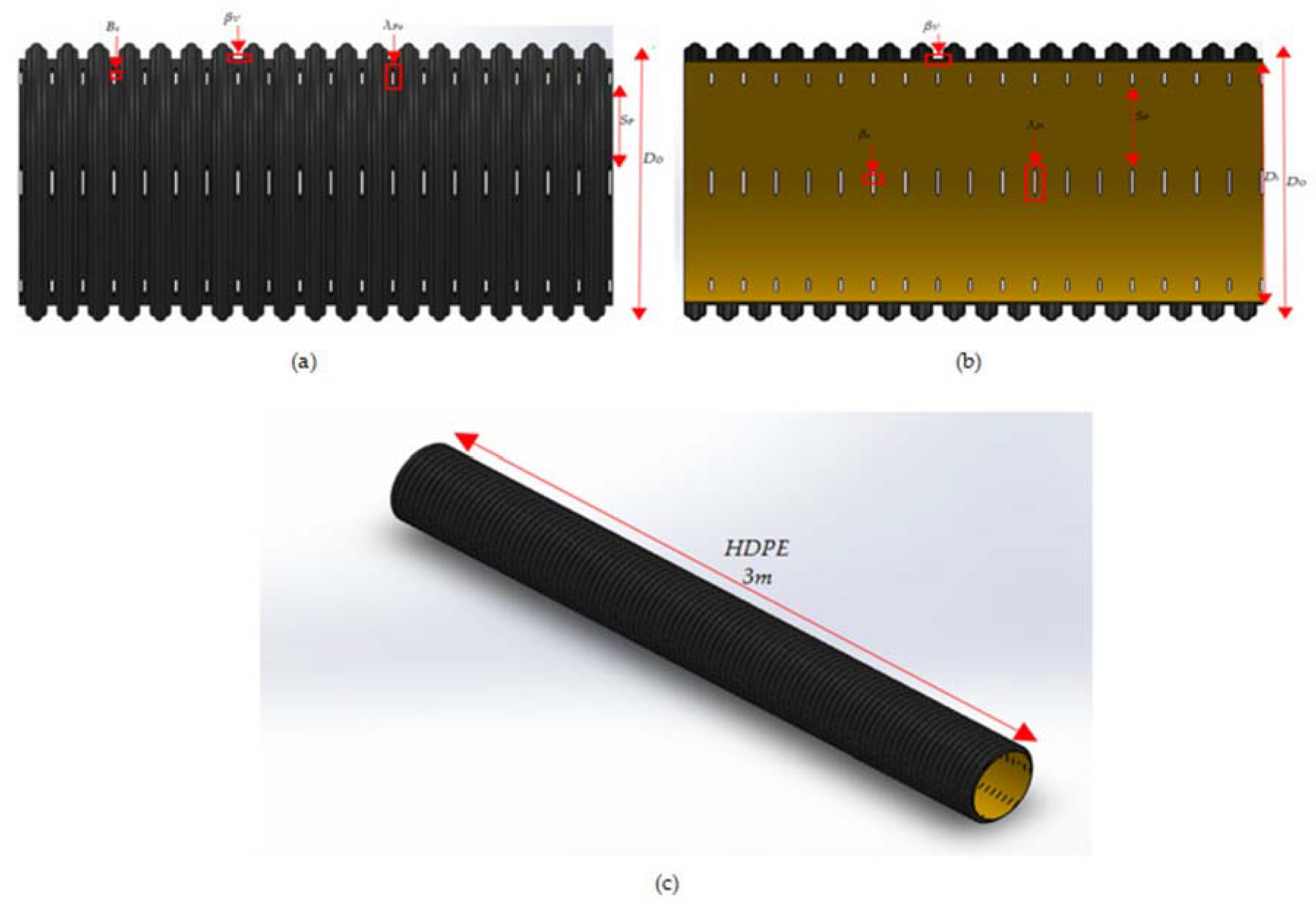
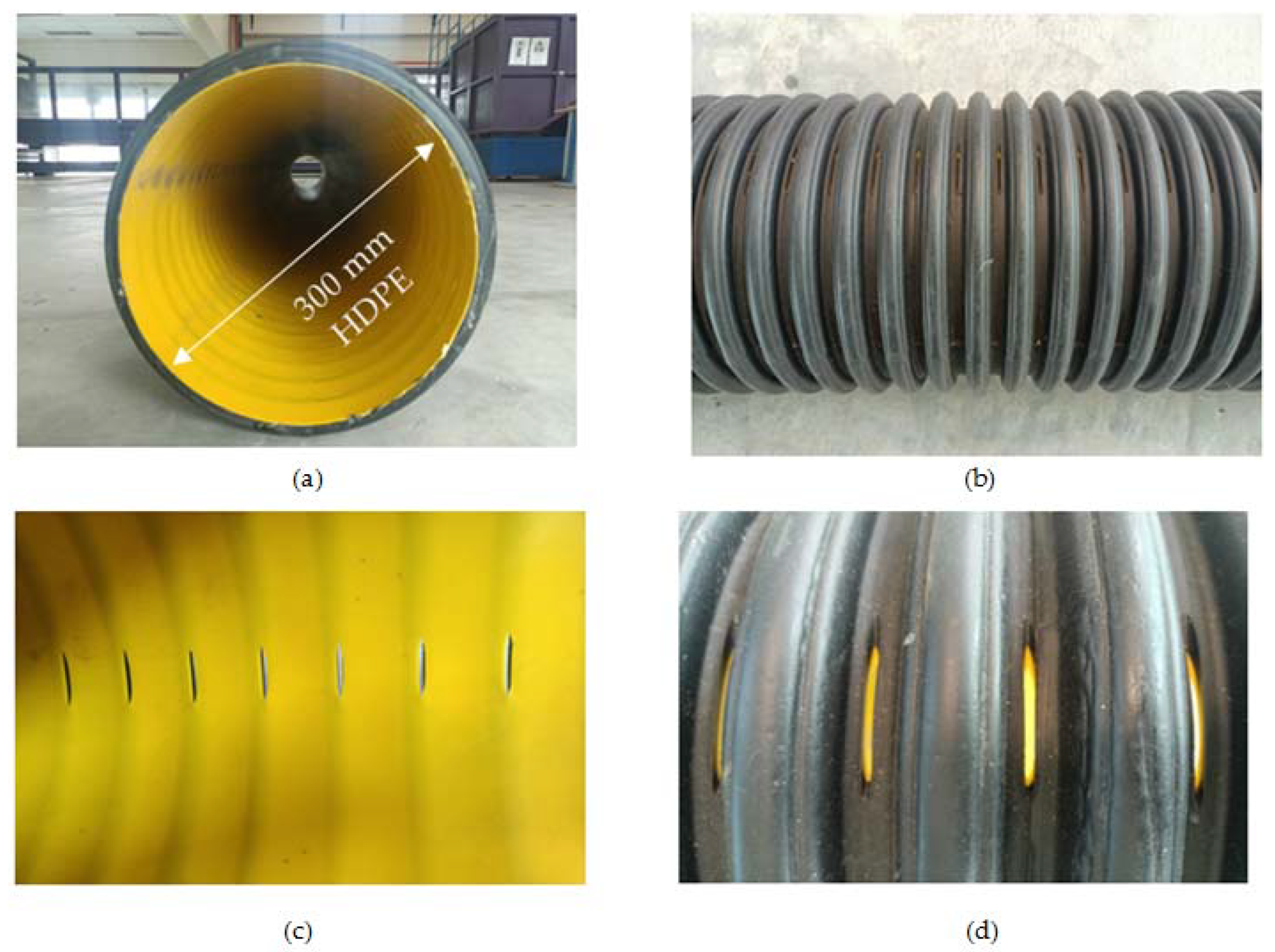
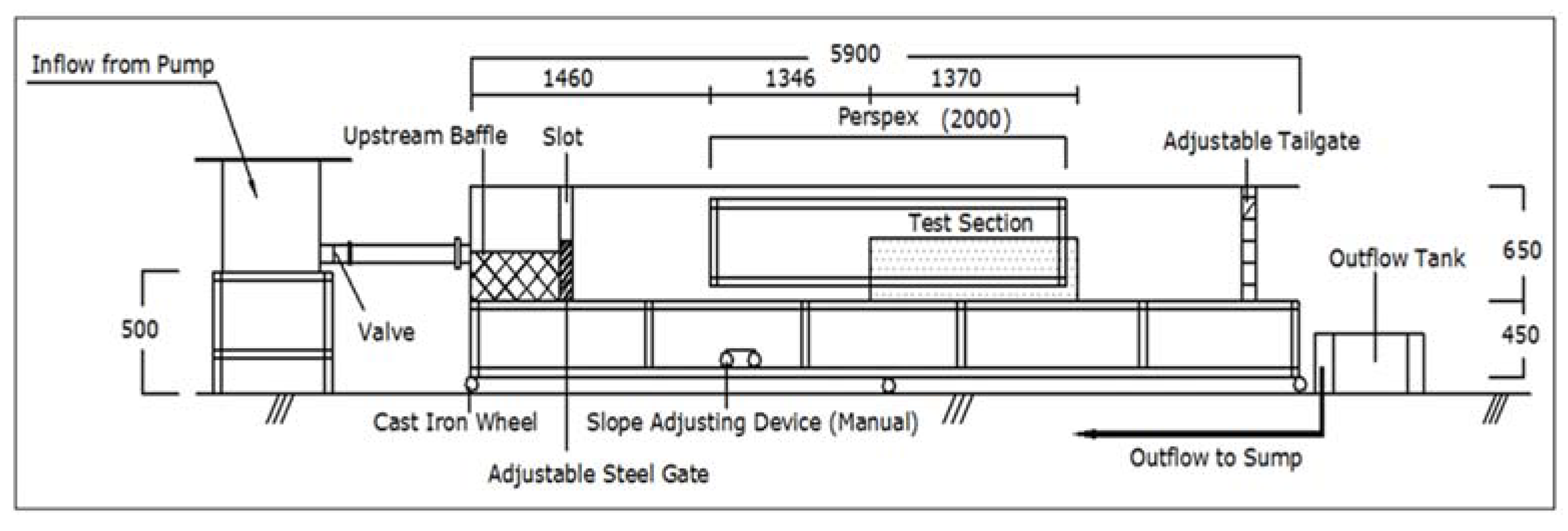
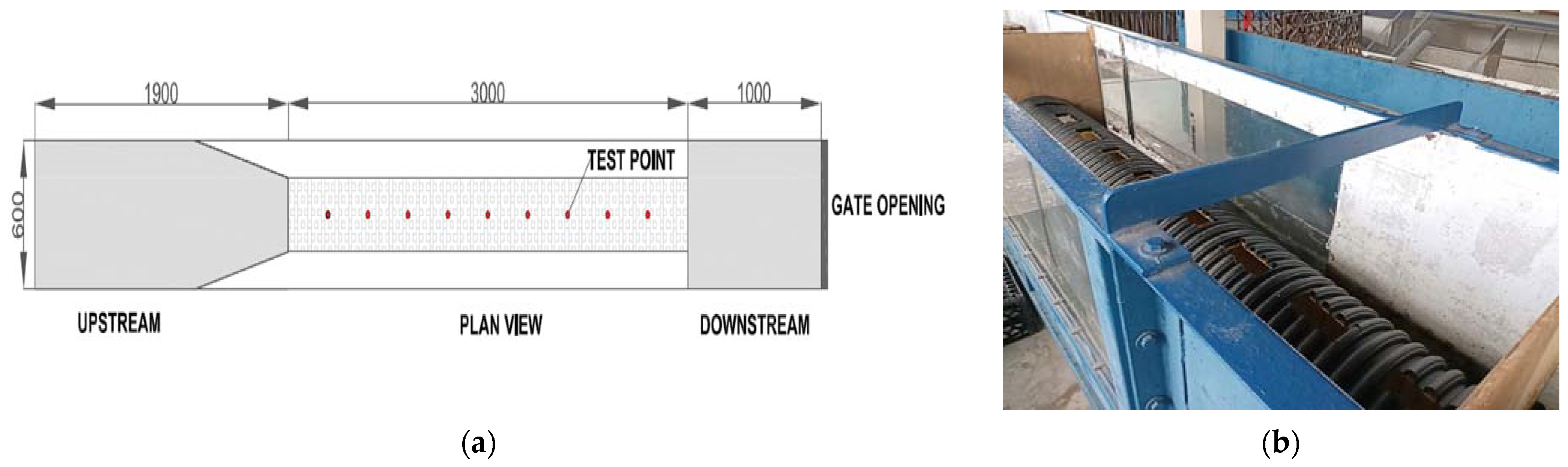

| Flow Parameter | Range | |||
|---|---|---|---|---|
| Perforated Pipe under GFO Conditions | Perforated Pipe under GPO Conditions | |||
| Slope 1:750 | Slope 1:1000 | Slope 1:750 | Slope 1:1000 | |
| Flow Rate, Q (m3/s) | 0.017–0.021 | 0.017–0.021 | 0.008–0.009 | 0.013–0.015 |
| Velocity, V (m/s) | 0.810–1.117 | 0.817–1.070 | 0.620–0.660 | 0.537–0.603 |
| Flow Depth, Y (m) | 0.080–0.120 | 0.080–0.120 | 0.100–0.110 | 0.110–0.120 |
| Hydraulic Radius, R (m) | 0.046–0.062 | 0.047–0.062 | 0.056–0.058 | 0.061–0.062 |
| Reynolds Number, Re | 57,912–64,801 | 57,124–64,393 | 41,041–43,103 | 38,252–43,579 |
| Froude Number, Fr | 0.763–1.261 | 0.769–1.193 | 0.611–0.666 | 0.508–0.568 |
| Parameter | Range | |||
|---|---|---|---|---|
| Perforated Pipe under GFO Conditions | Perforated Pipe under GPO Conditions | |||
| Slope 1:750 | Slope 1:1000 | Slope 1:750 | Slope 1:1000 | |
| Manning’n | 0.004–0.007 | 0.004–0.006 | 0.008–0.009 | 0.008–0.009 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abdullah, J.; Mohd Arif Zainol, M.R.R.; Riahi, A.; Zakaria, N.A.; Yusof, M.F.; Shaharuddin, S.; Alias, M.N.; Mohd Kasim, M.Z.; Abdul Aziz, M.S.; Mohamed Noor, N.; et al. Investigating the Relationship between the Manning Coefficients (n) of a Perforated Subsurface Stormwater Drainage Pipe and the Hydraulic Parameters. Sustainability 2023, 15, 6929. https://doi.org/10.3390/su15086929
Abdullah J, Mohd Arif Zainol MRR, Riahi A, Zakaria NA, Yusof MF, Shaharuddin S, Alias MN, Mohd Kasim MZ, Abdul Aziz MS, Mohamed Noor N, et al. Investigating the Relationship between the Manning Coefficients (n) of a Perforated Subsurface Stormwater Drainage Pipe and the Hydraulic Parameters. Sustainability. 2023; 15(8):6929. https://doi.org/10.3390/su15086929
Chicago/Turabian StyleAbdullah, Junaidah, Mohd Remy Rozainy Mohd Arif Zainol, Ali Riahi, Nor Azazi Zakaria, Mohd Fazly Yusof, Syafiq Shaharuddin, Muhammad Nurfasya Alias, Muhammad Zaki Mohd Kasim, Mohd Sharizal Abdul Aziz, Norazian Mohamed Noor, and et al. 2023. "Investigating the Relationship between the Manning Coefficients (n) of a Perforated Subsurface Stormwater Drainage Pipe and the Hydraulic Parameters" Sustainability 15, no. 8: 6929. https://doi.org/10.3390/su15086929
APA StyleAbdullah, J., Mohd Arif Zainol, M. R. R., Riahi, A., Zakaria, N. A., Yusof, M. F., Shaharuddin, S., Alias, M. N., Mohd Kasim, M. Z., Abdul Aziz, M. S., Mohamed Noor, N., Zawawi, M. H., & Ikhsan, J. (2023). Investigating the Relationship between the Manning Coefficients (n) of a Perforated Subsurface Stormwater Drainage Pipe and the Hydraulic Parameters. Sustainability, 15(8), 6929. https://doi.org/10.3390/su15086929








