Triple-Frequency Code-Phase Combination Determination: A Comparison with the Hatch-Melbourne-Wübbena Combination Using BDS Signals
Abstract
:1. Introduction
- (1)
- The noise levels of three original code measurements are no longer identical [29], thus, the calculated total noise should be modified;
- (2)
2. Materials and Methods
2.1. General Functional Model
2.2. GF Code-Phase Combination
2.3. A Two-Step Method
3. Results
3.1. Optimal and Suboptimal Combinations
3.2. Data Description
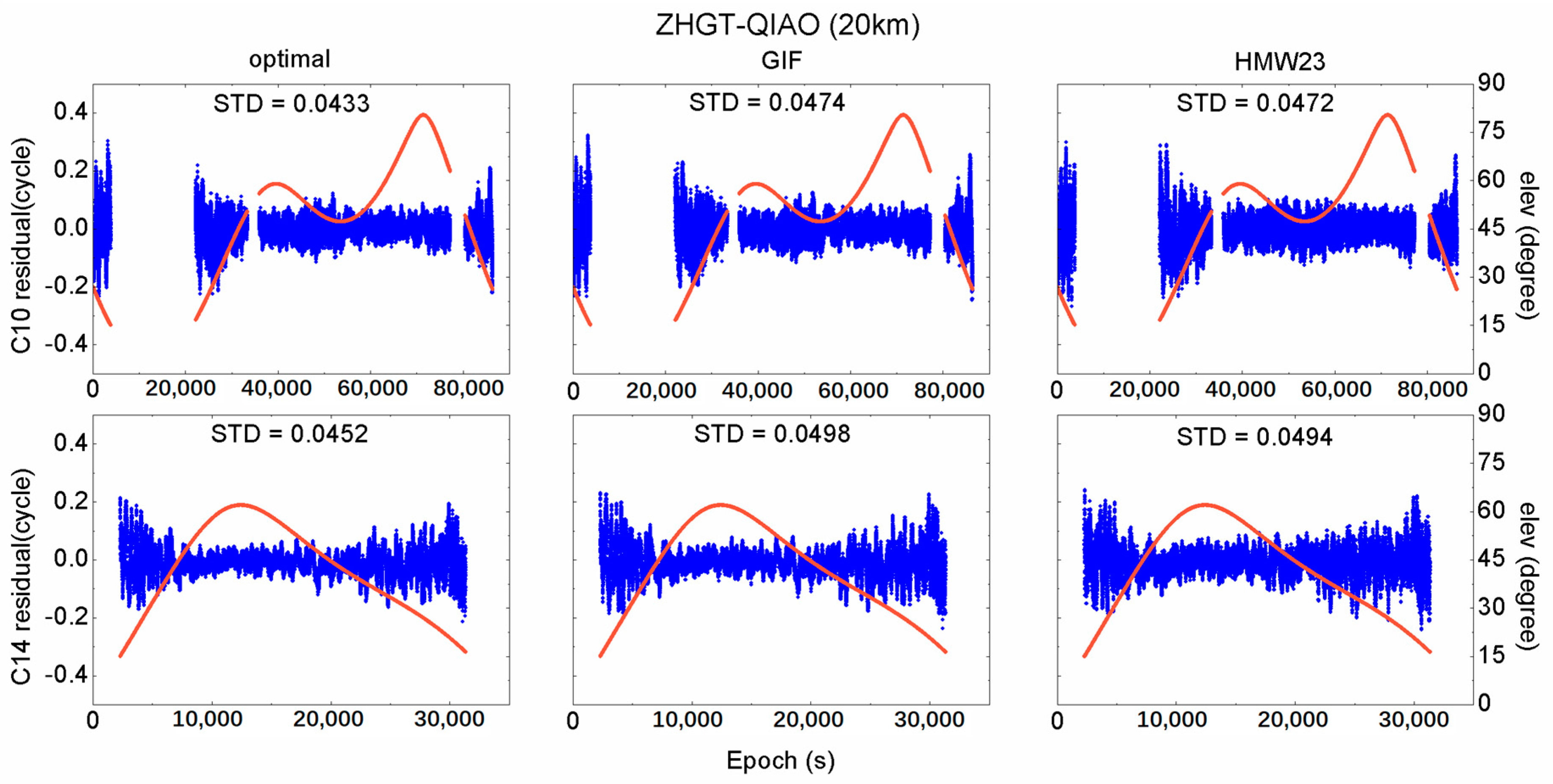
3.3. Optimal Combination
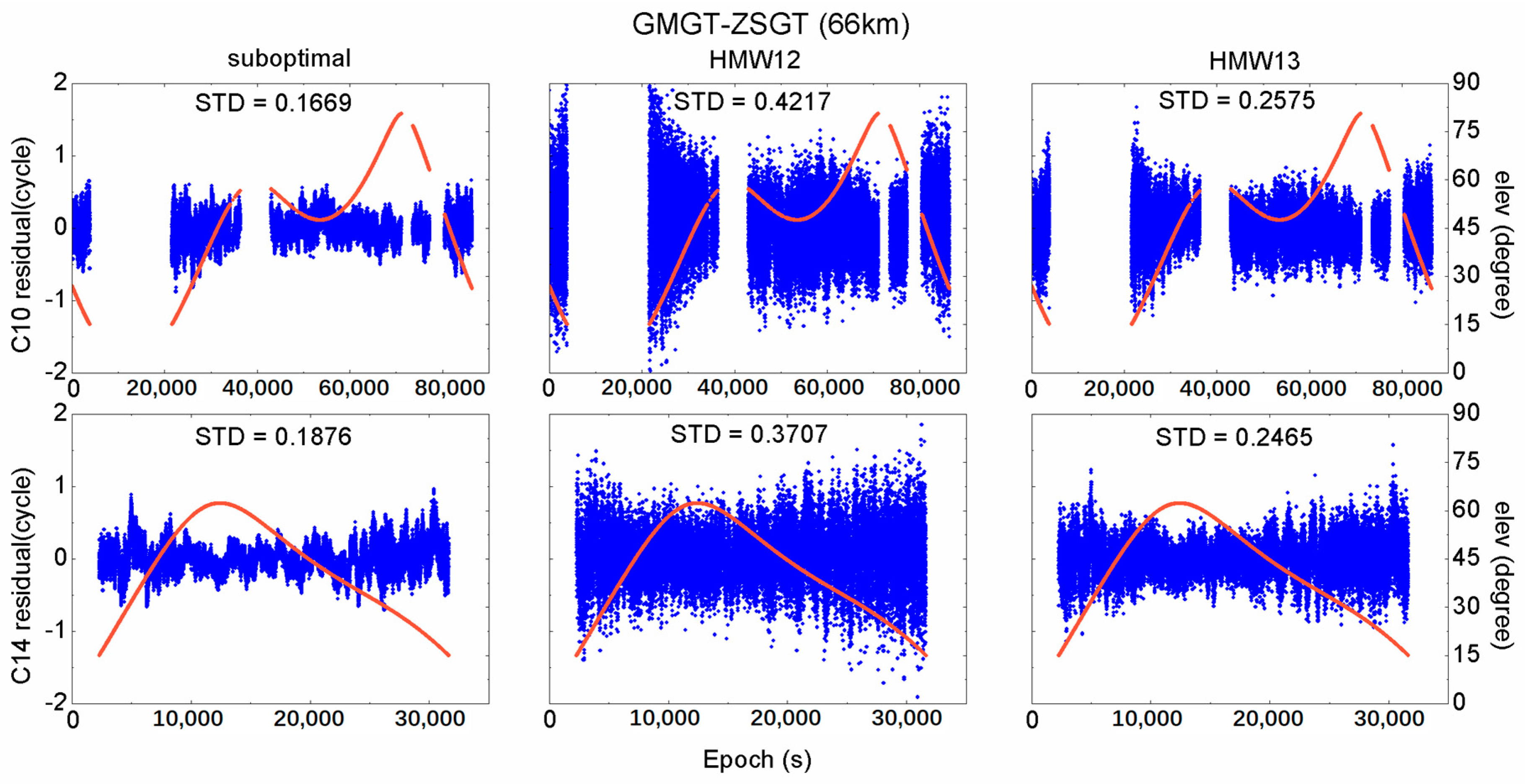
3.4. Suboptimal Combination
4. Discussion
5. Conclusions
- Although the optimal combination and the HMW23 combination can both achieve almost 100% AR success rate by rounding, the STD values of the ambiguity residual for the optimal combination are slightly better than those of the HMW23 combination, especially when the satellite elevation angle is higher than 45°. Specifically, an improvement of nearly 25% can be achieved for C10 satellite in the medium-long baseline.
- The suboptimal combination can achieve an AR success rate around 99% by rounding. Compared with the HMW12 and HMW13 combinations, the suboptimal combination achieves best results in all baselines, with a dramatic improvement of about 50% and 20%, respectively.
- Using the correctly fixed optimal and suboptimal ambiguities, the WL ambiguity can then be instantaneously determined and the ionosphere delay on B1 can be estimated. Therefore, decimeter-level kinematic positioning service can be provided.
Acknowledgments
Author Contributions
Conflicts of Interest
Appendix A. Calculation of the Theoretical AR Success Rate
References
- Lacy, M.; Reguzzoni, M.; Sansò, F. Real-time cycle slip detection in triple-frequency GNSS. GPS Solut. 2012, 16, 353–362. [Google Scholar] [CrossRef]
- Zhao, Q.; Sun, B.; Dai, Z.; Hu, Z.; Shi, C.; Liu, J. Real-time detection and repair of cycle slips in triple- frequency GNSS measurements. GPS Solut. 2015, 19, 381–391. [Google Scholar] [CrossRef]
- Huang, L.; Lu, Z.; Zhai, G.; Ouyang, Y.; Huang, M.; Lu, X.; Wu, T.; Li, K. A new triple-frequency cycle slip detecting algorithm validated with BDS data. GPS Solut. 2016, 20, 761–769. [Google Scholar] [CrossRef]
- Hatch, R.; Jung, J.; Enge, P.; Pervan, B. Civilian GPS: The benefits of three frequencies. GPS Solut. 2000, 3, 1–9. [Google Scholar] [CrossRef]
- Jia, C.; Zhao, L.; Li, L.; Li, H.; Cheng, J.; Li, Z. Improving the triple-carrier ambiguity resolution with a new ionosphere-free and variance-restricted method. Remote Sens. 2017, 9, 1108. [Google Scholar] [CrossRef]
- Li, B.; Feng, Y.; Gao, W.; Li, Z. Real-time kinematic positioning over long baselines using triple-frequency Beidou signals. IEEE Trans. Aerosp. Electr. Syst. 2016, 51, 3254–3269. [Google Scholar] [CrossRef]
- Li, B.; Feng, Y.; Shen, Y. Three carrier ambiguity resolution: Distance-independent performance demonstrated using semi-generated triple frequency GPS signals. GPS Solut. 2010, 14, 177–184. [Google Scholar] [CrossRef]
- Xu, Y.; Ji, S.; Chen, W.; Weng, D. A new ionosphere-free ambiguity resolution method for long-range baseline with GNSS triple-frequency signals. Adv. Space Res. 2015, 56, 1600–1612. [Google Scholar] [CrossRef]
- Guo, F.; Zhang, X.; Wang, J.; Ren, X. Modeling and assessment of triple-frequency BDS precise point positioning. J. Geod. 2016, 90, 1223–1235. [Google Scholar] [CrossRef]
- Geng, J.; Bock, Y. Triple-frequency GPS precise point positioning with rapid ambiguity resolution. J. Geod. 2013, 87, 449–460. [Google Scholar] [CrossRef]
- Cocard, M.; Geiger, A. Systematic search for all possible widelanes. In Proceedings of the 6th International Geodesy Symposium on Satellite Positioning, Columbus, OH, USA, 17–20 March 1992; pp. 312–318. [Google Scholar]
- Richert, T.; El-Sheimy, N. Optimal linear combinations of triple frequency carrier phase data from future global navigation satellite systems. GPS Solut. 2007, 11, 11–19. [Google Scholar] [CrossRef]
- Cocard, M.; Bourgon, S.; Kamali, O.; Collins, P. A systematic investigation of optimal carrier-phase combinations for modernized triple-frequency GPS. J. Geod. 2008, 82, 555–564. [Google Scholar] [CrossRef]
- Urquhart, L. An Analysis of Multi-Frequency Carrier Phase Linear Combinations for Gnss; Technol Report No. 263; Department of Geodesy and Geomatics Engineering, University of New Brunswick: Fredericton, NB, Canada, 2009; p. 71. [Google Scholar]
- Li, J.; Yang, Y.; He, H.; Guo, H. An analytical study on the carrier-phase linear combinations for triple-frequency GNSS. J. Geod. 2017, 91, 151–166. [Google Scholar] [CrossRef]
- Zhang, X.; He, X. BDS triple-frequency carrier-phase linear combination models and their characteristics. Sci. China Earth Sci. 2015, 58, 896–905. [Google Scholar] [CrossRef]
- Han, S.; Rizos, C. The impact of two additional civilian GPS frequencies on ambiguity resolution strategies. In Proceedings of the ION Annual Technical Meeting, Cambridge, MA, USA, 29 June–1 July 1992. [Google Scholar]
- Feng, Y. GNSS three carrier ambiguity resolution using ionosphere-reduced virtual signals. J. Geod. 2008, 82, 847–862. [Google Scholar] [CrossRef]
- Hatch, R. The synergism of GPS code and carrier measurements. In Proceedings of the third international symposium on satellite Doppler positioning, Las Cruces, NM, USA, 8–12 February 1982; pp. 1213–1231. [Google Scholar]
- Melbourne, W. The case for ranging in GPS based geodetic systems. In Proceedings of the 1st International Symposium on Precise Positioning with the Global Positioning System, Rockville, MD, USA, 15–19 April 1985; pp. 373–386. [Google Scholar]
- Wübbena, G. Software developments for geodetic positioning with GPS using TI 4100 code and carrier measurements. In Proceedings of the 1st International Symposium on Precise Positioning with the Global Positioning System, Rockville, MD, USA, 15–19 April 1985; pp. 403–412. [Google Scholar]
- Geng, J.; Teferle, F.; Meng, X.; Dodson, A. Towards PPP-RTK: Ambiguity resolution in real-time precise point positioning. Adv. Space Res. 2011, 47, 1664–1673. [Google Scholar] [CrossRef]
- Henkel, P.; Günther, C. Reliable integer ambiguity resolution: Multi-frequency code carrier linear combinations and statistical a priori knowledge of attitude. Navigation 2012, 59, 90–103. [Google Scholar] [CrossRef]
- Wang, K.; Rothacher, M. Ambiguity resolution for triple-frequency geometry-free and ionosphere-free combination tested with real data. J. Geod. 2013, 87, 539–553. [Google Scholar] [CrossRef]
- Deo, M.; El-Mowafy, A. Triple-frequency GNSS models for PPP with float ambiguity estimation: Performance comparison using GPS. Surv. Rev. 2016, 1–13. [Google Scholar] [CrossRef]
- Zhao, D.; Roberts, G.; Lau, L.; Hancock, C.; Bai, R. A theoretical and empirical integrated method to select the optimal combined signals for geometry-free and geometry-based three-carrier ambiguity resolution. Sensors 2016, 16. [Google Scholar] [CrossRef] [PubMed]
- Zhao, Q.; Dai, Z.; Hu, Z.; Sun, B.; Shi, C.; Liu, J. Three-carrier ambiguity resolution using the modified TCAR method. GPS Solut. 2015, 19, 589–599. [Google Scholar] [CrossRef]
- Li, J.; Yang, Y.; Xu, J.; He, H.; Guo, H. Real-time cycle-slip detection and repair based on code-phase combinations for GNSS triple-frequency un-differenced observations. Acta Geod. Cartogr. Sin. 2011, 40, 717–722. (In Chinese) [Google Scholar]
- Tang, W.; Deng, C.; Shi, C.; Liu, J. Triple-frequency carrier ambiguity resolution for Beidou navigation satellite system. GPS Solut. 2014, 18, 335–344. [Google Scholar] [CrossRef]
- Wanninger, L.; Beer, S. BeiDou satellite-induced code pseudorange variations: Diagnosis and therapy. GPS Solut. 2015, 19, 639–648. [Google Scholar] [CrossRef]
- Zhang, X.; He, X.; Liu, W. Characteristics of systematic errors in the BDS Hatch–Melbourne–Wübbena combination and its influence on wide-lane ambiguity resolution. GPS Solut. 2017, 21, 265–277. [Google Scholar] [CrossRef]
- Lou, Y.; Gong, X.; Gu, S.; Zheng, F.; Feng, Y. Assessment of code bias variations of BDS triple-frequency signals and their impacts on ambiguity resolution for long baselines. GPS Solut. 2017, 21, 177–186. [Google Scholar] [CrossRef]
- BeiDou Navigation Satellite System Signal in Space Interface Control Document: Open Service Signals B1C (Version 1.0). Available online: http://www.beidou.gov.cn/xt/gfxz/201712/P020171226741342013031.pdf (accessed on 27 December 2017).
- BeiDou Navigation Satellite System Signal in Space Interface Control Document: Open Service Signals B2a (Version 1.0). Available online: http://www.beidou.gov.cn/xt/gfxz/201712/P020171226742357364174.pdf (accessed on 27 December 2017).
- Feng, Y.; Li, B. Wide area real time kinematic decimetre positioning with multiple carrier GNSS signals. Sci. China Earth Sci. 2010, 53, 731–740. [Google Scholar] [CrossRef]
- He, X.; Zhang, X.; Tang, L.; Liu, W. Instantaneous real-time kinematic decimeter-level positioning with BeiDou triple-frequency signals over medium baselines. Sensors, 2016, 16, 1–19. [Google Scholar] [CrossRef] [PubMed]
- Li, B.; Li, Z.; Zhang, Z.; Tan, Y. ERTK: Extra-wide-lane RTK of triple-frequency GNSS signals. J. Geod. 2017, 91, 1031–1047. [Google Scholar] [CrossRef]
- Gao, W.; Gao, C.; Pan, S.; Wang, D.; Wang, S. Single-epoch positioning method in network RTK with BDS triple-frequency widelane combinations. Acta Geod. Cartogr. Sin. 2015, 44, 641–648. [Google Scholar]
- Teunissen, P.J.G. An optimality property of the integer least-squares estimator. J. Geod. 1999, 73, 587–593. [Google Scholar] [CrossRef]
- Teunissen, P.J.G. The least-squares ambiguity decorrelation adjustment: A method for fast GPS integer ambiguity estimation. J. Geod. 1995, 70, 65–82. [Google Scholar] [CrossRef]
- Jazaeri, S.; Amiri-Simkooei, A.; Sharifi, M. Fast integer least-squares estimation for GNSS high-dimensional ambiguity resolution using lattice theory. J. Geod. 2012, 86, 123–136. [Google Scholar] [CrossRef]
- Wang, J.; Feng, Y. Reliability of partial ambiguity fixing with multiple GNSS constellations. J. Geod. 2013, 87, 1–14. [Google Scholar] [CrossRef]
- Li, J.; Yang, Y.; Xu, J.; He, H.; Guo, H. GNSS multi-carrier fast partial ambiguity resolution strategy tested with real BDS/GPS dual- and triple-frequency observations. GPS Solut. 2015, 19, 5–13. [Google Scholar] [CrossRef]
- Paziewski, J.; Wielgosz, P. Investigation of some selected strategies for multi-GNSS instantaneous RTK positioning. Adv. Space Res. 2017, 51, 12–23. [Google Scholar] [CrossRef]
- Verhagen, S. Integer ambiguity validation: An open problem? GPS Solut. 2004, 8, 36–43. [Google Scholar] [CrossRef]
- Teunissen, P. Success probability of integer GPS ambiguity rounding and bootstrapping. J. Geod. 1998, 72, 606–612. [Google Scholar] [CrossRef]
- Zou, X.; Li, Z.; Li, M.; Tang, W.; Deng, C.; Chen, L.; Wang, C.; Shi, C. Modeling BDS pseudorange variations and models assessment. GPS Solut. 2017, 21, 1661–1668. [Google Scholar] [CrossRef]





| Reference | Characteristics | Limitation |
|---|---|---|
| [12,13,14,15,16] | (1) Theoretically discussed the factors that effects the performance of combinations (2) Gave the general selection procedure and specific useful combinations | (1) Only carrier phase is used (2) The ambiguity should be searched due to the used geometry-based model |
| [10,17,18] | select combinations that reduce or eliminate the effect of ionosphere delay | |
| [23,24] | (1) Mixed code-phase combinations (2) Minimize the noise level of the combinations and fix ambiguity by rounding with high success rate | No BDS data are validated |
| [8,26,27] | (1) Mixed code-phase combinations (2) Fix ambiguity by rounding | WL ambiguity fixing relies on the resolved EWL ambiguity |
| [3,28] | (1) Mixed code-phase combinations (2) Fix ambiguity by rounding | no specific discussion of the code coefficients in the combination |
| (m) | (i, j, k) | (m) | (Cycle) | (%) | ||||
|---|---|---|---|---|---|---|---|---|
| 0.0 | (0, −1, 1) | 4.8842 | −0.09 | 0.0370 | 0.0370 | 0.9259 | 0.0423 | 100.00 |
| 0.1 | (0, −1, 1) | 4.8842 | −0.09 | 0.0367 | 0.0372 | 0.9262 | 0.0423 | 100.00 |
| 0.5 | (0, −1, 1) | 4.8842 | −0.08 | 0.0191 | 0.0432 | 0.9378 | 0.0431 | 100.00 |
| 1.0 | (0, −1, 1) | 4.8842 | −0.05 | −0.0337 | 0.0612 | 0.9725 | 0.0445 | 100.00 |
| (m) | (i, j, k) | (m) | (Cycle) | (%) | ||||
|---|---|---|---|---|---|---|---|---|
| 0.0 | (1, 1, −2) | 1.2967 | 0.37 | 0.0370 | 0.0370 | 0.9259 | 0.1099 | 99.999 |
| 0.1 | (1, 1, −2) | 1.2967 | 0.36 | 0.0484 | 0.0332 | 0.9185 | 0.1134 | 99.999 |
| 0.5 | (1, 1, −2) | 1.2967 | 0.31 | 0.1363 | 0.0031 | 0.8605 | 0.1696 | 99.681 |
| 1.0 | (1, 0, −1) | 1.0247 | 0.15 | 0.2493 | −0.0354 | 0.7861 | 0.2308 | 96.973 |
| Baseline | Dist (km) | Optimal Combination | Suboptimal Combination | ||
|---|---|---|---|---|---|
| (i, j, k) | (, , ) | (i, j, k) | (, , ) | ||
| ZHGT-QIAO | 20 | (0, −1, 1) | (0.0367, 0.0372, 0.9262) | (1, 1, −2) | (0.0484, 0.0332, 0.9185) |
| GMGT-ZSGT | 66 | (0, −1, 1) | (0.0191, 0.0432, 0.9378) | (1, 1, −2) | (0.1363, 0.0031, 0.8605) |
| YFGT-ZSGT | 147 | (0, −1, 1) | (−0.0337, 0.0612, 0.9725) | (1, 0, −1) | (0.2493, −0.0354, 0.7861) |
| Baseline | Dist (km) | STD (Cycle) | (%) | (Cycle) | ||
|---|---|---|---|---|---|---|
| Optimal | GIF | HMW23 | ||||
| ZHGT-QIAO | 20 | 0.0472 | 0.0539 | 0.0520 | 9.231 | 0.0067 |
| GMGT-ZSGT | 66 | 0.0555 | 0.0613 | 0.0667 | 16.792 | 0.0058 |
| YFGT-ZSGT | 147 | 0.0580 | 0.0618 | 0.0666 | 12.913 | 0.0038 |
| Baseline | Dist (km) | STD (Cycle) | (%) | (%) | (%) | ||
|---|---|---|---|---|---|---|---|
| Suboptimal | HMW12 | HMW13 | |||||
| ZHGT-QIAO | 20 | 0.1963 | 0.3112 | 0.2241 | 98.914 | 36.922 | 12.405 |
| GMGT-ZSGT | 66 | 0.1872 | 0.4156 | 0.2677 | 99.244 | 54.957 | 30.071 |
| YFGT-ZSGT | 147 | 0.2107 | 0.4167 | 0.2704 | 98.236 | 49.436 | 22.078 |
| Type | Baseline | Dist (km) | STD (Cycle) | ||||
|---|---|---|---|---|---|---|---|
| Optimal | HMW23 | Suboptimal | HMW12 | HMW13 | |||
| medium | ZHGT-QIAO | 20 | 0.0472 | 0.0520 | 0.1963 | 0.3112 | 0.2241 |
| GMGT-SSGT | 30 | 0.0533 | 0.0581 | 0.2099 | 0.3133 | 0.2364 | |
| SDGT-ZSGT | 32 | 0.0489 | 0.0537 | 0.2260 | 0.3445 | 0.2567 | |
| JMGT-ZSGT | 35 | 0.0513 | 0.0559 | 0.2019 | 0.3009 | 0.2272 | |
| medium-long | SSGT-SDGT | 57 | 0.0552 | 0.0647 | 0.2189 | 0.4551 | 0.3055 |
| SSGT-QYGT | 59 | 0.0531 | 0.0609 | 0.2105 | 0.4128 | 0.2698 | |
| GMGT-ZSGT | 66 | 0.0555 | 0.0667 | 0.1872 | 0.4156 | 0.2677 | |
| YFGT-FKGT | 78 | 0.0597 | 0.0660 | 0.2043 | 0.3252 | 0.2433 | |
| long | SSGT-ZHGT | 123 | 0.0587 | 0.0670 | 0.2180 | 0.4173 | 0.2737 |
| QYGT-ZSGT | 132 | 0.0573 | 0.0640 | 0.2221 | 0.3744 | 0.2662 | |
| YFGT-ZSGT | 147 | 0.0580 | 0.0666 | 0.2107 | 0.4167 | 0.2704 | |
| QYGT-FKGT | 161 | 0.0629 | 0.0697 | 0.2187 | 0.3608 | 0.2633 | |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Deng, C.; Tang, W.; Cui, J.; Shen, M.; Li, Z.; Zou, X.; Zhang, Y. Triple-Frequency Code-Phase Combination Determination: A Comparison with the Hatch-Melbourne-Wübbena Combination Using BDS Signals. Remote Sens. 2018, 10, 353. https://doi.org/10.3390/rs10020353
Deng C, Tang W, Cui J, Shen M, Li Z, Zou X, Zhang Y. Triple-Frequency Code-Phase Combination Determination: A Comparison with the Hatch-Melbourne-Wübbena Combination Using BDS Signals. Remote Sensing. 2018; 10(2):353. https://doi.org/10.3390/rs10020353
Chicago/Turabian StyleDeng, Chenlong, Weiming Tang, Jianhui Cui, Mingxing Shen, Zongnan Li, Xuan Zou, and Yongfeng Zhang. 2018. "Triple-Frequency Code-Phase Combination Determination: A Comparison with the Hatch-Melbourne-Wübbena Combination Using BDS Signals" Remote Sensing 10, no. 2: 353. https://doi.org/10.3390/rs10020353
APA StyleDeng, C., Tang, W., Cui, J., Shen, M., Li, Z., Zou, X., & Zhang, Y. (2018). Triple-Frequency Code-Phase Combination Determination: A Comparison with the Hatch-Melbourne-Wübbena Combination Using BDS Signals. Remote Sensing, 10(2), 353. https://doi.org/10.3390/rs10020353






