Using Satellite Altimetry to Calibrate the Simulation of Typhoon Seth Storm Surge off Southeast China
Abstract
:1. Introduction
2. Data and Methods
2.1. T/P Data
2.2. Tide-Gauge Data
2.3. FVCOM (3.2.1) Ocean Circulation Model
2.4. Modification of NCEP Winds
2.5. Calibration of the Stom Surge Model against Altimetry Data
3. Results
3.1. Simulated Tides
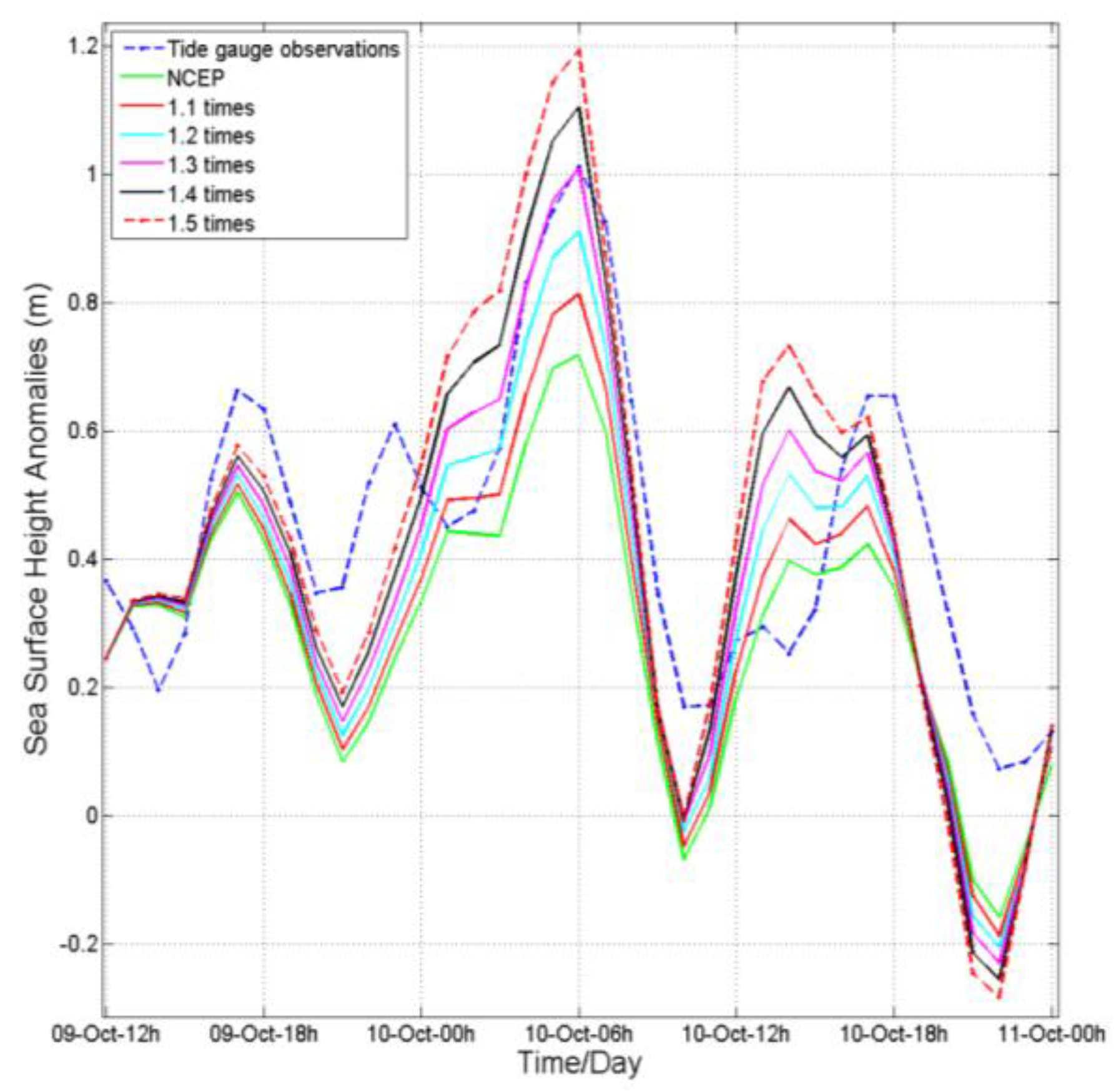
3.2. Comparison of Model Storm Surge under NCEP Winds with Tide-Gauge Data
3.3. Storm Surge from T/P Observations
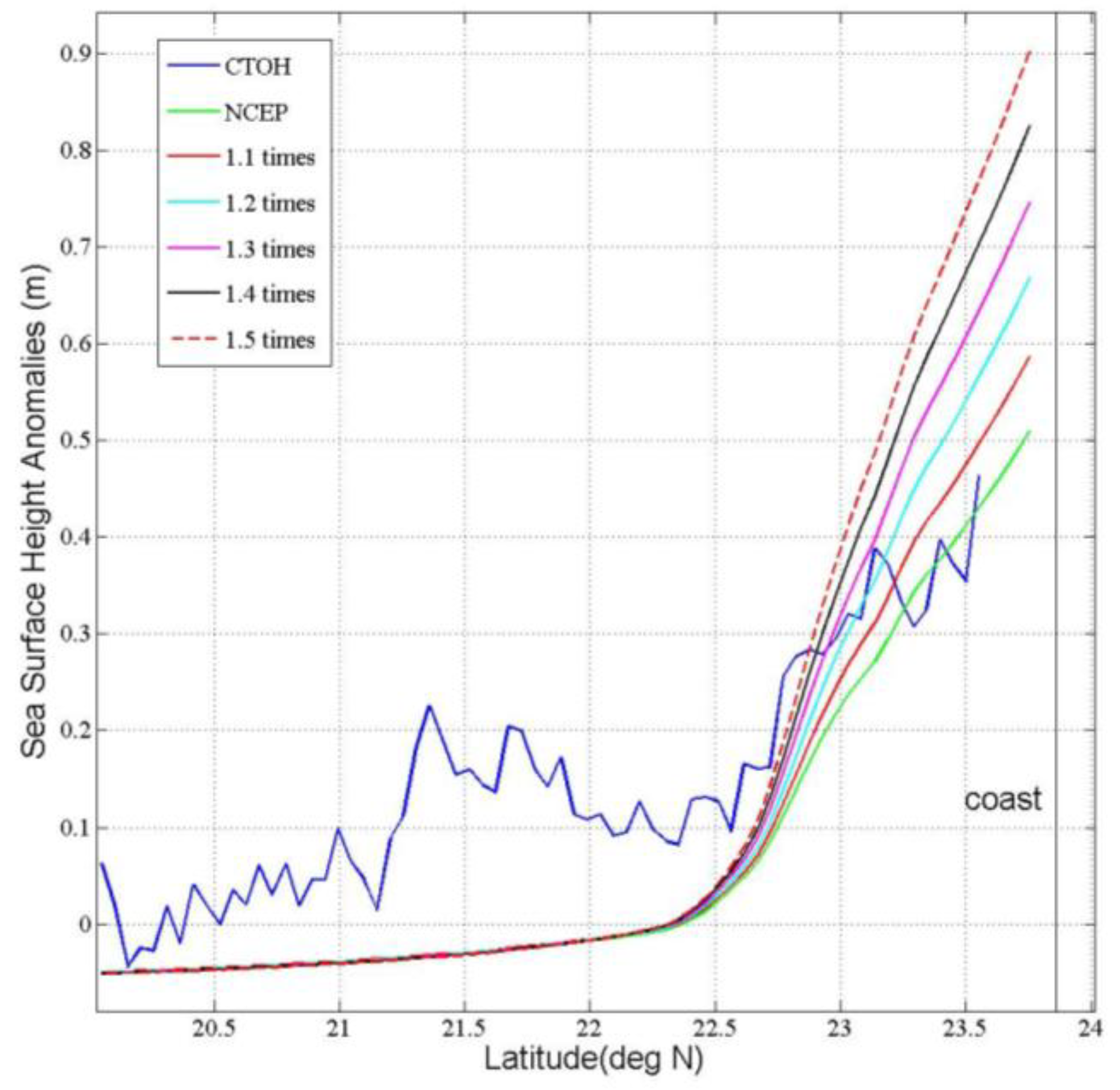
3.4. Comparison of Model Storm Surge under NCEP Winds with T/P Observations
3.5. Calibration against Altimetric Observations
4. Discussion
4.1. Effect of Tide-Surge Nonlinear Interactions
4.2. Storm Surge as a Coastally-Trapped Kelvin Wave
4.3. Strength and Limitation
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Brown, J.D.; Spencer, T.; Moeller, I. Modeling storm surge flooding of an urban area with particular reference to modeling uncertainties: A case study of Canvey Island, United Kingdom. Water Resour. Res. 2007, 43, W6402. [Google Scholar] [CrossRef]
- Price, J.F. Upper Ocean Response to a Hurricane. J. Phys. Oceanogr. 1981, 2, 153–175. [Google Scholar] [CrossRef]
- Sanford, T.B.; Black, P.G.; Haustein, J.R.; Feeney, J.W.; Forristall, G.Z. Ocean Response to a Hurricane. Part I: Observations. J. Phys. Oceanogr. 1987, 11, 2065–2083. [Google Scholar] [CrossRef]
- Han, G.; Ma, Z.; Chen, D.; Deyoung, B.; Chen, N. Observing storm surges from space: Hurricane Igor off Newfoundland. Sci. Rep. 2012, 2, 1010. [Google Scholar] [CrossRef] [PubMed]
- Shutler, J.D.; Quartly, G.D.; Donlon, C.J.; Sathyendranath, S.; Platt, T.; Chapron, B.; Johannessen, J.A.; Girard-Ardhuin, F.; Nightingale, P.D.; Woolf, D.K.; et al. Progress in satellite remote sensing for studying physical processes at the ocean surface and its borders with the atmosphere and sea ice. Prog. Phys. Geogr. 2016, 40, 215–246. [Google Scholar] [CrossRef]
- Chen, N.; Han, G.; Yang, J.; Chen, D. Hurricane Sandy storm surges observed by HY-2A satellite altimetry and tide-gauges. J. Geophys. Res. Oceans 2014, 119, 4542–4548. [Google Scholar] [CrossRef]
- Scharroo, R.; Smith, W.; Lillibridge, J.L. Satellite altimetry and the intensification of Hurricane Katrina. Eos Trans. Am. Geophys. Union 2005, 40, 366. [Google Scholar] [CrossRef]
- Lillibridge, J.; Lin, M.; Shum, C.K. Hurricane Sandy Storm Surge Measured by Satellite Altimetry. Oceanography 2013, 2, 8–9. [Google Scholar] [CrossRef]
- Fenoglio-Marc, L.; Scharroo, R.; Annunziato, A.; Mendoza, L.; Becker, M. Cyclone Xaver seen by geodetic observations. Geophys. Res. Lett. 2015, 22, 9925–9932. [Google Scholar] [CrossRef]
- Han, G.; Ma, Z.; Chen, N.; Chen, N.; Yang, J. Hurricane Isaac storm surges off Florida observed by Jason-1 and Jason-2 satellite altimeters. Remote Sens. Environ. 2017, 198, 244–253. [Google Scholar] [CrossRef]
- De Biasio, F.; Bajo, M.; Vignudelli, S.; Umgiesser, G.; Zecchetto, S. Improvements of storm surge forecasting in the Gulf of Venice exploiting the potential of satellite data: The ESA DUE eSurge-Venice project. Eur. J. Remote Sens. 2017, 50, 428–441. [Google Scholar] [CrossRef]
- Bajo, M.; De Biasio, F.; Umgiesser, G.; Vignudelli, S.; Zecchetto, S. Impact of using scatterometer and altimeter data on storm surge forecasting. Ocean Model. 2017, 113, 85–94. [Google Scholar] [CrossRef]
- De Biasio, F.; Vignudelli, S.; Della Valle, A.; Umgiesser, G.; Bajo, M.; Zecchetto, S. Exploiting the Potential of Satellite Microwave Remote Sensing to Hindcast the Storm Surge in the Gulf of Venice. IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2016, 9, 5089–5105. [Google Scholar] [CrossRef]
- Ye, A.; Chen, Z.; Yu, Y. Numerical inverstigation of three-dimensional semidiurnal tidal waves in Taiwan Strait and its adjacent areas. Oceanol. Limnol. Sin. 1985, 6, 439–450. [Google Scholar]
- Li, Y.; Cai, W.; Li, L.; Lin, M. The tide characteristics of the seas adjacent to Fujian and Taiwan derived from TOPEX/POSEIDON altimeter data. Acta Oceanol. Sin. 2002, 24, 154–162. [Google Scholar]
- Zhang, W.Z.; Hong, H.S.; Shang, S.P.; Chen, D.W.; Chai, F. A two-way nested coupled tide-surge model for the Taiwan Strait. Cont. Shelf Res. 2007, 27, 1548–1567. [Google Scholar] [CrossRef]
- Zhang, W.Z.; Shi, F.; Hong, H.S.; Shang, S.P.; Kirby, J.T. Tide-surge Interaction Intensified by the Taiwan Strait. J. Geophys. Res. 2010. [Google Scholar] [CrossRef]
- Prandle, D.; Wolf, J. Surge-Tide Interaction in the Southern North Sea. In Elsevier Oceanography Series; Elsevier: New York, NY, USA, 1978; pp. 161–185. [Google Scholar]
- Zhen, W.; Chen, F.; Chen, X. Tides and tidal currents in the Taiwan Strait. J. Oceanogr. Taiwan Strait 1982, 2, 1–4. [Google Scholar]
- Pan, H.; Liu, F. A numerical study of the tide-surge interaction in the East China Sea and the South China Sea. Chin. J. Oceanol. Limnol. 1994, 1, 13–21. [Google Scholar]
- Chen, C.; Liu, H.; Beardsley, R.C. An Unstructured Grid, Finite-Volume, Three-Dimensional, Primitive Equations Ocean Model: Application to Coastal Ocean and Estuaries. J. Atmos. Ocean. Technol. 2003, 20, 159–186. [Google Scholar] [CrossRef]
- Birol, F.; Fuller, N.; Lyard, F.; Cancet, M.; Nino, F.; Delebecque, C.; Fleury, S.; Toublanc, F.; Melet, A.; Saraceno, M.; et al. Coastal Applications from Nadir Altimetry: Example of the X-TRACK Regional Products. Adv. Space Res. 2016. [Google Scholar] [CrossRef]
- Pawlowicz, R.; Beardsley, B.; Lentz, S. Classical tidal harmonic analysis including error estimates in MATLAB using T_TIDE. Comput. Geosci. 2002, 28, 929–937. [Google Scholar] [CrossRef]
- Amante, C.; Eakins, B.W. ETOPO1 1 Arc-Minute Global Relief Model_Procedures, Data Sources and Analysis. NOAA Tech. Memo. NESDIS NGDC-24 2008. [Google Scholar] [CrossRef]
- Egbert, G.D.; Erofeeva, S.Y. Efficient Inverse Modeling of Barotropic Ocean Tides. J. Atmos. Ocean. Technol. 2002, 19, 183–204. [Google Scholar] [CrossRef]
- Weisberg, R.H.; Zheng, L. Circulation of Tampa Bay driven by buoyancy, tides and winds, as simulated using a Finite Volume Coastal Ocean Model. J. Geophys. Res. 2008, 111, C1005. [Google Scholar] [CrossRef]
- Ma, Z.; Han, G.; de Young, B. Oceanic responses to hurricane Igor over the Grand Banks: A modelling study. J. Geophys. Res. Oceans 2015, 120. [Google Scholar] [CrossRef]
- Su, J.; Yuan, Y. (Eds.) Hydrography in the Chinese Seas; Ocean Press: Beijing, China, 2005; p. 367. (In Chinese) [Google Scholar]
- Rego, J.O.L.; Li, C. Nonlinear terms in storm surge predictions: Effect of tide and shelf geometry with case study from Hurricane Rita. J. Geophys. Res. 2010, 115. [Google Scholar] [CrossRef]
- Tang, C.L.; Gui, Q.; De Tracey, B.M. Barotropic response of the Labrador/Newfoundland Shelf to a moving storm. J. Phys. Oceanogr. 1998, 17, 1152–1172. [Google Scholar] [CrossRef]
- Thiebaut, S.; Vennell, R. Observation of a fast continental shelf wave generated by a storm impacting Newfoundland using wavelet and cross-wavelet analyses. J. Phys. Oceanogr. 2010, 40, 417–428. [Google Scholar] [CrossRef]
- Brink, K.H.; Chapman, D.C. Programs for Computing Properties of Coastal-Trapped Waves and Wind-Driven Motions over the Continental Shelf and Slope; WHOI Tech. Rep. WHOI-87-24; Woods Hole Oceanographic Institution: Woods Hole, MA, USA, 1987; 119p. [Google Scholar]










| Tide | Simulated Amplitude | Observed Amplitude | Simulated Phase | Observed Phase |
|---|---|---|---|---|
| Q1 | 0.06 | 0.05 | 120 | 125 |
| O1 | 0.30 | 0.28 | 128 | 140 |
| P1 | 0.11 | 0.11 | 162 | 172 |
| K1 | 0.35 | 0.34 | 163 | 175 |
| N2 | 0.30 | 0.37 | 112 | 129 |
| M2 | 1.67 | 1.85 | 132 | 148 |
| S2 | 0.52 | 0.54 | 169 | 194 |
| K2 | 0.14 | 0.15 | 156 | 192 |
| MN4 | 0.03 | 0.03 | 266 | 289 |
| M4 | 0.09 | 0.08 | 282 | 309 |
| MS4 | 0.06 | 0.05 | 319 | 353 |
| RMS (22.3 to 23.55°N) | |
|---|---|
| NCEP | 0.15 m |
| modified by 1.1 | 0.13 m |
| modified by 1.2 | 0.11 m |
| modified by 1.3 | 0.10 m |
| modified by 1.4 | 0.11 m |
| modified by 1.5 | 0.12 m |
| 9 October, T12 | 9 October, T18 | 10 October, T00 | 10 October, T06 | 10 October, T12 | 10 October, T18 | 11 October, T00 | |
|---|---|---|---|---|---|---|---|
| NCEP | 33 | 31 | 29 | 30 | 27 | 22 | 22 |
| Modified by 1.1 | 36 | 34 | 32 | 33 | 30 | 24 | 25 |
| Modified by 1.2 | 40 | 38 | 34 | 36 | 32 | 27 | 27 |
| Modified by 1.3 | 43 | 41 | 37 | 39 | 35 | 29 | 29 |
| Modified by 1.4 | 46 | 44 | 40 | 42 | 38 | 31 | 31 |
| Modified by 1.5 | 49 | 47 | 43 | 45 | 40 | 33 | 33 |
| best-track | 45 | 40 | 40 | 40 | 35 | 30 | 30 |
| ntimes | NCEP | 1.1 | 1.2 | 1.3 | 1.4 | 1.5 |
|---|---|---|---|---|---|---|
| RMS | 9.6 | 6.7 | 3.9 | 1.6 | 2.1 | 4.5 |
| ntimes | 9 October, T12 | 9 October, T18 | 10 October, T00 | 10 October, T06 | 10 October, T12 | 10 October, T18 | 11 October, T00 | Mean |
|---|---|---|---|---|---|---|---|---|
| NCEP | 8.9 | 13.1 | 15.0 | 19.1 | 13.6 | 14.9 | 11.9 | 13.8 |
| 1.1 | 8.9 | 13.3 | 15.9 | 20.5 | 14.8 | 16.3 | 12.8 | 14.6 |
| 1.2 | 8.9 | 13.3 | 16.7 | 21.9 | 15.9 | 17.7 | 13.7 | 15.4 |
| 1.3 | 8.9 | 13.5 | 17.5 | 23.4 | 17.1 | 19.1 | 14.6 | 16.3 |
| 1.4 | 8.9 | 13.7 | 18.3 | 24.9 | 18.2 | 20.6 | 15.5 | 17.2 |
| 1.5 | 8.9 | 13.8 | 19.1 | 26.3 | 19.4 | 21.9 | 16.4 | 18.0 |
| Dachen | 9.3 | 12.0 | 14.0 | 22 | 25.0 | 22 | 12 | 16.6 |
| RMS | |
|---|---|
| NCEP | 0.21 m |
| modified by 1.1 | 0.19 m |
| modified by 1.2 | 0.18 m |
| modified by 1.3 | 0.18 m |
| modified by 1.4 | 0.19 m |
| modified by 1.5 | 0.21 m |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, X.; Han, G.; Yang, J.; Chen, D.; Zheng, G.; Chen, N. Using Satellite Altimetry to Calibrate the Simulation of Typhoon Seth Storm Surge off Southeast China. Remote Sens. 2018, 10, 657. https://doi.org/10.3390/rs10040657
Li X, Han G, Yang J, Chen D, Zheng G, Chen N. Using Satellite Altimetry to Calibrate the Simulation of Typhoon Seth Storm Surge off Southeast China. Remote Sensing. 2018; 10(4):657. https://doi.org/10.3390/rs10040657
Chicago/Turabian StyleLi, Xiaohui, Guoqi Han, Jingsong Yang, Dake Chen, Gang Zheng, and Nan Chen. 2018. "Using Satellite Altimetry to Calibrate the Simulation of Typhoon Seth Storm Surge off Southeast China" Remote Sensing 10, no. 4: 657. https://doi.org/10.3390/rs10040657
APA StyleLi, X., Han, G., Yang, J., Chen, D., Zheng, G., & Chen, N. (2018). Using Satellite Altimetry to Calibrate the Simulation of Typhoon Seth Storm Surge off Southeast China. Remote Sensing, 10(4), 657. https://doi.org/10.3390/rs10040657






