Design of Tracking, Telemetry, Command (TT&C) and Data Transmission Integrated Signal in TDD Mode
Abstract
:1. Introduction
- (1)
- High spectrum utilization;
- (2)
- Small amplitude fluctuation which can effectively resist nonlinear distortion;
- (3)
- The constellation is circular, which requires less power;
- (4)
- It is easy to realize variable rate modulation and meet the hierarchical transmission requirements of satellite communication.
- (1)
- Considering the lack of spectrum resources, this paper proposes the BOCcos and APSK integrated signal to achieve TT&C and data transmission services.
- (2)
- Considering the shortage of frequency resources in the case of large numbers of nodes, we propose the high precision measurement method for the TDD mode.
2. The Modulation System for the BOCcos and APSK Integrated Signal
2.1. Signal Mode
2.2. The Concept of Modulation and Demodulation
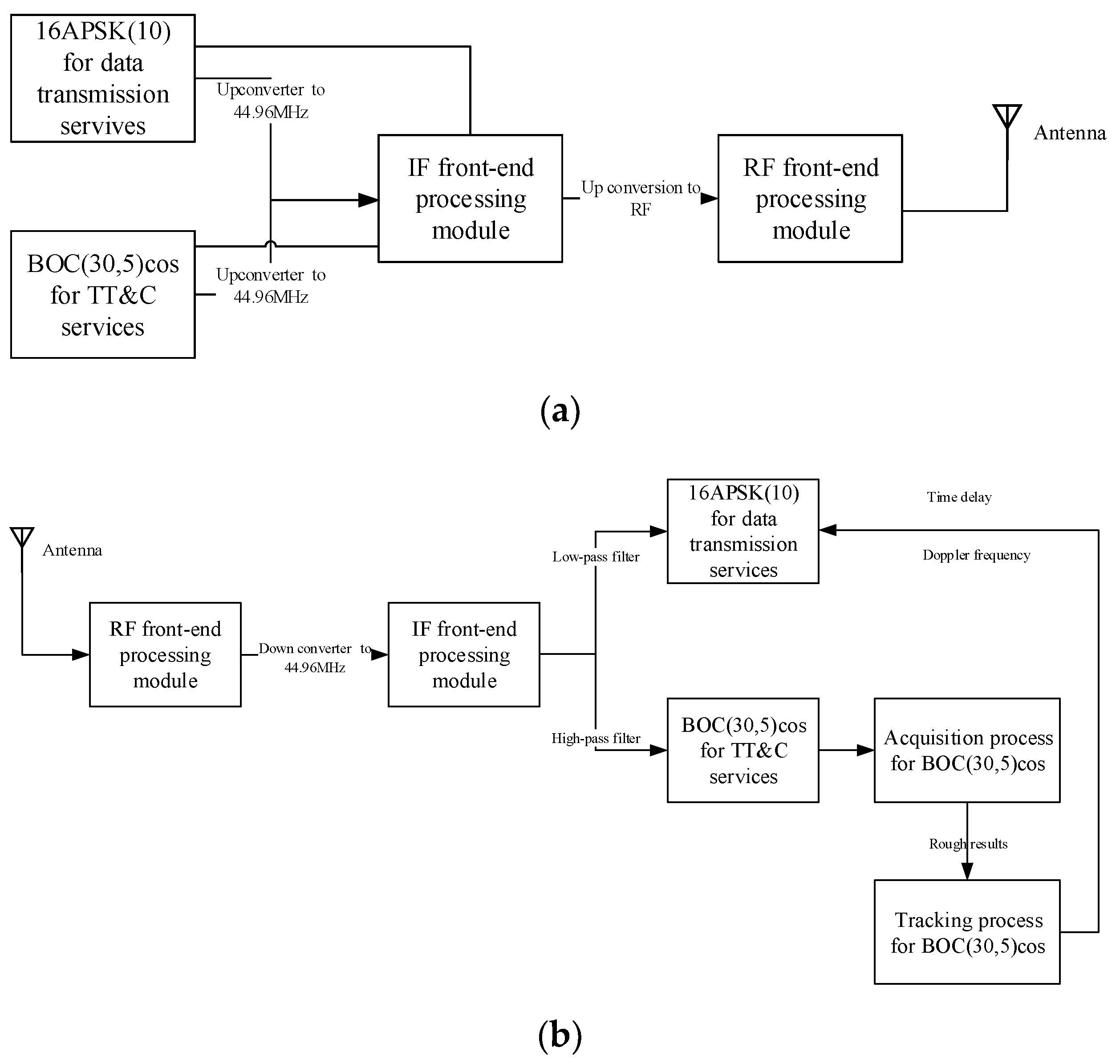
3. The Receiving Method of the Integrated Signal in the TDD Mode
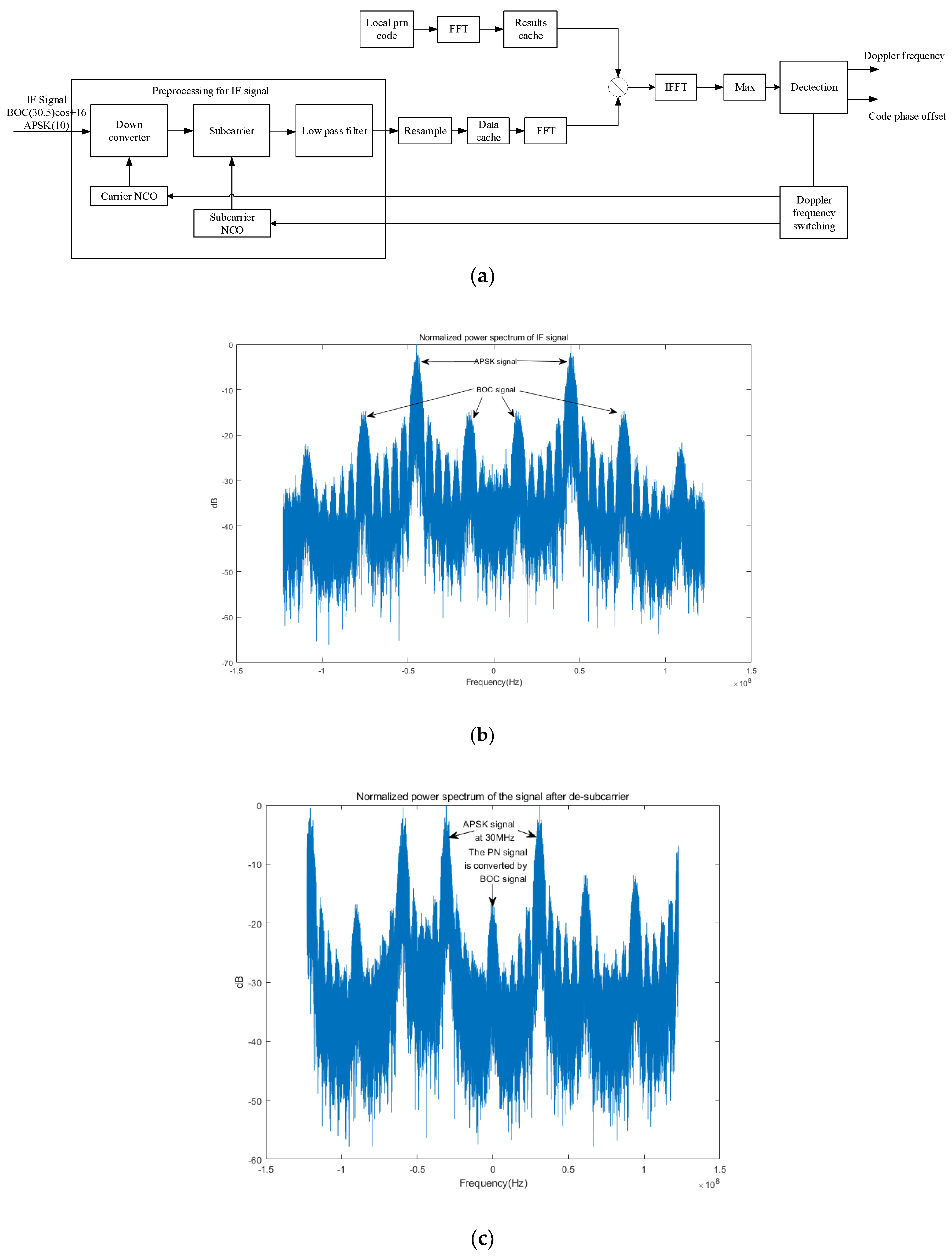
3.1. The Acquisition Process of Integrated Signal
3.2. Motion Compensation Method in TDD Mode
4. Performance Analysis of the TT&C Signal and Data Transmission Signal
4.1. The Design of the Simulation Scenario
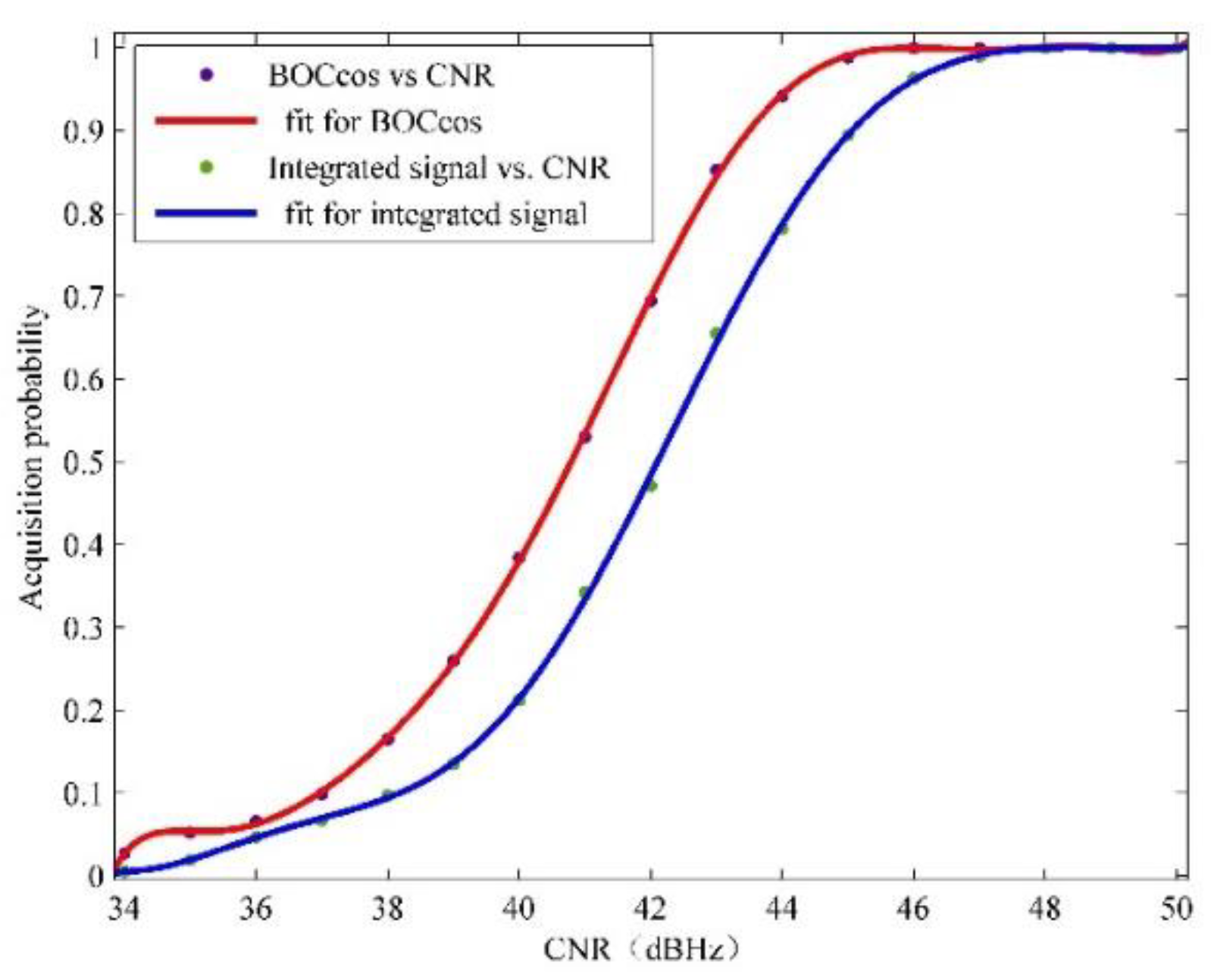
4.2. Acquisition Performance of the TT&C Signal
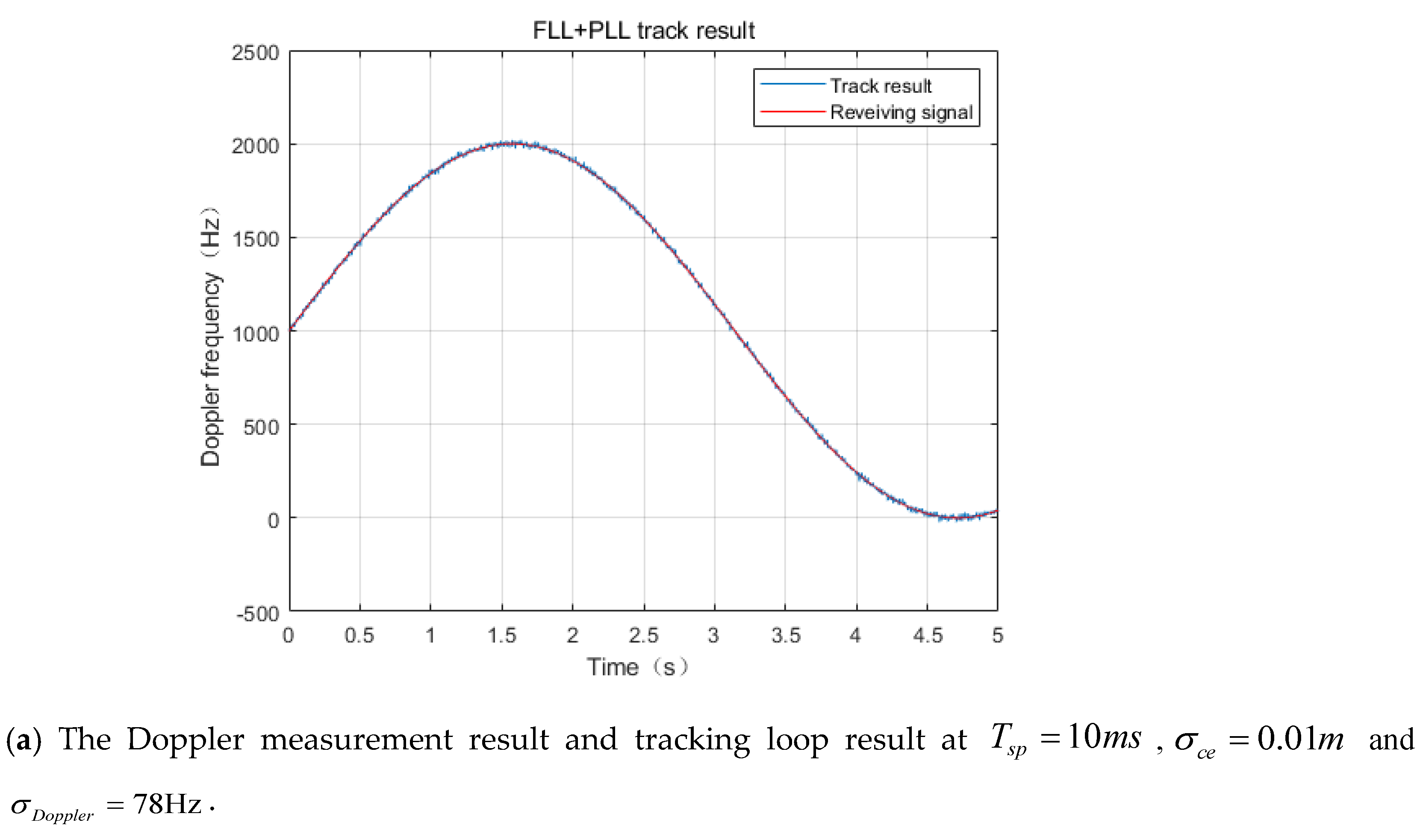
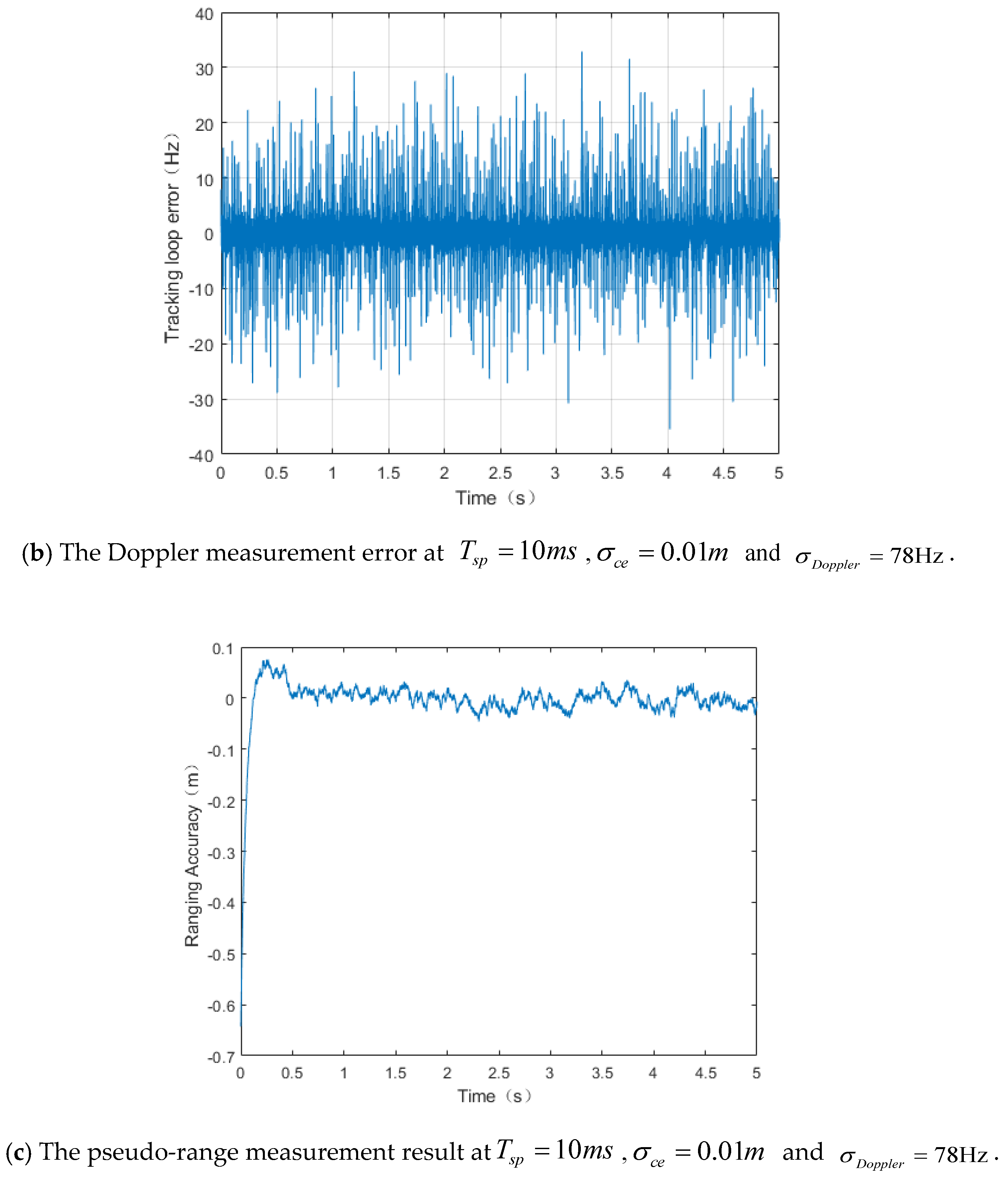
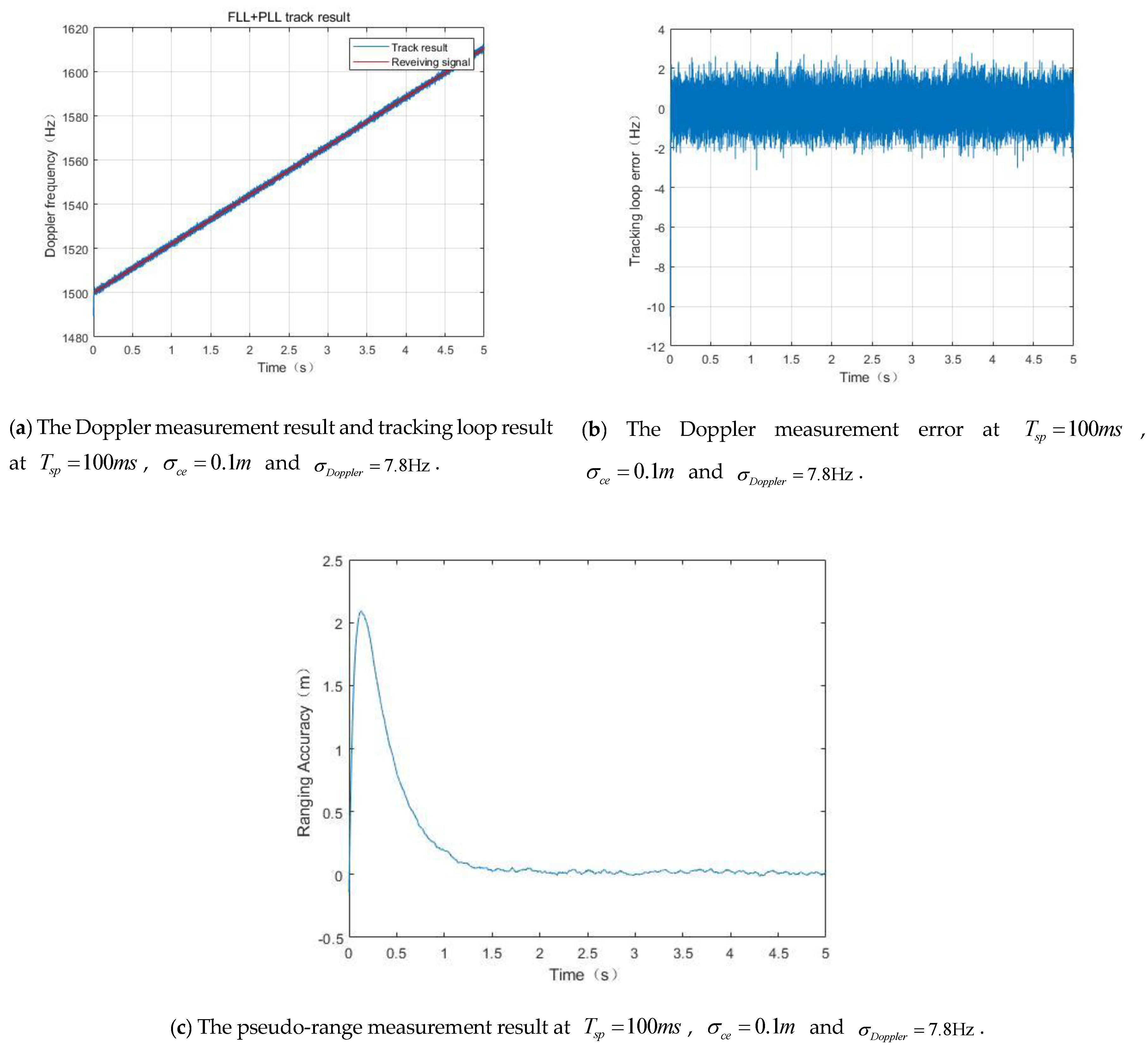
4.3. Tracking Performance for the TT&C Signal
5. Discussion
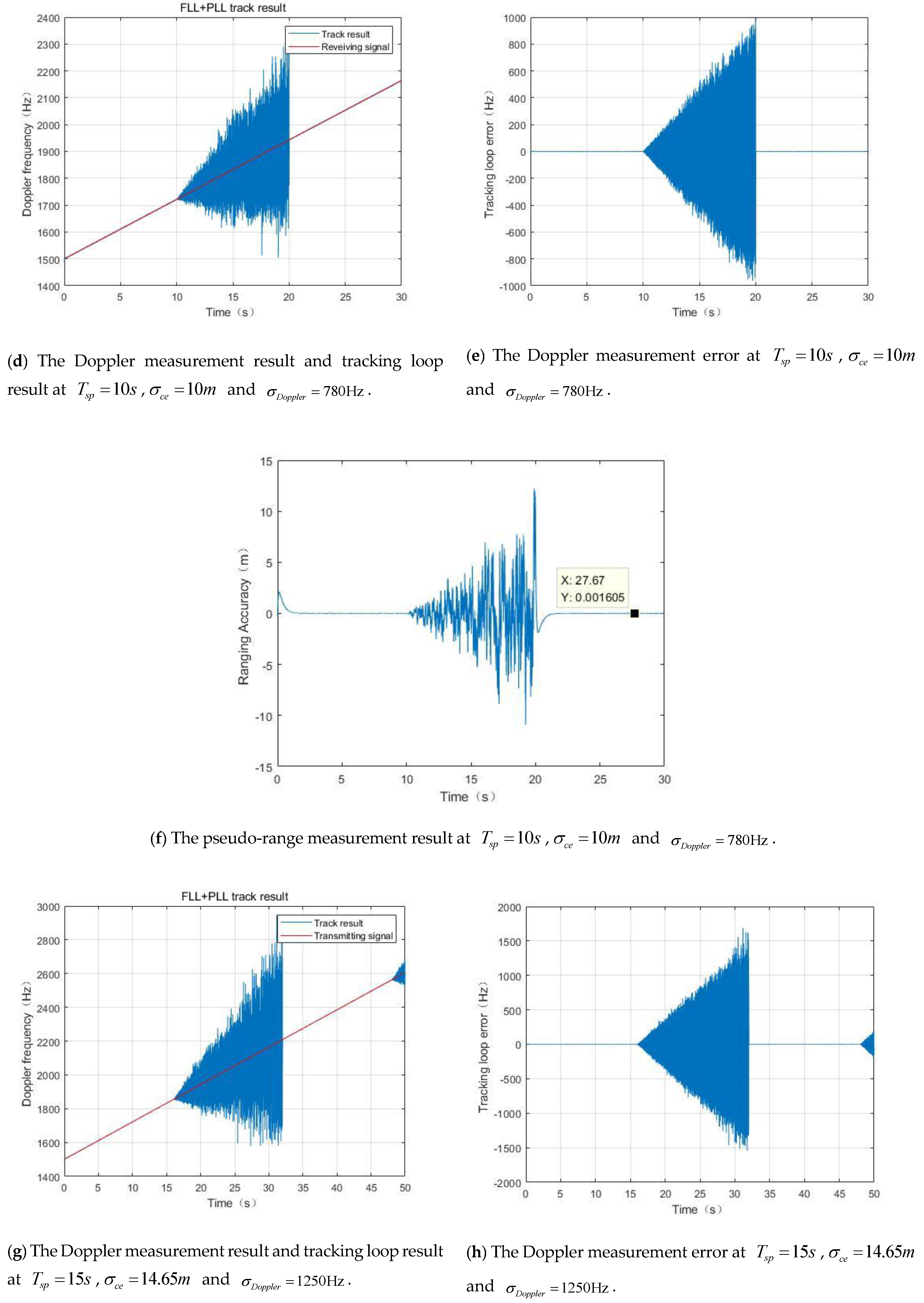
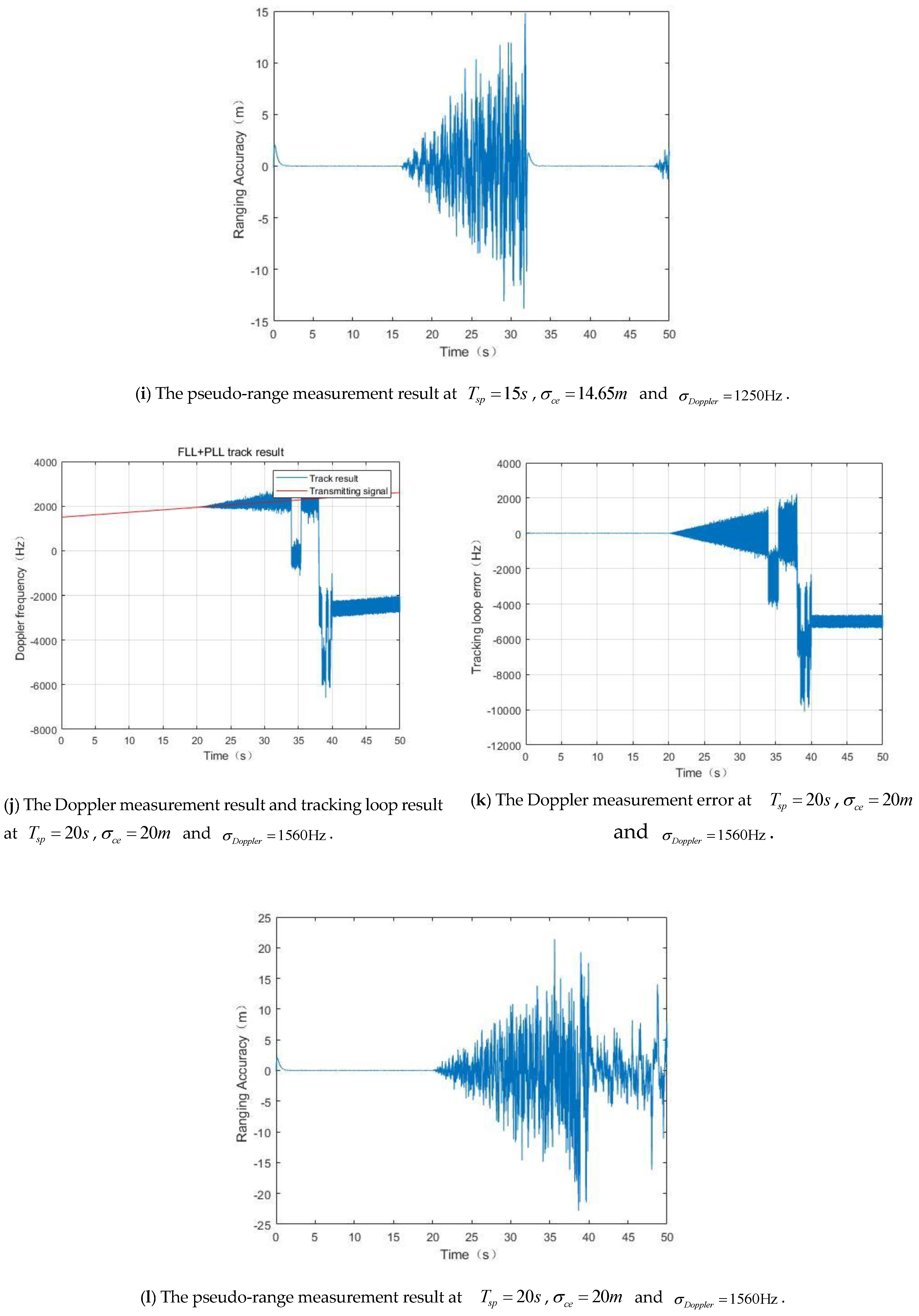
5.1. Ranging and Doppler Measurement Performance for Integrated Signals
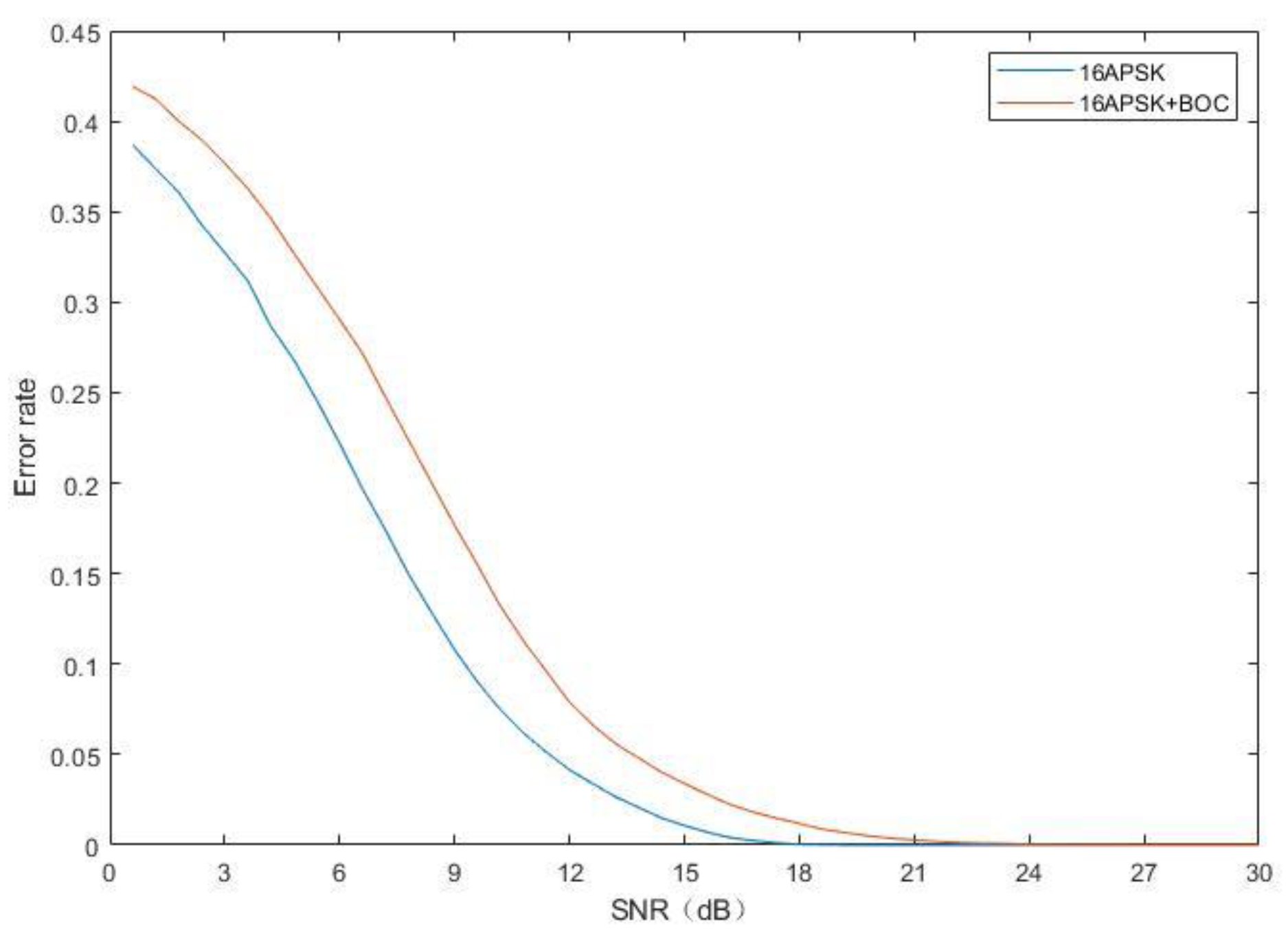
5.2. Bit Error Rate (BER) for Integrated Signals
5.3. Summary of Error Suppression Methods
- (1)
- Improve the PN code rate, which will reduce .
- (2)
- Increase the length of the PN code. The method can increase the integration period.
- (3)
- Increase the integration period.
- (4)
- Reduce the bandwidth of the loop. However, the stability of the loop will be weakened, so the error and SNR in the extrapolation process should be considered comprehensively.
- (5)
- Reduce the switching period of the TDD mode. This method can effectively reduce the extrapolation errors.
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A
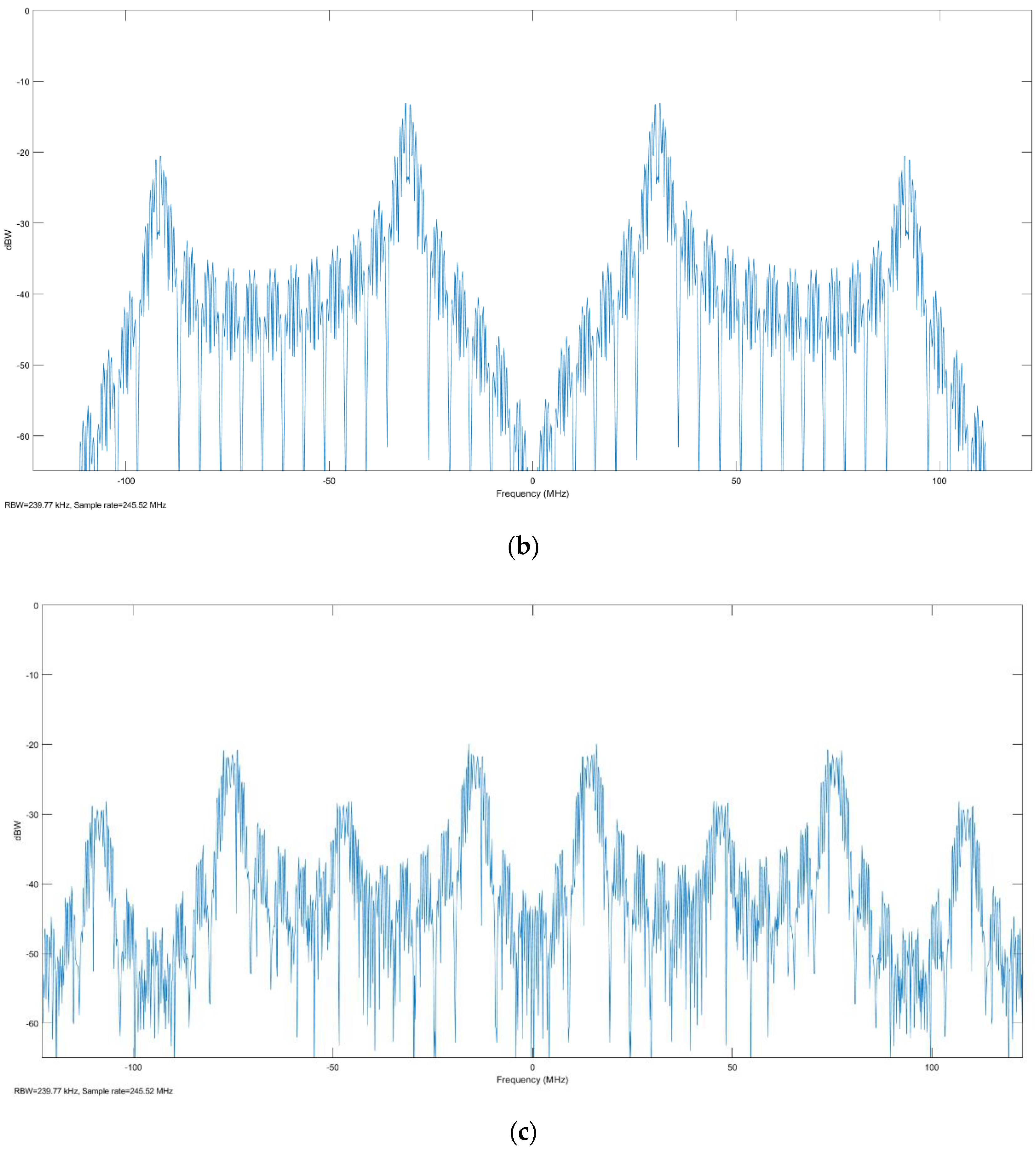
References
- Crisan, A.-M.; Martian, A.; Cacoveanu, R.; Coltuc, D. Evaluation of synchronization techniques for inter-satellite links. In Proceedings of the 2016 International Conference on Communications (COMM), Bucharest, Romania, 9–10 June 2016; pp. 463–468. [Google Scholar]
- Wu, W.R.; Dong, G.L.; Li, H.T. Engineering and Technology of Deep Space TT&C System; China Science Publishing & Media Ltd.: Beijing, China, 2013; pp. 143–194. [Google Scholar]
- Wu, W.; Li, H.; Li, Z.; Wang, G.; Tang, Y. Status and prospect of China’s deep space TT&C network. Sci. Sin. Inf. 2020, 50, 87–127. [Google Scholar] [CrossRef]
- Veres, P.M.; Gabányi, K.É.; Frey, S. Very Long Baseline Interferometry Observations of the Proposed Radio Counterpart of an EGRET Source. Symmetry 2020, 12, 1516. [Google Scholar] [CrossRef]
- Kinman, P. Doppler tracking of planetary spacecraft. IEEE Trans. Microw. Theor. Tech. 1992, 40, 1199–1204. [Google Scholar] [CrossRef]
- Del Portillo, I.; Cameron, B.G.; Crawley, E.F. A technical comparison of three low earth orbit satellite constellation systems to provide global broadband. Acta Astronaut. 2019, 159, 123–135. [Google Scholar] [CrossRef]
- Foreman, V.L.; Siddiqi, A.; De Weck, O.L. Large Satellite Constellation Orbital Debris Impacts: Case Studies of OneWeb and SpaceX Proposals. AIAA SPACE Astronaut. Forum Expos. 2017, 5200. [Google Scholar] [CrossRef]
- Li, Y.; Wang, Z.; Tan, W. The frequency spectrum management for aerospace TT&C system. In Proceedings of the 2013 5th IEEE International Symposium on Microwave, Antenna, Propagation and EMC Technologies for Wireless Communications, Chengdu, China, 29–31 October 2013; pp. 595–600. [Google Scholar] [CrossRef]
- Gu, X.; Bai, J.; Zhang, C.; Gao, H. Study on TT&C resources scheduling technique based on inter-satellite link. Acta Astronaut. 2014, 104, 26–32. [Google Scholar] [CrossRef]
- Tianjiao, Z.; Jing, L.; Zexi, L.; Ming, X. An Algorithm Research of Ground-Space Integrated Scheduling TT&C Resources of Orbit Determination of GNSS Constellation. In Proceedings of the 2013 Fourth International Conference on Intelligent Systems Design and Engineering Applications, Zhangjiajie, China, 6–7 November 2013; pp. 69–73. [Google Scholar]
- Yan, L. The Design and Implement of TT&C and Data Transmission Integrated System of Satellite; Nanjing University of Science & Technology: Nanjing, China, 2018. [Google Scholar]
- Abdullah, A.; Elnoubi, S.; Banna, M.; Nasr, M. Implementation of multi-user detection for ds-cdma communications. In Proceedings of the Twenty-Second National Radio Science Conference, NRSC 2005, Cairo, Egypt, 15–17 March 2005; pp. 403–410. [Google Scholar]
- Jovic, B.; Unsworth, C.; Sandhu, G.; Berber, S.M. A robust sequence synchronization unit for multi-user DS-CDMA chaos-based communication systems. Signal Process. 2007, 87, 1692–1708. [Google Scholar] [CrossRef]
- Nie, S.J.; He, B.Z.; Wang, H.Z.; Qin, Y.F. Design of integration channel of ranging and data transmission. Mod. Electron. Tech. 2013, 36, 57–62. [Google Scholar]
- Chen, S.; Sun, S.; Wang, Y.; Xiao, G.; Tamrakar, R. A comprehensive survey of TDD-based mobile communication systems from TD-SCDMA 3G to TD-LTE(A) 4G and 5G directions. China Commun. 2015, 12, 40–60. [Google Scholar] [CrossRef]
- Aslam, M.; Jiao, X.; Liu, W.; Moerman, I. An Approach to Achieve Zero Turnaround Time in TDD Operation on SDR Front-End. IEEE Access 2018, 6, 75461–75470. [Google Scholar] [CrossRef]
- Betz, J.W. Binary offset carrier modulation for radio navigation. Navigation 2001, 48, 227–246. [Google Scholar] [CrossRef]
- Wang, H.-Y.; Juang, J.-C. Retrieval of Ocean Surface Wind Speed Using Reflected BPSK/BOC Signals. Remote Sens. 2020, 12, 2698. [Google Scholar] [CrossRef]
- Pickholtz, R.; Schilling, D.; Milstein, L. Theory of Spread-Spectrum Communications—A Tutorial. IEEE Trans. Commun. 1982, 30, 855–884. [Google Scholar] [CrossRef] [Green Version]
- Rong, L.; Yingfeng, X. A multi-aircraft cooperative UAV observe and control system. J. Terahertz Sci. Electron. Inf. Technol. 2016, 14, 706–711. [Google Scholar]
- Liuqing, Y.; Qiangui, X. A UAV TT&C System for One Station Controlling Several Vehicles. Electron. Optics Control 2013, 20, 6–15. [Google Scholar]
- Guo, S.; Huang, G.; Liu, B.; Gao, Y. Research of MAPSK modulation based on the nonlinear satellite channel. In Proceedings of the 2010 3rd IEEE International Conference on Broadband Network and Multimedia Technology (IC-BNMT), Beijing, China, 26–28 October 2010; pp. 1197–1201. [Google Scholar]
- Manqian, Z.; Jian, L.; Bo, Y.; Guangnan, Z. Research on high order modulation for satellite communications. Electronic. Des. Eng. 2014, 22, 114–117. [Google Scholar]
- Zhang, J.; Zhu, L.; Guo, Y.; Gou, X. A new method of demodulation for 16APSK/32APSK. In Proceedings of the Proceedings of 2012 5th Global Symposium on Millimeter-Waves, Harbin, China, 27–30 May 2012; pp. 477–481. [Google Scholar]
- Saxena, T.; Jadon, J.S. Spectral analysis of Sine and Cosine BOC modulated signals. In Proceedings of the 2014 International Conference on Signal Processing and Integrated Networks (SPIN), Noida, India, 20–21 February 2014; pp. 734–738. [Google Scholar]
- Lohan, E.S.; Lakhzouri, A.; Renfors, M. Binary-offset-carrier modulation techniques with applications in satellite navigation systems. Wirel. Commun. Mob. Comput. 2007, 7, 767–779. [Google Scholar] [CrossRef]
- Machida, M.; Handa, S.; Oshita, S. Theoretical analysis of symbol error rates of (4,12) circular-signal-set constellation for some detection schemes. Electron. Commun. Jpn. Part I Commun. 2000, 83, 19–28. [Google Scholar] [CrossRef]
- Bhuiyan, M.Z.H.; Söderholm, S.; Thombre, S.; Ruotsalainen, L.; Kuusniemi, H. Implementation of a Software-Defined BeiDou Receiver. In Proceedings of the China Satellite Navigation Conference (CSNC) 2014 Proceedings, Nanjing, China, 21–23 May 2014; Springer: Berlin, Germany, 2014; Volume 303, pp. 751–762. [Google Scholar]
- Meng, S.; Yang, W.; Lu, W.; Liu, J.; Yu, J. Code tracking performance of DS/FH spread spectrum signal for TT&C. In Proceedings of the 2010 2nd IEEE International Conference on Information Management and Engineering, Chengdu, China, 16–18 April 2010; pp. 491–495. [Google Scholar]
- Yang, W.; Meng, S.; Wang, J.; Liu, J. Acquisition performance analysis of a synchronization scheme of DS/FH hybrid spread spectrum signals for TT&C. In Proceedings of the IEEE 2011 10th International Conference on Electronic Measurement & Instruments, Beijing, China, 16–19 August 2009; pp. 4–395. [Google Scholar]
- Wen, L.; Yue, X.; Zhongliang, D.; Jichao, J.; Lu, Y. Correlation combination ambiguity removing technology for acquisition of sine-phased BOC(kn,n) signals. China Commun. 2015, 12, 86–96. [Google Scholar] [CrossRef]
- Burian, A.; Lohan, E.S.; Renfors, M. BPSK-like Methods for Hybrid-Search Acquisition of Galileo Signals. In Proceedings of the 2006 IEEE International Conference on Communications, Istanbul, Turkey, 11–15 June 2006; Volume 11, pp. 5211–5216. [Google Scholar]
- Xie, G. Principles of GPS and Receiver Design; Publishing House of Electronics Industry: Beijing, China, 2009; pp. 349–393. [Google Scholar]
- Benedetto, F.; Giunta, G.; Lohan, E.S.; Renfors, M. A Fast Unambiguous Acquisition Algorithm for BOC-Modulated Signals. IEEE Trans. Veh. Technol. 2012, 62, 1350–1355. [Google Scholar] [CrossRef]
- Wang, C.; Zhou, M. Novel Approach to Intersatellite Distance Measurement with High Accuracy. J. Guid. Control. Dyn. 2015, 38, 944–949. [Google Scholar] [CrossRef]
- Kuang, K.; Zhang, S.; Li, J. Real-time GPS satellite orbit and clock estimation based on OpenMP. Adv. Space Res. 2019, 63, 2378–2386. [Google Scholar] [CrossRef]
- Maciuk, K.; Lewińska, P. High-Rate Monitoring of Satellite Clocks Using Two Methods of Averaging Time. Remote Sens. 2019, 11, 2754. [Google Scholar] [CrossRef] [Green Version]
- El-Mowafy, A. Impact of predicting real-time clock corrections during their outages on precise point positioning. Surv. Rev. 2017, 51, 183–192. [Google Scholar] [CrossRef]
- Liu, G.; Guo, F.; Wang, J.; Du, M.; Qu, L. Triple-Frequency GPS Un-Differenced and Uncombined PPP Ambiguity Resolution Using Observable-Specific Satellite Signal Biases. Remote Sens. 2020, 12, 2310. [Google Scholar] [CrossRef]
- Jia, X.; Zeng, T.; Ruan, R.; Mao, Y.; Xiao, G. Atomic Clock Performance Assessment of BeiDou-3 Basic System with the Noise Analysis of Orbit Determination and Time Synchronization. Remote Sens. 2019, 11, 2895. [Google Scholar] [CrossRef] [Green Version]
- Xiangqiang, M. Research and Application of Satellite Orbit Prediction Method Based on osculationg Kepler Element; University of Chinese Academy of Sciences: Beijing, China, 2017. [Google Scholar]
- Wenbing, W.; Rongfang, L. A new method of orbit prediction for LEO satellites using empirical accelerations. Chin. J. Space Sci. 2015, 35, 715–720. [Google Scholar]
- Liu, X.; Wang, D.; Zhong, X.; Meng, Y. Research on the Inversion Method of USO Frequency Stability Joining GNSS and Inter-satellite Distance Measurement. In Proceedings of the China Satellite Navigation Conference (CSNC) 2016 Proceedings, Changsha, China, 18–20 May 2016; Springer: Berlin, Germany, 2016; Volume 390, pp. 3–13. [Google Scholar]
- Hao, F.; Yu, B.; Gan, X.; Jia, R.; Zhang, H.; Huang, L.; Wang, B. Unambiguous Acquisition/Tracking Technique Based on Sub-Correlation Functions for GNSS Sine-BOC Signals. Sensors 2020, 20, 485. [Google Scholar] [CrossRef] [Green Version]
- Kaplan, E. Understanding GPS:Principles and Applications, 2nd ed.; Artech House: Boston, MA, USA, 2006; pp. 153–240. [Google Scholar]
- Parkinson, B.W.; Enge, P.; Axelrad, P.; Spilker, J.J., Jr. Global Positioning System: Theory and Applications; American Institute of Aeronautics and Astronautics (AIAA): Washington, DC, USA, 1996; pp. 409–433. [Google Scholar]
- Divsalar, D.; Net, M.S.; Cheung, K.-M. Acquisition and tracking for communications between Lunar South Pole and Earth. In Proceedings of the 2019 IEEE Aerospace Conference, Big Sky, MT, USA, 2–9 March 2019; pp. 1–14. [Google Scholar]




| Satellite number | 3364 |
| Orbit inclination | |
| Orbit altitude | 508 km |
| Orbital plane | 58 |
| The satellites in each plane | 58 |
| Number | 3364 |
| Process | Clock Cycle |
|---|---|
| Downsampling | 4096 |
| FFT of the downsampled signal | 409612 |
| Complex multiplication between the local PN code and downsampled signal | 4096 |
| IFFT of the multiplication result | 409612 |
| Maximum searches | 4096 |
| Threshold calculation | 1280 |
| Total | 111,872 |
| Threshold | Whether the Tracking Process Requires Reacquisition |
|---|---|
| And | No |
| Or | Yes |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xue, L.; Li, X.; Wu, W.; Yang, Y. Design of Tracking, Telemetry, Command (TT&C) and Data Transmission Integrated Signal in TDD Mode. Remote Sens. 2020, 12, 3340. https://doi.org/10.3390/rs12203340
Xue L, Li X, Wu W, Yang Y. Design of Tracking, Telemetry, Command (TT&C) and Data Transmission Integrated Signal in TDD Mode. Remote Sensing. 2020; 12(20):3340. https://doi.org/10.3390/rs12203340
Chicago/Turabian StyleXue, Linshan, Xue Li, Weiren Wu, and Yikang Yang. 2020. "Design of Tracking, Telemetry, Command (TT&C) and Data Transmission Integrated Signal in TDD Mode" Remote Sensing 12, no. 20: 3340. https://doi.org/10.3390/rs12203340
APA StyleXue, L., Li, X., Wu, W., & Yang, Y. (2020). Design of Tracking, Telemetry, Command (TT&C) and Data Transmission Integrated Signal in TDD Mode. Remote Sensing, 12(20), 3340. https://doi.org/10.3390/rs12203340





