Ionization in the Earth’s Atmosphere Due to Isotropic Energetic Electron Precipitation: Ion Production and Primary Electron Spectra
Abstract
:1. Introduction
2. Computation of EEP Ionization Rates
3. Atmospheric Ionization Yield Function for EEP with Isotropic Incidence
4. Spectra Function for Energetic Electron Precipitation
4.1. Exponential Spectral Distribution
4.2. Maxwellian Spectral Distribution
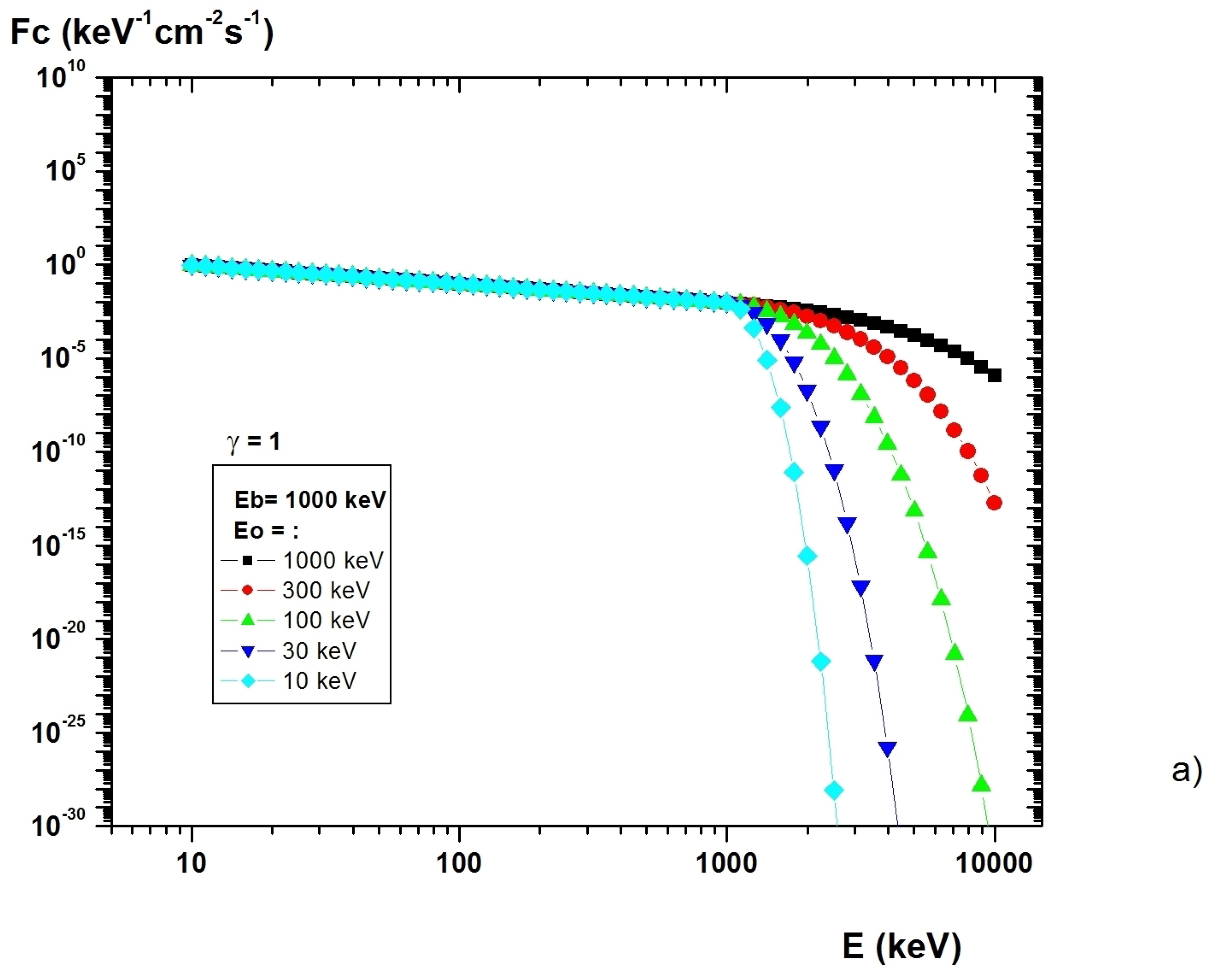
4.3. The Generalized Lorentzian (or -) Spectral Distribution
4.4. Power-Law Spectral Distribution
4.5. Combined Spectral Distribution
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Lam, M.M.; Horne, R.B.; Meredith, N.P.; Glauert, S.A.; Moat-Grin, T.; Green, J.C. Origin of energetic electron precipitation >30 keV into the atmosphere. J. Geophys. Res. 2010, 115, A00F08. [Google Scholar] [CrossRef]
- Rodger, C.J.; Clilverd, M.A.; Green, J.C.; Lam, M.M. Use of POES SEM-2 observations to examine radiation belt dynamics and energetic electron precipitation into the atmosphere. J. Geophys. Res. 2010, 115, A04202. [Google Scholar] [CrossRef] [Green Version]
- Millan, R.M.; Lin, R.P.; Smith, D.M.; McCarthy, M.P. Observation of relativistic electron precipitation during a rapid decrease of trapped relativistic electron flux. Geophys. Res. Lett. 2007, 34, L10101. [Google Scholar] [CrossRef]
- Makhmutov, V.; Bazilevskaya, G.; Stozhkov, Y.; Svirzhevskaya, A.; Svirzhevsky, N. Catalogue of electron precipitation events as observed in the long-duration cosmic ray balloon experiment. J. Atmos. Sol.-Terr. Phys. 2016, 149, 258–276. [Google Scholar] [CrossRef]
- Mironova, I.A.; Artamonov, A.A.; Bazilevskaya, G.A.; Rozanov, E.V.; Kovaltsov, G.A.; Makhmutov, V.S.; Mishev, A.L.; Karagodin, A.V. Ionization of the Polar Atmosphere by Energetic Electron Precipitation Retrieved From Balloon Measurements. Geophys. Res. Lett. 2019, 46, 990–996. [Google Scholar] [CrossRef] [Green Version]
- Mironova, I.A.; Bazilevskaya, G.A.; Kovaltsov, G.A.; Artamonov, A.A.; Rozanov, E.V.; Mishev, A.; Makhmutov, V.S.; Karagodin, A.V.; Golubenko, K.S. Spectra of high energy electron precipitation and atmospheric ionization rates retrieval from balloon measurements. Sci. Total Environ. 2019, 693, 133–242. [Google Scholar] [CrossRef] [PubMed]
- Lejeune, G.; Lathuillere, C.; Kofman, W. On the possibility to measure the high altitude light ion concentrations with EISCAT. Ann. Geophys. 1982, 38, 467–471. [Google Scholar]
- Sinnhuber, M.; Nieder, H.; Wieters, N. Energetic Particle Precipitation and the Chemistry of the Mesosphere/Lower Thermosphere. Surv. Geophys. 2012, 33, 1281–1334. [Google Scholar] [CrossRef]
- Mironova, I.; Aplin, K.; Arnold, F.; Bazilevskaya, G.; Harrison, R.; Krivolutsky, A.; Nicoll, K.; Rozanov, E.; Turunen, E.; Usoskin, I. Energetic Particle Influence on the Earth’s Atmosphere. Space Sci. Rev. 2015, 194, 1–96. [Google Scholar] [CrossRef] [Green Version]
- Rozanov, E.; Calisto, M.; Egorova, T.; Peter, T.; Schmutz, W. Influence of the Precipitating Energetic Particles on Atmospheric Chemistry and Climate. Surv. Geophys. 2012, 33, 483–501. [Google Scholar] [CrossRef] [Green Version]
- Arsenovic, P.; Rozanov, E.; Stenke, A.; Funke, B.; Wissing, J.; Mursula, K.; Tummon, F.; Peter, T. The influence of Middle Range Energy Electrons on atmospheric chemistry and regional climate. J. Atmos. Sol.-Terr. Phys. 2016, 149, 180–190. [Google Scholar] [CrossRef] [Green Version]
- Eyring, V.; Bony, S.; Meehl, G.A.; Senior, C.A.; Stevens, B.; Stouffer, R.J.; Taylor, K.E. Overview of the Coupled Model Intercomparison Project Phase 6 (CMIP6) experimental design and organization. Geosci. Model Dev. 2016, 9, 1937–1958. [Google Scholar] [CrossRef] [Green Version]
- Matthes, K.; Funke, B.; Andersson, M.E.; Barnard, L.; Beer, J.; Charbonneau, P.; Clilverd, M.A.; Dudok de Wit, T.; Haberreiter, M.; Hendry, A.; et al. Solar forcing for CMIP6 (v3.2). Geosci. Model Dev. 2017, 10, 2247–2302. [Google Scholar] [CrossRef] [Green Version]
- Rees, M. Auroral ionization and excitation by incident energetic electrons. Planet. Space Sci. 1963, 11, 1209–1218. [Google Scholar] [CrossRef]
- Lazarev, V.I. Absorption of the energy of an electron beam in the upper atmosphere. Geomagn. Aeron. 1967, 7, 219. [Google Scholar]
- Berger, M.J.; Seltzer, S.M. Bremsstrahlung in the atmosphere. J. Atmos. Sol.-Terr. Phys. 1972, 34, 85–108. [Google Scholar] [CrossRef]
- Roble, R.G.; Ridley, E.C. An auroral model for the NCAR thermospheric general circulation model (TGCM). Ann. Geophys. 1987, 5A, 369. [Google Scholar]
- Sergienko, T.E.; Ivanov, V.E. A new approach to calculate the excitation of atmospheric gases by auroral electron impact. Ann. Geophys. 1993, 11, 717–727. [Google Scholar]
- Fang, X.; Randall, C.E.; Lummerzheim, D.; Solomon, S.C.; Mills, M.J.; Marsh, D.R.; Jackman, C.H.; Wang, W.; Lu, G. Electron impact ionization: A new parameterization for 100 eV to 1 MeV electrons. J. Geophys. Res. 2008, 113, A09311. [Google Scholar] [CrossRef]
- Fang, X.; Randall, C.E.; Lummerzheim, D.; Wang, W.; Lu, G.; Solomon, S.C.; Frahm, R.A. Parameterization of monoenergetic electron impact ionization. Geophys. Res. Lett. 2010, 37, L22106. [Google Scholar] [CrossRef]
- Artamonov, A.A.; Mishev, A.L.; Usoskin, I.G. Model CRAC:EPII for atmospheric ionization due to precipitating electrons: Yield function and applications. J. Geophys. Res. (Space Phys.) 2016, 121, 1736–1743. [Google Scholar] [CrossRef] [Green Version]
- Artamonov, A.; Mironova, I.; Kovaltsov, G.; Mishev, A.; Plotnikov, E.; Konstantinova, N. Calculation of atmospheric ionization induced by relativistic electrons with non-vertical precipitation: Update of model CRAC:EPII. Adv. Space Res. 2017, 59, 2295–2300. [Google Scholar] [CrossRef] [Green Version]
- Xu, W.; Marshall, R.A.; Tyssøy, H.N.; Fang, X. A generalized method for calculating atmospheric ionization by energetic electron precipitation. J. Geophys. Res. (Space Phys.) 2020, 125, e2020JA028482. [Google Scholar] [CrossRef]
- Xu, W.; Marshall, R.A.; Tobiska, W.K. A Method for Calculating Atmospheric Radiation Produced by Relativistic Electron Precipitation. Space Weather 2021. [Google Scholar] [CrossRef]
- Usoskin, I.G.; Kovaltsov, G.A.; Mironova, I.A. Cosmic ray induced ionization model CRAC:CRII: An extension to the upper atmosphere. J. Geophys. Res. 2010, 115, D10302. [Google Scholar] [CrossRef] [Green Version]
- Porter, H.S.; Jackman, C.H.; Green, A.E.S. Efficiencies for production of atomic nitrogen and oxygen by relativistic proton impact in air. J. Chem. Phys. 1976, 65, 154–167. [Google Scholar] [CrossRef]
- Picone, J.M.; Hedin, A.E.; Drob, D.P.; Aikin, A.C. NRLMSISE-00 empirical model of the atmosphere: Statistical comparisons and scientific issues. J. Geophys. Res. (Space Phys.) 2002, 107, 1468. [Google Scholar] [CrossRef]
- Mironova, I.; Sinnhuber, M.; Rozanov, E. Energetic electron precipitation and their atmospheric effect. E3S Web Conf. 2020, 196. [Google Scholar] [CrossRef]
- Goldberg, R.A.; Jackman, C.H.; Barcus, J.R.; Soraas, F. Nighttime auroral energy deposition in the middle atmosphere. J. Geophys. Res. 1984, 89, 5581–5596. [Google Scholar] [CrossRef] [Green Version]
- Comess, M.D.; Smith, D.M.; Selesnick, R.S.; Millan, R.M.; Sample, J.G. Duskside relativistic electron precipitation as measured by SAMPEX: A statistical survey. J. Geophys. Res. (Space Phys.) 2013, 118, 5050–5058. [Google Scholar] [CrossRef]
- Lummerzheim, D.; Rees, M.H.; Romick, G.J. The application of spectroscopic studies of the aurora to thermospheric neutral composition. Planet. Space Sci. 1990, 38, 67–78. [Google Scholar] [CrossRef]
- Codrescu, M.V.; Fuller-Rowell, T.J.; Roble, R.G.; Evans, D.S. Medium energy particle precipitation influences on the mesosphere and lower thermosphere. J. Geophys. Res. 1997, 102, 19977–19988. [Google Scholar] [CrossRef]
- Mori, H.; Ishii, M.; Murayama, Y.; Kubota, M.; Sakanoi, K.; Yamamoto, M.; Monzen, Y.; Lummerzheim, D.; Watkins, B. Energy distribution of precipitating electrons estimated from optical and cosmic noise absorption measurements. Ann. Geophys. 2004, 22, 1613–1622. [Google Scholar] [CrossRef] [Green Version]
- Robinson, R.; Vondrak, R.; Miller, K.; Dabbs, T.; Hardy, D. On calculating ionospheric conductances from the flux and energy of precipitating electrons. J. Geophys. Res. 1987, 92, 2565–2570. [Google Scholar] [CrossRef]
- Frahm, R.; Winningham, J.; Sharber, J.; Link, R.; Crowley, G.; Gaines, E.; Chenette, D.; Anderson, B.; Potemra, T. The diffuse aurora: A significant source of ionization in the middle atmosphere. J. Geophys. Res. 1997, 102, 28203–28214. [Google Scholar] [CrossRef]
- Solomon, S.C. Auroral particle transport using Monte Carlo and hybrid methods. J. Geophys. Res. 2001, 106, 107–116. [Google Scholar] [CrossRef] [Green Version]
- Pierrard, V.; Lazar, M. Kappa distributions: Theory and applications in space plasmas. Sol. Phys. 2010, 267, 153–174. [Google Scholar] [CrossRef] [Green Version]
- Sharber, J.R.; Frahm, R.A.; Link, R.; Crowley, G.; Winningham, J.D.; Gaines, E.E.; Nightingale, R.W.; Chenette, D.L.; Anderson, B.J.; Gurgiolo, C.A. UARS particle environment monitor observations during the November 1993 storm: Auroral morphology, spectral characterization, and energy deposition. J. Geophys. Res. 1998, 103, 26307–26322. [Google Scholar] [CrossRef]
- Vasyliunas, V.M. A survey of low-energy electrons in the evening sector of the magnetosphere with OGO 1 and OGO 3. J. Geophys. Res. 1968, 73, 2839–2884. [Google Scholar] [CrossRef]
- Vampola, A.L.; Gorney, D.J. Electron energy deposition in the middle atmosphere. J. Geophys. Res. 1983, 88, 6267–6274. [Google Scholar] [CrossRef]
- Schroter, J.; Heber, B.; Steinhilber, F.; Kallenrode, M.B. Energetic particles in the atmosphere: A Monte-carlo simulation. Adv. Space Res. 2006, 37, 1597–1601. [Google Scholar] [CrossRef]
- Wissing, J.; Kallenrode, M.-B. Atmospheric ionization module Osnabrück (AIMOS): A 3-D model to determine atmospheric ionization by energetic charged particles from different populations. J. Geophys. Res. 2009, 114, A06104. [Google Scholar] [CrossRef] [Green Version]
- Clilverd, M.A.; Rodger, C.J.; Gamble, R.J.; Ulich, T.; Raita, T.; Seppälxax, A.; Green, J.C.; Thomson, N.R.; Sauvaud, J.-A.; Parrot, M. Ground-based estimates of outer radiation belt energetic electron precipitation fluxes into the atmosphere. J. Geophys. Res. (Space Phys.) 2010, 115, A12304. [Google Scholar] [CrossRef] [Green Version]
- van de Kamp, M.; Seppälxax, A.; Clilverd, M.A.; Rodger, C.J.; Verronen, P.T.; Whittaker, I.C. A model providing long-term data sets of energetic electron precipitation during geomagnetic storms. J. Geophys. Res. (Atmos.) 2016, 121, 12520–12540. [Google Scholar] [CrossRef] [Green Version]
- Birch, M.J.; Hargreaves, J.; Bromage, B.J.I.; Evans, D. Effects of high-speed solar wind on energetic electron activity in the auroral regions during July 1–2, 2005. J. Atmos. Sol.-Terr. Phys. 2009, 71, 1190–1209. [Google Scholar] [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mironova, I.; Kovaltsov, G.; Mishev, A.; Artamonov, A. Ionization in the Earth’s Atmosphere Due to Isotropic Energetic Electron Precipitation: Ion Production and Primary Electron Spectra. Remote Sens. 2021, 13, 4161. https://doi.org/10.3390/rs13204161
Mironova I, Kovaltsov G, Mishev A, Artamonov A. Ionization in the Earth’s Atmosphere Due to Isotropic Energetic Electron Precipitation: Ion Production and Primary Electron Spectra. Remote Sensing. 2021; 13(20):4161. https://doi.org/10.3390/rs13204161
Chicago/Turabian StyleMironova, Irina, Gennadiy Kovaltsov, Alexander Mishev, and Anton Artamonov. 2021. "Ionization in the Earth’s Atmosphere Due to Isotropic Energetic Electron Precipitation: Ion Production and Primary Electron Spectra" Remote Sensing 13, no. 20: 4161. https://doi.org/10.3390/rs13204161
APA StyleMironova, I., Kovaltsov, G., Mishev, A., & Artamonov, A. (2021). Ionization in the Earth’s Atmosphere Due to Isotropic Energetic Electron Precipitation: Ion Production and Primary Electron Spectra. Remote Sensing, 13(20), 4161. https://doi.org/10.3390/rs13204161





