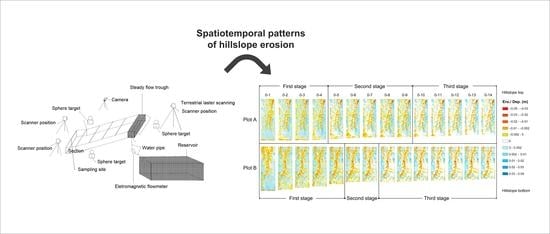
Spatiotemporal Patterns of Hillslope Erosion Investigated Based on Field Scouring Experiments and Terrestrial Laser Scanning
Abstract
1. Introduction
2. Materials and Methods
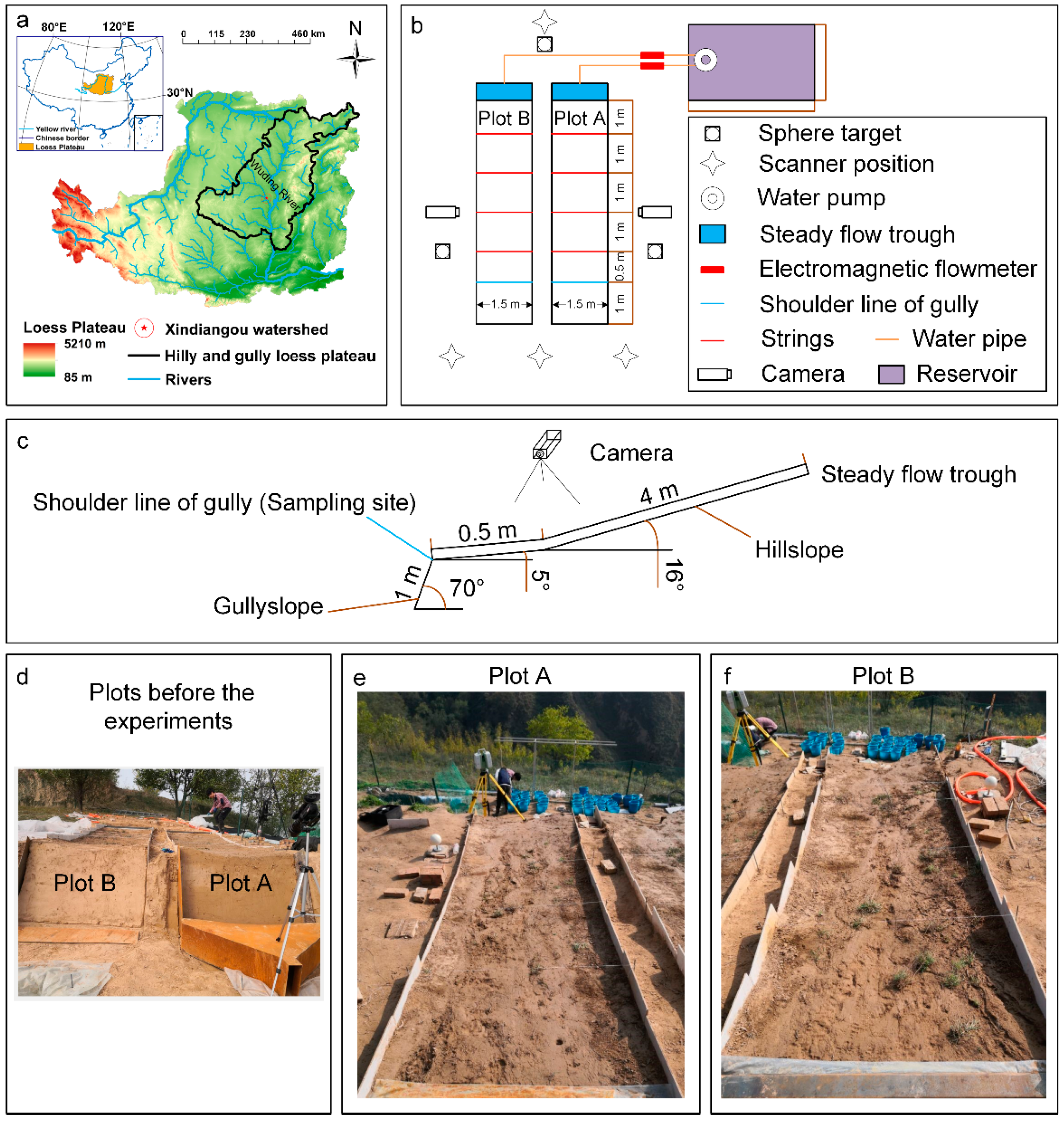
2.1. Study Site
2.2. Experimental Design and Establishment of Plots
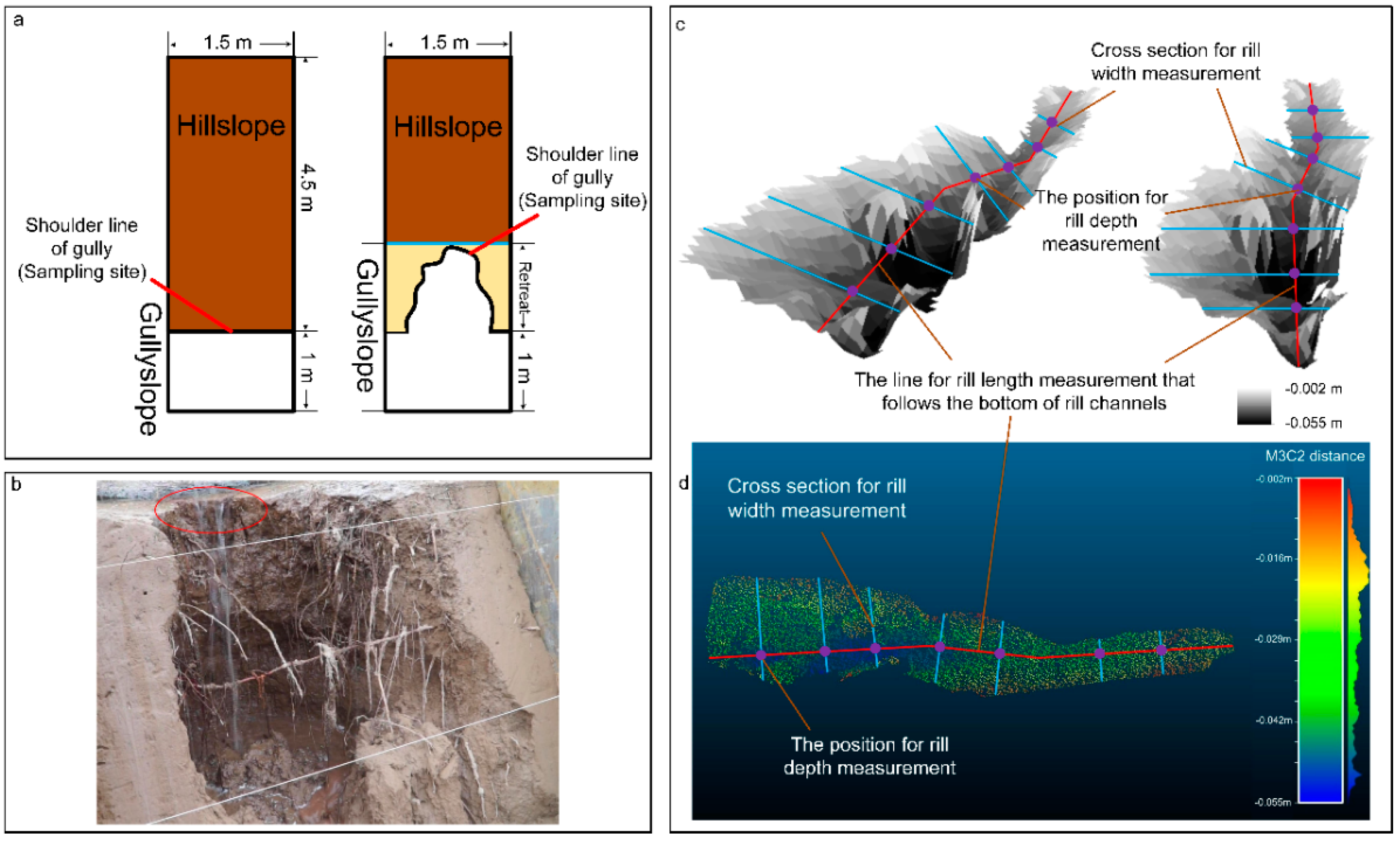
2.3. Data Collection and Processing
2.3.1. Collection of Sediment and Runoff
2.3.2. Acquisition and Processing of LiDAR Point Clouds
- LiDAR point cloud acquisition
- Registration of LiDAR point clouds
- Derivation of volumetric changes and soil erosion mass
- Derivation of rill dimensions
3. Results
3.1. Accuracy of the Sediment Yield Derived using Terrestrial Laser Scanning
3.2. Spatiotemporal Patterns of Erosion Processes
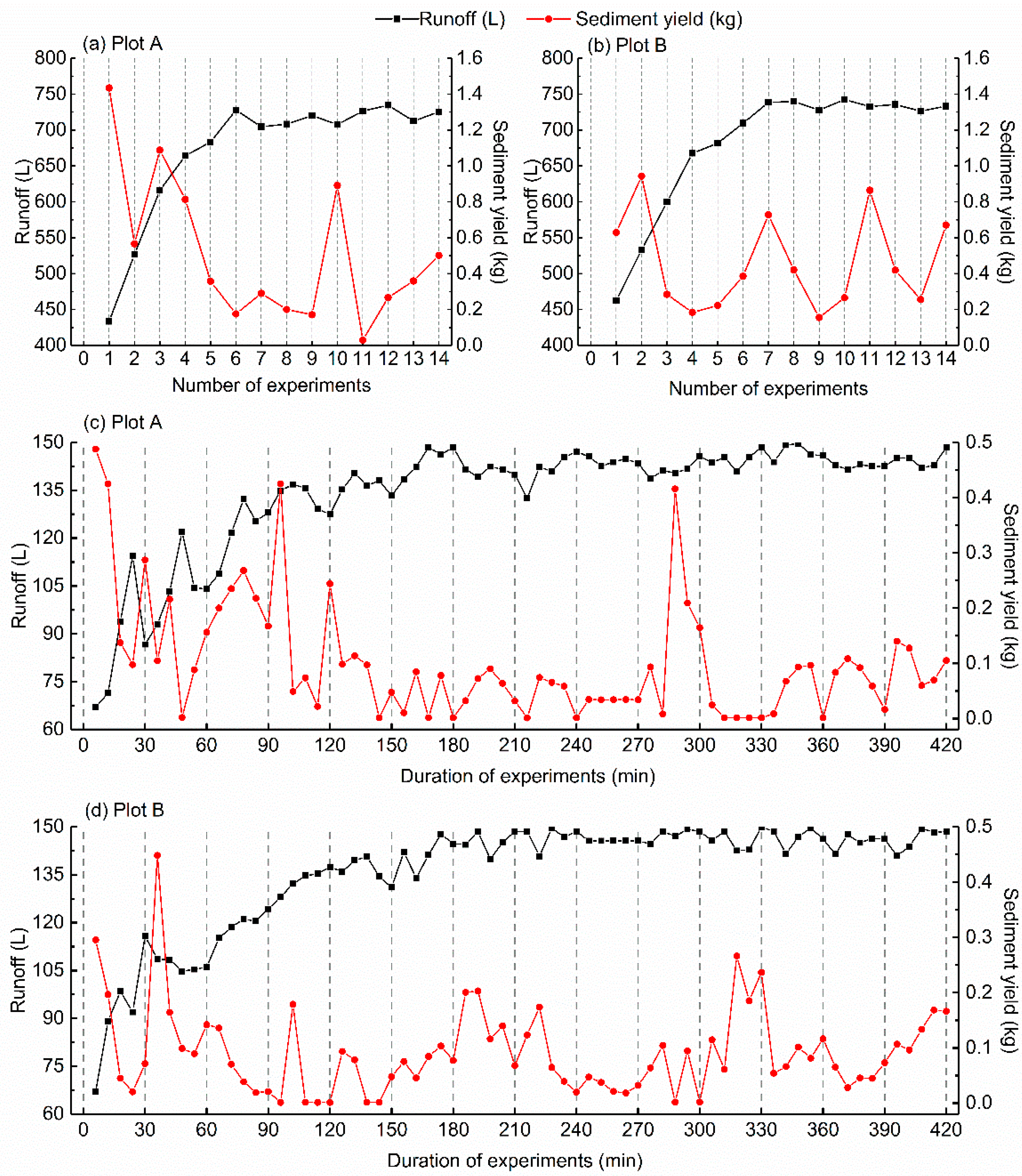
3.2.1. Measured Runoff and Sediment Discharge from Hillslopes
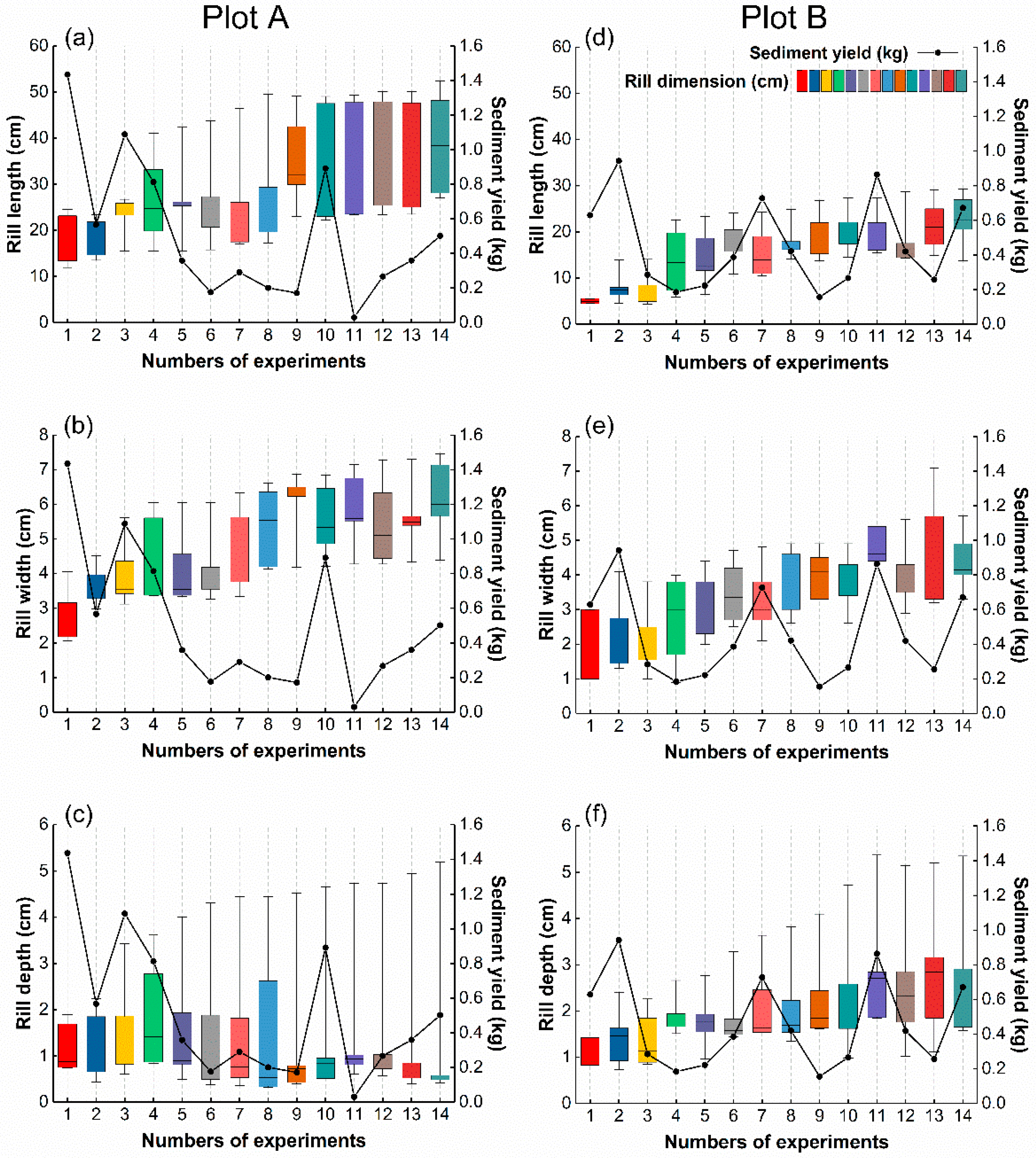
3.2.2. Spatiotemporal Development of Hillslope Erosion Processes
4. Discussion
4.1. The Design of the Study
4.2. Uncertainty in TLS Results
4.3. Spatiotemporal Patterns of the Soil Erosion Processes
4.4. Implications
5. Conclusions
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Borrelli, P.; Robinson, D.A.; Fleischer, L.R.; Lugato, E.; Ballabio, C.; Alewell, C.; Meusburger, K.; Modugno, S.; Schütt, B.; Ferro, V.; et al. An assessment of the global impact of 21st century land use change on soil erosion. Nat. Commun. 2017, 8, 2013. [Google Scholar] [CrossRef]
- Borrelli, P.; Robinson, D.A.; Panagos, P.; Lugato, E.; Yang, J.E.; Alewell, C.; Wuepper, D.; Montanarella, L.; Ballabio, C. Land use and climate change impacts on global soil erosion by water (2015–2070). Proc. Natl. Acad. Sci. USA 2020, 117, 21994–22001. [Google Scholar] [CrossRef] [PubMed]
- De Vries, F.T.; Liiri, M.E.; Bjornlund, L.; Bowker, M.A.; Christensen, S.; Setala, H.; Bardgett, R.D. Land use alters the resistance and resilience of soil food webs to drought. Nat. Clim. Chang. 2012, 2, 276–280. [Google Scholar] [CrossRef]
- Rodrigo-Comino, J.; Terol, E.; Mora, G.; Giménez-Morera, A.; Cerdà, A. Vicia sativa Roth. can reduce soil and water losses in recently planted vineyards (Vitis vinifera L.). Earth Syst. Environ. 2020, 4, 827–842. [Google Scholar] [CrossRef]
- Tsunekawa, A.; Liu, G.; Yamanaka, N.; Du, S. Restoration and Development of the Degraded Loess Plateau, China; Springer: Tokyo, Japan, 2014; pp. 507–508. [Google Scholar]
- Clarke, M.L.; Rendell, H.M. The impact of the farming practice of remodelling hillslope topography on badland morphology and soil erosion processes. Catena 2000, 40, 229–250. [Google Scholar] [CrossRef]
- Hales, T.; Scharer, K.; Wooten, R. Southern Appalachian hillslope erosion rates measured by soil and detrital radiocarbon in hollows. Geomorphology 2012, 138, 121–129. [Google Scholar] [CrossRef]
- Guo, D.W.; Yu, B.F.; Fu, X.D.; Li, T.J. Improved Hillslope Erosion Module for the Digital Yellow-River Model. J. Hydrol. Eng. 2014, 20, 1–11. [Google Scholar] [CrossRef]
- Yang, X. State and trends of hillslope erosion across New South Wales, Australia. Catena 2020, 186, 104361. [Google Scholar] [CrossRef]
- Zhang, L.T.; Gao, Z.L.; Li, Z.B.; Tian, H.W. Downslope runoff and erosion response of typical engineered landform to variable inflow rate patterns from upslope. Nat. Hazards 2016, 80, 775–796. [Google Scholar] [CrossRef]
- Xu, X.; Zheng, F.; Wilson, G.V.; Zhang, X.C.; Qin, C.; He, X. Quantification of upslope and lateral inflow impacts on runoff discharge and soil loss in ephemeral gully systems under laboratory conditions. J. Hydrol. 2019, 579, 124174. [Google Scholar] [CrossRef]
- Xu, J.X. Erosion caused by hyperconcentrated flow on the Loess Plateau of China. Catena 1999, 36, 1–19. [Google Scholar]
- Van Maren, D.S.; Winterwerp, J.C.; Wu, B.S.; Zhou, J.J. Modelling hyperconcentrated flow in the Yellow River. Earth Surf. Process. Landf. 2009, 34, 596–612. [Google Scholar] [CrossRef]
- Neugirg, F.; Kaiser, A.; Huber, A.; Heckmann, T.; Schindewolf, M.; Schmidt, J.; Becht, M.; Haas, F. Using terrestrial LiDAR data to analyse morphodynamics on steep unvegetated slopes driven by different geomorphic processes. Catena 2016, 142, 269–280. [Google Scholar] [CrossRef]
- Nouwakpo, S.K.; Weltz, M.A.; McGwire, K. Assessing the performance of structure-from-motion photogrammetry and terrestrial LiDAR for reconstructing soil surface microtopography of naturally vegetated plots. Earth Surf. Process. Landf. 2016, 41, 308–322. [Google Scholar] [CrossRef]
- Li, L.; Nearing, M.A.; Nichols, M.H.; Polyakov, V.O.; Cavanaugh, M.L. Using terrestrial LiDAR to measure water erosion on stony plots under simulated rainfall. Earth Surf. Process Landf. 2020, 45, 484–495. [Google Scholar] [CrossRef]
- Wang, P.; Jin, X.; Li, S.D. Application of Handheld 3D Scanner in Quantitative Study of Slope Soil Erosion. IOP Conf. Ser. Earth Environ. Sci. 2018, 170, 022178. [Google Scholar] [CrossRef]
- Wirtz, S.; Seeger, M.; Ries, J.B. Field experiments for understanding and quantification of rill erosion processes. Catena 2012, 91, 21–34. [Google Scholar] [CrossRef]
- Qin, C.; Zheng, F.L.; Xu, X.M.; Wu, H.Y.; Shen, H.O. A laboratory study on rill network development and morphological characteristics on loessial hillslope. J. Soils Sedim. 2018, 18, 1679–1690. [Google Scholar] [CrossRef]
- Michael, C.S.; Rorke, B.B. Hydraulic conditions for rill incision under simulated rainfall: A laboratory experiment. Earth Surf. Process. Landf. 1992, 17, 127–146. [Google Scholar]
- Nearing, M.A.; Foster, G.R.; Lane, L.J.; Finkner, S.C. A process-based soil erosion model for USDA-Water Erosion Prediction Project technology. Trans. ASAE 1989, 32, 1587–1593. [Google Scholar] [CrossRef]
- Hessel, R. Consequences of hyperconcentrated flow for process-based soil erosion modelling on the Chinese Loess Plateau. Earth Surf. Process. Landf. 2006, 31, 1100–1114. [Google Scholar] [CrossRef]
- Yang, X.; Yu, B.; Zhu, Q. Climate change impacts on rainfall erosivity and hillslope erosion in NSW. In Proceedings of the 21st International Congress on Modelling and Simulation, Gold Coarst, Australia, 29 November–4 December 2015. [Google Scholar]
- Evans, M.; Lindsay, J. High resolution quantification of gully erosion in upland peatlands at the landscape scale. Earth Surf. Process. Landf. 2010, 35, 876–886. [Google Scholar] [CrossRef]
- Chico, G.; Clutterbuck, B.; Midgley, N.G.; Labadz, J. Application of terrestrial laser scanning to quantify surface changes in restored and degraded blanket bogs. Mires Peat 2019, 24, 1–24. [Google Scholar]
- Goodwin, N.R.; Armston, J.D.; Muir, J.; Stiller, I. Monitoring gully change: A comparison of airborne and terrestrial laser scanning using a case study from Aratula, Queensland. Geomorphology 2017, 282, 195–208. [Google Scholar] [CrossRef]
- Telling, J.; Lyda, A.; Hartzell, P.; Glennie, C. Review of Earth science research using terrestrial laser scanning. Earth Sci. Rev. 2017, 169, 35–68. [Google Scholar] [CrossRef]
- Usmanov, B.; Yermolaev, O.; Gafurov, A. Estimates of slope erosion intensity utilizing terrestrial laser scanning. Proc. Int. Assoc. Hydrol. Sci. 2015, 367, 59–65. [Google Scholar] [CrossRef]
- Eltner, A.; Mulsow, C.; Maas, H. Quantitative measurement of soil erosion from TLS and UAV data. Int. Arch. Photogramm. Remote Sens. Spat. Inf. Sci. 2013, 40, 4–6. [Google Scholar] [CrossRef]
- Eltner, A.; Maas, H.; Faust, D. Soil micro-topography change detection at hillslopes in fragile Mediterranean landscapes. Geoderma 2018, 313, 217–232. [Google Scholar] [CrossRef]
- Zhao, G.; Mu, X.; Wen, Z.; Wang, F.; Gao, P. Soil erosion, conservation, and eco-environment changes in the loess plateau of China. Land Degrad. Dev. 2013, 24, 499–510. [Google Scholar] [CrossRef]
- Zhao, G.; Gao, P.; Tian, P.; Sun, W.; Hu, J.; Mu, X. Assessing sediment connectivity and soil erosion by water in a representative catchment on the Loess Plateau. Catena 2020, 185, 104284. [Google Scholar] [CrossRef]
- Dang, W.; Dang, T. Analysis of landscape and aesthetic beauty of soil and water conservation technology demonstration park in the Xindian Gully. In Proceedings of the Cross-Strait Symposium on Soil and Water Conservation, Taiyuan, China, 2 September 2015. (In Chinese with English Abstract). [Google Scholar]
- Li, M.; Yao, W.Y.; Ding, W.F.; Yang, J.F.; Chen, J.N. Effect of grass coverage on sediment yield in the hillslope-gully side erosion system. J. Geogr. Sci. 2009, 19, 321–330. [Google Scholar] [CrossRef]
- Gong, J.; Jia, Y.; Zhou, Z.; Wang, Y.; Wang, W.; Peng, H. An experimental study on dynamic processes of ephemeral gully erosion in loess landscapes. Geomorphology 2011, 125, 203–213. [Google Scholar] [CrossRef]
- Yellow River Conservancy Commission [YRCC]. 1954—1963 Water and Soil Conservation Runoff Test Data in the Middle Reaches of the Yellow River, Tianshui, Xifeng, Suide Station Small Watershed Parts; Yellow River Conservancy Commission [YRCC]: Xi’an, China, 1966. (In Chinese) [Google Scholar]
- Cucchiaro, S.; Fallu, D.J.; Zhang, H.; Walsh, K.; Van, O.K.; Brown, A.G.; Tarolli, P. Multiplatform-SfM and TLS data fusion for monitoring agricultural terraces in complex topographic and landcover conditions. Remote Sens. 2020, 12, 1946. [Google Scholar] [CrossRef]
- Brandyk, T.; Dodd, V.; Grace, P. An assessment of some unsaturated hydraulic conductivity characteristics for a range of Irish peat soils. Ir. J. Agric. Res. 1985, 24, 237–251. [Google Scholar]
- Lane, S.N.; Westaway, R.M.; Murray, D. Estimation of erosion and deposition volumes in a large, gravel-bed, braided river using synoptic remote sensing. Earth Surf. Process. Landf. 2003, 28, 249–271. [Google Scholar] [CrossRef]
- Wheaton, J.M.; Brasington, J.; Darby, S.E.; Sear, D.A. Accounting for uncertainty in DEMs from repeat topographic surveys: Improved sediment budgets. Earth Surf. Process. Landf. 2010, 35, 136–156. [Google Scholar] [CrossRef]
- Antoniazza, G.; Bakker, M.; Lane, S.N. Revisiting the morphological method in two-dimensions to quantify bed-material transport in braided rivers. Earth Surf. Process.Landf. 2019, 44, 2251–2267. [Google Scholar] [CrossRef]
- Girardeau-Montaut, D.; Roux, M.; Marc, R.; Thibault, G. Change detection on points cloud data acquired with a ground laser scanner. Int. Archi. Photogramm. Remote Sens. Spat. Inf. Sci. 2005, 36, W19. [Google Scholar]
- Cignoni, P.; Rocchini, C.; Scopigno, R. Metro: Measuring Error on Simplified Surfaces. Comput. Graph. Forum 1998, 17, 167–174. [Google Scholar] [CrossRef]
- Lague, D.; Brodu, N.; Leroux, J. Accurate 3D comparison of complex topography with terrestrial laser scanner: Application to the Rangitikei canyon (N-Z). ISPRS J. Photogramm. Remote Sens. 2013, 82, 10–26. [Google Scholar] [CrossRef]
- Vericat, D.; Smith, M.W.; Brasington, J. Patterns of topographic change in sub-humid badlands determined by high resolution multi-temporal topographic surveys. Catena 2014, 120, 164–176. [Google Scholar] [CrossRef]
- Zhang, J.; Zheng, F.L.; Wen, L.L.; Yu, F.Y.; An, J.; Li, G.F. Methodology of Dynamic Monitoring Gully Erosion Process Using 3D Laser Scanning Technology. Bull. Soil Water Conserv. 2011, 31, 89–94, (In Chinese with English Abstract). [Google Scholar]
- Xiao, H.; Xia, Z.; Zhu, X.; Yang, Y.; Li, Y. Application of Three-dimensional Laser Scanner on Research of Slope Soil Erosion. Bull. Soil Water Conserv. 2014, 34, 198–200, (In Chinese with English Abstract). [Google Scholar]
- Huff, T.P.; Feagin, R.A.; Delgado, A. Understanding Lateral Marsh Edge Erosion with Terrestrial Laser Scanning (TLS). Remote Sens. 2019, 11, 2208. [Google Scholar] [CrossRef]
- Zang, Y.F.; Yang, B.S.; Li, J.P.; Guan, H.Y. An accurate TLS and UAV image point clouds registration method for deformation detection of chaotic hillside areas. Remote Sens. 2019, 11, 647. [Google Scholar] [CrossRef]
- Tan, K.; Xiao, J.C. Correction of incidence angle and distance effects on TLS intensity data based on reference targets. Remote Sens. 2016, 8, 251. [Google Scholar] [CrossRef]
- Fang, H.; Yang, X.; Zhang, X.; Liang, A. Using 137Cs tracer technique to evaluate erosion and deposition of black soil in Northeast China. Pedosphere 2006, 16, 201–209. [Google Scholar] [CrossRef]
- An, J.; Zheng, F.; Wang, B. Using 137 Cs technique to investigate the spatial distribution of erosion and deposition regimes for a small catchment in the black soil region, Northeast China. Catena 2014, 123, 243–251. [Google Scholar] [CrossRef]
- Bookhagen, B.; Strecker, M.R. Spatiotemporal trends in erosion rates across a pronounced rainfall gradient: Examples from the southern Central Andes. Earth Planet. Sci. Lett. 2012, 327–328, 97–110. [Google Scholar] [CrossRef]
- Bryan, R.B.; Poesen, J. Laboratory experiments on the influence of slope length on runoff, percolation and rill development. Earth Surf. Process. Landf. 2010, 14, 211–231. [Google Scholar] [CrossRef]
- Besl, P.J.; McKay, N.D. A Method for Registration of 3-D Shapes. IEEE Trans. Pattern Anal. Mach. Intell. 1992, 14, 239–256. [Google Scholar] [CrossRef]
- Hancock, G.R.; Lowry, J.B. Hillslope erosion measurement—A simple approach to a complex process. Hydrol. Process. 2015, 29, 4809–4816. [Google Scholar] [CrossRef]
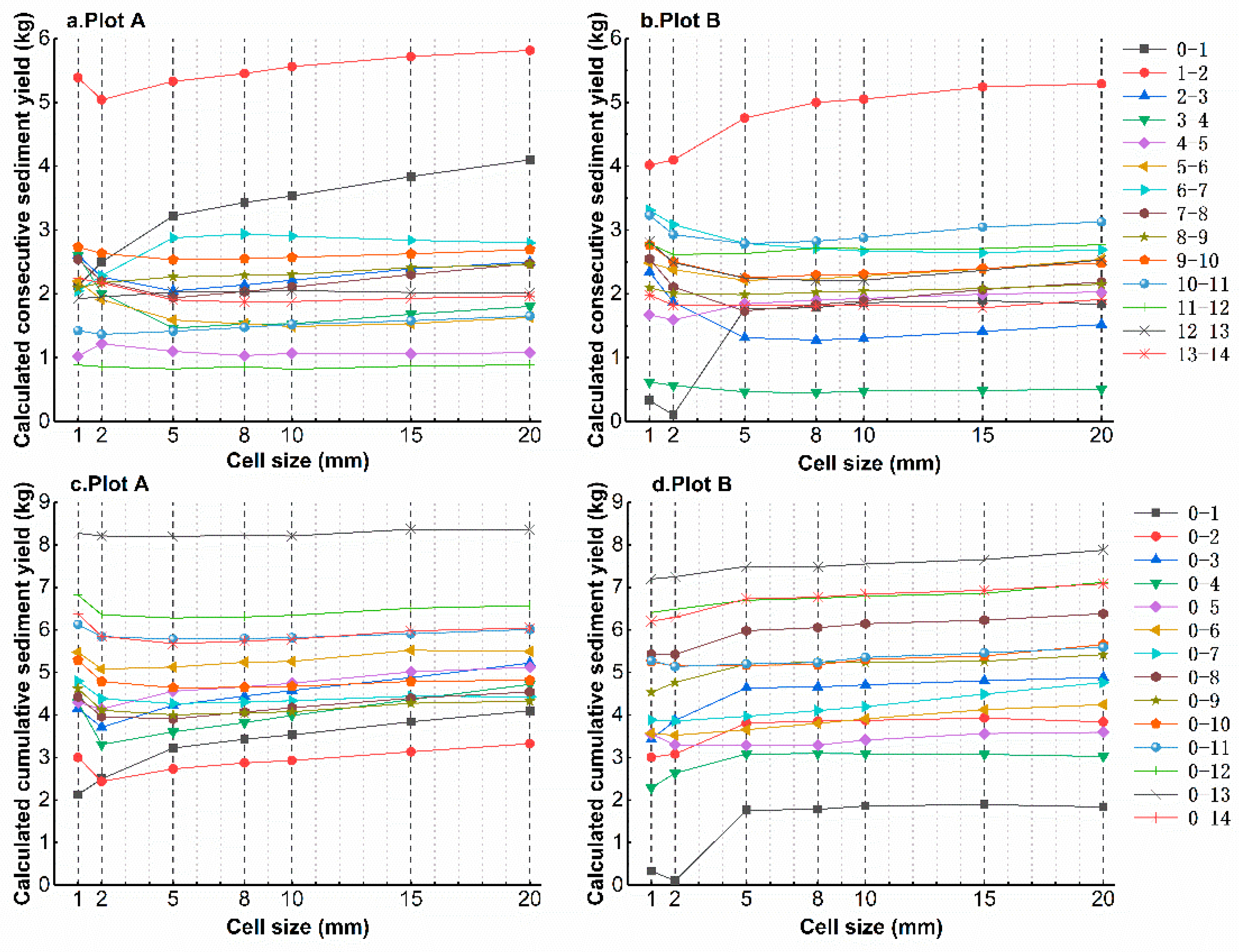


| Plot | Cell Size (mm) | Magnitude of Absolute Errors (kg) | Magnitude of Relative Errors (%) | Linear Relation | ||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Max | Min | Mean | Max | Min | Mean | R2 | p | |||
| Consecutive sediment yield | A | 5 | 4.76 | 0.56 | 1.67 | 4705.44 | 80.21 | 784.12 | 0.11 | 0.245 |
| 8 | 4.88 | 0.60 | 1.72 | 4905.49 | 87.61 | 805.28 | 0.13 | 0.211 | ||
| 10 | 4.99 | 0.55 | 1.74 | 5070.74 | 88.55 | 820.23 | 0.14 | 0.196 | ||
| 15 | 5.15 | 0.60 | 1.83 | 5266.4 | 106.65 | 853.71 | 0.16 | 0.150 | ||
| 20 | 5.25 | 0.62 | 1.91 | 5518.67 | 121.92 | 888.86 | 0.19 | 0.123 | ||
| B | 5 | 3.81 | 0.28 | 1.73 | 1180.65 | 153.15 | 466.21 | 0.47 | 0.006 | |
| 8 | 4.06 | 0.27 | 1.76 | 1204.23 | 145.84 | 473.67 | 0.46 | 0.007 | ||
| 10 | 4.11 | 0.29 | 1.79 | 1216.81 | 160.46 | 480.71 | 0.47 | 0.007 | ||
| 15 | 4.30 | 0.30 | 1.86 | 1242.75 | 165.11 | 502.07 | 0.45 | 0.009 | ||
| 20 | 4.35 | 0.32 | 1.94 | 1286.78 | 175.74 | 524.83 | 0.44 | 0.010 | ||
| Cumulative sediment yield | A | 5 | 1.79 | 0.004 | 0.87 | 124.49 | 0.06 | 25.02 | 0.61 | <0.001 |
| 8 | 2.00 | 0.02 | 0.89 | 139.22 | 0.33 | 26.65 | 0.58 | 0.001 | ||
| 10 | 2.10 | 0.06 | 0.90 | 146.26 | 0.94 | 27.54 | 0.58 | 0.002 | ||
| 15 | 2.40 | 0.11 | 0.98 | 167.59 | 1.80 | 31.19 | 0.55 | 0.003 | ||
| 20 | 2.67 | 0.01 | 1.04 | 185.79 | 0.12 | 34.32 | 0.50 | 0.005 | ||
| B | 5 | 2.78 | 0.12 | 1.26 | 180.06 | 2.34 | 56.82 | 0.82 | <0.001 | |
| 8 | 2.80 | 0.16 | 1.30 | 183.55 | 3.06 | 58.48 | 0.82 | <0.001 | ||
| 10 | 2.85 | 0.27 | 1.37 | 195.96 | 5.39 | 61.23 | 0.83 | <0.001 | ||
| 15 | 2.95 | 0.38 | 1.47 | 200.81 | 7.46 | 64.64 | 0.83 | <0.001 | ||
| 20 | 3.03 | 0.51 | 1.59 | 192.27 | 10.07 | 66.73 | 0.84 | <0.001 | ||
| Plot | Rill Dimension (cm) | TLS-Derived Erosion (kg) | TLS-Derived Deposition (kg) | TLS-Derived Sediment Yield (kg) | Measured Sediment Yield (kg) | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Slope | R2 | p | Slope | R2 | p | Slope | R2 | p | Slope | R2 | p | ||
| A | Max. rill length | 0.08 | 0.32 | 0.037 * | −0.01 | 0.03 | 0.574 | 0.09 | 0.39 | 0.016 * | 0.15 | 0.87 | <0.001 ** |
| Min. rill length | 0.18 | 0.36 | 0.023 * | −0.03 | 0.07 | 0.350 | 0.21 | 0.50 | 0.005 * | 0.34 | 0.86 | <0.001 ** | |
| Ave. rill length | 0.13 | 0.44 | 0.010 * | −0.02 | 0.05 | 0.464 | 0.15 | 0.56 | 0.002 * | 0.23 | 0.90 | <0.001 ** | |
| Max. rill width | 0.96 | 0.48 | 0.006 * | −0.05 | 0.01 | 0.727 | 1.01 | 0.54 | 0.003 * | 1.62 | 0.96 | <0.001 ** | |
| Min. rill width | 1.15 | 0.32 | 0.035 * | −0.14 | 0.03 | 0.550 | 1.29 | 0.40 | 0.015 * | 2.26 | 0.86 | <0.001 ** | |
| Ave. rill width | 0.73 | 0.27 | 0.046 * | −0.06 | 0.01 | 0.695 | 0.79 | 0.32 | 0.034 * | 1.51 | 0.83 | <0.001 ** | |
| Max. rill depth | 0.99 | 0.47 | 0.007 ** | −0.05 | 0.01 | 0.755 | 1.04 | 0.53 | 0.003 * | 1.66 | 0.93 | <0.001 ** | |
| Min. rill depth | −0.54 | 0.003 | 0.855 | 1.10 | 0.08 | 0.316 | −1.64 | 0.03 | 0.573 | −3.82 | 0.10 | 0.266 | |
| Ave. rill depth | 1.69 | 0.09 | 0.303 | 1.19 | 0.30 | 0.042 * | 0.50 | 0.01 | 0.766 | 1.34 | 0.04 | 0.500 | |
| B | Max. rill length | 0.19 | 0.36 | 0.023 * | −0.11 | 0.20 | 0.107 | 0.17 | 0.54 | 0.003 * | 0.22 | 0.74 | <0.001 ** |
| Min. rill length | 0.42 | 0.67 | <0.001 ** | −0.01 | 0.07 | 0.360 | 0.29 | 0.63 | <0.001 ** | 0.36 | 0.80 | <0.001 ** | |
| Ave. rill length | 0.27 | 0.45 | 0.008 * | −0.13 | 0.20 | 0.107 | 0.21 | 0.54 | 0.003 * | 0.28 | 0.80 | <0.001 ** | |
| Max. rill width | 2.08 | 0.60 | 0.001 * | −0.49 | 0.06 | 0.397 | 1.74 | 0.81 | <0.001 ** | 1.97 | 0.87 | <0.001 ** | |
| Min. rill width | 1.53 | 0.55 | 0.003 * | −0.36 | 0.05 | 0.426 | 1.02 | 0.47 | 0.007 * | 1.37 | 0.72 | <0.001 ** | |
| Ave. rill width | 1.93 | 0.58 | 0.001 * | −0.66 | 0.12 | 0.217 | 1.35 | 0.55 | 0.002 * | 1.8 | 0.84 | <0.001 ** | |
| Max. rill depth | 1.32 | 0.60 | 0.001 * | −0.33 | 0.07 | 0.370 | 1.06 | 0.74 | <0.001 ** | 1.31 | 0.96 | <0.001 ** | |
| Min. rill depth | 0.71 | 0.02 | 0.674 | −2.06 | 0.23 | 0.079 | 0.61 | 0.02 | 0.615 | 1.82 | 0.16 | 0.150 | |
| Ave. rill depth | 2.77 | 0.51 | 0.004 * | −1.02 | 0.13 | 0.214 | 2.24 | 0.64 | <0.001 ** | 2.88 | 0.90 | <0.001 ** | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, P.; Hao, M.; Hu, J.; Gao, C.; Zhao, G.; Chan, F.K.S.; Gao, J.; Dang, T.; Mu, X. Spatiotemporal Patterns of Hillslope Erosion Investigated Based on Field Scouring Experiments and Terrestrial Laser Scanning. Remote Sens. 2021, 13, 1674. https://doi.org/10.3390/rs13091674
Li P, Hao M, Hu J, Gao C, Zhao G, Chan FKS, Gao J, Dang T, Mu X. Spatiotemporal Patterns of Hillslope Erosion Investigated Based on Field Scouring Experiments and Terrestrial Laser Scanning. Remote Sensing. 2021; 13(9):1674. https://doi.org/10.3390/rs13091674
Chicago/Turabian StyleLi, Pengfei, Mingkui Hao, Jinfei Hu, Chendi Gao, Guangju Zhao, Faith Ka Shun Chan, Jianjian Gao, Tianmin Dang, and Xingmin Mu. 2021. "Spatiotemporal Patterns of Hillslope Erosion Investigated Based on Field Scouring Experiments and Terrestrial Laser Scanning" Remote Sensing 13, no. 9: 1674. https://doi.org/10.3390/rs13091674
APA StyleLi, P., Hao, M., Hu, J., Gao, C., Zhao, G., Chan, F. K. S., Gao, J., Dang, T., & Mu, X. (2021). Spatiotemporal Patterns of Hillslope Erosion Investigated Based on Field Scouring Experiments and Terrestrial Laser Scanning. Remote Sensing, 13(9), 1674. https://doi.org/10.3390/rs13091674