Evolution of Maritime GNSS and RNSS Performance Standards
Abstract
1. Introduction
- –
- currently binding performance standards for shipborne GNSS receivers set by the resolutions MSC.112(73) on GPS [2], MSC.113(73) on GLONASS [3], MSC.114(73) on DGPS and DGLONASS [4], MSC.115(73) on Combined GPS and GLONASS [5], MSC.233(82) on Galileo [6], MSC.379(93) on Beidou [7], MSC.401(95) on Multi System Shipborne Radionavigation Receivers [8] with MSC.432(98) amendments [9], MSC.449(99) on IRNSS [10], MSC.480(102) on QZSS [11], and the revised resolution A.1046(27) on World Wide Radionavigation System (WWRNS) [12],
- –
2. IMO GNSS Policy
- Accuracy: The degree of conformance between the estimated or measured parameter of a craft at a given time and its true parameter at that time (parameters in this context may be position coordinates, velocity, time, angle, etc.). Absolute accuracy (geodetic or geographic accuracy) is the accuracy of a position estimate with respect to the geographic or geodetic coordinates of the Earth in WGS84.
- Integrity: The ability to provide users with warnings within a specified time when the system should not be used for navigation.
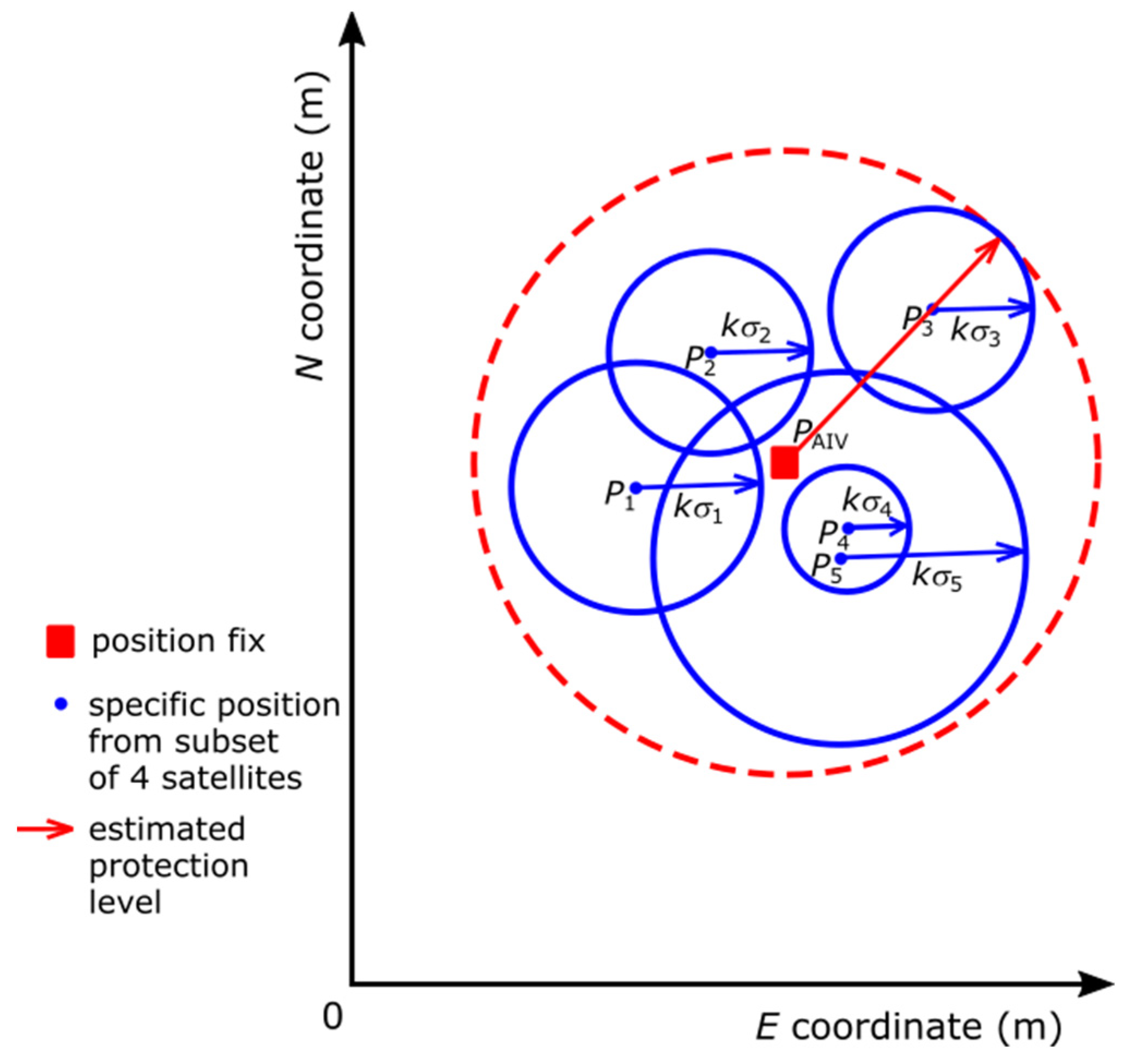
- Craft autonomous integrity monitoring (CAIM): This is a technique whereby various navigation sensor information available on the craft is autonomously processed to monitor the integrity of the navigation signals.
- Receiver autonomous integrity monitoring (RAIM): A technique whereby the redundant information available at a GNSS receiver is autonomously processed to monitor the integrity of the navigation signals.
- Continuity: The probability that, assuming a fault-free receiver, a user will be able to determine position with specified accuracy and is able to monitor the integrity of the determined position over the (short) time interval applicable for a particular operation within a limited part of the coverage area.
- Availability: The percentage of time that an aid, or system of aids, is performing a required function under stated conditions where system availability is the availability of a system to a user, including signal availability and the performance of the user’s receiver.
- Alert limit (AL): The maximum allowable error (or threshold value) in the measured position, during integrity monitoring, before an alarm is triggered.
- Time to alarm (TTA): The time elapsed between the occurrence of a failure in the system and its presentation on the ship’s bridge.
- Integrity risk (IR): The probability that a user will experience a position error larger than AL without an alarm being raised within the specified TTA at any instant of time at any location in the coverage area.
- Coverage: The coverage provided by a radionavigation system is that surface area or space volume in which the signals are adequate to permit the user to determine position to a specified level of performance.
- Latency: The time lag between the navigation observations and the presented navigation solution.
- Chart error (CE): Position errors in the chart caused by inaccuracies in surveying and chart generation, and by errors in the reference geodetic system.
- Navigation system error (NSE): The combined error of the CE and GNSS position estimate (PE) usually referenced to a common consistent reference point (CCRP) and formulated by (1):
- Vessel Technical Error (VTE): This is the difference between the indicated craft position and the indicated command or desired position. It is a measure of the accuracy the craft is controlled with. Components are cross track error (XTE) and along track error (ATE).
- Total System Error (TSE): The overall navigation performance can be described by the TSE. Assuming the contributions to TSE from NSE and VTE are random, the TSE can be described as (2) (Figure 1):

- Gross errors: Or “outliers”, are errors other than random errors or systematic errors. They are often large and, by definition, unpredictable. They are typically caused by sudden changes in the prevailing physical circumstances, by system faults or operator errors.
- Marginally detectable bias (MDB): The minimum size of gross error in an observation that may be detected with given probabilities of type 1 and type 2 errors. A type 1 error occurs when an observation without a gross error is wrongly rejected, and a type 2 error occurs when an observation with a gross error is wrongly accepted.
- Marginally detectable error (MDE): The maximum position-offset caused by a MDB in one of the observations.
- Reliability of a position fix: A measure of the propagation of a non-detected gross error (outlier) in an observation to the position fix. This “external” reliability is usually expressed in terms of MDE.
- 10 m accuracy (95%) and 25 m AL for most applications.
- 10 s TTA.
- 10−5 IR per 3 h.
- 99.97% continuity over 3 h.
- 99.8% overall availability (considered per 30 days).
| Area | Minimum Maritime User Requirements for General Navigation | |||||||
|---|---|---|---|---|---|---|---|---|
| System Level Parameters | Service Level Parameters | |||||||
| Absolute Accuracy | Integrity | Availability (%) (per 30 Days) | Continuity (%) (over 3 h) | Coverage | Fix Interval (s) | |||
| Horizontal 95% (m) | AL (m) | TTA (s) | IR (per 3 h) | |||||
| Ocean | 10 | 25 | 10 | 1.00 × 10−5 | 99.8 | - | Global | 1 |
| Coastal | 10 | 25 | 10 | 1.00 × 10−5 | 99.8 | - | Global | 1 |
| Port approach and restricted waters | 10 | 25 | 10 | 1.00 × 10−5 | 99.8 | 99.97 | Regional | 1 |
| Port | 1 | 2.5 | 10 | 1.00 × 10−5 | 99.8 | 99.97 | Local | 1 |
| Inland waterways | 10 | 25 | 10 | 1.00 × 10−5 | 99.8 | 99.97 | Regional | 1 |
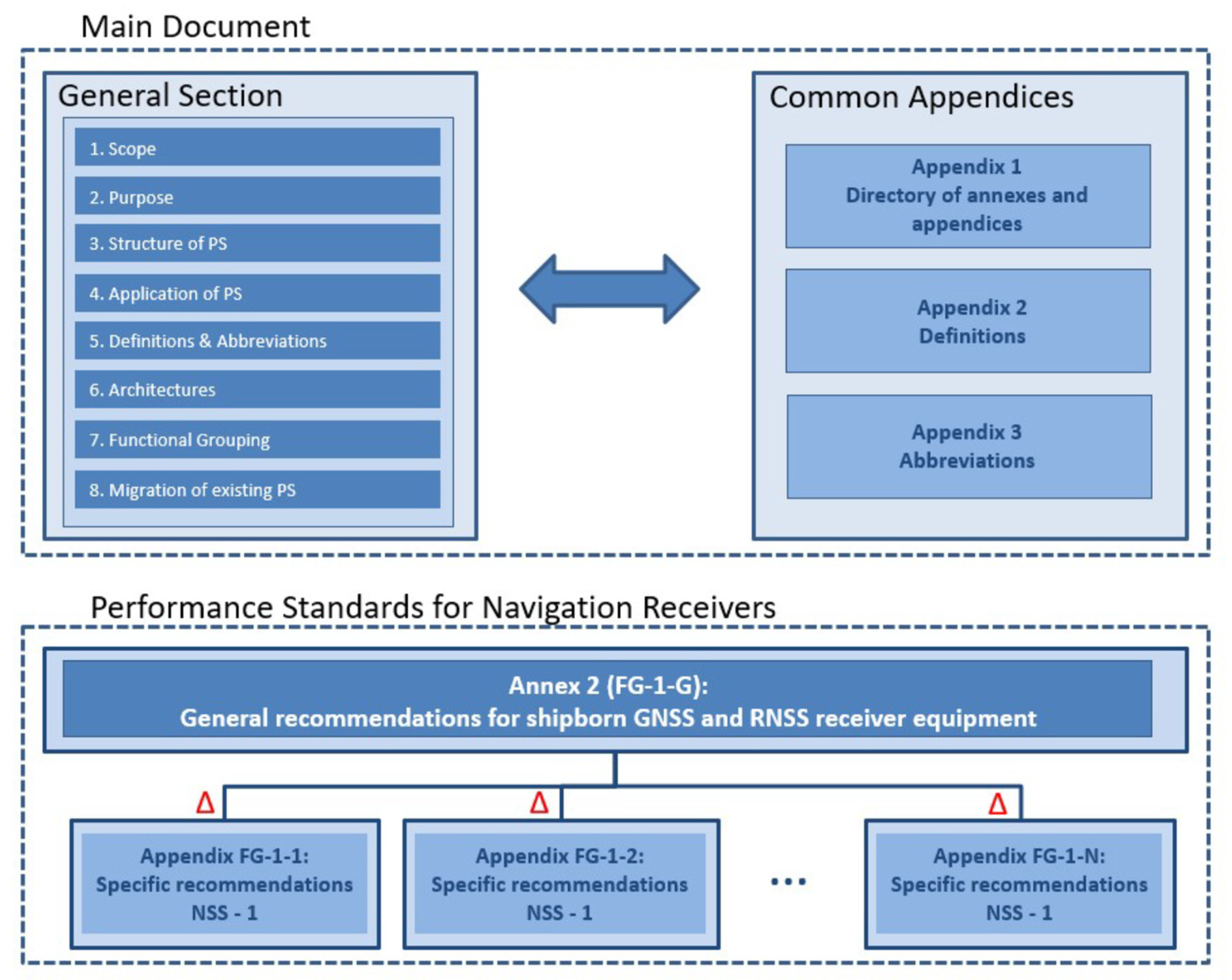
3. IMO GNSS Performance Standards
- Ocean waters where a radionavigation system is used to assist in the navigation of ships and provides positional information with an error not greater than 100 m with a probability of 95%, and an integrity warning of system malfunction, non-availability or discontinuity is provided to users as soon as practicable by Maritime Safety Information (MSI) systems;
- harbor entrances, harbor approaches, and coastal waters where a radionavigation system provides positional information with an error not greater than 10 m with a probability of 95%, and when the system is available, the service continuity should be ≥99.97% over a period of 15 min and an integrity warning of system malfunction, non-availability or discontinuity should be provided to users within 10 s.
- GPS: static accuracy such that the position of the antenna is determined to within 100 m (95%) with horizontal dilution of precision HDOP = 4 (or position dilution of precision PDOP = 6), dynamic accuracy such that the position of the ship is determined to within 100 m (95%) with HDOP = 4 (or PDOP = 6) under the conditions of sea states and ship’s motion likely to be experienced in ships;
- GLONASS: static accuracy such that the position of the antenna is determined to within 45 m (95%) with HDOP = 4 (PDOP = 6), dynamic accuracy such that the position of the antenna is determined to within 45 m (95%) with HDOP = 4 (PDOP = 6) under the conditions of sea states and ship’s motion likely to be experienced in ships;
- DGPS and DGLONASS: static and dynamic accuracies should be 10 m (95%);
- Combined GPS and GLONASS: static accuracy such that the position of the antenna is determined to within 35 m (95%) in non-differential mode and 10 m (95%) in differential mode with HDOP < 4 or PDOP < 6, dynamic accuracy such that the position of the ship is determined to within 35 m (95%) in non-differential mode and 10 m (95%) in differential mode with HDOP < 4 or PDOP < 6 under the conditions of sea states and ship’s motion likely to be experienced in ships;
- all systems receivers: integrity status and alarm for differential IALA modes.
- Have static and dynamic accuracy such that the position of the antenna is determined to within: (i) 15 m horizontal (95%) and 35 m vertical (95%) for single frequency operations on the L1 frequency; (ii) 10 m horizontal (95%) and 10 m vertical (95%) for dual frequency operations on L1 and E5a or L1 and E5b frequencies.
- Provide a warning within 5 s of loss of position or if a new position based on the information provided by the Galileo constellation has not been calculated for more than 1 s for conventional craft and 0.5 s for high-speed craft. Under such conditions, the last known position and the time of last valid fix, with the explicit indication of the state so that no ambiguity can exist, should be output until normal operation is resumed.
- Use RAIM to provide integrity performance appropriate to the operation being undertaken.
- Provide a self-test function.
- For receivers having the capability to process the Galileo Safety of Life Service, integrity monitoring and alerting algorithms should be based on a suitable combination of the Galileo integrity message and RAIM. The receiver should provide an alarm within 10 s TTA of the start of an event if an AL of 25 m Horizontal Alert Limit (HAL) is exceeded for a period of at least 3 s. The probability of detection of the event should be better that 99.999% over a 3 h period (IR ≤ 10−5 through 3 h).
- have static and dynamic accuracy such that the position of the antenna is determined to be within 25 m horizontally (95%) and 30 m vertically (95%).
- static accuracy such that, for the service area, where a horizontal dilution of precision (HDOP) is equal to or less than 6.7, the position of the antenna should be determined to within 50.4 m horizontal (95%), dynamic accuracy under the conditions of sea states and ships’ motion likely to be experienced in ships, such that for the service area where a HDOP is equal to or less than 6.7, the position of the antenna should be determined to within 50.4 m horizontal (95%).
4. Research and Future Development of Maritime GNSS Standards
- σE2 is the estimated variance of the antenna receiver Easting measurement in the local reference frame centered on the GPS antenna (East, North, Up, ENU) (m2);
- σN2 is the estimated variance of antenna receiver Northing measurement in the local reference frame (ENU) (m2);
- (1)
- Consistency tests using two sensors or model of ship’s movement.
- (2)
- Determination of PL by RAIM using such tests.
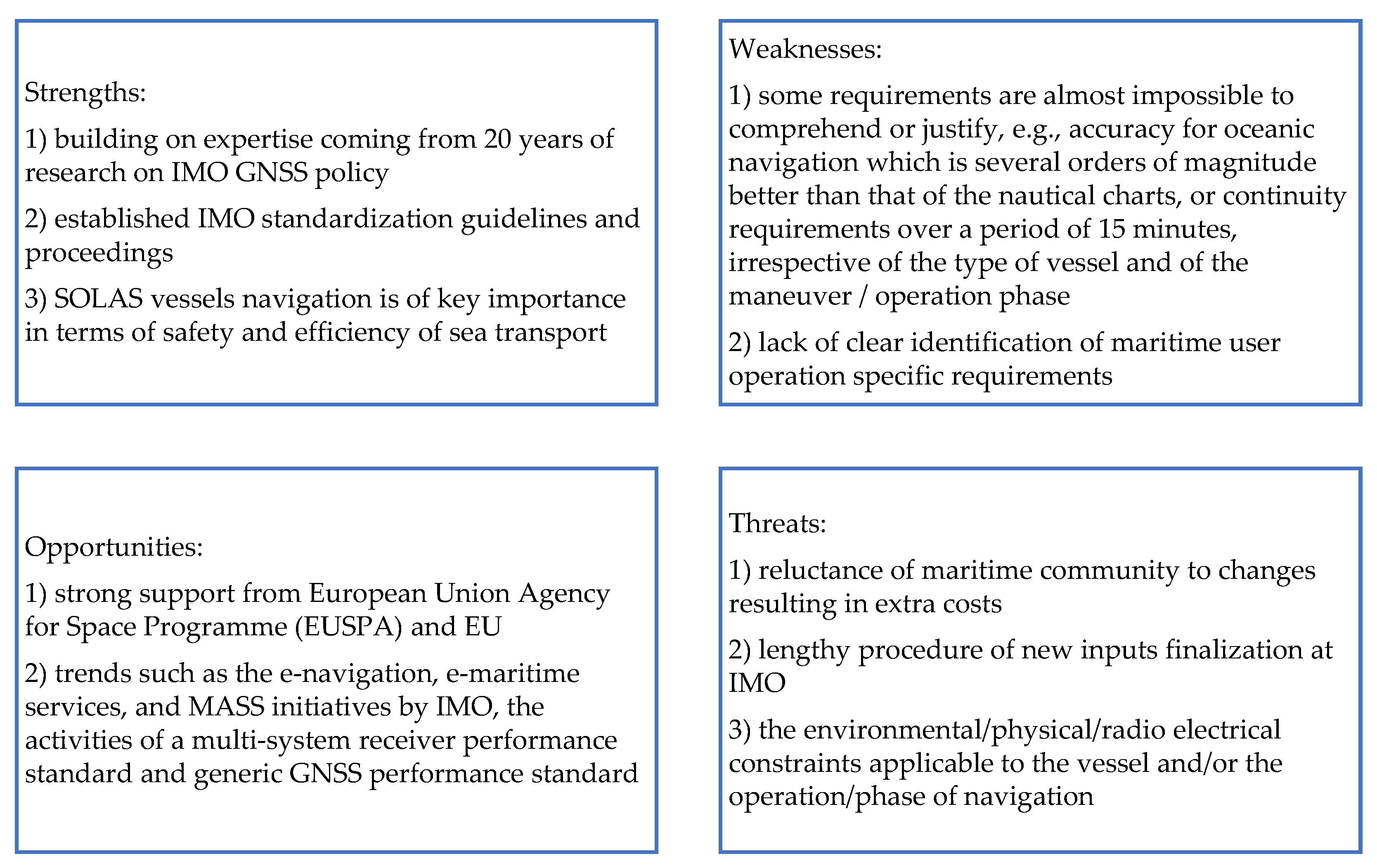
5. Discussion
- (a)
- a predictive annunciation that the GNSS data delivered to the shipborne system is going to exceed the required navigation performance thresholds specified for the current and planned operational tasks (GNSS alert status),
- (b)
- a reactive annunciation that the GNSS data delivered to the shipborne system has exceeded the required navigation performance thresholds specified for the current operational task (GNSS fault status),
- (c)
- in case of predictive annunciation, a fixed minimum time allowed for the caution flag to be provided to the user before the onset of a GNSS fault resulting in an unsafe condition,
- (d)
- in case of reactive annunciation, the maximum time allowed from the moment a GNSS fault resulting in an unsafe condition is detected to the moment that the shipborne system provides a warning flag to the user.
- (1)
- building awareness of maritime community on operation specific GNSS availability, continuity and integrity,
- (2)
- (3)
- approving recommended algorithms of maritime 2D and 3D protection levels,
- (4)
- further standardization of maritime GNSS receivers in scope of supported data formats and interoperability,
- (5)
- utilization of VHF Data Exchange System (VDES) evolving on AIS for augmentation,
- (6)
- utilization of GNSS services going beyond open ones, for instance Galileo Safety of Life or High Accuracy services,
- (7)
- fusion of GNSS data with INS positioning and attitude sensors,
- (8)
- fusion of GNSS data with ship accurate hydrodynamic model.
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
| Abbreviation or Acronym | Definition |
|---|---|
| AIS | Automatic Identification System |
| AIV | All in View |
| AL | Alert Limit |
| ATE | Along Track Error |
| BDS | Chinese GNSS Beidou |
| CAIM | Craft Autonomous Integrity Monitoring |
| CCRP | Common Consistent Reference Point |
| CE | Chart Error |
| CG | Correspondence Group |
| DGLONASS | Differential GLONASS |
| DGPS | Differential GPS |
| DoF | Degrees of Freedom |
| DOP | Dilution of Precision |
| DP | Dynamic Positioning |
| DRMS | Distance Root Mean Squared |
| ECDIS | Electronic Chart Display & Information System |
| EGNOS | European Geostationary Navigation Overlay System |
| ENU | East, North, Up Reference |
| EU | European Union |
| Galileo | European GNSS |
| GBAS | Ground Based Augmentation System |
| GLONASS | Russian GNSS |
| GNSS | Global Navigation Satellite System |
| GPS | US Global Positioning System |
| HAL | Horizontal Alert Limit |
| HDOP | Horizontal Dilution of Precision |
| HPL | Horizontal Protection Level |
| IALA | International Association of Marine Aids to Navigation and Lighthouse Authorities |
| ICAO | UN International Civil Aviation Organization |
| IMO | UN International Maritime Organization |
| IR | Integrity Risk |
| LEO | Low Earth Orbit |
| LPME | Line of Position Mean Error |
| MDB | Marginally Detectable Bias |
| MDE | Marginally Detectable Error |
| MSC | IMO Maritime Safety Committee |
| MSI | Maritime Safety Information |
| MVPA | Maritime Vessel Protection Area |
| NCSR | IMO Navigation, Communication, and Search & Rescue Subcommittee |
| NSE | Navigation System Error |
| PDOP | Position Dilution of Precision |
| PE | Position Estimate / Error or Protection Ellipse |
| PL | Protection Level |
| PNT | Position, Navigation, Timing |
| PNT DP | PNT Data Processing |
| PPP | Precise Point Positioning |
| QZSS | Japanese Quasi Zenith Satellite System |
| RAIM | Receiver Autonomous Integrity Monitoring |
| RF | Radio Frequency |
| RNSS | Regional Navigation Satellite System |
| RTCA | Radio Technical Commission for Aeronautics |
| RTCM | Radio Technical Commission for Maritime Services |
| RTK | Real Time Kinematics |
| SBAS | Satellite Based Augmentation System |
| SOLAS | IMO Convention on Safety of Life at Sea |
| SV | Satellite Vehicle |
| SWOT | Analysis of Strengths, Weaknesses, Opportunities, Threats |
| TSE | Total System Error |
| TTA | Time to Alarm |
| UN | United Nations |
| VDES | VHF Data Exchange System |
| VHF | Very High Frequency |
| VTE | Vessel Technical Error |
| VTS | Vessel Traffic Services |
| WAAS | US Wide Area Augmentation System |
| WWRNS | Worldwide Radionavigation System |
| XTE | Cross Track Error |
Appendix B
- λa and λb represent the eigenvalues of the covariance matrix.
- vλ
- represents the eigenvector of the covariance matrix that corresponds to the largest eigenvalue of x and y coordinate respectively.
References
- IMO. A.666(16), World Wide Radionavigation System; International Maritime Organization: London, UK, 1989. [Google Scholar]
- IMO. MSC.112(73), Adoption of the Revised Performance Standards for Shipborne Global Positioning System (GPS) Receiver Equipment; International Maritime Organization: London, UK, 2000. [Google Scholar]
- IMO. MSC.113(73), Adoption of the Revised Performance Standards for Shipborne GLONASS Receiver Equipment; International Maritime Organization: London, UK, 2000. [Google Scholar]
- IMO. MSC.114(73), Adoption of the Revised Performance Standards for Shipborne DGPS and DGLONASS Maritime Radio Beacon Receiver Equipment; International Maritime Organization: London, UK, 2000. [Google Scholar]
- IMO. MSC.115(73), Adoption of the Revised Performance Standards for Shipborne Combined GPS/GLONASS Receiver Equipment; International Maritime Organization: London, UK, 2000. [Google Scholar]
- IMO. MSC.233(82), Adoption of the Performance Standards for Shipborne Galileo Receiver Equipment; International Maritime Organization: London, UK, 2006. [Google Scholar]
- IMO. MSC.379(93), Performance Standards for Shipborne Beidou Satellite Navigation System (BDS) Receiver Equipment; International Maritime Organization: London, UK, 2014. [Google Scholar]
- IMO. MSC.401(95), Performance Standards for Multi-System Shipborne Radionavigation Receivers; International Maritime Organization: London, UK, 2015. [Google Scholar]
- IMO. MSC.432(98), Amendments to Performance Standards for Multi-System Shipborne Radionavigation Receivers (RESOLUTION MSC.401(95)); International Maritime Organization: London, UK, 2017. [Google Scholar]
- IMO. MSC.449(99), Performance Standards for Shipborne Indian Regional Navigation Satellite System (IRNSS) Receiver Equipment; International Maritime Organization: London, UK, 2018. [Google Scholar]
- IMO. MSC.480(102), Performance Standards for Shipborne Japanese Quasi-Zenith Satellite System (QZSS) Receiver Equipment; International Maritime Organization: London, UK, 2020. [Google Scholar]
- IMO. A.1046(27), World Wide Radionavigation System; International Maritime Organization: London, UK, 2011. [Google Scholar]
- IMO. A.915(22), Revised Maritime Policy and Requirements for A Future GNSS; International Maritime Organization: London, UK, 2001. [Google Scholar]
- IMO. MSC.1/Circ.1575, Guidelines for Shipborne Position, Navigation and Timing (PNT) Data Processing; International Maritime Organization: London, UK, 2017. [Google Scholar]
- IMO. A.860(20), Maritime Policy for A Future Global Navigation Satellite System (GNSS); International Maritime Organization: London, UK, 1997. [Google Scholar]
- RTCM. RTCM 10403.3, Differential GNSS (Global Navigation Satellite Systems) Services-Version 3 + Amendment 3; Radio Technical Commission for Maritime Services: Washington, DC, USA, 2021; Available online: https://rtcm.myshopify.com/products/rtcm-10403-3-differential-gnss-global-navigation-satellite-systems-services-version-3-amendment-2-may-20-2021?_pos=2&_sid=4b86b96f6&_ss=r (accessed on 19 October 2022).
- Walter, T.; Blanch, J.; Choi, M.J.; Reid, T.; Enge, P. Incorporating GLONASS into Aviation RAIM Receivers. In Proceedings of the 2013 International Technical Meeting of The Institute of Navigation, San Diego, CA, USA, 27–29 January 2013; pp. 239–249. [Google Scholar]
- Teunissen, P.J.G.; Imparato, D.; Tiberius, C.C.J.M. Does RAIM with Correct Exclusion Produce Unbiased Positions? Sensors 2017, 17, 1508. [Google Scholar] [CrossRef] [PubMed]
- ICAO. Standards and Recommended Practices (SARPs), Volume 1–Annex 10, Amendments 1-81; International Civil Aviation Organization: Montreal, Canada, 2006. [Google Scholar]
- Sabatini, R.; Moore, T. A New Avionics-Based GNSS Integrity Augmentation System: Part 1–Fundamentals. J. Navig. 2013, 66, 363–384. [Google Scholar] [CrossRef]
- Sabatini RMoore, T.; Hill, C. A New Avionics-Based GNSS Integrity Augmentation System: Part 2–Integrity Flags. J. Navig. 2013, 66, 501–522. [Google Scholar] [CrossRef]
- RTCA. Minimum Operational Performance Standards for Global Positioning System/Satellite-Based Augmentation System Airborne Equipment. DO-229E, SC-159; Radio Technical Commission for Aeronautics: Washington, DC, USA, 2016; Available online: https://my.rtca.org/productdetails?id=a1B3600000211rIEAQ (accessed on 19 October 2022).
- IEC. Maritime Navigation and Radio-communication Equipment and Systems–Global Navigation Satellite Systems (GNSS)–Part 4: Ship-borne DGPS and DGLONASS Maritime Radio Beacon Receiver Equipment–Performance Requirements, Methods of Testing and Required Test Results, International Electrotechnical Commission, Reference number IEC 61108-4:2004(E), 1st ed.; International Electrotechnical Commission: Geneva, Switzerland, 2004; Available online: https://webstore.iec.ch/publication/4518 (accessed on 20 August 2022).
- IMO. A.953(23), World Wide Radionavigation System; International Maritime Organization: London, UK, 2003. [Google Scholar]
- Lopez-Martinez, M.; Alvarez, J.-M.; Lorenzo, J.-M.; Daroca, C.G. SBAS/EGNOS for Maritime. J. Mar. Sci. Eng. 2020, 8, 764. [Google Scholar] [CrossRef]
- Schlüter, S.; Hoque, M.M. An SBAS Integrity Model to Overbound Residuals of Higher-Order Ionospheric Effects in the Ionosphere-Free Linear Combination. Remote Sens. 2020, 12, 2467. [Google Scholar] [CrossRef]
- Innac, A.; Angrisano, A.; Del Pizzo, S.; Cappello, G.; Gaglione, S. The EGNOS Augmentation in Maritime Navigation. Sensors 2022, 22, 775. [Google Scholar] [CrossRef] [PubMed]
- EC. European Radio Navigation Plan, ERNP ver. 1.1. 2018. Available online: https://ec.europa.eu/docsroom/documents/33024 (accessed on 20 August 2022).
- Klepsvik, J.; Ober, P.; Baldauf, M. A Critical Look at the IMO Requirements for GNSS. In Proceedings of the 20th International Technical Meeting of the Satellite Division of The Institute of Navigation (ION GNSS 2007), Fort Worth, TX, USA, September 2007; pp. 1931–1942. [Google Scholar]
- Hargreaves, C.; Williams, P. Maritime Integrity Concept. In Proceedings of the European Navigation Conference ENC 2018, Abstracts and Technical Papers, Chalmers, Gothenburg, Sweden, 14–17 May 2018; pp. 117–124. Available online: https://research.chalmers.se/en/publication/503272 (accessed on 20 August 2022).
- Zalewski, P. Integrity Concept for Maritime Autonomous Surface Ships’ Position Sensors. Sensors 2020, 20, 2075. [Google Scholar] [CrossRef] [PubMed]
- Office of the US. Department of Defense, GPS SPS Performance Standard, 5th Ed. 2020. Available online: https://www.gps.gov/technical/ps/ (accessed on 31 August 2022).
- Chen, L.; Gao, W.; Hu, Z.; Cao, Y.; Pei, L.; Liu, C.; Zhou, W.; Liu, X.; Chen, L.; Yang, R. BDS-3 Integrity Risk Modeling and Probability Evaluation. Remote Sens. 2022, 14, 944. [Google Scholar] [CrossRef]
- Du, Z.; Wu, Z.; Yang, J. Error Ellipsoid Analysis for the Diameter Measurement of Cylindroid Components Using a Laser Radar Measurement System. Sensors 2016, 16, 714. [Google Scholar] [CrossRef] [PubMed]
- Hilton, S.; Cairola, F.; Gardi, A.; Sabatini, R.; Pongsakornsathien, N.; Ezer, N. Uncertainty Quantification for Space Situational Awareness and Traffic Management. Sensors 2019, 19, 4361. [Google Scholar] [CrossRef] [PubMed]
- Specht, M. Determination of Navigation System Positioning Accuracy Using the Reliability Method Based on Real Measurements. Remote Sens. 2021, 13, 4424. [Google Scholar] [CrossRef]
- IMO. NCSR 4/INF.16/Rev.2.2017, Report on EGNOS Application as Effective Augmentation System for Marine Positioning in Inland and Pilot Navigation; International Maritime Organization: London, UK, 2017. [Google Scholar]
- Tossaint, M.; Samson, J.; Toran, F.; Ventura-Traveset, J.; Hernández-Pajares, M.; Juan, J.M.; Sanz, J.; Ramos-Bosch, P. The Stanford-ESA Integrity Diagram: A new tool for the user domain SBAS integrity assessment. Navig. J. Inst. Navig. 2007, 54, 153–162. [Google Scholar] [CrossRef]
- Porretta, M.; Jimenez-Banos, D.; Crisci, M.; Solari, G.; Fiumara, A.; Hein, G. GNSS Evolutions for Maritime An Incremental Approach, working paper, InsideGNSS, May/June. 2016. Available online: https://insidegnss-com.exactdn.com/wp-content/uploads/2018/01/mayjune16-WP.pdf (accessed on 20 August 2022).
- Zalewski, P. Real-time GNSS Spoofing Detection in Maritime Code Receivers. Sci. J. Marit. Univ. Szczec. 2014, 38, 118–124. [Google Scholar]
- Zou, X.; Li, Z.; Wang, Y.; Deng, C.; Li, Y.; Tang, W.; Fu, R.; Cui, J.; Liu, J. Multipath Error Fusion Modeling Methods for Multi-GNSS. Remote Sens. 2021, 13, 2925. [Google Scholar] [CrossRef]
- Krzykowska-Piotrowska, K.; Dudek, E.; Wielgosz, P.; Milanowska, B.; Batalla, J.M. On the Correlation of Solar Activity and Troposphere on the GNSS/EGNOS Integrity. Fuzzy Logic Approach. Energies 2021, 14, 4534. [Google Scholar] [CrossRef]
- IMCA. Guidance on Satellite-Based Positioning systems for Offshore Applications, International Marine Contractors Association, Rev. 0.1. September 2021. Available online: https://www.imca-int.com/product/guidance-on-satellite-based-positioning-systems-for-offshore-applications/ (accessed on 20 August 2022).
- Gottschalg, G.; Leinen, S. Comparison and Evaluation of Integrity Algorithms for Vehicle Dynamic State Estimation in Different Scenarios for an Application in Automated Driving. Sensors 2021, 21, 1458. [Google Scholar] [CrossRef] [PubMed]
- IMO. NCSR 9/5, Generic Performance Standards for Shipborne Satellite Navigation System Receiver Equipment, Report of the Correspondence Group on Development of Generic Performance Standards for Shipborne Satellite Navigation System Receiver Equipment; International Maritime Organization: London, UK, 2022. [Google Scholar]
- Koch, P.; Gewies, S. Worldwide Availability of Maritime Medium-Frequency Radio Infrastructure for R-Mode-Supported Navigation. J. Mar. Sci. Eng. 2020, 8, 209. [Google Scholar] [CrossRef]
- Bronk, K.; Koncicki, P.; Lipka, A.; Niski, R.; Wereszko, B. Concept, Signal Design And Measurement Studies of the R-Mode Baltic System. Navig.–J. Inst. Navig. 2021, 68, 465–483. [Google Scholar] [CrossRef]
- IALA. IALA Guideline G1129 The Retransmission of SBAS Corrections Using MF-Radio Beacon and AIS, Edition 2.0 urn:mrn:iala:pub:g1129:ed2.0. 2022. Available online: https://www.iala-aism.org/product/g1129/ (accessed on 20 August 2022).
- RTCM. RTCM 10410.1, Standard for Networked Transport of RTCM via Internet Protocol (Ntrip) Version 2.0 with Amendment 2; Radio Technical Commission for Maritime Services: Washington, DC, USA, January 2021; Available online: https://rtcm.myshopify.com/products/rtcm-10410-1-standard-for-networked-transport-of-rtcm-via-internet-protocol-ntrip-version-2-0-with-amendment-1-june-28-2011 (accessed on 19 October 2022).
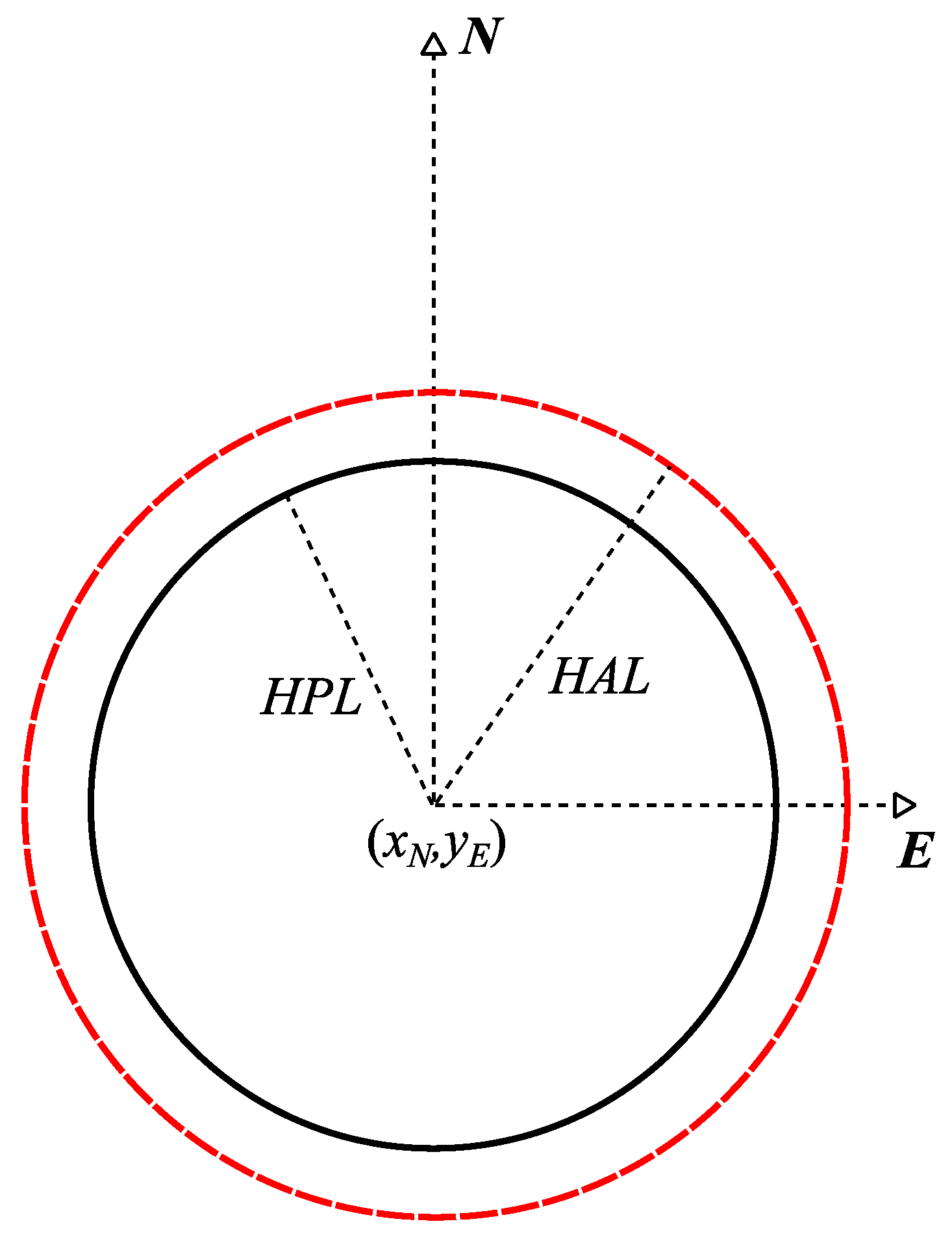

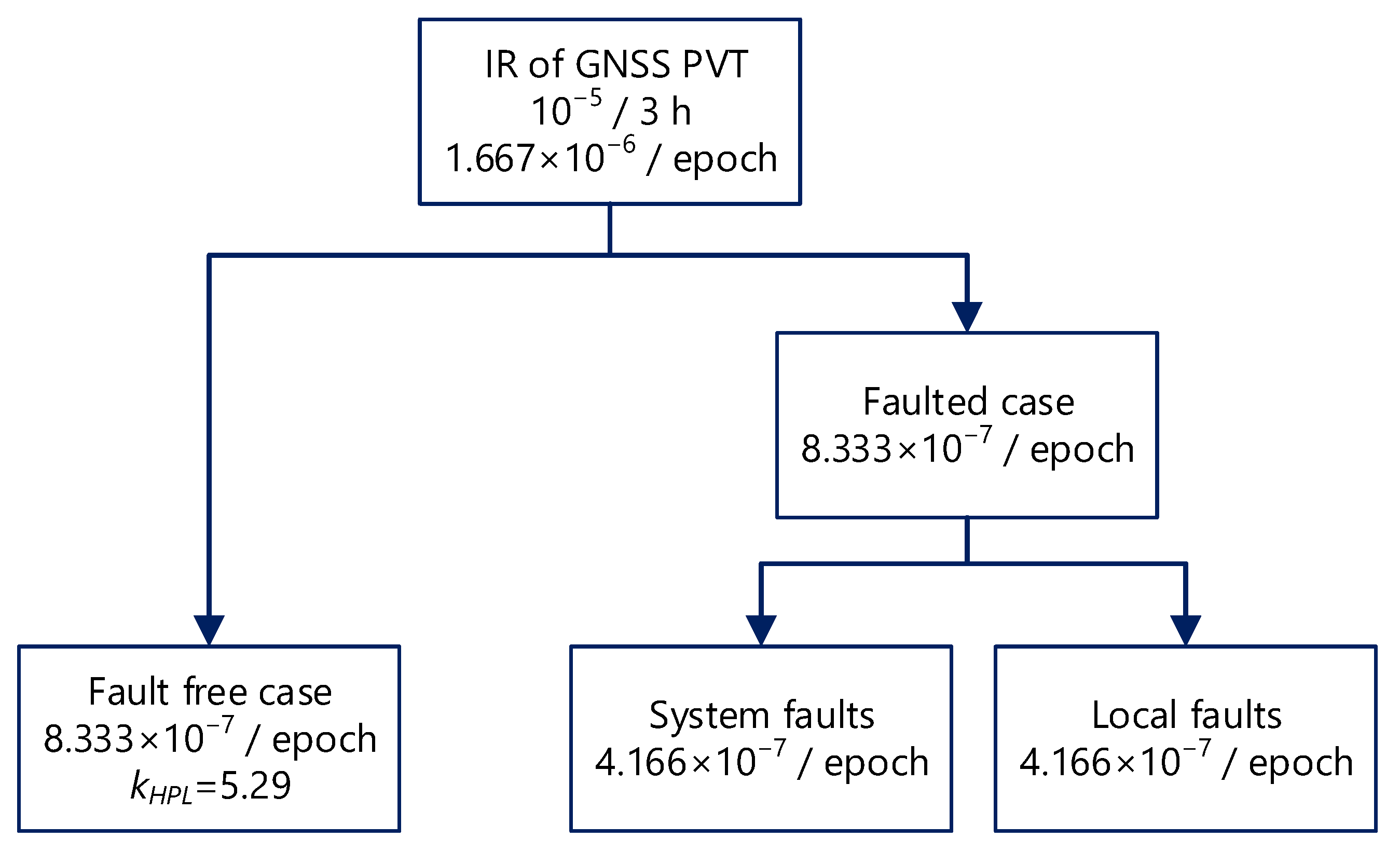

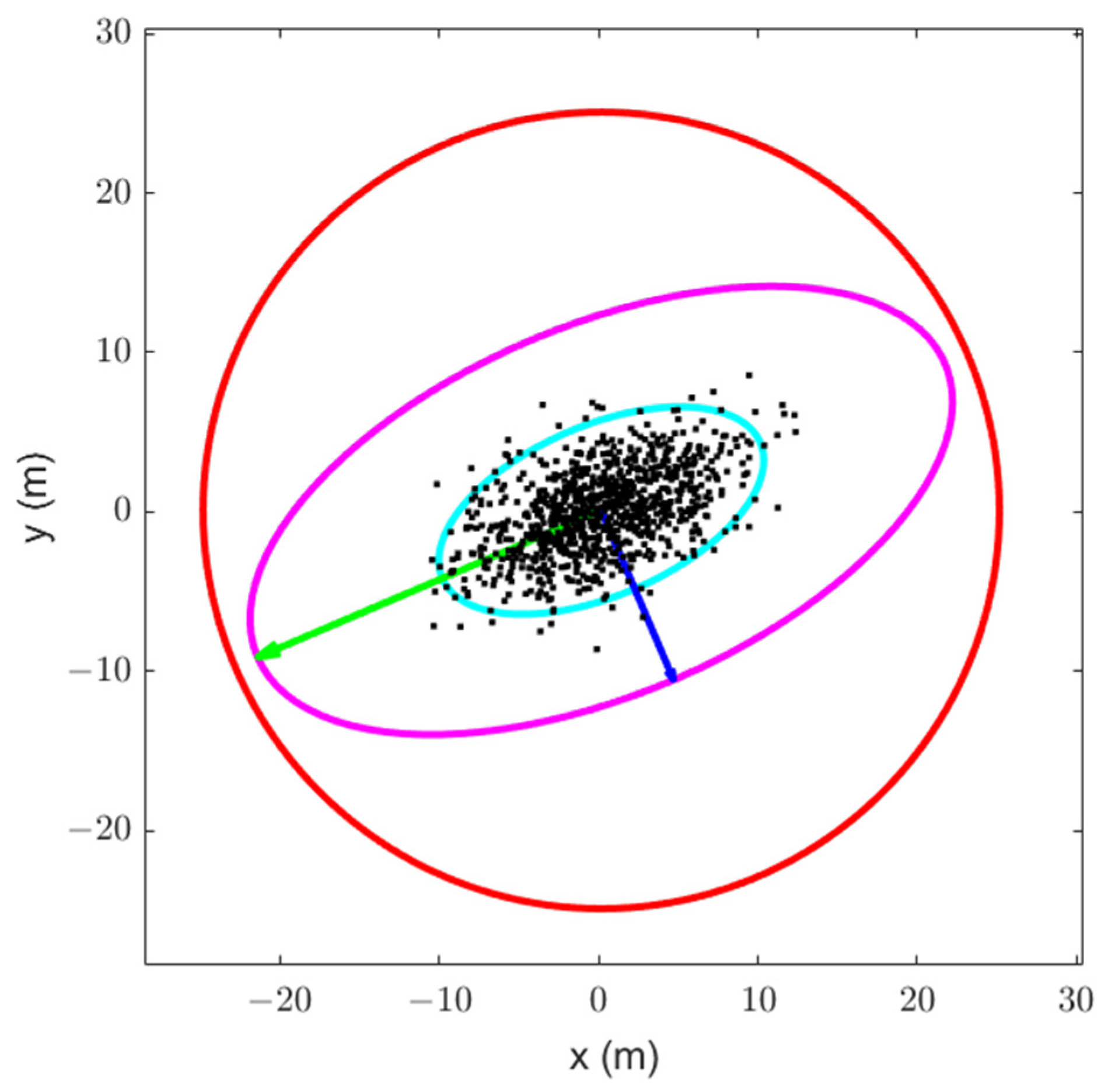
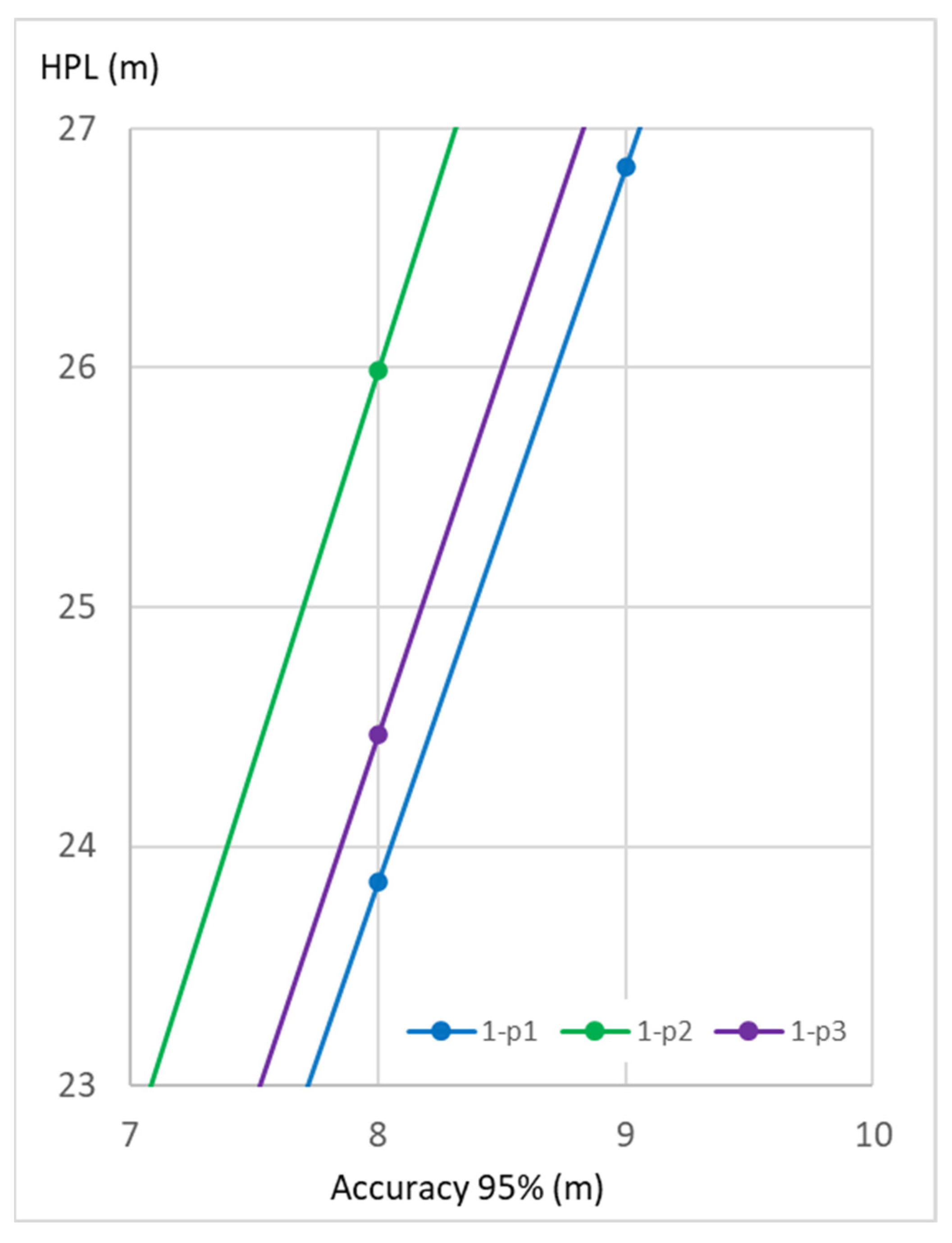
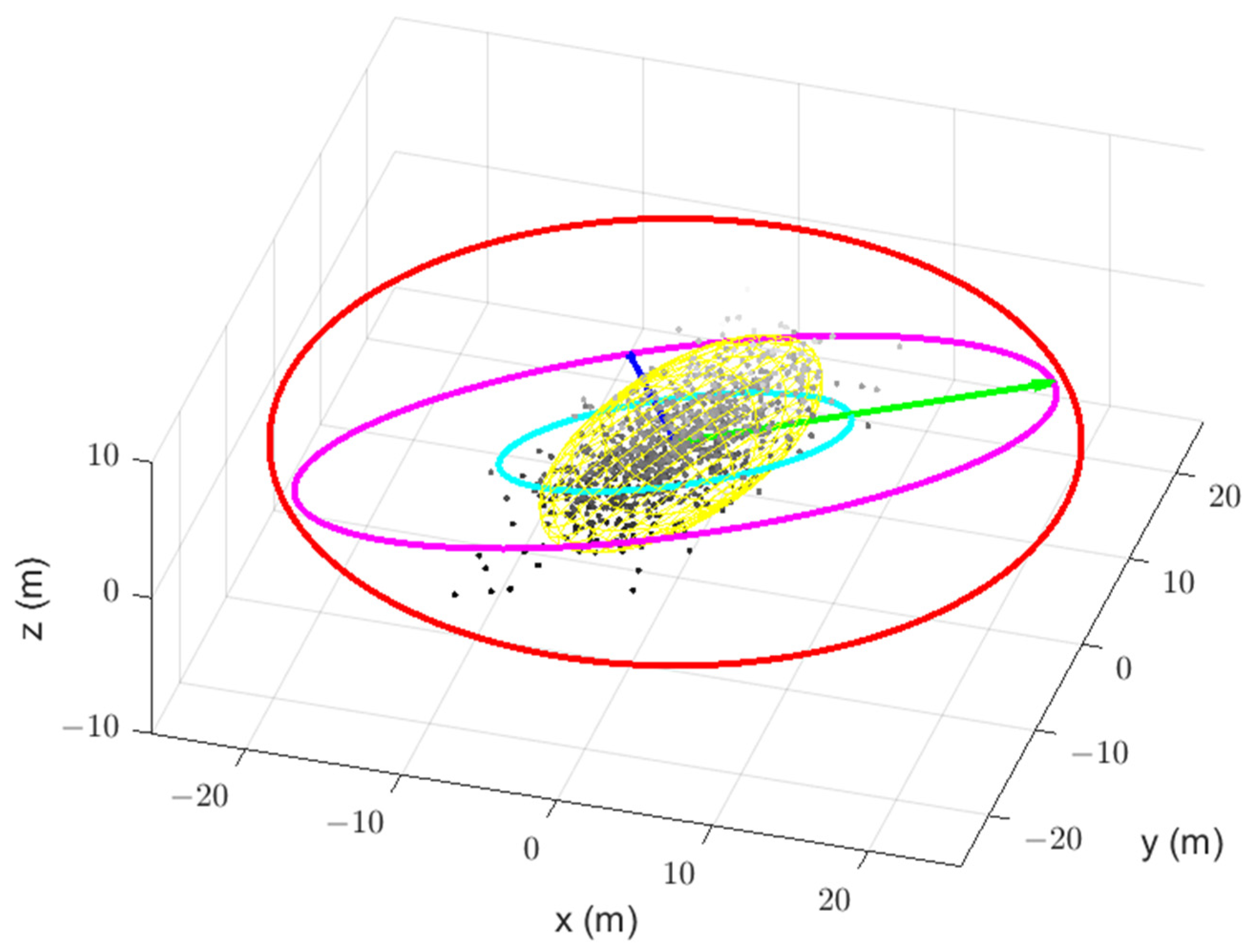
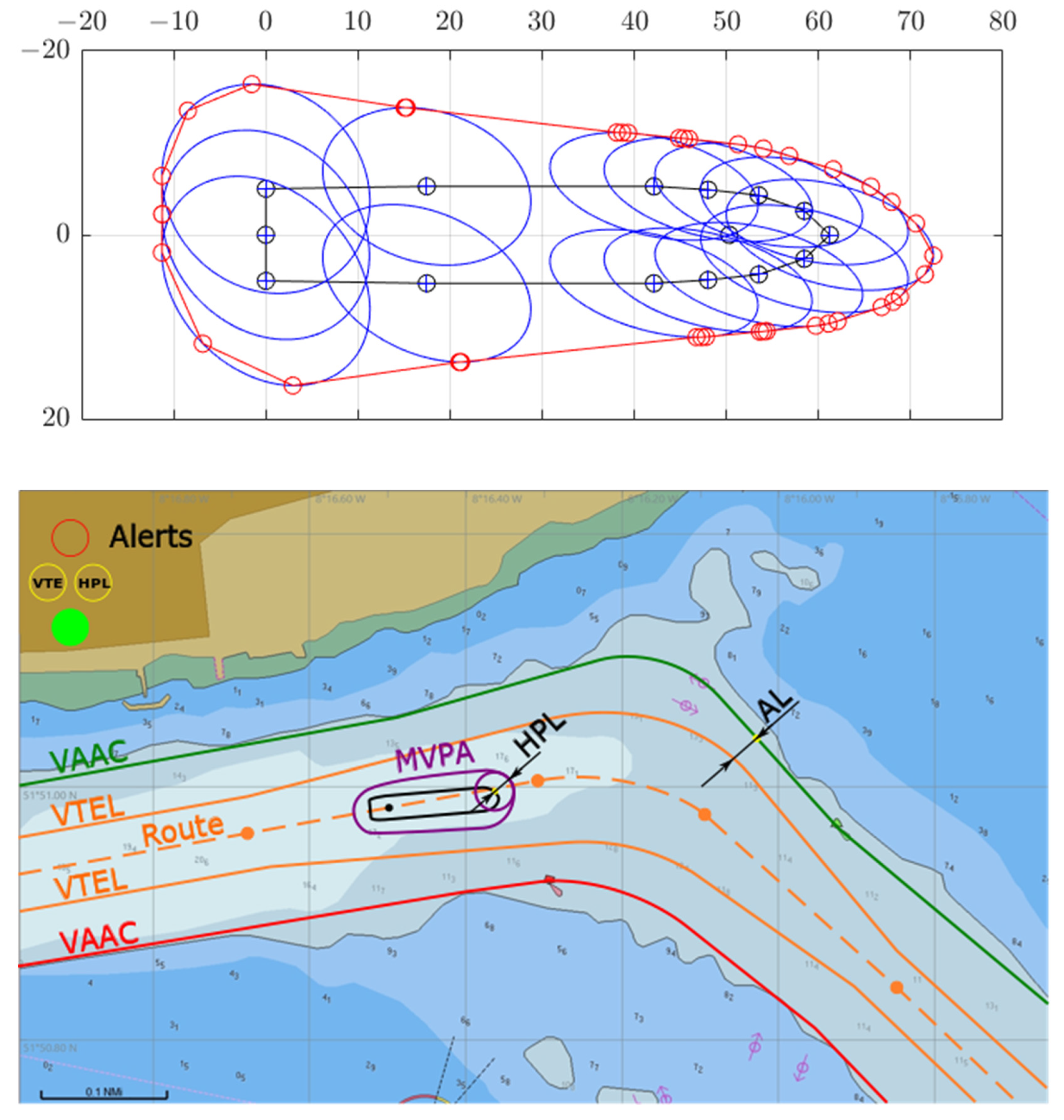

| Parameter | Unit | Value |
|---|---|---|
| Number of recorded positions | 900 | |
| Average x relative coordinate | m | 0.16 |
| Average y relative coordinate | m | 0.08 |
| 1σ of x coordinates | m | 4.17 |
| 1σ of y coordinates | m | 2.67 |
| 95% ellipse semimajor axis | m | 10.86 |
| 95% ellipse semiminor axis | m | 5.35 |
| 1.73 × DRMS (95% horizontal error) | m | 8.55 |
| HPL (99.99992%) | m | 26.16 |
| PL ellipse (PE) semimajor axis | m | 23.47 |
| PL ellipse (PE) semiminor axis | m | 11.57 |
| AL | m | 25 |
| Position/Time Accuracy Standard | Value | Conditions and Constraints |
|---|---|---|
| Global Average Position Accuracy | ≤8 m 95% Horizontal Error ≤13 m 95% Vertical Error | Defined for a position/time solution meeting the representative user conditions. Position/time solution is available. |
| Worst Site Position Accuracy | ≤15 m 95% Horizontal Error ≤33 m 95% Vertical Error |
| p (%) | 1-p | Accuracy (m) | HPL1 (m) | HPL2 (m) | HPL3 (m) |
|---|---|---|---|---|---|
| 95% | 1-p1 | 1-p2 | 1-p3 | ||
| 99.999833% | 1.67 × 10−6 | 1 | 2.98174975 | 3.24828662 | 3.05843521 |
| 99.999986% | 1.39 × 10−7 | 2 | 5.96349951 | 6.49657324 | 6.11687042 |
| 99.999917% | 8.33 × 10−7 | 3 | 8.94524926 | 9.74485986 | 9.17530563 |
| 4 | 11.92699901 | 12.99314647 | 12.23374084 | ||
| 5 | 14.90874877 | 16.24143309 | 15.29217605 | ||
| 6 | 17.89049852 | 19.48971971 | 18.35061126 | ||
| 7 | 20.87224827 | 22.73800633 | 21.40904647 | ||
| 8 | 23.85399803 | 25.98629295 | 24.46748169 | ||
| 9 | 26.83574778 | 29.23457957 | 27.52591690 | ||
| 10 | 29.81749753 | 32.48286618 | 30.58435211 |
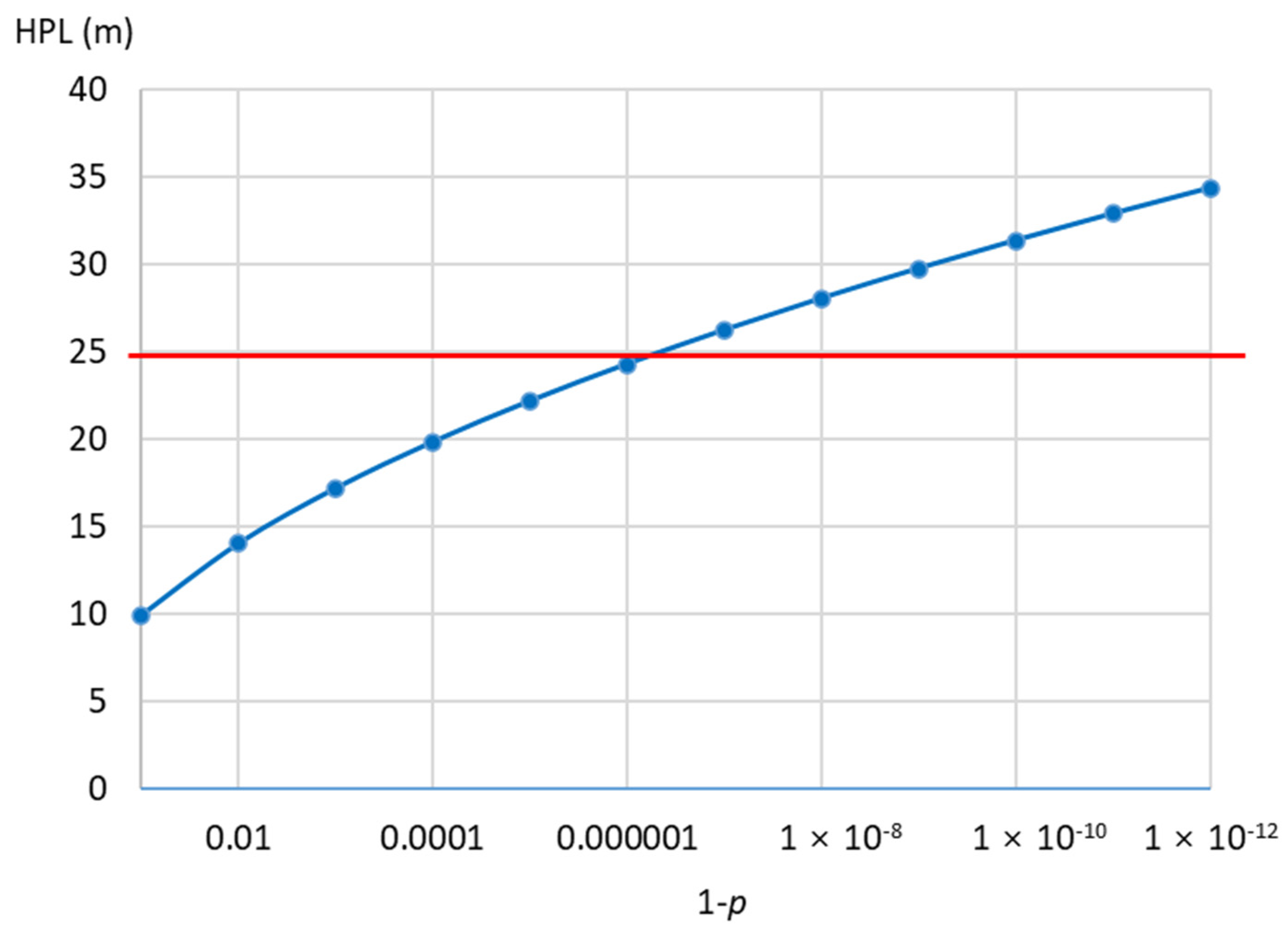
| Accuracy (m) | 1-p | HPL |
|---|---|---|
| 95% (k = 2.4477) | ||
| 8 | 1.00 × 10−2 | 14.03400813 |
| 1.00 × 10−3 | 17.18807949 | |
| 1.00 × 10−4 | 19.84708464 | |
| 1.00 × 10−5 | 22.18971520 | |
| 1.00 × 10−6 | 24.30761512 | |
| 1.00 × 10−7 | 26.25522510 | |
| 1.00 × 10−8 | 28.06801626 | |
| 1.00 × 10−9 | 29.77062697 | |
| 1.00 × 10−10 | 31.38099613 | |
| 1.00 × 10−11 | 32.91266641 | |
| 1.00 × 10−12 | 34.37617273 |
| Parameter | Unit | Value |
|---|---|---|
| Number of recorded positions | 900 | |
| Average x relative coordinate | m | −0.15 |
| Average y relative coordinate | m | −0.06 |
| Average z relative coordinate | m | −0.19 |
| 1σ of x coordinates | m | 3.63 |
| 1σ of y coordinates | m | 2.79 |
| 1σ of z coordinates | m | 2.77 |
| 95% ellipsoid a axis | m | 11.21 |
| 95% ellipsoid b axis | m | 4.75 |
| 95% ellipsoid c axis | m | 4.82 |
| 1.73 × DRMS (95% horizontal error) | m | 7.92 |
| 1.56 × MRSE (95% 3D sphere) | m | 8.35 |
| HPL (99.99992%) | m | 24.21 |
| PL ellipsoid (PE) a axis | m | 24.24 |
| PL ellipsoid (PE) b axis | m | 10.27 |
| PL ellipsoid (PE) c axis | m | 10.42 |
| AL | m | 25 |
| Parameter | Description | Effect on Position | Reason of Change |
|---|---|---|---|
| HDOP | Loss in precision due to satellite geometry. | Normally slow change in solution in biased direction due to changing geometry; can introduce a small jump in position when constellation changes. | Change in SV position, constellation change |
| LPME (Line of Position Mean Error) | Mean of the residuals from Least Squares process. | Position becomes noisier as residuals increase. | Multipath or noisy pseudo-ranges. |
| No. of SVs | Number of satellite vehicles (SV) used in position. | Change in geometry, reduced multi GNSS and available quality control. | Masking; RF interference; faulty hardware. |
| Age of data | Average age of differential correction data being used. | Gradual position wander de-grading little up to 15 s. At the point where corrections are no longer used (usually 20–30 s), position could jump up to 10 m. | Degradation or loss in differential link |
| Signal quality | Signal and augmentation signal quality indicator based on RF quality or quality and amount of data received. | Average age of data increases. Health flags raised. | Shadowing, RF interference, faulty hardware, jamming, spoofing, degradation, or loss in augmentation link. |
| F-Test | F-Test tests the stochastic model and detects a solution with pseudo-range variances performing outside expectations. | First process in determining the presence of blunders or outliers in the observations. | Noisy pseudo-range, a-priori variances incorrectly set in DGNSS software (e.g., too optimistic). |
| W-Test (unit variance) | If the F-test fails, the W-test is used to locate the pseudo-range(s) that is (are) causing the failure of the F-test. | When an outlier is detected, it is rejected, improving the precision of the position. The number of SVs used will be reduced and will cause the geometry to degrade. | Pseudo-range values exceed the expected ones (e.g., bias in pseudo-range). |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zalewski, P.; Bąk, A.; Bergmann, M. Evolution of Maritime GNSS and RNSS Performance Standards. Remote Sens. 2022, 14, 5291. https://doi.org/10.3390/rs14215291
Zalewski P, Bąk A, Bergmann M. Evolution of Maritime GNSS and RNSS Performance Standards. Remote Sensing. 2022; 14(21):5291. https://doi.org/10.3390/rs14215291
Chicago/Turabian StyleZalewski, Paweł, Andrzej Bąk, and Michael Bergmann. 2022. "Evolution of Maritime GNSS and RNSS Performance Standards" Remote Sensing 14, no. 21: 5291. https://doi.org/10.3390/rs14215291
APA StyleZalewski, P., Bąk, A., & Bergmann, M. (2022). Evolution of Maritime GNSS and RNSS Performance Standards. Remote Sensing, 14(21), 5291. https://doi.org/10.3390/rs14215291









