Noise Robust High-Speed Motion Compensation for ISAR Imaging Based on Parametric Minimum Entropy Optimization
Abstract
:1. Introduction
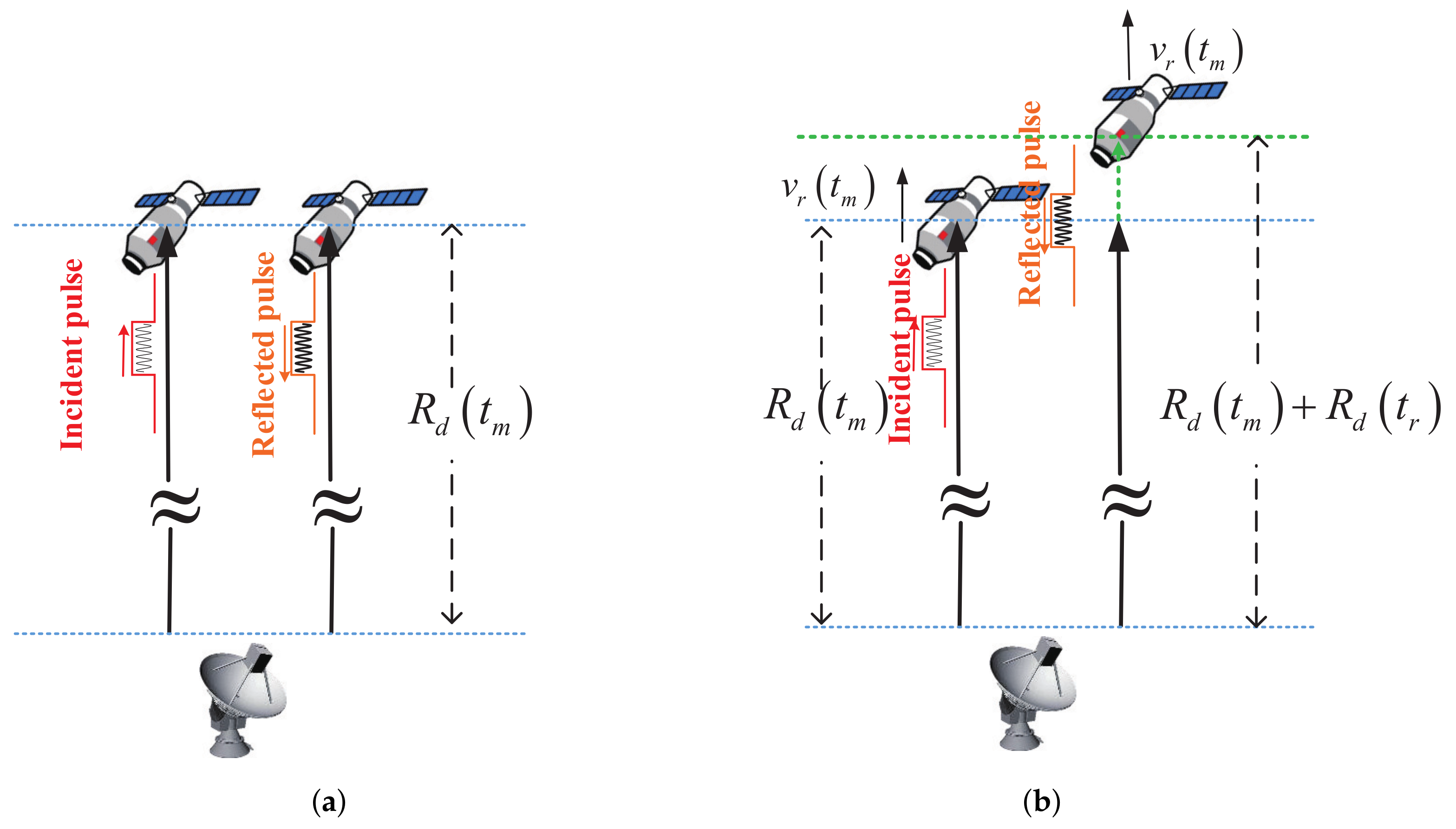
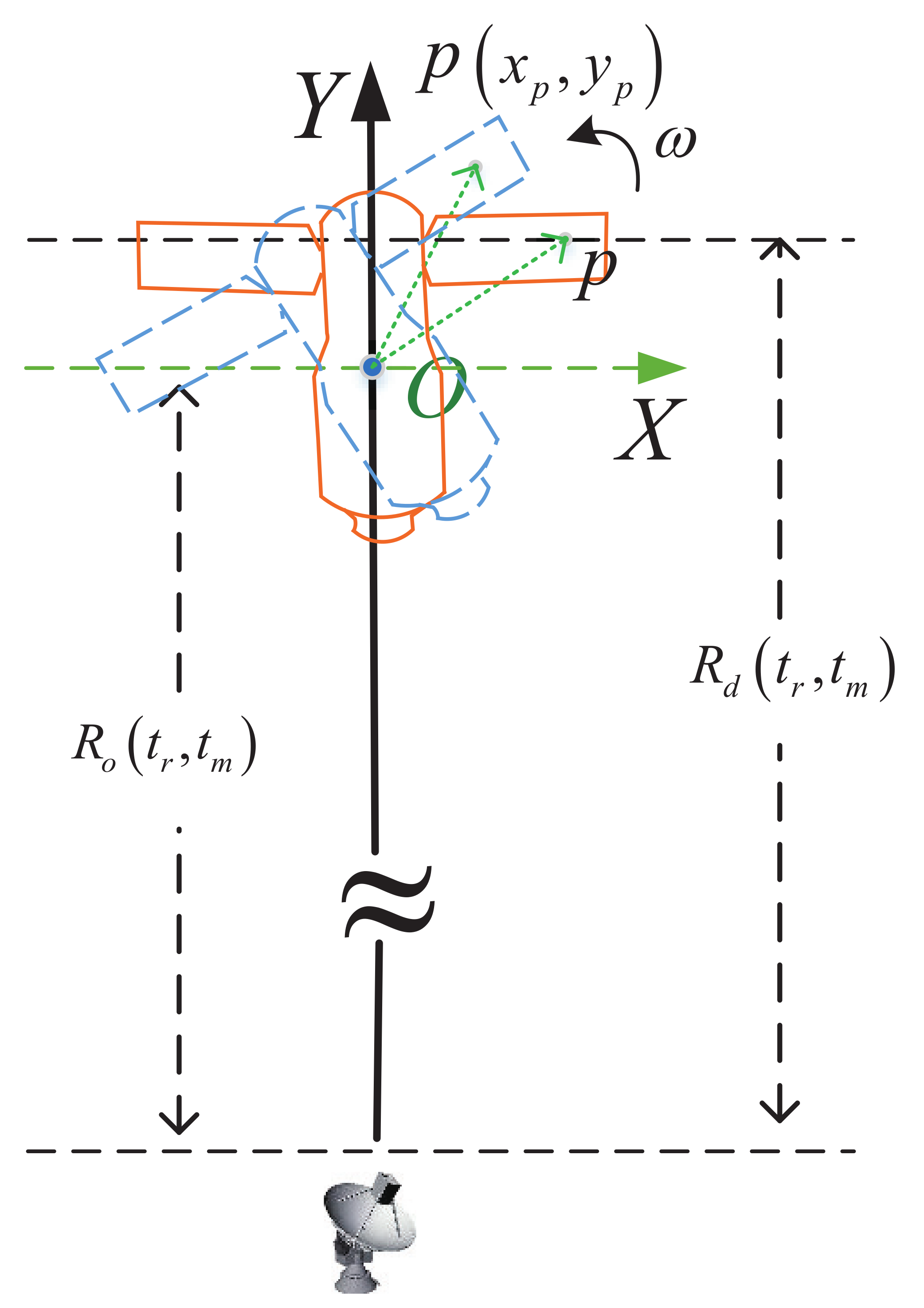
2. De-Chirp Signal Model for High-Speed Moving Targets
3. Optimal Compensation for High-Speed Motion
3.1. Optimization Based on Parametric Minimum Entropy
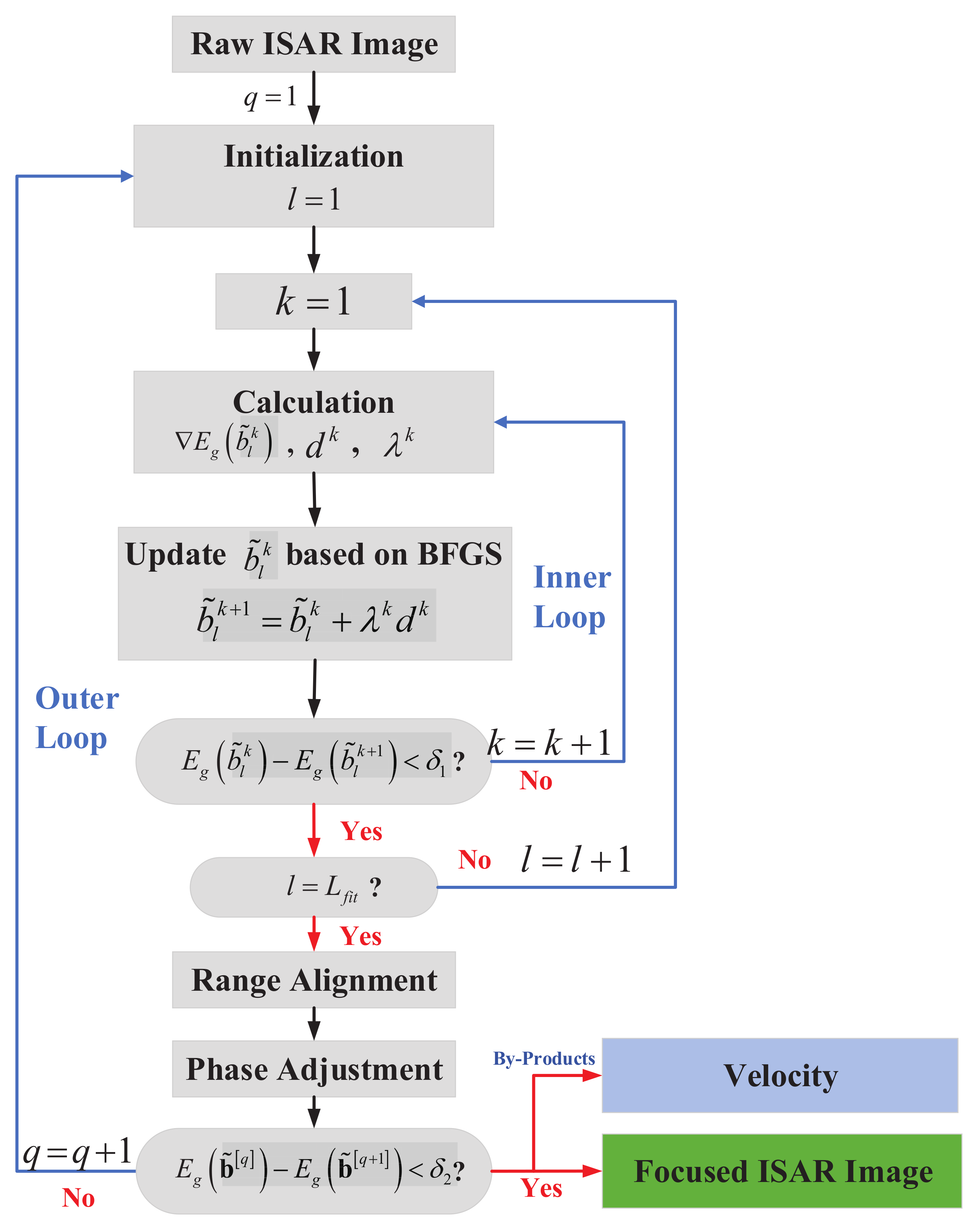
3.2. Parameter Optimization Based on Fast Iteration
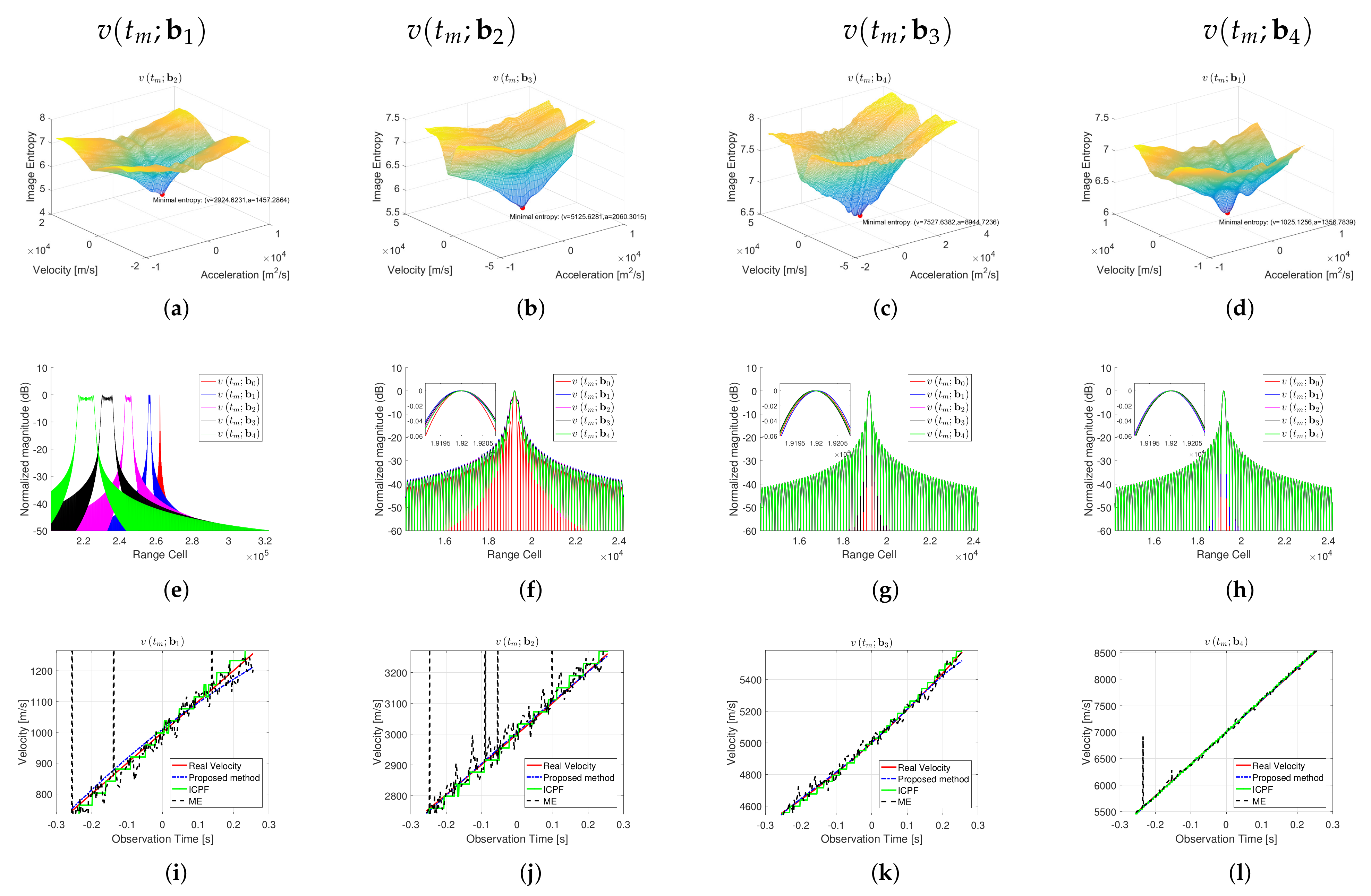
4. Experiment Analysis
4.1. Experiments Based on Point Array Simulation
4.2. Experiments Based on TG-I’s Electromagnetic Simulation
4.3. Performance under Different SNRs
4.4. Experiment Using Measured Yak-42 Data
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Walker, J.L. Range-Doppler imaging of rotating objects. IEEE Trans. Aerosp. Electron. Syst. 1980, AES-16, 23–52. [Google Scholar] [CrossRef]
- Xu, G.; Xing, M.D.; Zhang, L.; Duan, J.; Chen, Q.Q.; Bao, Z. Sparse apertures ISAR imaging and scaling for maneuvering targets. IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2014, 7, 2942–2956. [Google Scholar] [CrossRef]
- Yang, L.; Xing, M.; Zhang, L.; Sun, G.C.; Gao, Y.; Zhang, Z.; Bao, Z. Integration of rotation estimation and high-order compensation for ultrahigh-resolution microwave photonic isar imagery. IEEE Trans. Geosci. Remote Sens. 2020, 59, 2095–2115. [Google Scholar] [CrossRef]
- Ma, J.T.; Gao, M.G.; Guo, B.F.; Dong, J.; Xiong, D.; Feng, Q. High resolution inverse synthetic aperture radar imaging of three-axis-stabilized space target by exploiting orbital and sparse priors. Chin. Phys. B 2017, 26, 108401. [Google Scholar] [CrossRef]
- Jakowatz, C.V.; Wahl, D.E.; Eichel, P.H.; Ghiglia, D.C.; Thompson, P.A. Spotlight-Mode Synthetic Aperture Radar: A Signal Processing Approach: A Signal Processing Approach; Springer: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Chen, C.C.; Andrews, H.C. Target-motion-induced radar imaging. IEEE Trans. Aerosp. Electron. Syst. 1980, AES-16, 2–14. [Google Scholar] [CrossRef]
- Caputi, W.J. Stretch: A time-transformation technique. IEEE Trans. Aerosp. Electron. Syst. 1971, AES-7, 269–278. [Google Scholar] [CrossRef]
- Wehner, D.R. High resolution radar. In Norwood; Artech House: London, UK, 1987. [Google Scholar]
- Tian, B.; Chen, Z.; Xu, S.; Liu, Y. ISAR imaging compensation of high speed targets based on integrated cubic phase function. In MIPPR 2013: Multispectral Image Acquisition, Processing, and Analysis; International Society for Optics and Photonics: Bellingham, WA, USA, 2013; Volume 8917, p. 89170B. [Google Scholar]
- Kun-Fan, Z.; Zhi-Hong, F.; De-Bao, M. Study on a method of compensation for the range profile of high velocity spatial targets. In Proceedings of the 2010 International Conference on Image Analysis and Signal Processing, Zhejiang, China, 9–11 April 2010; pp. 450–453. [Google Scholar]
- Tian, B.; Lu, Z.; Liu, Y.; Li, X. High velocity motion compensation of IFDS data in ISAR imaging based on adaptive parameter adjustment of matched filter and entropy minimization. IEEE Access 2018, 6, 34272–34278. [Google Scholar] [CrossRef]
- Gu, F.F.; Fu, M.H.; Chen, C.H.; Yang, M.; Zhang, Y. A novel ISAR imaging method for high speed moving target based on parametric sparse representation. In Proceedings of the 2017 16th International Conference on Optical Communications and Networks (ICOCN), Wuzhen, China, 7–10 August 2017; pp. 1–3. [Google Scholar]
- Zhiping, Y.; Zhen, F.; Dongjin, W.; Weidong, C. ISAR imaging of fast-moving target based on FRFT range compression. In Proceedings of the 2007 1st Asian and Pacific Conference on Synthetic Aperture Radar, Huangshan, China, 5–9 November 2007; pp. 306–309. [Google Scholar]
- Cao, M.; Fu, Y.; Jiang, W.; Li, X.; Zhuang, Z. High resolution range profile imaging of high speed moving targets based on fractional Fourier transform. In MIPPR 2007: Automatic Target Recognition and Image Analysis; and Multispectral Image Acquisition; International Society for Optics and Photonics: Bellingham, WA, USA, 2007; Volume 6786, p. 678654. [Google Scholar]
- Zhang, S.; Sun, S.; Zhang, W.; Zong, Z.; Yeo, T.S. High-resolution bistatic ISAR image formation for high-speed and complex-motion targets. IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2015, 8, 3520–3531. [Google Scholar] [CrossRef]
- Wang, F.; Jiang, D.; Chen, H. High range resolution profile construction exploiting modified fractional Fourier transformation. Math. Probl. Eng. 2015, 2015, 321878. [Google Scholar] [CrossRef] [Green Version]
- Liu, Y.; Zhang, S.; Zhu, D.; Li, X. A novel speed compensation method for ISAR imaging with low SNR. Sensors 2015, 15, 18402–18415. [Google Scholar] [CrossRef] [Green Version]
- Wang, Y.; Kang, J.; Jiang, Y. ISAR imaging of maneuvering target based on the local polynomial Wigner distribution and integrated high-order ambiguity function for cubic phase signal model. IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2014, 7, 2971–2991. [Google Scholar] [CrossRef]
- Brinkman, W.; Thayaparan, T. Focusing ISAR images using the AJTF optimized with the GA and the PSO algorithm-comparison and results. In Proceedings of the 2006 IEEE Conference on Radar, Verona, NY, USA, 24–27 April 2006. [Google Scholar]
- He, C.; Daiying, Z. High speed motion compensation based on the range profile. In Proceedings of the 2013 IEEE International Conference on Signal Processing, Communication and Computing (ICSPCC 2013), Kunming, China, 5–8 August 2013; pp. 1–4. [Google Scholar]
- Sheng, J.; Fu, C.; Wang, H.; Liu, Y. High speed motion compensation for terahertz ISAR imaging. In Proceedings of the 2017 International Applied Computational Electromagnetics Society Symposium (ACES), Suzhou, China, 1–4 August 2017; pp. 1–2. [Google Scholar]
- Guo, B.; Li, Z.; Xiao, Y.; Shi, L.; Han, N.; Zhu, X. ISAR Speed Compensation Algorithm for High-speed Moving Target Based on Simulate Anneal. In Proceedings of the 2019 IEEE 19th International Conference on Communication Technology (ICCT), Xi’an, China, 16–19 October 2019; pp. 1595–1599. [Google Scholar]
- Zhu, D.; Wang, L.; Yu, Y.; Tao, Q.; Zhu, Z. Robust ISAR range alignment via minimizing the entropy of the average range profile. IEEE Geosci. Remote Sens. Lett. 2009, 6, 204–208. [Google Scholar]
- Liu, L.; Zhou, F.; Tao, M.; Sun, P.; Zhang, Z. Adaptive translational motion compensation method for ISAR imaging under low SNR based on particle swarm optimization. IEEE J.Sel. Top. Appl. Earth Obs. Remote Sens. 2015, 8, 5146–5157. [Google Scholar] [CrossRef]
- Zhang, L.; Sheng, J.l.; Duan, J.; Xing, M.D.; Qiao, Z.J.; Bao, Z. Translational motion compensation for ISAR imaging under low SNR by minimum entropy. EURASIP J. Adv. Signal Process. 2013, 2013, 33. [Google Scholar] [CrossRef] [Green Version]
- Xi, L.; Guosui, L.; Ni, J. Autofocusing of ISAR images based on entropy minimization. IEEE Trans. Aerosp. Electron. Syst. 1999, 35, 1240–1252. [Google Scholar] [CrossRef]
- Wang, J.; Liu, X.; Zhou, Z. Minimum-entropy phase adjustment for ISAR. IEE Proc.-Radar Sonar Navig. 2004, 151, 203–209. [Google Scholar] [CrossRef]
- Kragh, T.J.; Kharbouch, A.A. Monotonic iterative algorithm for minimum-entropy autofocus. In Proceedings of the Adaptive Sensor Array Processing (ASAP) Workshop, Lexington, KY, USA, 6–7 June 2006; Volume 40, pp. 1147–1159. [Google Scholar]
- Wang, J.; Zhang, L.; Du, L.; Yang, D.; Chen, B. Noise-robust motion compensation for aerial maneuvering target ISAR imaging by parametric minimum entropy optimization. IEEE Trans. Geosci. Remote Sens. 2019, 57, 4202–4217. [Google Scholar] [CrossRef]
- Li, Y.; Wu, R.; Xing, M.; Bao, Z. Inverse synthetic aperture radar imaging of ship target with complex motion. IET Radar Sonar Navig. 2008, 2, 395–403. [Google Scholar] [CrossRef]
- Liu, Y.; Li, G.; Tian, B.; Chen, Z.P. ISAR imaging at low SNR level based on polarimetric whitening filter. In MIPPR 2013: Multispectral Image Acquisition, Processing, and Analysis; International Society for Optics and Photonics: Bellingham, WA, USA, 2013; Volume 8917, p. 891703. [Google Scholar]
- Barbarossa, S.; Di Lorenzo, P.; Vecchiarelli, P. Parameter estimation of 2D multi-component polynomial phase signals: An application to SAR imaging of moving targets. IEEE Trans. Signal Process. 2014, 62, 4375–4389. [Google Scholar] [CrossRef]
- Cantoni, A.; Martorella, M. Fourier-based ISAR imaging using 2D polynomials. IET Radar Sonar Navig. 2017, 11, 1216–1227. [Google Scholar] [CrossRef] [Green Version]
- Cantoni, A.; Martorella, M. ISAR image autofocus using 2D-polynomials. In Proceedings of the 2016 IEEE Radar Conference (RadarConf), Philadelphia, PA, USA, 2–6 May 2016; pp. 1–6. [Google Scholar]
- Nocedal, J.; Wright, S. Numerical Optimization; Springer: Berlin/Heidelberg, Germany, 2006. [Google Scholar]
- Shao, S.; Zhang, L.; Liu, H.; Zhou, Y. Spatial-variant contrast maximization autofocus algorithm for ISAR imaging of maneuvering targets. Sci. China Inf. Sci. 2019, 62, 40303. [Google Scholar] [CrossRef] [Green Version]
- Shao, S.; Zhang, L.; Liu, H.; Zhou, Y. Accelerated translational motion compensation with contrast maximisation optimisation algorithm for inverse synthetic aperture radar imaging. IET Radar Sonar Navig. 2019, 13, 316–325. [Google Scholar] [CrossRef]
- Chen, V.C.; Lipps, R. ISAR imaging of small craft with roll, pitch and yaw analysis. In Proceedings of the Record of the IEEE 2000 International Radar Conference, Alexandria, VA, USA, 12 May 2000; pp. 493–498. [Google Scholar]
- Chen, V.C.; Miceli, W. Time-varying spectral analysis for radar imaging of manoeuvring targets. IEE Proc.-Radar Sonar Navig. 1998, 145, 262–268. [Google Scholar] [CrossRef]
- Li, Y.; Xing, M.; Su, J.; Quan, Y.; Bao, Z. A new algorithm of ISAR imaging for maneuvering targets with low SNR. IEEE Trans. Aerosp. Electron. Syst. 2013, 49, 543–557. [Google Scholar] [CrossRef]
- Martorella, M.; Berizzi, F.; Haywood, B. Contrast maximisation based technique for 2-D ISAR autofocusing. IEE Proc.-Radar Sonar Navig. 2005, 152, 253–262. [Google Scholar] [CrossRef] [Green Version]
- Wang, J.; Kasilingam, D. Global range alignment for ISAR. IEEE Trans. Aerosp. Electron. Syst. 2003, 39, 351–357. [Google Scholar] [CrossRef]
- Boag, A. A fast physical optics (FPO) algorithm for high frequency scattering. IEEE Trans. Antennas Propag. 2004, 52, AES-16, 197–204. [Google Scholar] [CrossRef]



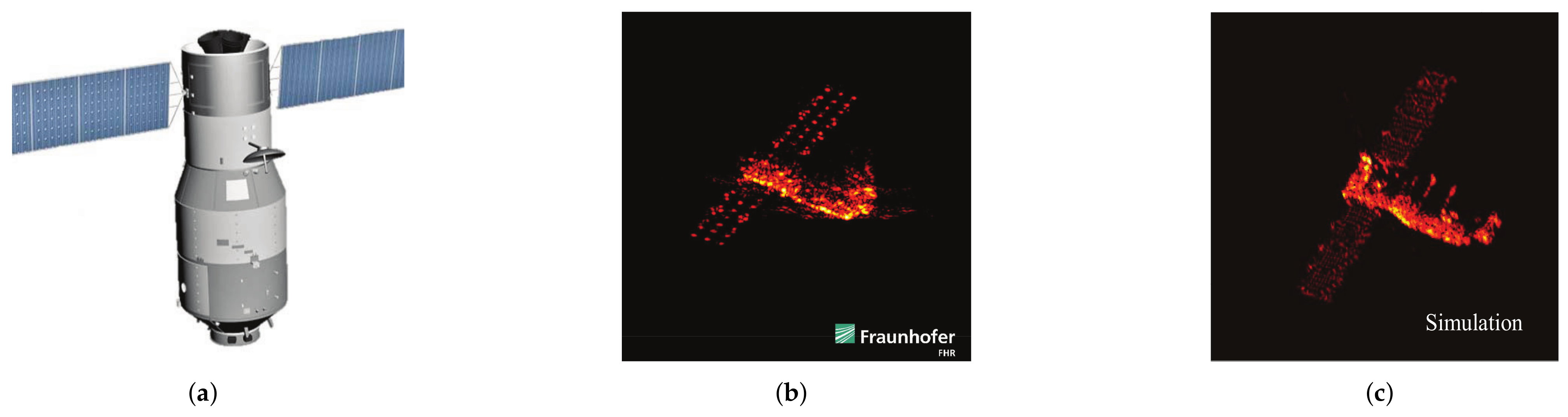

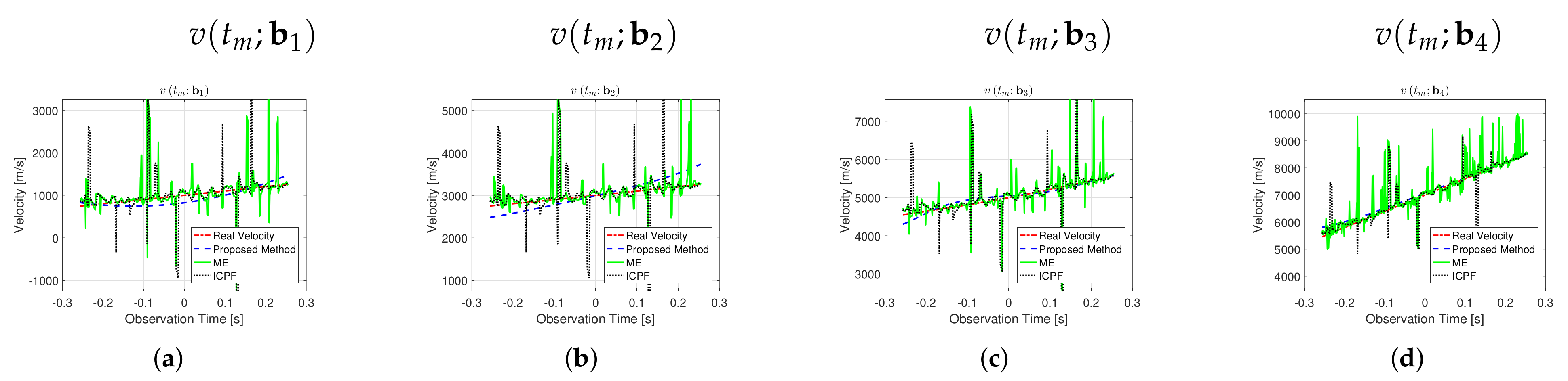
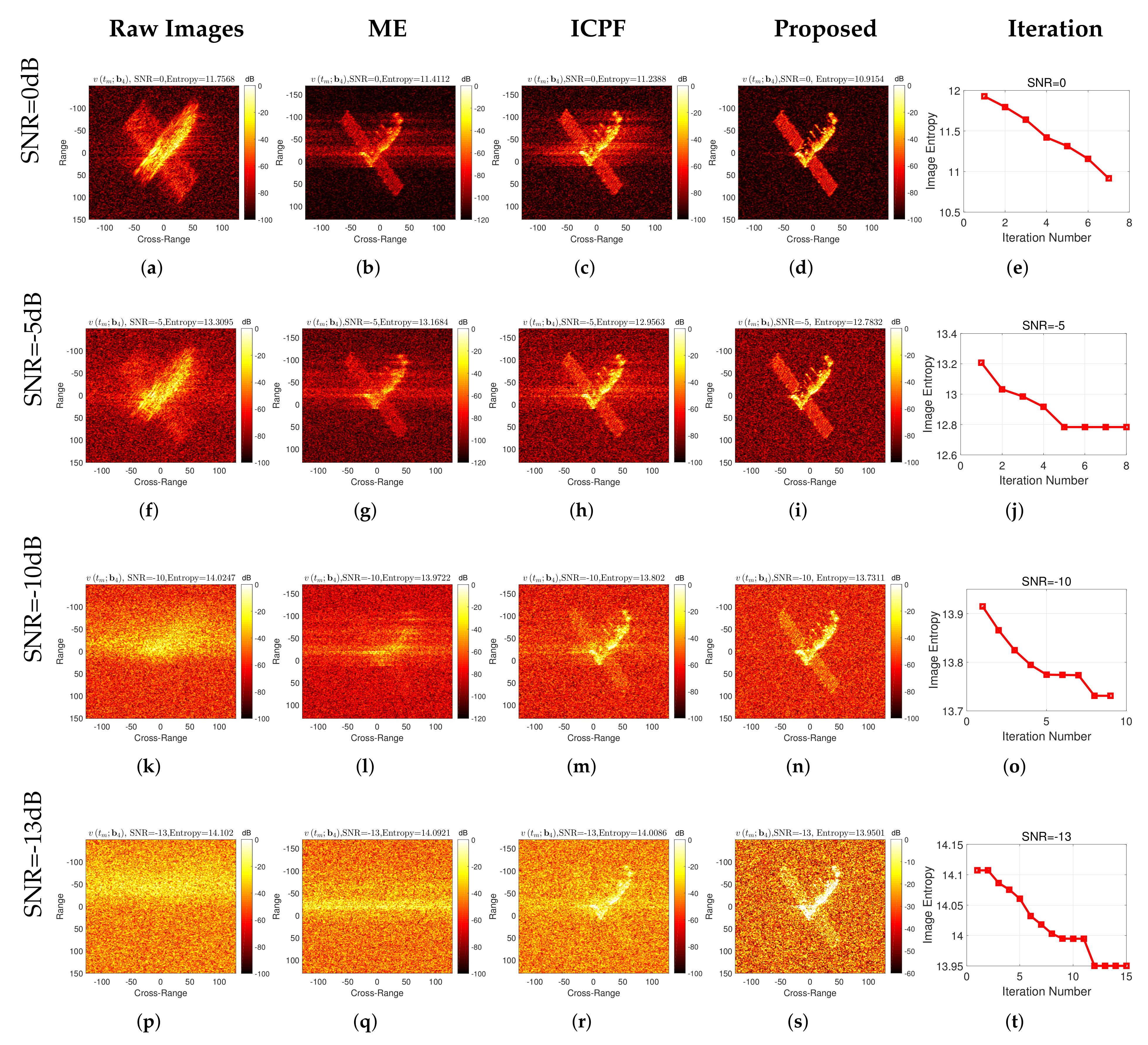

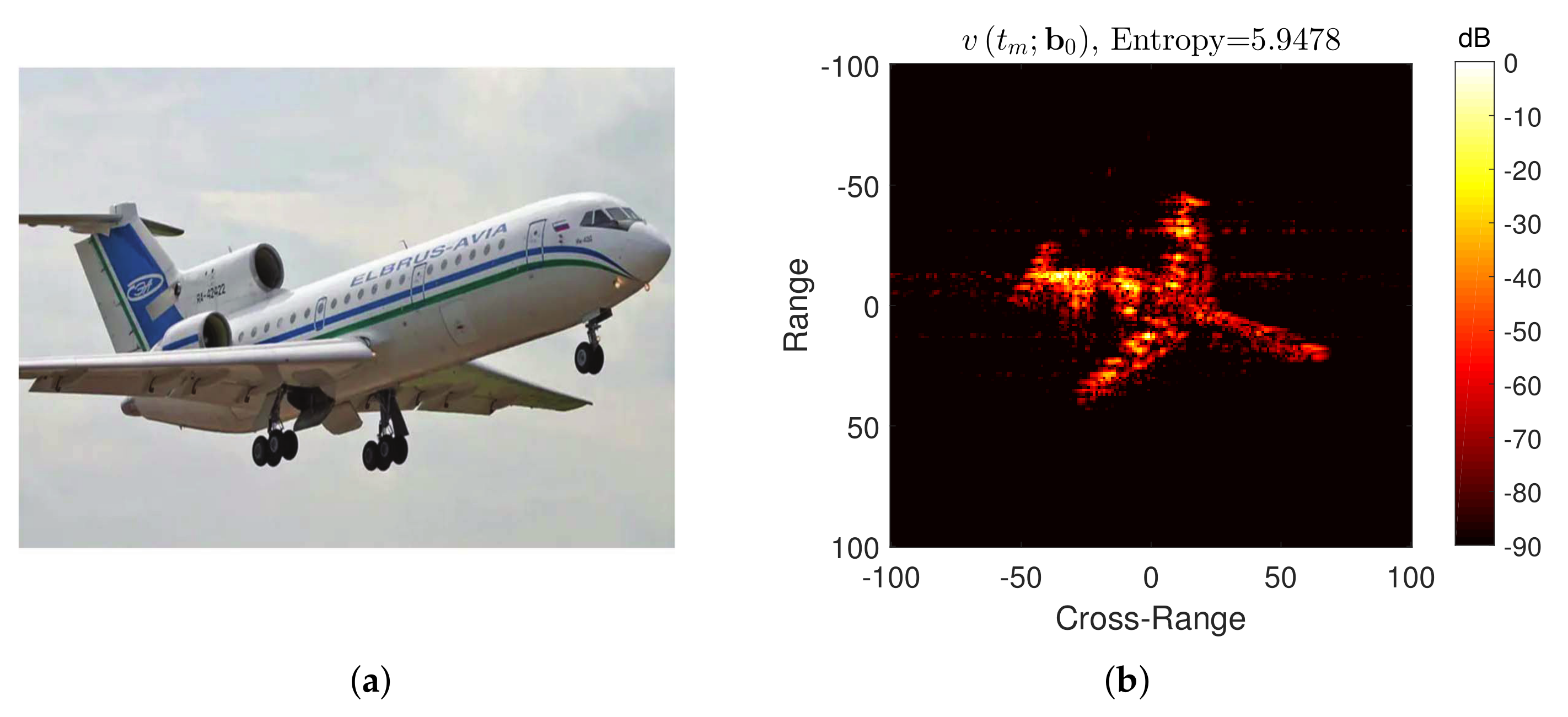




| Center Frequency | Pulse Repetition Frequency | Pulse Width | Band Width | Sample Frequency |
|---|---|---|---|---|
| 16 GHz | 1000 Hz | 400 us | 2 GHz | 10 MHz |
| b | b0 | b1 | b2 | |
|---|---|---|---|---|
| v | ||||
| 0 | 0 | 0 | ||
| 1000 | 1000 | 10 | ||
| 3000 | 1000 | 100 | ||
| 5000 | 2000 | 1000 | ||
| 7000 | 6000 | 10 | ||
| Image Entropy | ||||
|---|---|---|---|---|
| Ideal Image | 4.3252 | |||
| Raw Image | 6.2026 | 5.5213 | 5.9747 | 6.593 |
| ME | 4.4224 | 4.4917 | 4.3975 | 4.3981 |
| ICPF | 4.3911 | 4.3853 | 4.3897 | 4.3821 |
| Proposed Method | 4.3347 | 4.3328 | 4.3256 | 4.3422 |
| RMSE | ||||
|---|---|---|---|---|
| ME | 217.41 | 254.57 | 27.27 | 65.99 |
| ICPF | 19.55 | 18.46 | 19.24 | 19.76 |
| Proposed Method | 17.35 | 4.40 | 12.83 | 6.46 |
| Image Entropy | ||||
|---|---|---|---|---|
| Ideal Image | 6.3441 | |||
| Raw Images | 6.8023 | 7.3338 | 7.5326 | 7.8763 |
| ME | 7.181 | 7.177 | 7.134 | 7.8239 |
| ICPF | 6.9641 | 6.9599 | 6.9469 | 6.9511 |
| Proposed Method | 6.4277 | 6.3488 | 6.3703 | 6.3587 |
| RMSE | ||||
|---|---|---|---|---|
| ME | 492.3 | 414.1 | 454.8 | 549.5 |
| ICPF | 503.6 | 503.5 | 503.5 | 457.0 |
| Proposed Method | 125.2 | 202.8 | 83.4 | 123.7 |
| Image Entropy vs. SNR | ||||
|---|---|---|---|---|
| SNR | 0 dB | −5 dB | −10 dB | −13 dB |
| Raw Images | 11.7568 | 13.3095 | 14.0247 | 14.102 |
| ME | 11.4112 | 13.1684 | 13.9722 | 14.0921 |
| ICPF | 11.2388 | 12.9563 | 13.802 | 14.0086 |
| Proposed Method | 10.9154 | 12.7832 | 13.7311 | 13.9501 |
| Image Entropy | ||||
|---|---|---|---|---|
| Ideal Image | 5.9478 | |||
| Raw Images | 7.0153 | 8.1064 | 8.7729 | 9.0073 |
| ME | 6.3938 | 6.4513 | 6.9367 | 6.881 |
| ICPF | 6.6593 | 6.6628 | 6.6609 | 6.6615 |
| Proposed Method | 5.9983 | 5.9617 | 6.1039 | 6.0206 |
| RMSE | ||||
|---|---|---|---|---|
| ME | 146.99 | 93.78 | 194.24 | 252.78 |
| ICPF | 319.42 | 319.61 | 319.99 | 321.25 |
| Proposed Method | 35.28 | 49.79 | 66.59 | 49.87 |
| Image Entropy Vs SNR | ||||
|---|---|---|---|---|
| SNR | 0 dB | −5 dB | −10 dB | −13 dB |
| Raw Images | 9.0235 | 10.3065 | 11.0532 | 11.2426 |
| ME | 7.8333 | 9.3286 | 10.5286 | 11.2758 |
| ICPF | 8.0752 | 9.4778 | 10.5239 | 11.348 |
| Proposed Method | 7.69 | 8.9948 | 10.3366 | 10.4271 |
| Algorithms | ME | ICPF | Proposed Method |
|---|---|---|---|
| Computation time (s) | 70.91 | 224.77 | 5.52 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, J.; Li, Y.; Song, M.; Huang, P.; Xing, M. Noise Robust High-Speed Motion Compensation for ISAR Imaging Based on Parametric Minimum Entropy Optimization. Remote Sens. 2022, 14, 2178. https://doi.org/10.3390/rs14092178
Wang J, Li Y, Song M, Huang P, Xing M. Noise Robust High-Speed Motion Compensation for ISAR Imaging Based on Parametric Minimum Entropy Optimization. Remote Sensing. 2022; 14(9):2178. https://doi.org/10.3390/rs14092178
Chicago/Turabian StyleWang, Jiadong, Yachao Li, Ming Song, Pingping Huang, and Mengdao Xing. 2022. "Noise Robust High-Speed Motion Compensation for ISAR Imaging Based on Parametric Minimum Entropy Optimization" Remote Sensing 14, no. 9: 2178. https://doi.org/10.3390/rs14092178
APA StyleWang, J., Li, Y., Song, M., Huang, P., & Xing, M. (2022). Noise Robust High-Speed Motion Compensation for ISAR Imaging Based on Parametric Minimum Entropy Optimization. Remote Sensing, 14(9), 2178. https://doi.org/10.3390/rs14092178








