Experiences with the RTM Method in Local Quasi-Geoid Modeling
Abstract
:1. Introduction
2. RTM Corrections to Gravity Anomalies and Height Anomalies
3. Numerical Experiments
3.1. Data Sets
3.2. Parameter Settings
3.3. RTM Correction Comparison
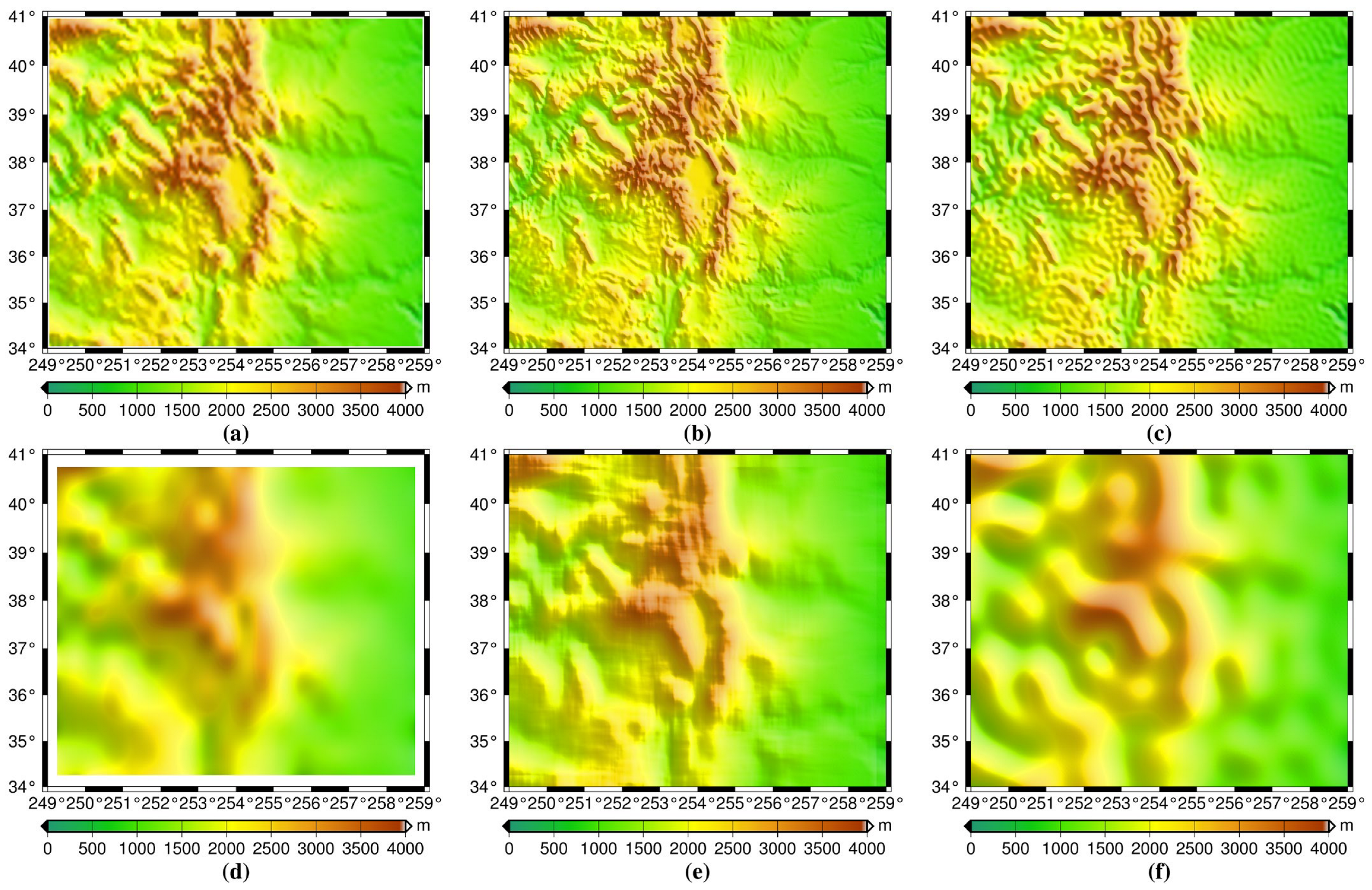
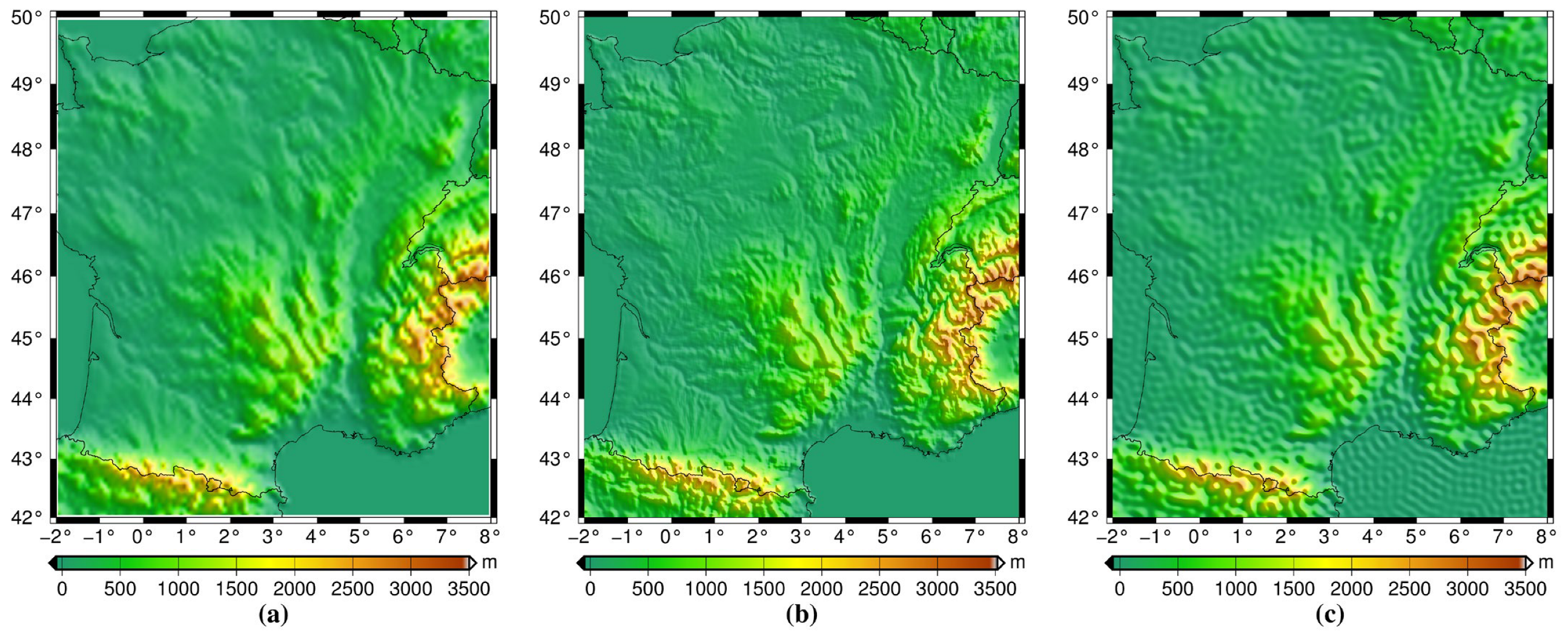
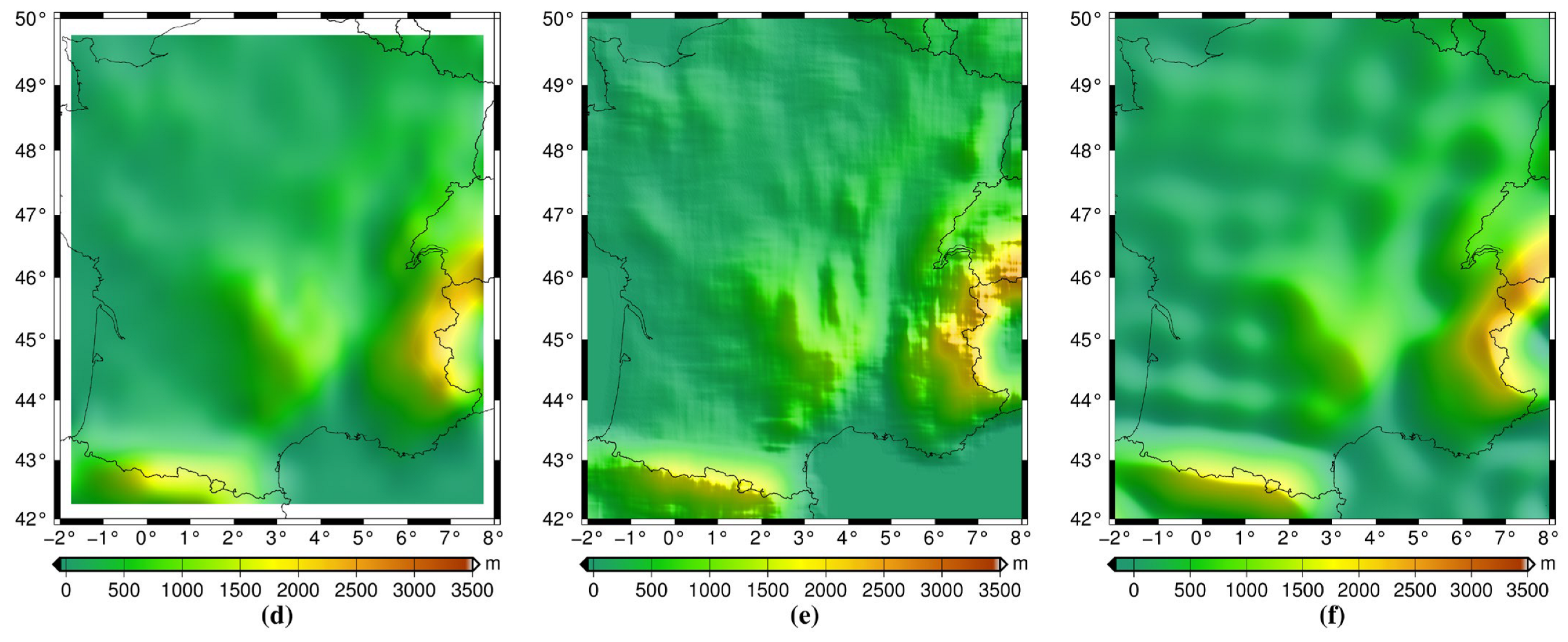
3.3.1. DEM Resolution Combination Effect on RTM Corrections
3.3.2. Integration Radius Effect on RTM Corrections
3.3.3. Reference Topography Effect on RTM Corrections
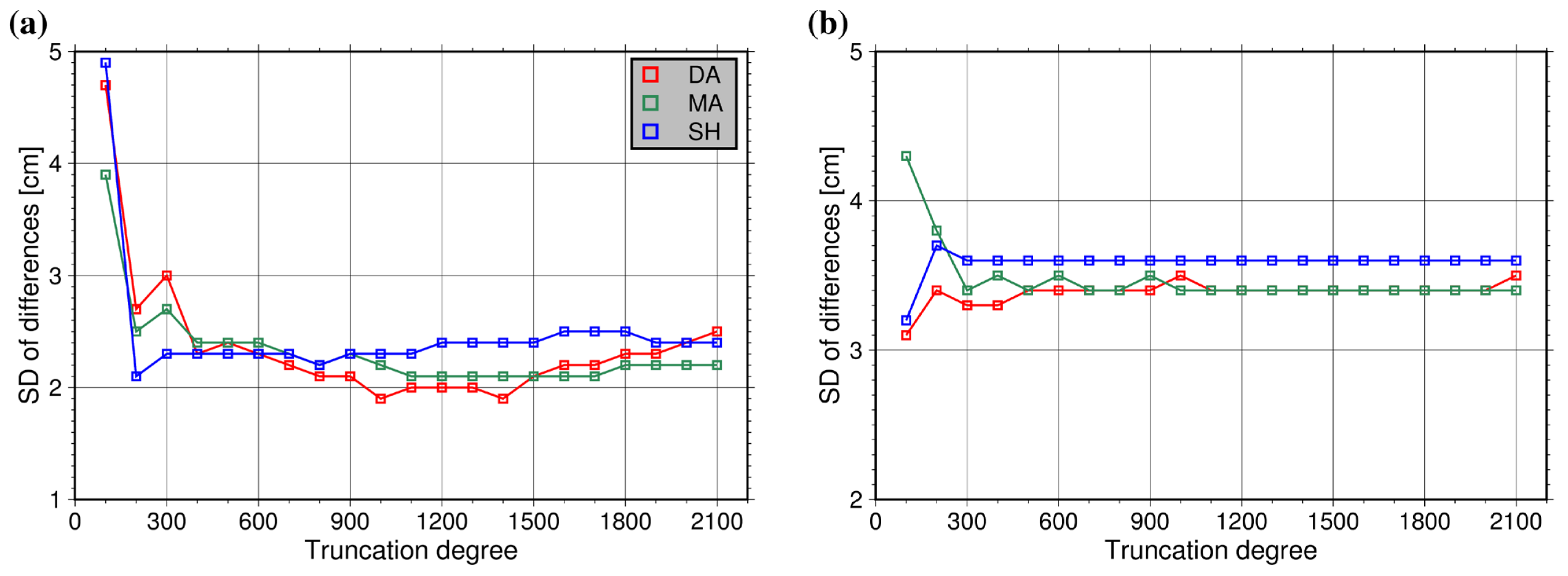
3.4. Local Quasi-Geoid Model Comparison
4. Summary and Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Heiskanen, W.; Moritz, H. Physical Geodesy; W.H. Freeman: San Francisco, CA, USA, 1967. [Google Scholar]
- Hofmann-Wellenhof, B.; Moritz, H. Physical Geodesy; Springer: Wien, Austria, 2006. [Google Scholar]
- Bucha, B.; Janák, J.; Papčo, J.; Bezděk, A. High-resolution regional gravity field modelling in a mountainous area from terrestrial gravity data. Geophys. J. Int. 2016, 207, 949–966. [Google Scholar] [CrossRef] [Green Version]
- Denker, H. Regional Gravity Field Modeling: Theory and Practical Results. In Science of Geodesy—II; Xu, G., Ed.; Springer: Berlin, Germany, 2013; pp. 185–291. [Google Scholar]
- Featherstone, W.E.; McCubbine, J.C.; Brown, N.J.; Claessens, S.J.; Filmer, M.S.; Kirby, J.F. The first Australian gravimetric quasigeoid model with location-specific uncertainty estimates. J. Geod. 2018, 92, 149–168. [Google Scholar] [CrossRef] [Green Version]
- Klees, R.; Tenzer, R.; Prutkin, I.; Wittwer, T. A data-driven approach to local gravity field modelling using spherical radial basis functions. J. Geod. 2008, 82, 457–471. [Google Scholar] [CrossRef] [Green Version]
- Slobbe, C.; Klees, R.; Farahani, H.; Huisman, L.; Alberts, B.; Voet, P.; De Doncker, F. The impact of noise in a GRACE/GOCE global gravity model on a local quasi-geoid. J. Geophys. Res. 2019, 124, 3219–3237. [Google Scholar] [CrossRef]
- Scheinert, M.; Müller, J.; Dietrich, R.; Damaske, D.; Damm, V. Regional geoid determination in Antarctica utilizing airborne gravity and topography data. J. Geod. 2008, 82, 403–414. [Google Scholar] [CrossRef]
- Vu, D.T.; Bruinsma, S.; Bonvalot, S. A high-resolution gravimetric quasigeoid model for Vietnam. Earth Planets Space 2019, 71, 65. [Google Scholar] [CrossRef]
- Li, X.; Ahlgren, K.; Hardy, R.; Krcmaric, J.; Wang, Y. The Development and Evaluation of the Experimental Gravimetric Geoid Model 2019. 2019. Available online: https://beta.ngs.noaa.gov/GEOID/xGEOID19/xGeoid19_tech_details.v10.pdf (accessed on 15 July 2021).
- Wang, Y.; Li, X.; Ahlgren, K.; Krcmaric, J. Colorado geoid modeling at the US National Geodetic Survey. J. Geod. 2020, 94, 106. [Google Scholar] [CrossRef]
- Jiang, T.; Dang, Y.; Zhang, C. Gravimetric geoid modeling from the combination of satellite gravity model, terrestrial and airborne gravity data: A case study in the mountainous area, Colorado. Earth Planets Space 2020, 72, 189. [Google Scholar] [CrossRef]
- Tscherning, C.C. Comparison of some methods for the detailed representation of the Earth’s gravity field. Rev. Geophys. 1981, 19, 213–221. [Google Scholar] [CrossRef]
- Tscherning, C.C. Geoid Determination by 3D Least-Squares Collocation. In Geoid Determination Theory and Methods; Sansò, F., Sideris, M.G., Eds.; Springer: Berlin, Germany, 2013; pp. 311–336. [Google Scholar]
- Grigoriadis, V.N.; Vergos, G.S.; Barzaghi, R.; Carrion, D.; Koç, Ö. Collocation and FFT-based geoid estimation within the Colorado 1 cm geoid experiment. J. Geod. 2021, 95, 52. [Google Scholar] [CrossRef]
- Li, X. Using radial basis functions in airborne gravimetry for local geoid improvement. J. Geod. 2018, 92, 471–485. [Google Scholar] [CrossRef]
- Lin, M.; Denker, H.; Müller, J. A comparison of fixed- and free-positioned point mass methods for regional gravity field modeling. J. Geodyn. 2019, 125, 32–47. [Google Scholar] [CrossRef]
- Schmidt, M.; Fengler, M.; Mayer-Guerr, T.; Eicker, A.; Kusche, J.; Sanchez, L.; Han, S.C. Regional gravity field modeling in terms of spherical base functions. J. Geod. 2007, 81, 17–38. [Google Scholar] [CrossRef] [Green Version]
- Forsberg, R.; Tscherning, C.C. The use of height data in gravity field approximation by collocation. J. Geophys. Res. 1981, 86, 7843–7854. [Google Scholar] [CrossRef]
- Forsberg, R. A Study of Terrain Reductions, Density Anomalies and Geophysical Inversion Methods in Gravity Field Modeling; OSU Report 355; Ohio State University: Columbus, OH, USA, 1984. [Google Scholar]
- Tziavos, I.N.; Vergos, G.S.; Grigoriadis, V.N. Investigation of topographic reductions and aliasing effects on gravity and the geoid over Greece based on various digital terrain models. Surv. Geophys. 2010, 31, 23–67. [Google Scholar] [CrossRef]
- Tziavos, I.N.; Sideris, M.G. Topographic Reductions in Gravity and Geoid Modeling. In Geoid Determination Theory and Methods; Sansò, F., Sideris, M.G., Eds.; Springer: Berlin, Germany, 2013; pp. 337–400. [Google Scholar]
- Rexer, M.; Hirt, C.; Bucha, B.; Holmes, S. Solution to the spectral filter problem of residual terrain modelling (RTM). J. Geod. 2018, 92, 675–690. [Google Scholar] [CrossRef]
- Farr, T.G.; Rosen, P.; Caro, E.; Crippen, R.; Duren, R.; Hensley, S.; Kobrick, M.; Paller, M.; Rodriguez, E.; Roth, L.; et al. The shuttle radar topography mission. Rev. Geophys. 2007, 45, RG2004. [Google Scholar] [CrossRef] [Green Version]
- Yamazaki, D.; Ikeshima, D.; Tawatari, R.; Yamaguchi, T.; O’Loughlin, F.; Neal, J.C.; Sampson, C.C.; Kanae, S.; Bates, P.D. A high-accuracy map of global terrain elevations. Geophys. Res. Lett. 2017, 44, 5844–5853. [Google Scholar] [CrossRef] [Green Version]
- Yang, M.; Hirt, C.; Tenzer, R.; Pail, R. Experiences with the use of mass-density maps in residual gravity forward modeling. Stud. Geophys. Geod. 2018, 62, 596–623. [Google Scholar] [CrossRef]
- Yang, M.; Hirt, C.; Rexer, M.; Pail, R.; Yamazaki, D. The tree-canopy effect in gravity forward modeling. Geophys. J. Int. 2019, 219, 271–289. [Google Scholar] [CrossRef]
- Yang, M.; Hirt, C.; Pail, R. TGF: A new MATLAB-based software for terrain-related gravity field calculations. Remote. Sens. 2020, 12, 1063. [Google Scholar] [CrossRef] [Green Version]
- Forsberg, R.; Tscherning, C.C. An Overview Manual for the GRAVSOFT Geodetic Gravity Field Modelling Programs; DTU: Copenhagen, Denmark, 2008. [Google Scholar]
- Lin, M.; Li, X. Impacts of using the rigorous topographic gravity modeling method and lateral density variation model on topographic reductions and geoid modeling: A case study in Colorado, USA. Surv. Geophys. 2022, 43, 1497–1538. [Google Scholar] [CrossRef]
- Olgun, S.; Üstün, A.; Akyılmaz, O. tc-cylinder: An optimized algorithm for accurate topography effect from high-resolution digital elevation models. Comput. Geosci. 2023, 170, 105264. [Google Scholar] [CrossRef]
- Bucha, B.; Hirt, C.; Yang, M.; Kuhn, M.; Rexer, M. Residual terrain modelling (RTM) in terms of the cap-modified spectral technique: RTM from a new perspective. J. Geod. 2019, 93, 2089–2108. [Google Scholar] [CrossRef]
- Hirt, C.; Bucha, B.; Yang, M.; Kuhn, M. A numerical study of residual terrain modeling (RTM) techniques and the harmonic correction using ultra-high-degree spectral gravity modelling. J. Geod. 2019, 93, 1469–1486. [Google Scholar] [CrossRef]
- Hirt, C. RTM gravity forward-modeling using topography/bathymetry data to improve high-degree global geopotential models in the coastal zone. Mar. Geod. 2013, 36, 183–202. [Google Scholar] [CrossRef] [Green Version]
- Hirt, C.; Yang, M.; Kuhn, M.; Bucha, B.; Kurzmann, A.; Pail, R. SRTM2gravity: An Ultrahigh Resolution Global Model of Gravimetric Terrain Corrections. Geophys. Res. Lett. 2019, 46, 4618–4627. [Google Scholar] [CrossRef] [Green Version]
- Klees, R.; Seitz, K.; Slobbe, D.C. The RTM harmonic correction revisited. J. Geod. 2022, 96, 39. [Google Scholar] [CrossRef]
- Vaníček, P.; Huang, J.; Novák, P.; Pagiatakis, S.; Véronneau, M.; Martinec, Z.; Featherstone, E. Determination of the boundary values for the Stokes-Helmert problem. J. Geod. 1999, 73, 180–192. [Google Scholar]
- Heck, B. On Helmert’s methods of condensation. J. Geod. 2003, 77, 155–170. [Google Scholar] [CrossRef]
- Ellmann, A.; Vaníček, P. UNB application of Stokes-Helmert’s approach to geoid computation. J. Geodyn. 2007, 43, 200–213. [Google Scholar] [CrossRef]
- Klees, R.; Seitz, K.; Slobbe, D.C. Exact closed-form expressions for the complete RTM correction. J. Geod. 2023, 97, 33. [Google Scholar] [CrossRef]
- Omang, O.C.; Tscherning, C.C.; Forsberg, R. Generalizing the Harmonic Reduction Procedure in Residual Topographic Modeling. In VII Hotine-Marussi Symposium on Mathematical Geodesy; Springer: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Yang, M.; Hirt, C.; Wu, B.; Deng, X.; Tsoulis, D.; Feng, W.; Wang, C.; Zhong, M. Residual Terrain Modelling: The Harmonic Correction for Geoid Heights. Surv. Geophys. 2022, 43, 1201–1231. [Google Scholar] [CrossRef]
- Wang, Y.; Sánchez, L.; Ågren, J.; Huang, J.; Forsberg, R.; Abd-Elmotaal, H.A.; Ahlgren, K.; Barzaghi, R.; Bašić, T.; Carrion, D.; et al. Colorado geoid computation experiment: Overview and summary. J. Geod. 2021, 95, 127. [Google Scholar] [CrossRef]
- Duquenne, H. A data set to test geoid computation methods. In Proceedings of the 1st International Symposium of the International Gravity Field Service “Gravity Field of the Earth”, Harita Dergisi, Istanbul, Turkey, 28 August–1 September 2006. [Google Scholar]
- Ågren, J.; Barzaghi, R.; Carrion, D.; Denker, H.; Grigoriadis, V.N.; Kiamehr, R.; Sona, G.; Tscherning, C.C.; Tziavos, I.N. Different geoid computation methods applied on a test dataset: Results and considerations. In Proceedings of the Hotine-Marussi Symposium, Rome, Italy, 6–12 July 2009. [Google Scholar]
- van Westrum, D.; Ahlgren, K.; Hirt, C.; Guillaume, S. A Geoid Slope Validation Survey (2017) in the rugged terrain of Colorado, USA. J. Geod. 2021, 95, 9. [Google Scholar] [CrossRef]
- Pavlis, N.K.; Holmes, S.A.; Kenyon, S.C.; Factor, J.K. The development and evaluation of the Earth Gravitational Model 2008 (EGM2008). J. Geophys. Res. 2012, 117, B04406. [Google Scholar] [CrossRef] [Green Version]
- Förste, C.; Bruinsma, S.L.; Abrikosov, O.; Lemoine, J.M.; Marty, J.C.; Flechtner, F.; Balmino, G.; Barthelmes, F.; Biancale, R. EIGEN-6C4 the Latest Combined Global Gravity Field Model Including GOCE Data Up to Degree and Order 2190 of GFZ Potsdam and GRGS Toulouse; GFZ Data Services: Potsdam, Germany, 2014. [Google Scholar]
- Wong, L.; Gore, R. Accuracy of geoid heights from modified Stokes kernels. Geophys. J. R. Astron. Soc. 1969, 18, 81–91. [Google Scholar] [CrossRef] [Green Version]
- Rapp, R.H. Use of potential coefficient models for geoid undulation determinations using a spherical harmonic representation of the height anomaly/geoid undulation difference. J. Geod. 1997, 71, 282–289. [Google Scholar] [CrossRef]
- Tenzer, R.; Chen, W.; Rathnayake, S.; Pitoňák, M. The effect of anomalous global lateral topographic density on the geoid-to-quasigeoid separation. J. Geod. 2021, 95, 12. [Google Scholar] [CrossRef]
- Pavlis, N.K.; Factor, J.K.; Holmes, S.A. Terrain-related gravimetric quantities computed for the next EGM. In Proceedings of the 1st International Symposium of the International Gravity Field Service (IGFS), Istanbul, Turkey, 28 August–1 September 2006; pp. 318–323. [Google Scholar]
- Hirt, C. Prediction of vertical deflections from high-degree spherical harmonic synthesis and residual terrain model data. J. Geod. 2010, 84, 179–190. [Google Scholar] [CrossRef] [Green Version]
- Wessel, P.; Smith, W.H.F. New, improved version of generic mapping tools released. Eos Trans. Am. Geophys. Union 1998, 79, 579. [Google Scholar] [CrossRef]

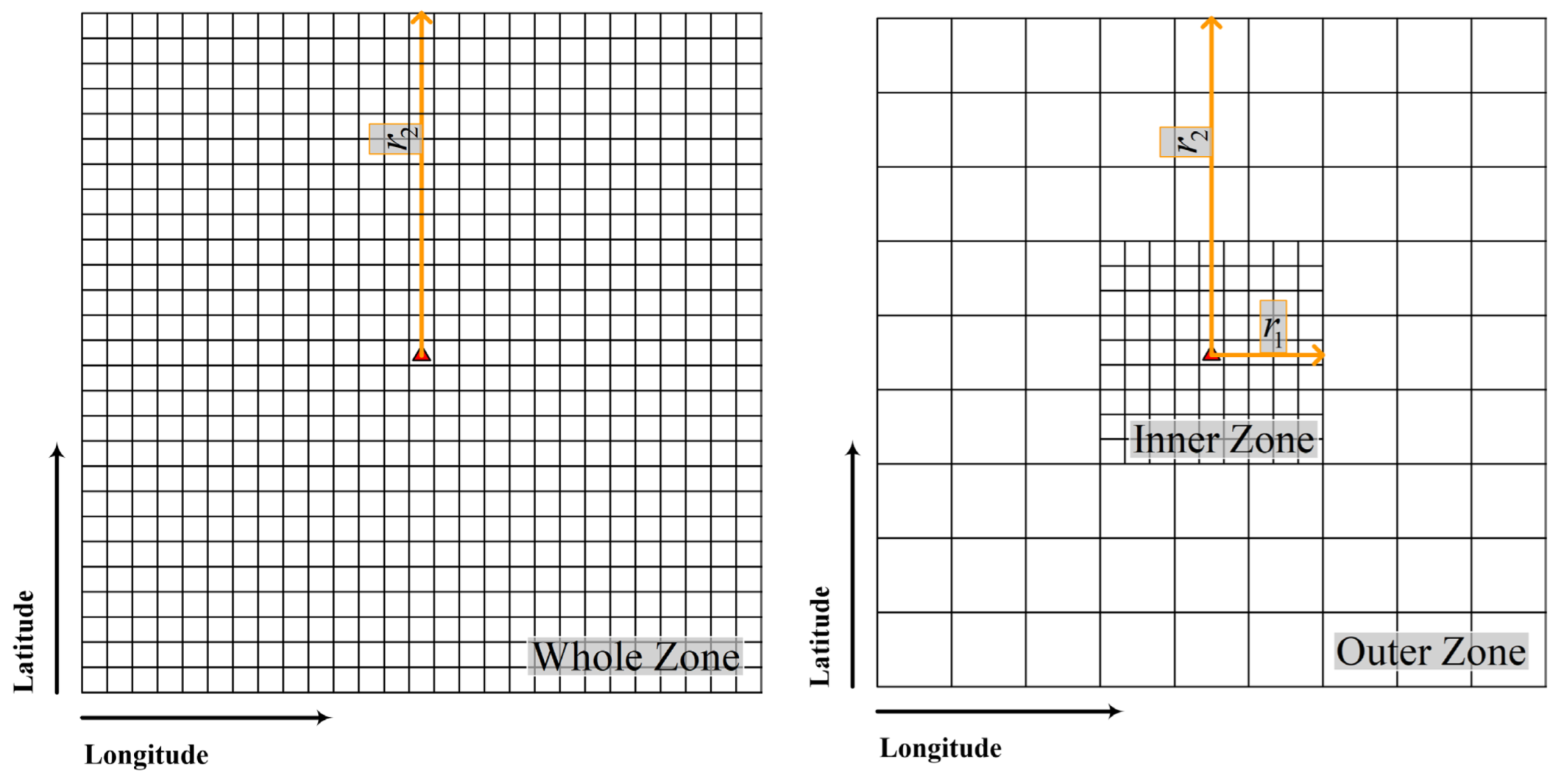



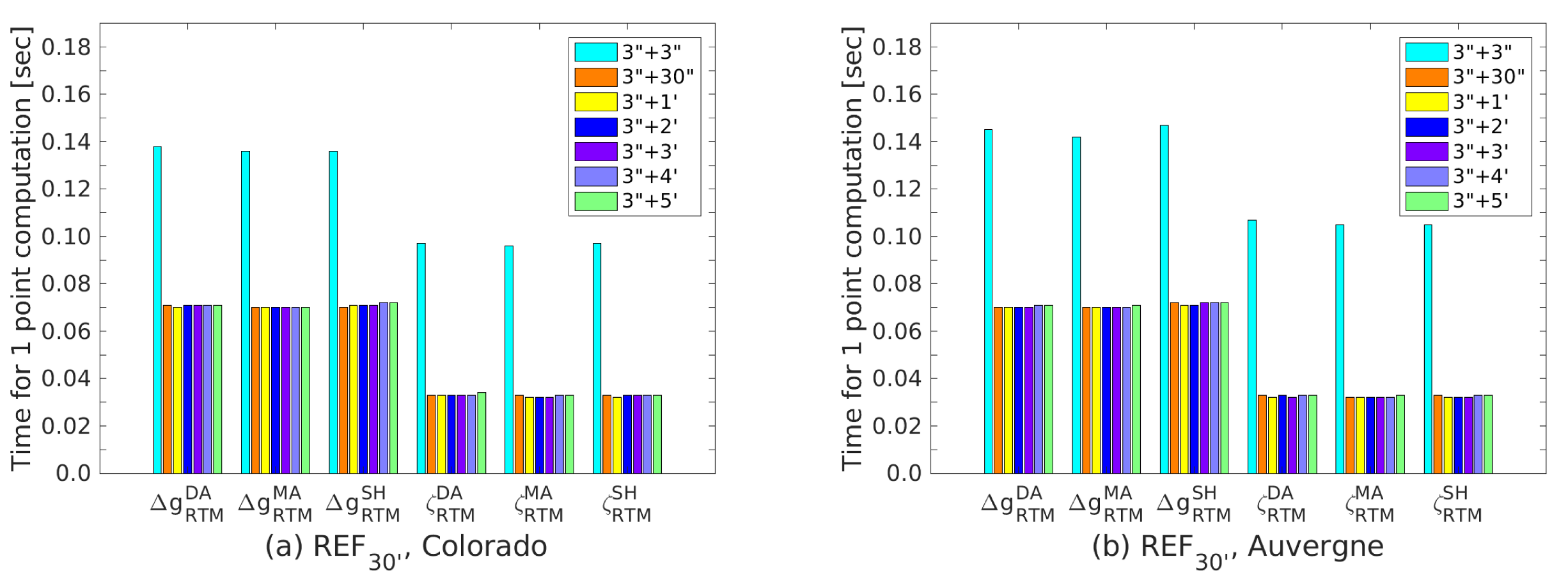

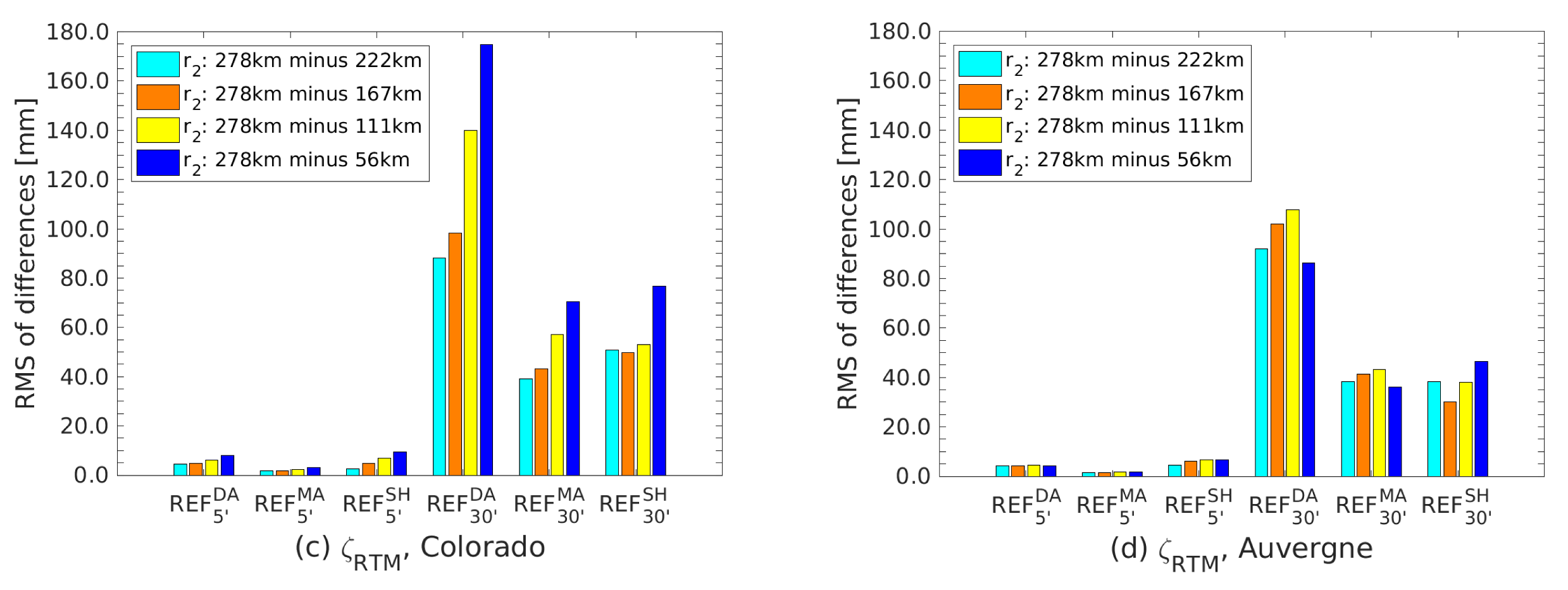

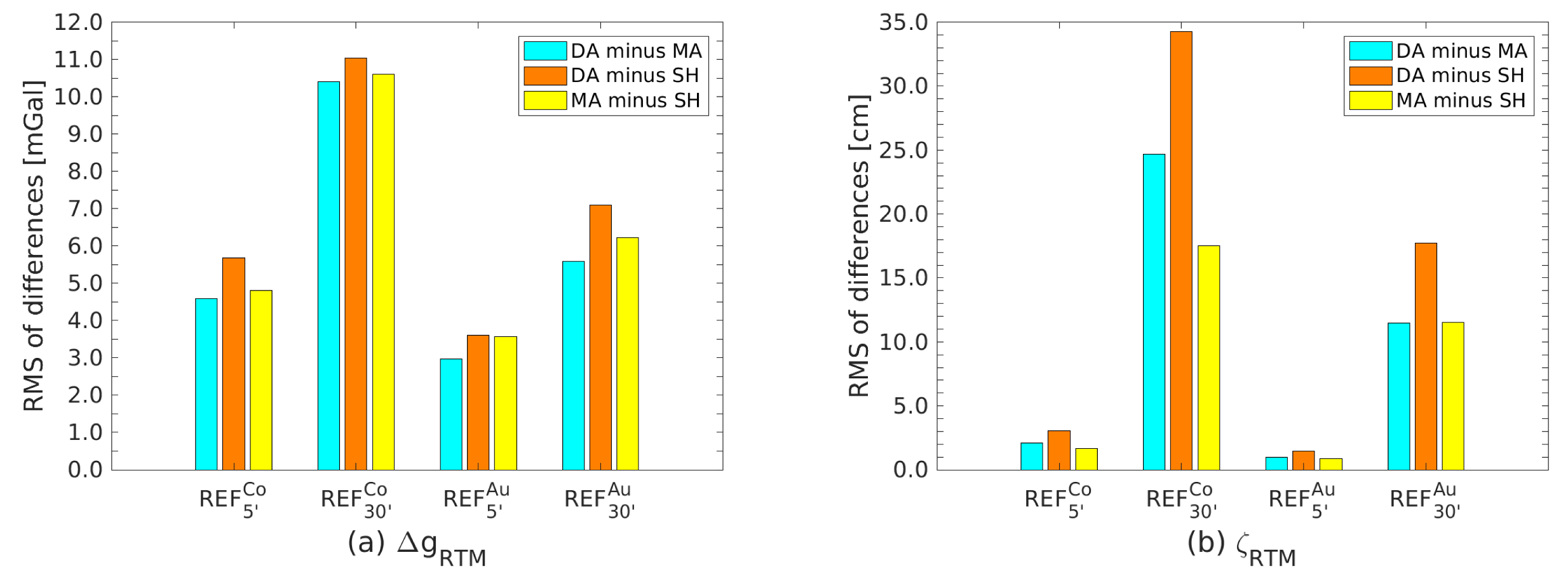

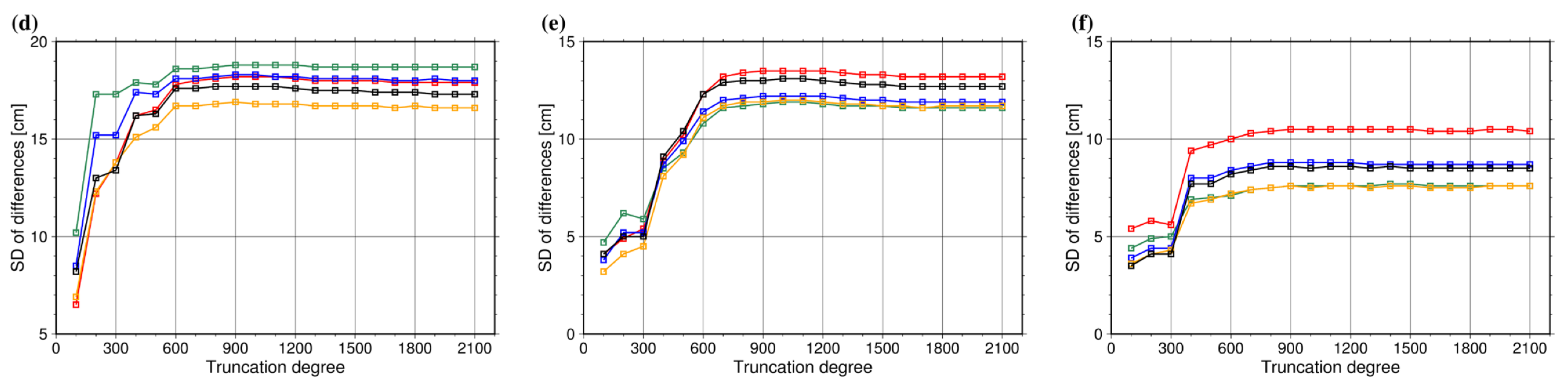
| Parameters | Scenarios | Remarks |
|---|---|---|
| DEM resolution combination | 3″ + 3″ 3″ + 30″ 3″ + 1′ 3″ + 2′ 3″ + 3′ 3″ + 4′ 3″ + 5′ | In this case, the values of r1 and r2, the type of reference topography are pre-fixed |
| Integration radius r1 | r1 = 10 km r1 = 20 km r1 = 30 km r1 = 40 km r1 = 50 km | In this case, the DEM resolution combination scenario, the value of r2, and the type of reference topography are pre-fixed |
| Integration radius r2 | r2 = 56 km r2 = 111 km r2 = 167 km r2 = 222 km r2 = 278 km | In this case, the DEM resolution combination scenario, the value of r1, and the type of reference topography are pre-fixed |
| Reference topography | DA approach MA approach SH approach | In this case, the DEM resolution combination scenario, the values of r1 and r2 are pre-fixed |
| Residual Gravity Anomaly | Reference Topography | Mean | SD | RMS | Min | Max |
|---|---|---|---|---|---|---|
| 1.369 | 5.961 | 6.117 | −39.698 | 43.531 | ||
| 0.283 | 5.450 | 5.458 | −33.184 | 38.287 | ||
| 0.344 | 3.527 | 3.544 | −27.436 | 29.674 | ||
| 0.287 | 15.681 | 15.684 | −57.729 | 54.017 | ||
| −1.953 | 14.332 | 14.464 | −51.354 | 54.711 | ||
| 0.201 | 10.007 | 10.009 | −40.868 | 55.576 |
| Residual Gravity Anomaly | Reference Topography | Mean | SD | RMS | Min | Max |
|---|---|---|---|---|---|---|
| 0.540 | 4.644 | 4.676 | −63.663 | 64.943 | ||
| 0.195 | 4.668 | 4.672 | −63.417 | 65.381 | ||
| 0.296 | 2.730 | 2.746 | −19.592 | 21.382 | ||
| 1.454 | 9.623 | 9.732 | −50.121 | 72.149 | ||
| −0.406 | 9.082 | 9.091 | −42.874 | 52.153 | ||
| −0.215 | 7.564 | 7.567 | −27.356 | 49.728 |
| Quasi-Geoid Model | Integration Radius r2 | Truncation Degree | Mean | SD | RMS | Min | Max |
|---|---|---|---|---|---|---|---|
| / | / | −31.3 | 22.2 | 38.3 | −73.1 | 29.8 | |
| 222 km | / | −46.3 | 24.0 | 52.1 | −95.6 | −5.0 | |
| 222 km | / | −28.2 | 16.1 | 32.5 | −58.5 | 4.3 | |
| 222 km | / | −22.0 | 12.0 | 25.1 | −47.9 | 3.2 | |
| 222 km | 100 | −47.8 | 9.5 | 48.7 | −65.3 | −24.5 | |
| 222 km | 200 | −24.5 | 6.3 | 25.3 | −39.5 | −10.4 | |
| 222 km | 200 | −16.4 | 4.5 | 17.0 | −25.9 | −3.5 |
| Quasi-Geoid Model | Integration Radius r2 | Truncation Degree | Mean | SD | RMS | Min | Max |
|---|---|---|---|---|---|---|---|
| / | / | −9.9 | 17.1 | 19.7 | −54.6 | 27.9 | |
| 56 km | / | −13.6 | 17.9 | 22.4 | −53.3 | 22.4 | |
| 222 km | / | −12.6 | 11.7 | 17.2 | −41.4 | 15.1 | |
| 278 km | / | −9.7 | 8.6 | 12.9 | −24.4 | 11.7 | |
| 56 km | 100 | −12.9 | 6.5 | 14.5 | −29.5 | −0.1 | |
| 222 km | 100 | −12.7 | 3.2 | 13.1 | −22.9 | −6.4 | |
| 278 km | 100 | −9.7 | 3.5 | 10.3 | −19.1 | −0.4 |
| Quasi-Geoid Model | Truncation Degree | Mean | SD | RMS | Min | Max |
|---|---|---|---|---|---|---|
| / | −11.9 | 3.6 | 12.4 | −21.8 | −1.4 | |
| / | −9.5 | 2.7 | 9.9 | −18.2 | −2.6 | |
| / | −10.6 | 2.8 | 11.0 | −17.9 | −2.7 | |
| / | −11.9 | 2.4 | 12.1 | −17.2 | −4.2 | |
| 1000 | −10.2 | 1.9 | 10.4 | −17.5 | −5.4 | |
| 1100 | −10.9 | 2.1 | 11.1 | −16.2 | −3.7 | |
| 200 | −11.7 | 2.1 | 11.9 | −17.9 | −4.1 |
| Quasi-Geoid Model | Truncation Degree | Mean | SD | RMS | Min | Max |
|---|---|---|---|---|---|---|
| / | −9.8 | 3.8 | 10.5 | −18.8 | 4.7 | |
| / | −10.0 | 3.6 | 10.6 | −18.2 | 3.0 | |
| / | −9.9 | 3.6 | 10.5 | −18.2 | 3.7 | |
| / | −9.7 | 3.6 | 10.3 | −18.4 | 0.9 | |
| 100 | −10.4 | 3.1 | 10.9 | −17.9 | −2.4 | |
| 300 | −9.9 | 3.4 | 10.5 | −19.1 | −0.9 | |
| 100 | −10.1 | 3.2 | 10.6 | −17.9 | −0.6 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lin, M.; Yang, M.; Zhu, J. Experiences with the RTM Method in Local Quasi-Geoid Modeling. Remote Sens. 2023, 15, 3594. https://doi.org/10.3390/rs15143594
Lin M, Yang M, Zhu J. Experiences with the RTM Method in Local Quasi-Geoid Modeling. Remote Sensing. 2023; 15(14):3594. https://doi.org/10.3390/rs15143594
Chicago/Turabian StyleLin, Miao, Meng Yang, and Jianjun Zhu. 2023. "Experiences with the RTM Method in Local Quasi-Geoid Modeling" Remote Sensing 15, no. 14: 3594. https://doi.org/10.3390/rs15143594
APA StyleLin, M., Yang, M., & Zhu, J. (2023). Experiences with the RTM Method in Local Quasi-Geoid Modeling. Remote Sensing, 15(14), 3594. https://doi.org/10.3390/rs15143594






