The Deep Atmospheric Composition of Jupiter from Thermochemical Calculations Based on Galileo and Juno Data
Abstract
:1. Introduction
2. Material and Methods
2.1. Chemistry Modelling
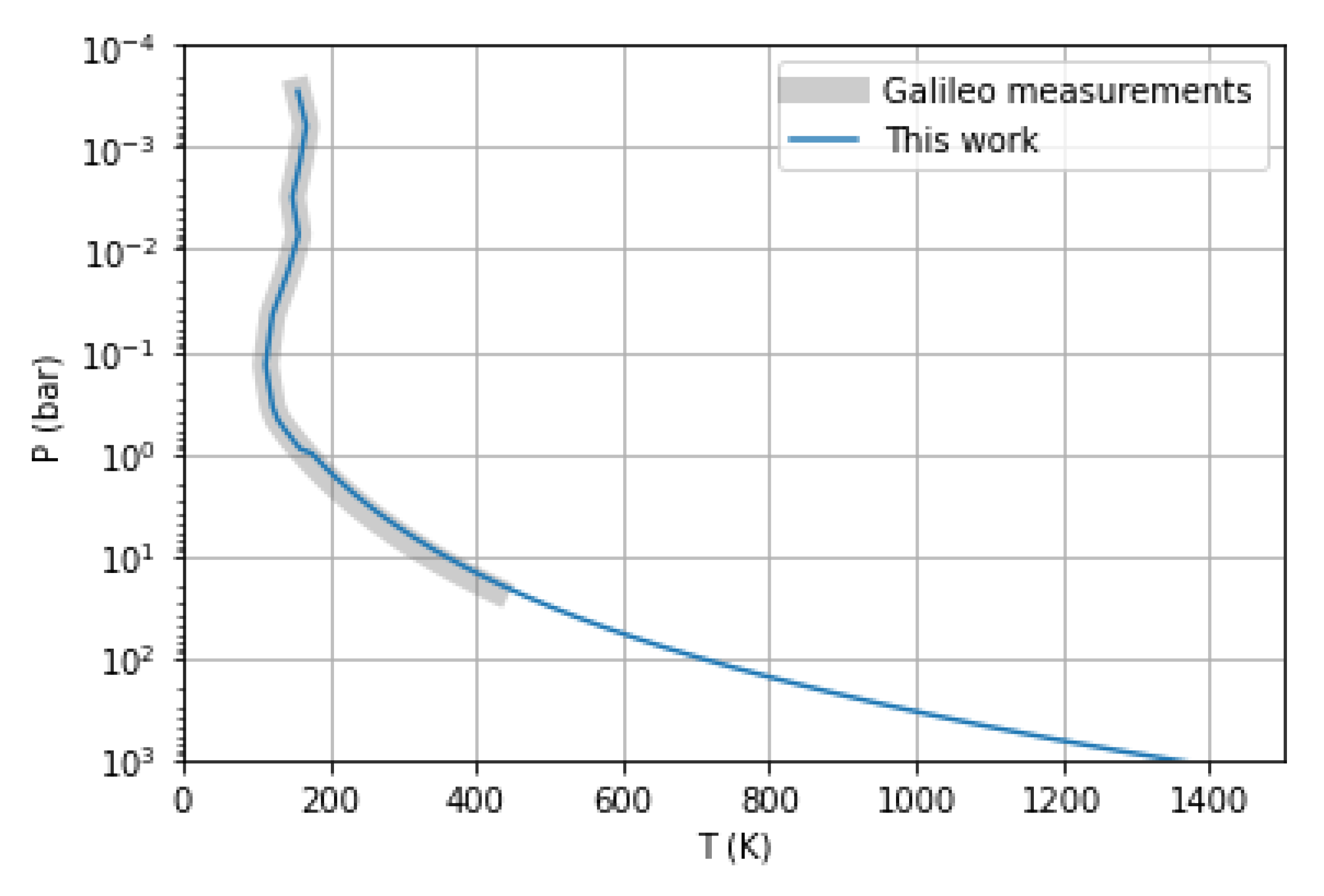
2.2. Thermal Profile
2.3. Elemental Abundances
| Molecule (g) | Galileo (1998) [37] * | Galileo (2004) [38] * | Juno (2020) [6] ** |
|---|---|---|---|
| HO | ≤0.033 ± 0.015 | 0.289 ± 0.096 | 2.7 |
| NH | ≤10 | 2.96 ± 1.13 | 2.76 |
| CH | 2.9 | 3.27 ± 0.78 | - |
| HS | 2.5 | 2.75 ± 0.66 | - |
2.4. Condensation Data
3. Results
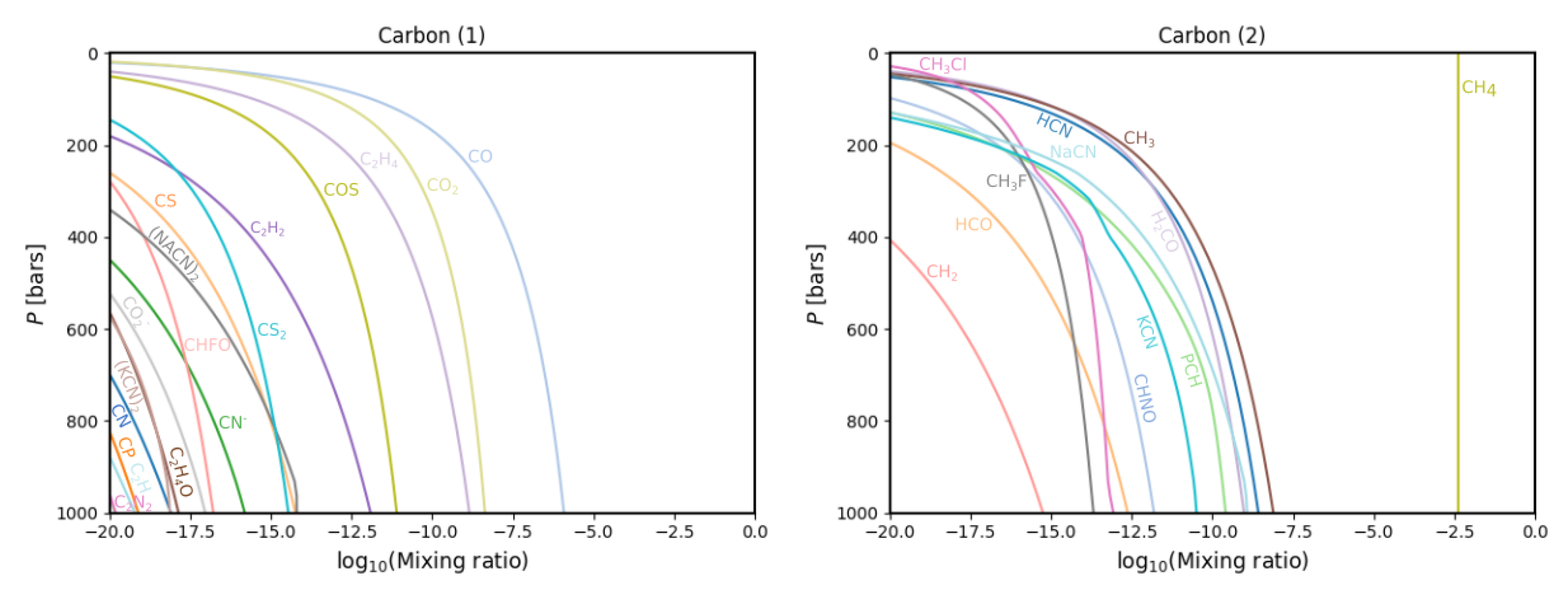
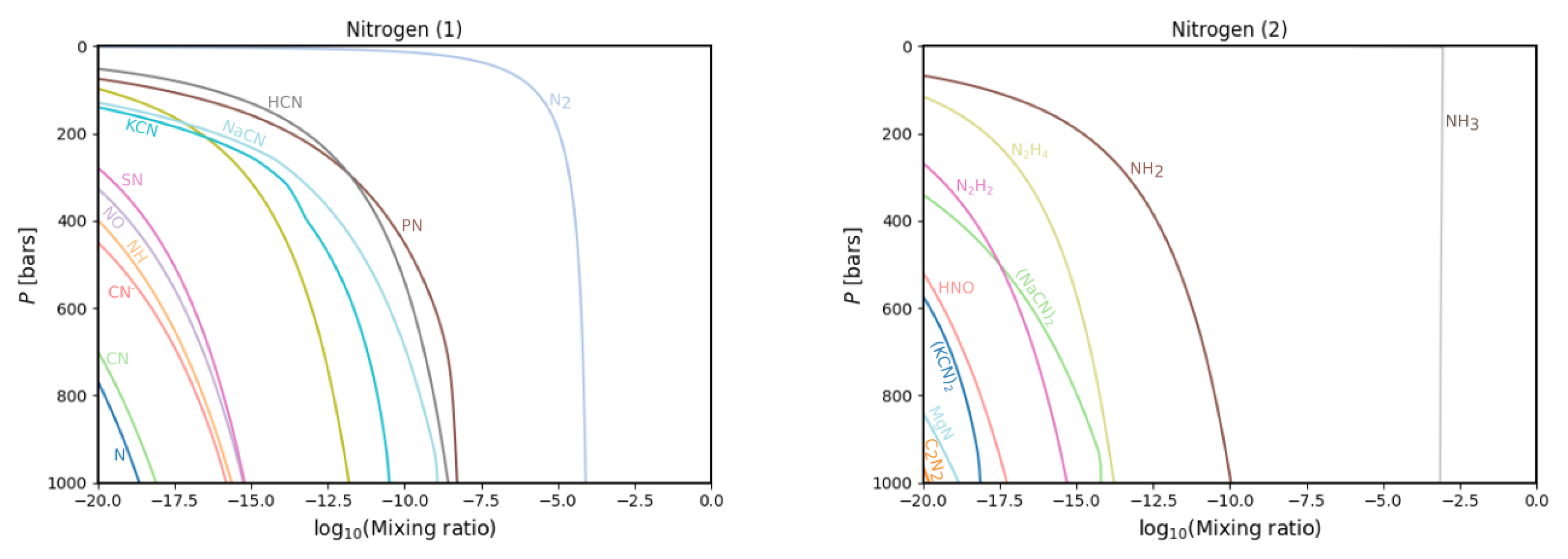
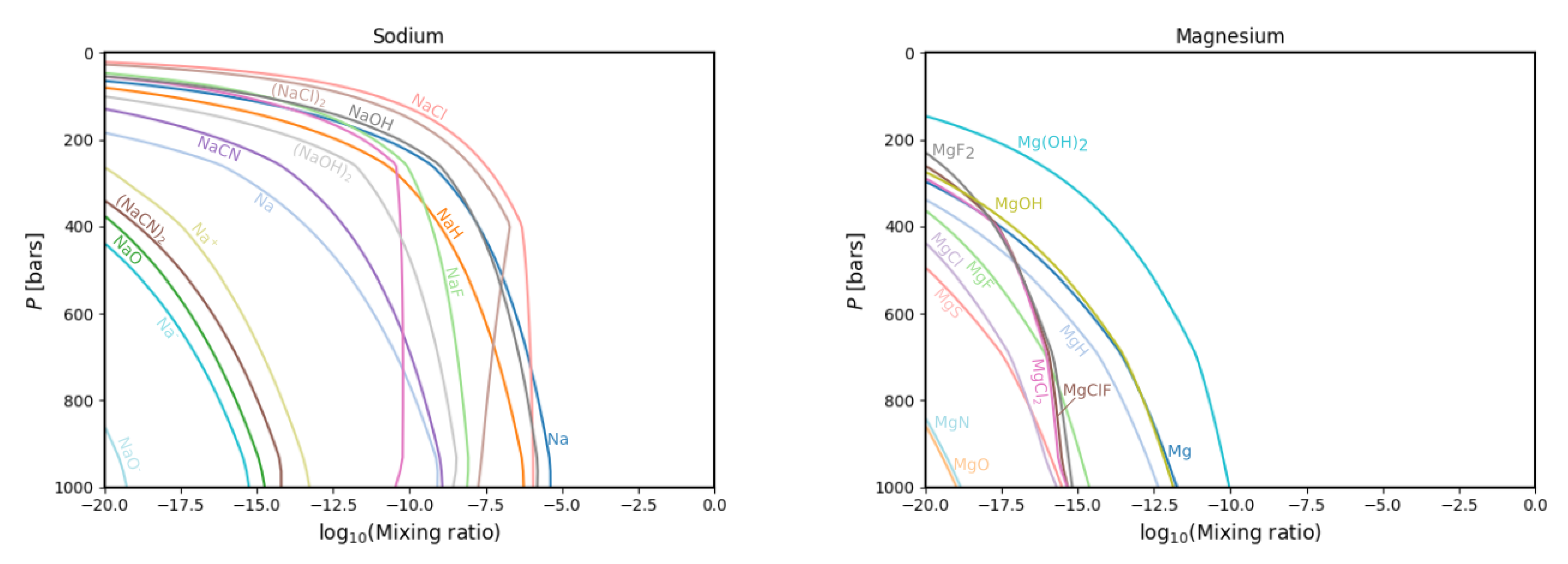
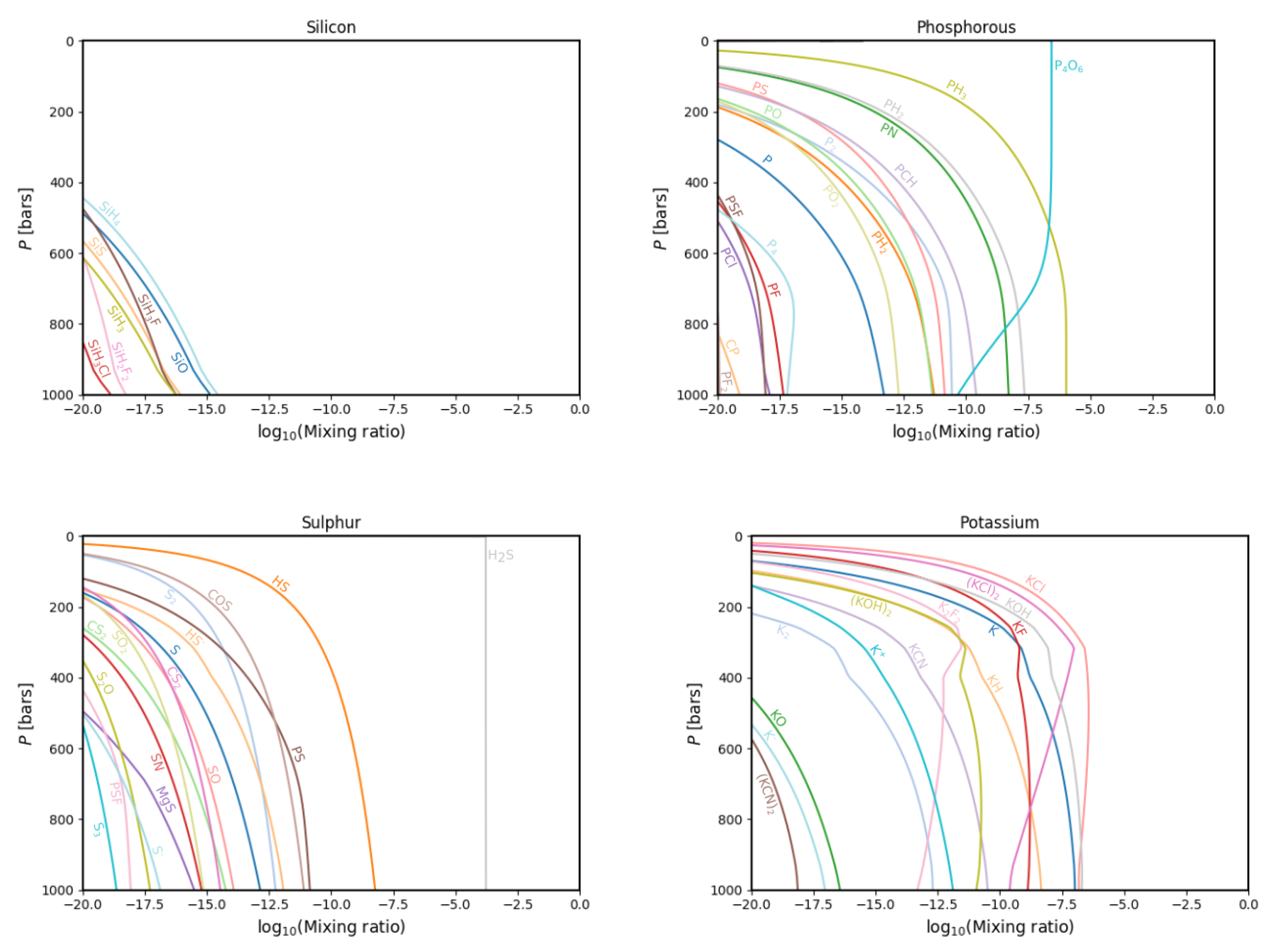
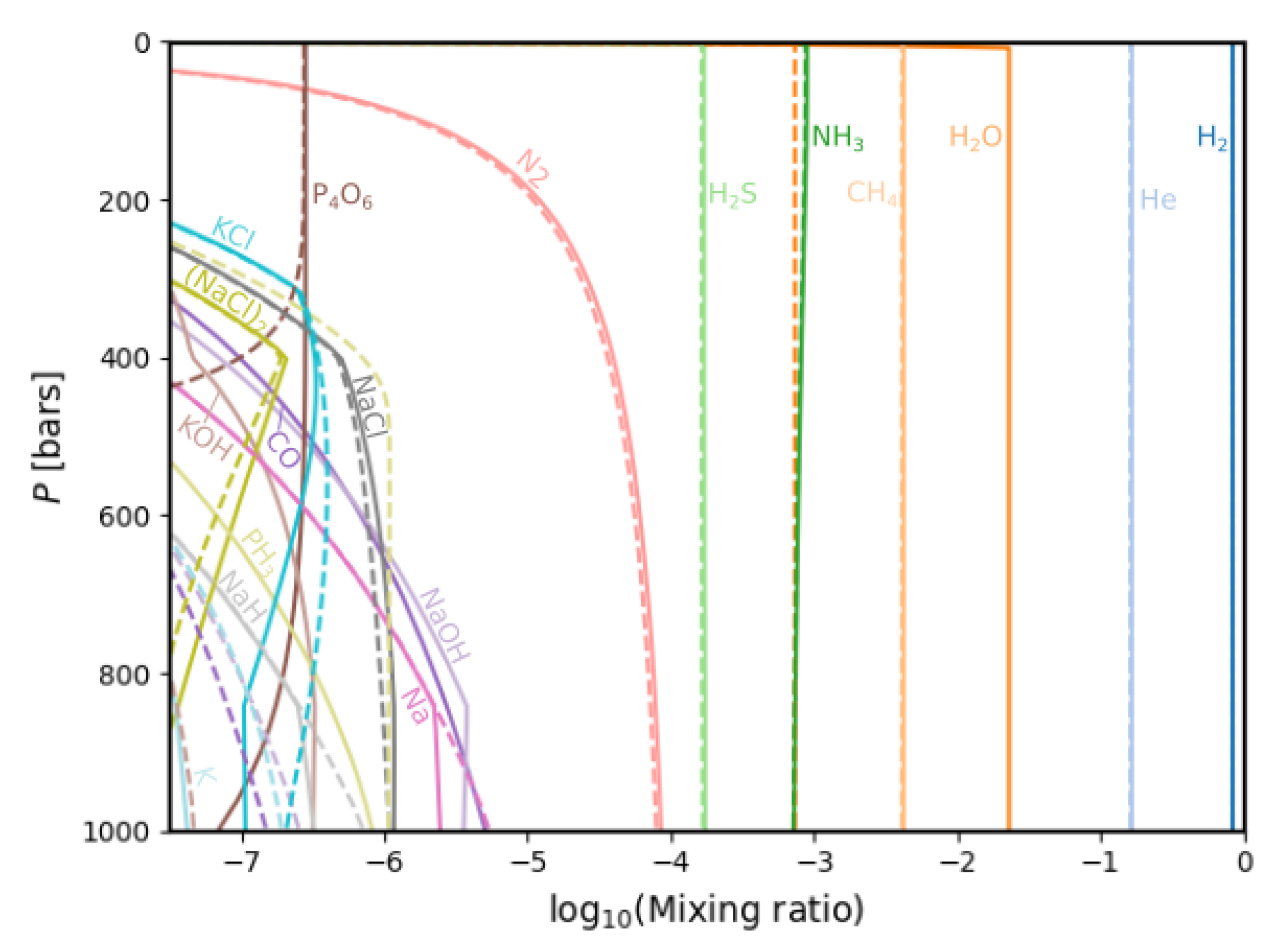
3.1. Gas Phase Chemistry
3.2. Comparisons with Previous Calculations
3.3. Condensation Chemistry
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. Elemental Abundances Used in the Calculations
| H | 12.0 |
| He | 10.98 |
| Li | 1.451727836017593 |
| C | 9.386275588677877 |
| N | 8.712636918082811 |
| O | 9.56309160017658 |
| F | 4.961727836017593 |
| Na | 6.641727836017593 |
| Mg | 8.001727836017594 |
| Al | 6.851727836017593 |
| Si | 7.911727836017593 |
| P | 5.811727836017593 |
| S | 8.001060529847855 |
| Cl | 5.901727836017593 |
| K | 5.431727836017593 |
| Ca | 6.741727836017593 |
| Ti | 5.351727836017593 |
| V | 4.331727836017593 |
| Cr | 6.041727836017593 |
| Mn | 5.831727836017593 |
| Fe | 7.901727836017593 |
| Co | 5.391727836017593 |
| Ni | 6.621727836017593 |
| Zr | 2.9817278360175923 |
| W | 1.2517278360175919 |
Appendix B. List of Condensed Species Considered in the Calculations
| Al2O3[s] | CORUNDUM(alpha) |
| Al2O3[l] | CORUNDUM(liquid) |
| MgAl2O4[s] | SPINEL |
| MgAl2O4[l] | SPINEL(liquid) |
| TiO2[s] | RUTILE |
| TiO2[l] | RUTILE(liquid) |
| Ti4O7[s] | TITANIUM-OXIDE |
| Ti4O7[l] | TITANIUM-OXIDE(liquid) |
| Mg2SiO4[s] | FOSTERITE |
| Mg2SiO4[l] | FOSTERITE(liquid) |
| MgSiO3[s] | ENSTATITE |
| MgSiO3[l] | ENSTATITE(liquid) |
| Fe[s] | IRON(alpha-delta) |
| Fe[l] | IRON(liquid) |
| Fe2SiO4[s] | FAYALITE |
| FeS[s] | TROILITE |
| FeS[l] | TROILITE(liquid) |
| MgTi2O5[s] | MG-DITITANATE |
| MgTi2O5[l] | MG-DITITANATE(liquid) |
| C[s] | GRAPHITE |
| TiC[s] | TITANIUM-CARBIDE |
| TiC[l] | TITANIUM-CARBIDE(liquid) |
| SiC[s] | SILICON-CARBIDE(alpha) |
| SiO[s] | SILICON-MONOXIDE |
| SiO2[s] | QUARTZ |
| SiO2[l] | QUARTZ(liquid) |
| Zr[s] | ZIRCONIUM(beta) |
| Zr[l] | ZIRCONIUM(liquid) |
| ZrO2[s] | BADDELEYITE |
| ZrO2[l] | BADDELEYITE(liquid) |
| ZrSiO4[s] | ZR-SILICATE |
| W[s] | TUNGSTEN |
| W[l] | TUNGSTEN(liquid) |
| WO3[s] | W-TRIOXIDE |
| WO3[l] | W-TRIOXIDE(liquid) |
| MgO[s] | PERICLASE |
| MgO[l] | PERICLASE(liquid) |
| FeO[s] | FERROPERICLASE |
| FeO[l] | FERROPERICLASE(liquid) |
| Na2SiO3[s] | NA-METASILICATE |
| Na2SiO3[l] | NA-METASILICATE(liquid) |
| H2O[s] | WATER-ICE |
| H2O[l] | WATER(liquid) |
| NH3[s] | AMONIA(liquid/solid) |
| CH4[s] | METHANE(liquid/solid) |
| CO[s] | C-MONOXIDE(liquid/solid) |
| CO2[s] | C-DIOXIDE(liquid/solid) |
| H2SO4[s] | SULPHURIC-ACID |
| H2SO4[l] | SULPHURIC-ACID(liquid) |
| Na[s] | SODIUM |
| Na[l] | SODIUM(liquid) |
| NaCl[s] | HALITE |
| NaCl[l] | HALITE(liquid) |
| KCl[s] | SYLVITE |
| KCl[l] | SYLVITE(liquid) |
| S[s] | SULPHUR |
| S[l] | SULPHUR(liquid) |
| MgS[s] | MG-SULPHIDE |
| LiCl[s] | LI-CHLORIDE |
| LiCl[l] | LI-CHLORIDE(liquid) |
| AlCl3[s] | AL-TRICHLORIDE |
| AlCl3[l] | AL-TRICHLORIDE(liquid) |
| CaO[s] | LIME |
| CaO[l] | LIME(liquid) |
| CaCl2[s] | CA-DICHLORIDE |
| CaCl2[l] | CA-DICHLORIDE(liquid) |
| LiH[s] | LI-HYDRIDE |
| LiH[l] | LI-HYDRIDE(liquid) |
| MgTiO3[s] | GEIKIELITE |
| MgTiO3[l] | GEIKIELITE(liquid) |
| K2SiO3[s] | K-SILICATE |
| K2SiO3[l] | K-SILICATE(liquid) |
| Ti[s] | TITANIUM(beta) |
| Ti[l] | TITANIUM(liquid) |
| TiO[s] | TI-MONOXIDE(beta) |
| TiO[l] | TI-MONOXIDE(liquid) |
| LiOH[s] | LI-HYDROXIDE |
| LiOH[l] | LI-HYDROXIDE(liquid) |
| VO[s] | V-MONOXIDE |
| VO[l] | V-MONOXIDE(liquid) |
| V2O3[s] | KARELIANITE |
| V2O4[s] | PARAMONTROSEITE |
| V2O5[s] | SHCHERBINAITE |
| CaS[s] | CALCIUM-SULFIDE |
| FeS2[s] | PYRITE |
| Na2S[s] | NA-SULFIDE |
| Mn[s] | MANGANESE(alpha-delta) |
| Mn[l] | MANGANESE(liquid) |
| MnS[s] | ALABANDITE |
| Ni[s] | NICKEL |
| Ni[l] | NICKEL(liquid) |
| Ni3S2[s] | HEAZLEWOODITE |
| Ni3S2[l] | HEAZLEWOODITE(liquid) |
| Cr[s] | CHROMIUM |
| Cr[l] | CHROMIUM(liquid) |
| CrN[s] | CARLSBERGITE |
| CaSiO3[s] | WOLLASTONITE |
| CaTiO3[s] | PEROVSKITE |
| NiS[s] | MILLERITE |
| NiS2[s] | VAESITE |
| Ca2Al2SiO7[s] | GEHLENITE |
| Ca3Al2Si3O12[s] | GROSSULAR |
| Ca2SiO4[s] | LARNITE |
| CaAl2SiO6[s] | Ca-TSCHERMAKS |
| Ca3Si2O7[s] | RANKINITE |
| Ca5P3O12F[s] | FLUORAPATITE |
| Ca3MgSi2O8[s] | MERWINITE |
| CaAl2Si2O8[s] | ANORTHITE |
| CaTiSiO5[s] | SPHENE |
| Ca2MgSi2O7[s] | AKERMANITE |
| Al2SiO5[s] | KYANITE |
| CaMgSiO4[s] | MONTICELLITE |
| CaMgSi2O6[s] | DIOPSIDE |
| MgAl2SiO6[s] | Mg-TSCHERMAKS |
| KMg3AlSi3O10F2[s] | FLUORPHLOGOPITE |
| Mg3Al2Si3O12[s] | PYROPE |
| Ca2Al3Si3O13H[s] | CLINOZOISITE |
| CaSi2O5[s] | CaSi-TITANITE |
| Ca5Si2CO11[s] | SPURRITE |
| KAlSi3O8[s] | MICROCLINE |
| Ca5P3O13H[s] | HYDROXYAPATITE |
| KAlSiO4[s] | KALSILITE |
| KAlSi2O6[s] | LEUCITE |
| NaAlSi3O8[s] | ALBITE |
| NaAlSi2O6[s] | JADEITE |
| NaAlSiO4[s] | NEPHELINE |
| Ca2MnAl2Si3O13H[s] | PIEMONTITE(ORDERED) |
| CaAl4Si2O12H2[s] | MARGARITE |
| Ca2Al2Si3O12H2[s] | PREHNITE |
| Ca2FeAl2Si3O13H[s] | EPIDOTE(ORDERED) |
| Ca5Si2C2O13[s] | TILLEYITE |
| Ca3Fe2Si3O12[s] | ANDRADITE |
| KMg2Al3Si2O12H2[s] | EASTONITE |
| Mn3Al2Si3O12[s] | SPESSARTIN |
| CaFeSi2O6[s] | HEDENBERGITE |
| Mg3Cr2Si3O12[s] | KNORRINGITE |
| K2Si4O9[s] | Si-WADEITE |
| Mg2Al2Si3O12H2[s] | TSCHERMAK-TALC |
| KAl3Si3O12H2[s] | MUSCOVITE |
| KMg3AlSi3O12H2[s] | PHLOGOPITE |
| NaAl3Si3O12H2[s] | PARAGONITE |
| AlSi2O6H[s] | PYROPHYLLITE |
| NaMg3AlSi3O12H2[s] | SODAPHLOGOPITE |
| FeAl2O4[s] | HERCYNITE |
| Mg3Si4O12H2[s] | TALC |
| KMgAlSi4O12H2[s] | CELADONITE |
| NaCrSi2O6[s] | KOSMOCHLOR |
| Ca2FeAlSi3O12H2[s] | FERRI-PREHNITE |
| MnTiO3[s] | PYROPHANITE |
| Ca2Fe2AlSi3O13H[s] | Fe-EPIDOTE |
| MgAl2SiO7H2[s] | Mg-CHLORITOID |
| MnSiO3[s] | PYROXMANGITE |
| CaAl2Si4O14H4[s] | WAIRAKITE |
| KAlSi3O9H2[s] | K-CYMRITE |
| Fe3Al2Si3O12[s] | ALMANDINE |
| Al2SiO6H2[s] | HYDROXY-TOPAZ |
| KFeAlSi4O12H2[s] | FERROCELADONITE |
| NaFeSi2O6[s] | ACMITE |
| MnAl2SiO7H2[s] | Mn-CHLORITOID |
| NaAlSi2O7H2[s] | ANALCITE |
| CaAl2Si2O10H4[s] | LAWSONITE |
| MgCr2O4[s] | PICROCHROMITE |
| Mn2SiO4[s] | TEPHROITE |
| KMn3AlSi3O12H2[s] | Mn-BIOTITE |
| MgAl2Si2O10H4[s] | MAGNESIOCARPHOLITE |
| FeTiO3[s] | ILMENITE |
| AlO2H[s] | DIASPORE |
| FeAl2SiO7H2[s] | Fe-CHLORITOID |
| Mg7Si2O14H6[s] | PHASEA |
| CaCO3[s] | CALCITE |
| Mg3Si2O9H4[s] | LIZARDITE |
| Al2Si2O9H4[s] | KAOLINITE |
| FeSiO3[s] | FERROSILITE |
| CaSO4[s] | ANHYDRITE |
| KFe3AlSi3O12H2[s] | ANNITE |
| CaMgC2O6[s] | DOLOMITE |
| CaAl2Si4O16H8[s] | LAUMONTITE |
| FeAl2Si2O10H4[s] | FERROCARPHOLITE |
| Fe3Si4O12H2[s] | MINNESOTAITE |
| Cr2O3[s] | ESKOLAITE |
| MgCO3[s] | MAGNESITE |
| Fe2TiO4[s] | ULVOSPINEL |
| MgFe2O4[s] | MAGNESIOFERRITE |
| CaFeC2O6[s] | ANKERITE |
| MnO[s] | MANGANOSITE |
| NaAlCO5H2[s] | DAWSONITE |
| Mn2O3[s] | BIXBYITE |
| MgO2H2[s] | BRUCITE |
| Fe3Si2O9H4[s] | GREENALITE |
| MnCO3[s] | RHODOCHROSITE |
| Fe2O3[s] | HEMATITE |
| Fe3O4[s] | MAGNETITE |
| FeCO3[s] | SIDERITE |
| FeO2H[s] | GOETHITE |
| NiO[s] | NICKEL |
| CuO[s] | TENORITE |
| Cu2O[s] | CUPRITE |
| Cu[s] | COPPER |
| NH4Cl[s] | AMMONIUM-CHLORIDE |
| NH4SH[s] | AMMONIUM-HYDROSULFIDE |
| H2S[s] | HYDROGEN-SULFIDE(liquid/solid) |
| S2[s] | Disulfer(liquid/solid) |
| S8[s] | Octasulfur(liquid/solid) |
| P[s] | PHOSPHORUS_WHITE |
| P[l] | PHOSPHORUS(liquid) |
| P4O10[s] | PHOSPHORUS-OXIDE |
| P4S3[s] | PHOSPHORUS-SULFIDE |
| P4S3[l] | PHOSPHORUS-SULFIDE(liquid) |
| Zn[s] | ZINC |
| Zn[l] | ZINC(liquid) |
| ZnSO4[s] | ZINC-SULFATE |
| ZnS[s] | SPHALERITE/WURTZITE |
| H3PO4[s] | PHOSPHORIC-ACID |
| H3PO4[l] | PHOSPHORIC-ACID |
| Mg3P2O8[s] | MAGNESIUM-PHOSPHATE |
| P3N5[s] | PHOSPHORUS-NITRIDE |
| AlF3[s] | ALUMINUM-FLUORIDE |
| CaF2[s] | FLUORITE |
| KF[s] | POTASSIUM-FLUORIDE |
| NaF[s] | SODIUM-FLUORIDE |
| FeF2[s] | IRON-FLUORIDE |
| MgF2[s] | MAGNESIUM-FLUORIDE |
| MgF2[l] | MAGNESIUM-FLUORIDE |
| HF2K[s] | POTASSIUM-BIFLUORIDE |
| AlF6Na3[s] | CRYOLITE |
| Li2SiO3[s] | LI-SILICATE |
| Li2SiO3[l] | LI-SILICATE |
| Li2Si2O5[s] | LI-SILICATE |
| Li2Si2O5[l] | LI-SILICATE |
| Li2TiO3[s] | LI-TITANATE |
| Li2TiO3[l] | LI-TITANATE |
| Co[s] | COBALT |
| Co[l] | COBALT(liquid) |
| CoO[s] | COBALT-MONOOXIDE |
| Ti3O5[s] | TITANIUM-OXIDE |
| Ti3O5[l] | TITANIUM-OXIDE(liquid) |
| Mg2TiO4[s] | QANDILIT |
| Mg2TiO4[l] | QANDILIT(liquid) |
| TiN[s] | TITANIUM-NITRIDE |
| TiN[l] | TITANIUM-NITRIDE(liquid) |
| TiF4[s] | TITANIUM-TETRAFLUORIDE |
| TiF3[s] | TITANIUM-TRIFLUORIDE |
| TiCl4[s] | TITANIUM-TETRACHLORIDE |
| TiCl4[l] | TITANIUM-TETRACHLORIDE(liquid) |
| TiCl3[s] | TITANIUM-TRICHLORIDE |
| TiCl2[s] | TITANIUM-DICHLORIDE |
| TiH2[s] | TITANIUM-HYDRIDE |
| FeCl2[s] | IRON-DICHLORIDE |
| FeCl2[l] | IRON-DICHLORIDE(liquid) |
| FeCl3[s] | IRON-TRICHLORIDE |
| FeCl3[l] | IRON-TRICHLORIDE(liquid) |
| AlO3H3[s] | GIBBSITE |
| Fe3O4[l] | MAGNETITE(liquid) |
References
- Guillot, T.; Cheng, L.; Scott, J.; Brown, S.; Ingersoll, A.; Janssen, M.; Levin, S.; Lunine, J.; Orton, G.; Steffes, P.; et al. Storms and the Depletion of Ammonia in Jupiter: II. Explaining the Juno Observations. J. Geophys. Sci. Planets 2020, 125, e2020JE006404. [Google Scholar] [CrossRef]
- Guillot, T.; Cheng, L.; Scott, J.; Brown, S.; Ingersoll, A.; Janssen, M.; Levin, S.; Lunine, J.; Orton, G.; Steffes, P.; et al. Storms and the Depletion of Ammonia in Jupiter: I. Microphysics of ‘Mushballs’. J. Geophys. Sci. Planets 2020, 125, e2020JE006403. [Google Scholar] [CrossRef]
- Mousis, O.; Marboeuf, U.; Lunine, J.I.; Alibert, Y.; Fletcher, L.N.; Orton, G.S.; Pauzat, F.; Ellinger, Y. Determination of the Minimum Masses of Heavy Elements in the Envelopes of Jupiter and Saturn. Astrophys. J. 2009, 696, 1348–1354. [Google Scholar] [CrossRef]
- Bolton, S.J.; Adriani, A.; Adumitroaie, V.; Allison, M.; Anderson, J.; Atreya, S.; Bloxham, J.; Brown, S.; Connerney, J.E.P.; DeJong, E.; et al. Jupiter’s interior and deep atmosphere: The initial pole-to-pole passes with the Juno spacecraft. Science 2017, 356, 821–825. [Google Scholar] [CrossRef]
- Grassi, D.; Adriani, A.; Mura, A.; Atreya, S.K.; Fletcher, L.N.; Lunine, J.I.; Orton, G.S.; Bolton, S.; Plainaki, C.; Sindoni, G.; et al. On the Spatial Distribution of Minor Species in Jupiter’s Troposphere as Inferred From Juno JIRAM Data. J. Geophys. Res. (Planets) 2020, 125, e06206. [Google Scholar] [CrossRef]
- Li, C.; Ingersoll, A.; Bolton, S.; Levin, S.; Janssen, M.; Atreya, S.; Lunine, J.; Steffes, P.; Brown, S.; Guillot, T.; et al. The water abundance in Jupiter’s equatorial zone. Nat. Astron. 2020, 4, 609–616. [Google Scholar] [CrossRef]
- Visscher, C. Mapping Jupiter’s Mischief. J. Geophys. Res. Planets 2020, 125, e2020JE006526. [Google Scholar] [CrossRef]
- Li, C.; Ingersoll, A.; Janssen, M.; Levin, S.; Bolton, S.; Adumitroaie, V.; Allison, M.; Arballo, J.; Bellotti, A.; Brown, S.; et al. The distribution of ammonia on Jupiter from a preliminary inversion of Juno microwave radiometer data. Geophys. Res. Lett. 2007, 44, 5317–5325. [Google Scholar] [CrossRef]
- Helled, R.; Lunine, J. Measuring Jupiter’s water abundance by Juno: The link between interior and formation models. Mon. Not. R. Astron. Soc. 2014, 441, 2273–2279. [Google Scholar] [CrossRef]
- Bosman, A.D.; Cridland, A.J.; Miguel, Y. Jupiter formed as a pebble pile around the N2 ice line. Astron. Astrophys. 2019, 632, L11. [Google Scholar] [CrossRef] [Green Version]
- Öberg, K.I.; Wordsworth, R. Jupiter’s Composition Suggests its Core Assembled Exterior to the N2 Snowline. Astron. J. 2019, 158, 194. [Google Scholar] [CrossRef]
- Wang, D.; Lunine, J.; Mousis, O. Modeling the disequilibrium species for Jupiter and Saturn: Implications for Juno and Saturn entry probe. Icarus 2016, 276, 21–38. [Google Scholar] [CrossRef]
- Zhang, Z.; Adumitroaie, V.; Allison, M.; Arballo, J.; Atreya, S.; Bjoraker, G.; Bolton, S.; Brown, S.; Fletcher, L.; Guillot, T.; et al. Residual Study: Testing Jupiter Atmosphere Models Against Juno MWR Observations. Earth Space Sci. 2020, 7, e2020EA001229. [Google Scholar] [CrossRef]
- Freedman, R.; Lustig-Yeager, J.; Fortney, J.; Lupu, R.; Marley, M.; Lodders, K. Gaseous Mean Opacities for Giant Planet and Ultracool Dwarf Atmospheres over a Range of Metallicities and Temperatures. Astrophys. J. Suppl. Ser. 2014, 214, 25. [Google Scholar] [CrossRef]
- Guillot, T.; Gautier, D.; Chabrier, G.; Mosser, B. Are the Giant Planets Fully Convective? Icarus 1994, 112, 337–353. [Google Scholar] [CrossRef]
- Barshay, S.; Lewis, J. Chemical structure of the deep atmosphere of Jupiter. Icarus 1978, 33, 593–611. [Google Scholar] [CrossRef]
- Carlson, B.; Prather, M.; Rossow, W. Cloud chemistry on Jupiter. Astrophys. J. 1987, 322, 559. [Google Scholar] [CrossRef]
- Fegley, B., Jr.; Lodders, K. Chemical Models of the Deep Atmospheres of Jupiter and Saturn. Icarus 1994, 110, 117–154. [Google Scholar] [CrossRef]
- Lunine, J.; Helled, R.; Stevenson, D.; Bolton, S.; Nettelmann, N.; Atreya, S.; Guillot, T.; Militzer, B.; Miguel, Y.; Hubbard, W. Revelations on Jupiter’s formation, evolution and interior: Challenges from Juno results. Icarus 2022, 378, 114937. [Google Scholar]
- Bahn, G.S.; Zukoski, E.E. Kinetics, Equilibria and Performance of High Temperature Systems: Proceedings of the First Conference; Butterworths: Petersburg, VA, USA, 1960. [Google Scholar]
- Zeleznik, F.J.; Gordon, S. An Analytical Investigation of Three General Methods for of Calculating Chemical Equilibrium Compositions; NASA: Washington, DC, USA, 1968.
- Woitke, P.; Helling, C.; Hunter, G.H.; Millard, J.D.; Turner, G.E.; Worters, M.; Blecic, J.; Stock, J.W. Equilibrium chemistry down to 100 K. Impact of silicates and phyllosilicates on carbon/oxygen ratio. Astron. Astrophys. 2018, 614, A1. [Google Scholar] [CrossRef]
- Herbort, O.; Woitke, P.; Helling, C.; Zerkle, A. The atmospheres of rocky exoplanets: II. Influence of surface composition on the diversity of cloud condensates. Astron. Astrophys. 2022, 658, A180. [Google Scholar] [CrossRef]
- Blecic, J.; Harrington, J.; Bowman, O.M. TEA: A Code Calculating Thermochemical Equilibrium Abundances. Astrophys. J. Suppl. Ser. 2016, 225, 4. [Google Scholar] [CrossRef]
- Blecic, J.; Harrington, J.; Bowman, O.M.; Thermochemical Equilibrium Abundances (TEA). 2014–2016. Available online: https://github.com/dzesmin/TEA (accessed on 12 December 2022).
- Miguel, Y.; Bazot, M.; Guillot, T.; Howard, S.; Galanti, E.; Kaspi, Y.; Hubbard, W.; Militzer, B.; Helled, R.; Atreya, S.; et al. Jupiter’s inhomogeneous envelope. Astron. Astrophys. 2022, 662, A18. [Google Scholar] [CrossRef]
- Guillot, T.; Morel, P. CEPAM: A code for modeling the interior of giant planets. Astron. Astrophys. Suppl. 1995, 109, 109–123. [Google Scholar]
- Kaspi, Y.; Galanti, E.; Hubbard, W.B.; Stevenson, D.J.; Bolton, S.J.; Iess, L.; Guillot, T.; Bloxham, J.; Connerney, J.E.P.; Cao, H.; et al. Jupiter’s atmospheric jet streams extend thousands of kilometres deep. Nature 2018, 555, 223–226. [Google Scholar] [CrossRef] [PubMed]
- Guillot, T.; Miguel, Y.; Militzer, B.; Hubbard, W.; Kaspi, Y.; Galanti, E.; Cao, H.; Helled, R.; Wahl, S.M.; Iess, L.; et al. A suppression of differential rotation in Jupiter’s deep interior. Nature 2018, 555, 227–230. [Google Scholar] [CrossRef]
- Gupta, P.; Atreya, S.; Steffes, P.; Fletcher, L.; Guillot, T.; Allison, M.; Bolton, S.; Helled, R.; Levin, S.; Li, C. Jupiter’s Temperature Structure: A Reassessment of the Voyager Radio Occultation Measurements. Planet. Sci. J. 2022, 3, 159. [Google Scholar] [CrossRef]
- Guillot, T.; Fletcher, L.N.; Helled, R.; Ikoma, M.; Line, M.R.; Parmentier, V. Giant Planets from the Inside-Out. arXiv 2022, arXiv:2205.04100. [Google Scholar]
- Anders, E.; Grevesse, N. Abundances of the elements: Meteoritic and solar. Geochim. Cosmochim. Acta 1989, 53, 197–214. [Google Scholar] [CrossRef]
- Asplund, M.; Grevesse, N.; Jacques, S.; Pat, S. The Chemical Composition of the Sun. Annu. Rev. Astron. Astrophys. 2009, 47, 481–522. [Google Scholar] [CrossRef]
- West, R.; Baines, K.; Friedson, A.; Banfield, D.; Ragent, B.; Taylor, F. Jupiter. The Planet, Satellites and Magnetosphere; Cambridge University Press: Cambridge, UK, 2004; Chapter 5. [Google Scholar]
- Lodders, K. Brown Dwarfs—Faint at Heart, Rich in Chemistry. Science 2004, 303, 323–324. [Google Scholar] [CrossRef]
- Ingersoll, A.; Kanamori, H.; Dowling, T. Atmospheric gravity waver from the impact of comet Shoemaker-Levy with Jupiter. Geophys. Res. Lett. 1994, 21, 1083–1086. [Google Scholar] [CrossRef]
- Niemann, H.; Atreya, S.; Carignan, G.; Donahue, T.; Haverman, J.; Harpold, D.; Hartle, R.; Hunten, D.; Kasprzak, W.; Mahaffy, P.; et al. The composition of the jovian atmosphere as determined by the Galileo probe mass spectrometer. J. Geophys. Res. 1998, 103, 22831–22845. [Google Scholar] [CrossRef]
- Wong, M.; Mahaffy, P.; Atreya, S.; Niemann, H.; Owen, C. Updated Galileo probe mass spectrometer measurements of carbon, oxygen, nitrogen and sulfur on Jupiter. Icarus 2004, 171, 153–170. [Google Scholar] [CrossRef]
- Herbort, O.; Woitke, P.; Helling, C.; Zerkle, A. The atmospheres of rocky exoplanets: I. Outgassing of common rock and the stability of liquid water. Astron. Astrophys. 2020, 636, A71. [Google Scholar] [CrossRef]
- Chase, M., Jr. NIST-JANAF Thermochemical Tables; American Institute of Physics for the National Institute of Standards and Technology: New York, NY, USA, 1998. [Google Scholar]
- Zimmer, K.; Zhang, Y.; Lu, P.; Chen, Y.; Zhang, G.; Dalkilic, M.; Zhu, C. SUPCRTBL: A revised and extended thermodynamic dataset and software package of SUPCRT92. Comput. Geosci. 2016, 90, 97–111. [Google Scholar] [CrossRef]
- Johnson, J.; Oelkers, E.; Helgeson, H. SUPCRT92—A software package for calculating the standard thermodynamic properties of minerals, gases, aqueous species, and reactions from 1 to bar to 5000-bar and 0C to 1000C. Comput. Geosci. 1992, 18, 899–947. [Google Scholar] [CrossRef]
- Miguel, Y.; Guillot, T.; Fayon, L. Jupiter internal structure: The effect of different equations of state. Astron. Astrophys. 2016, 596, A114. [Google Scholar] [CrossRef] [Green Version]


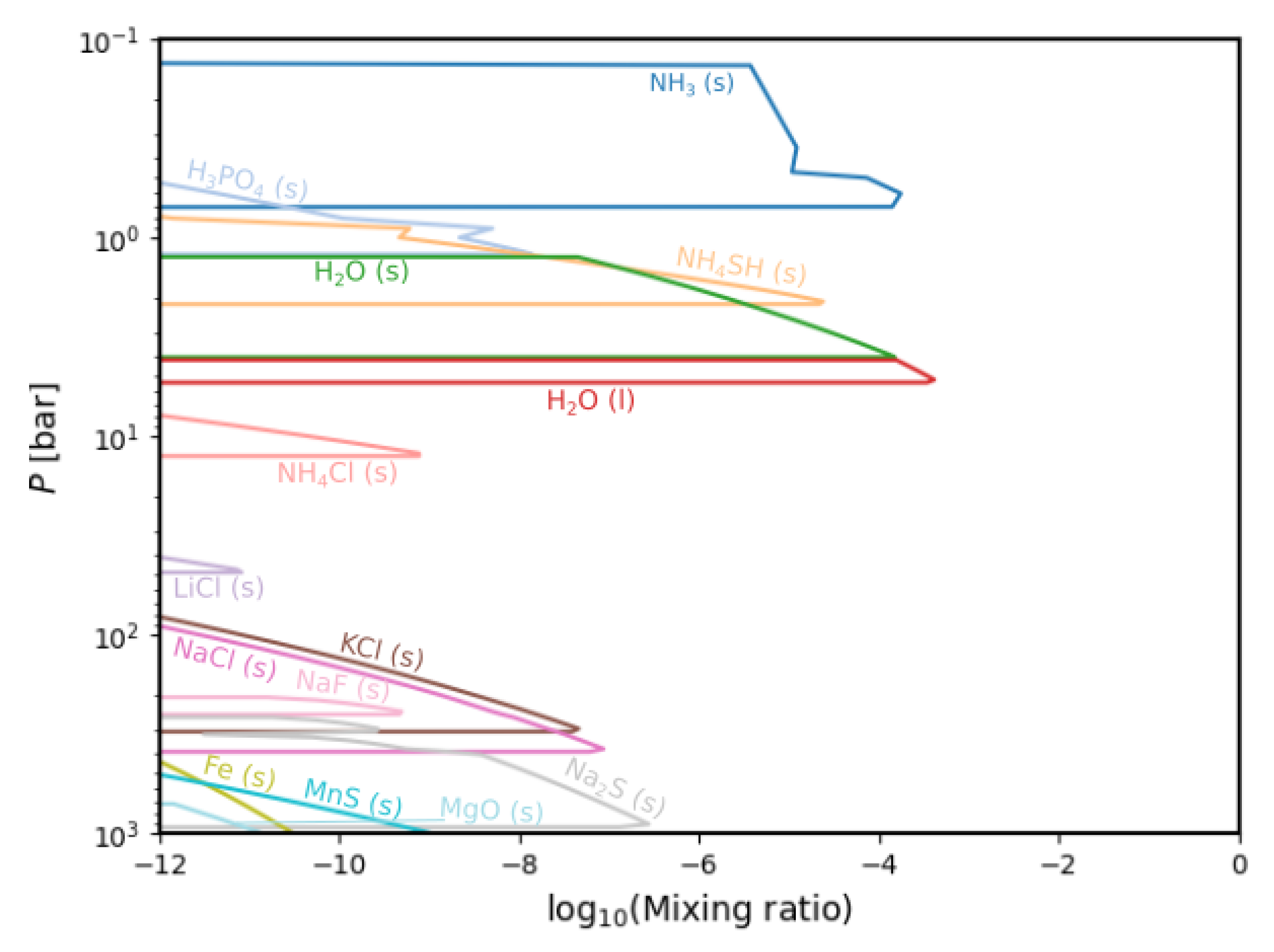

| Condensate | Formation Pressure (bar) | Formation Temperature (K) |
|---|---|---|
| NH (s) | 0.70 | 148.04 |
| HPO (s) | 1.212 | 187.30 |
| NHSH (s) | 2.16 | 224.24 |
| HO (s) | 4.01 | 187.30 |
| HO (l) | 5.37 | 295.93 |
| NHCl (s) | 12.60 | 381.83 |
| LiCl (s) | 48.59 | 568.66 |
| NaF (s) | 251.94 | 919.88 |
| KCl (s) | 307.64 | 973.44 |
| NaCl (s) | 388.65 | 1093.48 |
| NaS (s) | 931.29 | 1321.77 |
| MgO (s) | 1262.16 | 1434.49 |
| MnS (s) | 1834.39 | 1583.10 |
| Fe (s) | 3176.41 | 1820.93 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rensen, F.; Miguel , Y.; Zilinskas, M.; Louca, A.; Woitke, P.; Helling, C.; Herbort, O. The Deep Atmospheric Composition of Jupiter from Thermochemical Calculations Based on Galileo and Juno Data. Remote Sens. 2023, 15, 841. https://doi.org/10.3390/rs15030841
Rensen F, Miguel Y, Zilinskas M, Louca A, Woitke P, Helling C, Herbort O. The Deep Atmospheric Composition of Jupiter from Thermochemical Calculations Based on Galileo and Juno Data. Remote Sensing. 2023; 15(3):841. https://doi.org/10.3390/rs15030841
Chicago/Turabian StyleRensen, Frank, Yamila Miguel , Mantas Zilinskas, Amy Louca, Peter Woitke, Christiane Helling, and Oliver Herbort. 2023. "The Deep Atmospheric Composition of Jupiter from Thermochemical Calculations Based on Galileo and Juno Data" Remote Sensing 15, no. 3: 841. https://doi.org/10.3390/rs15030841
APA StyleRensen, F., Miguel , Y., Zilinskas, M., Louca, A., Woitke, P., Helling, C., & Herbort, O. (2023). The Deep Atmospheric Composition of Jupiter from Thermochemical Calculations Based on Galileo and Juno Data. Remote Sensing, 15(3), 841. https://doi.org/10.3390/rs15030841






