Geolocation Accuracy Validation of High-Resolution SAR Satellite Images Based on the Xianning Validation Field
Abstract
1. Introduction
- The geometric performance of five representative high-resolution SAR satellites: ALOS, TerraSAR-X, Cosmo-SkyMed, RadarSat-2, and Chinese YG-3, is evaluated on the same benchmark with the rational function model (RFM) based on the Xianning validation field. The experimental analysis concerns the geolocation accuracies of the above satellite products and provides a reference for the application in the field of spaceborne SAR.
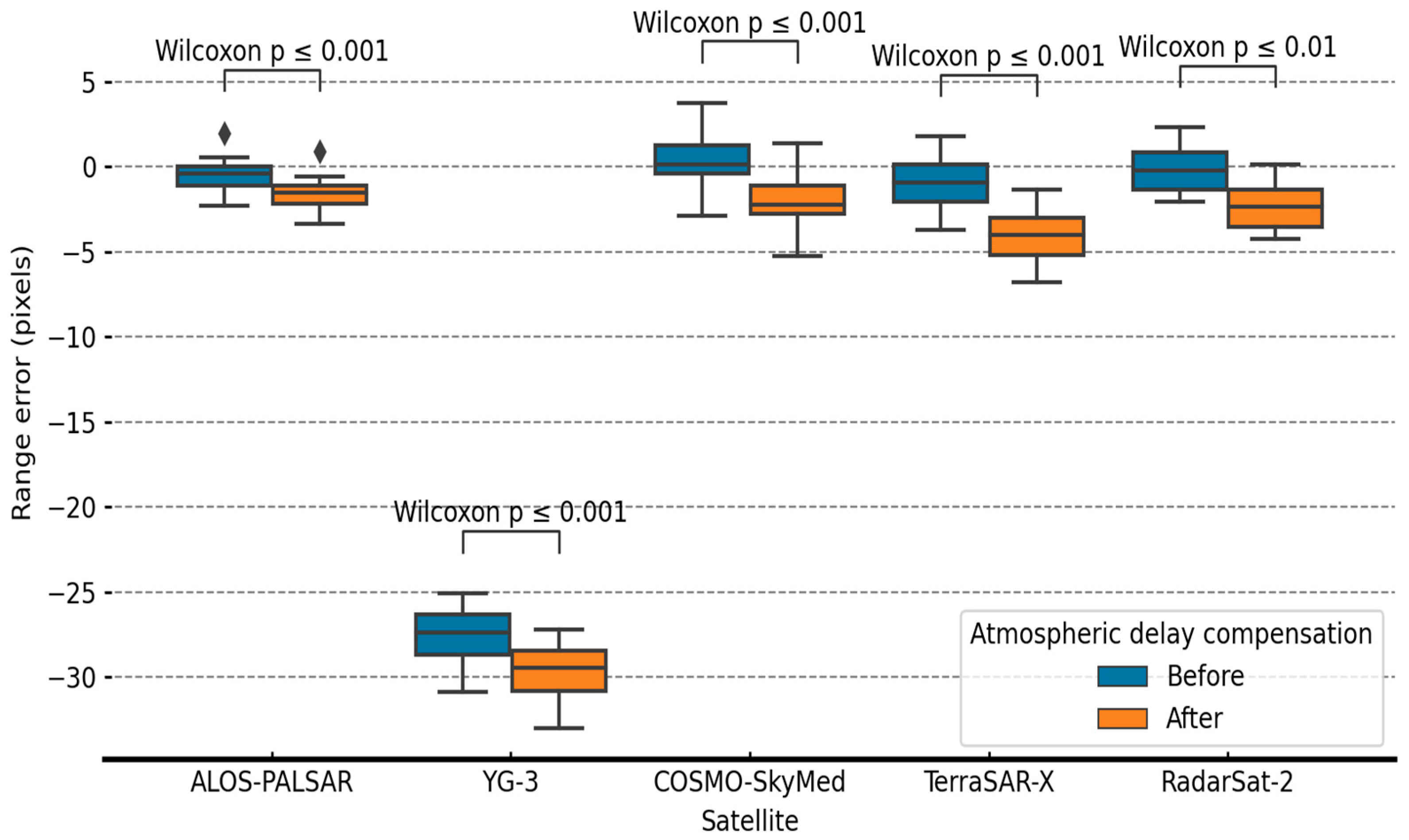
- An atmospheric delay correction model is used to evaluate the APD, and the experimental results show that a rough atmospheric correction result might have been added to the parameters of each satellite product in advance.
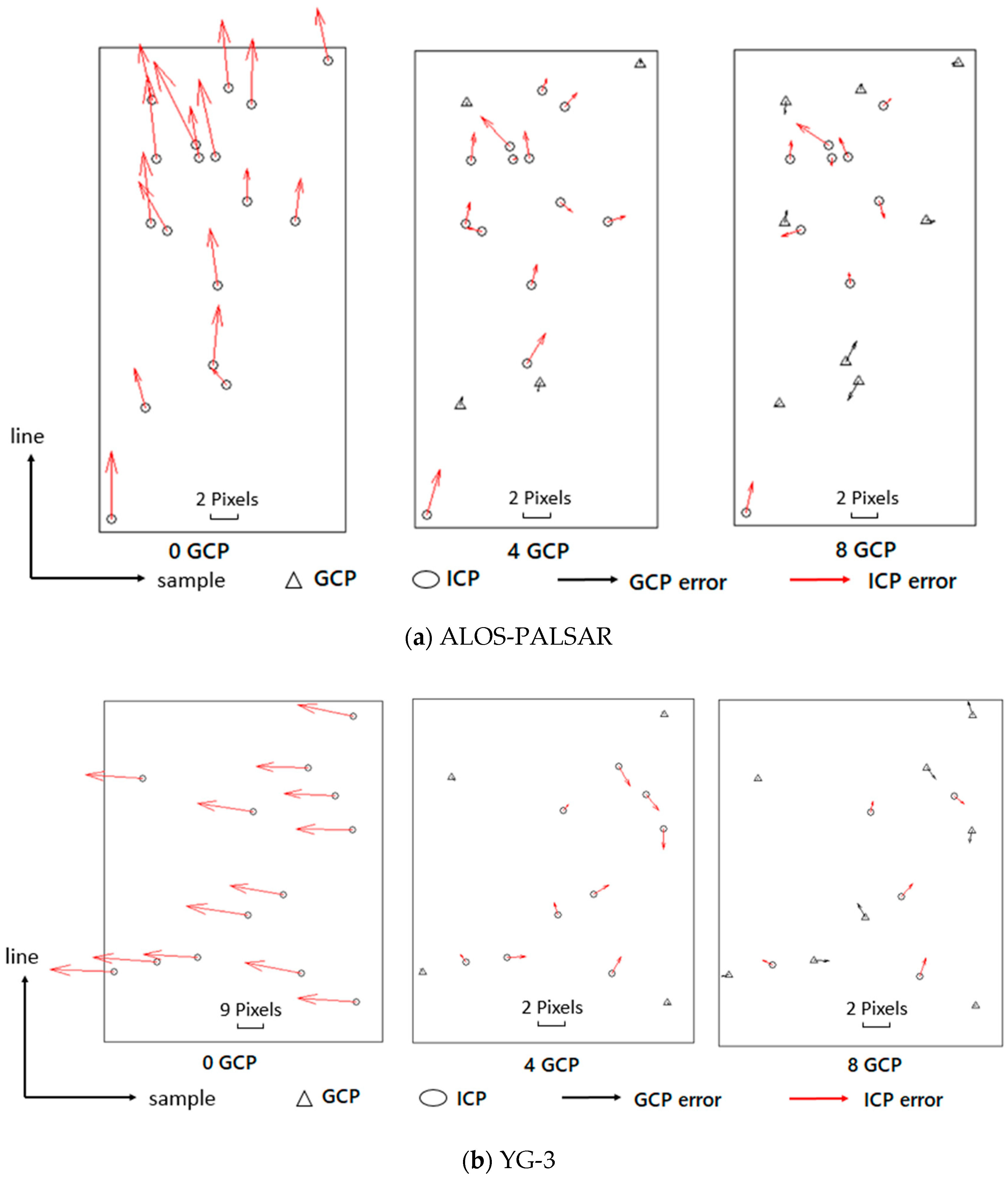
- A simulated images-based method is proposed to obtain ground control points (GCPs) for SAR images. It is proven effective by the experiment results, which provides an option for improving the geolocation accuracy on rough terrain areas.
2. Methods
2.1. Rational Function Model for SAR Images
2.2. Estimation of Atmospheric Propagation Delay
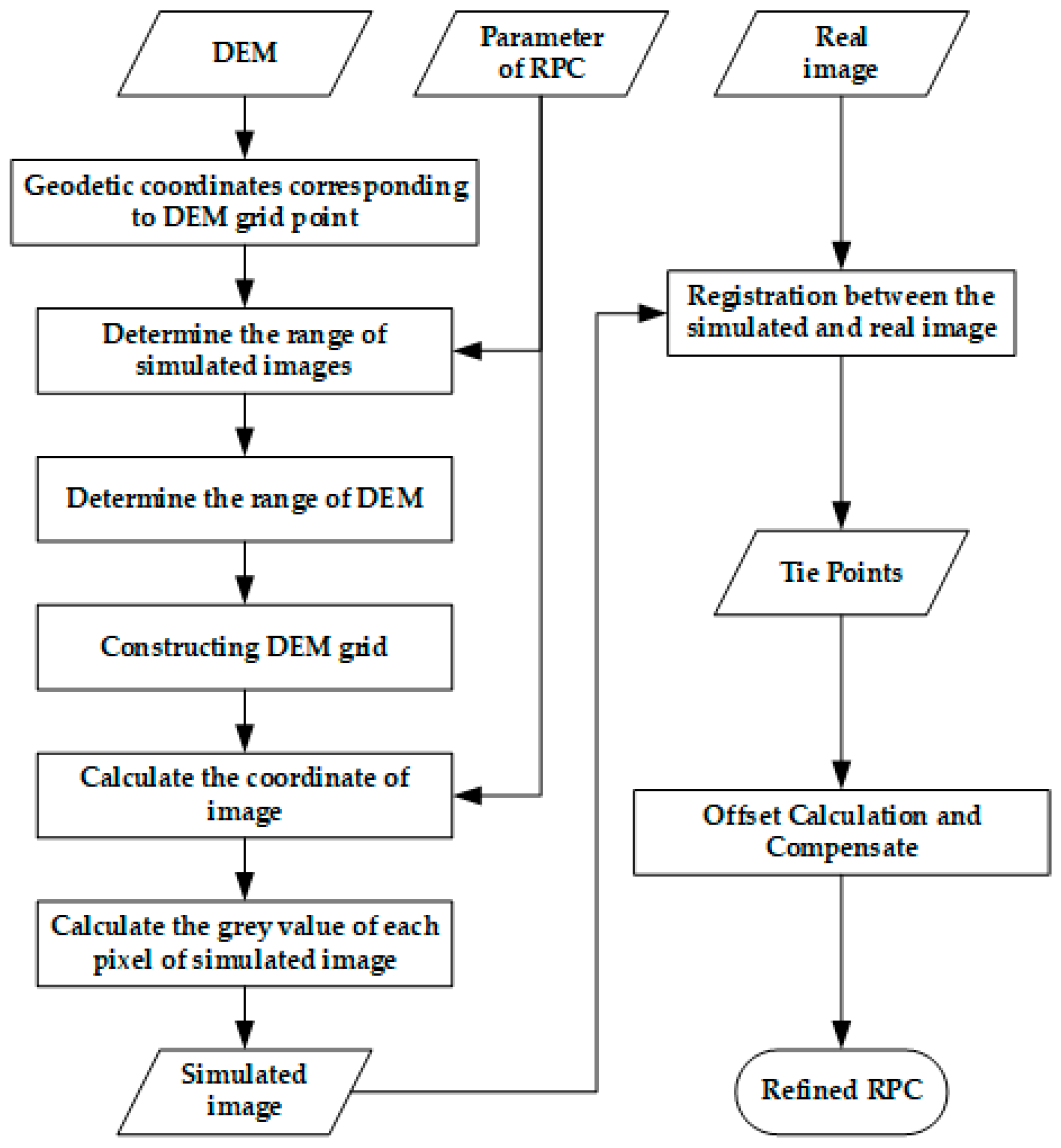
2.3. DEM-Based SAR Image Simulation
- Determine the range of the simulated image and the used DEM. Firstly, extract the covering areas of the DEM and convert them to geodetic coordinates under the WGS84 coordinate system via a transformation based on the RFM. Then, determine the range of the simulated image by combining the covering area of the DEM and real image.
- DEM interpolation and simulated image coordinate solution: Since the resolutions of the DEM and the real SAR image are inconsistent, a DEM with the same resolution as the real image is generated via bilinear interpolation. Then, the SAR image coordinates corresponding to each point on the DEM are solved by using the RFM, building the pixel-corresponding relation between the DEM and the simulated SAR image.
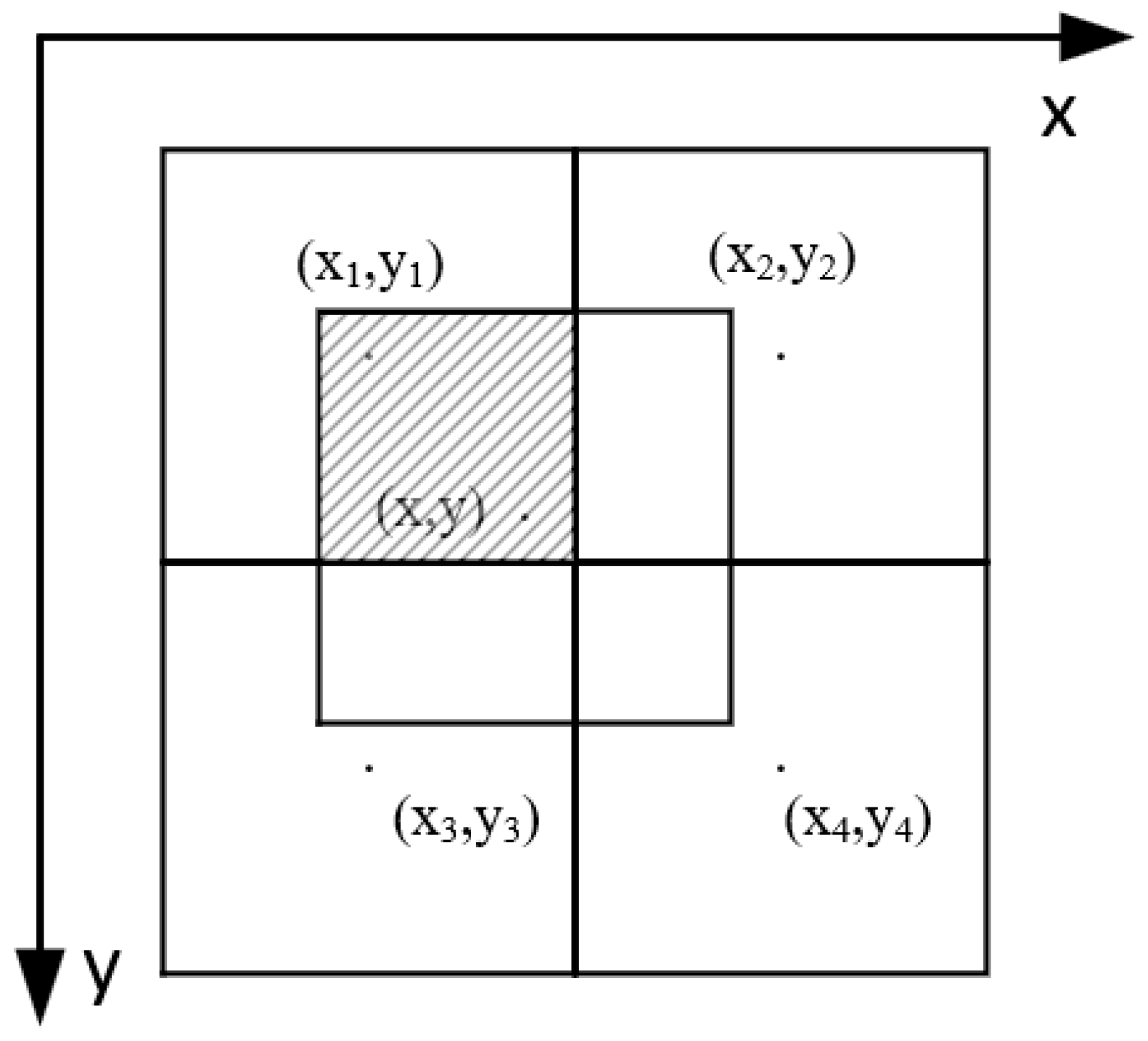
- Determine the backscattered power (grey value) of the simulated image. For one cell (X, Y, Z) in DEM, the coordinate of the corresponding pixel (x, y) in the simulated image can be calculated by using the RFM, and are grid points surrounding (x, y) (as shown in Figure 3). For each pixel in DEM, its contribution of grey value to four adjacent pixels is based on the size of the intersection area. As highlighted in Figure 3, the contribution of (x, y) to pixel is . Due to the imaging characteristics of SAR and the geometric distortions such as layover, perspective shrinkage, and shadow, the actual relationship may not be a one-to-one correspondence. Some pixels may have higher brightness when one pixel in the simulated image consists of multiple ground units [39].
3. Experiment and Result Analysis
3.1. Dataset and Test-Field Area
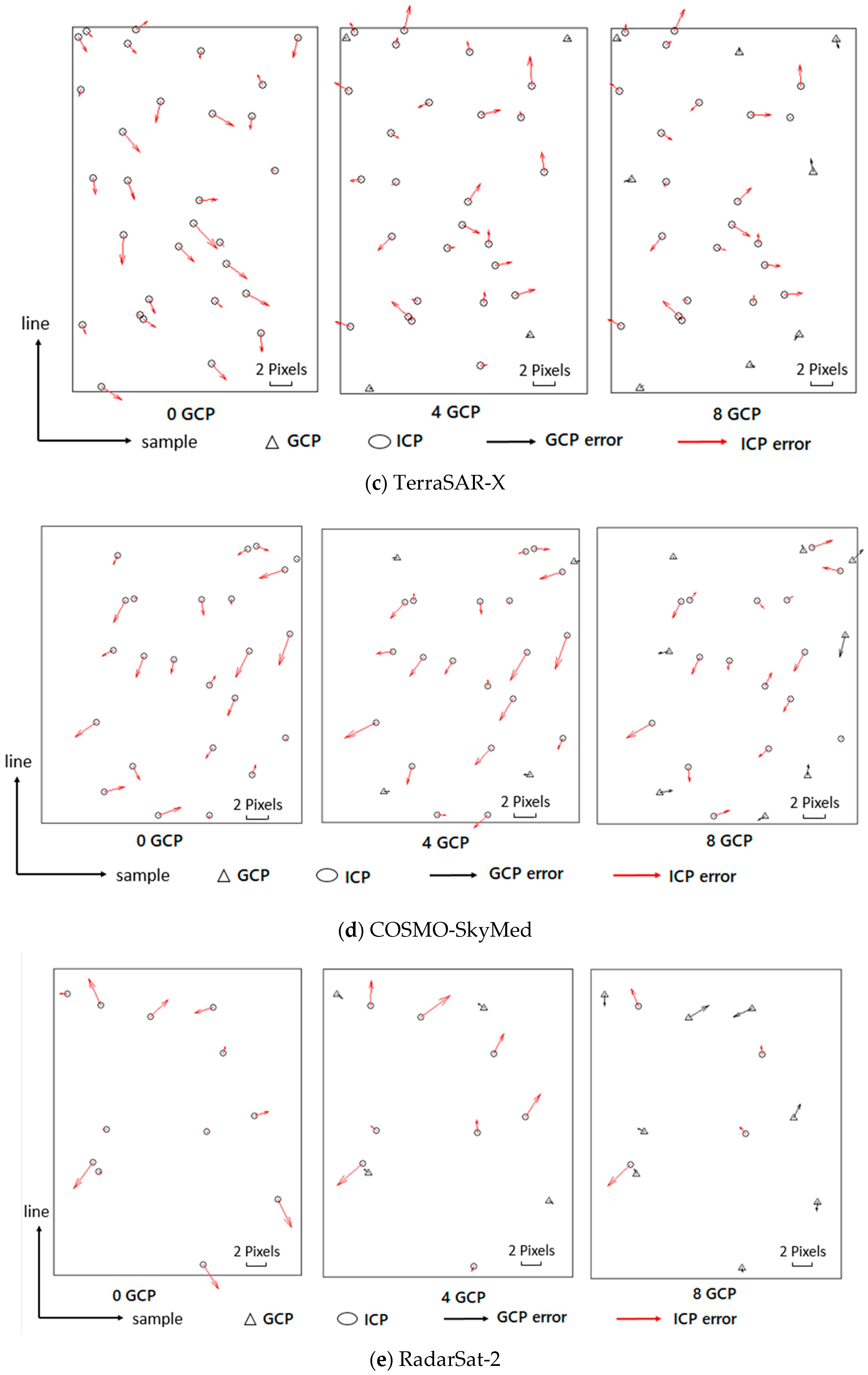
3.2. Accuracy Assessment with GPS Measured GCPs
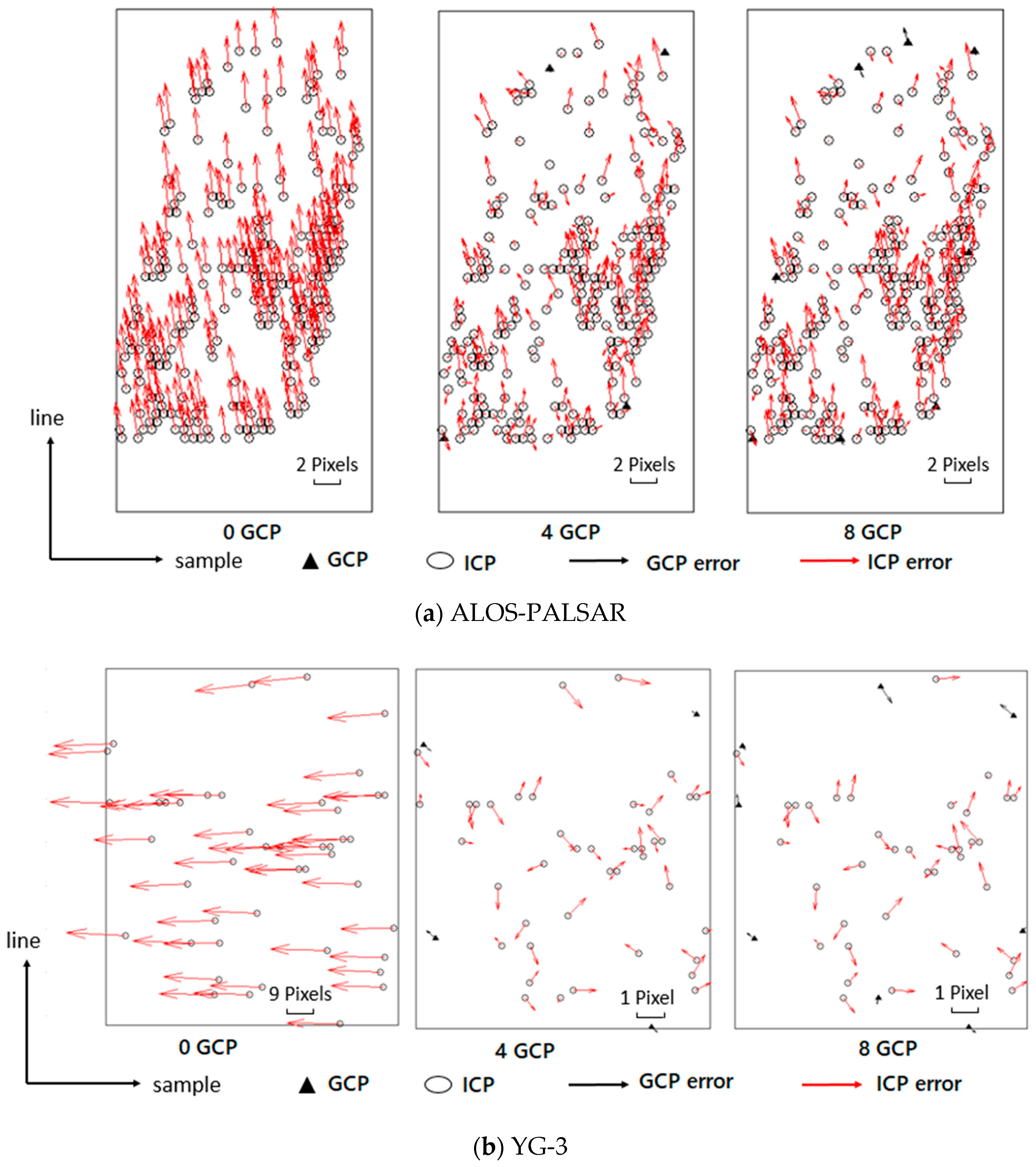
3.3. Accuracy Assessment with Simulated GCPs from Simulated Image
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Tang, L.; Tang, W.; Qu, X.; Han, Y.; Wang, W.; Zhao, B. A Scale-Aware Pyramid Network for Multi-Scale Object Detection in SAR Images. Remote Sens. 2022, 14, 973. [Google Scholar] [CrossRef]
- Zhao, B.; Han, Y.; Wang, H.; Tang, L.; Liu, X.; Wang, T. Robust Shadow Tracking for Video SAR. IEEE Geosci. Remote Sens. Lett. 2021, 18, 821–825. [Google Scholar] [CrossRef]
- Yoon, Y.T.; Eineder, M.; Yague-Martinez, N.; Montenbruck, O. TerraSAR-X Precise Trajectory Estimation and Quality Assessment. IEEE Trans. Geosci. Remote Sens. 2009, 47, 1859–1868. [Google Scholar] [CrossRef]
- Schubert, A.; Jehle, M.; Small, D.; Meier, E. Influence of Atmospheric Path Delay on the Absolute Geolocation Accuracy of TerraSAR-X High-Resolution Products. IEEE Trans. Geosci. Remote Sens. 2010, 48, 751–758. [Google Scholar] [CrossRef]
- Balss, U.; Eineder, M.; Fritz, T.; Breit, H.; Minet, C. Techniques for high accuracy relative and absolute localization of TerraSAR-X/TanDEM-X data. In Proceedings of the 2011 IEEE International Geoscience and Remote Sensing Symposium, IEEE, Vancouver, BC, Canada, 24–29 July 2011; pp. 2464–2467. [Google Scholar] [CrossRef]
- Eineder, M.; Minet, C.; Steigenberger, P.; Cong, X.; Fritz, T. Imaging Geodesy—Toward Centimeter-Level Ranging Accuracy with TerraSAR-X. IEEE Trans. Geosci. Remote Sens. 2011, 49, 661–671. [Google Scholar] [CrossRef]
- Cong, X.; Balss, U.; Eineder, M.; Fritz, T. Imaging Geodesy—Centimeter-Level Ranging Accuracy with TerraSAR-X: An Update. IEEE Geosci. Remote Sens. Lett. 2012, 9, 948–952. [Google Scholar] [CrossRef]
- Shimada, M. Ortho-Rectification and Slope Correction of SAR Data Using DEM and Its Accuracy Evaluation. IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2010, 3, 657–671. [Google Scholar] [CrossRef]
- Luscombe, A. Image quality and calibration of RADARSAT-2. In Proceedings of the 2009 IEEE International Geoscience and Remote Sensing Symposium, Cape Town, South Africa, 12–17 July 2009; Volume 2, pp. 757–760. [Google Scholar] [CrossRef]
- Williams, D.; LeDantec, P.; Chabot, M.; Hillman, A.; James, K.; Caves, R.; Thompson, A.; Vigneron, C.; Wu, Y. RADARSAT-2 Image Quality and Calibration Update. In Proceedings of the EUSAR 2014; 10th European Conference on Synthetic Aperture Radar, Berlin, Germany, 3–5 June 2014; pp. 1–4. [Google Scholar]
- Schubert, A.; Miranda, N.; Geudtner, D.; Small, D. Sentinel-1A/B Combined Product Geolocation Accuracy. Remote Sens. 2017, 9, 607. [Google Scholar] [CrossRef]
- Deng, M.; Zhang, G.; Zhao, R.; Li, S.; Li, J. Improvement of Gaofen-3 Absolute Positioning Accuracy Based on Cross-Calibration. Sensors 2017, 17, 2903. [Google Scholar] [CrossRef]
- Wang, W.; Liu, J.; Qiu, X. Decimeter-Level Geolocation Accuracy Updated by a Parametric Tropospheric Model with GF-3. Sensors 2018, 18, 2197. [Google Scholar] [CrossRef]
- Deng, M.; Zhang, G.; Cai, C.; Xu, K.; Zhao, R.; Guo, F.; Suo, J. Improvement and Assessment of the Absolute Positioning Accuracy of Chinese High-Resolution SAR Satellites. Remote Sens. 2019, 11, 1465. [Google Scholar] [CrossRef]
- Deng, M.; Zhang, G.; Zhao, R.; Zhang, Q.; Li, D.; Li, J. Assessment of the geolocation accuracy of YG-13A high-resolution SAR data. Remote Sens. Lett. 2018, 9, 101–110. [Google Scholar] [CrossRef]
- Zhang, G.; Zhao, R.; Li, S.; Deng, M.; Guo, F.; Xu, K.; Wang, T.; Jia, P.; Hao, X. Stability Analysis of Geometric Positioning Accuracy of YG-13 Satellite. IEEE Trans. Geosci. Remote Sens. 2022, 60, 1–12. [Google Scholar] [CrossRef]
- Yunjun, Z.; Fattahi, H.; Pi, X.; Rosen, P.; Simons, M.; Agram, P.; Aoki, Y. Range Geolocation Accuracy of C-/L-Band SAR and its Implications for Operational Stack Coregistration. IEEE Trans. Geosci. Remote Sens. 2022, 60, 1–19. [Google Scholar] [CrossRef]
- Schubert, A.; Small, D.; Jehle, M.; Meier, E. COSMO-skymed, TerraSAR-X, and RADARSAT-2 geolocation accuracy after compensation for earth-system effects. In Proceedings of the 2012 IEEE International Geoscience and Remote Sensing Symposium, IEEE, Munich, Germany, 22–27 July 2012; pp. 3301–3304. [Google Scholar] [CrossRef]
- Nitti, D.O.; Nutricato, R.; Lorusso, R.; Lombardi, N.; Bovenga, F.; Bruno, M.F.; Chiaradia, M.T.; Milillo, G. On the geolocation accuracy of COSMO-SkyMed products. In Proceedings of the SAR Image Analysis, Modeling, and Techniques XV, SPIE, Toulouse, France, 21–24 September 2015; Volume 9642, pp. 69–80. [Google Scholar] [CrossRef]
- Dowman, I.; Tao, V. An update on the use of rational functions for photogrammetric restitution. ISPRS Highlights 2002, 7, 22–29. [Google Scholar]
- Zhang, G.; Zhu, X. A study of the RPC model of TerraSAR-X and COSMO-SkyMed SAR imagery. Int. Arch. Photogramm. Remote Sens. Spat. Inf. Sci. 2008, 37, 321–324. [Google Scholar]
- Zhang, G.; Fei, W.; Li, Z.; Zhu, X.; Li, D. Evaluation of the RPC Model for Spaceborne SAR Imagery. Photogramm. Eng. Remote Sens. 2010, 76, 727–733. [Google Scholar] [CrossRef]
- He, X.; Wei, X.; Zhang, L.; Balz, T.; Liao, M. RPC modeling for spaceborne SAR and its aplication in radargrammetry. In Proceedings of the 2010 IEEE International Geoscience and Remote Sensing Symposium, Honolulu, HI, USA, 25–30 July 2010; pp. 3600–3603. [Google Scholar] [CrossRef]
- Zhang, L.; Balz, T.; Liao, M. Satellite SAR geocoding with refined RPC model. ISPRS J. Photogramm. Remote Sens. 2012, 69, 37–49. [Google Scholar] [CrossRef]
- Jiang, W.; Yu, A.; Dong, Z.; Wang, Q. Comparison and Analysis of Geometric Correction Models of Spaceborne SAR. Sensors 2016, 16, 973. [Google Scholar] [CrossRef]
- Bagheri, H.; Schmitt, M.; d’Angelo, P.; Zhu, X.X. A framework for SAR-optical stereogrammetry over urban areas. ISPRS J. Photogramm. Remote Sens. 2018, 146, 389–408. [Google Scholar] [CrossRef]
- Akiki, R.; Marí, R.; De Franchis, C.; Morel, J.-M.; Facciolo, G. Robust rational polynomial camera modelling for SAR and pushbroom imaging. In Proceedings of the 2021 IEEE International Geoscience and Remote Sensing Symposium IGARSS, Brussels, Belgium, 11–16 July 2021; pp. 7908–7911. [Google Scholar] [CrossRef]
- Zhang, G.; Li, Z.; Pan, H.; Qiang, Q.; Zhai, L. Orientation of Spaceborne SAR Stereo Pairs Employing the RPC Adjustment Model. IEEE Trans. Geosci. Remote Sens. 2011, 49, 2782–2792. [Google Scholar] [CrossRef]
- Curlander, J.C.; McDonough, R.N. Synthetic Aperture Radar; Wiley: Hoboken, NJ, USA, 1991; Volume 11. [Google Scholar]
- Zhao, R.; Zhang, G.; Deng, M.; Yang, F.; Chen, Z.; Zheng, Y. Multimode Hybrid Geometric Calibration of Spaceborne SAR Considering Atmospheric Propagation Delay. Remote Sens. 2017, 9, 464. [Google Scholar] [CrossRef]
- Hanssen, R.F. Radar Interferometry Data Interpretation and Error Analysis. In Radar Interferometry Data Interpretation and Error Analysis; Springer Science & Business: Berlin, Germany, 2001. [Google Scholar]
- Raggam, H.; Perko, R.; Gutjahr, K.; Kiefl, N. Accuracy Assessment of 3D Point Retrieval from TerraSAR-X Data Sets. In Proceedings of the European Conference on Synthetic Aperture Radar, Eurogress Aachen, Germany, 7–10 June 2010. [Google Scholar]
- Zhao, R.; Jiang, Y.; Zhang, G.; Deng, M.; Yang, F. Geometric Accuracy Evaluation of YG-18 Satellite Imagery Based on RFM. Photogramm. Rec. 2017, 32, 33–47. [Google Scholar] [CrossRef]
- Franceschetti, G.; Migliaccio, M.; Riccio, D. The SAR simulation: An overview. In Proceedings of the International Geoscience & Remote Sensing Symposium, Firenze, Italy, 10–14 July 1995. [Google Scholar]
- Guindon, B.; Adair, M. Analytic Formulation of Spaceborne SAR Image Geocoding and “Value-Added” Product Generation Procedures using Digital Elevation Data. Can. J. Remote Sens. 1992, 18, 2–12. [Google Scholar] [CrossRef]
- Kimura, H.; Kodaira, N. Simulation and comparison of JERS-1 and ERS-1 SAR images. In Proceedings of the International Geoscience & Remote Sensing Symposium, Tokyo, Japan, 18–21 August 1993. [Google Scholar]
- Gelautz, M.; Frick, H.; Raggam, J.; Burgstaller, J.; Leberl, F. SAR image simulation and analysis of alpine terrain. ISPRS J. Photogramm. Remote Sens. 1998, 53, 17–38. [Google Scholar] [CrossRef]
- Zhang, G.; Qiang, Q.; Luo, Y.; Zhu, Y.; Gu, H.; Zhu, X. Application of RPC Model in Orthorectification of Spaceborne SAR Imagery: Application of RPC model in orthorecitification of spaceborne SAR imagery. Photogramm. Rec. 2012, 27, 94–110. [Google Scholar] [CrossRef]
- Wang, H.; Cheng, Q.; Wang, T.; Zhang, G.; Wang, Y.; Li, X.; Jiang, B. Layover Compensation Method for Regional Spaceborne SAR Imagery Without GCPs. IEEE Trans. Geosci. Remote Sens. 2021, 59, 8367–8381. [Google Scholar] [CrossRef]
- Zheng, X.; Huang, Q.; Wang, J.; Wang, T.; Zhang, G. Geometric Accuracy Evaluation of High-Resolution Satellite Images Based on Xianning Test Field. Sensors 2018, 18, 2121. [Google Scholar] [CrossRef]
- Bruyninx, C. The EUREF Permanent Network: A multi-disciplinary network serving surveyors as well as scientists. GeoInformatics 2004, 7, 32–35. [Google Scholar]
- Woolson, R.F. Wilcoxon Signed-rank Test. Wiley Encycl. Clin. Trials 2007, 1–3. [Google Scholar]
- Eineder, M.; Schattler, B.; Breit, H.; Fritz, T.; Roth, A. TerraSAR-X SAR products and processing algorithms. In Proceedings of the 2005 IEEE International Geoscience and Remote Sensing Symposium, 2005, IGARSS ’05, Seoul, Republic of Korea, 25–29 July 2005; Volume 7, pp. 4870–4873. [Google Scholar] [CrossRef]
- Small, D.; Zuberbühler, L.; Schubert, A.; Meier, E. Terrain-flattened gamma nought Radarsat-2 backscatter. Can. J. Remote Sens. 2011, 37, 493–499. [Google Scholar] [CrossRef]

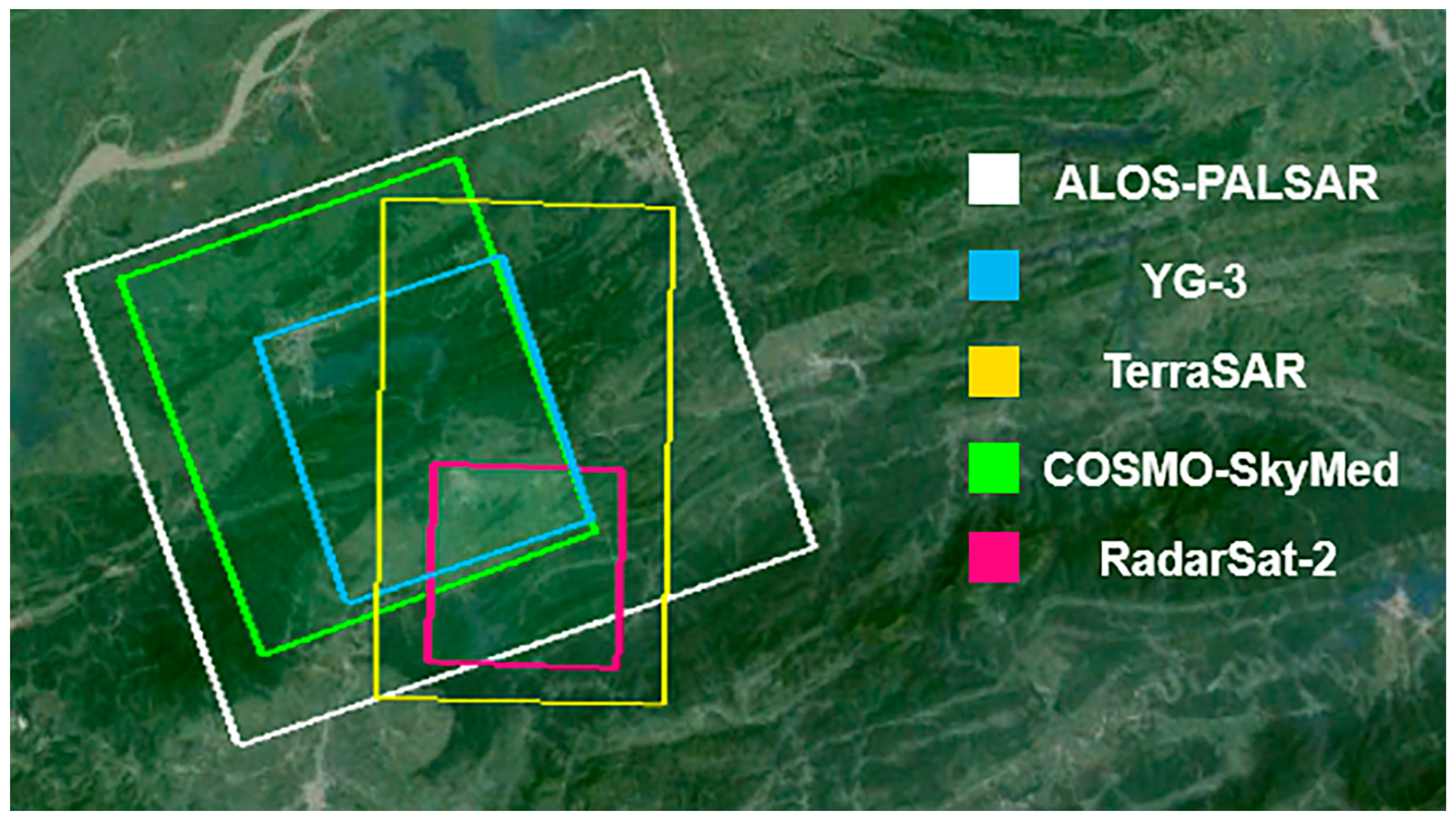

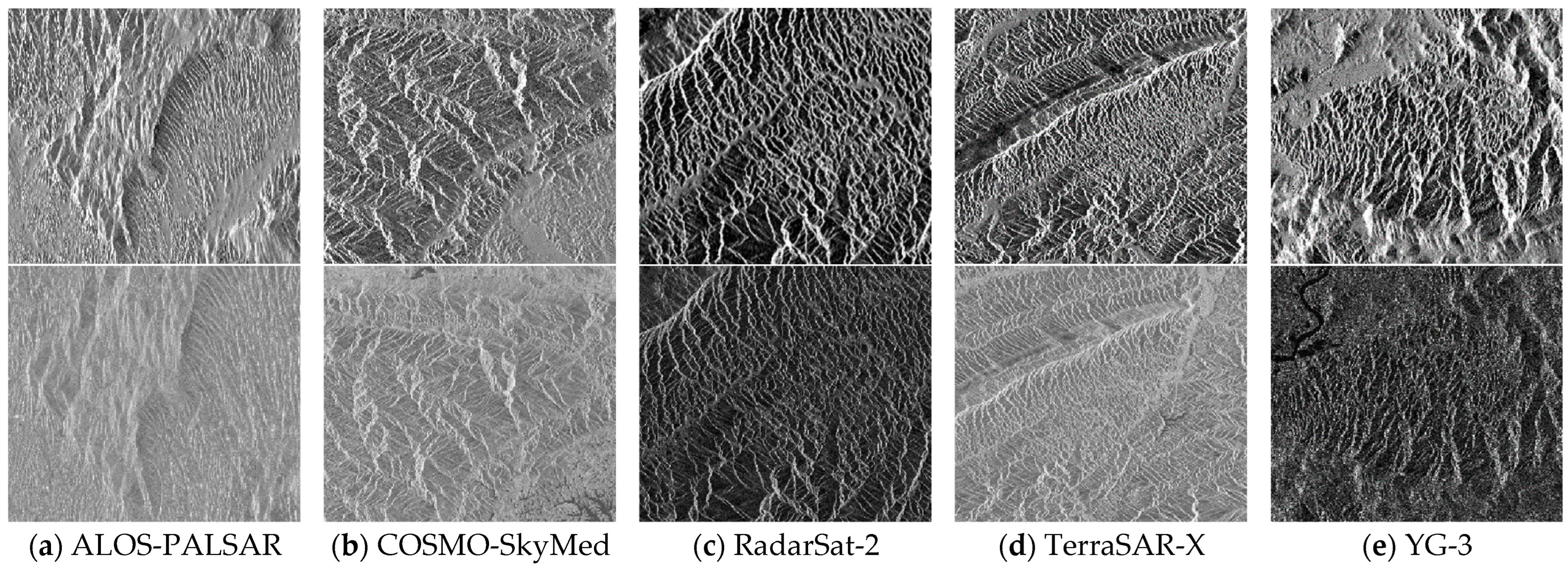

| Satellite | ALOS | COSMO-SkyMed | RadarSat-2 | TerraSAR-X | YG-3 |
|---|---|---|---|---|---|
| Country | Japan | Italy | Canada | Germany | China |
| Orbit height | 692 km | 620 km | 800 km | 515 km | 600 km |
| Imaging time | 22 December 2008 | 22 July 2012 | 18 March 2013 | 24 July 2012 | 27 April 2012 |
| Range spacing | 5.0 m | 3.0 m | 1.6 m | 1.2 m | 3.0 m |
| Azimuth spacing | 3.0 m | 2.5 m | 2.8 m | 3.3 m | 3.0 m |
| Width of image | 50 km × 70 km | 40 km × 40 km | 20 km × 20 km | 30 km × 50 km | 30 km × 30 km |
| Incident angle | 34° | 32° | 31° | 30° | 30° |
| Orbit direction and Look | Descending, right | Descending, right | Descending, right | Descending, right | Descending, right |
| Imaging mode | Fine Resolution | Himage | UltraFine | StripMap | Strip |
| Data level | Level 1.1 | SCS | SLC | SSC | L1B |
| Polarization modes | HH | HH | HH | HH | HH |
| Image size | 9344 × 18,432 | 19,604 × 22,334 | 8794 × 10,861 | 18,700 × 27,694 | 13,469 × 16,426 |
| Satellite | Mean Ionospheric Slant Delay (m) | Mean Tropospheric Slant Delay (m) | Slant Range RMSE of CPs after Compensation (Pixels) |
|---|---|---|---|
| ALOS-PALSAR | 1.7854 | 3.2194 | 1.923 |
| YG-3 | 0.0569 | 2.6988 | 29.765 |
| COSMO-SkyMed | 0.0558 | 2.7848 | 2.540 |
| TerraSAR-X | 0.0369 | 2.6785 | 4.323 |
| RadarSat-2 | 0.1256 | 2.7071 | 2.665 |
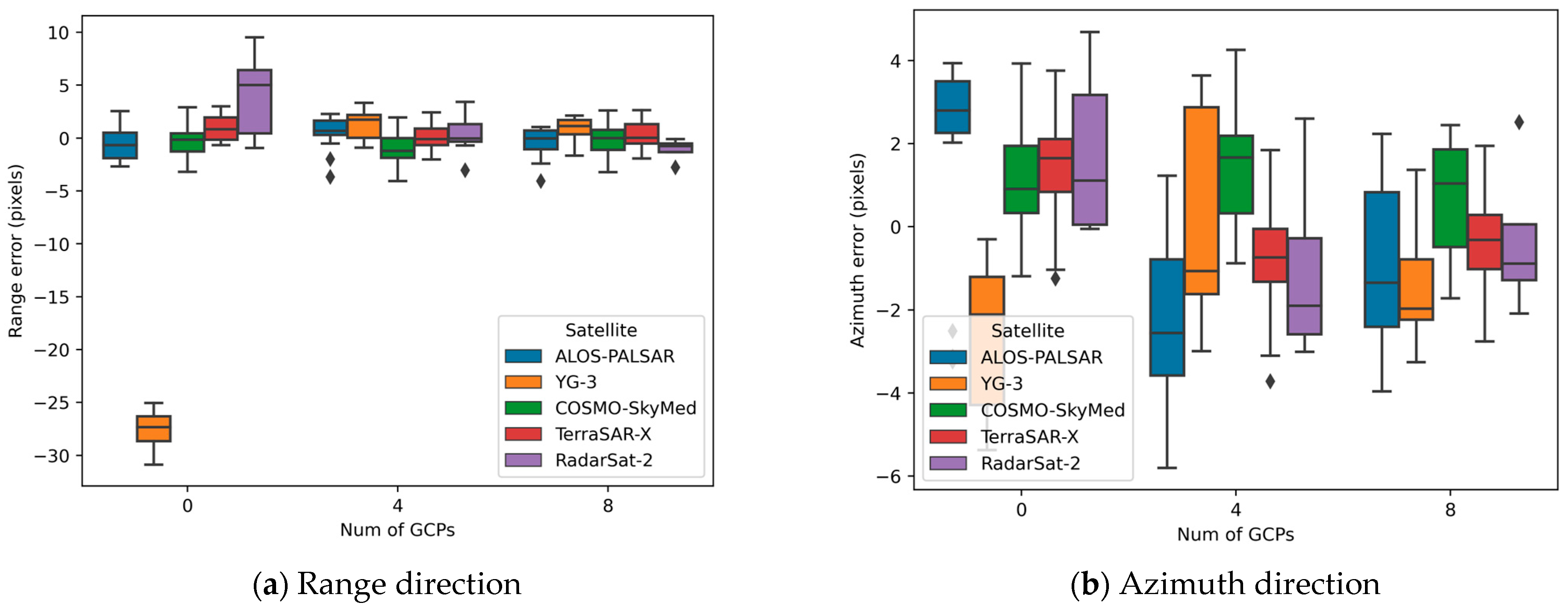
| Satellite | Number of GCPs | Number of CPs | RMSE of GCPs (Pixels) | RMSE of CPs (Pixels) | ||||
|---|---|---|---|---|---|---|---|---|
| Range | Azimuth | Plane | Range | Azimuth | Plane | |||
| ALOS-PALSAR | 0 | 17 | —— | —— | —— | 1.870 | 3.278 | 3.773 |
| 4 | 13 | 0.211 | 0.858 | 0.884 | 1.693 | 2.916 | 3.372 | |
| 8 | 9 | 0.846 | 1.506 | 1.728 | 1.702 | 2.219 | 2.796 | |
| YG-3 | 0 | 13 | —— | —— | —— | 27.645 | 3.129 | 27.822 |
| 4 | 9 | 0.411 | 0.486 | 0.637 | 1.905 | 2.425 | 3.084 | |
| 8 | 5 | 1.301 | 1.627 | 2.083 | 1.524 | 2.098 | 2.593 | |
| COSMO-SkyMed | 0 | 24 | —— | —— | —— | 1.448 | 1.708 | 2.240 |
| 4 | 20 | 0.699 | 0.004 | 0.699 | 1.728 | 1.942 | 2.599 | |
| 8 | 12 | 0.977 | 1.257 | 1.592 | 1.467 | 1.442 | 2.057 | |
| TerraSAR-X | 0 | 30 | —— | —— | —— | 1.506 | 1.826 | 2.367 |
| 4 | 26 | 0.327 | 0.056 | 0.332 | 1.324 | 1.477 | 1.984 | |
| 8 | 22 | 0.548 | 0.805 | 0.974 | 1.371 | 1.263 | 1.864 | |
| RadarSat-2 | 0 | 12 | —— | —— | —— | 1.493 | 1.936 | 2.445 |
| 4 | 8 | 0.601 | 0.460 | 0.757 | 1.801 | 2.176 | 2.824 | |
| 8 | 4 | 1.189 | 1.082 | 1.489 | 1.489 | 1.752 | 2.300 | |
| Satellite | Number of GCPs | Number of CPs | RMSE of GCP (Pixels) | RMSE of CP (Pixels) | ||||
|---|---|---|---|---|---|---|---|---|
| Range | Azimuth | Plane | Range | Azimuth | Plane | |||
| ALOS-PALSAR | 0 | 238 | —— | —— | —— | 1.104 | 8.065 | 8.140 |
| 4 | 234 | 0.080 | 0.178 | 0.196 | 0.341 | 1.038 | 1.092 | |
| 8 | 230 | 0.180 | 0.469 | 0.502 | 0.339 | 1.093 | 1.197 | |
| YG-3 | 0 | 45 | —— | —— | —— | 28.885 | 1.253 | 28.912 |
| 4 | 41 | 0.427 | 0.345 | 0.549 | 0.741 | 0.849 | 1.127 | |
| 8 | 37 | 0.452 | 0.554 | 0.715 | 0.701 | 0.909 | 1.148 | |
| COSMO-SkyMed | 0 | 29 | —— | —— | —— | 2.644 | 1.120 | 2.872 |
| 4 | 25 | 0.221 | 0.249 | 0.333 | 1.091 | 0.791 | 1.348 | |
| 8 | 21 | 0.483 | 0.455 | 0.664 | 0.932 | 0.819 | 1.240 | |
| TerraSAR-X | 0 | 84 | —— | —— | —— | 5.630 | 1.635 | 5.863 |
| 4 | 80 | 0.325 | 0.430 | 0.538 | 0.836 | 0.937 | 1.255 | |
| 8 | 76 | 0.420 | 0.511 | 0.661 | 0.783 | 0.876 | 1.175 | |
| RadarSat-2 | 0 | 183 | —— | —— | —— | 5.648 | 3.317 | 6.550 |
| 4 | 179 | 0.175 | 0.409 | 0.445 | 0.778 | 0.867 | 1.165 | |
| 8 | 175 | 0.445 | 0.598 | 0.745 | 0.704 | 0.812 | 1.075 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jiang, B.; Dong, X.; Deng, M.; Wan, F.; Wang, T.; Li, X.; Zhang, G.; Cheng, Q.; Lv, S. Geolocation Accuracy Validation of High-Resolution SAR Satellite Images Based on the Xianning Validation Field. Remote Sens. 2023, 15, 1794. https://doi.org/10.3390/rs15071794
Jiang B, Dong X, Deng M, Wan F, Wang T, Li X, Zhang G, Cheng Q, Lv S. Geolocation Accuracy Validation of High-Resolution SAR Satellite Images Based on the Xianning Validation Field. Remote Sensing. 2023; 15(7):1794. https://doi.org/10.3390/rs15071794
Chicago/Turabian StyleJiang, Boyang, Xiaohuan Dong, Mingjun Deng, Fangqi Wan, Taoyang Wang, Xin Li, Guo Zhang, Qian Cheng, and Shuying Lv. 2023. "Geolocation Accuracy Validation of High-Resolution SAR Satellite Images Based on the Xianning Validation Field" Remote Sensing 15, no. 7: 1794. https://doi.org/10.3390/rs15071794
APA StyleJiang, B., Dong, X., Deng, M., Wan, F., Wang, T., Li, X., Zhang, G., Cheng, Q., & Lv, S. (2023). Geolocation Accuracy Validation of High-Resolution SAR Satellite Images Based on the Xianning Validation Field. Remote Sensing, 15(7), 1794. https://doi.org/10.3390/rs15071794






