Cascaded-Filter-Based Reverberation Suppression Method of Short-Pulse Continuous Wave for Active Sonar
Abstract
1. Introduction
- The formation mechanism of high-frequency-oscillating decay characteristics of the reverberation envelope of the short-pulse-width CW signals is modeled and analyzed based on the cell scattering model. The oscillation frequency is theoretically deduced;
- Based on the experimental data from the lake trial, the underlying causes of the high-frequency oscillation of the reverberation envelope of short-pulse-width CW signals are analyzed;
- A cascaded filter is designed to improve the target detection capability under the non-steadily-decay reverberation background while simultaneously reducing the false alarm rate. Specifically, before applying CA-CFAR to filter out low-frequency oscillations, an α filter is designed based on the high-frequency oscillation components of the reverberation envelope and the pulse width of the transmitted signal, with the purpose of suppressing high-frequency oscillations.
2. Problem Statement
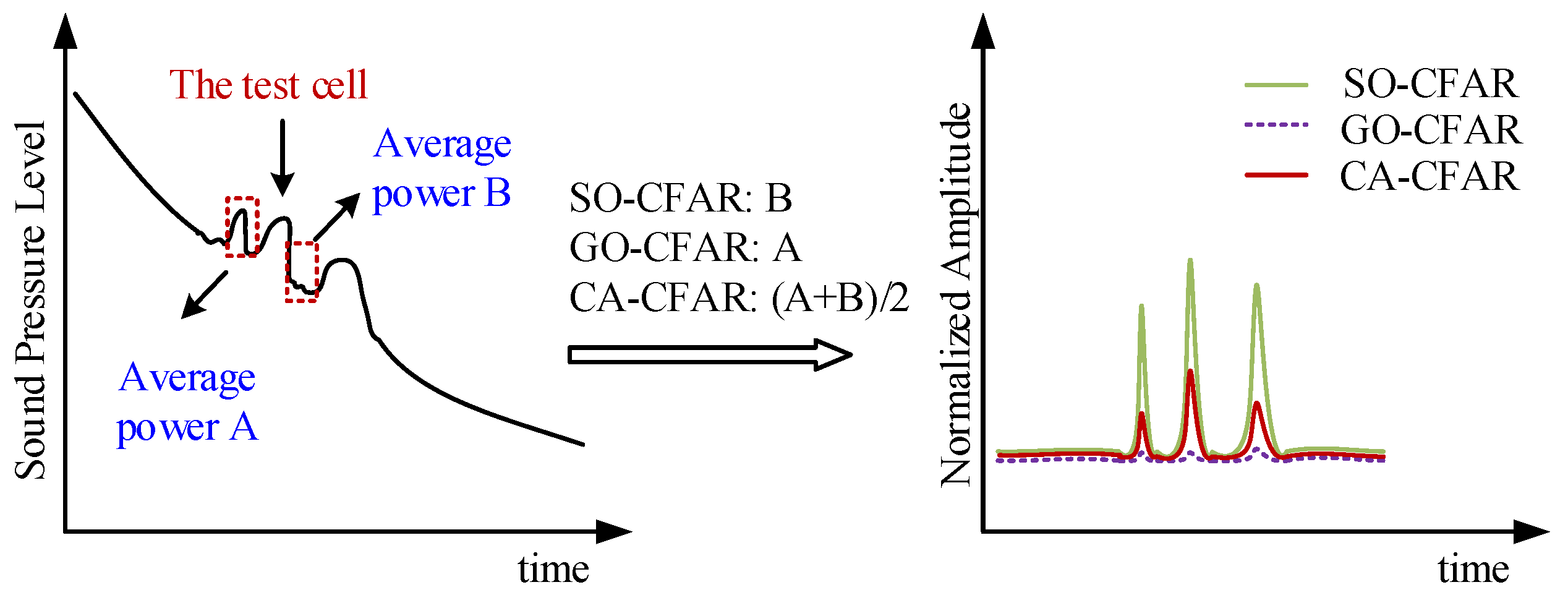
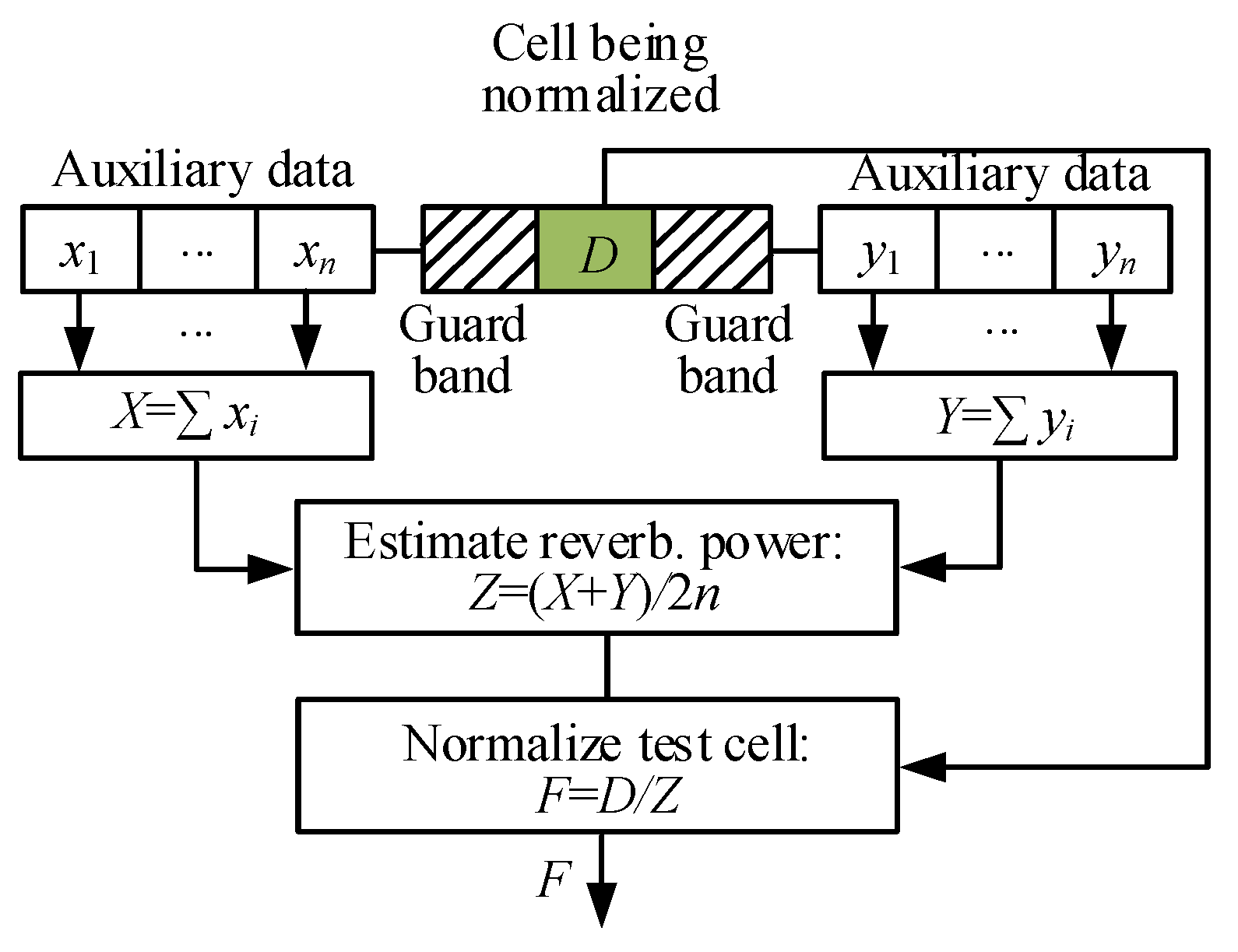
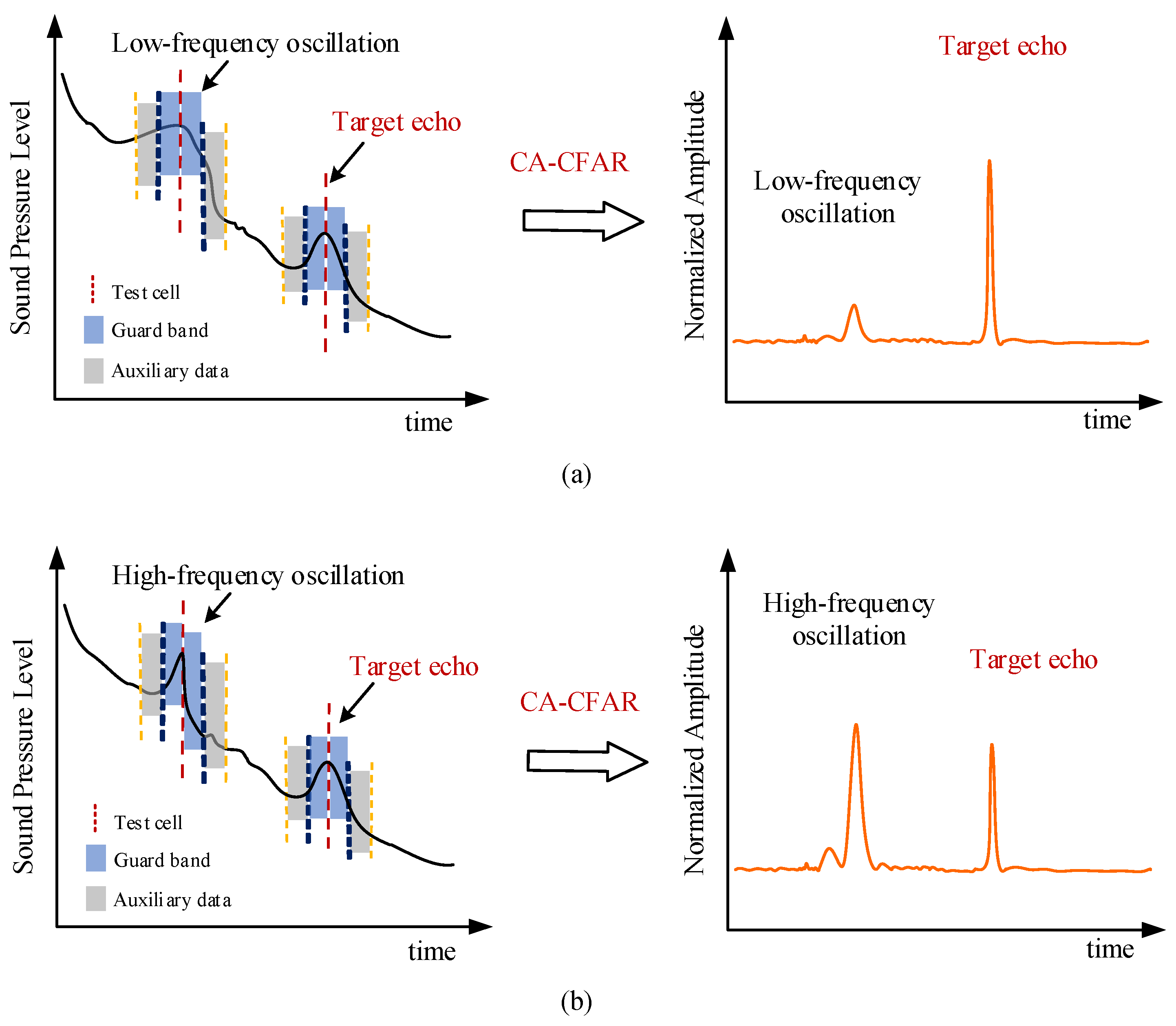
2.1. Traditional Reverberation Background Target Detection Method Using CA-CFAR
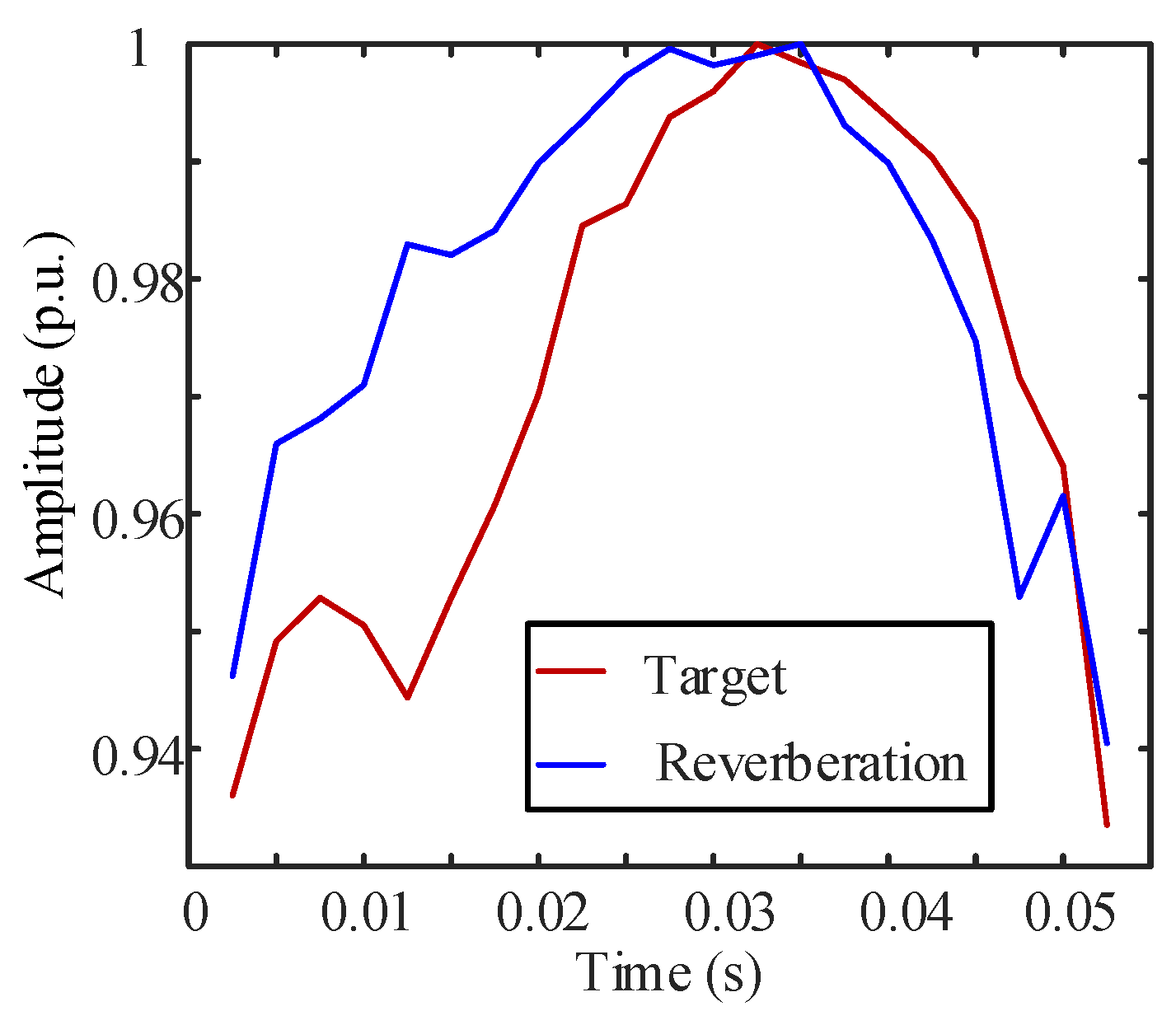
2.2. High-Frequency-Oscillating Phenomenon of Reverberation Envelope of Short-Pulse-Width CW Signals
2.2.1. High-Frequency-Oscillating Phenomenon
2.2.2. Adequacy Analysis of Traditional CA-CFAR
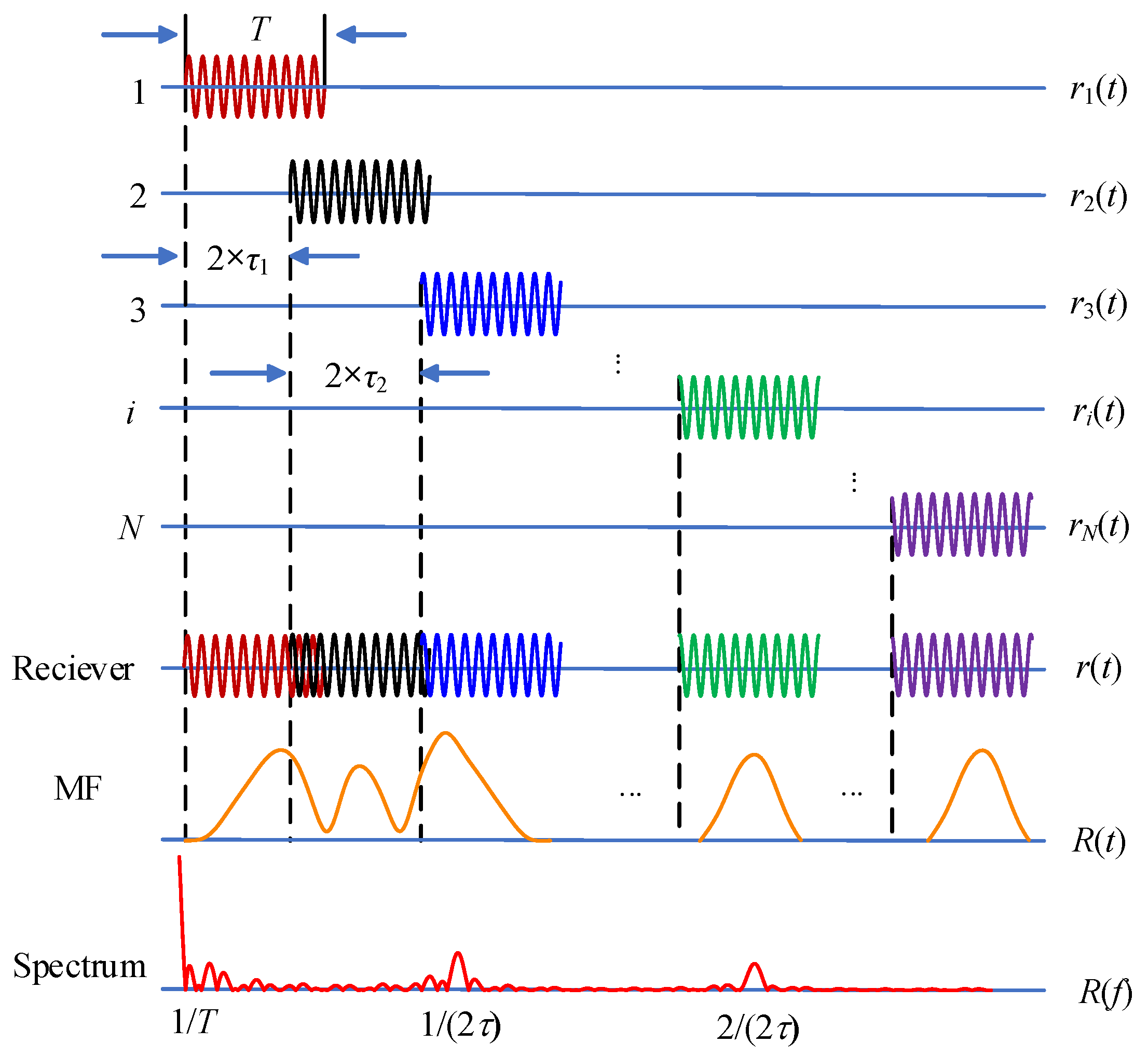
3. Modeling of Reverberation Oscillation Characteristics
- (1)
- The scattering cells are uniformly distributed within the directional angle range of the receiving array;
- (2)
- The scattering bodies are distributed on the seabed according to the Gaussian distribution, so the time delay of the scatter echo signals follows a Gaussian distribution;
- (3)
- The time delay difference of different scattered echo signals is equal, i.e., τ1 = τ2 = … = τN = τ;
- (4)
- The volume of the scatterer bodies is assumed to be small and the pulse broadening phenomenon during scattering is neglected.
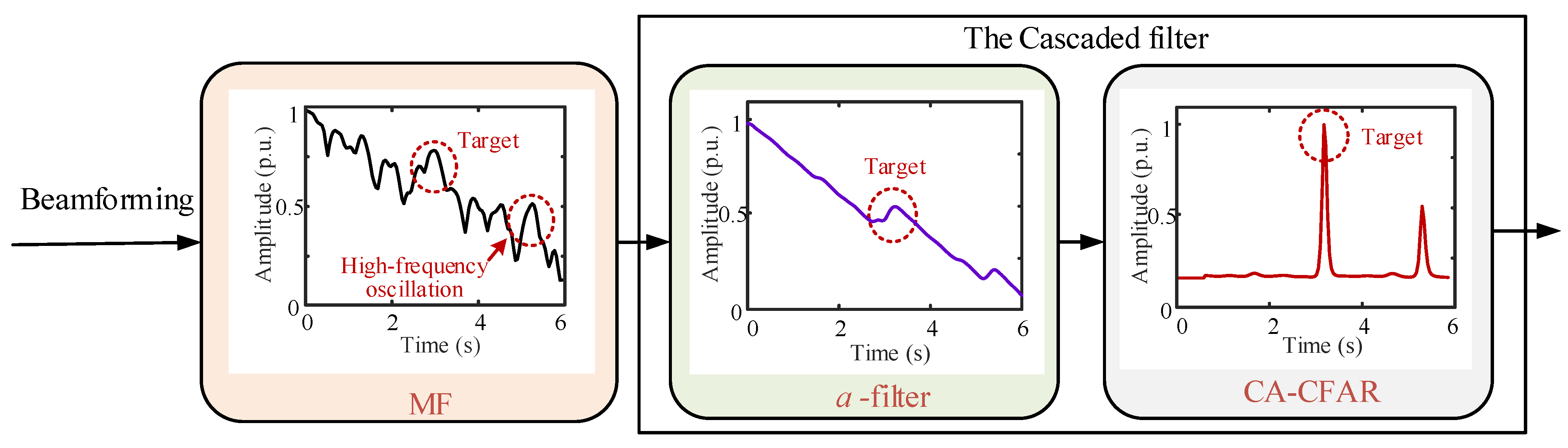
4. Operation Principles of Cascaded Reverberation Suppression Algorithm
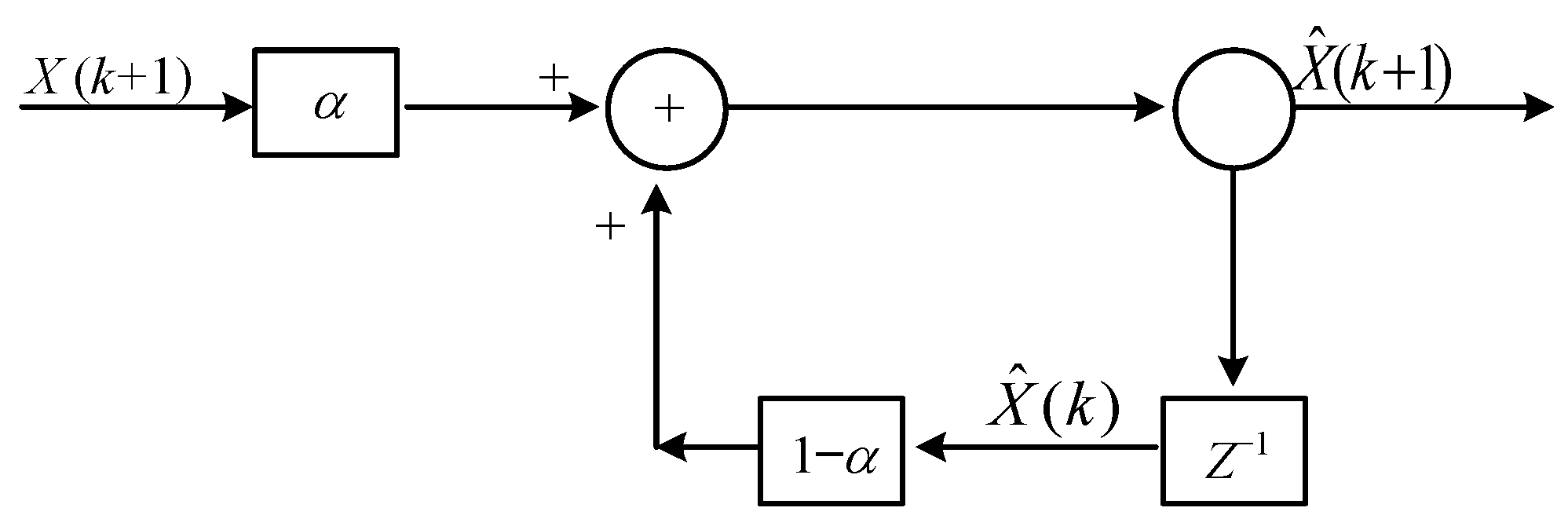
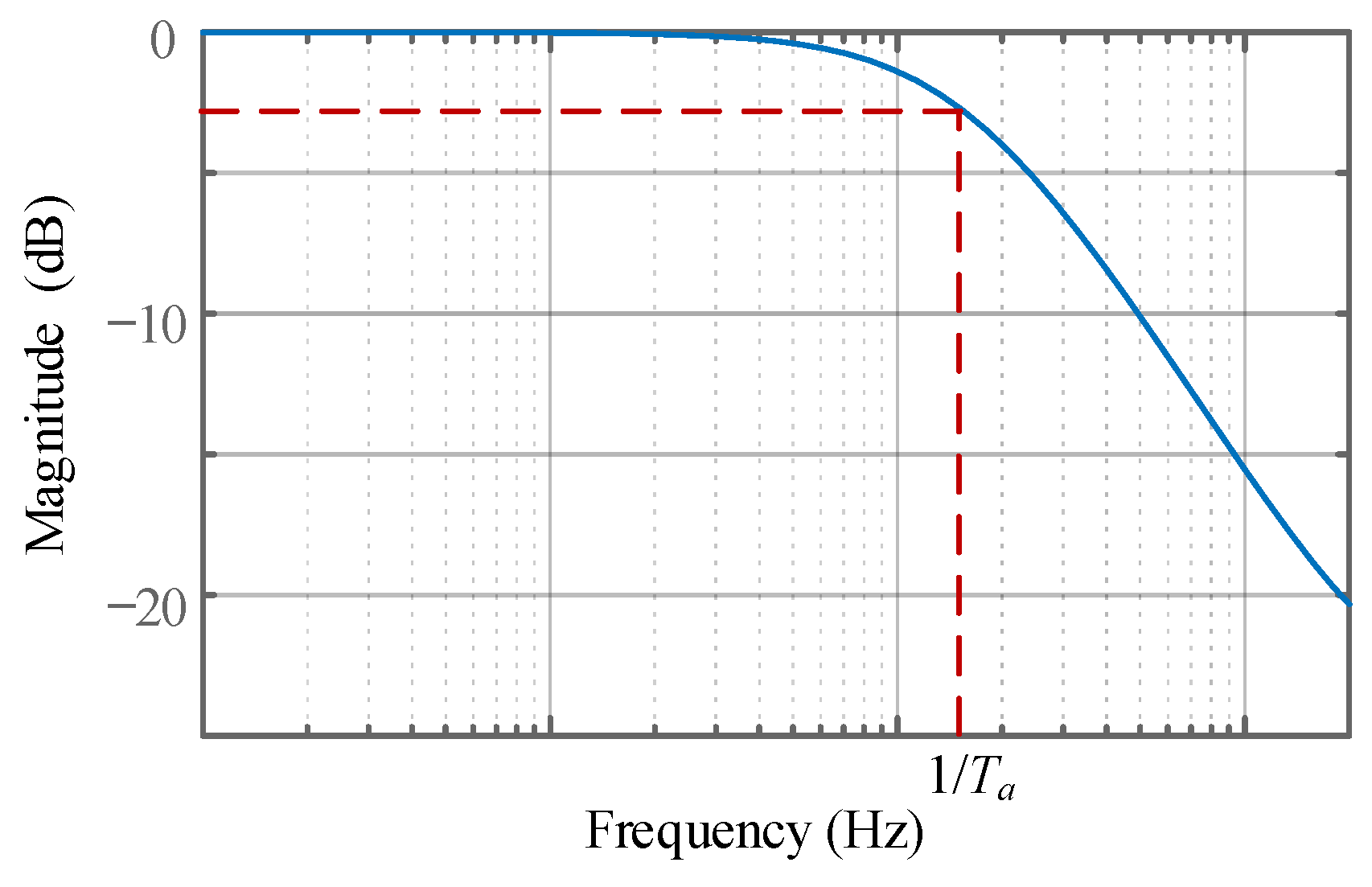
4.1. The Design of α Filter
4.2. The Data Processing Logic Based on the Cascaded Filter
- The data received from the active sonar are processed through beamforming and the MF;
- The α filter is designed based on the pulse width of the transmitting CW signal, and the result of the MF is passed through the α filter;
- The auxiliary data length and guard band length of CA-CFAR are designed based on the pulse width of the transmitting CW signal, and the output of the α filter is used as the input of CA-CFAR;
- The bearing-time record is displayed.
5. Performance Analysis
5.1. Simulation Experiment Test
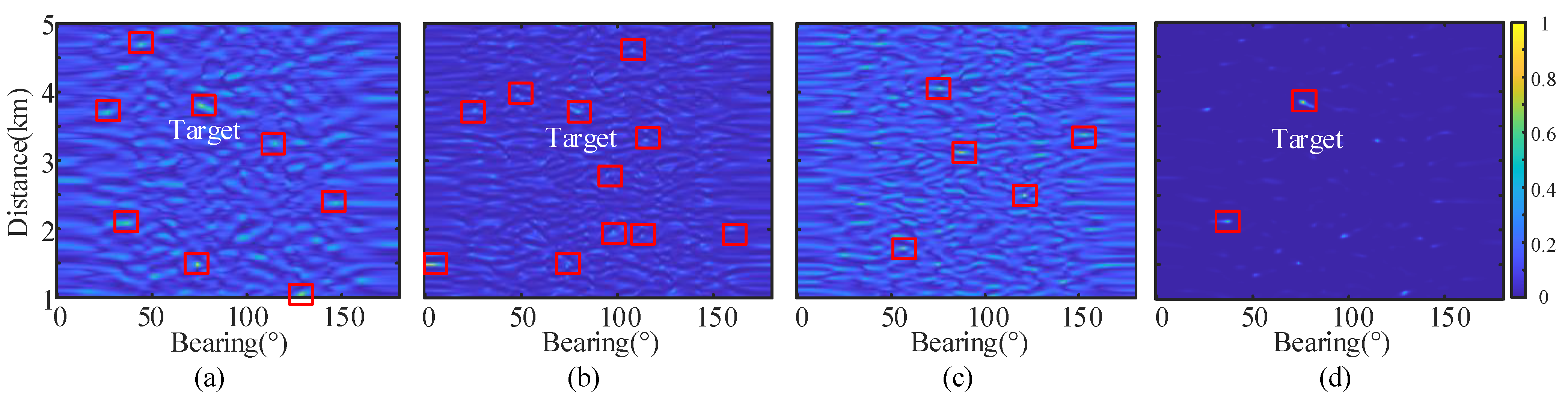
5.1.1. Simulation Comparison
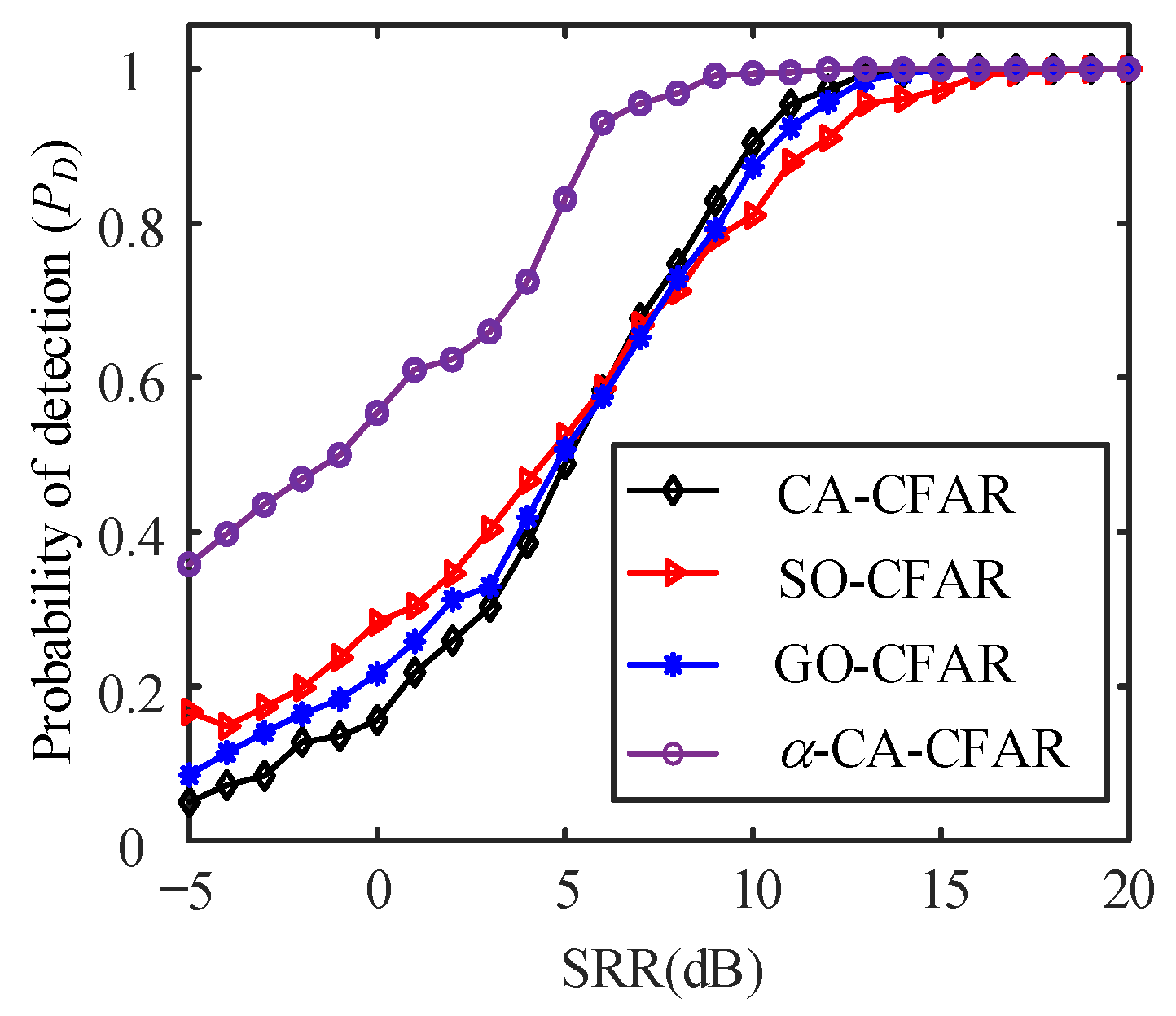
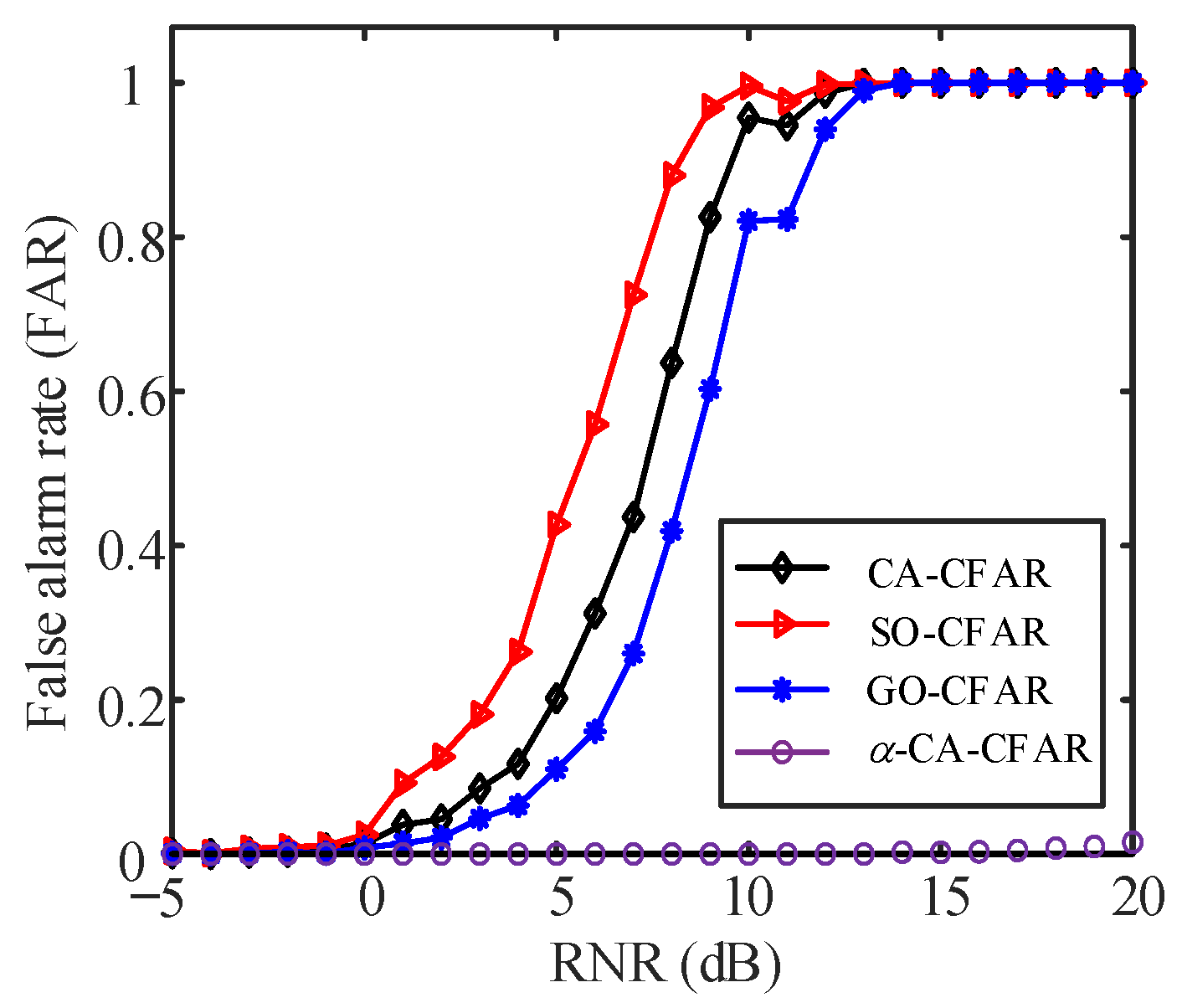
5.1.2. Monte-Carlo Simulations
Target Detection Performance under Strong Non-Steadily-Decay Reverberation Background Interference
False Alarm Suppression Performance against High-Frequency Oscillations of the Reverberation Envelope
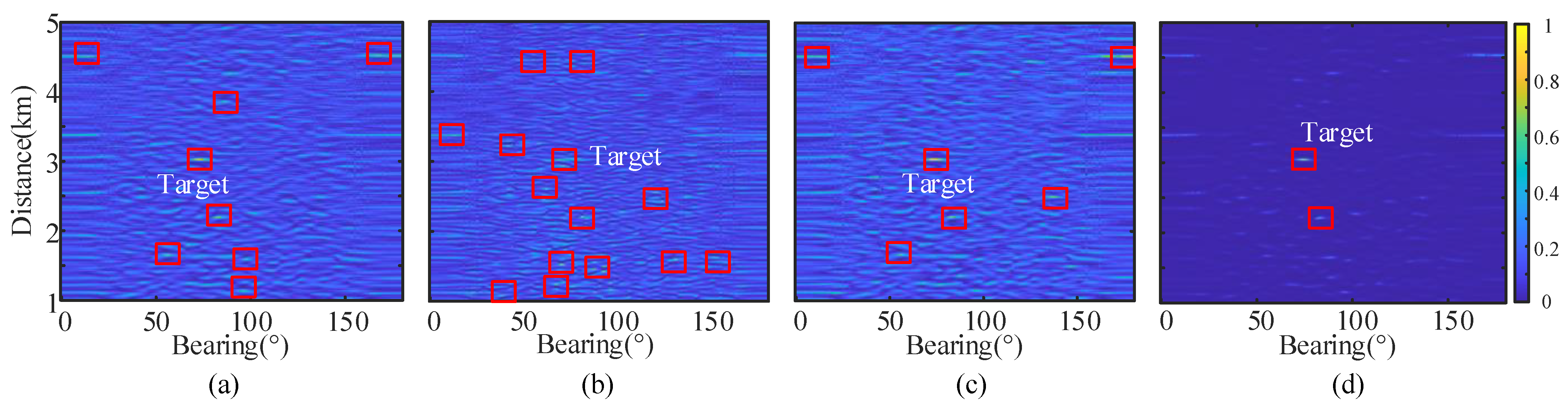
5.2. Actual Lake Trials Data Test
5.2.1. Experimental Conditions
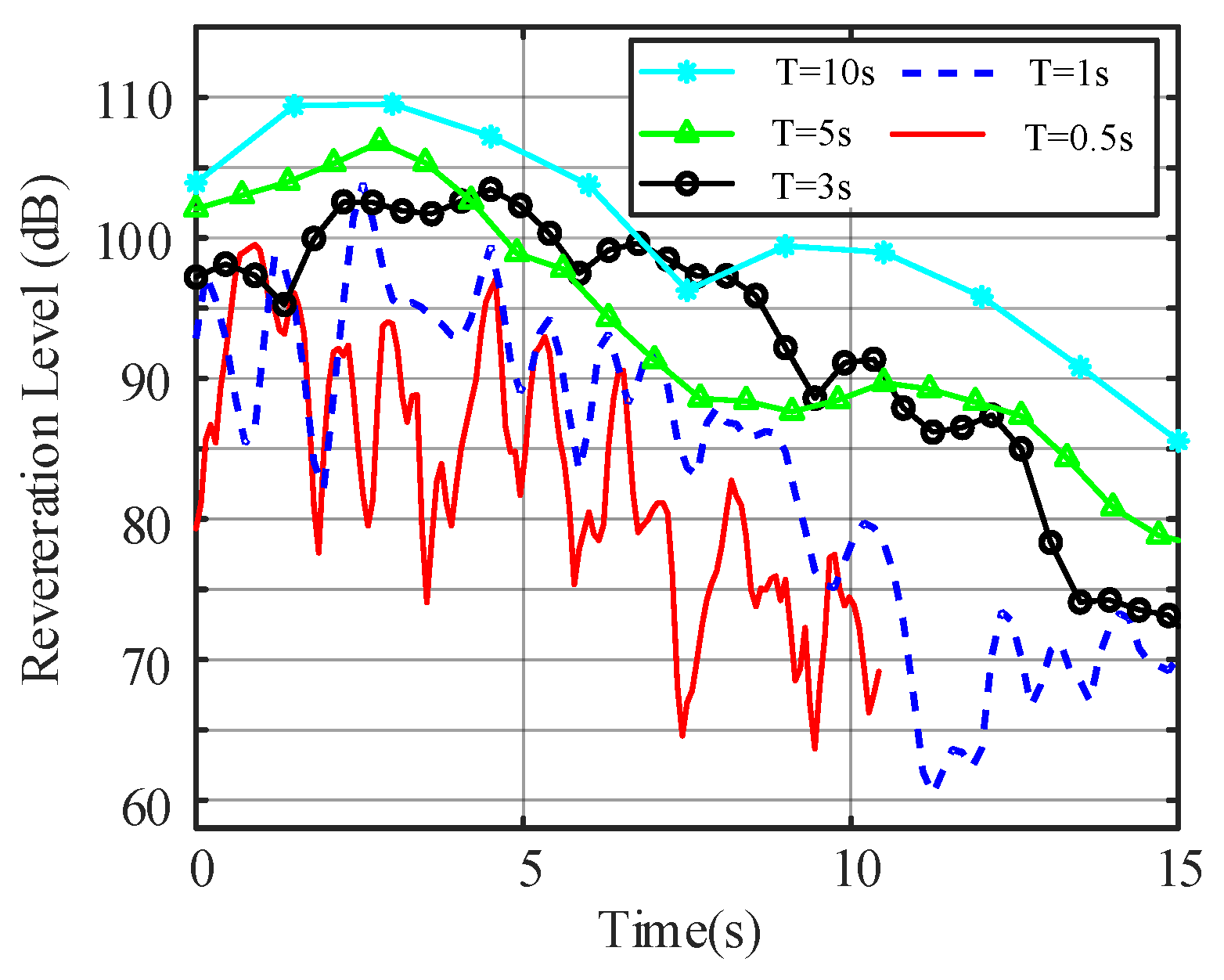
5.2.2. Analysis Results
Trial Results with T = 0.05 s
Trial Results with T = 0.1 s
Trial Results with T = 0.2 s
Trial Results with T = 0.5 s
6. Discussion
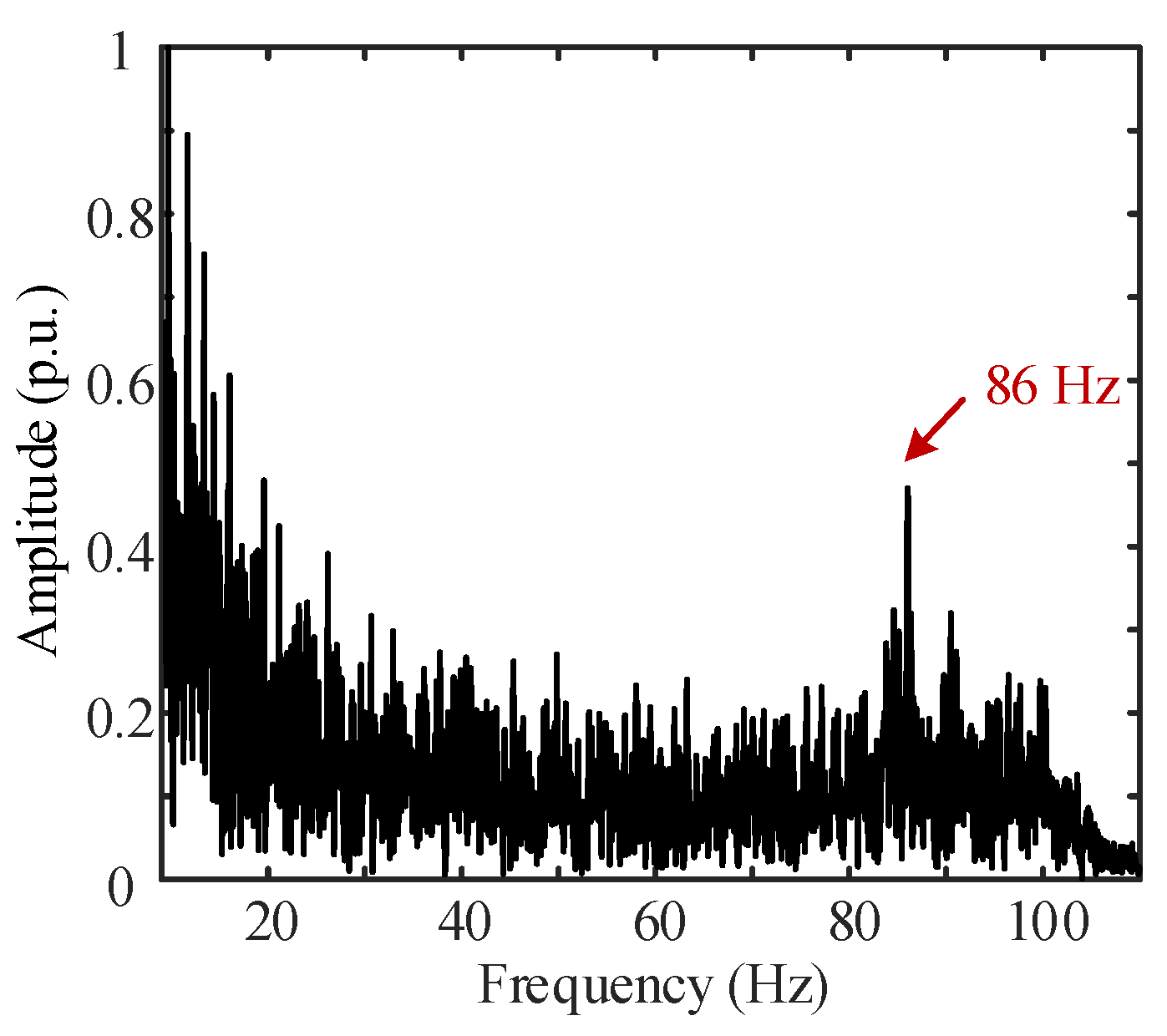
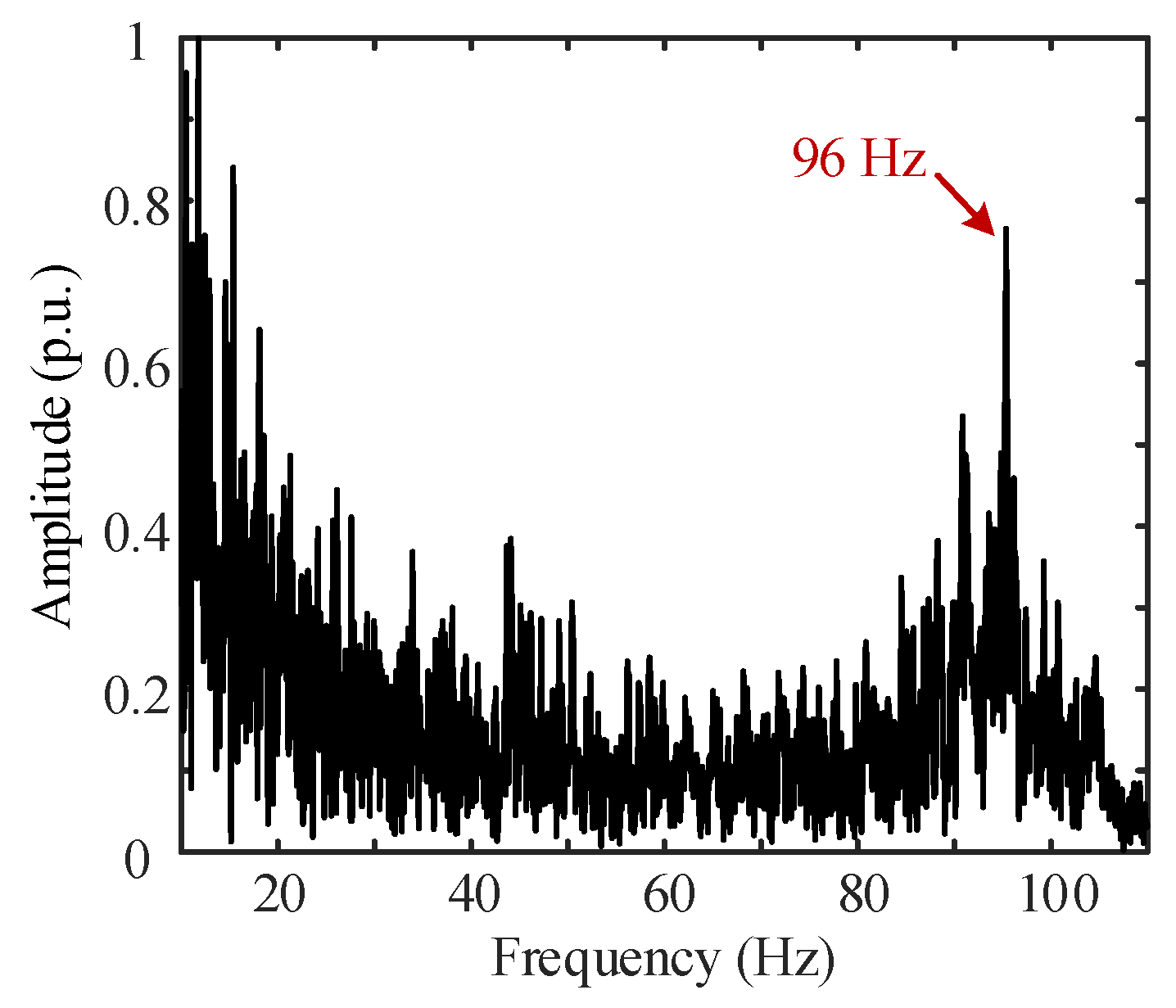
6.1. Analysis of the Causes of High-Frequency Oscillations of the Reverberation Envelope of Short-Pulse-Width CW Signal
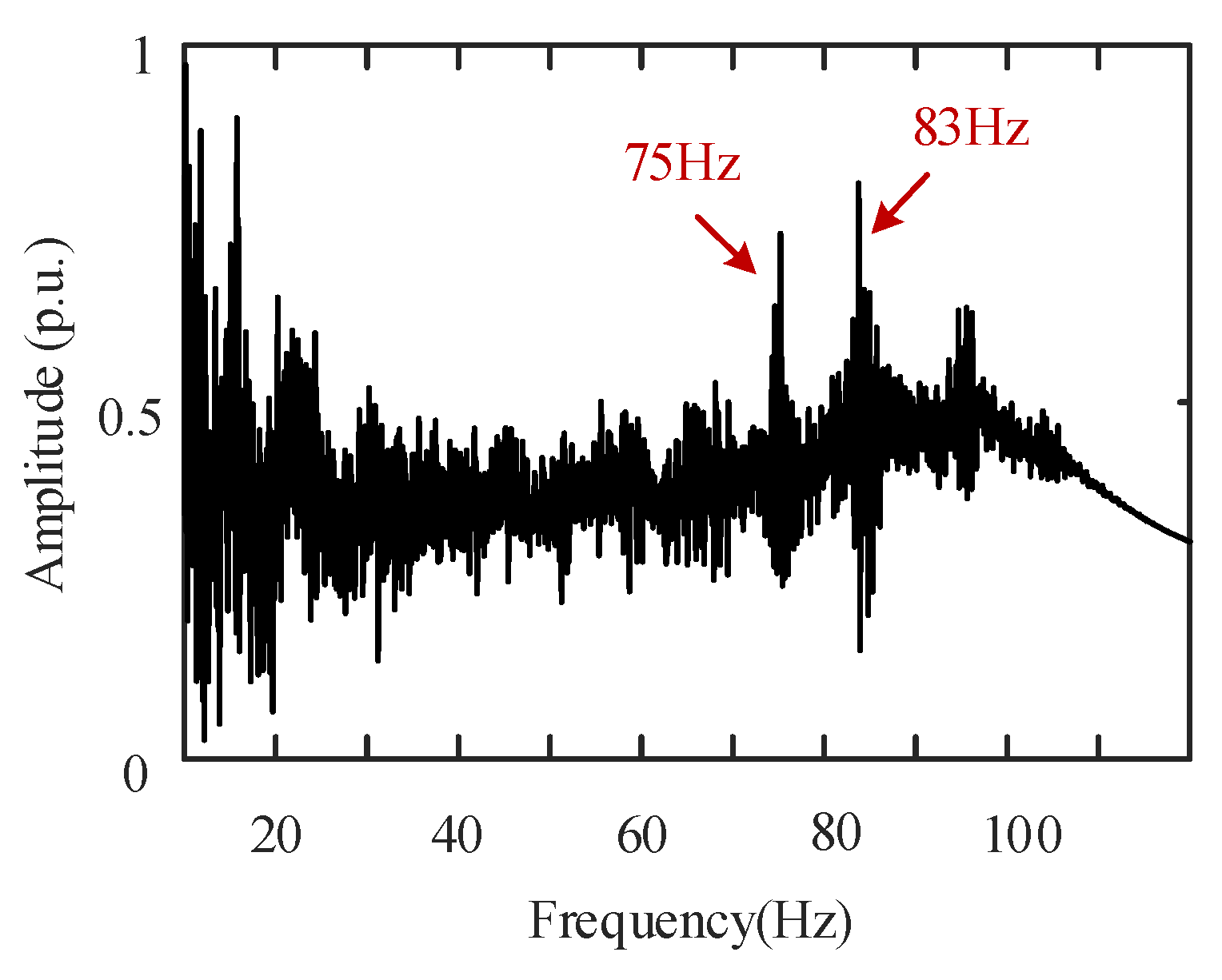
6.1.1. Simulated Target Signal and Its Frequency Spectrum Emitted by the Transponder
6.1.2. The Reverberation Envelope and Its Spectrum of the Long-Pulse-Width CW Signals
6.2. Analysis of Lake Trial Results
7. Conclusions
- (1)
- By modeling the formation mechanism of high-frequency oscillations in the reverberation envelope of a short-pulse CW signal, an analysis reveals that the frequency component of the high-frequency oscillations is m/2τ (m = 1, 2, …). Based on this, an α-filter is designed to suppress high-frequency oscillations in the reverberation envelope with frequencies greater than 1/T. Subsequently, the CA-CFAR is applied to suppress low-frequency oscillations in the reverberation envelope with frequencies less than 1/T;
- (2)
- The underlying factor determining the high-frequency oscillations of the reverberation envelope of short-pulse CW signals is the duration of the transmitted CW. Specifically, the shorter the pulse width, the more severe the oscillation phenomenon becomes;
- (3)
- Simulation and lake trials confirm the effectiveness of the proposed method. Monte Carlo simulation experiments indicate that, when the SRR is less than 15 dB, the average detection probability of the proposed α-CA-CFAR method is 20% higher compared to CA-CFAR, SO-CFAR, and GO-CFAR methods. The lake trial results demonstrate the method’s strong robustness;
- (4)
- Although the proposed method can effectively suppress high-frequency and low-frequency oscillations, it is limited for interference signals with the same time scale as the target echo. To further improve the suppression ability of reverberation interference, considering the scattering characteristics of the target and different interference sources, small changes in the temporal variation features used to identify the target echo, such as the temporal amplitude and frequency variations, will be part of our future investigation.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Jia, H.; Li, X. Underwater reverberation suppression based on non-negative matrix factorization. J. Sound Vib. 2021, 506, 116166. [Google Scholar] [CrossRef]
- Chen, D.; Tao, Z. Design of a short pulse width CW signal generator. Mod. Nav. 2012, 3, 190–192. [Google Scholar]
- Lee, S.; Lim, J. Reverberation suppression using non-negative matrix factorization to detect low-Doppler target with continuous wave active sonar. EURASIP J. Adv. Signal Process. 2019, 2019, 11. [Google Scholar] [CrossRef]
- Hao, P.; Yan, S.; Xu, D.; Shi, B. Active Sonar Constant False Alarm Processing Technology, 1st ed.; Posts & Telecom Press: Beijing, China, 2021; pp. 28–34. [Google Scholar]
- Kim, G.; Lee, K.; Lee, S. Linear Frequency Modulated Reverberation Suppression Using Non-Negative Matrix Factorization Methods, Dechirping Transformation and Modulo Operation. IEEE Access 2020, 8, 110720–110737. [Google Scholar] [CrossRef]
- Finn, H.M.; Johnson, R.S. Adaptive detection mode with threshold control as a function spatially sampled clutter-level estimates. RCA Rev. 1968, 29, 414–464. [Google Scholar]
- Cai, J.; Hu, S.; Jin, Y.; Mo, J.; Yan, T.; Xia, M. A CA-CFAR-Like detector using the Gerschgorin circle theorem for bistatic space based radar. IEEE Trans. Aerosp. Electron. Syst. 2021, 57, 4137–4148. [Google Scholar] [CrossRef]
- Trunk, G.V. Range resolution of targets using automatic detectors. IEEE Trans. AES 1978, 14, 750–755. [Google Scholar] [CrossRef]
- Hansen, V.G. Constant false alarm rate processing in search radars. In Proceedings of the IEE International Radar, London, UK, 20–23 October 1973; pp. 325–332. [Google Scholar]
- Smith, M.E.; Varshney, P.K. Intelligent CFAR processor based on data variability. IEEE Trans. Aerosp. Electron. Syst. 2000, 36, 837–847. [Google Scholar] [CrossRef]
- Patel, V.; Madhukar, H.; Ravichandran, S. Variability index constant false alarm rate for marine target detection. In Proceedings of the 2018 Conference on Signal Processing and Communication Engineering Systems (SPACES), Vijayawada, India, 4–5 January 2018; pp. 171–175. [Google Scholar]
- Ji, Y.; Liu, A.; Chen, X.; Wang, J.; Yu, C. Target Detection Method for High-Frequency Surface Wave Radar RD Spectrum Based on (VI)CFAR-CNN and Dual-Detection Maps Fusion Compensation. Remote Sens. 2024, 16, 332. [Google Scholar] [CrossRef]
- Yang, J.; Tang, D.; Hefner, B.T.; Williams, K.L.; Preston, J.R. Overview of mid-frequency reverberation data acquired during the target and reverberation experiment 2013. IEEE J. Ocean. Eng. 2018, 43, 563–585. [Google Scholar] [CrossRef]
- Cole, B.; Davis, J.; Leen, W.; Powers, W.; Hanrahan, J. Coherent bottom reverberation: Modeling and comparisons with at-sea measurements. J. Acoust. Soc. Am. 2004, 116, 1985–1994. [Google Scholar] [CrossRef]
- Li, F.; Jin, G.; Zhang, R. The coherent theory and an oscillation phenomenon of the reverberation in shallow water. Sci. China Ser. A. 2000, 30, 560–566. [Google Scholar]
- Li, F.; Jin, G.; Zhang, R.; Liu, J.; Guo, L. An oscillation phenomenon of reverberation in the shallow water with a thermocline. J. Sound Vib. 2002, 252, 457–468. [Google Scholar] [CrossRef]
- Li, F.; Liu, J.; Li, Z.; Zhang, R. An oscillation phenomenon of low-frequency reverberation in the shallow water and its physical explanation. Sci. China Ser. G 2005, 48, 413–421. [Google Scholar] [CrossRef]
- Yin, L.; Wu, J.; Hou, Q. Fluctuation of short-range reverberation in shallow water. J. Appl. Acoust. 2021, 40, 828–832. [Google Scholar]
- Yin, L.; Wu, J.; Hou, Q.; Chen, J. A simulation of finite element and analysis of short-range reverberation intensity in shallow water. Tech. Acoust. 2019, 38, 481–483. [Google Scholar]
- Choo, Y.; Lee, K.; Hong, W.; Byun, S.-H.; Yang, H. Active underwater target detection using a shallow neural network with spectrogram-based temporal variation features. IEEE J. Ocean. Eng. 2022, 49, 279–293. [Google Scholar] [CrossRef]
- Li, Y.; Xu, Y.; Zhou, Z. Analysis of characteristics and research of modeling simulation on marine reverberation. Ship Electron. Eng. 2018, 38, 86–88+182. [Google Scholar]
- Jiang, Y.; Luo, Y.; Shi, J. The research on simulation of seabed reverberation model based on multi-beam sounding system. In Proceedings of the 2016 IEEE/OES China Ocean Acoustics (COA), Harbin, China, 9–11 January 2016; pp. 1–5. [Google Scholar]
- Holland, C.W.; Smith, C.M.; Lowe, Z.; Dorminy, J. Seabed Observations at the New England Mud Patch: Reflection and Scattering Measurements and Direct Geoacoustic Information. IEEE J. Ocean. Eng. 2022, 47, 578–593. [Google Scholar] [CrossRef]
- Luo, J.; Zhu, P.; Zhang, Z.; Chen, Y. Seabed characterization based on the statistical classification using the seabed reflection amplitudes of sub-bottom profiler data. Cont. Shelf Res. 2024, 279, 105293. [Google Scholar] [CrossRef]
- Xu, X.; Zhang, Y.; Li, H.; Yang, S. A model of seafloor reverberation simulation. Acta Acust. 1998, 23, 141–148. [Google Scholar]
- Yang, Y.; Shao, X.; Shi, Y.; Zhang, W. Back-stepping Robust Control for Flexible Air-breathing Hypersonic Vehicle via α-filter-based Uncertainty and Disturbance Estimator. Int. J. Control Autom. Syst. 2021, 19, 753–766. [Google Scholar] [CrossRef]
- Lu, S.; Hu, P.; Ding, F. Robust sonar CFAR detection algorithm in complex non-homogeneous background. Tech. Acoust. 2020, 39, 744–751. [Google Scholar]
- Ruppel, C.D.; Weber, T.C.; Staaterman, E.R.; Labak, S.J.; Hart, P.E. Categorizing Active Marine Acoustic Sources Based on Their Potential to Affect Marine Animals. J. Mar. Sci. Eng. 2022, 10, 1278. [Google Scholar] [CrossRef]





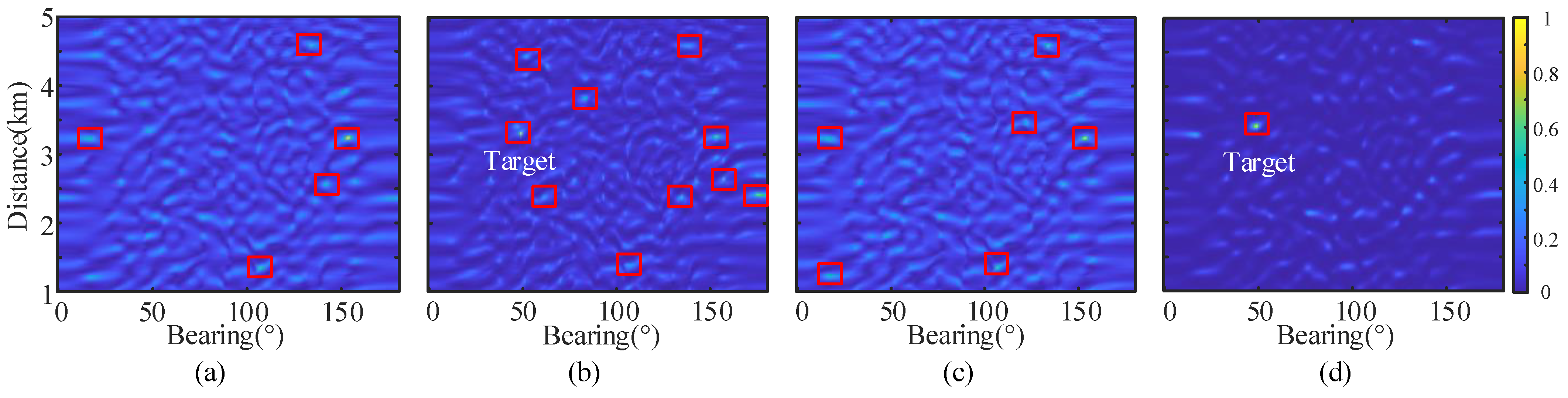
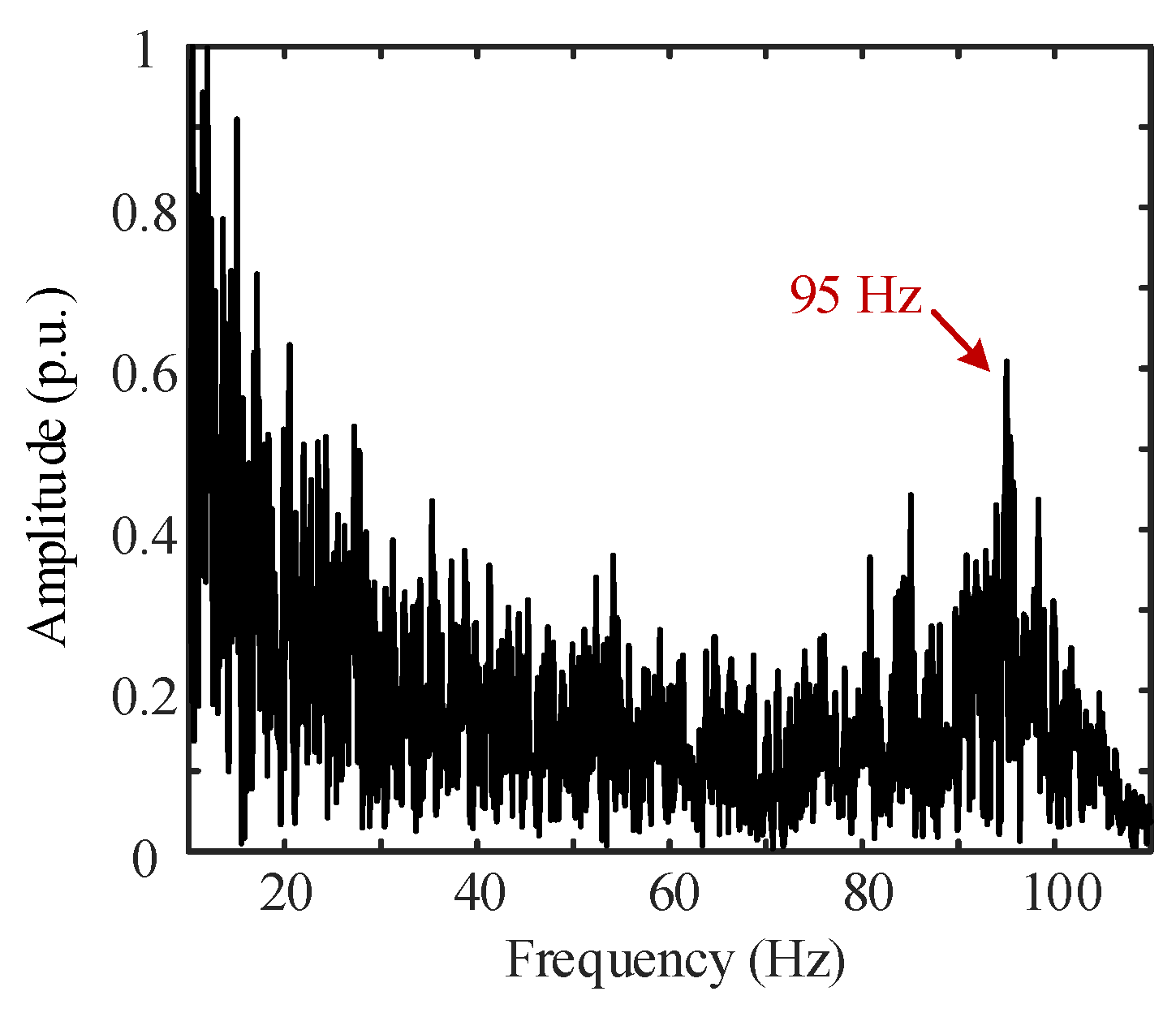


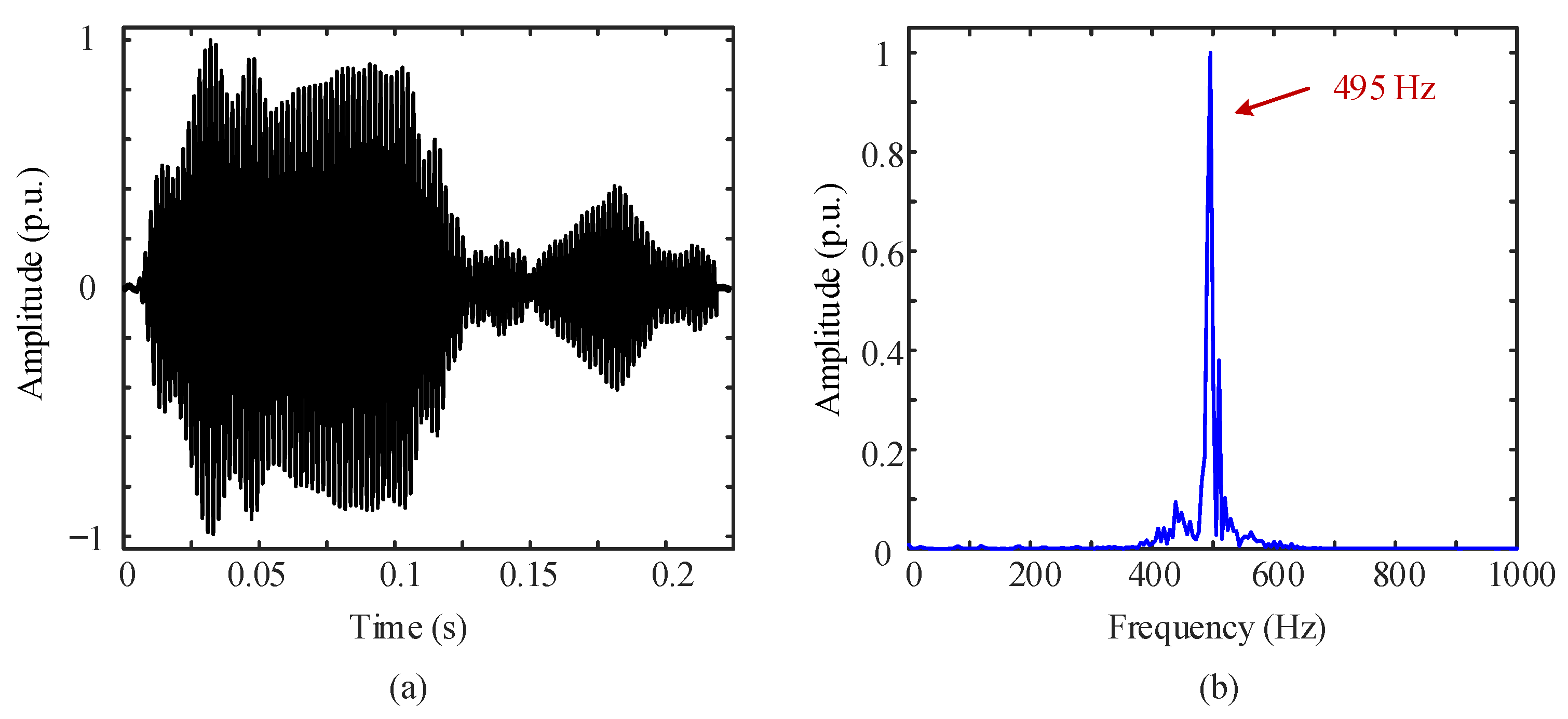
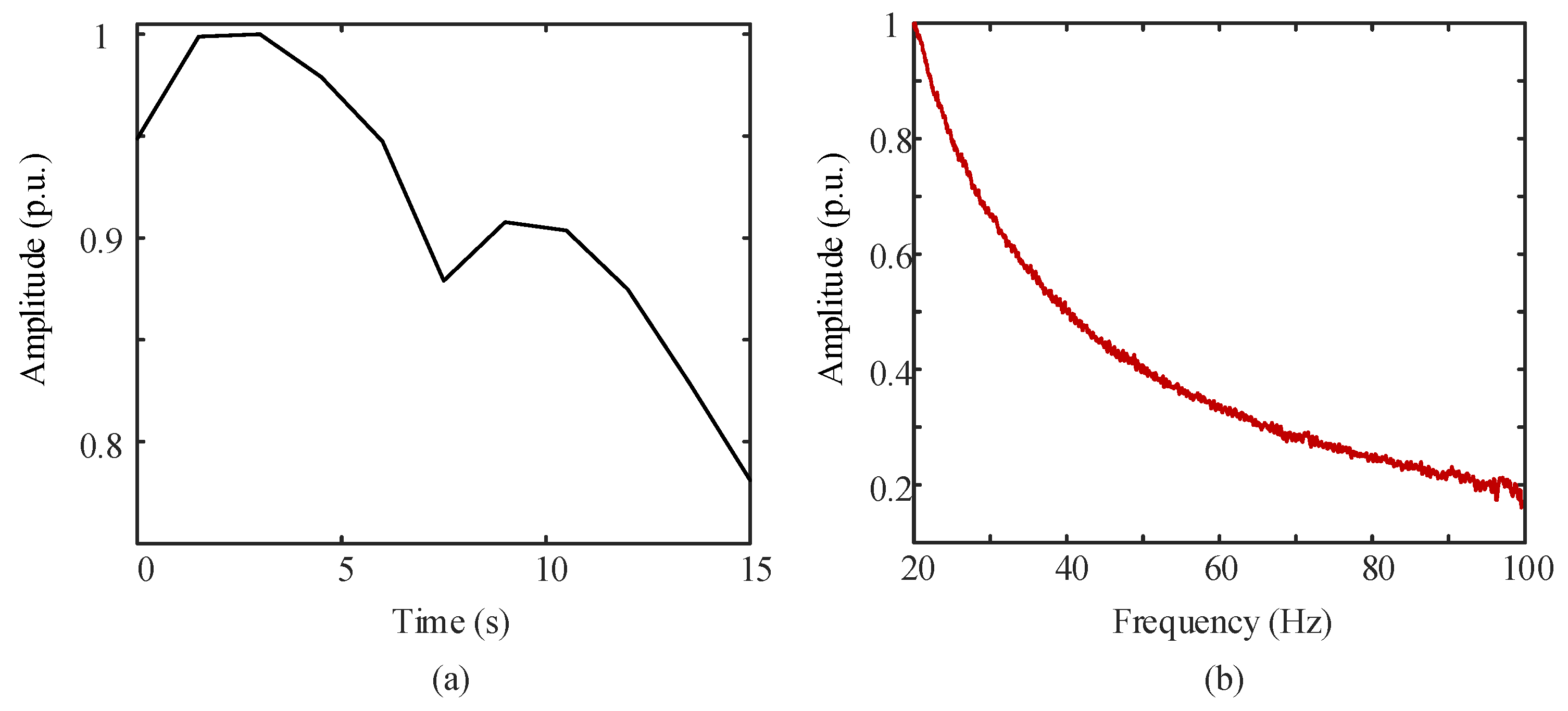
| Method | CA-CFAR | SO-CFAR | GO-CFAR | α-CA-CFAR |
|---|---|---|---|---|
| Low-frequency oscillation (p.u.) | 0.78 | 0.66 | 0.81 | 0.66 |
| High-frequency oscillation (p.u.) | 0.98 | 0.89 | 0.9 | 0.55 |
| Target (p.u.) | 1 | 0.8 | 1 | 1 |
| Target/Low-frequency oscillation | 1.28 | 1.21 | 1.23 | 1.52 |
| Target/High-frequency oscillation | 1.02 | 0.82 | 1.11 | 1.82 |
| SRR\Method | CA-CFAR | SO-CFAR | GO-CFAR | α-CA-CFAR |
|---|---|---|---|---|
| 15 dB | 1 | 0.973 | 0.999 | 1 |
| 10 dB | 0.904 | 0.810 | 0.873 | 0.995 |
| 5 dB | 0.488 | 0.524 | 0.507 | 0.850 |
| 0 dB | 0.156 | 0.283 | 0.216 | 0.575 |
| −5 dB | 0.05 | 0.167 | 0.085 | 0.385 |
| RNR\Method | CA-CFAR | SO-CFAR | GO-CFAR | α-CA-CFAR |
|---|---|---|---|---|
| 15 dB | 1 | 1 | 1 | 0.023 |
| 10 dB | 0.952 | 0.990 | 0.827 | 0.012 |
| 5 dB | 0.194 | 0.422 | 0.102 | 0.008 |
| 0 dB | 0.010 | 0.024 | 0.005 | 0 |
| −5 dB | 0 | 0 | 0 | 0 |
| System Parameters | Parameter Settings |
|---|---|
| Water depth | 80 m |
| Receive distance | 3 km |
| Signal frequency | 495 Hz |
| Sound source level | 212 dB |
| Waveform | CW |
| Pulse width | 0.05 s/0.1 s/0.2 s/0.5 s |
| Target strength | −20 dB |
| Source depth | 40 m |
| Transponder depth | 40 m |
| Array depth | 30 m |
| Array elements | 16 |
| Array form | Line |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cui, Y.; Liao, S.; Gao, J.; Zhu, H.; Zhao, N.; Luo, A. Cascaded-Filter-Based Reverberation Suppression Method of Short-Pulse Continuous Wave for Active Sonar. Remote Sens. 2024, 16, 2949. https://doi.org/10.3390/rs16162949
Cui Y, Liao S, Gao J, Zhu H, Zhao N, Luo A. Cascaded-Filter-Based Reverberation Suppression Method of Short-Pulse Continuous Wave for Active Sonar. Remote Sensing. 2024; 16(16):2949. https://doi.org/10.3390/rs16162949
Chicago/Turabian StyleCui, Yonglin, Shuhan Liao, Juncheng Gao, Haidong Zhu, Nengtong Zhao, and An Luo. 2024. "Cascaded-Filter-Based Reverberation Suppression Method of Short-Pulse Continuous Wave for Active Sonar" Remote Sensing 16, no. 16: 2949. https://doi.org/10.3390/rs16162949
APA StyleCui, Y., Liao, S., Gao, J., Zhu, H., Zhao, N., & Luo, A. (2024). Cascaded-Filter-Based Reverberation Suppression Method of Short-Pulse Continuous Wave for Active Sonar. Remote Sensing, 16(16), 2949. https://doi.org/10.3390/rs16162949





