A Simple Path to the Small Perturbation Method for Scattering from Slightly Rough Dielectric Surfaces
Abstract
1. Introduction
2. Volumetric Perturbative Reciprocal Approach
3. Scattering from a Slightly Rough Surface
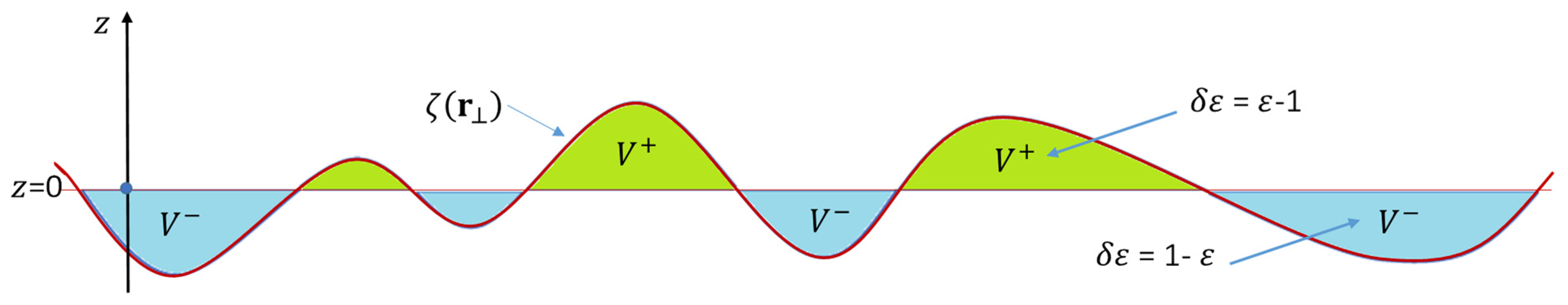
3.1. Medium Perturbation
3.2. Internal Field Approximation
3.3. Unperturbed Fields
3.4. Scattered Fields
3.5. Normalised Radar Cross-Section
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Rice, S.O. Reflection of Electromagnetic Waves from Slightly Rough Surfaces. Commun. Pure 1951, 4, 361–378. [Google Scholar] [CrossRef]
- Rayleigh, L. Theory of Sound; Macmillan: London, UK, 1920; Volume II, pp. 89–96. [Google Scholar]
- Ulaby, F.T.; Moore, R.K.; Fung, A.K. Microwave Remote Sensing; Artech House: Reading, MA, USA, 1982; Volume II. [Google Scholar]
- Tsang, L.; Kong, J.A. Scattering of Electromagnetic Waves: Advanced Topics; Wiley: New York, NY, USA, 2001. [Google Scholar]
- Wright, J. A new model for sea clutter. IEEE Trans. Antennas Propagat. 1968, 16, 217–223. [Google Scholar] [CrossRef]
- Valenzuela, G.R. Scattering of electromagnetic waves from a tilted slightly rough surface. Radio Sci. 1968, 3, 1057–1066. [Google Scholar] [CrossRef]
- Di Martino, G.; Di Simone, A.; Iodice, A.; Riccio, D. Bistatic scattering from anisotropic rough surfaces via a closed-form two-scale model. IEEE Trans. Geosci. Remote Sens. 2021, 59, 3656–3671. [Google Scholar] [CrossRef]
- Tabatabaeenejad, A.; Moghaddam, M. Study of Validity Region of Small Perturbation Method for Two-Layer Rough Surfaces. IEEE Geosci. Remote Sens. Lett. 2010, 7, 319–323. [Google Scholar] [CrossRef]
- Imperatore, P. Perturbation Theory for Electromagnetic Wave Scattering in Random Layered Structures. Ph.D. Thesis, University of Naples Federico II, Napoli, Italy, 2010. [Google Scholar]
- Imperatore, P.; Iodice, A.; Riccio, D. Electromagnetic Wave Scattering from Layered Structures with an Arbitrary Number of Rough Interfaces. IEEE Trans. Geosci. Remote Sens. 2009, 47, 1056–1072. [Google Scholar] [CrossRef]
- Imperatore, P.; Iodice, A.; Riccio, D. Transmission Through Layered Media with Rough Boundaries: First-Order Perturbative Solution. IEEE Trans. Antennas Propagat. 2009, 57, 1481–1494. [Google Scholar] [CrossRef]
- Imperatore, P.; Iodice, A.; Riccio, D. Physical Meaning of Perturbative Solutions for Scattering from and Through Multilayered Structures with Rough Interfaces. IEEE Trans. Antennas Propagat. 2010, 58, 2710–2724. [Google Scholar] [CrossRef]
- Voronovich, A. Small-slope approximation for electromagnetic wave scattering at a rough interface of two dielectric half-spaces. Waves Random Media 1994, 4, 337–367. [Google Scholar] [CrossRef]
- Sarabandi, K.; Chiu, T. Electromagnetic scattering from slightly rough surface with inhomogeneous dielectric profiles. IEEE Trans. Antennas Propagat. 1997, 45, 1419–1430. [Google Scholar] [CrossRef]
- Hill, N.R. Integral-equation perturbative approach to optical scattering from rough surfaces. Phys. Rev. B 1981, 24, 7112. [Google Scholar] [CrossRef]
- Guérin, C.; Sentenac, A. Second-order perturbation theory for scattering from heterogeneous rough surfaces. J. Opt. Soc. Am. A 2004, 21, 1251–1260. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Imperatore, P.; Iodice, A.; Riccio, D. Reciprocity, Coupling and Scattering: A New Look at SPM for Rough Surface. In Proceedings of the European Microwave Conference, Rome, Italy, 29 September–1 October 2009; pp. 994–997. [Google Scholar]
- Imperatore, P.; Iodice, A.; Riccio, D. Consistency and Validity of Perturbative Formulations for Scattering from Rough Multilayers. IEEE Trans. Antennas Propagat. 2012, 60, 2019–2027. [Google Scholar] [CrossRef]
- Freeman, A.; Durden, S.L. A Three-Component Scattering Model for Polarimetric SAR Data. IEEE Trans. Geosci. Remote Sens. 1998, 36, 963–973. [Google Scholar] [CrossRef]
- Chen, S.W.; Wang, X.S.; Xiao, S.P.; Sato, M. General Polarimetric Model-Based Decomposition for Coherency Matrix. IEEE Trans. Geosci. Remote Sens. 2014, 52, 1843–1855. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Iodice, A.; Imperatore, P. A Simple Path to the Small Perturbation Method for Scattering from Slightly Rough Dielectric Surfaces. Remote Sens. 2024, 16, 3035. https://doi.org/10.3390/rs16163035
Iodice A, Imperatore P. A Simple Path to the Small Perturbation Method for Scattering from Slightly Rough Dielectric Surfaces. Remote Sensing. 2024; 16(16):3035. https://doi.org/10.3390/rs16163035
Chicago/Turabian StyleIodice, Antonio, and Pasquale Imperatore. 2024. "A Simple Path to the Small Perturbation Method for Scattering from Slightly Rough Dielectric Surfaces" Remote Sensing 16, no. 16: 3035. https://doi.org/10.3390/rs16163035
APA StyleIodice, A., & Imperatore, P. (2024). A Simple Path to the Small Perturbation Method for Scattering from Slightly Rough Dielectric Surfaces. Remote Sensing, 16(16), 3035. https://doi.org/10.3390/rs16163035






