Near-Inertial Oscillations Induced by Winter Monsoon Onset in the Southwest Taiwan Strait
Abstract
:1. Introduction
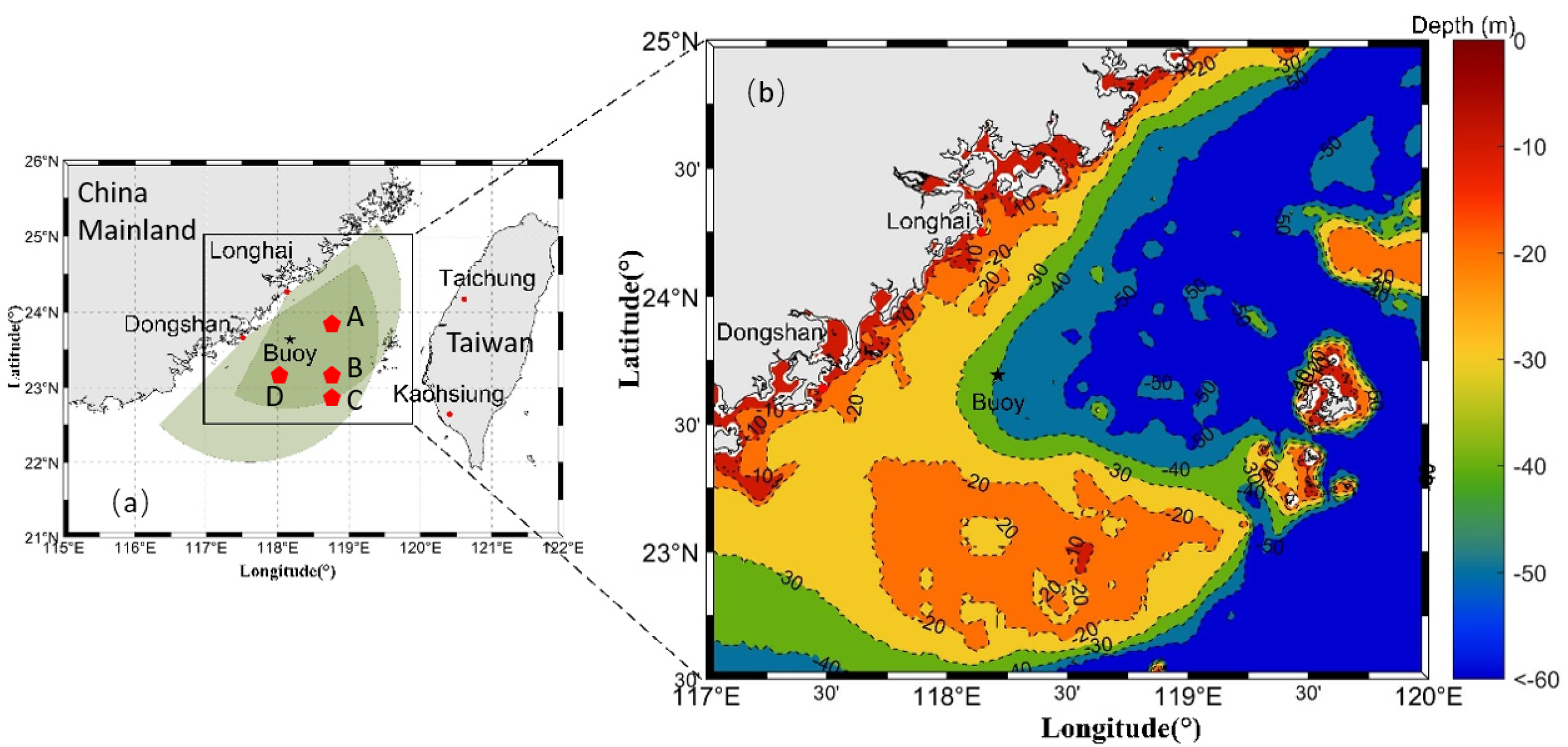
2. Data and Method
2.1. Ocean Surface Current
2.2. Local Wind
2.3. Air and Sea Surface Temperature
2.4. Complex Demodulation
2.5. Slab Simulation
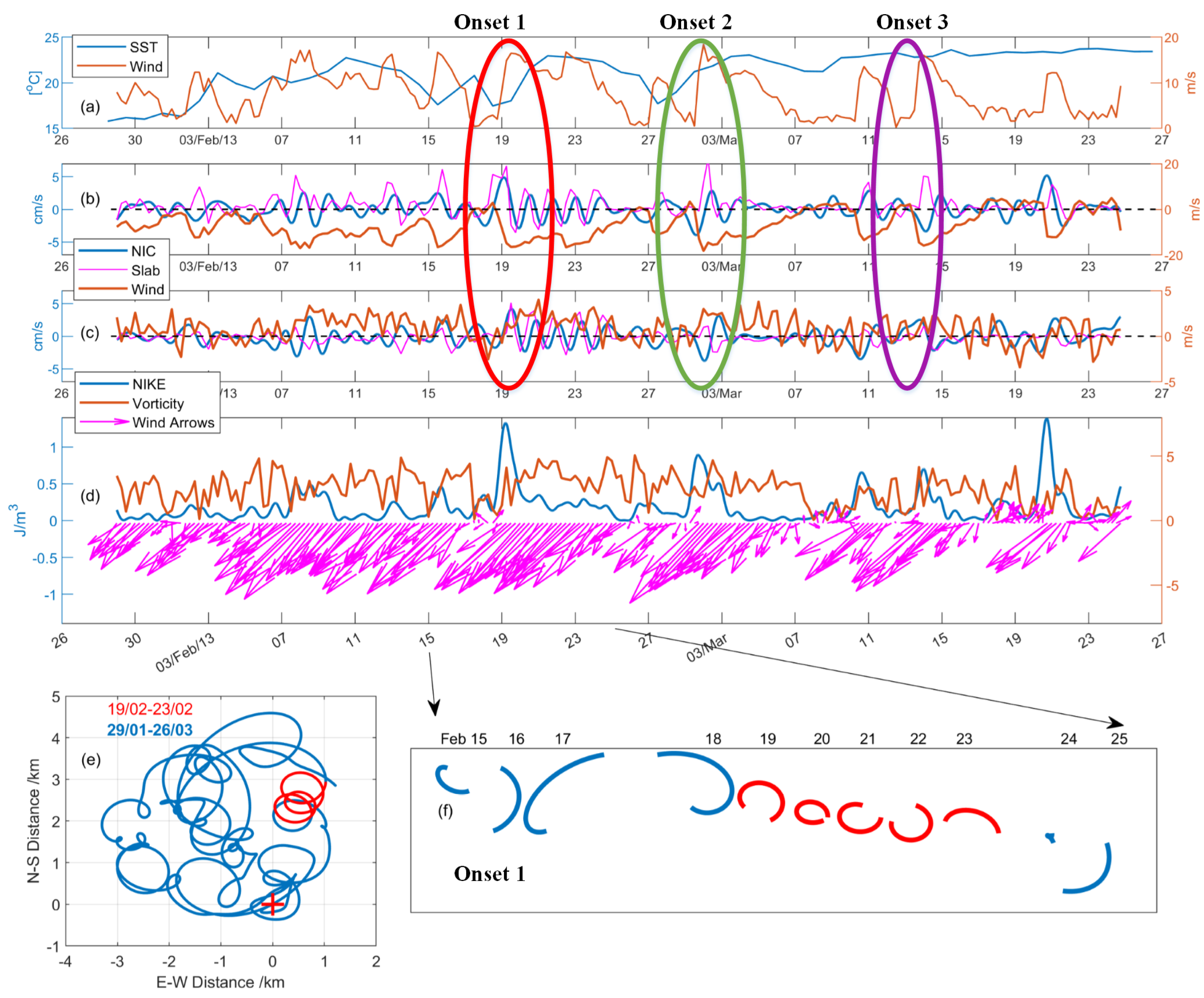
3. Results
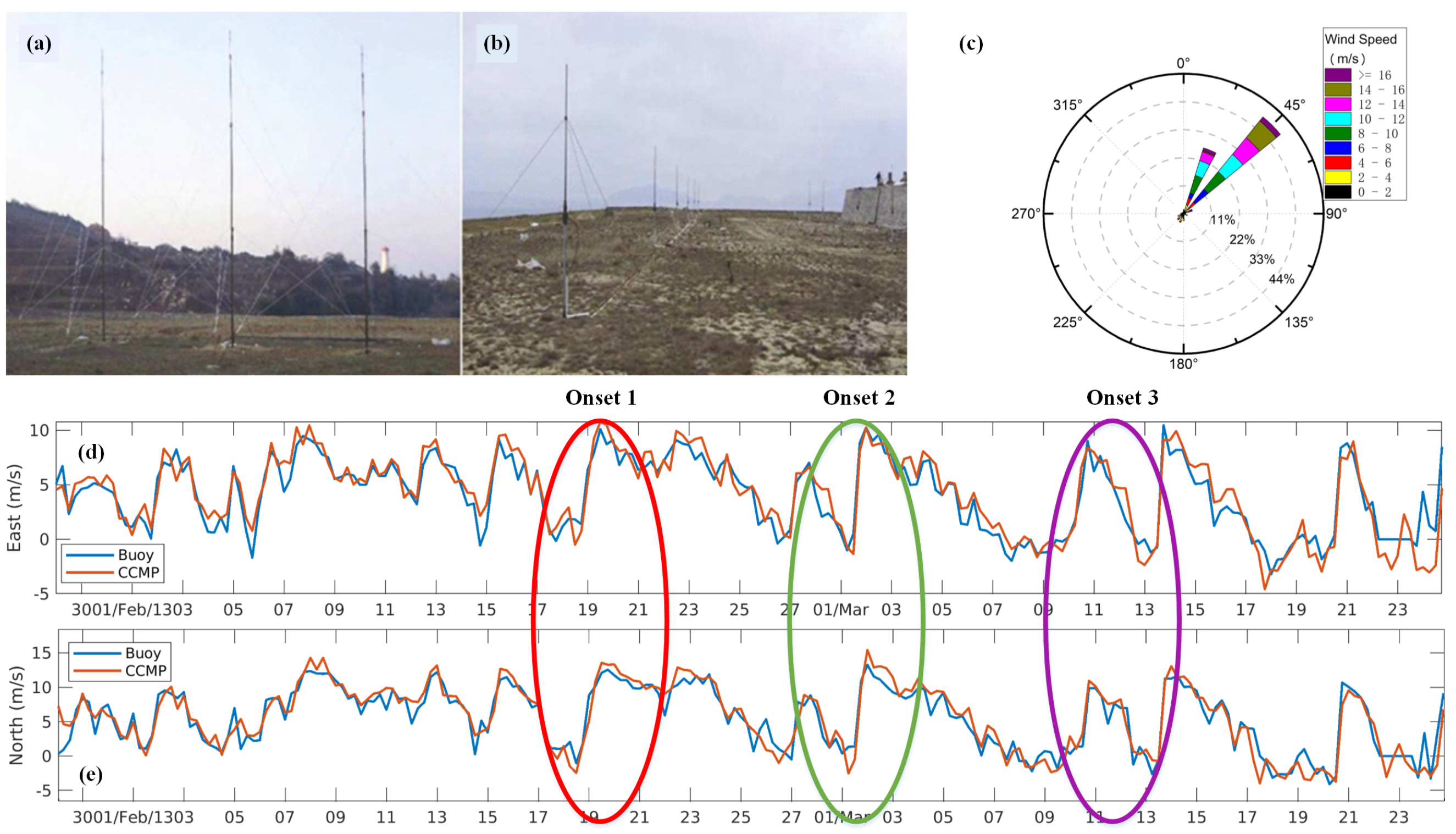
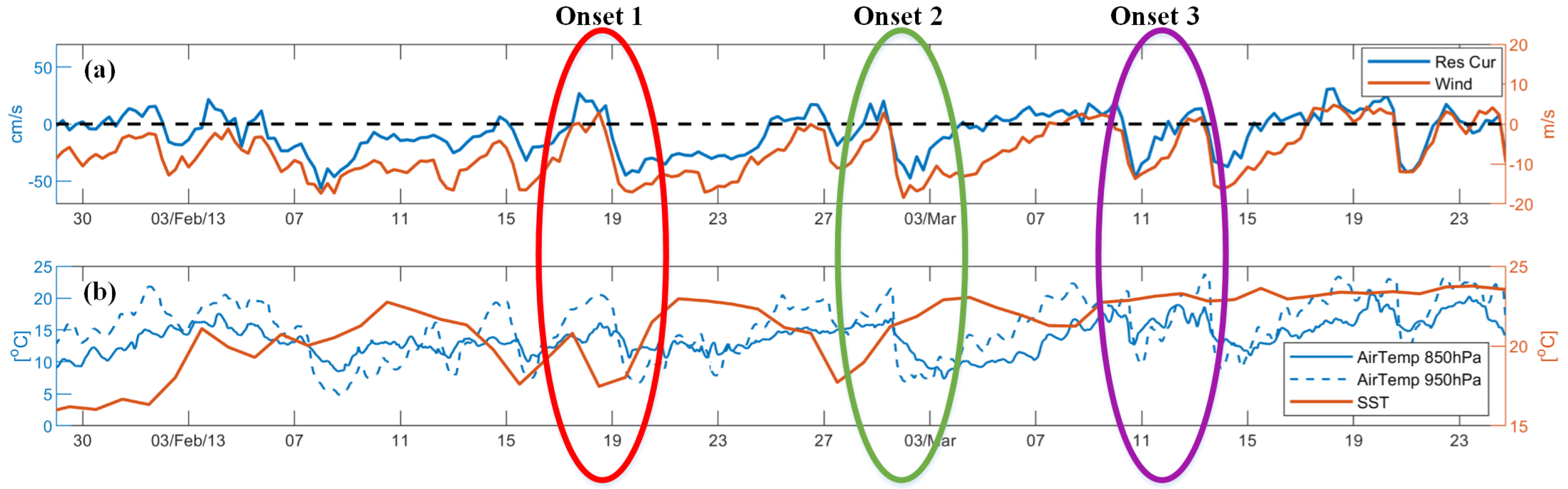
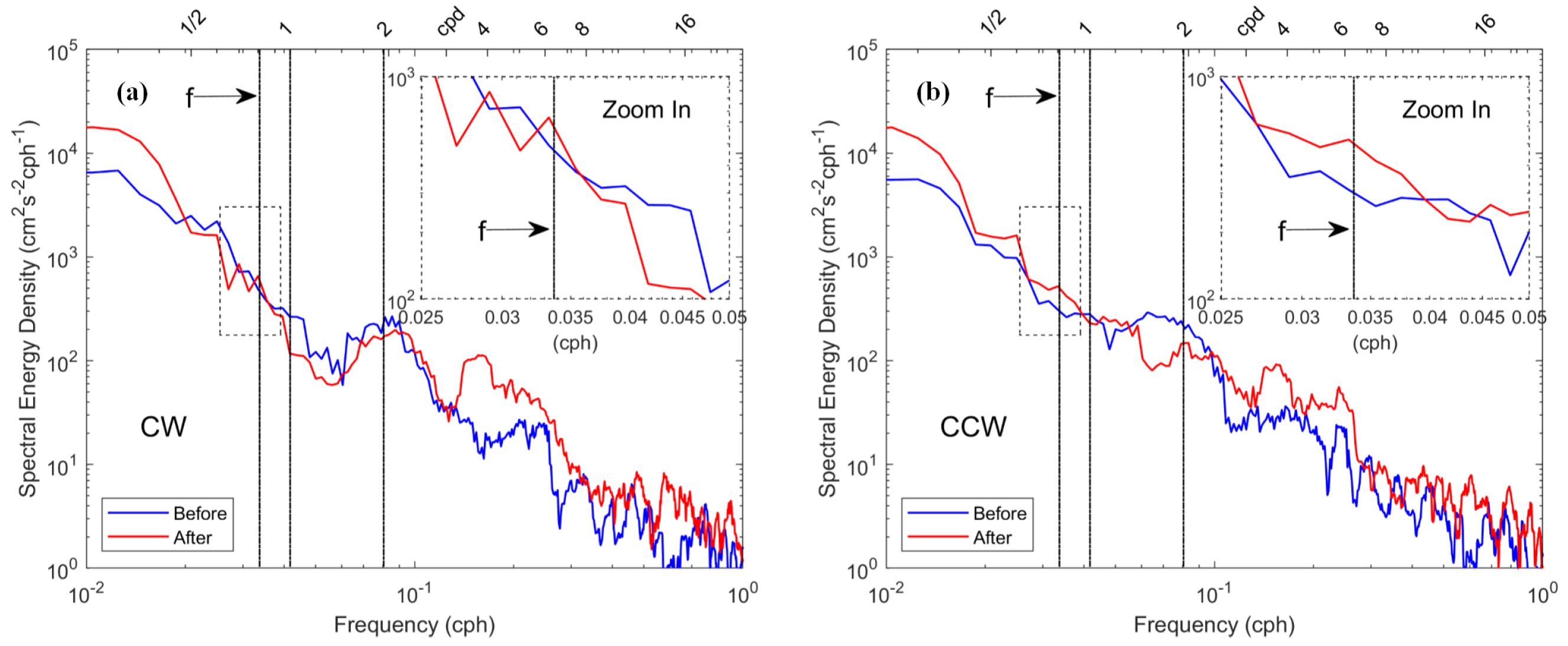
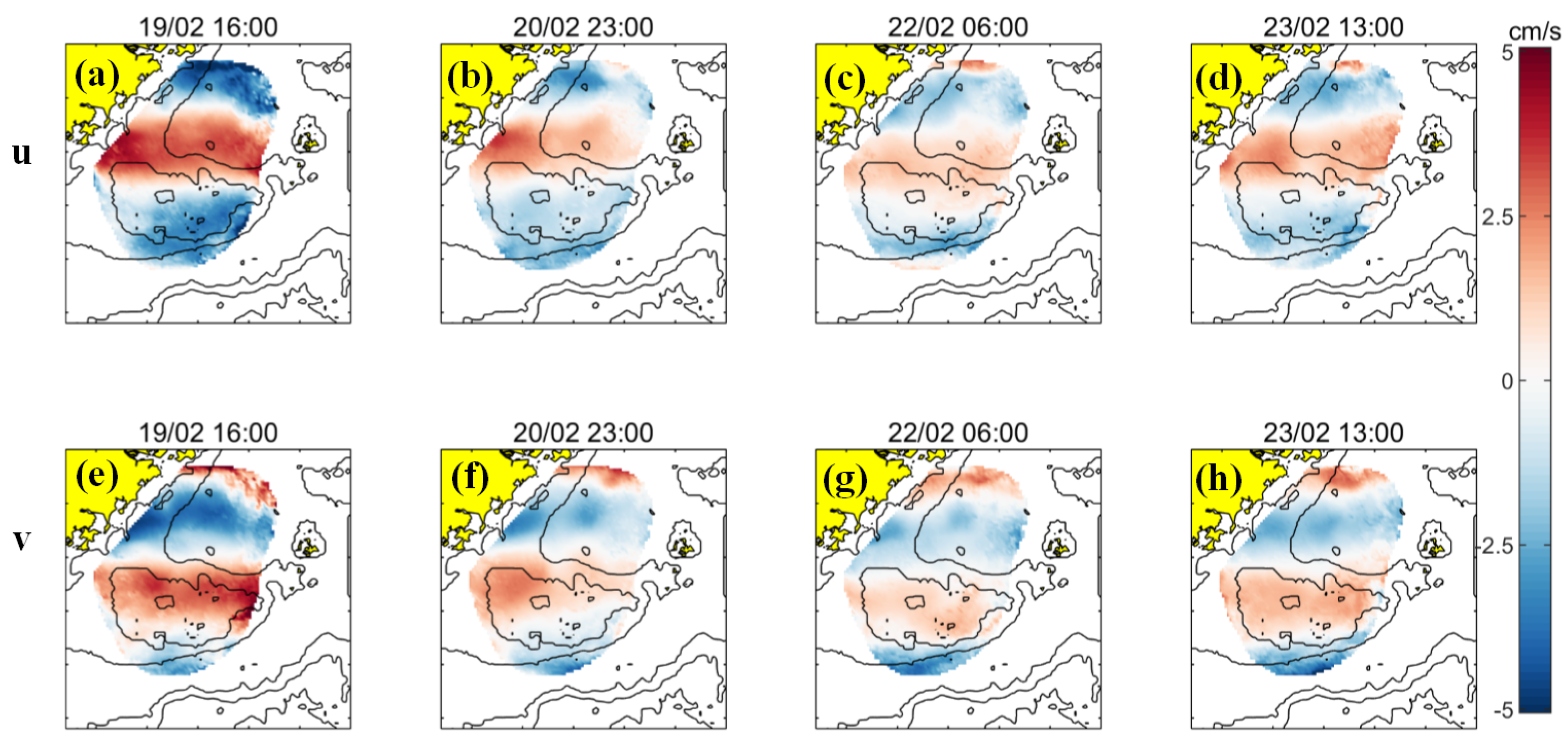
3.1. Characteristics of NIOs Induced by Monsoon Onset
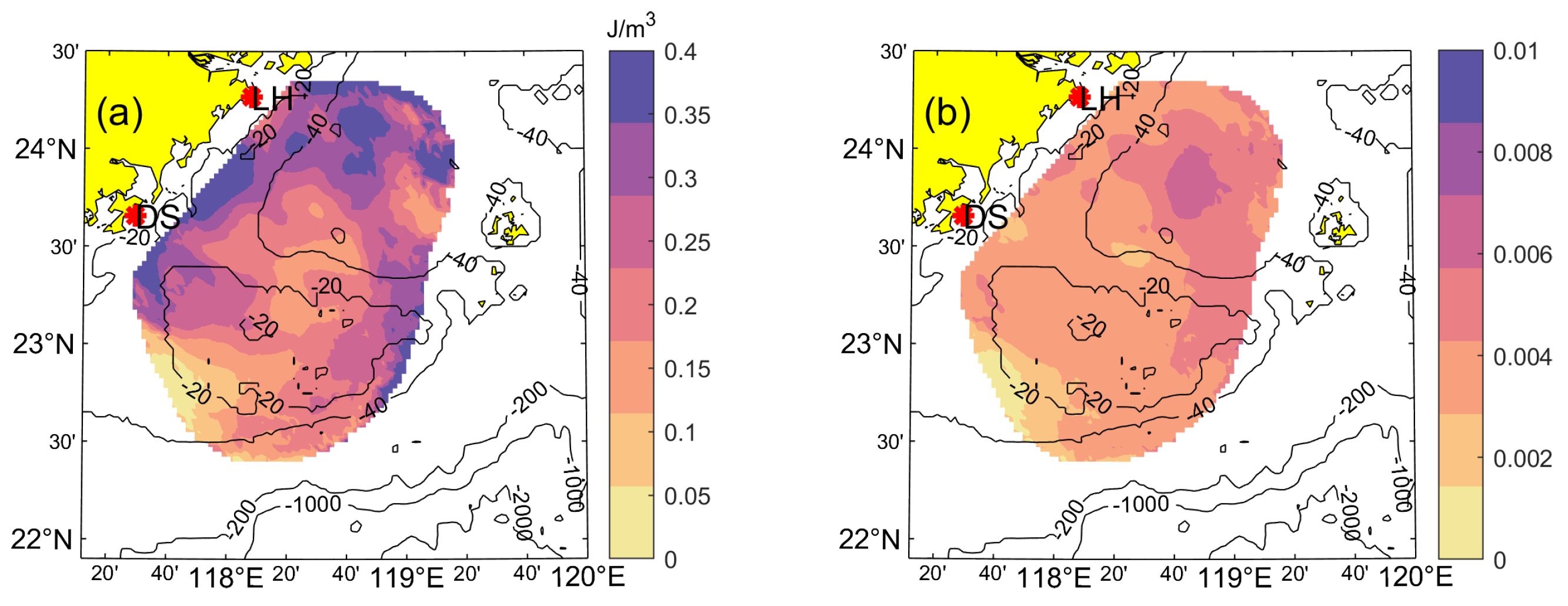
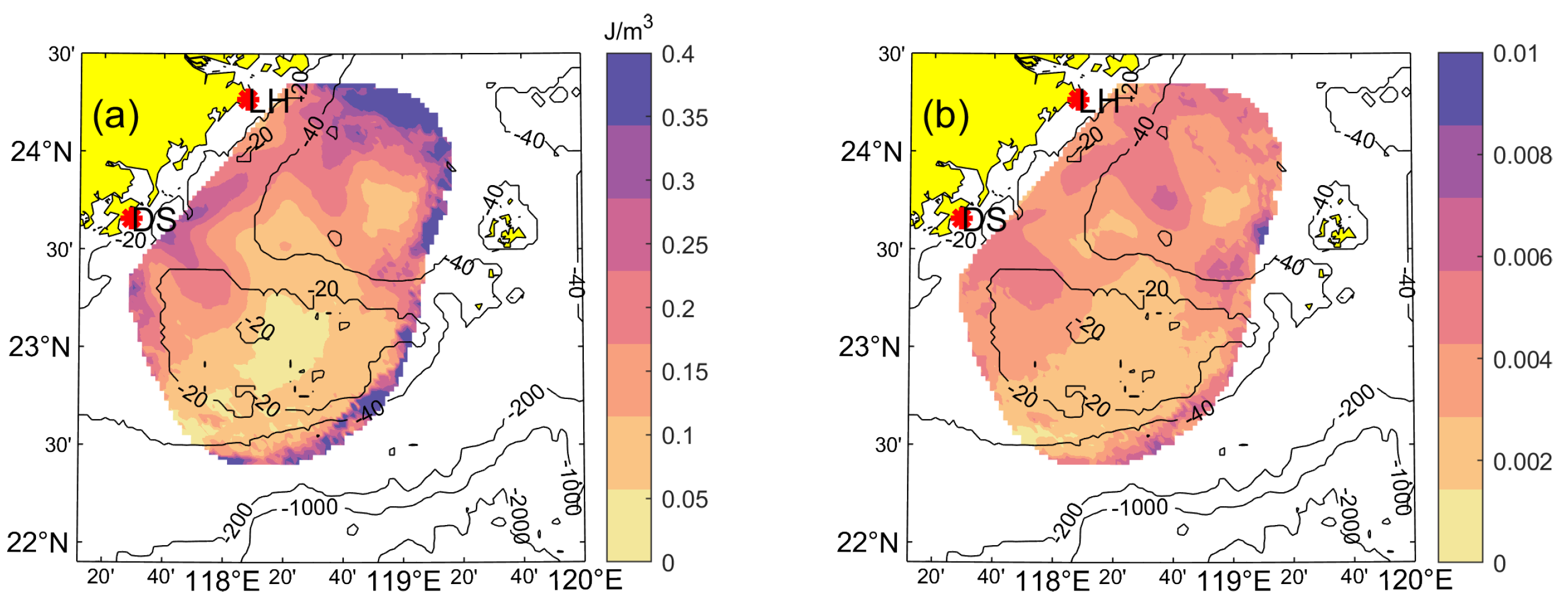
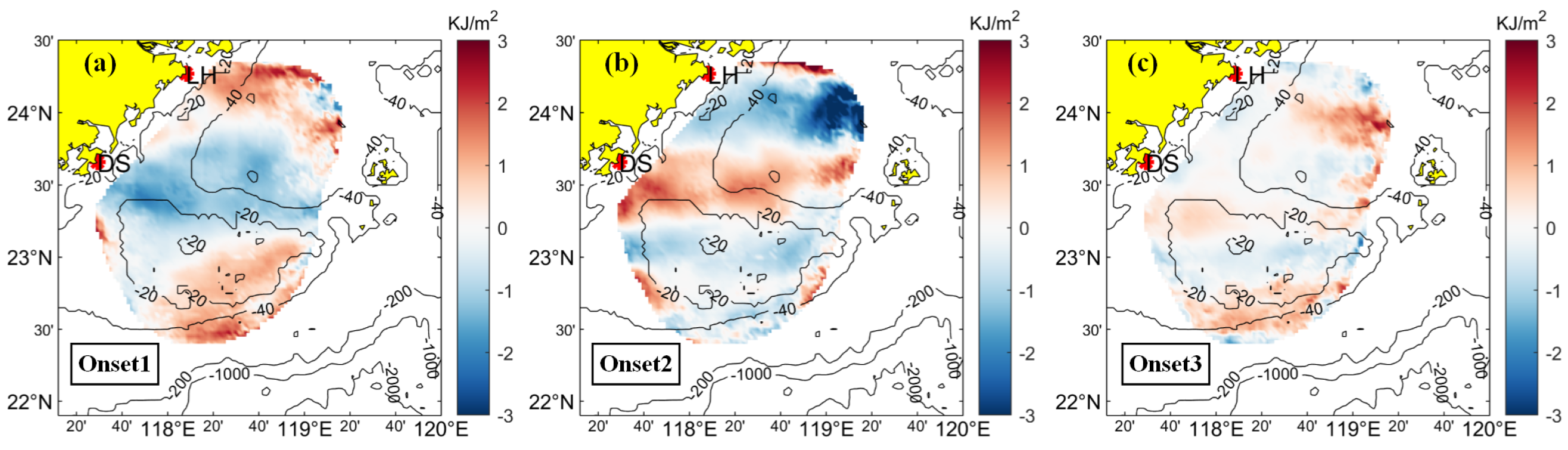
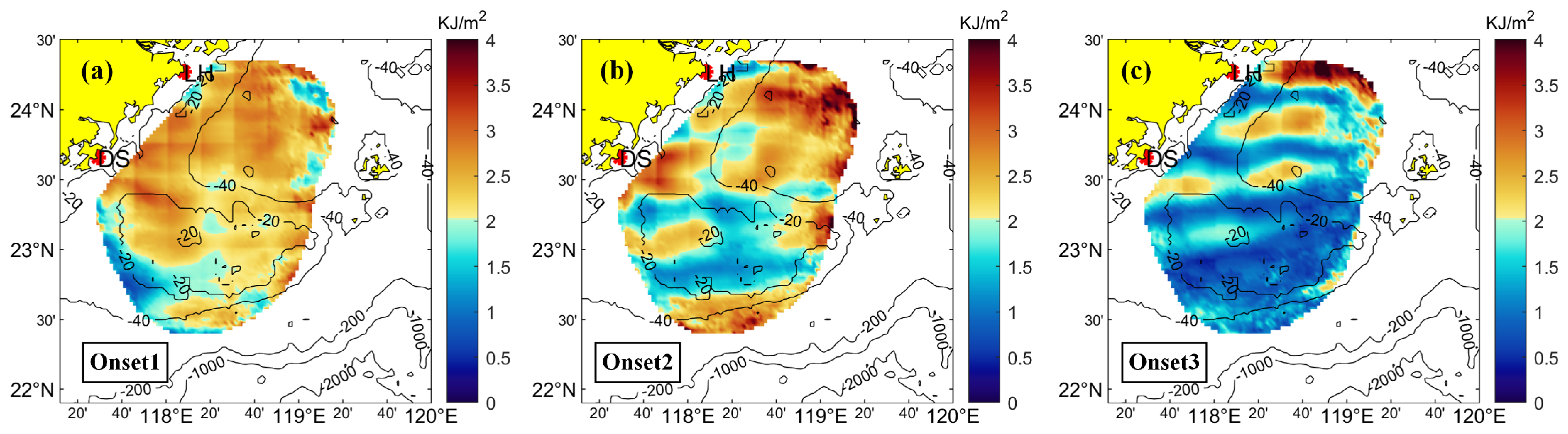
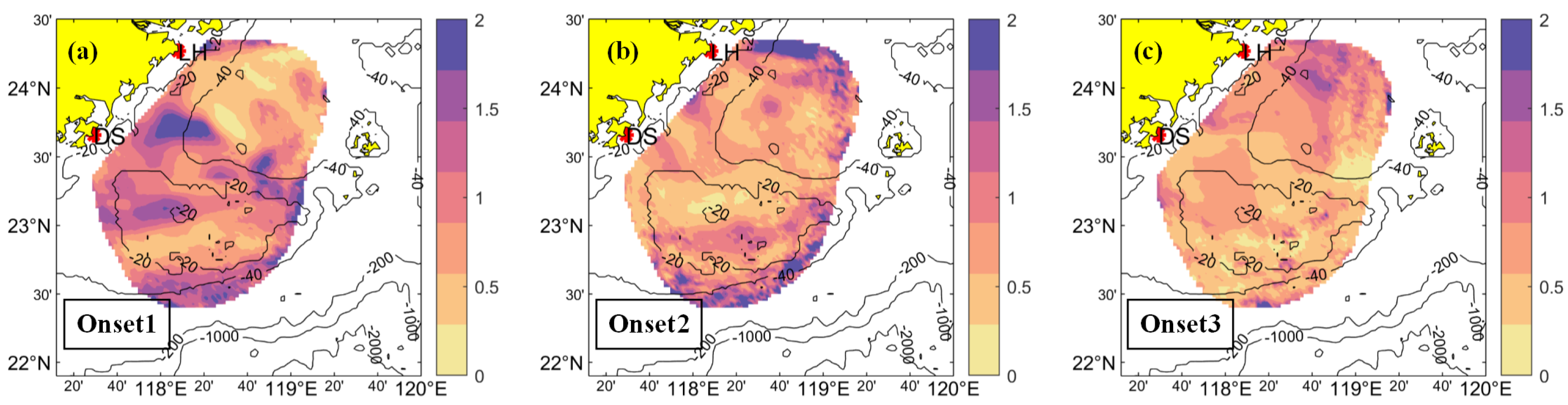
3.2. Spatial Distribution of NIKE
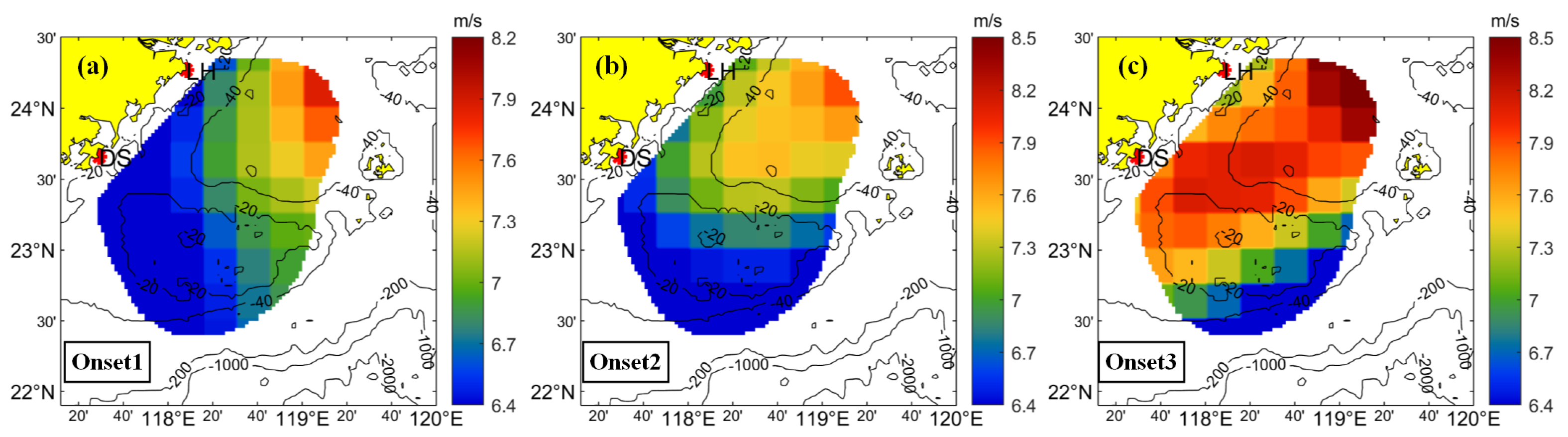
3.3. Variation in Wind and SST
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Yang, B.; Hu, P.; Hou, Y. Observed near-inertial waves in the Northern South China Sea. Remote Sens. 2021, 13, 3223. [Google Scholar]
- Alford, M.H. Internal swell generation: The spatial distribution of energy flux from the wind to mixed layer near-inertial motions. J. Phys. Oceanogr. 2001, 31, 2359–2368. [Google Scholar]
- Comby, C.; Petrenko, A.; Estournel, C.; Marsaleix, P.; Ulses, C.; Bosse, A.; Doglioli, A.; Barrillon, S. Near Inertial Oscillations and Vertical Velocities Modulating Phytoplankton After a Storm in the Mediterranean Sea. J. Water Resour. Ocean. Sci. 2023, 12, 31–37. [Google Scholar]
- Tan, Z.; Wang, J.; Guo, J.; Liu, C.; Zhang, M.; Ma, S. Improving satellite-retrieved cloud base height with ground-based cloud radar measurements. Adv. Atmos. Sci. 2024, 41, 2131–2140. [Google Scholar]
- Dippe, T.; Zhai, X.; Greatbatch, R.J.; Rath, W. Interannual variability of wind power input to near-inertial motions in the North Atlantic. Ocean Dyn. 2015, 65, 859–875. [Google Scholar]
- Sun, B.; Wang, S.; Yuan, M.; Wang, H.; Jing, Z.; Chen, Z.; Wu, L. Energy flux into near-inertial internal waves below the surface boundary layer in the global ocean. J. Phys. Oceanogr. 2021, 51, 2315–2328. [Google Scholar]
- Wang, J.; Torres, H.; Klein, P.; Wineteer, A.; Zhang, H.; Menemenlis, D.; Ubelmann, C.; Rodriguez, E. Increasing the observability of near inertial oscillations by a future ODYSEA satellite mission. Remote Sens. 2023, 15, 4526. [Google Scholar]
- Hisaki, Y.; Naruke, T. Horizontal variability of near-inertial oscillations associated with the passage of a typhoon. J. Geophys. Res. Oceans 2003, 108. [Google Scholar]
- Song, D.; Gao, G.; Xia, Y.; Ren, Z.; Liu, J.; Bao, X.; Yin, B. Near-inertial oscillations in seasonal highly stratified shallow water. Estuarine, Coast. Shelf Sci. 2021, 258, 107445. [Google Scholar]
- Niu, P.; Liao, G. Near-surface dynamic structure in the northern South China Sea and Northwest Pacific revealed using Lagrangian data. J. Oceanogr. 2023, 79, 445–460. [Google Scholar]
- Zhang, Y.; Yang, W.; Zhao, W.; Zhang, Y.; Shen, J.; Wei, H. Spatial and seasonal variations of near-inertial kinetic energy in the upper Ross Sea and the controlling factors. Front. Mar. Sci. 2023, 10, 1173900. [Google Scholar] [CrossRef]
- Liu, Y.; Weisberg, R.H.; Shay, L.K. Current patterns on the West Florida Shelf from joint self-organizing map analyses of HF radar and ADCP data. J. Atmos. Ocean. Technol. 2007, 24, 702–712. [Google Scholar] [CrossRef]
- Rubio, A.; Reverdin, G.; Fontán, A.; González, M.; Mader, J. Mapping near-inertial variability in the SE Bay of Biscay from HF radar data and two offshore moored buoys. Geophys. Res. Lett. 2011, 38. [Google Scholar] [CrossRef]
- Cosoli, S.; Gačić, M.; Mazzoldi, A. Surface current variability and wind influence in the northeastern Adriatic Sea as observed from high-frequency (HF) radar measurements. Cont. Shelf Res. 2012, 33, 1–13. [Google Scholar] [CrossRef]
- Wang, G.; Li, D.; Wei, Z.; Li, S.; Wang, Y.; Xu, T. Observed near inertial waves in the Wake of Typhoon Linfa (2015) in the Northern South China Sea. J. Ocean Univ. China 2019, 18, 1013–1021. [Google Scholar] [CrossRef]
- Kovačević, V.; Gačić, M.; Mosquera, I.M.; Mazzoldi, A.; Marinetti, S. HF radar observations in the northern Adriatic: Surface current field in front of the Venetian Lagoon. J. Mar. Syst. 2004, 51, 95–122. [Google Scholar] [CrossRef]
- Zhu, D. Near inertial oscillations in shelf-break of northern South China Sea after passage of typhoon Wayne. J. Trop. Oceanogr. 2007, 26, 1–7. [Google Scholar]
- Shrira, V.I.; Forget, P. On the nature of near-inertial oscillations in the uppermost part of the ocean and a possible route toward HF radar probing of stratification. J. Phys. Oceanogr. 2015, 45, 2660–2678. [Google Scholar] [CrossRef]
- Ma, Y.; Wang, D.; Shu, Y.; Chen, J.; He, Y.; Xie, Q. Bottom-reached near-inertial waves induced by the tropical cyclones, Conson and Mindulle, in the South China Sea. J. Geophys. Res. Oceans 2022, 127, e2021JC018162. [Google Scholar] [CrossRef]
- Kamli, E.; Chavanne, C.; Dumont, D. Experimental assessment of the performance of high-frequency CODAR and WERA radars to measure ocean currents in partially ice-covered waters. J. Atmos. Ocean. Technol. 2016, 33, 539–550. [Google Scholar] [CrossRef]
- Zheng, H.; Zhu, X.H.; Zhao, R.; Chen, J.; Wang, M.; Ren, Q.; Liu, Y.; Nan, F.; Yu, F.; Park, J.H. Near-inertial waves reaching the deep basin in the South China Sea after typhoon Mangkhut (2018). J. Phys. Oceanogr. 2023, 53, 2435–2454. [Google Scholar] [CrossRef]
- Li, R.; Chen, C.; Dong, W.; Beardsley, R.C.; Wu, Z.; Gong, W.; Liu, Y.; Liu, T.; Xu, D. Slope-intensified storm-induced near-inertial oscillations in the South China sea. J. Geophys. Res. Oceans 2021, 126, e2020JC016713. [Google Scholar] [CrossRef]
- Hou, H.; Xu, T.; Li, B.; Yang, B.; Wei, Z.; Yu, F. Different types of near-inertial internal waves observed by lander in the intermediate-deep layers of the South China Sea and their generation mechanisms. J. Mar. Sci. Eng. 2022, 10, 594. [Google Scholar] [CrossRef]
- Hu, Y.; Yu, F.; Chen, Z.; Si, G.; Liu, X.; Nan, F.; Ren, Q. Two near-inertial peaks in antiphase controlled by stratification and tides in the Yellow Sea. Front. Mar. Sci. 2023, 9, 1081869. [Google Scholar] [CrossRef]
- Lin, S.; Wang, Y.; Zhang, W.Z.; Ni, Q.B.; Chai, F. Tropical Cyclones Related Wind Power on Oceanic Near-Inertial Oscillations. Geophys. Res. Lett. 2023, 50, e2023GL105056. [Google Scholar] [CrossRef]
- Shu, Y.; Pan, J.; Wang, D.; Chen, G.; Sun, L.; Yao, J. Generation of near-inertial oscillations by summer monsoon onset over the South China Sea in 1998 and 1999. Deep. Sea Res. Part I Oceanogr. Res. Pap. 2016, 118, 10–19. [Google Scholar] [CrossRef]
- Shen, Z.; Wu, X.; Lin, H.; Chen, X.; Xu, X.A.; Li, L. Spatial distribution characteristics of surface tidal currents in the southwest of Taiwan Strait. J. Ocean Univ. China 2014, 13, 971–978. [Google Scholar] [CrossRef]
- Wang, L.; Pawlowicz, R.; Wu, X.; Yue, X. Wintertime variability of currents in the southwestern Taiwan Strait. J. Geophys. Res. Oceans 2021, 126, e2020JC016586. [Google Scholar] [CrossRef]
- Wei, G.; He, Z.; Xie, Y.; Shang, S.; Dai, H.; Wu, J.; Liu, K.; Lin, R.; Wan, Y.; Lin, H.; et al. Assessment of HF radar in mapping surface currents under different sea states. J. Atmos. Ocean. Technol. 2020, 37, 1403–1422. [Google Scholar] [CrossRef]
- Guan, B.; Fang, G. Winter counter-wind currents off the southeastern China coast: A review. J. Oceanogr. 2006, 62, 1–24. [Google Scholar] [CrossRef]
- Wang, D.; Hong, B.; Gan, J.; Xu, H. Numerical investigation on propulsion of the counter-wind current in the northern South China Sea in winter. Deep. Sea Res. Part I Oceanogr. Res. Pap. 2010, 57, 1206–1221. [Google Scholar] [CrossRef]
- Atlas, R.; Hoffman, R.N.; Ardizzone, J.; Leidner, S.M.; Jusem, J.C.; Smith, D.K.; Gombos, D. A cross-calibrated, multiplatform ocean surface wind velocity product for meteorological and oceanographic applications. Bull. Am. Meteorol. Soc. 2011, 92, 157–174. [Google Scholar]
- de Souza, J.M.A.C.; Couto, P.; Soutelino, R.; Roughan, M. Evaluation of four global ocean reanalysis products for New Zealand waters–A guide for regional ocean modelling. New Zealand J. Mar. Freshw. Res. 2021, 55, 132–155. [Google Scholar] [CrossRef]
- Bellotti, G.; Franco, L.; Cecioni, C. Regional downscaling of copernicus ERA5 wave data for coastal engineering activities and operational coastal services. Water 2021, 13, 859. [Google Scholar] [CrossRef]
- Pawlowicz, R.; Beardsley, B.; Lentz, S. Classical tidal harmonic analysis including error estimates in MATLAB using T_TIDE. Comput. Geosci. 2002, 28, 929–937. [Google Scholar] [CrossRef]
- Pollard, R.T.; Millard, R. Comparison between observed and simulated wind-generated inertial oscillations. In Deep Sea Research and Oceanographic Abstracts; Elsevier, 1970; Volume 17, pp. 813–821. [Google Scholar]
- Large, W.; Pond, S. Open ocean momentum flux measurements in moderate to strong winds. J. Phys. Oceanogr. 1981, 11, 324–336. [Google Scholar] [CrossRef]
- Gong, Q.; Wang, Q.; Chen, L.; Diao, Y.; Xiong, X.; Sun, J.; Lv, X. Observation of near-inertial waves in the wake of four typhoons in the northern South China Sea. Sci. Rep. 2023, 13, 3147. [Google Scholar]
- Senhui, J.; Zewen, W.; Xiejun, S. Characteristics of Near-inertial Oscillation Influenced by Western Boundary Current of South China Sea. Adv. Earth Sci. 2018, 33, 270. [Google Scholar]
- Chang, S.; Deng, Y.; Zhang, Y.; Zhao, Q.; Wang, R.; Zhang, K. An advanced scheme for range ambiguity suppression of spaceborne SAR based on blind source separation. IEEE Trans. Geosci. Remote Sens. 2022, 60, 1–12. [Google Scholar]
- Oey, L.Y.; Chang, Y.L.; Lin, Y.C.; Chang, M.C.; Varlamov, S.; Miyazawa, Y. Cross flows in the Taiwan Strait in winter. J. Phys. Oceanogr. 2014, 44, 801–817. [Google Scholar] [CrossRef]
- Shen, J.; Qiu, Y.; Guo, X.; Pan, A.; Zhou, X. The spatio-temporal variation of wintertime subtidal currents in the western Taiwan Strait. Acta Oceanol. Sin. 2017, 36, 4–13. [Google Scholar]
- Li, L.; Guo, X.; Liao, E.; Jiang, Y. Subtidal variability in the Taiwan Strait induced by combined forcing of winter monsoon and topography. Sci. China Earth Sci. 2018, 61, 483–493. [Google Scholar]
- Jan, S.; Chern, C.S.; Wang, J.; Chao, S.Y. The anomalous amplification of M2 tide in the Taiwan Strait. Geophys. Res. Lett. 2004, 31. [Google Scholar] [CrossRef]
- Jan, S.; Sheu, D.D.; Kuo, H.M. Water mass and throughflow transport variability in the Taiwan Strait. J. Geophys. Res. Oceans 2006, 111. [Google Scholar] [CrossRef]
- Lin, S.C.; Shih, Y.C.; Chiau, W.Y. An impact analysis of destructive fishing and offshore oil barges on marine living resources in Taiwan Strait. Ocean Coast. Manag. 2013, 80, 119–131. [Google Scholar]
- Shen, Y.T.; Lai, J.W.; Leu, L.G.; Lu, Y.C.; Chen, J.M.; Shao, H.J.; Chen, H.W.; Chang, K.T.; Terng, C.T.; Chang, Y.C.; et al. Applications of ocean currents data from high-frequency radars and current profilers to search and rescue missions around Taiwan. J. Oper. Oceanogr. 2019, 12, S126–S136. [Google Scholar]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Peng, X.; Wang, L.; Wu, X.; Ai, W. Near-Inertial Oscillations Induced by Winter Monsoon Onset in the Southwest Taiwan Strait. Remote Sens. 2024, 16, 4284. https://doi.org/10.3390/rs16224284
Peng X, Wang L, Wu X, Ai W. Near-Inertial Oscillations Induced by Winter Monsoon Onset in the Southwest Taiwan Strait. Remote Sensing. 2024; 16(22):4284. https://doi.org/10.3390/rs16224284
Chicago/Turabian StylePeng, Xiaolin, Li Wang, Xiongbin Wu, and Weihua Ai. 2024. "Near-Inertial Oscillations Induced by Winter Monsoon Onset in the Southwest Taiwan Strait" Remote Sensing 16, no. 22: 4284. https://doi.org/10.3390/rs16224284
APA StylePeng, X., Wang, L., Wu, X., & Ai, W. (2024). Near-Inertial Oscillations Induced by Winter Monsoon Onset in the Southwest Taiwan Strait. Remote Sensing, 16(22), 4284. https://doi.org/10.3390/rs16224284






