Complex Deformation Monitoring over the Linfen–Yuncheng Basin (China) with Time Series InSAR Technology
Abstract
:1. Introduction
2. StaMPS Method
3. Data Collection and Processing
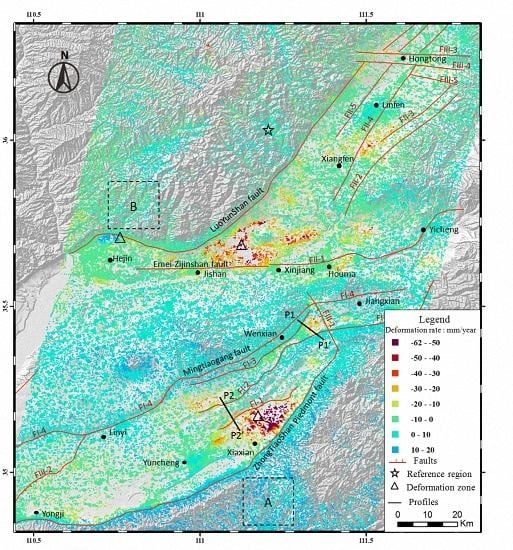
4. Results and Discussion
4.1. Deformation Characteristics Analysis
4.2. Fault Activity Analysis
4.3. Relationship Analysis between Underground Water and Ground Subsidence
5. Hejin Abnormal Deformation Analysis
5.1. Interferogram Analysis
- (1)
- Fringes that persist in time (e.g., Figure 10A–H) show deformation anomalies. These fringes are unlikely to be atmospheric artifacts because the interferograms were produced from independent SAR images. Additionally, the signals cannot be attributed to DEM errors because the baselines of these interferograms are short, making them insensitive to any plausible errors in the DEM.
- (2)
- The deformation patterns are different during the periods spanning 2003–2006 (Figure 10A–D) and 2009–2010 (Figure 10E–H). The color changes from blue–red–yellow–blue along the direction of the arrow during 2003–2006 (Figure 10A–D). However, the color change shows the opposite trend along the direction of the arrow (yellow–red–blue–yellow) during 2009–2010 (Figure 10E–H). Another phenomenon of note is that the deformation center moved to the west during 2009–2010 (Figure 10E–H).
5.2. Deformation and Mechanism Inversion
5.3. Discussion of Abnormal Deformation
6. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Wang, X. Recent crustal deformation field and time-space evolution character in Linfen basin. Earthq. Res. Shanxi 1995, 83, 80–86. [Google Scholar]
- Liu, R.; Ji, L. Present-day crustal deformation of Linfen basin and its boundary faults. J. Geodesy Geodyn. 2014, 34, 28–31. [Google Scholar]
- Liu, R.; Han, S. The current seismic activity characteristics and seismic risk analysis of the Linfen basin, Shanxi, and its periphery. Earthq. Res. Shanxi 2013, 153, 1–3. [Google Scholar]
- Seismological Bureau of Shanxi Province. 2015. Available online: http://www.sxeq.com.cn/n16/index.html (accessed on 30 January 2016).
- Chang’an University. Monitoring Land Subsidence and Ground Fissures over Fenwei Basin by using InSAR and GPS Techniques; Chang’an University: Xi’an, China, 2014. [Google Scholar]
- Shanxi Geological Disaster Environment Monitoring Center. Report on Land Subsidence and Ground Fissure Monitoring within the Shanxi Basin; Geological Disaster Environment Monitoring Center of Shanxi Province: Taiyuan, China, 2013. [Google Scholar]
- Massonnet, D.; Rossi, M.; Carmona, C.; Adragna, F.; Peltzer, G.; Feigl, K.; Rabaute, T. The displacement field of the Landers earthquake mapped by radar interferometry. Nature 1993, 364, 138–142. [Google Scholar] [CrossRef]
- Zebker, H.A.; Rosen, P.A.; Goldstein, R.M.; Gabriel, A.; Werner, C.L. On the derivation of coseismic displacement fields using differential radar interferometry: The Landers earthquake. J. Geogr. Res. 1994, 99, 19617–19634. [Google Scholar]
- Lu, Z.; Patrick, M.; Fielding, E.J.; Trautwein, C. Lava volume from the 1997 eruption of Okmok Volcano, Alaska, estimated from spaceborne and airborne interferometric synthetic aperture radar. IEEE Trans. Geosci. Remote Sens. 2003, 41, 1428–1436. [Google Scholar]
- Zhao, C.Y.; Lu, Z.; Zhang, Q.; de La Fuente, J. Large-area landslides detection and monitoring with ALOS/PALSAR imagery data over Northern California and Southern Oregon, USA. Remote Sens. Environ. 2012, 124, 348–359. [Google Scholar] [CrossRef]
- Yang, C.-S.; Zhang, Q.; Zhao, C.-Y.; Wang, Q.L.; Ji, L.-Y. Monitoring land subsidence and fault deformation using the small baseline subset InSAR technique: A case study in the Datong Basin, China. J. Geodyn. 2014, 75, 34–40. [Google Scholar] [CrossRef]
- Hanssen, R. Radar Interferometry: Data Interpretation and Error Analysis; Springer: New York, NY, USA, 2001. [Google Scholar]
- Lanari, R.; Mora, O.; Manunta, M.; Mallorqu, J.J.; Berardino, P.; Sansosti, E. A small-baseline approach for investigating deformations on full-resolution differential SAR interferograms. IEEE Trans. Geosci. Remote Sens. 2004, 42, 1377–1386. [Google Scholar] [CrossRef]
- Lanari, R.; Casu, F.; Manzo, M.; Zeni, G.; Berardino, P.; Manunta, M.; Pepe, A. An overview of the small BAseline subset algorithm: A DInSAR technique for surface deformation analysis. Pure Appl. Geophys. 2007, 164, 637–661. [Google Scholar] [CrossRef]
- Usai, S. A least squares database approach for SAR interferometry data. IEEE Trans. Geosci. Remote Sens. 2003, 41, 753–760. [Google Scholar] [CrossRef]
- Berardino, P. A new algorithm for surface deformation monitoring based on small baseline differential SAR interferograms. IEEE Trans. Geosci. Remote Sens. 2002, 40, 2375–2383. [Google Scholar] [CrossRef]
- Hooper, A.; Zebker, H.; Segall, P.; Kampes, B. A new method for measuring deformation on volcanoes and other natural terrains using InSAR persistent scatterers. Geophys. Res. Lett. 2004, 31, L23611. [Google Scholar] [CrossRef]
- Hooper, A.; Zebker, H.A. Phase unwrapping in three dimensions with application to InSAR time series. J. Opt. Soc. Am. A: Opt. Image Sci. Vis. 2007, 24, 2737–2747. [Google Scholar] [CrossRef]
- Ferretti, A.; Prati, C.; Rocca, F. Permanent scatterers in SAR interferometry. IEEE Tans. Geosci. Remote Sens. 2001, 39, 8–20. [Google Scholar] [CrossRef]
- Hooper, A. A multi-temporal InSAR method incorporating both peristent scatterer and small baseline approaches. Geophys. Res. Lett. 2008, 35, L16302. [Google Scholar] [CrossRef]
- Hooper, A.; Spaans, K.; Bekaert, D.; Arikan, M. Recent advances in SAR interferometry time series analysis for measuring crustal deformation. Tectonophysics 2012, 514–517, 1–13. [Google Scholar] [CrossRef]
- Hooper, A.; Bekaert, D.; Spaans, K. Stamps/MTI Manual Version 3.3b1; University of Leeds: Leeds, UK, 2013; Available online: http://homepages.see.leeds.ac.uk/~earahoo/stamps/index.html (accessed on 30 January 2016).
- Li, Z.; Fielding, E.; Cross, P.; Muller, J.P. Interferometric synthetic aperture radar atmospheric correction: GPS topography-dependent turbulence model. J. Geophys. Res.: Solid Earth 2006, 111, B02404. [Google Scholar] [CrossRef]
- Doin, M.P.; Lasserre, C.; Peltzer, G.; Cavalie, O.; Doubre, C. Corrections of stratified tropospheric delays in SAR interferometry: Validation with global atmospheric models. J. Appl. Geophys. 2009, 69, 35–50. [Google Scholar] [CrossRef]
- Lin, Y.N.; Simons, M.; Hetland, E.A.; Muse, P.; DiCaprio, C. A multi-scale approach to estimating topographically-correlated propagation delays in radar interferograms. Geochem. Geophys. Geosys. 2010, 11, Q09002. [Google Scholar] [CrossRef]
- Béjar-Pizarro, M.; Socquet, A.; Armijo, R.; Carrizo, D.; Genrich, J.; Simons, M. Interseismic coupling and Andean structure in the north Chile subduction zone. Nat. Geosci. 2013, 6, 462–467. [Google Scholar] [CrossRef]
- Bekaert, D.P.S.; Walters, R.J.; Wright, T.J.; Hooper, A.J.; Parker, D.J. Statistical comparison of InSAR tropospheric correction techniques. Remote Sens. Environ. 2015, 170, 40–47. [Google Scholar] [CrossRef]
- Beauducel, F.; Briole, P.; Froger, J. Volcano-wide fringes in ERS synthetic aperture radar interferograms of Etna (1992–1998): Deformation or tropospheric effect? J. Geophys. Res. 2000, 105, 16391–16402. [Google Scholar] [CrossRef]
- Chaussard, E.; Wdowinski, S.; Cabral-Cano, E.; Amelung, F. Land subsidence in central Mexico detected by ALOS InSAR time-series. Remote Sens. Environ. 2014, 140, 94–106. [Google Scholar] [CrossRef]
- Okada, Y. Surface deformation due to shear and tensile faults in a half-space. Bull. Seismol. Soc. Am. 1985, 75, 1135–1154. [Google Scholar]
- Massonnet, D.; Feigl, K. Radar interferometry and its application to changes in the Earth’s surface. Rev. Geophys. 1998, 36, 441–500. [Google Scholar] [CrossRef]
- Press, W.; Teukolsky, S.; Vetterling, W.; Flannery, B. Numerical Recipes in C, the Art of Scientific Computing; Cambridge Univ. Press: New York, NY, USA, 1992; p. 994. [Google Scholar]












| Parameter | Deflation | Inflation |
|---|---|---|
| Length (km) | 5.2 ± 0.9 | 5.4 ± 0.5 |
| Width (km) | 2.5 ± 0.4 | 1.6 ± 0.2 |
| Depth (km) | 1.5 ± 0.5 | 1.4 ± 0.3 |
| Strike (°) | 104.1 ± 1.4 | 78.3 ± 8.7 |
| X (km) | 7.7 ± 0.3 | 7.0 ± 1.0 |
| Y (km) | 3.7 ± 0.3 | 4.7 ± 0.1 |
| Open (mm) | −34.5 ± 14.5 | 38.6 ± 9.8 |
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons by Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, C.-s.; Zhang, Q.; Xu, Q.; Zhao, C.-y.; Peng, J.-b.; Ji, L.-y. Complex Deformation Monitoring over the Linfen–Yuncheng Basin (China) with Time Series InSAR Technology. Remote Sens. 2016, 8, 284. https://doi.org/10.3390/rs8040284
Yang C-s, Zhang Q, Xu Q, Zhao C-y, Peng J-b, Ji L-y. Complex Deformation Monitoring over the Linfen–Yuncheng Basin (China) with Time Series InSAR Technology. Remote Sensing. 2016; 8(4):284. https://doi.org/10.3390/rs8040284
Chicago/Turabian StyleYang, Cheng-sheng, Qin Zhang, Qiang Xu, Chao-ying Zhao, Jian-bing Peng, and Ling-yun Ji. 2016. "Complex Deformation Monitoring over the Linfen–Yuncheng Basin (China) with Time Series InSAR Technology" Remote Sensing 8, no. 4: 284. https://doi.org/10.3390/rs8040284
APA StyleYang, C.-s., Zhang, Q., Xu, Q., Zhao, C.-y., Peng, J.-b., & Ji, L.-y. (2016). Complex Deformation Monitoring over the Linfen–Yuncheng Basin (China) with Time Series InSAR Technology. Remote Sensing, 8(4), 284. https://doi.org/10.3390/rs8040284