Comparison of Small Baseline Interferometric SAR Processors for Estimating Ground Deformation
Abstract
:1. Introduction and Motivation
2. Theoretical Basis of Small-Baseline Interferometry Approaches
2.1. Small-Baseline Interferogram Selection Criteria and Phase Unwrapping
2.2. Distribued Scatterer Pixel Selection
2.3. Inversion of Interferograms to Individual SAR Scenes
2.4. Mitigation of Non-Deformation Residuals
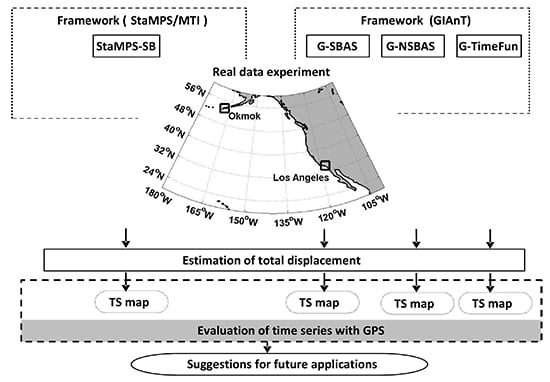
3. Real Data Experiment
3.1. Test Sites and Dataset
3.1.1. Geodetic Setting of Study Areas and SAR Imagery
3.1.2. GPS Data at Test Sites
3.2. Small Baseline Interferograms Selection
3.3. DS Point Selection and Coverage Evaluation
3.4. Estimation of Surface Deformation
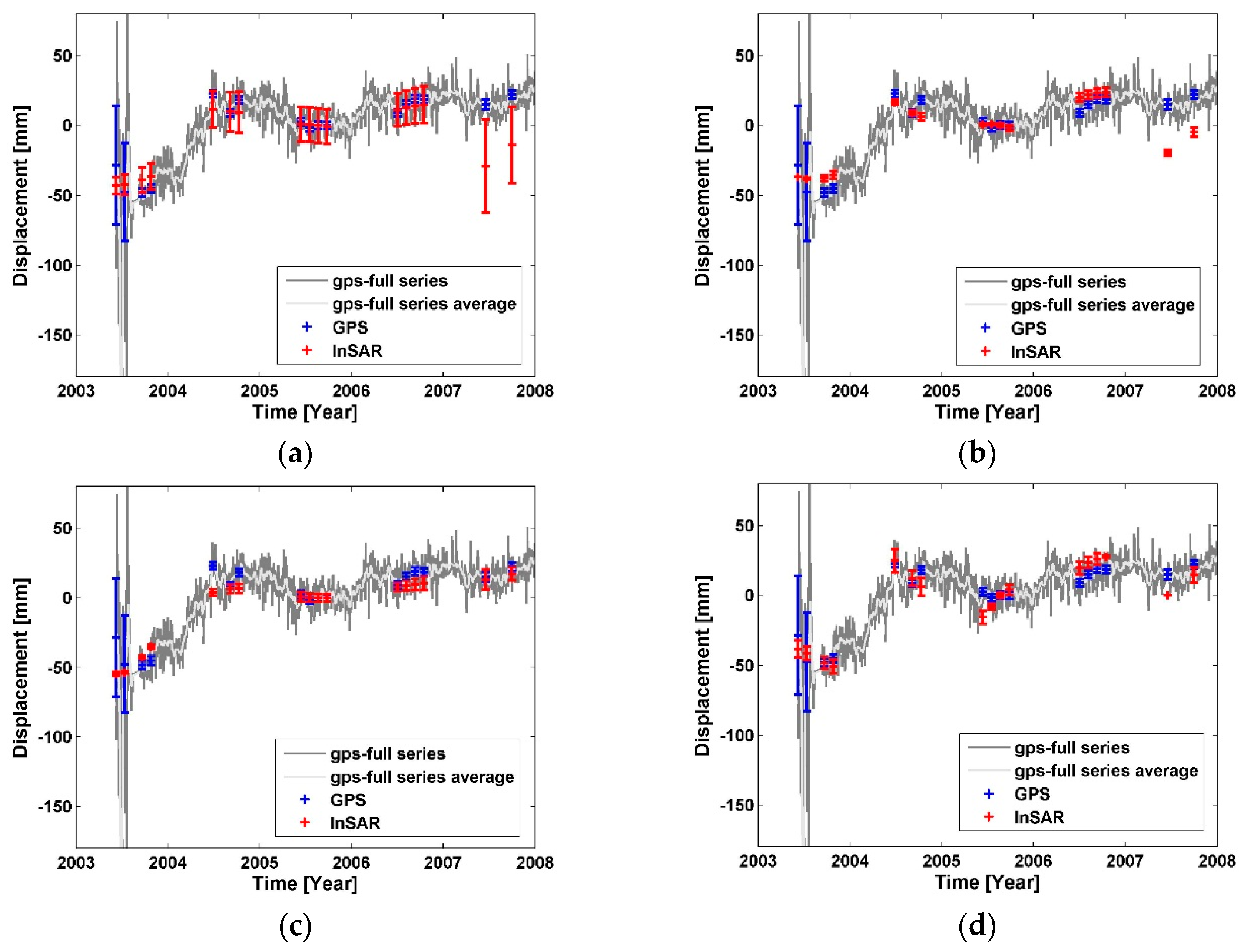
3.4.1. Comparison of Small Baseline InSAR and GPS Measurements for the LA Site
3.4.2. Comparison of Small Baseline InSAR and GPS Measurements for the Okmok Site
4. Discussion
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Costantini, M.; Falco, S.; Malvarosa, F.; Minati, F. A New Method for Identification and Analysis of Persistent Scatterers in Series of SAR Images. In Proceedings of the Geoscience and Remote Sensing Symposium, Boston, MA, USA, 7–11 July 2008; IEEE International: Boston, MA, USA, 2008; pp. II-449–II-452. [Google Scholar]
- Ferretti, A.; Prati, C.; Rocca, F. Permanent scatterers in SAR interferometry. IEEE Trans. Geosci. Remote Sens. 2001, 39, 8–20. [Google Scholar] [CrossRef]
- Hooper, A.; Segall, P.; Zebker, H. Persistent scatterer interferometric synthetic aperture radar for crustal deformation analysis, with application to Volcán Alcedo, Galápagos. J. Geophys. Res. 2007. [Google Scholar] [CrossRef]
- Kampes, B. Radar Interferometry: Persistent Scatterer Technique; Springer: Dordrecht, The Netherlands, 2006; Volume 12. [Google Scholar]
- Werner, C.; Wegmuller, U.; Strozzi, T.; Wiesmann, A. Interferometric Point Target Analysis for Deformation Mapping. In Proceedings of the Geoscience and Remote Sensing Symposium, Toulouse, France, 21–25 July 2003; IEEE International: Toulouse, France, 2003; Volume 7, pp. 4362–4364. [Google Scholar]
- Berardino, P.; Fornaro, G.; Lanari, R.; Sansosti, E. A new algorithm for surface deformation monitoring based on small baseline differential SAR interferograms. IEEE Trans. Geosci. Remote Sens. 2002, 40, 2375–2383. [Google Scholar] [CrossRef]
- Doin, M.P.; Guillaso, S.; Jolivet, R.; Lasserre, C.; Lodge, F.; Ducret, G. Presentation of the small baseline NSBAS processing chain on a case example: The Etna deformation monitoring from 2003 to 2010 using Envisat data. In Proceedings of the ESA FRINGE 2011 Conference, Frascati, Italy, 19–23 September 2011.
- Hetland, E.A.; Muse, P.; Simons, M.; Lin, Y.N.; Agram, P.S.; Di Caprio, C.J. Multiscale InSAR Time Series (MInTS) analysis of surface deformation. J. Geophys. Res. Solid Earth 2012. [Google Scholar] [CrossRef]
- Lanari, R.; Mora, O.; Manunta, M.; Mallorqui, J.J.; Berardino, P.; Sansosti, E. A small-baseline approach for investigating deformations on full-resolution differential SAR interferograms. IEEE Trans. Geosci. Remote Sens. 2004. [Google Scholar] [CrossRef]
- Mora, O.; Mallorqui, J.J.; Broquetas, A. Linear and nonlinear terrain deformation maps from a reduced set of interferometric SAR images. IEEE Trans. Geosci. Remote Sens. 2003, 41, 2243–2253. [Google Scholar] [CrossRef]
- Ferretti, A.; Fumagalli, A.; Novali, F.; Prati, C.; Rocca, F.; Rucci, A. A New Algorithm for Processing Interferometric Data-Stacks: SqueeSAR. IEEE Trans. Geosci. Remote Sens. 2011, 49, 3460–3470. [Google Scholar] [CrossRef]
- Gong, W.; Meyer, F. Persistent Scatterer Coherence Analysis over the Valley of Ten Thousand Smokes, Katmai. In Proceedings of the American Geophysical Union Fall Meeting 2011, San Francisco, CA, USA, 5–9 December 2011.
- Perissin, D.; Ferretti, A. Urban-target recognition by means of repeated spaceborne SAR images. IEEE Trans. Geosci. Remote Sens. 2007, 45, 4043–4058. [Google Scholar] [CrossRef]
- Riddick, S.N.; Schmidt, D.A.; Deligne, N.I. An analysis of terrain properties and the location of surface scatterers from persistent scatterer interferometry. ISPRS J. Photogr. Remote Sens. 2012, 73, 50–57. [Google Scholar] [CrossRef]
- Osmanoğlu, B.; Sunar, F.; Wdowinski, S.; Cabral-Cano, E. Time series analysis of InSAR data: Methods and trends. ISPRS J. Photogr. Remote Sens. 2015. [Google Scholar] [CrossRef]
- Hanssen, R. Radar Interferometry: Data Interpretation and Error Analysis, 1st ed.; Kluwer Academic Publishers: Amsterdam, The Netherlands, 2001; Volume 2. [Google Scholar]
- Lanari, R.; Casu, F.; Manzo, M.; Zeni, G.; Berardino, P.; Manunta, M.; Pepe, A. An Overview of the Small BAseline Subset Algorithm: A DInSAR Technique for Surface Deformation Analysis. In Deformation and Gravity Change: Indicators of Isostasy, Tectonics, Volcanism, and Climate Change; Wolf, D., Fernández, J., Eds.; Birkhäuser: Basel, Switzerland, 2007; pp. 637–661. [Google Scholar]
- Hooper, A.; Bekaert, D.; Spaans, K.; Arıkan, M. Recent advances in SAR interferometry time series analysis for measuring crustal deformation. Tectonophysics 2012, 514–517, 1–13. [Google Scholar] [CrossRef]
- Crosetto, M.; Monserrat, O.; Cuevas-González, M.; Devanthéry, N.; Crippa, B. Persistent Scatterer Interferometry: A review. ISPRS J. Photogr. Remote Sens. 2015. [Google Scholar] [CrossRef]
- Iglesias, R.; Monells, D.; Lopez-Martinez, C.; Mallorqui, J.J.; Fabregas, X.; Aguasca, A. Polarimetric Optimization of Temporal Sublook Coherence for DInSAR Applications. IEEE Geosci. Remote Sens. Lett. 2015, 12, 87–91. [Google Scholar] [CrossRef]
- Usai, S. A least squares database approach for SAR interferometric data. IEEE Trans. Geosci. Remote Sens. 2003, 41, 753–760. [Google Scholar] [CrossRef]
- López-Quiroz, P.; Doin, M.-P.; Tupin, F.; Briole, P.; Nicolas, J.-M. Time series analysis of Mexico City subsidence constrained by radar interferometry. J. Appl. Geophys. 2009, 69, 1–15. [Google Scholar] [CrossRef]
- Schmidt, D.A.; Burgmann, R. Time-dependent land uplift and subsidence in the Santa Clara valley, California, from a large interferometric synthetic aperture radar data set. J. Geophys. Res. Solid Earth 2003, 108, Article Number 2416. [Google Scholar] [CrossRef]
- Hooper, A. A multi-temporal InSAR method incorporating both persistent scatterer and small baseline approaches. Geophys. Res. Lett. 2008, 35, Article Number L16302. [Google Scholar] [CrossRef]
- Jolivet, R.; Lasserre, C.; Doin, M.P.; Guillaso, S.; Peltzer, G.; Dailu, R.; Sun, J.; Shen, Z.-K.; Xu, X. Shallow creep on the Haiyuan Fault (Gansu, China) revealed by SAR Interferometry. J. Geophys. Res. Solid Earth 2012. [Google Scholar] [CrossRef]
- Lee, C.W.; Lu, Z.; Jung, H.S.; Won, J.S.; Dzurisin, D. Surface deformation of Augustine Volcano, 1992–2005, from multiple-interferogram processing using a refined small baseline subset (SBAS) interferometric synthetic aperture radar (InSAR) approach. In The 2006 Eruption of Augustine Volcano, Alaska: U.S. Geological Survey Professional Paper; Power, J.A., Coombs, M.L., Freymueller, J.T., Eds.; USGS: Reston, VA, USA; Volume 1769, Chapter 17. Available online: http://pubs.usgs.gov/pp/1769/chapters/p1769_chapter17.pdf (accessed on 1 December 2014).
- Lanari, R.; Lundgren, P.; Manzo, M.; Casu, F. Satellite radar interferometry time series analysis of surface deformation for Los Angeles, California. Geophys. Res. Lett. 2004, 31, Article Number L23613. [Google Scholar] [CrossRef]
- Biggs, J.; Wright, T.; Lu, Z.; Parsons, B. Multi-interferogram method for measuring interseismic deformation: Denali fault, Alaska. Geophys. J. Int. 2007, 170, 1165–1179. [Google Scholar] [CrossRef]
- Agram, P.S.; Jolivet, R.; Riel, B.; Lin, Y.N.; Simons, M.; Hetland, E.; Doin, M.-P.; Lasserrre, C. New Radar Interferometric Time Series Analysis Toolbox Released. Eos Trans. Am. Geophys. Union 2013, 94, 69–70. [Google Scholar] [CrossRef]
- Agram, P.; Jolivet, R.; Simons, M. Generic InSAR Analysis Toolbox (GIAnT), User Guide, ed.; Available online: http://earthdef.caltech.edu (accessed on 31 March 2016).
- Sousa, J.J.; Hooper, A.J.; Hanssen, R.F.; Bastos, L.C.; Ruiz, A.M. Persistent Scatterer InSAR: A comparison of methodologies based on a model of temporal deformation vs. spatial correlation selection criteria. Remote Sens. Environ. 2011, 115, 2652–2663. [Google Scholar] [CrossRef]
- Agram, S.P.; Casu, F.; Zebker, H.A.; Lanari, R. Comparison of Persistent Scatterers and Small Baseline Time-Series InSAR Results: A Case Study of the San Francisco Bay Area. IEEE Geosci. Remote Sens. Lett. 2011, 8, 592–596. [Google Scholar] [CrossRef]
- Lauknes, T.R.; Dehls, J.; Larsen, Y.; Høgda, K.A.; Weydahl, D.J. A Comparison of SBAS and PS ERS InSAR for Subsidence Monitoring in Oslo, Norway. In Fringe Workshop; European Space Agency: Frascati, Italy, 2005; p. 58. [Google Scholar]
- Bamler, R.; Hartl, P. Synthetic aperture radar interferometry. Inverse Probl. 1998, 14, 54. [Google Scholar] [CrossRef]
- Rosen, P.A.; Hensley, S.; Joughin, I.R.; Li, F.K.; Madsen, S.N.; Rodriguez, E.; Goldstein, R.M. Synthetic aperture radar interferometry—Invited paper. Proc. IEEE 2000, 88, 333–382. [Google Scholar] [CrossRef]
- Chen, C.W.; Zebker, H.A. Network approaches to two-dimensional phase unwrapping: Intractability and two new algorithms (vol. 17, p 401, 2000). J. Opt. Soc. Am. Optics Image Sci. Vis. 2001, 18, 1192. [Google Scholar] [CrossRef]
- Goldstein, R.M.; Zebker, H.A.; Werner, C.L. Satellite Radar Interferometry—Two-Dimensional Phase Unwrapping. Radio Sci. 1988, 23, 713–720. [Google Scholar] [CrossRef]
- Costantini, M. A novel phase unwrapping method based on network programming. IEEE Trans. Geosc. Remote Sens. 1998, 36, 813–821. [Google Scholar] [CrossRef]
- Pepe, A.; Lanari, R. On the extension of the minimum cost flow algorithm for phase unwrapping of multitemporal differential SAR interferograms. IEEE Trans. Geosc. Remote Sens. 2006, 44, 2374–2383. [Google Scholar] [CrossRef]
- Hooper, A.; Zebker, H.A. Phase unwrapping in three dimensions with application to InSAR time series. J. Opt. Soc. Am. Optics Image Sci. Vis. 2007, 24, 2737–2747. [Google Scholar] [CrossRef]
- Shanker, A.P.; Zebker, H. Edgelist phase unwrapping algorithm for time series InSAR analysis. J. Opt. Soc. Am. Optics Image Sci. Vis. 2010, 27, 605–612. [Google Scholar] [CrossRef] [PubMed]
- Parizzi, A.; Brcic, R. Adaptive InSAR Stack Multilooking Exploiting Amplitude Statistics: A Comparison Between Different Techniques and Practical Results. IEEE Geosci. Remote Sens. Lett. 2011, 8, 441–445. [Google Scholar] [CrossRef]
- Goel, K.; Adam, N. Fusion of Monostatic/Bistatic InSAR Stacks for Urban Area Analysis via Distributed Scatterers. IEEE Geosci. Remote Sens. Lett. 2014, 11, 733–737. [Google Scholar] [CrossRef]
- Ferretti, A.; Fumagalli, A.; Novali, F.; Prati, C.; Roca, R.; Rucci, A. Exploitation of distributed scatterers in interferometric data stacks. In Geoscience Remote Sensing Symposium; IEEE International: Cape Town, South Africa, 2009. [Google Scholar]
- Ferretti, A.; Fumagalli, A.; Novali, F.; Prati, C.; Roca, R.; Rucci, A. The second generation PSInSAR approach: SqueeSAR. In Workshop ERS SAR Interferometry (FRINGE); European Space Agency: Frascati, Italy, 2009. [Google Scholar]
- Fry, J.; Xian, G.; Jin, S.; Dewitz, J.; Homer, C.; Yang, L.; Barnes, C.; Herold, N.; Wickham, J. Completion of the 2006 National Land Cover Database for the Conterminous United States. Photogramm. Eng. Remote Sens. 2011, 77, 858–864. [Google Scholar]
- Fournier, T.; Freymueller, J.; Cervelli, P. Tracking magma volume recovery at Okmok volcano using GPS and an unscented Kalman filter. J. Geophys. Res. Solid Earth 2009, 114, Article Number B02405. [Google Scholar] [CrossRef]
- Watson, K.M.; Bock, Y.; Sandwell, D.T. Satellite interferometric observations of displacements associated with seasonal groundwater in the Los Angeles basin. J. Geophys. Res. Solid Earth 2002, 107, Article Number 2074. [Google Scholar] [CrossRef]
- UNAVCO. (2013). GNSS Data Archive Interface Version 2 (DAI v2). Available online: http://facility.unavco.org/data/dai2/app/dai2.html (accessed on 31 March 2016).
- Google. Google Earth Version 6 ed. Available online: http://www.google.com/earth/download/ (accessed on 31 March 2016).
- Bawden, G.W.; Thatcher, W.; Stein, R.S.; Hudnut, K.W.; Peltzer, G. Tectonic contraction across Los Angeles after removal of groundwater pumping effects. Nature 2001, 412, 812–815. [Google Scholar] [CrossRef] [PubMed]
- Brooks, B.A.; Merrifield, M.A.; Foster, J.; Werner, C.L.; Gomez, F.; Bevis, M.; Gill, F. Space geodetic determination of spatial variability in relative sea level change, Los Angeles basin. Geophys. Res. Lett. 2007, 34. [Google Scholar] [CrossRef]
- Zhang, L.; Lu, Z.; Ding, X.; Jung, H.-S.; Feng, G.; Lee, C.-W. Mapping ground surface deformation using temporarily coherent point SAR interferometry: Application to Los Angeles Basin. Remote Sens. Environ. 2012, 117, 429–439. [Google Scholar] [CrossRef]
- Qu, F.; Lu, Z.; Poland, M.; Freymueller, J.; Zhang, Q.; Jung, H.-S. Post-Eruptive Inflation of Okmok Volcano, Alaska, from InSAR, 2008–2014. Remote Sens. 2015, 7, 16778–16794. [Google Scholar] [CrossRef]
- Lu, Z.; Dzurisin, D.; Biggs, J.; Wicks, C.; McNutt, S. Ground surface deformation patterns, magma supply, and magma storage at Okmok volcano, Alaska, from InSAR analysis: 1. Intereruption deformation, 1997–2008. J. Geophys. Res. Solid Earth 2010. [Google Scholar] [CrossRef]
- Lu, Z.; Dzurisin, D. Ground surface deformation patterns, magma supply, and magma storage at Okmok volcano, Alaska, from InSAR analysis: 2. Coeruptive deflation, July–August 2008. J. Geophys. Res. Solid Earth 2010, 115. [Google Scholar] [CrossRef]
- Lu, Z.; Masterlark, T.; Dzurisin, D. Interferometric synthetic aperture radar study of Okmok volcano, Alaska, 1992–2003: Magma supply dynamics and postemplacement lava flow deformation. J. Geophys. Res. Solid Earth 2005, 110, Article Number B02403. [Google Scholar] [CrossRef]
- Freymueller, J.; Kaufman, A.M. Changes in the magma system during the 2008 eruption of Okmok volcano, Alaska, based on GPS measurements. J. Geophys. Res. Solid Earth 2010, 115, Article Number B12415. [Google Scholar] [CrossRef]
- Lu, Z.; Freymueller, J.T. Synthetic aperture radar interferometry coherence analysis over Katmai volcano group, Alaska. J. Geophys. Res. 1998, 103, 29887–29894. [Google Scholar] [CrossRef]
- Gong, W.; Meyer, F.J.; Liu, S.; Hanssen, R.F. Temporal Filtering of InSAR Data Using Statistical Parameters From NWP Models. IEEE Trans. Geosci. Remote Sens. 2015, 53, 4033–4044. [Google Scholar] [CrossRef]
- Bock, Y.; Nikolaidis, R.M.; de Jonge, P.J.; Bevis, M. Instantaneous geodetic positioning at medium distances with the Global Positioning System. J. Geophys. Res. Solid Earth 2000, 105, 28223–28253. [Google Scholar] [CrossRef]
- Van Zyl, J.J. The Shuttle Radar Topography Mission (SRTM): A breakthrough in remote sensing of topography. Acta Astronaut. 2001, 48, 559–565. [Google Scholar] [CrossRef]
- Rodriguez, E.; Martin, J.M. Theory and design of interferometric synthetic aperture radars. IEE Proc. Rad. Signal Proc. 1992, 139, 147–159. [Google Scholar] [CrossRef]
- Falge, R.; Thiele, A.; Wenyu, G.; Hinz, S.; Meyer, F.J. Analyzing the spatial distribution of coherent points in SAR Interferograms. In Proceedings of the IEEE International Geoscience and Remote Sensing Symposium, Quebec City, QC, Canada, 13–18 July 2014; IEEE International: Quebec, QC, Canada, 2014. [Google Scholar]
- Casu, F.; Manzo, M.; Lanari, R. A quantitative assessment of the SBAS algorithm performance for surface deformation retrieval from DInSAR data. Remote Sens. Environ. 2006, 102, 195–210. [Google Scholar] [CrossRef]
- Lu, Z.; Dzurisin, D. InSAR Imaging of Aleutian Volcanoes: Monitoring a Volcanic Arc from Space; Springer: Berlin, Germany, 2014. [Google Scholar]











| Feature | Los Angeles (CA, USA) | Okmok (AK, USA) |
|---|---|---|
| Climate zone | subtropical zone | sub-arctic zone |
| Land cover | urban/forest/mountain | volcanic sediment/snow/scrubs |
| Deformation | periodical plus linear trend | none linear |
| SAR satellite | ERS-1/2 | Envisat |
| Acquisition time | 1995–2000 | 2003–2008 |
| NO. of SLCs. | 48 | 20 |
| NO. of total IFGs.1 | 123 | 35 |
| Coherence threshold (G-SBAS/Timefun) | 0.1 | 0.1 |
| Coherence threshold (G-NSBAS) | 0.2 | 0.2 |
| NO. of valid IFGs 1 | 105 | 30 |
| γ | 1 × 10−4 | 1 |
| Gaussian filter length (year) | 0.1 | 0.2 |
| Site Name | SBAS Method | Point Density (DS/km2) | Coverage Ratio (km2) 1 | σ Triangle Size (km2) 2 | Short Arc. Median (km) |
|---|---|---|---|---|---|
| OK Ice/Snow and scrub | G-NSABS | 53.50 | 0.73 | 97.02 | 0.09 |
| G-SBAS G-TimeFun | 34.01 | 0.71 | 112.47 | 0.10 | |
| StaMPS-SB 3 | 12.07 | 0.72 | 226.47 | 0.16 | |
| LA-1 Forest and Scrub | G-NSABS | 15.63 | 0.61 | 86.80 | 0.09 |
| G-SBAS G-TimeFun | 0.55 | 0.43 | 1602.80 | 0.39 | |
| StaMPS-SB 3 | 19.68 | 0.95 | 58.67 | 0.17 | |
| LA-2 Developed, low to medium intensity and open space | G-NSABS | 162.26 | 0.99 | 0.76 | 0.08 |
| G-SBAS G-TimeFun | 134.54 | 0.99 | 4.69 | 0.08 | |
| StaMPS-SB 3 | 89.13 | 0.99 | 3.43 | 0.11 | |
| LA-3 | G-NSABS | 163.63 | 1.00 | 2.07 | 0.08 |
| Developed, medium to high intensity | G-SBAS G-TimeFun | 119.69 | 0.99 | 7.39 | 0.08 |
| StaMPS-SB 3 | 91.72 | 1.00 | 3.08 | 0.11 |
| Arc 1 | Method | Mean Distance 2 (m) | Residual 3 (mm) | |||
|---|---|---|---|---|---|---|
| Point 1 | Point 2 | Point 1 | Point 2 | Offset | σ | |
| LEEP | HOLP | G-NSBAS | 572.72 | 32.95 | −15.42 | 14.95 |
| G-SBAS | 758.32 | 71.46 | −10.57 | 15.27 | ||
| G-TimeFun | 758.32 | 71.46 | −8.09 | 8.96 | ||
| StaMPS-SB | 144.00 | 70.68 | 8.74 | 8.81 | ||
| WHC1 | LEEP | G-NSBAS | 42.27 | 572.72 | 0.86 | 7.24 |
| G-SBAS | 42.27 | 758.32 | −1.27 | 8.41 | ||
| G-TimeFun | 42.27 | 758.32 | −1.21 | 7.44 | ||
| StaMPS-SB | 59.07 | 144.00 | −2.78 | 8.46 | ||
| LBC2 | LBC1 | G-NSBAS | 49.33 | 31.88 | 1.40 | 8.53 |
| G-SBAS | 49.33 | 31.88 | 2.82 | 9.42 | ||
| G-TimeFun | 49.33 | 31.88 | −1.40 | 4.35 | ||
| StaMPS-SB | 59.40 | 58.51 | −2.43 | 9.17 | ||
| Arc | Method | Mean Distance [m] | Residual [mm] | |||
|---|---|---|---|---|---|---|
| Point 1 | Point 2 | Point 1 | Point 2 | Offset | σ | |
| OKCE | OKFG | G-NSBAS | 196.75 | 145.68 | 5.92 | 14.47 |
| G-SBAS | 196.72 | 224.00 | 1.91 | 12.92 | ||
| G-TimeFun | 196.72 | 224.00 | 4.77 | 8.57 | ||
| StaMPS-SB | 168.35 | 453.61 | 1.37 | 9.00 | ||
| OKCD | OKSO | G-NSBAS | 58.41 | 72.84 | 0.77 | 13.20 |
| G-SBAS | 88.88 | 72.84 | 0.96 | 12.32 | ||
| G-TimeFun | 88.88 | 72.84 | 0.04 | 11.42 | ||
| StaMPS-SB | 112.32 | 123.57 | 7.18 | 15.68 | ||
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons by Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gong, W.; Thiele, A.; Hinz, S.; Meyer, F.J.; Hooper, A.; Agram, P.S. Comparison of Small Baseline Interferometric SAR Processors for Estimating Ground Deformation. Remote Sens. 2016, 8, 330. https://doi.org/10.3390/rs8040330
Gong W, Thiele A, Hinz S, Meyer FJ, Hooper A, Agram PS. Comparison of Small Baseline Interferometric SAR Processors for Estimating Ground Deformation. Remote Sensing. 2016; 8(4):330. https://doi.org/10.3390/rs8040330
Chicago/Turabian StyleGong, Wenyu, Antje Thiele, Stefan Hinz, Franz J. Meyer, Andrew Hooper, and Piyush S. Agram. 2016. "Comparison of Small Baseline Interferometric SAR Processors for Estimating Ground Deformation" Remote Sensing 8, no. 4: 330. https://doi.org/10.3390/rs8040330
APA StyleGong, W., Thiele, A., Hinz, S., Meyer, F. J., Hooper, A., & Agram, P. S. (2016). Comparison of Small Baseline Interferometric SAR Processors for Estimating Ground Deformation. Remote Sensing, 8(4), 330. https://doi.org/10.3390/rs8040330