Multi-Physics Fields Based Nonlinear Dynamic Behavior Analysis of Air Bearing Motorized Spindle
Abstract
1. Introduction
2. Mathematical Modeling
2.1. Modeling for Restoring Force of Air Journal Bearing
2.2. Modeling for UMF of PMSM
2.3. Modeling for Rotor Dynamic System
3. Numeric Simulation of the Mathematical Model
3.1. Simulation of the PMSM Model
3.2. Simulation of Nonlinear Dynamic Behavior of the ABMS
4. Discussion
5. Conclusions
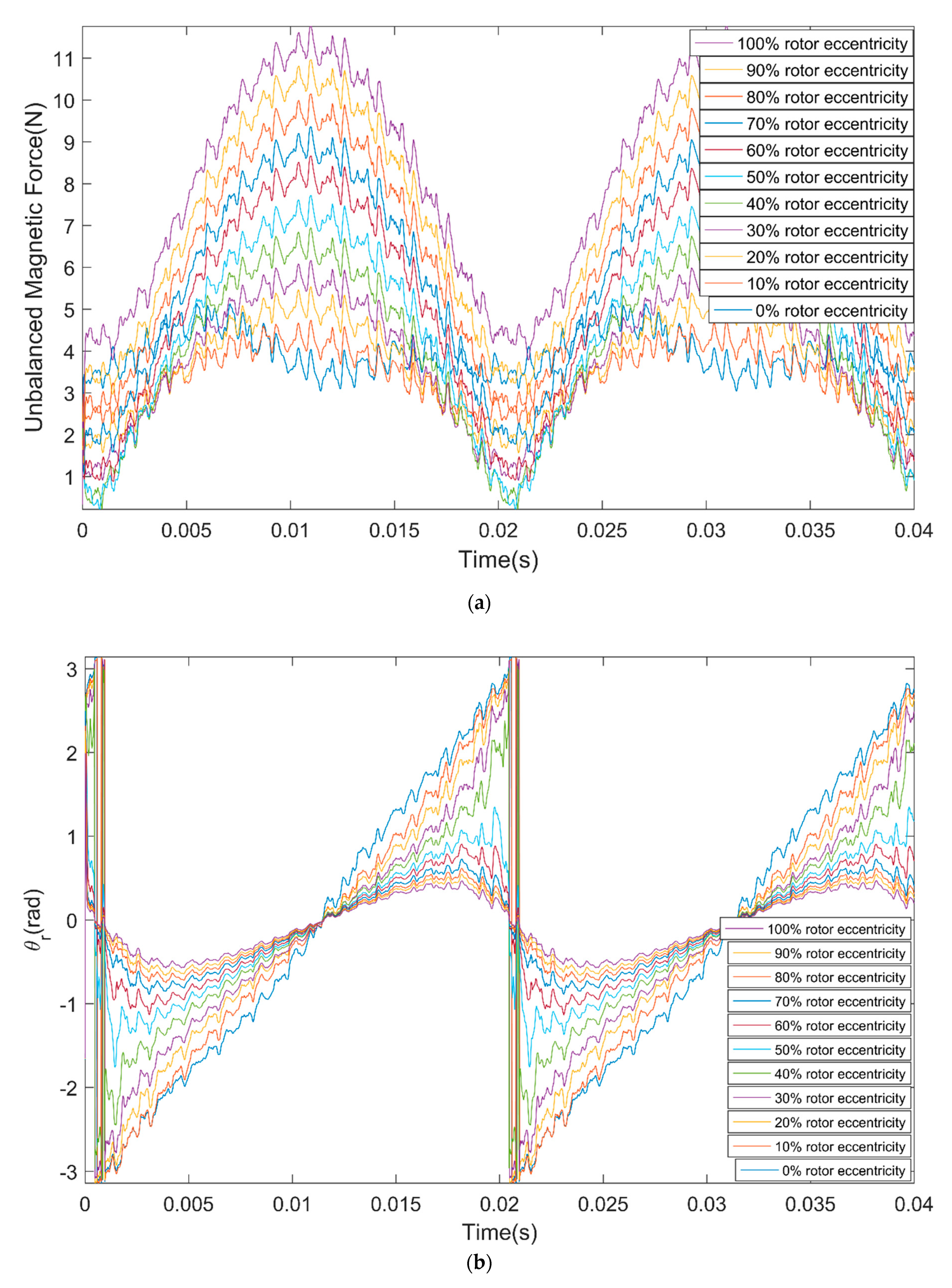
- When the PMSM is at small rotor eccentricity status, the unbalanced magnetic shows quadratic variation trend with rotor eccentricity while it changes linearly at large rotor eccentricity status;
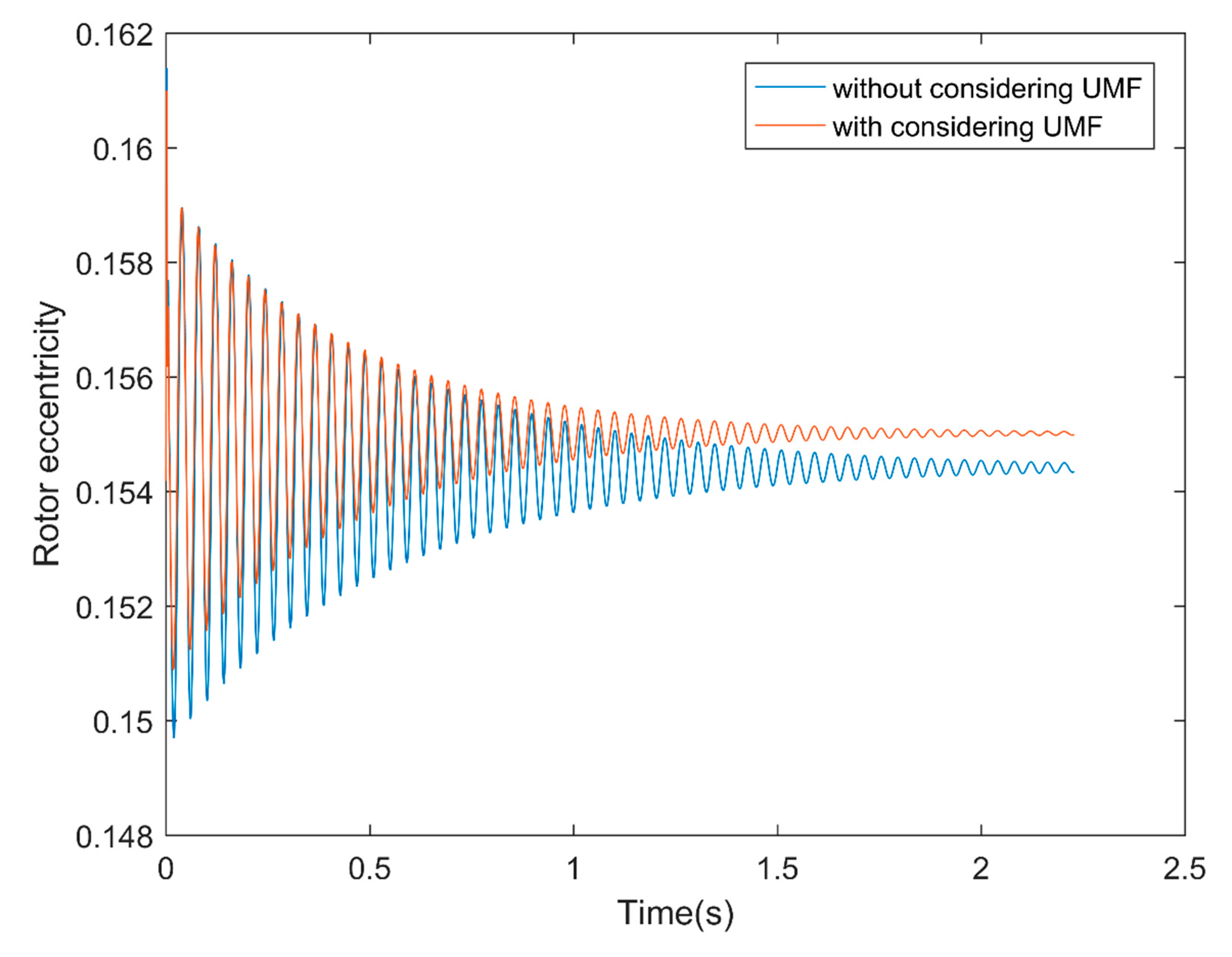
- The existence of the UMF can slightly change the final convergent position of the rotor center and it can accelerate the convergence of the rotor center to some extent;
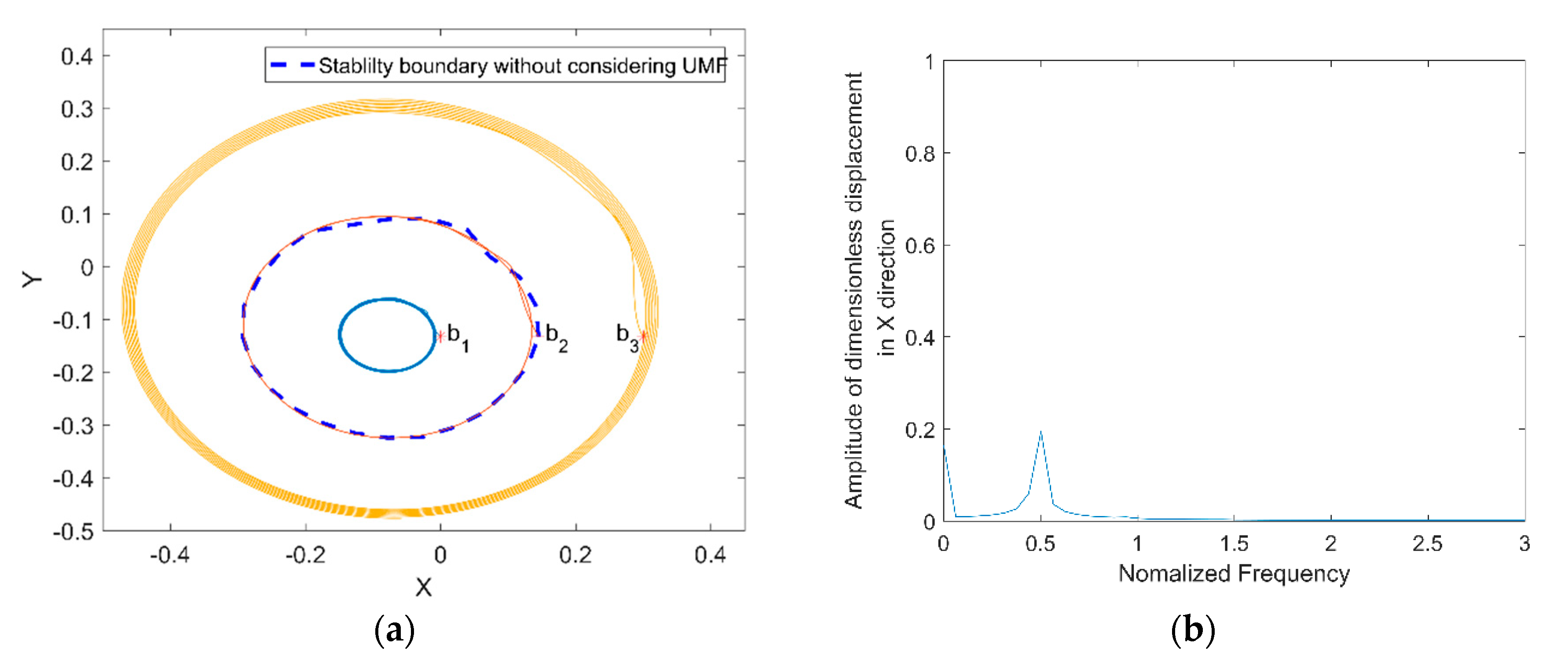
- The UMF can change the bifurcation threshold of rotor mass at a certain external load. The other form of its manifestation is that the UMF can enlarge the stability boundary at certain rotor mass and external load.
Author Contributions
Funding
Conflicts of Interest
References
- Nguyen, Q.-D.; Ueno, S. Modeling and Control of Salient-Pole Permanent Magnet Axial-Gap Self-Bearing Motor. IEEE/ASME Trans. Mechatronics 2010, 16, 518–526. [Google Scholar] [CrossRef]
- Gao, Q.; Lu, L.; Chen, W.; Wang, G. Influence of air-induced vibration of aerostatic bearing on the machined surface quality in ultra-precision flycutting. Proc. Inst. Mech. Eng. Part J. J. Eng. Tribol. 2017, 232, 117–125. [Google Scholar] [CrossRef]
- Jiang, S.; Xu, C. Dynamics characteristics of a rotary table motorized spindle with externally pressurized air bearings. J. Vibroengineering 2017, 19, 801–811. [Google Scholar] [CrossRef][Green Version]
- Gao, S.; Cheng, K.; Ding, H.; Hongya, F. Multiphysics-based design and analysis of the high-speed aerostatic spindle with application to micro-milling. Proc. Inst. Mech. Eng. Part J. J. Eng. Tribol. 2015, 230, 852–871. [Google Scholar] [CrossRef]
- Gao, Q.; Chen, W.; Lu, L.; Huo, D.; Cheng, K. Aerostatic bearings design and analysis with the application to precision engineering: State-of-the-art and future perspectives. Tribol. Int. 2019, 135, 1–17. [Google Scholar] [CrossRef]
- Chen, G.; Ju, B.; Fang, H.; Chen, Y.; Yu, N.; Wan, Y. Air bearing: Academic insights and trend analysis. Int. J. Adv. Manuf. Technol. 2019, 106, 1191–1202. [Google Scholar] [CrossRef]
- Yu, M.; Lv, P.; Xu, T.; Tan, X.; Li, H. Manufacturing of a Compact Micro Air Bearing Device for Power Micro Electro Mechanical System (MEMS) Applications Using Silica Film Assisted Processing. Micromachines 2018, 9, 166. [Google Scholar] [CrossRef]
- Zhang, S.; To, S.; Cheung, C.F.; Wang, H. Dynamic characteristics of an aerostatic bearing spindle and its influence on surface topography in ultra-precision diamond turning. Int. J. Mach. Tools Manuf. 2012, 62, 1–12. [Google Scholar] [CrossRef]
- Li, J.; Liu, P. Dynamic analysis of 5-DOFs aerostatic spindles considering tilting motion with varying stiffness and damping of thrust bearings. J. Mech. Sci. Technol. 2019, 33, 5199–5207. [Google Scholar] [CrossRef]
- Zhang, G.-H.; Sun, Y.; Liu, Z.-S.; Zhang, M.; Yan, J.-J. Dynamic characteristics of self-acting gas bearing–flexible rotor coupling system based on the forecasting orbit method. Nonlinear Dyn. 2011, 69, 341–355. [Google Scholar] [CrossRef]
- Chen, D.; Li, N.; Pan, R.; Han, J. Analysis of aerostatic spindle radial vibration error based on microscale nonlinear dynamic characteristics. J. Vib. Control. 2019, 25, 2043–2052. [Google Scholar] [CrossRef]
- Zhao, X.-L.; Dong, H.; Fang, Z.; Chen, D.-D.; Zhang, J.-A. Study on Dynamic Characteristics of Aerostatic Bearing with Elastic Equalizing Pressure Groove. Shock. Vib. 2018, 2018, 1–12. [Google Scholar] [CrossRef]
- Yang, D.-W.; Chen, C.-H.; Kang, Y.; Hwang, R.-M.; Shyr, S.-S. Influence of orifices on stability of rotor-aerostatic bearing system. Tribol. Int. 2009, 42, 1206–1219. [Google Scholar] [CrossRef]
- Hassini, M.A.; Arghir, M. A Simplified Nonlinear Transient Analysis Method for Gas Bearings. J. Tribol. 2012, 134, 011704. [Google Scholar] [CrossRef]
- Shiau, T.N.; Hsu, W.C.; Deng, B.W. Nonlinear Dynamic Analysis of a Rotor System with Aerodynamic Journal Bearings. In Proceedings of the Volume 6: Turbo Expo 2007, Parts A and B; ASME International: New York, NY, USA, 2007; pp. 913–921. [Google Scholar]
- Xu, C.; Jiang, S. Dynamic Analysis of a Motorized Spindle With Externally Pressurized Air Bearings. J. Vib. Acoust. 2015, 137, 041001. [Google Scholar] [CrossRef]
- Chen, C.-H.; Tsai, T.-H.; Yang, D.-W.; Kang, Y.; Chang, Y.-P. Inherent restriction on stability of rotor-aerostatic bearing system. Ind. Lubr. Tribol. 2011, 63, 277–292. [Google Scholar] [CrossRef]
- Lihua, Y.; Shemiao, Q.; Yu, L. Numerical Analysis on Dynamic Coefficients of Self-Acting Gas-Lubricated Tilting-Pad Journal Bearings. J. Tribol. 2007, 130, 011006. [Google Scholar] [CrossRef]
- Yang, P.; Zhu, K.-Q.; Wang, X.-L. On the non-linear stability of self-acting gas journal bearings. Tribol. Int. 2009, 42, 71–76. [Google Scholar] [CrossRef]
- Nagaraju, T.; Sharma, S.C.; Jain, S. Study of orifice compensated hole-entry hybrid journal bearing considering surface roughness and flexibility effects. Tribol. Int. 2006, 39, 715–725. [Google Scholar] [CrossRef]
- Du, J.; Yang, G.; Ge, W.; Liu, T. Nonlinear Dynamic Analysis of a Rigid Rotor Supported By a Spiral-Grooved Opposed-Hemisphere Gas Bearing. Tribol. Trans. 2016, 59, 781–800. [Google Scholar] [CrossRef]
- Wang, C.-C. Application of a hybrid numerical method to the nonlinear dynamic analysis of a micro gas bearing system. Nonlinear Dyn. 2009, 59, 695–710. [Google Scholar] [CrossRef]
- Wang, C.-C. Nonlinear dynamic behavior and bifurcation analysis of a rigid rotor supported by a relatively short externally pressurized porous gas journal bearing system. Acta Mech. 2006, 183, 41–60. [Google Scholar] [CrossRef]
- Guang-Hui, Z.; Yi, S.; Zhan-Sheng, L.; Fang-Cheng, X.; Jia-Jia, Y. Application of a forecasting coupling method to the non-linear dynamic analysis of a flexible rotor supported by externally pressurized orifices hybrid gas bearings. Proc. Inst. Mech. Eng. Part J: J. Eng. Tribol. 2011, 225, 704–717. [Google Scholar] [CrossRef]
- Wang, C.-C. Application of a hybrid method to the nonlinear dynamic analysis of a flexible rotor supported by a spherical gas-lubricated bearing system. Nonlinear Anal. Theory, Methods Appl. 2009, 70, 2035–2053. [Google Scholar] [CrossRef]
- Wang, C.-C.; Lee, T.-E. Nonlinear dynamic analysis of bi-directional porous aero-thrust bearing systems. Adv. Mech. Eng. 2017, 9, 1–11. [Google Scholar] [CrossRef]
- Khonsari, M.; Chang, Y.J. Stability Boundary of Non-Linear Orbits Within Clearance Circle of Journal Bearings. J. Vib. Acoust. 1993, 115, 303–307. [Google Scholar] [CrossRef]
- Li, J.; Chen, R.; Cao, H.; Tian, Z. Numerical study of nonlinear stability boundaries for orifice-compensated hole-entry hybrid journal bearings. Proc. Inst. Mech. Eng. Part J. J. Eng. Tribol. 2019, 1–14. [Google Scholar] [CrossRef]
- Wang, J.P.; Lieu, D.K. Magnetic lumped parameter modeling of rotor eccentricity in brushless permanent-magnet motors. IEEE Trans. Magn. 1999, 35, 4226–4231. [Google Scholar] [CrossRef]
- Wang, J.; Jing, M.; Fan, H.; Liu, H.; Shi, B. Study on Unbalance Magnetic Pull of the motorized spindle under different rotor eccentricities. In Proceedings of the 2016 13th International Conference on Ubiquitous Robots and Ambient Intelligence (URAI), Xi’an, China, 19–22 August 2016; Institute of Electrical and Electronics Engineers (IEEE): Piscataway, NJ, USA, 2016; pp. 781–785. [Google Scholar]
- Han, X.; Palazzolo, A. Unstable force analysis for induction motor eccentricity. J. Sound Vib. 2016, 370, 230–258. [Google Scholar] [CrossRef]
- Wu, Q.; Sun, Y.; Chen, W.; Chen, G.; Bai, Q.; Zhang, Q. Effect of motor rotor eccentricity on aerostatic spindle vibration in machining processes. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2017, 232, 1331–1342. [Google Scholar] [CrossRef]
- Wu, Q.; Sun, Y.; Chen, W.; Wang, Q.; Chen, G. Theoretical prediction and experimental verification of the unbalanced magnetic force in air bearing motor spindles. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2019, 233, 2330–2344. [Google Scholar] [CrossRef]
- Meessen, K.J.; Paulides, J.; Lomonova, E.E. Force Calculations in 3-D Cylindrical Structures Using Fourier Analysis and the Maxwell Stress Tensor. IEEE Trans. Magn. 2013, 49, 536–545. [Google Scholar] [CrossRef]
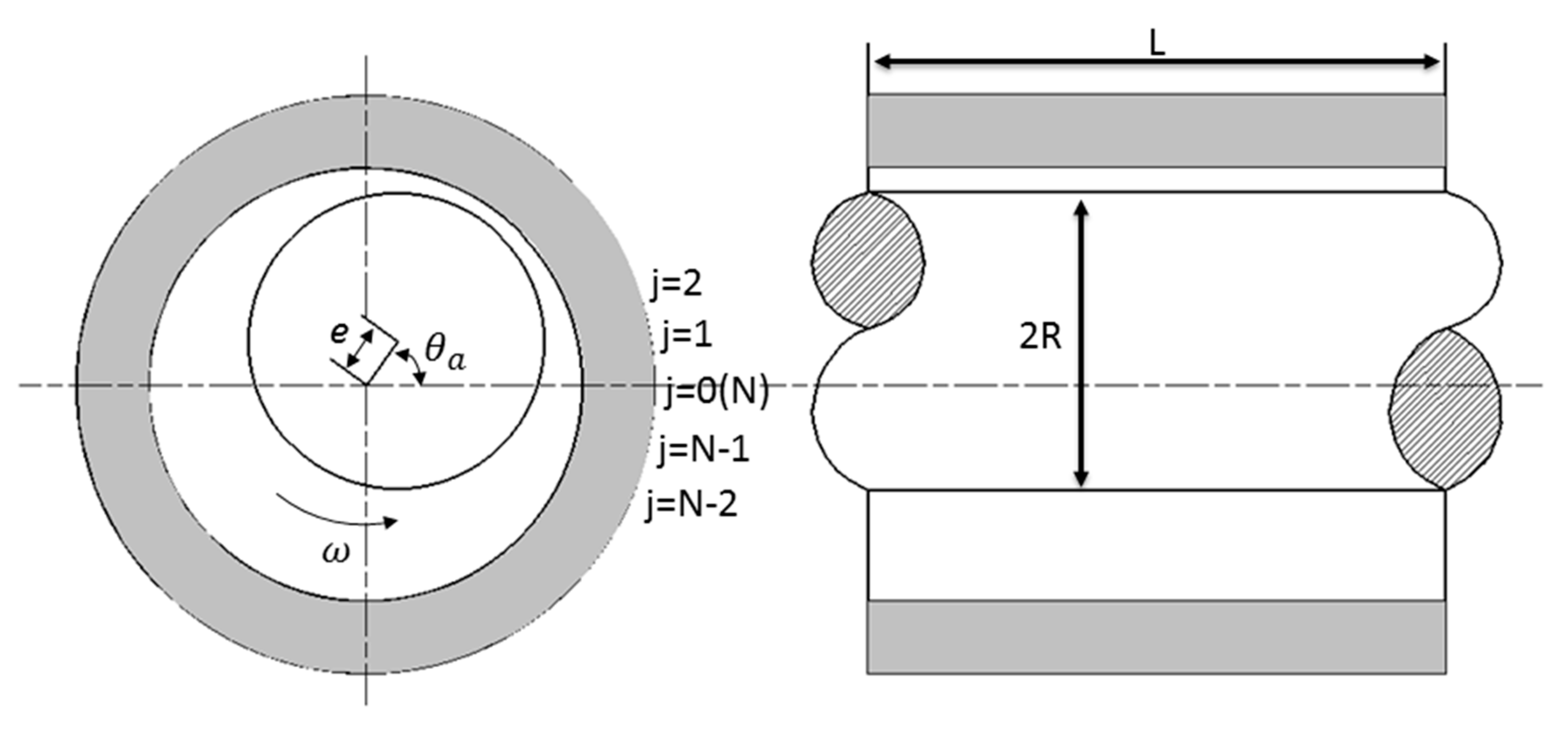
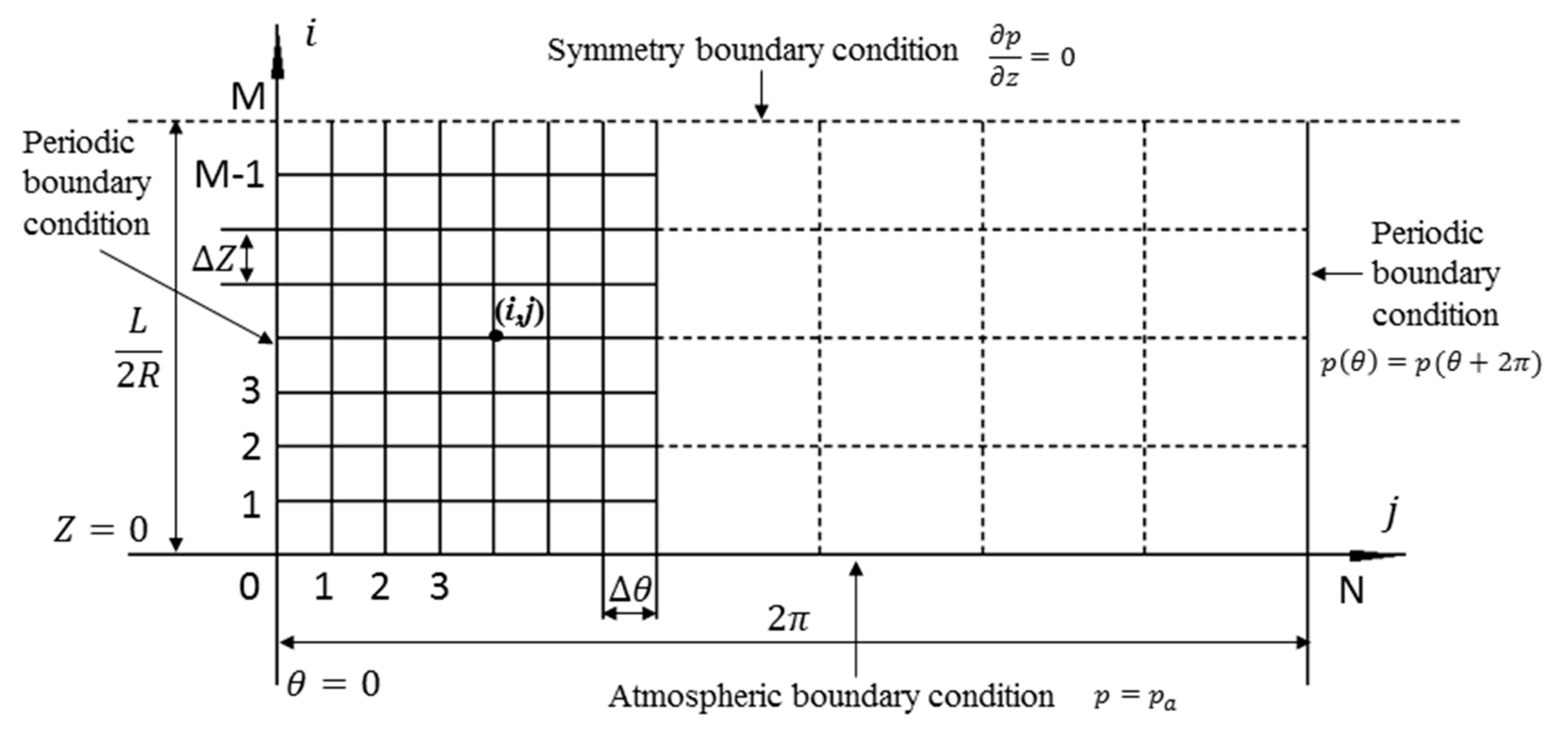
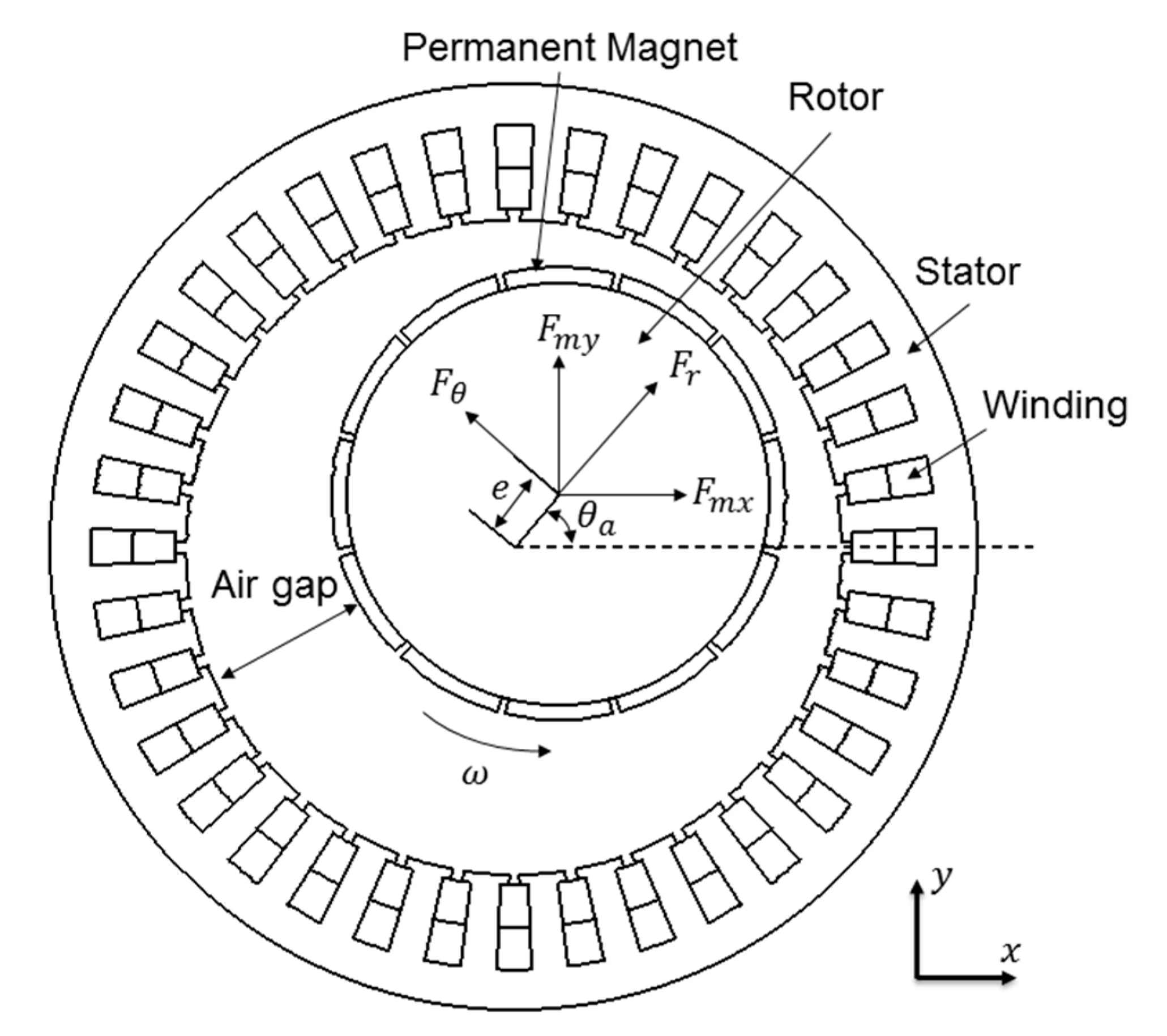


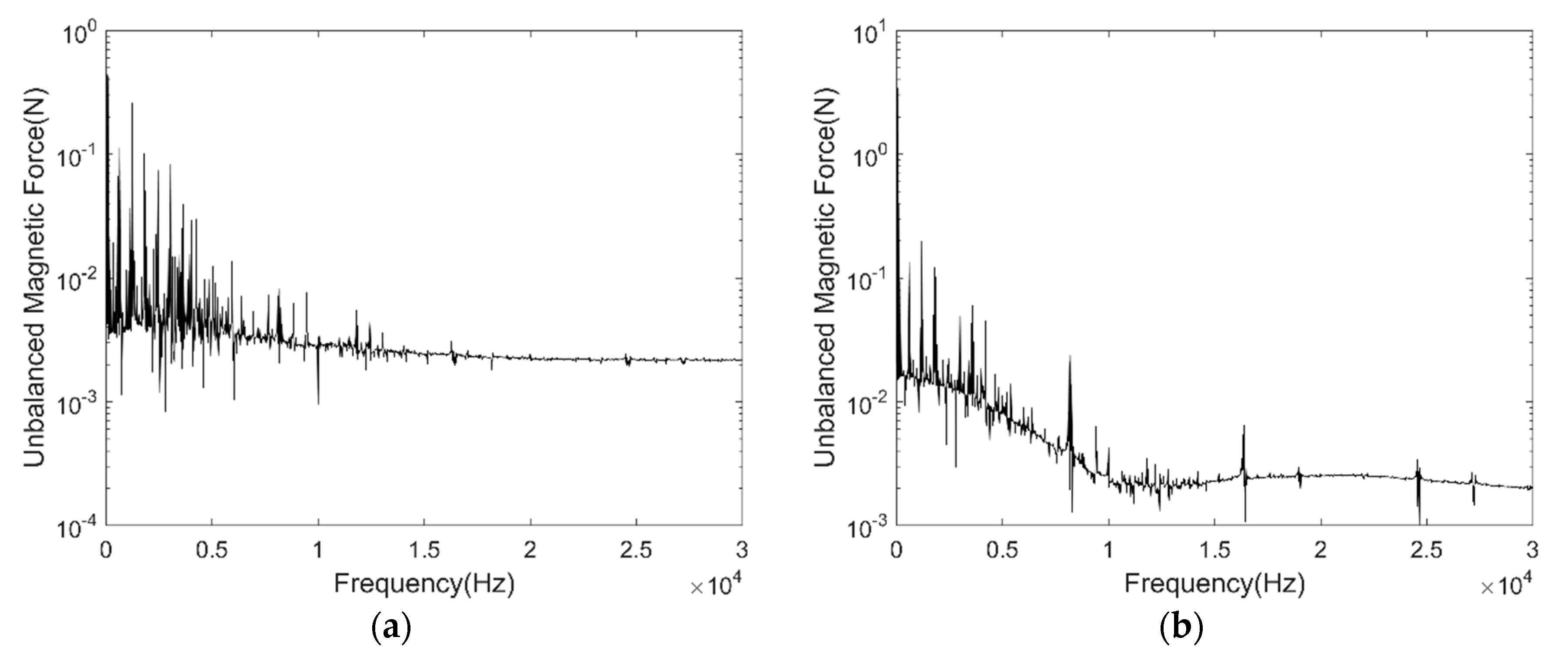
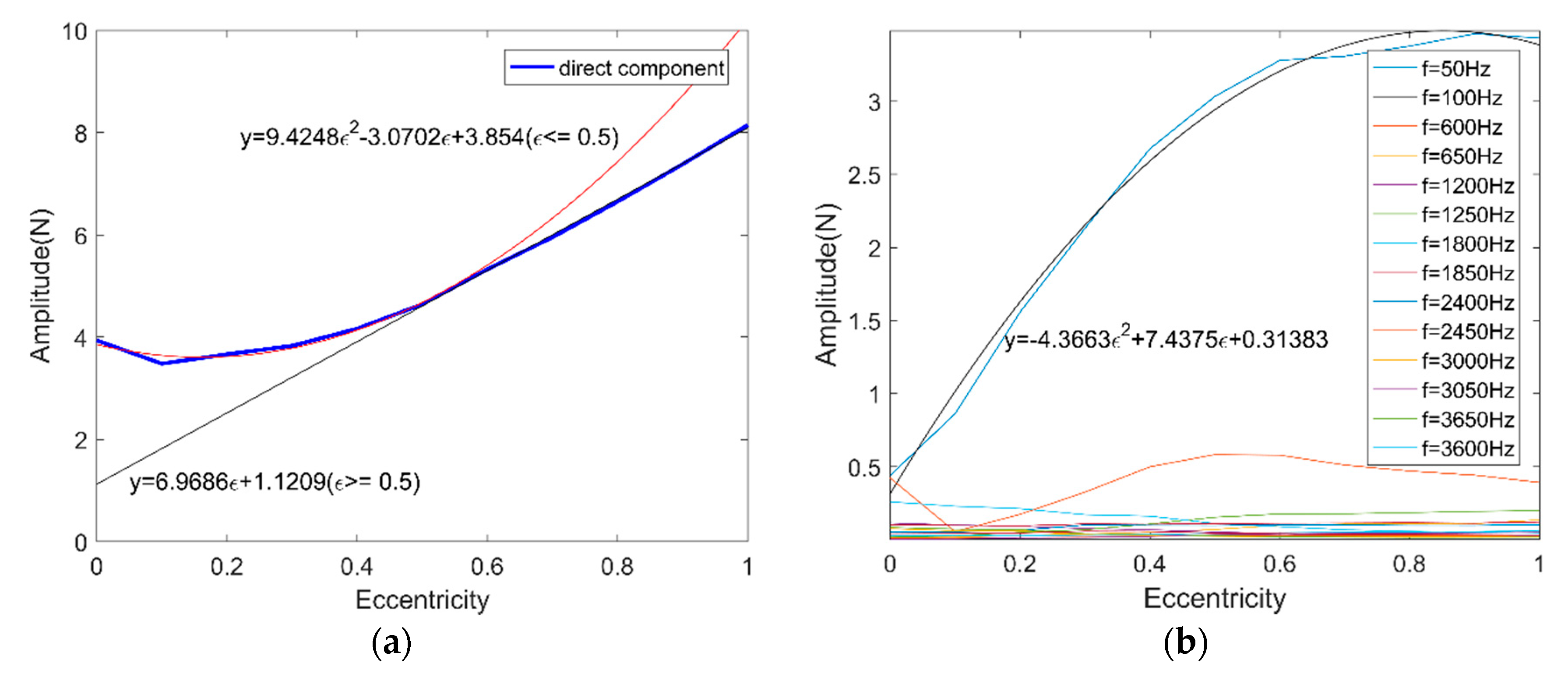

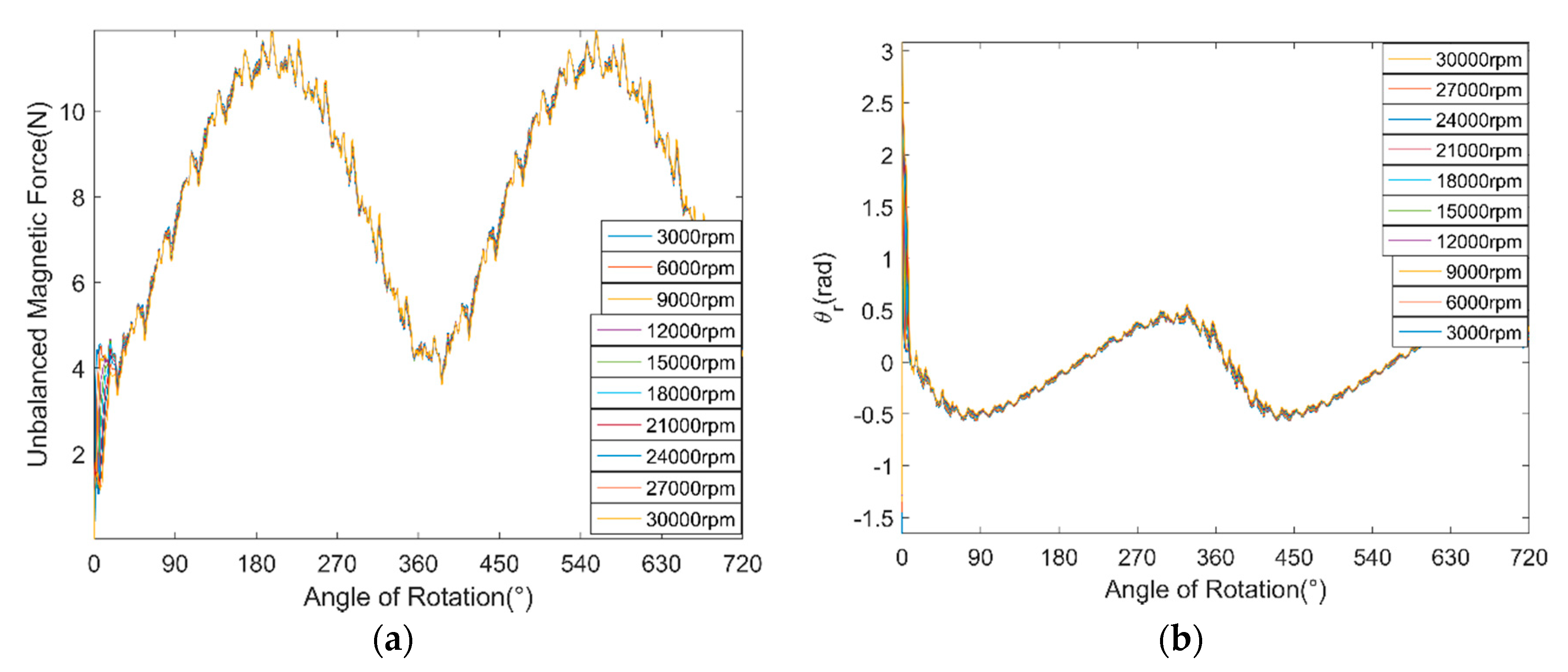
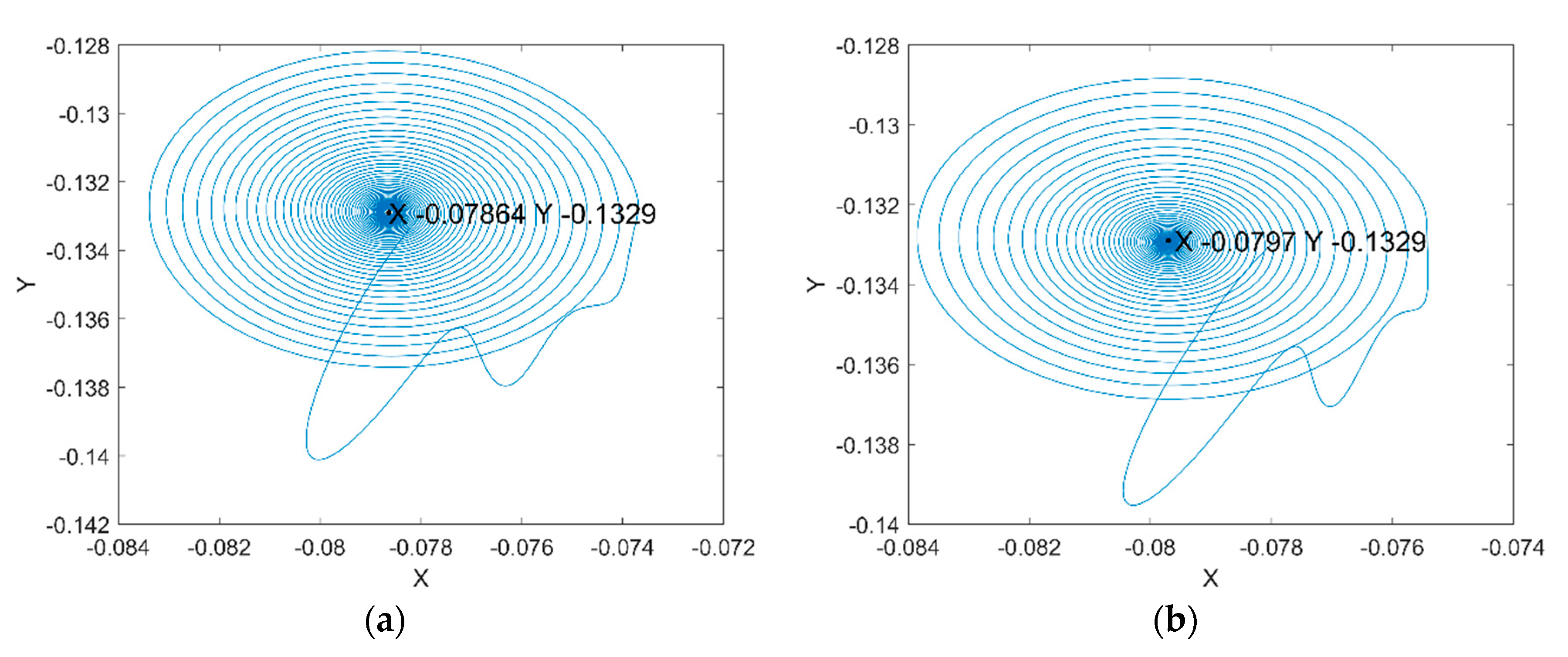



| Parameters (Unit) | Rotor | Stator |
|---|---|---|
| Outer stator diameter (mm) | 150 | 100 |
| Inner rotor diameter (mm) | 106 | – |
| Number of slots | 36 | |
| Number of poles | 12 | |
| Motor effective length (mm) | 80 | |
| Rated speed (r/min) | 3000 | |
| Air gap (mm) | 2 | |
| Winding form | Three phases of double layer winding | |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, G.; Chen, Y.; Lu, Q.; Wu, Q.; Wang, M. Multi-Physics Fields Based Nonlinear Dynamic Behavior Analysis of Air Bearing Motorized Spindle. Micromachines 2020, 11, 723. https://doi.org/10.3390/mi11080723
Chen G, Chen Y, Lu Q, Wu Q, Wang M. Multi-Physics Fields Based Nonlinear Dynamic Behavior Analysis of Air Bearing Motorized Spindle. Micromachines. 2020; 11(8):723. https://doi.org/10.3390/mi11080723
Chicago/Turabian StyleChen, Guoda, Yijie Chen, Qi Lu, Quanhui Wu, and Minghuan Wang. 2020. "Multi-Physics Fields Based Nonlinear Dynamic Behavior Analysis of Air Bearing Motorized Spindle" Micromachines 11, no. 8: 723. https://doi.org/10.3390/mi11080723
APA StyleChen, G., Chen, Y., Lu, Q., Wu, Q., & Wang, M. (2020). Multi-Physics Fields Based Nonlinear Dynamic Behavior Analysis of Air Bearing Motorized Spindle. Micromachines, 11(8), 723. https://doi.org/10.3390/mi11080723






