A Lubricating Oil Condition Monitoring System Based on Wear Particle Kinematic Analysis in Microfluid for Intelligent Aeroengine
Abstract
:1. Introduction
2. Design and Fabrication of the WKAS
2.1. Theoretical Calculation of the Wear Particle Velocity
2.2. Design of Microfluidic Channel
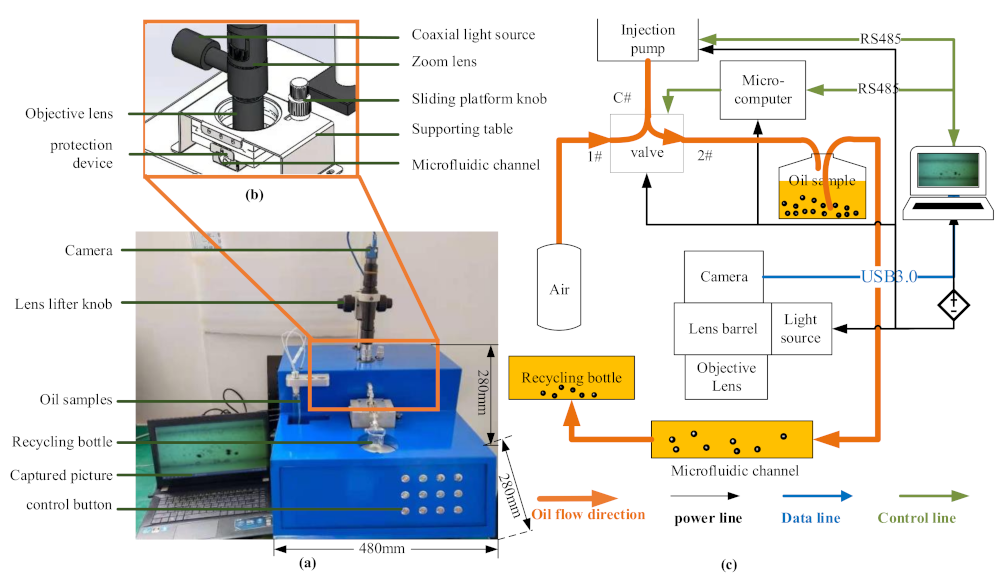
2.3. Fabrication of the WKAS Hardware System
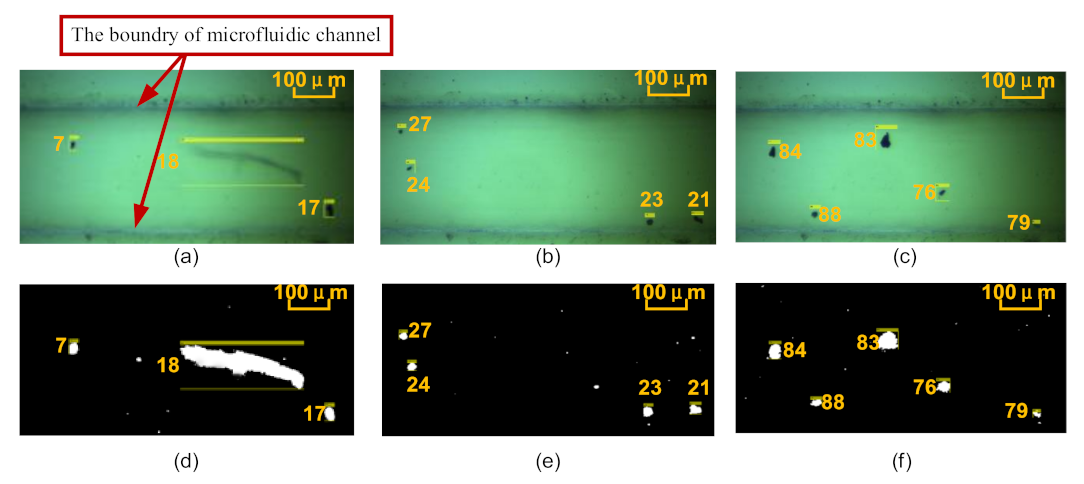
2.4. Particle Tracking and Velocity Measurement Algorithm
3. Experimental Section
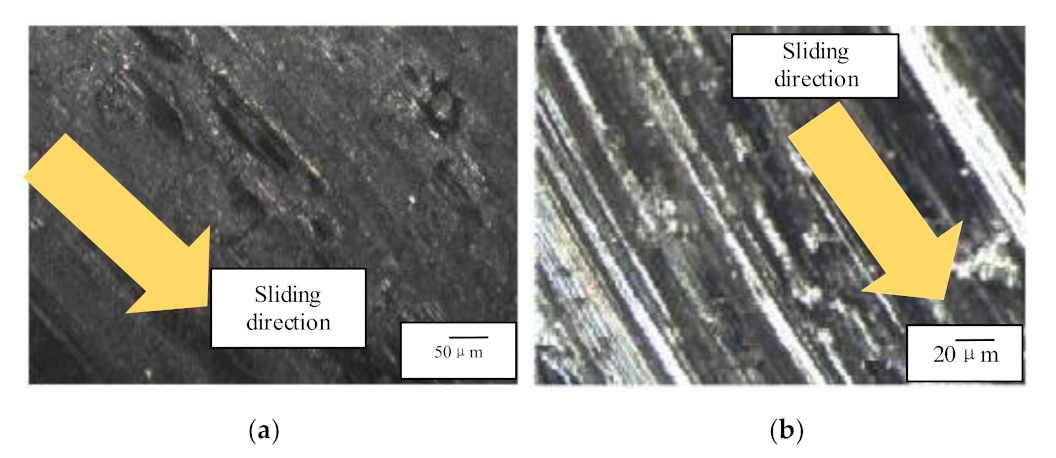
3.1. Experimental Setup
3.2. Results and Discussion
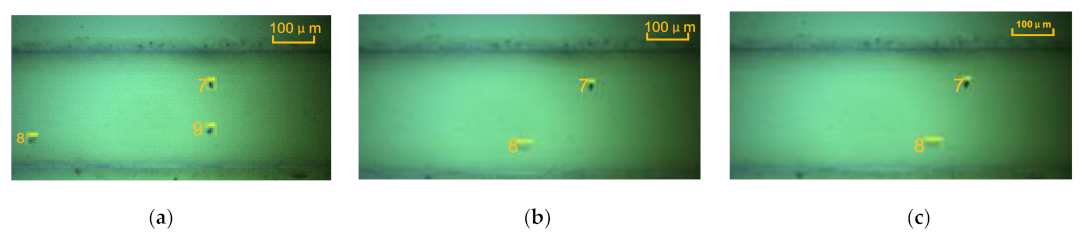
3.2.1. Monitoring of Particle Diameter Based on Average Velocity in the Lubricating Oil
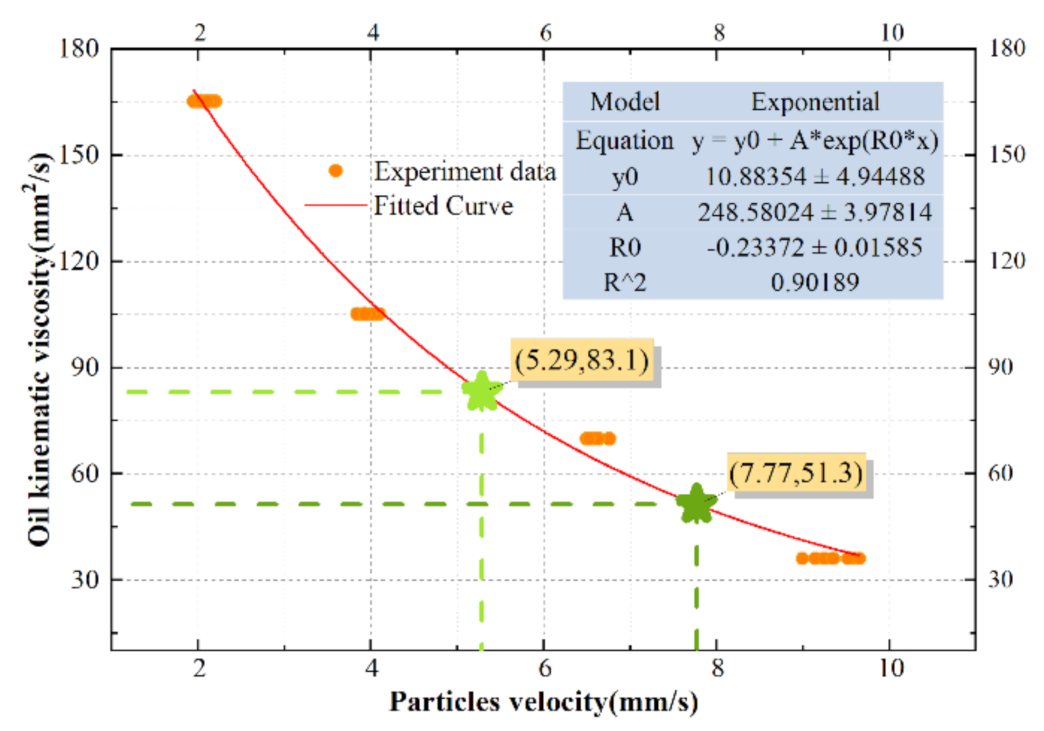
3.2.2. Monitoring of Lubricating Oil Kinematic Viscosity Based on Average Particle Velocity
3.2.3. Establishing Oil Viscosity Measurement Curve Based on Velocities of Particles with Fixed Diameter
4. Conclusions
- (1)
- The influencing factors of wear particle velocity were systematically studied. It was found that the wear particle velocity is closely related to the diameter of the particle and the viscosity. Furthermore, the effect of microfluidic channel size on the oil flow rate was analyzed, which was optimized as 3 cm × 300 μm × 200 μm.
- (2)
- In view of the wear particle motion image characteristics, a particle velocity measurement algorithm based on GMM and blob was developed, and the tracking results showed that the algorithm has high robustness.
- (3)
- As demonstrated experimentally, WKAS can be used to characterize the changes in particle diameter and oil viscosity. A larger particle diameter or greater oil viscosity results in lower average particle velocity. Notably, compared with other traditional oil condition monitoring methods, WKAS not only provides multidimensional oil condition monitoring information but also reflects wear severity. Thus, it is obvious that WKAS has great potential application in the condition monitoring of intelligent aeroengines.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| Symbol | Unit | Description |
| Width of the microfluidic channel | ||
| Height of the microfluidic channel | ||
| Length of the microfluidic channel | ||
| D | Equivalent diameter of the microfluidic channel | |
| p | Oil pressure | |
| Shear stress | ||
| Oil flow | ||
| Wear particle size | ||
| / | Reynolds number | |
| Oil density | ||
| Particle density | ||
| Oil dynamic viscosity coefficient | ||
| Oil kinematic viscosity coefficient | ||
| Oil instantaneous velocity | ||
| Oil average velocity | ||
| Particle velocity | ||
| s | Time | |
| Acceleration of gravity | ||
| Gravity | ||
| Buoyancy | ||
| Drag force | ||
| Additional mass force | ||
| Basset force | ||
| Magnus force | ||
| Saffman force |
References
- Maria, H.D.S.; Frogeri, R.F.; Piurcosky, F.P.; Prado, L. Áurea Remotely Piloted Aircraft: Analysis of the Deployment in Aeronautical Accident Investigation Bureau. J. Aerosp. Technol. Manag. 2021, 13, 0121. [Google Scholar] [CrossRef]
- Akli, B.M.; Abdelkrim, N.; Fatima, H.; Fethi, D. Moving objects detection and tracking with camera motion compensation. In Proceedings of the International Conference on Electrical Engineering and Control Applications, Singapore, Singapore, 10–13 June 2021; pp. 1193–1210. [Google Scholar]
- Wu, T.; Wu, H.; Du, Y.; Peng, Z. Progress and trend of sensor technology for online oil monitoring. Sci. China Ser. E Technol. Sci. 2013, 56, 2914–2926. [Google Scholar] [CrossRef]
- Xie, C.; Zhang, P.; Yan, Z. Correlation analysis of aeroengine operation monitoring using deep learning. Soft Comput. 2021, 25, 551–562. [Google Scholar] [CrossRef]
- Wang, J.; Wang, G.; Cheng, L. Texture extraction of wear particles based on improved random Hough transform and visual saliency. Eng. Fail. Anal. 2020, 109. [Google Scholar] [CrossRef]
- Zhu, X.; Zhong, C.; Zhe, J. Lubricating oil conditioning sensors for online machine health monitoring—A review. Tribol. Int. 2017, 109, 473–484. [Google Scholar] [CrossRef] [Green Version]
- Mao, H.; Zuo, H.; Wang, H. Electrostatic Sensor Application for online Monitoring of Wind Turbine Gearboxes. Sensors 2018, 18, 3574. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ma, L.; Xu, Z.; Zhang, H.; Qiao, W.; Chen, H. Multifunctional Detection Sensor and Sensitivity Improvement of a Double Solenoid Coil Sensor. Micromachines 2019, 10, 377. [Google Scholar] [CrossRef] [Green Version]
- Dingxin, Y.; Zheng, H.; Jianwei, X. Research on capacitive sensor for online oil monitoring. In 2011 Prognostics and System Health Managment Conference; IEEE: New York, NY, USA, 2011; pp. 1–4. [Google Scholar] [CrossRef]
- Du, L.; Zhu, X.; Han, Y.; Zhe, J. High Throughput Wear Debris Detection in Lubricants Using a Resonance Frequency Division Multiplexed Sensor. Tribol. Lett. 2013, 51, 453–460. [Google Scholar] [CrossRef]
- Du, L.; Zhe, J. An integrated ultrasonic–inductive pulse sensor for wear debris detection. Smart Mater. Struct. 2012, 22, 025003. [Google Scholar] [CrossRef]
- Druy, M.A.; Crocombe, R.A.; Suresh, P. Portable, real-time alloy identification of metallic wear debris from machinery lubrication systems: Laser-induced breakdown spectroscopy versus x-ray fluorescence. In Next-Generation Spectroscopic Technologies VII; SPIE: Bern, Switzerland, 2014. [Google Scholar]
- Fan, H.; Gao, S.; Zhang, X.; Cao, X.; Ma, H.; Liu, Q. Intelligent Recognition of Ferrographic Images Combining Optimal CNN With Transfer Learning Introducing Virtual Images. IEEE Access 2020, 8, 137074–137093. [Google Scholar] [CrossRef]
- Khan, M.; Cheema, A.F.; Khan, S.Z.; Qureshi, S.-U.-R. Image based portable wear debris analysis tool. Ind. Lubr. Tribol. 2015, 67, 389–398. [Google Scholar] [CrossRef]
- Cao, W.; Zhang, H.; Wang, N.; Wang, H.W.; Peng, Z.X. The gearbox wears state monitoring and evaluation based on on-line wear debris features. Wear 2019, 426-427, 1719–1728. [Google Scholar] [CrossRef]
- Peng, Y.; Wu, T.; Wang, S.; Du, Y.; Kwok, N.; Peng, Z. A microfluidic device for three-dimensional wear debris imaging in online condition monitoring. Proc. Inst. Mech. Eng. J. Eng. Tribol. 2017, 231, 965–974. [Google Scholar] [CrossRef]
- Peng, Y.; Cai, J.; Wu, T.; Cao, G.; Kwok, N.; Zhou, S.; Peng, Z. Online wear characterisation of rolling element bearing using wear particle morphological features. Wear 2019, 430–431, 369–375. [Google Scholar] [CrossRef]
- Peng, P.; Wang, J. Wear particle classification considering particle overlapping. Wear 2019, 422–423, 119–127. [Google Scholar] [CrossRef]
- Peng, Y.; Wu, T.; Wang, S.; Peng, Z. Wear state identification using dynamic features of wear debris for online purpose. Wear 2017, 376–377, 1885–1891. [Google Scholar] [CrossRef]
- Wu, T.H.; Mao, J.H.; Wang, J.T.; Wu, J.Y.; Xie, Y.B. A New On-Line Visual Ferrograph. Tribol. Trans. 2009, 52, 623–631. [Google Scholar] [CrossRef]
- Visitskii, Y.; Petrov, A.; Shunderyuk, M. The motion of a particle in a viscous fluid under gravity, vibration and Basset’s force. J. Appl. Math. Mech. 2009, 73, 548–557. [Google Scholar] [CrossRef]
- Khatibi, M.; Time, R.W.; Rabenjafimanantsoa, H. Particles falling through viscoelastic non-Newtonian flows in a horizontal rectangular channel analyzed with PIV and PTV techniques. J. Non-Newton. Fluid Mech. 2016, 235, 143–153. [Google Scholar] [CrossRef]
- Han, Y.-F.; Mei, N. Theoretical and experimental studies of the transport process of micro-particles in static water. J. Hydrodyn. 2014, 26, 875–881. [Google Scholar] [CrossRef]
- Mala, G.M.; Li, D. Flow characteristics of water in microtubes. Int. J. Heat Fluid Flow 1999, 20, 142–148. [Google Scholar] [CrossRef]
- Yang, L.; Zhang, J.; Yuan, H.; Mei, N. Analysis of the motion of small-scale ellipsoidal particles in a horizontal laminar flow field. Particuology 2018, 40, 44–51. [Google Scholar] [CrossRef]
- Joseph, P.; Tabeling, P. Direct measurement of the apparent slip length. Phys. Rev. E 2005, 71, 035303. [Google Scholar] [CrossRef]
- Lauga, E. Apparent Slip Due to the Motion of Suspended Particles in Flows of Electrolyte Solutions. Langmuir 2004, 20, 8924–8930. [Google Scholar] [CrossRef] [Green Version]
- Lumma, D.; Best, A.; Gansen, A.; Feuillebois, F.; Rädler, J.O.; Vinogradova, O.I. Flow profile near a wall measured by double-focus fluorescence cross-correlation. Phys. Rev. E 2003, 67, 056313. [Google Scholar] [CrossRef]
- Lemley, E.C.; Handy, T.A.; Papavassiliou, D.V.; Neeman, H.J. Laminar entrance length in microtubes. In Proceedings of the Fluids Engineering Division Summer Meeting, Vail, CO, USA, 2–6 August 2009; pp. 549–554. [Google Scholar] [CrossRef]
- Mukherjee, D.; Wu, Q.M.J.; Nguyen, T.M. Gaussian Mixture Model with Advanced Distance Measure Based on Support Weights and Histogram of Gradients for Background Suppression. IEEE Trans. Ind. Inform. 2013, 10, 1086–1096. [Google Scholar] [CrossRef]





| Ratio | |
|---|---|
| 1 | |
| Name | Corporation | Model |
|---|---|---|
| Microchannel | Huarui Chip Technology Co., Ltd., Zhenjiang, China | Quartz glass |
| Slide platform | Super Eye Technology Co., Ltd., Shenzhen, China | Z006 |
| Objective lens/lens lifter | Jiangnan Novel Optics Co., Ltd., Nanjing, China | 10X/SHL-10A |
| Zoom lens barrel | Shanghai Optical Co., Ltd., Shanghai, China | 2× |
| Camera/video capture software | Daheng Imaging Co., Ltd., Beijing, China | MER2-301-125U3C/StreamPix |
| Injection pump/valve | Runze Fluid Co., Ltd., Nanjing, China | MiNi-SY04/Mrv-01B |
| Microcomputer | All Controller Aviation Technology Co., Ltd., Nanjing, China | MC9S08DZ128 |
| Test Piece | Material | Ingredient | Size/mm | Surface Roughness (μm) | Surface Hardness (HRC) |
|---|---|---|---|---|---|
| Pin | GCr15 | 1.01% C; 1.50% Cr; 0.30% Mn; 0.25% Si; ≤0.02% S; ≤0.027% P | 6 | 0.04 | 60 |
| Disc | GCr15 | 1.01% C; 1.50% Cr; 0.30% Mn; 0.25% Si; ≤0.02% S; ≤0.027% P | 80 × 8 | 0.4 | 10 |
| Run | Diameter (μm) | Velocity (mm/s) | Viscosity (mm2/s) |
|---|---|---|---|
| 1 | 49.252 | 9.613 | 36.06 |
| 49.381 | 9.548 | 36.06 | |
| 49.674 | 9.604 | 36.06 | |
| 50.246 | 9.142 | 36.06 | |
| 50.678 | 9.354 | 36.06 | |
| 51.054 | 9.523 | 36.06 | |
| 48.263 | 9.648 | 36.06 | |
| 49.947 | 9.249 | 36.06 | |
| 49.324 | 9.345 | 36.06 | |
| 50.126 | 8.994 | 36.06 | |
| 2 | 50.134 | 6.813 | 69.87 |
| 50.249 | 6.799 | 69.87 | |
| 50.631 | 6.802 | 69.87 | |
| 50.247 | 6.854 | 69.87 | |
| 49.958 | 6.792 | 69.87 | |
| 50.854 | 6.757 | 69.87 | |
| 49.594 | 6.838 | 69.87 | |
| 49.246 | 6.743 | 69.87 | |
| 48.937 | 6.817 | 69.87 | |
| 50.133 | 6.728 | 69.87 | |
| 3 | 50.168 | 4.256 | 105.1 |
| 49.624 | 4.195 | 105.1 | |
| 51.029 | 4.213 | 105.1 | |
| 50.847 | 4.152 | 105.1 | |
| 49.581 | 4.094 | 105.1 | |
| 49.874 | 3.996 | 105.1 | |
| 48.249 | 3.958 | 105.1 | |
| 50.319 | 3.942 | 105.1 | |
| 50.197 | 4.013 | 105.1 | |
| 51.273 | 3.934 | 105.1 | |
| 4 | 50.156 | 2.199 | 165.2 |
| 51.217 | 2.056 | 165.2 | |
| 51.349 | 2.183 | 165.2 | |
| 50.487 | 2.158 | 165.2 | |
| 50.149 | 2.059 | 165.2 | |
| 49.638 | 1.953 | 165.2 | |
| 49.826 | 1.995 | 165.2 | |
| 49.659 | 2.037 | 165.2 | |
| 49.593 | 2.105 | 165.2 | |
| 50.164 | 2.112 | 165.2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Z.; Liu, Y.; Zuo, H.; Wang, H.; Fei, H. A Lubricating Oil Condition Monitoring System Based on Wear Particle Kinematic Analysis in Microfluid for Intelligent Aeroengine. Micromachines 2021, 12, 748. https://doi.org/10.3390/mi12070748
Liu Z, Liu Y, Zuo H, Wang H, Fei H. A Lubricating Oil Condition Monitoring System Based on Wear Particle Kinematic Analysis in Microfluid for Intelligent Aeroengine. Micromachines. 2021; 12(7):748. https://doi.org/10.3390/mi12070748
Chicago/Turabian StyleLiu, Zhenzhen, Yan Liu, Hongfu Zuo, Han Wang, and Hang Fei. 2021. "A Lubricating Oil Condition Monitoring System Based on Wear Particle Kinematic Analysis in Microfluid for Intelligent Aeroengine" Micromachines 12, no. 7: 748. https://doi.org/10.3390/mi12070748
APA StyleLiu, Z., Liu, Y., Zuo, H., Wang, H., & Fei, H. (2021). A Lubricating Oil Condition Monitoring System Based on Wear Particle Kinematic Analysis in Microfluid for Intelligent Aeroengine. Micromachines, 12(7), 748. https://doi.org/10.3390/mi12070748







