Dynamic Modeling and Flow Distribution of Complex Micron Scale Pipe Network
Abstract
:1. Introduction
2. Model Development
- (a)
- No power source in the fluid network model.
- (b)
- The fluid state in the nodes is uniform (the internal pressure is equal).
- (c)
- The flow resistance only takes the equivalent frictional resistance along the pipeline into account and keeps the flow resistance coefficient constant.
- (d)
- The cross-sectional area in the same branch pipe remains unchanged, and the working medium parameters are represented by the weighted average of the connected node parameters.
- (a)
- Nodes, including pipe transitions and other essential components in the microfluidic network.
- (b)
- Branches, a connecting component between two nodes.
3. Results and Discussions
3.1. Model Design and Electrical Equivalent
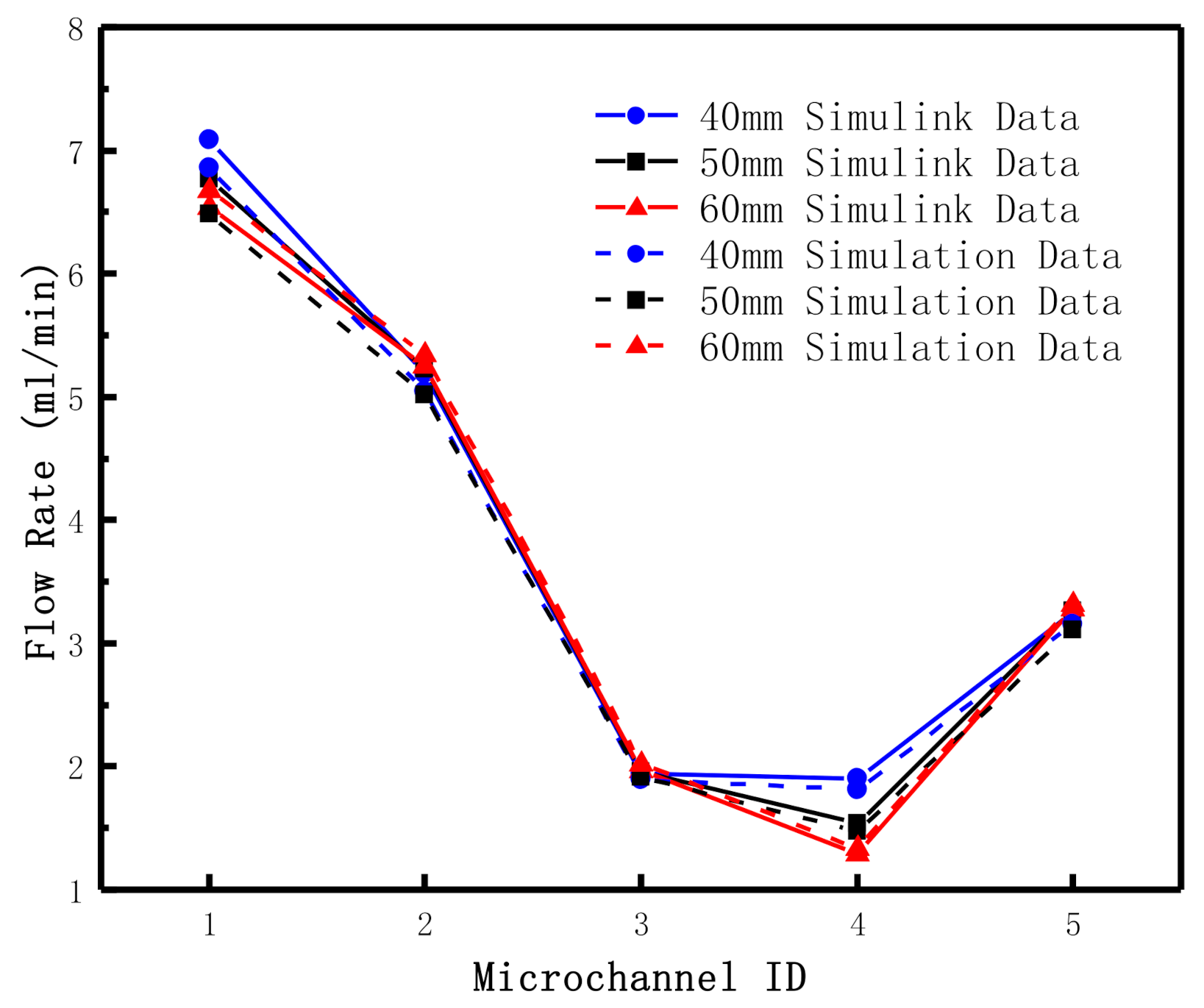
3.2. Model Calculation Results
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Gou, Y.; Jia, Y.; Wang, P.; Sun, C. Progress of Inertial Microfluidics in Principle and Application. Sensors 2018, 18, 1762. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zhang, K.; Jiang, L.; Gao, Z.; Zhai, C.; Yan, W.; Wu, S. Design and Numerical Study of Micropump Based on Induced Electroosmotic Flow. J. Nanotechnol. 2018, 2018, 4018503. [Google Scholar] [CrossRef] [Green Version]
- Aracil, C.; Perdigones, F.; Moreno, J.M.; Luque, A.; Quero, J.M. Portable Lab-on-PCB platform for autonomous micromixing. Microelectron. Eng. 2014, 131, 13–18. [Google Scholar] [CrossRef]
- Okkels, F.; Bruus, H. Design of Micro-Fluidic Bio-Reactors Using Topology Optimization. In Proceedings of the European Conference on Computational Fluid Dynamics, Mekelweg, The Netherlands, 5–8 September 2006. [Google Scholar]
- Mathew, B.; John, T.J.; Hegab, T.J. Effect of Manifold Design on Flow Distribution in Multichanneled Microfluidic Devices. In Proceedings of the Asme Fluids Engineering Division Summer Meeting, Vail, CO, USA, 2–6 August 2009. [Google Scholar]
- Delsman, E.R.; Pierik, A.; De Croon, M.H.J.M.; Kramer, G.J.; Schouten, J.C. Microchannel Plate Geometry Optimization for Even Flow Distribution at High Flow Rates. Chem. Eng. Res. Des. 2004, 82, 267–273. [Google Scholar] [CrossRef]
- Alharbi, A.Y.; Pence, D.V.; Cullion, R.N. Fluid Flow Through Microscale Fractal-Like Branching Channel Networks. J. Fluids Eng. 2003, 125, 1051–1057. [Google Scholar] [CrossRef]
- Jing, D.; Yi, S. Electroosmotic Flow in Tree-like Branching Microchannel Network. Fractals 2019, 9, 1950095. [Google Scholar] [CrossRef]
- Chen, Y.; Cheng, P. Heat transfer and pressure drop in fractal tree-like microchannel nets. Int. J. Heat Mass Transf. 2002, 45, 2643–2648. [Google Scholar] [CrossRef]
- Bassiouny, M.K.; Martin, H. Flow Distribution and Pressure Drop in Plate Heat Exchangers I U-Type Arrangement. Chem. Eng. Sci. 1984, 39, 693–700. [Google Scholar] [CrossRef]
- Bassiouny, M.K.; Martin, H. Flow distribution and pressure drop in plate heat exchangers II Z-type arrangement. Chem. Eng. Sci. 1984, 39, 701–704. [Google Scholar] [CrossRef]
- Kim, S.; Choi, E.; Cho, Y.I. The effect of header shapes on the flow distribution in a manifold for electronic packaging applications. Int. Commun. Heat Mass Transf. 1995, 22, 329–341. [Google Scholar] [CrossRef]
- Wang, J. Pressure drop and flow distribution in parallel-channel configurations of fuel cells: Z-type arrangement. Int. J. Hydrogen Energy 2010, 35, 5498–5509. [Google Scholar] [CrossRef]
- Idelchik, I.E. Handbook of Hydraulic Resistance, 4th Edition Revised and Augmented; Begell House Inc.: Moscow, Russia, 2008. [Google Scholar]
- Bruus, H. Theoretical Microfluidics; Oxford University Press: Oxford, UK, 2008. [Google Scholar]



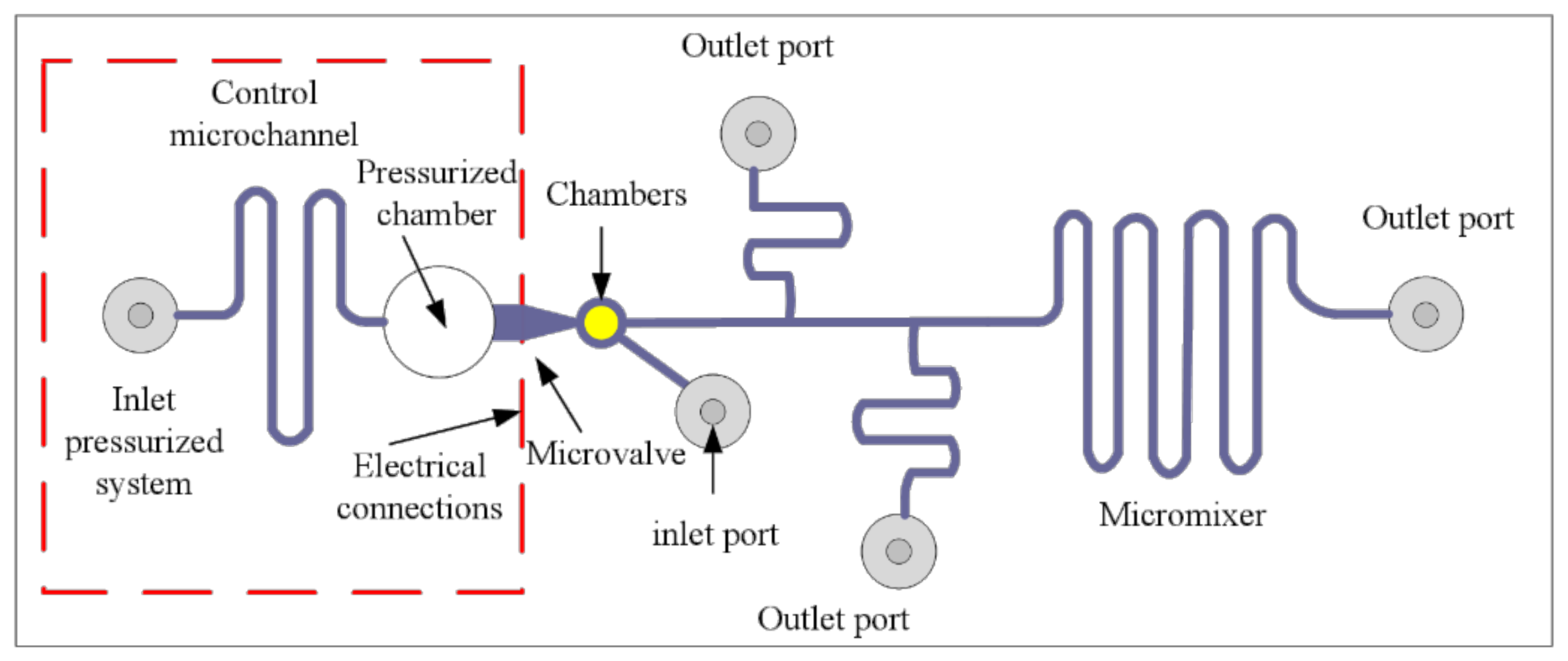
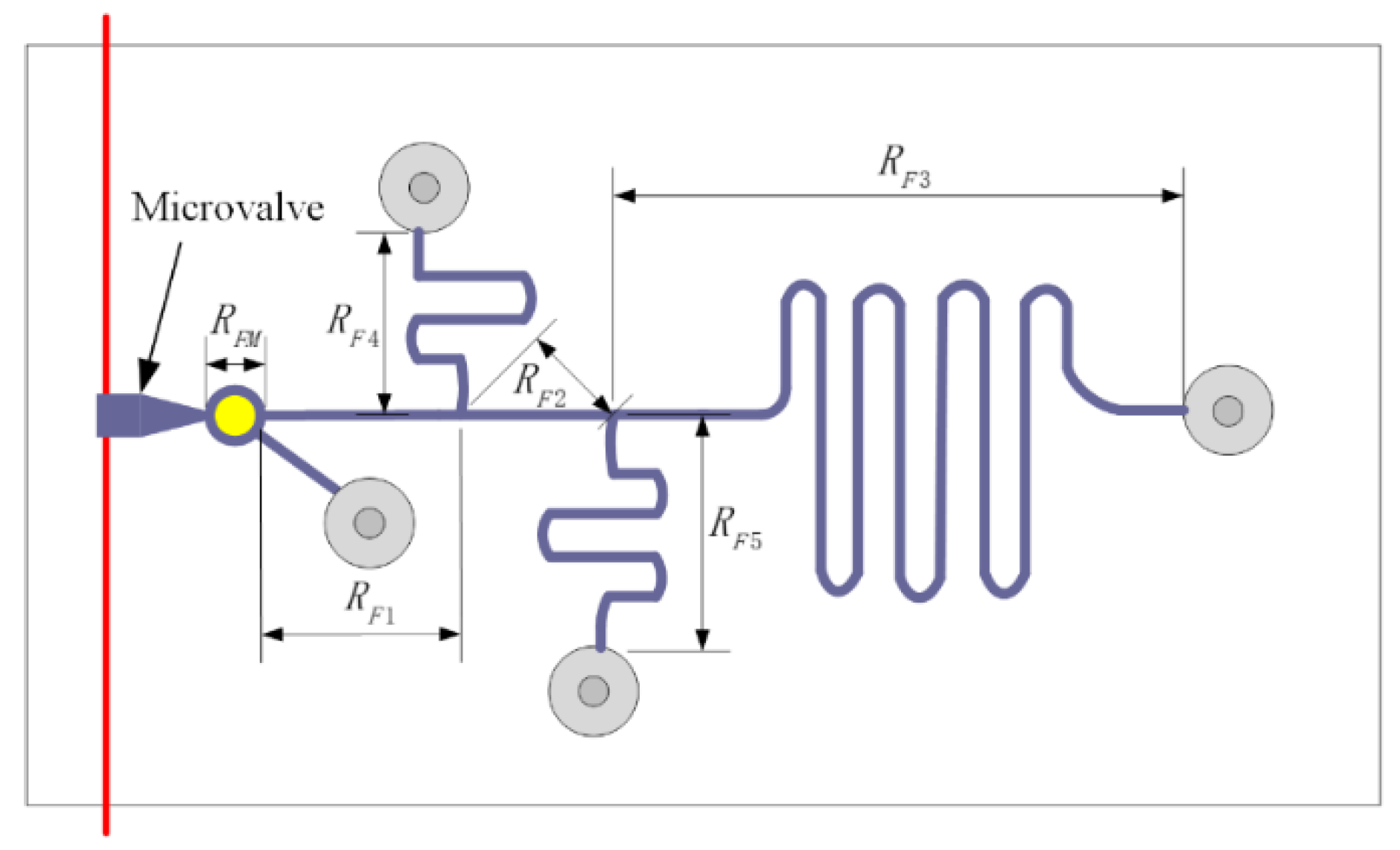
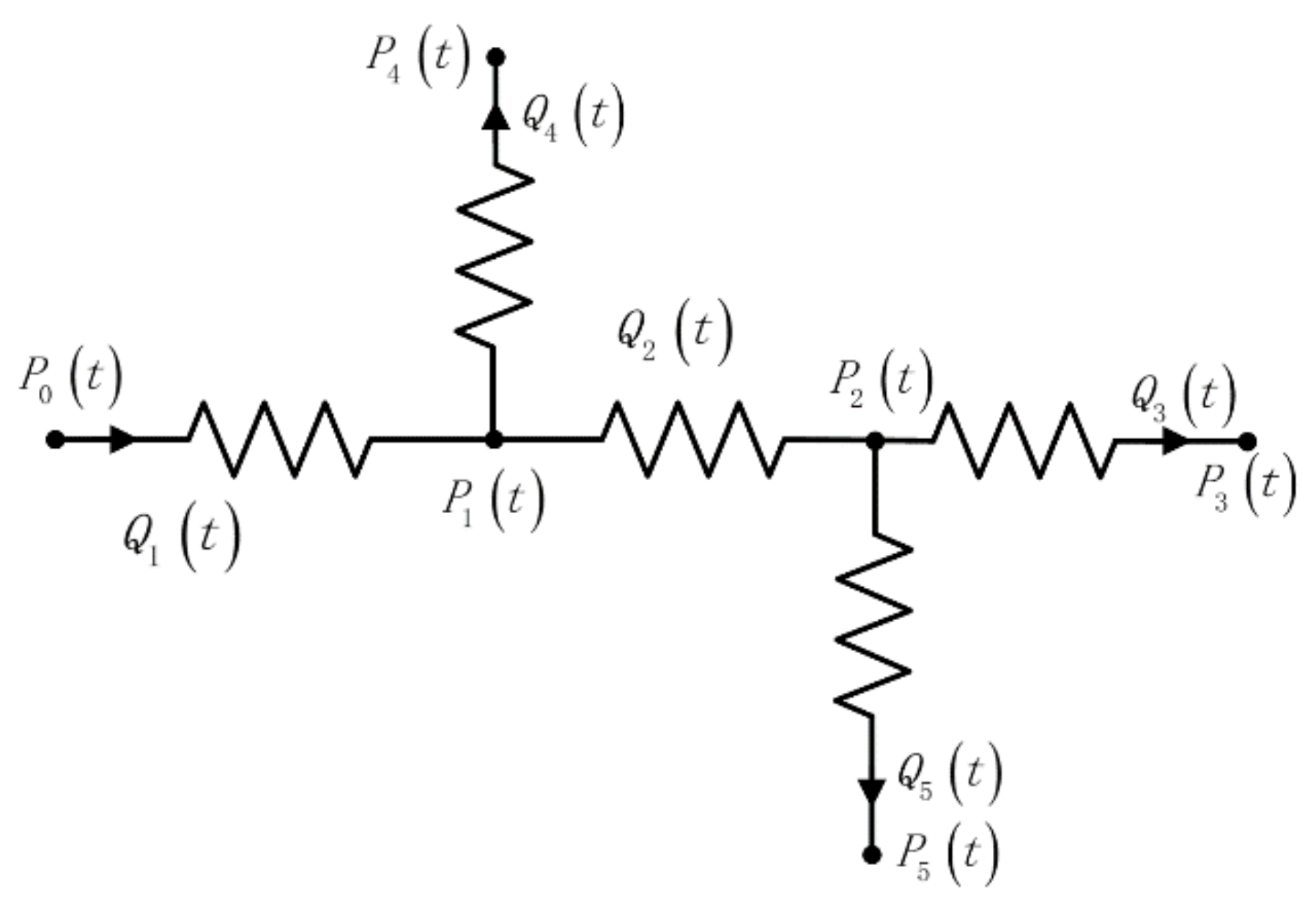

| Parameter | |||||
|---|---|---|---|---|---|
| ) | 500 | 500 | 350 | 350 | 350 |
| ) | 500 | 500 | 350 | 350 | 350 |
| Length (mm) | 10 | 8 | 50 | 30 | 30 |
| ) | 4.64 | 3.71 | 96.6 | 57.9 | 57.9 |
| Entrance Pressure (KPa) | 15 | 18 | 20 | |
|---|---|---|---|---|
| (mL/min) | Simulink Data | 7.623 | 13.990 | 18.235 |
| Simulation Data | 7.767 | 13.812 | 17.945 | |
| Relative Error (%) | 1.883 | 1.272 | 1.585 | |
| (mL/min) | Simulink Data | 5.126 | 8.895 | 11.407 |
| Simulation Data | 5.217 | 8.802 | 11.246 | |
| Relative Error (%) | 1.772 | 1.054 | 1.414 | |
| (mL/min) | Simulink Data | 1.921 | 3.333 | 4.275 |
| Simulation Data | 2.000 | 3.211 | 4.086 | |
| Relative Error (%) | 4.100 | 3.671 | 4.421 | |
| (mL/min) | Simulink Data | 2.497 | 5.095 | 6.827 |
| Simulation Data | 2.550 | 5.011 | 6.700 | |
| Relative Error (%) | 2.111 | 1.653 | 1.871 | |
| (mL/min) | Simulink Data | 3.205 | 5.561 | 7.132 |
| Simulation Data | 3.217 | 5.590 | 7.160 | |
| Relative Error (%) | 0.378 | 0.515 | 0.388 | |
| Microchannel 4 Length (mm) | 40 | 50 | 60 | |
|---|---|---|---|---|
| ) | 7.73 | 9.66 | 11.6 | |
| (mL/min) | Simulink Data | 7.090 | 6.764 | 6.541 |
| Simulation Data | 6.855 | 6.488 | 6.680 | |
| Relative Error (%) | 3.305 | 4.078 | 2.104 | |
| (mL/min) | Simulink Data | 5.188 | 5.226 | 5.252 |
| Simulation Data | 5.047 | 5.016 | 5.342 | |
| Relative Error (%) | 2.733 | 4.014 | 1.721 | |
| (mL/min) | Simulink Data | 1.944 | 1.958 | 1.968 |
| Simulation Data | 1.894 | 1.912 | 2.026 | |
| Relative Error (%) | 2.580 | 2.369 | 2.943 | |
| (mL/min) | Simulink Data | 1.903 | 1.538 | 1.290 |
| Simulation Data | 1.81 | 1.472 | 1.337 | |
| Relative Error (%) | 4.863 | 4.297 | 3.663 | |
| Simulink Data | 3.244 | 3.267 | 3.284 | |
| Simulation Data | 3.152 | 3.104 | 3.316 | |
| Relative Error (%) | 2.825 | 5.000 | 0.989 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, Y.; Zhang, K.; Guo, F.; Yang, M. Dynamic Modeling and Flow Distribution of Complex Micron Scale Pipe Network. Micromachines 2021, 12, 763. https://doi.org/10.3390/mi12070763
Zhao Y, Zhang K, Guo F, Yang M. Dynamic Modeling and Flow Distribution of Complex Micron Scale Pipe Network. Micromachines. 2021; 12(7):763. https://doi.org/10.3390/mi12070763
Chicago/Turabian StyleZhao, Yao, Kai Zhang, Fengbei Guo, and Mingyue Yang. 2021. "Dynamic Modeling and Flow Distribution of Complex Micron Scale Pipe Network" Micromachines 12, no. 7: 763. https://doi.org/10.3390/mi12070763
APA StyleZhao, Y., Zhang, K., Guo, F., & Yang, M. (2021). Dynamic Modeling and Flow Distribution of Complex Micron Scale Pipe Network. Micromachines, 12(7), 763. https://doi.org/10.3390/mi12070763







