Modified Hexagonal Split Ring Resonator Based on an Epsilon-Negative Metamaterial for Triple-Band Satellite Communication
Abstract
:1. Introduction
2. Design of the Unit Cell and Simulation
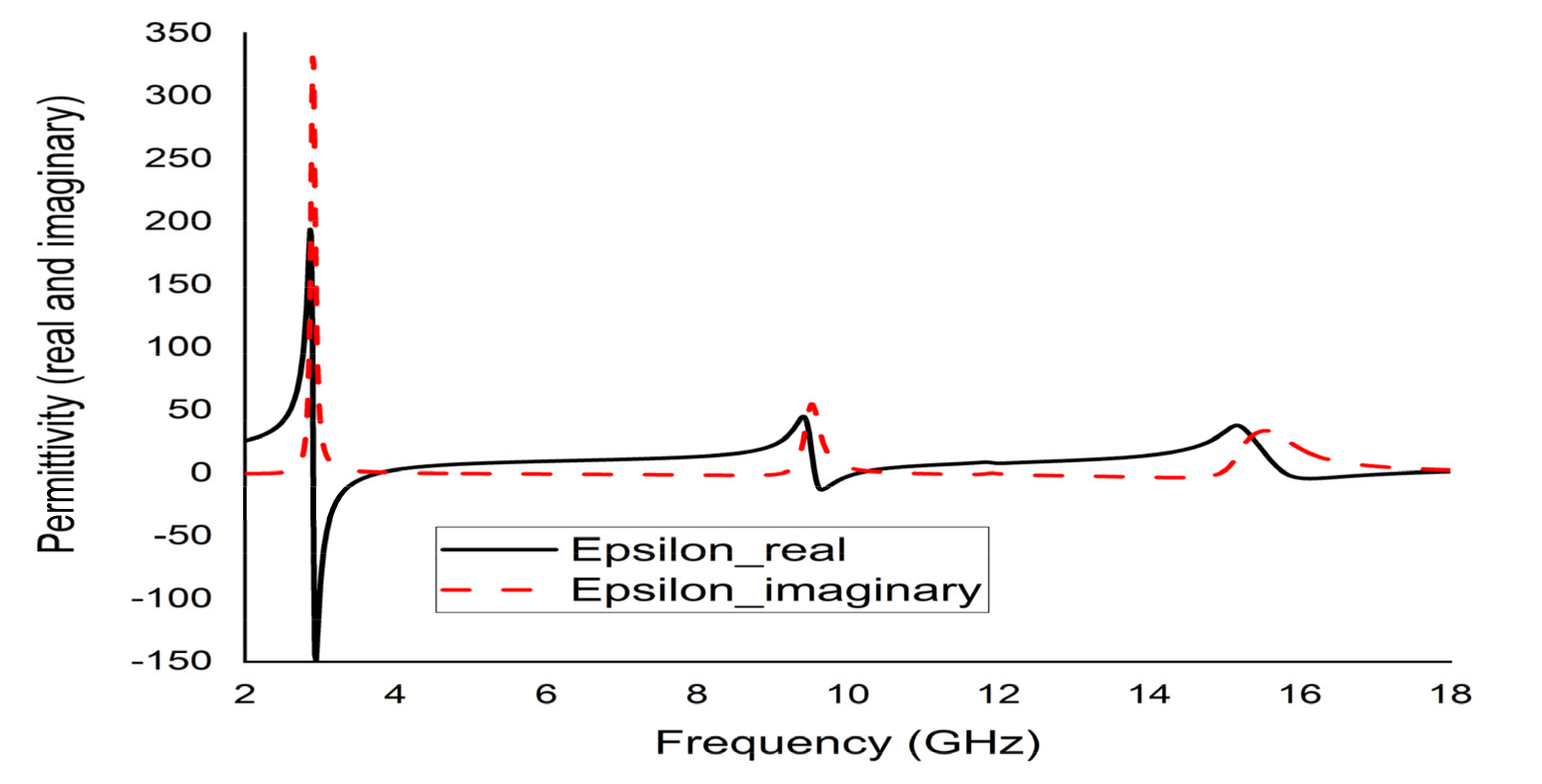
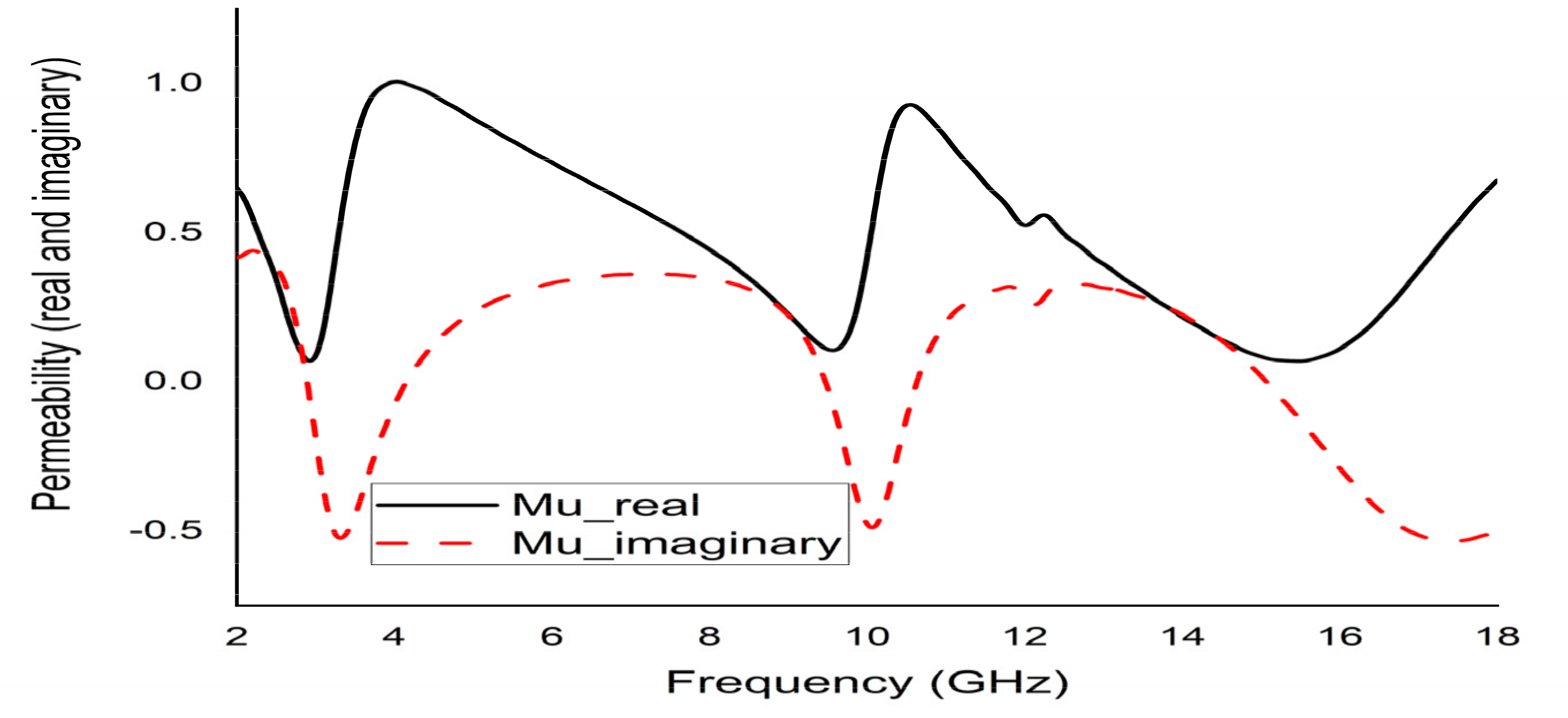
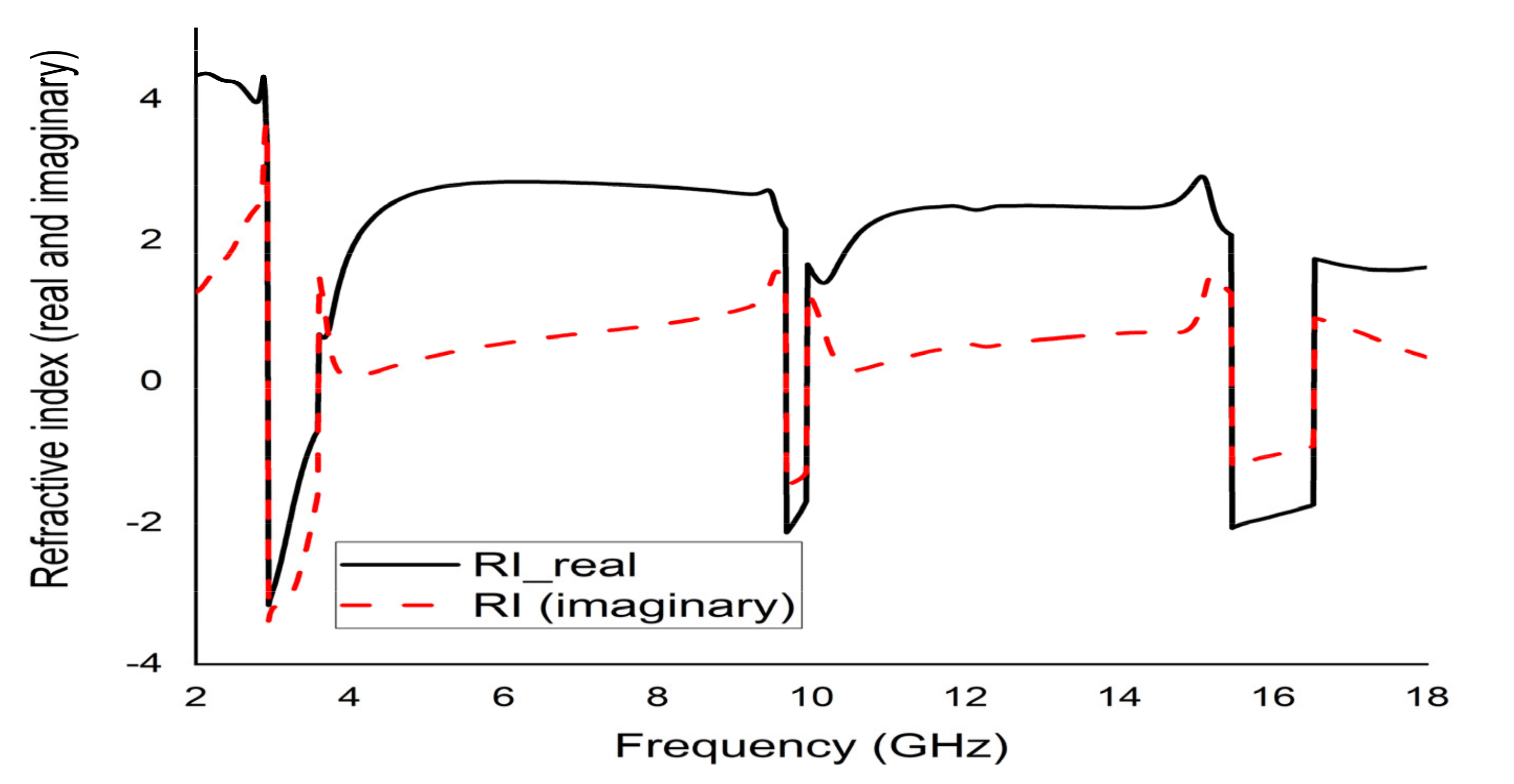
3. Effective Medium Parameters Extraction Method
4. Parametric Study
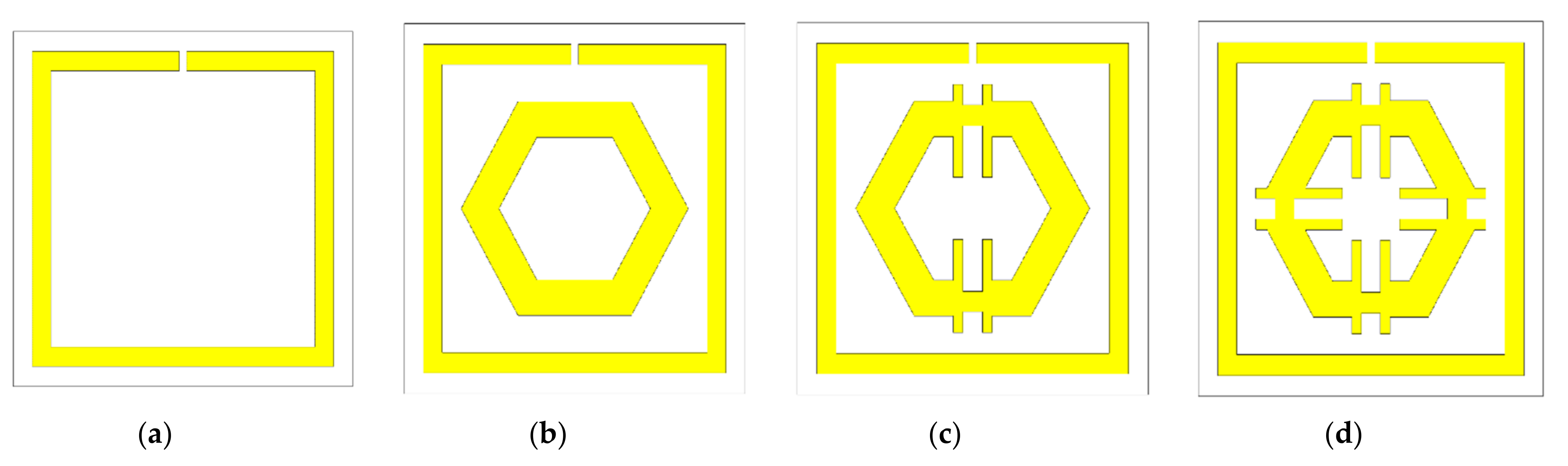
4.1. Design Procedure
4.2. Effects of Changing of the Unit Cell Size
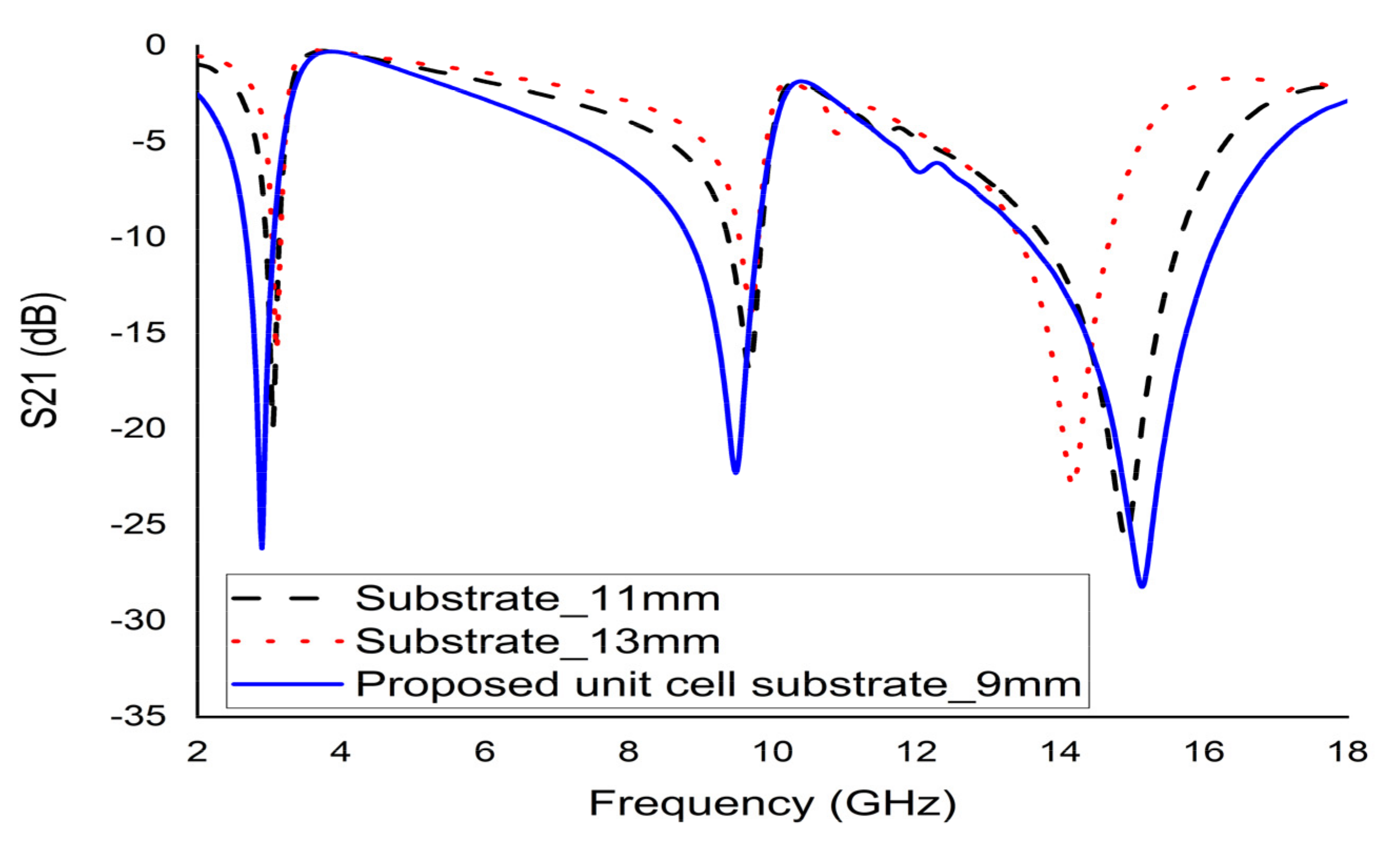
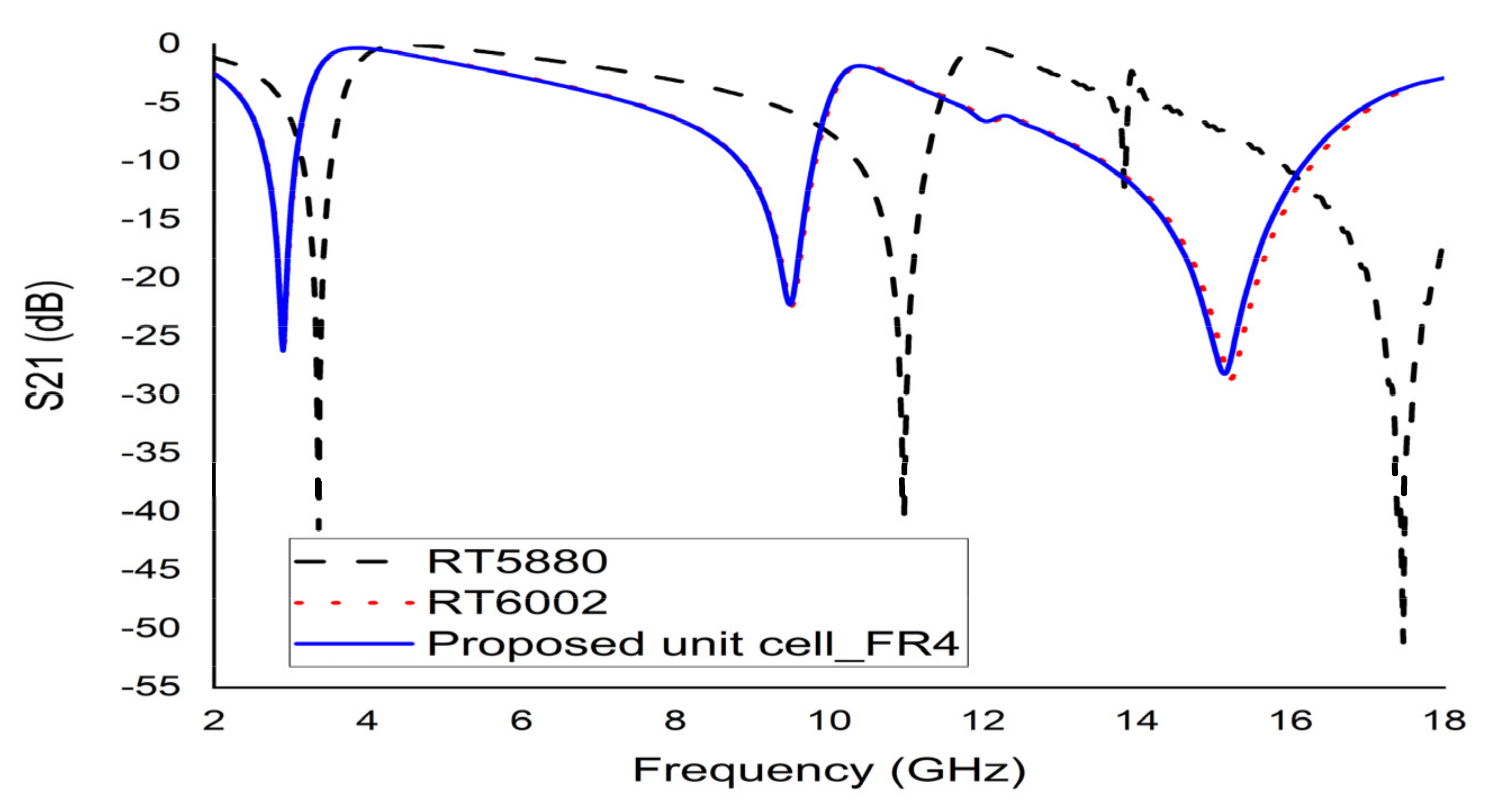
4.3. Effects of Changing Substrate Properties
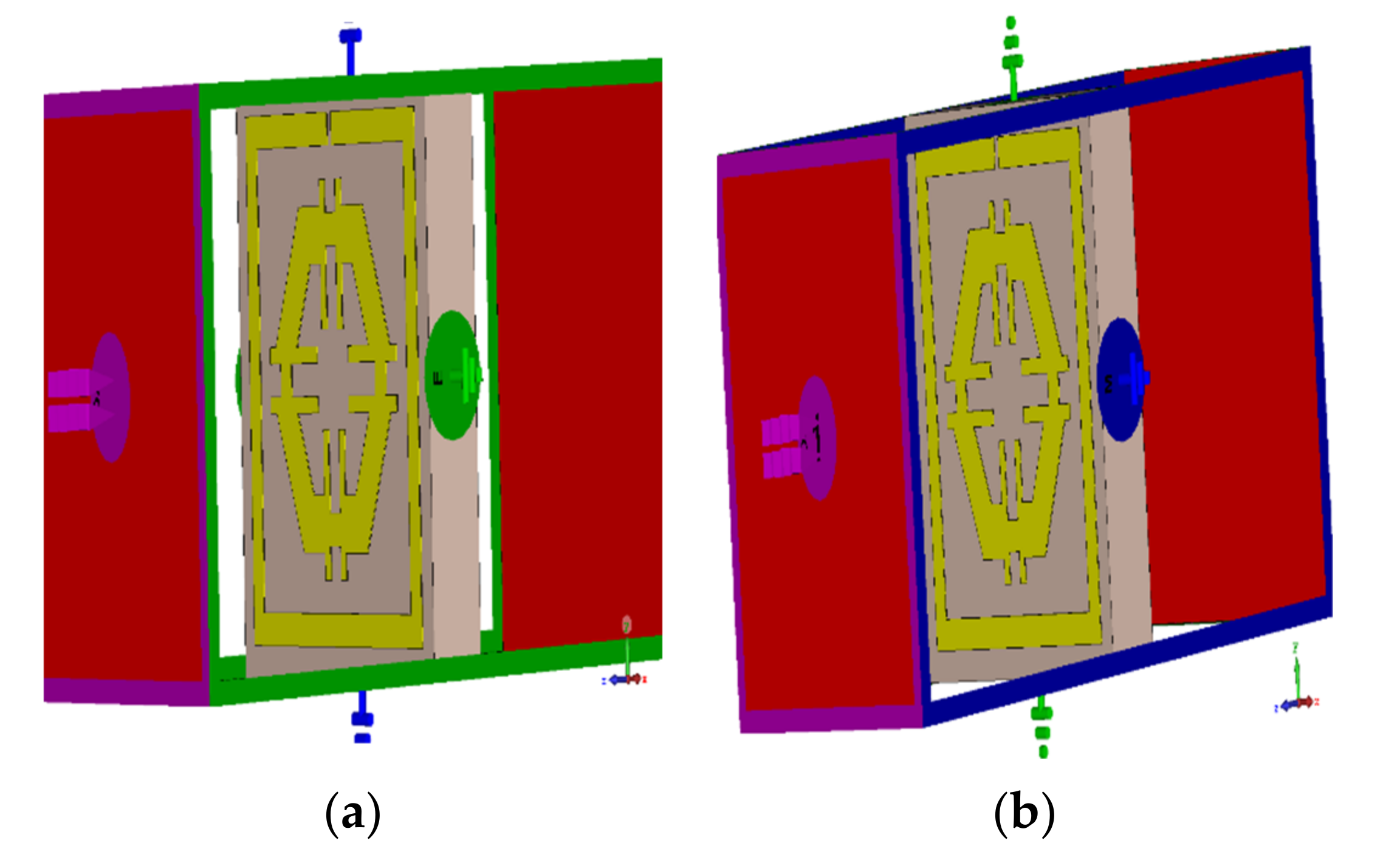
4.4. Effects of Changing the EM Field Propagation Direction
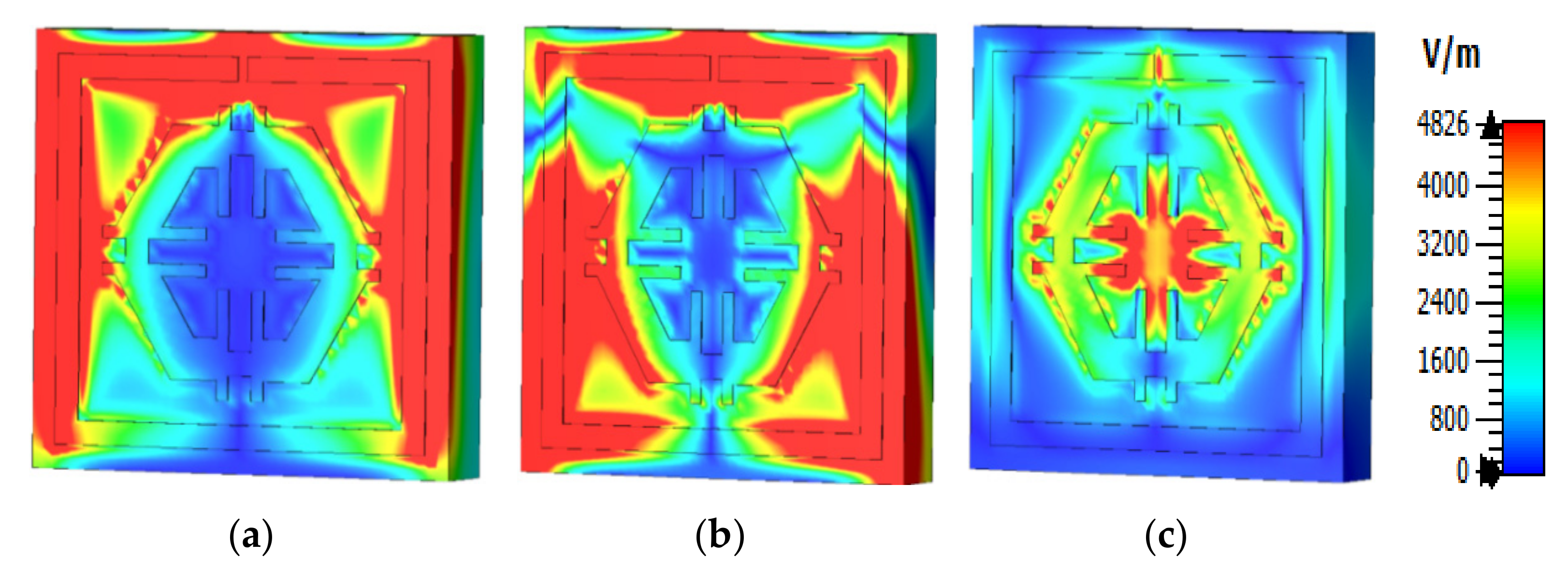
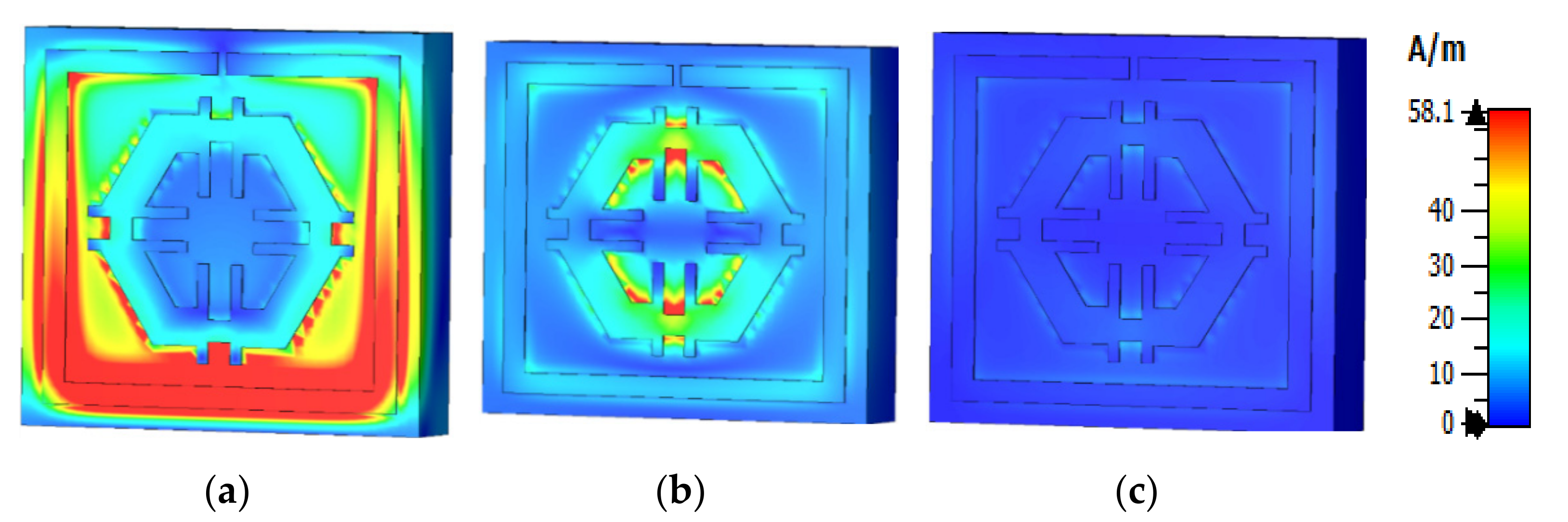
5. Surface Current, Electric Field, and Magnetic Field Analysis
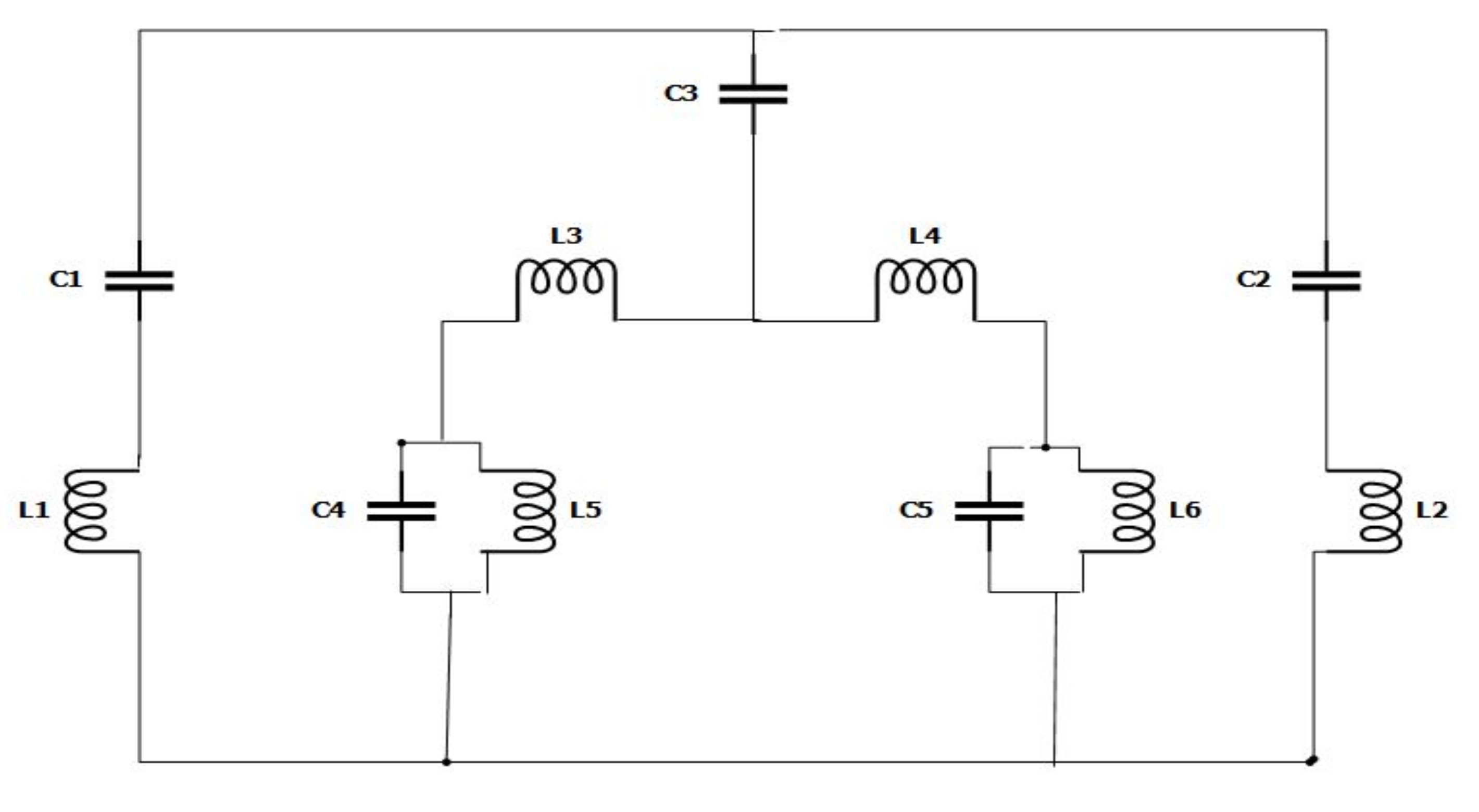
6. Circuit Model of the Projected Metamaterial Unit Cell
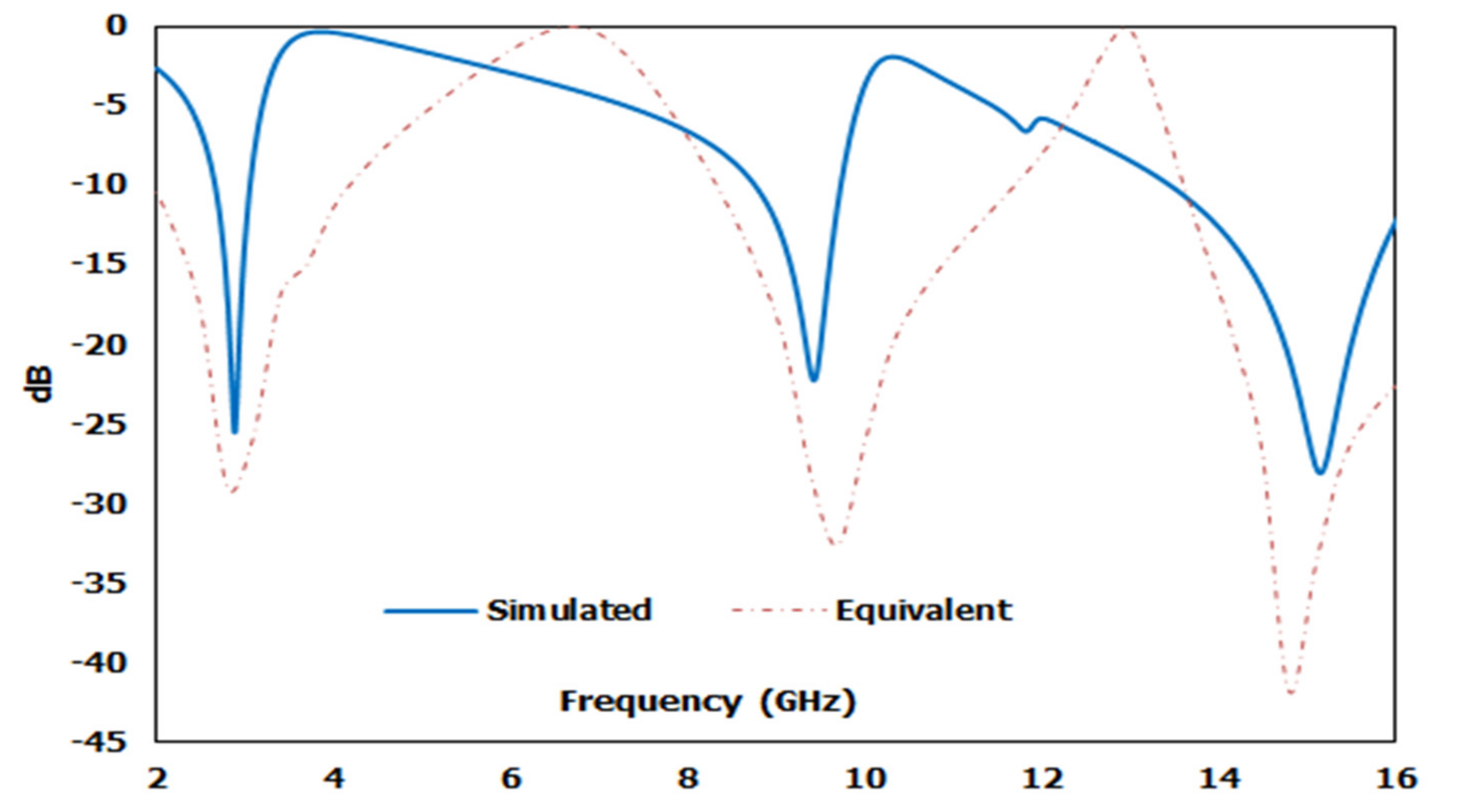
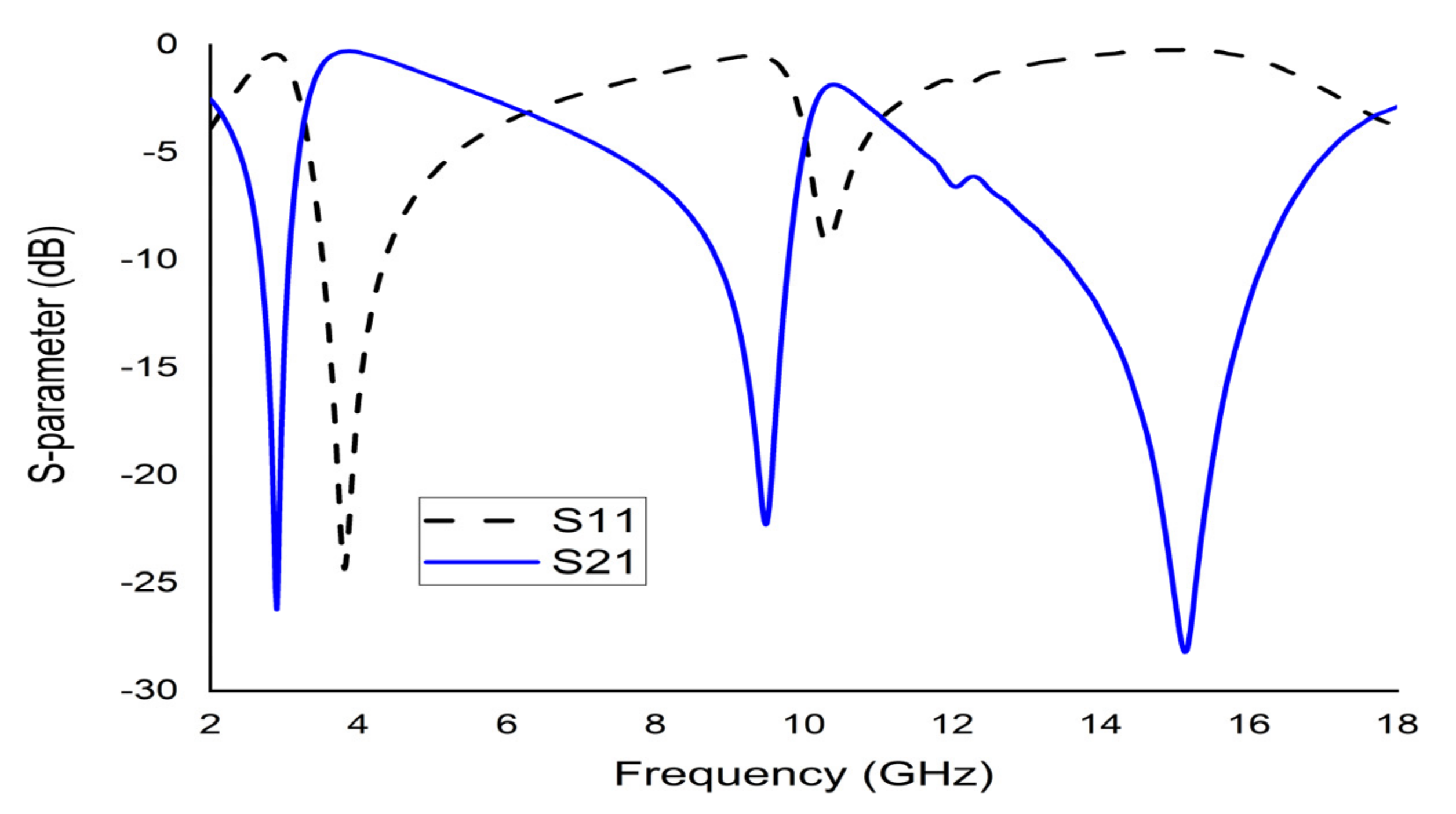
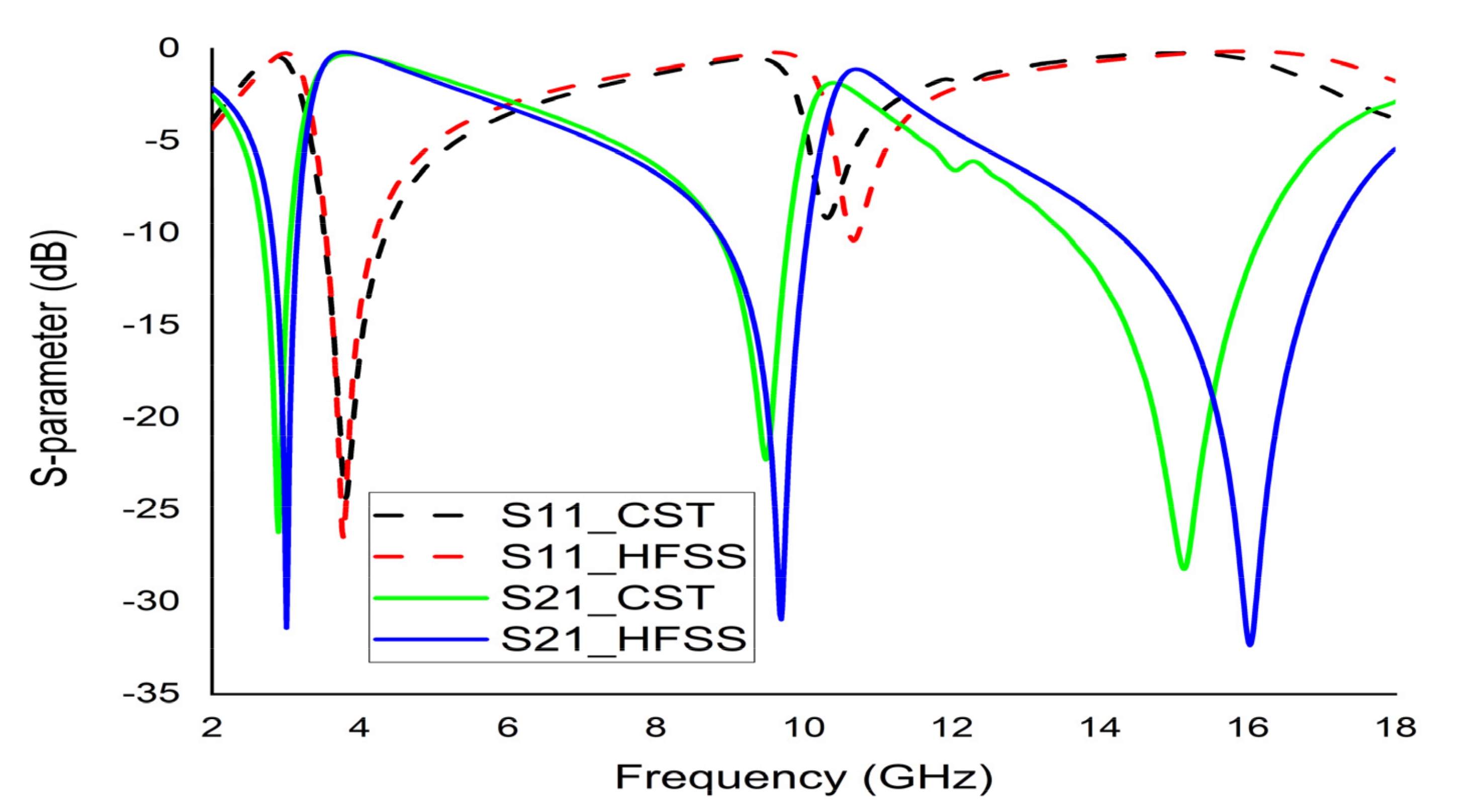
7. Results and Discussion
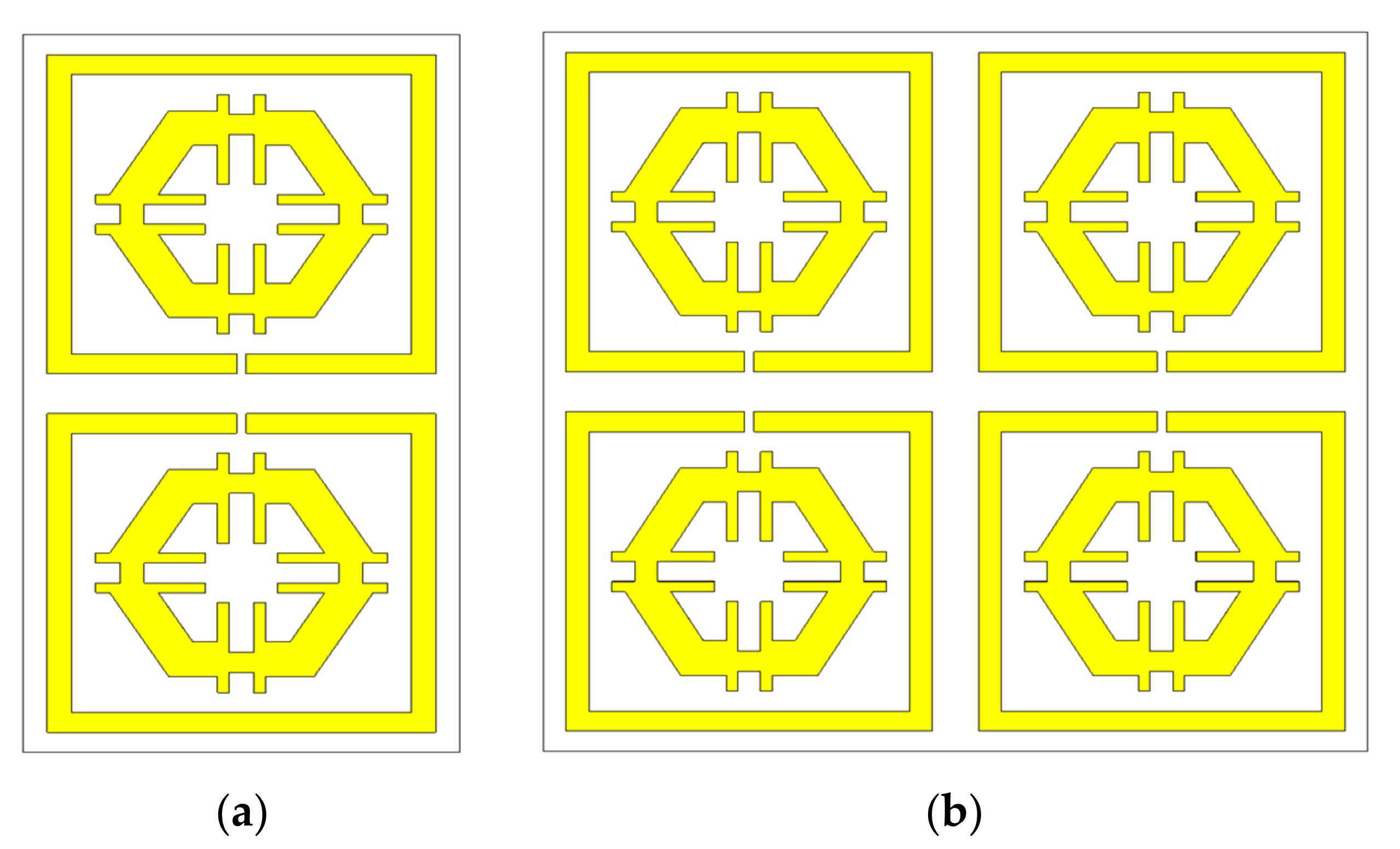
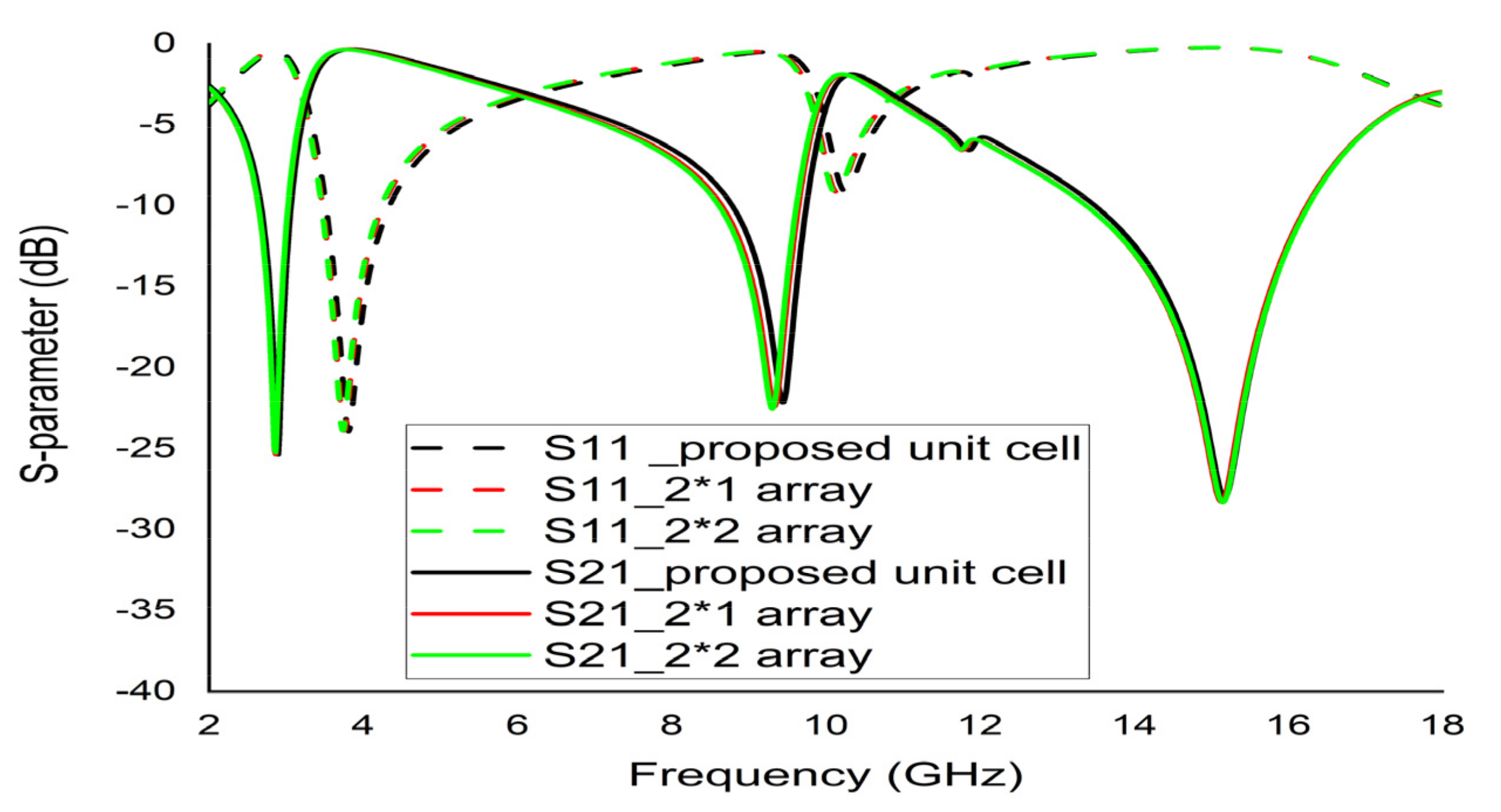
Array Structure
8. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Sikder, S.I.; Faruque, M.R.I.; Islam, M.T. A New Double Negative Metamaterial for C-Band Microwave Applications. Adv. Mater. Res. 2014, 974, 33–37. [Google Scholar] [CrossRef]
- Suzuki, T.; Harumi, A. Reflectionless zero refractive index metasurface in the terahertz waveband. Opt. Express 2020, 28, 21509–21521. [Google Scholar] [CrossRef] [PubMed]
- Islam, S.S.; Faruque, M.R.I.; Islam, M.T. An object-independent ENZ metamaterial-based wideband electromagnetic cloak. Sci. Rep. 2016, 6, 33624. [Google Scholar] [CrossRef] [Green Version]
- Hossain, M.J.; Faruque, M.R.I.; Islam, M.T. Effective medium ratio obeying wideband left-handed miniaturized meta-atoms for multi-band applications. J. Electron. Mater. 2018, 47, 1859–1870. [Google Scholar] [CrossRef]
- Hossain, M.J.; Faruque, M.R.I.; Islam, M.T. Correction: Perfect metamaterial absorber with high fractional bandwidth for solar energy harvesting. PLoS ONE 2019, 14, e0211751. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Emadi, R.; Reza, S.A.; Zeidaabadi, N. Plasmonic cloaking for irregular inclusions using an epsilon-near-zero region composed of a graphene–silica stack. JOSA B 2018, 35, 643–651. [Google Scholar] [CrossRef]
- Sabah, C.; Nesimoglu, T. Design and characterization of a resonator-based metamaterial and its sensor application using microstrip technology. Opt. Eng. 2016, 55, 027107. [Google Scholar] [CrossRef]
- Dalgac, S.; Karadağ, F.; Ünal, E.; Özkaner, V.; Bakır, M.; Akgöl, O.; Sevim, U.K.; Delihacıoğlu, K.; Öztürk, M.; Karaaslan, M.; et al. Metamaterial sensor application concrete material reinforced with carbon steel fiber. Mod. Phys. Lett. B 2020, 34, 2050097. [Google Scholar] [CrossRef]
- Veselago, V.G. The Electrodynamics of Substances with Simultaneously Negative Values of ε and μ. Phys. Uspekhi 1968, 10, 509–514. [Google Scholar] [CrossRef]
- Pendry, J.B.; Holden, A.; Stewart, W.; Youngs, I. Extremely low frequency plasmons in metallic mesostructures. Phys. Rev. Lett. 1996, 76, 4773. [Google Scholar] [CrossRef] [Green Version]
- Enoch, S.G.; Tayeb, G.; Pierre, S.N.; Guérin, P.V. A metamaterial for directive emission. Phys. Rev. Lett. 2002, 89, 213902. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hossain, M.J.; Faruque, M.R.I.; Islam, M.T. Design and analysis of a new composite double negative metamaterial for multi-band communication. Curr. Appl. Phys. 2017, 17, 931–939. [Google Scholar] [CrossRef]
- Zarghooni, B.; Abdolmehdi, D.; Tayeb, A.D. Greek-key pattern as a miniaturized multiband metamaterial unit-cell. IEEE Antennas Wirel. Propag. Lett. 2015, 14, 1254–1257. [Google Scholar] [CrossRef]
- Smith, K.L.; Adams, R.S. Spherical spiral metamaterial unit cell for negative permeability and negative permittivity. IEEE Trans. Antennas Propag. 2018, 66, 6425–6428. [Google Scholar] [CrossRef]
- Shelby, R.A.; Smith, D.R.; Seldon, S. Experimental verification of a negative index of refraction. Science 2001, 292, 77–79. [Google Scholar] [CrossRef] [Green Version]
- Priyanka, A. A Review paper on metamaterial. Int. J. Eng. Sci. Res. Technol. 2014, 3, 612–618. [Google Scholar]
- Islam, S.S.; Faruque, M.R.I.; Islam, M.T. An ENG metamaterial based wideband electromagnetic cloak. Microw. Opt. Technol. Lett. 2016, 58, 2522–2525. [Google Scholar] [CrossRef]
- Islam, M.S.; Samsuzzaman, M.; Beng, G.K.; Misran, N.; Amin, N.; Islam, M.T. A Gap Coupled Hexagonal Split Ring Resonator Based Metamaterial for S-Band and X-Band Microwave Applications. IEEE Access 2020, 8, 68239–68253. [Google Scholar] [CrossRef]
- Moniruzzaman, M.; Islam, M.T.; Islam, M.R.; Misran, N.; Samsuzzaman, M. Coupled ring split ring resonator (CR-SRR) based epsilon negative metamaterial for multiband wireless communications with high effective medium ratio. Results Phys. 2020, 18, 103248. [Google Scholar] [CrossRef]
- Almutairi, A.F.; Islam, M.S.; Samsuzzaman, M.; Islam, M.T.; Misran, N.; Islam, M.T. A complementary split ring resonator based metamaterial with effective medium ratio for C-band microwave applications. Results Phys. 2019, 15, 102675. [Google Scholar] [CrossRef]
- Hasan, M.M.; Faruque, M.R.I. Left-handed metamaterial using Z-shaped SRR for multiband application by azimuthal angular rotations. Mater. Res. Express 2017, 4, 045801. [Google Scholar] [CrossRef]
- Wartak, M.S.; Tsakmakidis, K.L.; Hess, O. Introduction to metamaterials. Phys. Can. 2011, 67, 30–34. [Google Scholar]
- Ahamed, E.; Faruque, M.R.I.; Mansor, M.F.B.; Islam, M.T. Polarization-dependent tunneled metamaterial structure with enhanced fields properties for X-band application. Results Phys. 2019, 15, 102530. [Google Scholar] [CrossRef]
- Pendry, J.B.; Holden, A.J.; Robbins, D.J.; Stewart, W. Magnetism from conductors and enhanced nonlinear phenomena. IEEE Trans. Microw. Theory Tech. 1999, 47, 2075–2084. [Google Scholar] [CrossRef] [Green Version]
- Dawar, P.; De, A. Bandwidth enhancement of RMPA using ENG metamaterials at THz. In Proceedings of the 4th International Conference on Computer and Communication Technology (ICCCT), Allahabad, India, 20–22 September 2013. [Google Scholar]
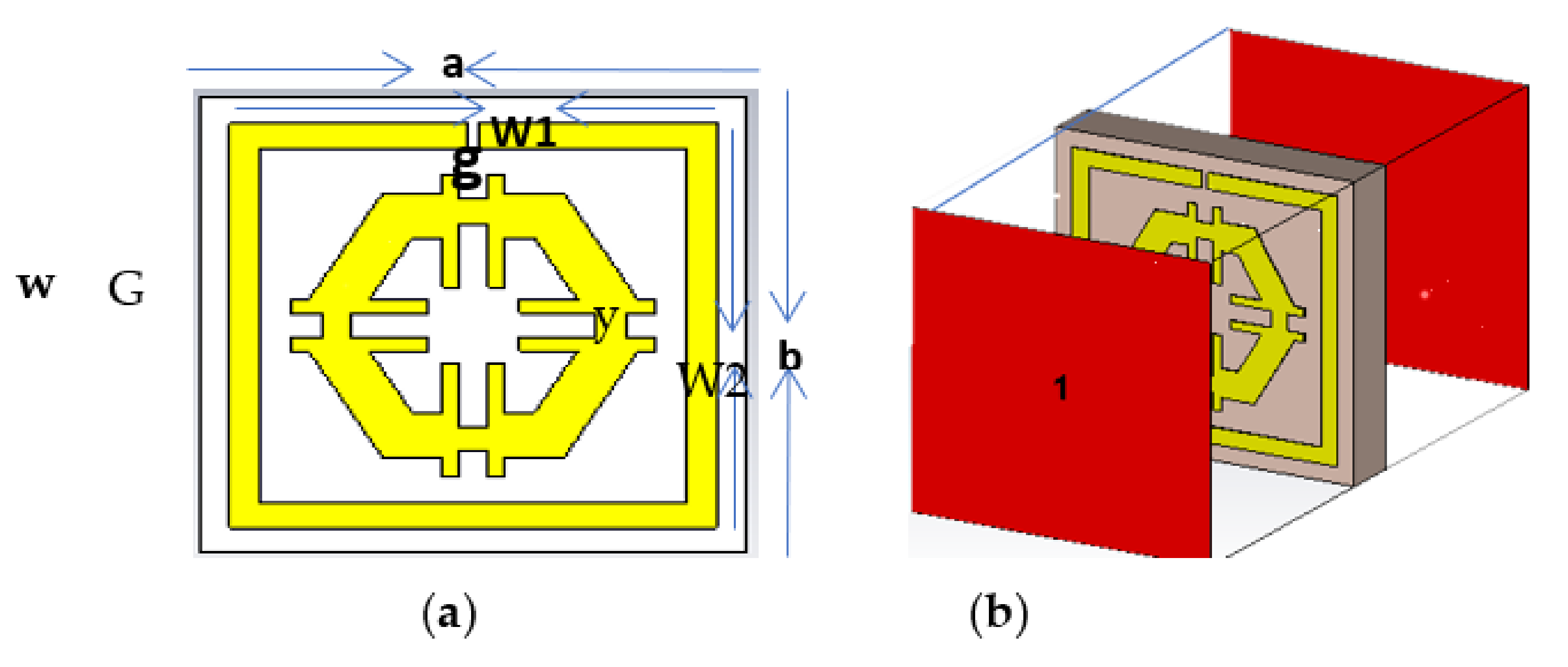



| Parameter | Dimension (mm) | Parameter Dimension (mm) |
|---|---|---|
| a | 9 | G 0.50 |
| b | 9 | g 0.20 |
| W1 | 8 | W 0.50 |
| W2 | 8 | y 1 |
| Subdesign | Resonance Frequency (GHz) | Types Structure | Resonance Apex (dB) | Covering Bands |
|---|---|---|---|---|
| Design-1 | 2.9, 10 | SSR | −27.44, −26.19 | S, X |
| Design-2 | 3, 9.8, 17.33 | SSR+ Hexagon | −25.76, −20.83, −28.28 | S, X, Ku |
| Design-3 | 2.89, 10, 17.2 | Modified hexagon | −27.4, −30, −29 | S, X, Ku |
| Proposed design | 2.89, 9.42, 15.16 | Formatted hexagon | −25, −22.24,−28 | S, X, Ku |
| Parameter | Frequency (GHz) | Extracted Property |
|---|---|---|
| Transmission coefficient, S21 | 2.89, 9.42, 15.16 | S21 < −20 dB |
| Permittivity, εr | 2.9, 9.54,15.36 | εr < 0 |
| Permeability, µr | 2.86, 9.47, 15.60 | µr ≈ 0 |
| Refractive index, n | 2.90, 9.60, 15.47 | n < 0 |
| Types of Simulation | Unit Cell | 2 × 1 Array | 2 × 2 Array | Max. Shift of Frequency (%) |
|---|---|---|---|---|
| 1st resonance (GHz) | 2.89 | 2.87 | 2.87 | 0.69 |
| 2nd resonance (GHz) | 9.42 | 9.32 | 9.29 | 1.3 |
| 3rd resonance (GHz) | 15.16 | 15.12 | 15.14 | 0.26 |
| Author Name | Design Shape | Dimension (mm3) | Metamaterial Type | EMR (λ/L) |
|---|---|---|---|---|
| Mallik et.al. | U | 25 × 25 × 1 | LHM | 1.99 |
| Zhou Z et.al. | Modified H | 9 × 9 × 1 | Chiral | 3.50 |
| Islam et.al | H | 30 × 30 × 1.6 | LHM | 3.65 |
| Hossain et.al. | G | 12 × 12 × 1.6 | NIM | 11.90 |
| Faruque et.al. | SSR | 10 × 10 × 1.6 | LHM | 9.10 |
| Hossain et.al. | C | 12 × 12 × 1.6 | NM | 7.44 |
| Hasan et.al. | Modified G | 10 × 10 × 1.6 | SNM | 3.98 |
| Proposed metamaterial | Formatted hexagon | 9 × 9 × 1.6 | ENG | 11.53 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Afsar, S.U.; Faruque, M.R.I.; Hossain, M.J.; Khandaker, M.U.; Osman, H.; Alamri, S. Modified Hexagonal Split Ring Resonator Based on an Epsilon-Negative Metamaterial for Triple-Band Satellite Communication. Micromachines 2021, 12, 878. https://doi.org/10.3390/mi12080878
Afsar SU, Faruque MRI, Hossain MJ, Khandaker MU, Osman H, Alamri S. Modified Hexagonal Split Ring Resonator Based on an Epsilon-Negative Metamaterial for Triple-Band Satellite Communication. Micromachines. 2021; 12(8):878. https://doi.org/10.3390/mi12080878
Chicago/Turabian StyleAfsar, Salah Uddin, Mohammad Rashed Iqbal Faruque, Mohammad Jakir Hossain, Mayeen Uddin Khandaker, Hamid Osman, and Sultan Alamri. 2021. "Modified Hexagonal Split Ring Resonator Based on an Epsilon-Negative Metamaterial for Triple-Band Satellite Communication" Micromachines 12, no. 8: 878. https://doi.org/10.3390/mi12080878
APA StyleAfsar, S. U., Faruque, M. R. I., Hossain, M. J., Khandaker, M. U., Osman, H., & Alamri, S. (2021). Modified Hexagonal Split Ring Resonator Based on an Epsilon-Negative Metamaterial for Triple-Band Satellite Communication. Micromachines, 12(8), 878. https://doi.org/10.3390/mi12080878









