Polishing Characteristics of Cemented Carbide Using Cubic Boron Nitride Magnetic Abrasive Powders
Abstract
1. Introduction
2. Experiment Procedures
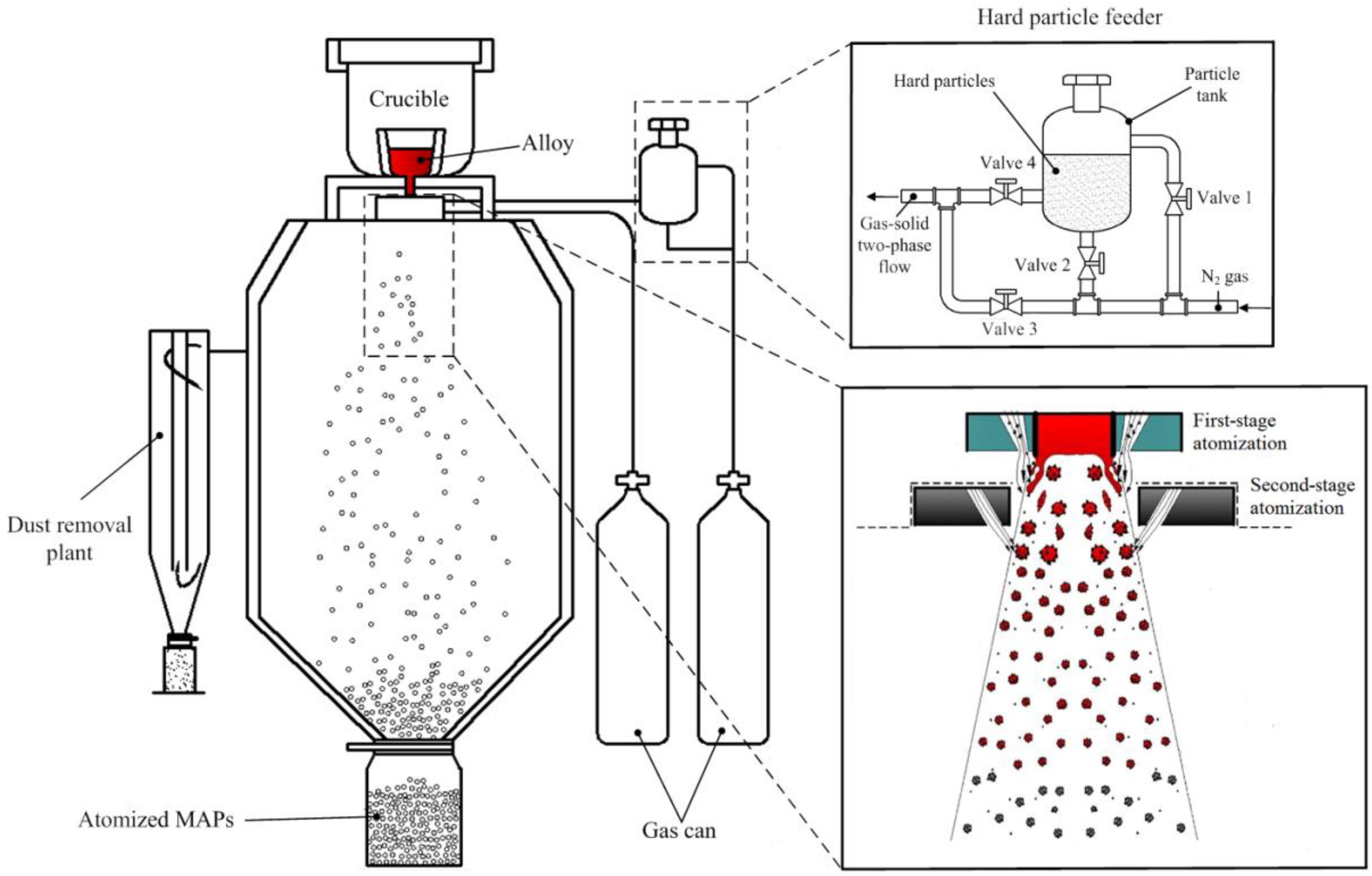
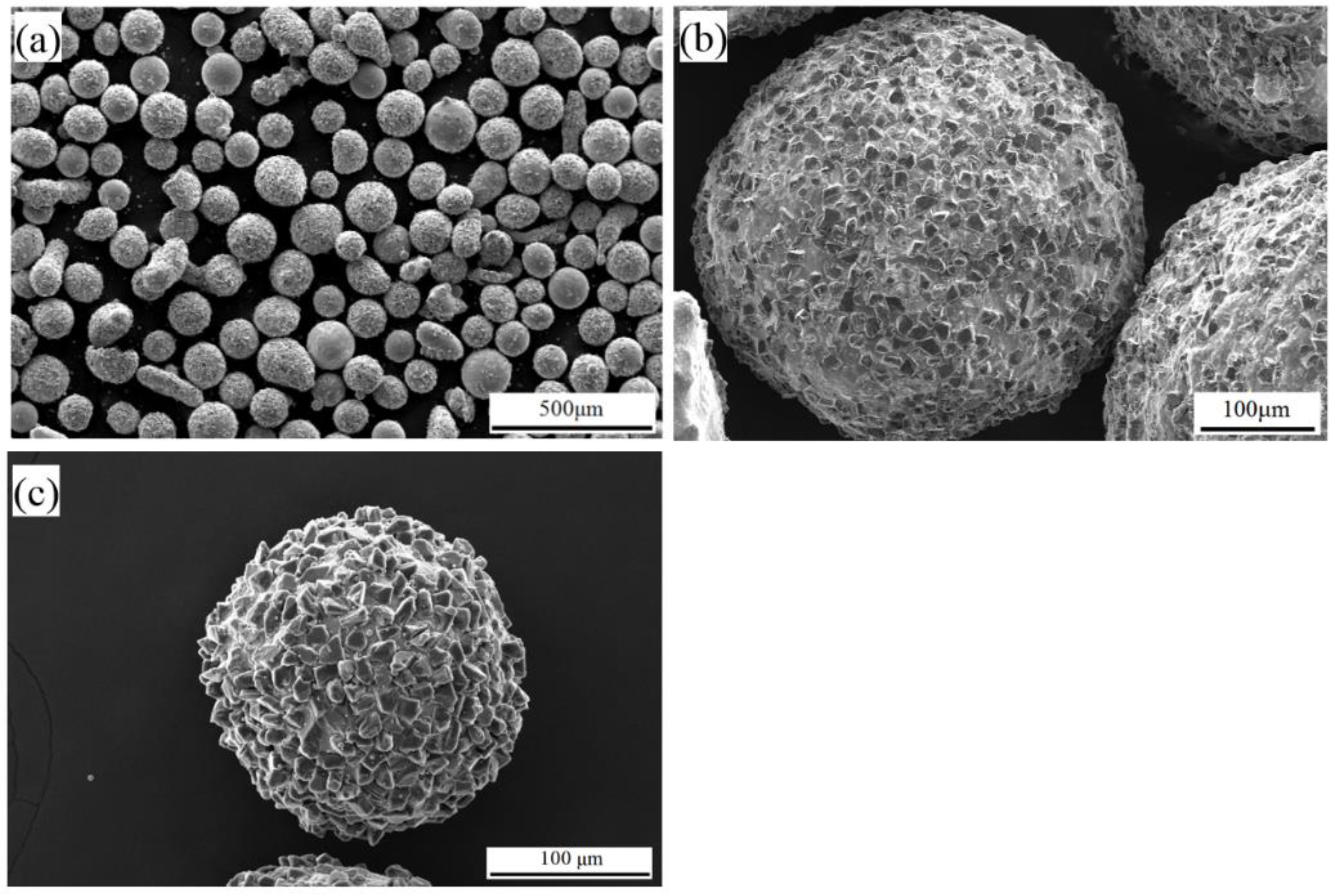
2.1. Preparation of MAP
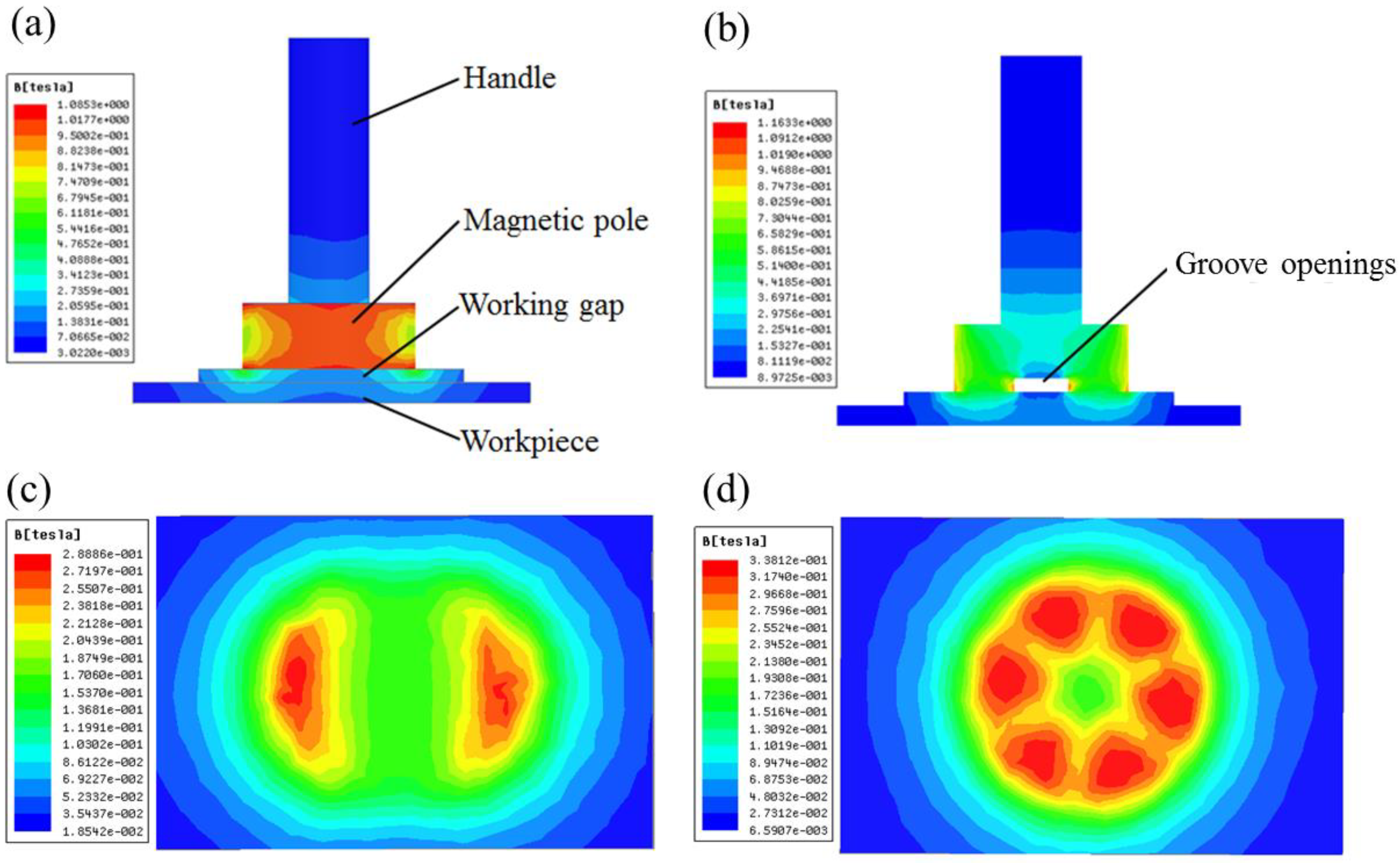
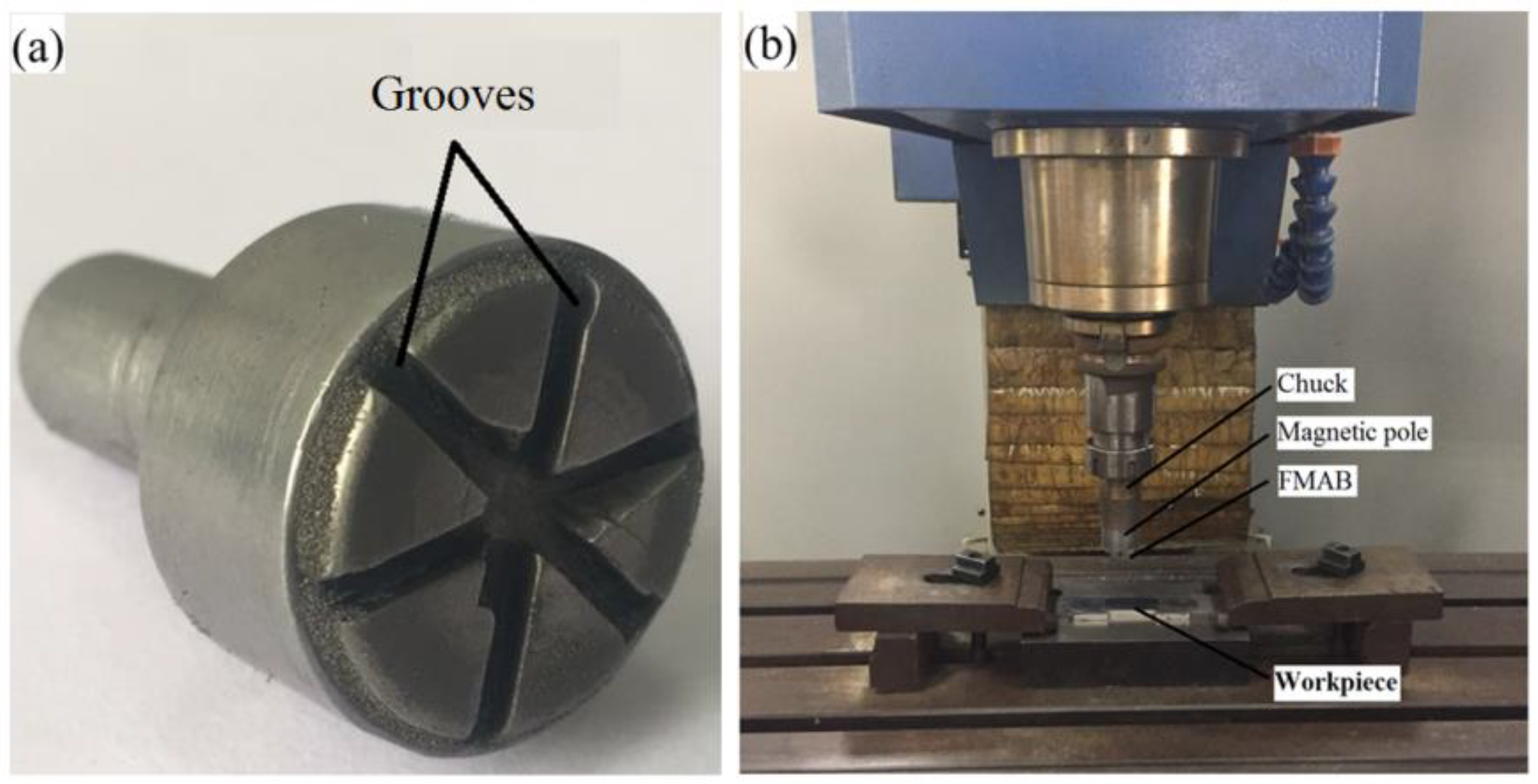
2.2. Magnetic Tool Design
2.3. Experimental Setup
2.4. Workpiece Preparation
3. Experiment Details
4. Results and Discussions
4.1. Statistical Model of %ΔRa
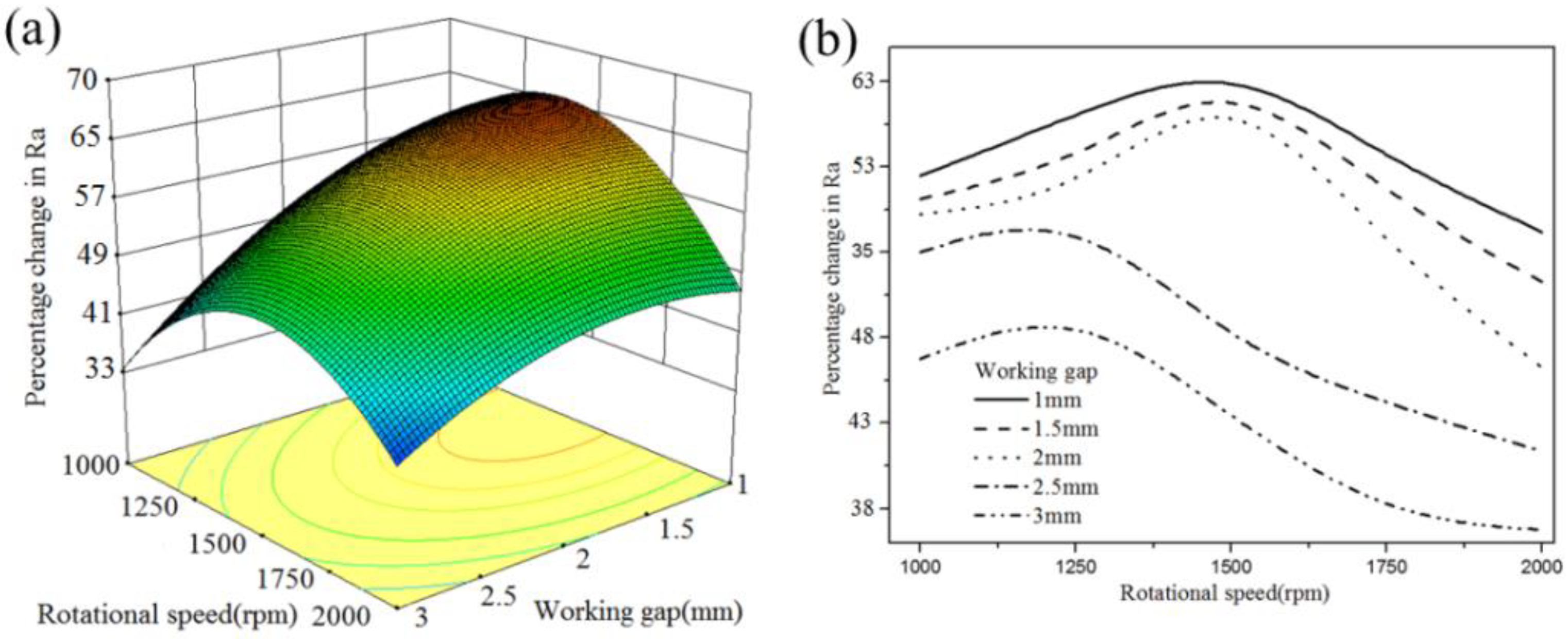
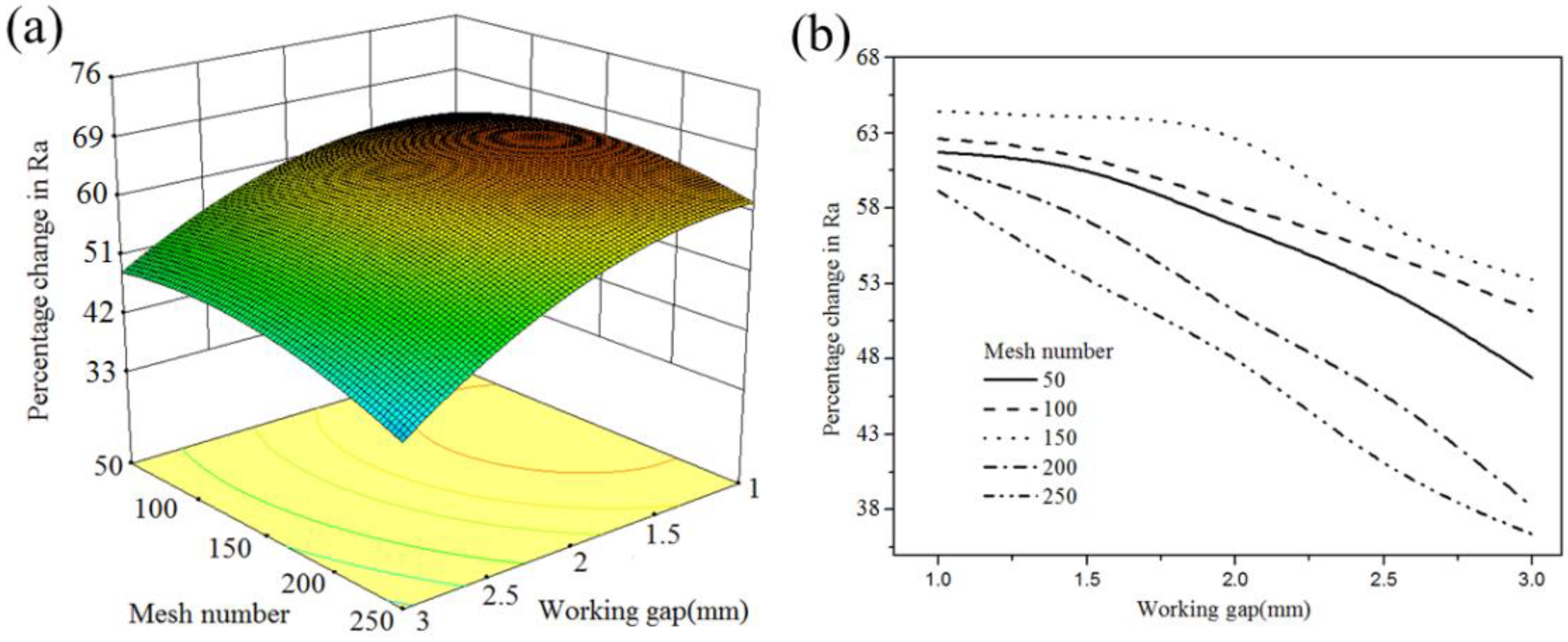
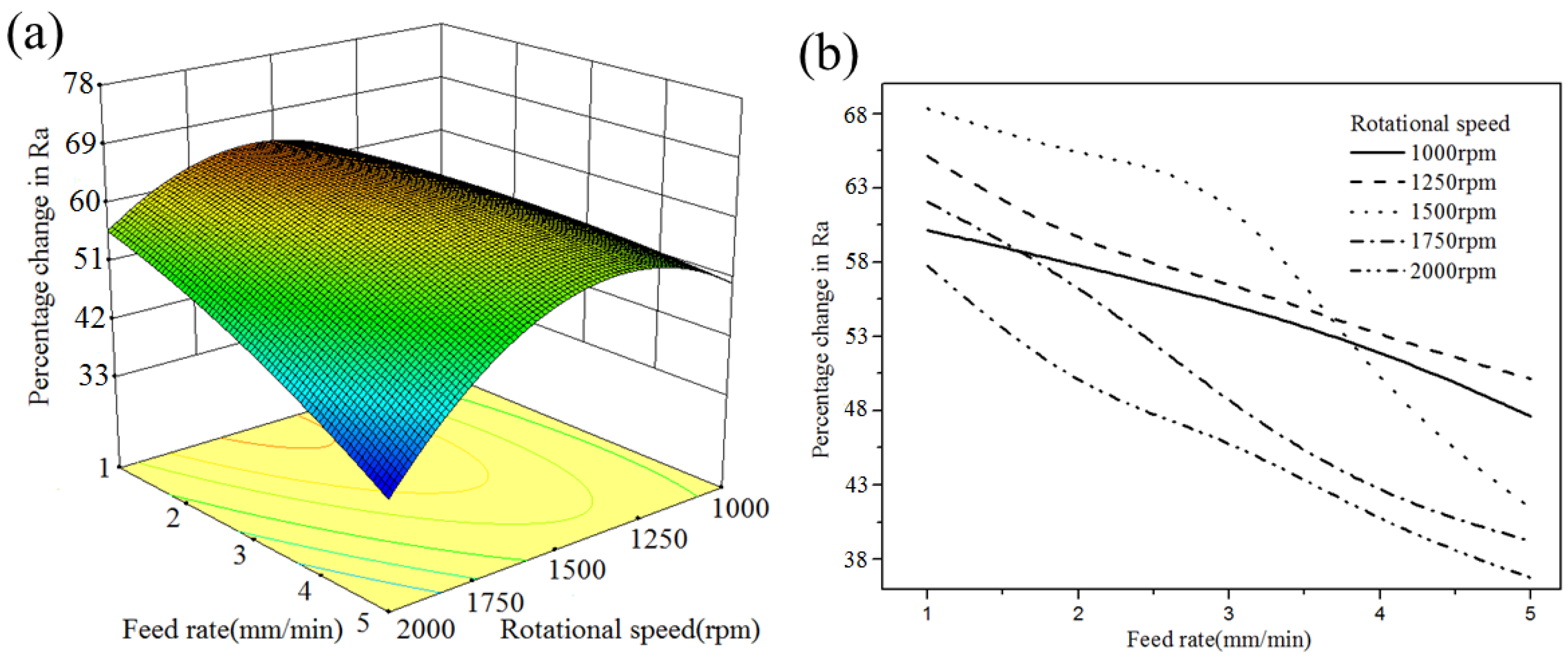
4.2. Interaction Effects of Process Parameters
4.3. Validation Test
4.4. Surface Quality of Cemented Carbide
4.5. Comparison of Polishing Performance of MAPs
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kansal, H.; Singh, A.K.; Grover, V. Magnetorheological nano-finishing of diamagnetic material using permanent magnets tool. Precis. Eng. 2018, 51, 30–39. [Google Scholar] [CrossRef]
- Jha, S.; Jain, V. Design and development of the magnetorheological abrasive flow finishing (MRAFF) process. Int. J. Mach. Tools Manuf. 2004, 44, 1019–1029. [Google Scholar] [CrossRef]
- Fan, Z.H.; Tian, Y.B.; Zhou, Q.; Shi, C.H. Enhanced magnetic abrasive finishing of Ti–6Al–4V using shear thickening fluids addi-tives. Precis. Eng. 2020, 64, 300–306. [Google Scholar] [CrossRef]
- Guo, C.; Zhang, D.; Li, X.; Liu, J.; Li, F. A permanent magnet tool in ultrasonic assisted magnetic abrasive finishing for 30CrMnSi grooves part. Precis. Eng. 2021, 72, 417–425. [Google Scholar] [CrossRef]
- Singh, G.; Kumar, H.; Kansal, H.K.; Sharma, K.; Kumar, R.; Chohan, J.S.; Singh, S.; Sharma, S.; Li, C.H.H.; Krolczyk, G.; et al. Multi-objective optimization of chemically assisted magnetic abrasive finishing (MAF) on Inconel 625 tubes using genetic algo-rithm: Modeling and microstructural analysis. Micromachines 2022, 13, 1168. [Google Scholar] [CrossRef]
- Sooraj, V.; Radhakrishnan, V. Fine finishing of internal surfaces using elastic abrasives. Int. J. Mach. Tools Manuf. 2014, 78, 30–40. [Google Scholar] [CrossRef]
- Jain, V.; Kumar, R.; Dixit, P.; Sidpara, A. Investigations into abrasive flow finishing of complex workpieces using FEM. Wear 2009, 267, 71–80. [Google Scholar] [CrossRef]
- Qian, C.; Fan, Z.; Tian, Y.; Liu, Y.; Han, J.; Wang, J. A review on magnetic abrasive finishing. Int. J. Adv. Manuf. Technol. 2021, 112, 619–634. [Google Scholar] [CrossRef]
- Kala, P.; Pandey, P.M. Comparison of finishing characteristics of two paramagnetic materials using double disc magnetic abrasive finishing. J. Manuf. Process. 2015, 17, 63–77. [Google Scholar] [CrossRef]
- Kala, P.; Pandey, P.M. Experimental study on finishing forces in double disk magnetic abrasive finishing process while fin-ishing paramagnetic workpiece. Proc. Mater. Sci. 2014, 5, 1677–1684. [Google Scholar] [CrossRef]
- Verma, G.C.; Kala, P.; Pandey, P.M. Experimental investigations into internal magnetic abrasive finishing of pipes. Int. J. Adv. Manuf. Technol. 2017, 88, 1657–1668. [Google Scholar] [CrossRef]
- Kajal, S.; Jain, V.K.; Ramkumar, J.; Nagdeve, L. Experimental and theoretical investigations into internal magnetic abrasive fin-ishing of a revolver barrel. Int. J. Adv. Manuf. Technol. 2017, 100, 1105–1122. [Google Scholar] [CrossRef]
- Mulik, R.S.; Pandey, P.M. Magnetic abrasive finishing of hardened AISI 52100 steel. Int. J. Adv. Manuf. Technol. 2011, 55, 501–515. [Google Scholar] [CrossRef]
- Mulik, R.S.; Pandey, P.M. Ultrasonic assisted magnetic abrasive finishing of hardened AISI 52100 steel using unbonded SiC abrasives. Int. J. Refract. Met. Hard Mater. 2011, 29, 68–77. [Google Scholar] [CrossRef]
- Lin, C.; Yang, L.; Chow, H. Study of magnetic abrasive finishing in free-form surface operations using the Taguchi method. Int. J. Adv. Manuf. Technol. 2007, 34, 122–130. [Google Scholar] [CrossRef]
- Wang, A.C.; Lee, S.J. Study the characteristics of magnetic finishing with gel abrasive. Int. J. Mach. Tools Manuf. 2009, 49, 1063–1069. [Google Scholar] [CrossRef]
- Hanada, K.; Yamaguchi, H. Development of Spherical Iron-Based Composite Powder with Carried Alumina Abrasive Grains by Plasma Spray. Adv. Mater. Res. 2009, 75, 43–46. [Google Scholar] [CrossRef]
- Zhang, G.-X.; Zhao, Y.-G.; Zhao, D.-B.; Yin, F.-S.; Zhao, Z.-D. Preparation of white alumina spherical composite magnetic abrasive by gas atomization and rapid solidification. Scr. Mater. 2011, 65, 416–419. [Google Scholar] [CrossRef]
- Gao, Y.; Zhao, Y.; Zhang, G. Preparation of Al2O3 magnetic abrasives by gas-solid two-phase double-stage atomization and rapid solidification. Mater. Lett. 2018, 215, 300–304. [Google Scholar] [CrossRef]
- Gao, Y.; Zhao, Y.; Zhang, G.; Zhang, G.; Yin, F. Polishing of paramagnetic materials using atomized magnetic abrasive powder. Mater. Manuf. Process. 2019, 34, 604–611. [Google Scholar] [CrossRef]
- Yang, L.-D.; Lin, C.-T.; Chow, H.-M. Optimization in MAF operations using Taguchi parameter design for AISI304 stainless steel. Int. J. Adv. Manuf. Technol. 2009, 42, 595–605. [Google Scholar] [CrossRef]
- Shinmura, T.; Takazawa, K.; Hatano, E.; Matsunaga, M.; Matsuo, T. Study on magnetic abrasive finishing. CIRP Ann. Manuf. Technol. 1990, 39, 325–328. [Google Scholar] [CrossRef]
- Zhao, Z.D.; Huang, H.Y.; Zhao, Y.G.; Yu, X.J. Research on preparation and properties of magnetic abrasive by conventional sol-id-state method. Key Eng. Mater 2009, 416, 553–557. [Google Scholar] [CrossRef]
- Liu, G.; Zhao, Y.; Meng, J.; Gao, Y.; Song, Z.; Zhang, X.; Liu, Q.; Cao, C. Preparation of Al2O3 magnetic abrasives by combining plasma molten metal powder with sprayed abrasive powder. Ceram. Int. 2022, 48, 21612–21619. [Google Scholar] [CrossRef]
- Li, W.; Li, J.; Cheng, B.; Zhang, X.J.; Song, Q.; Wang, Y.; Zhang, T.; Seniuts, U.; Belostrkovsky, M. Achieving in-situ alloy-hardening core-shell structured carbonyl iron powders for magnetic abrasive finishing. Mater. Des. 2021, 212, 110198. [Google Scholar]

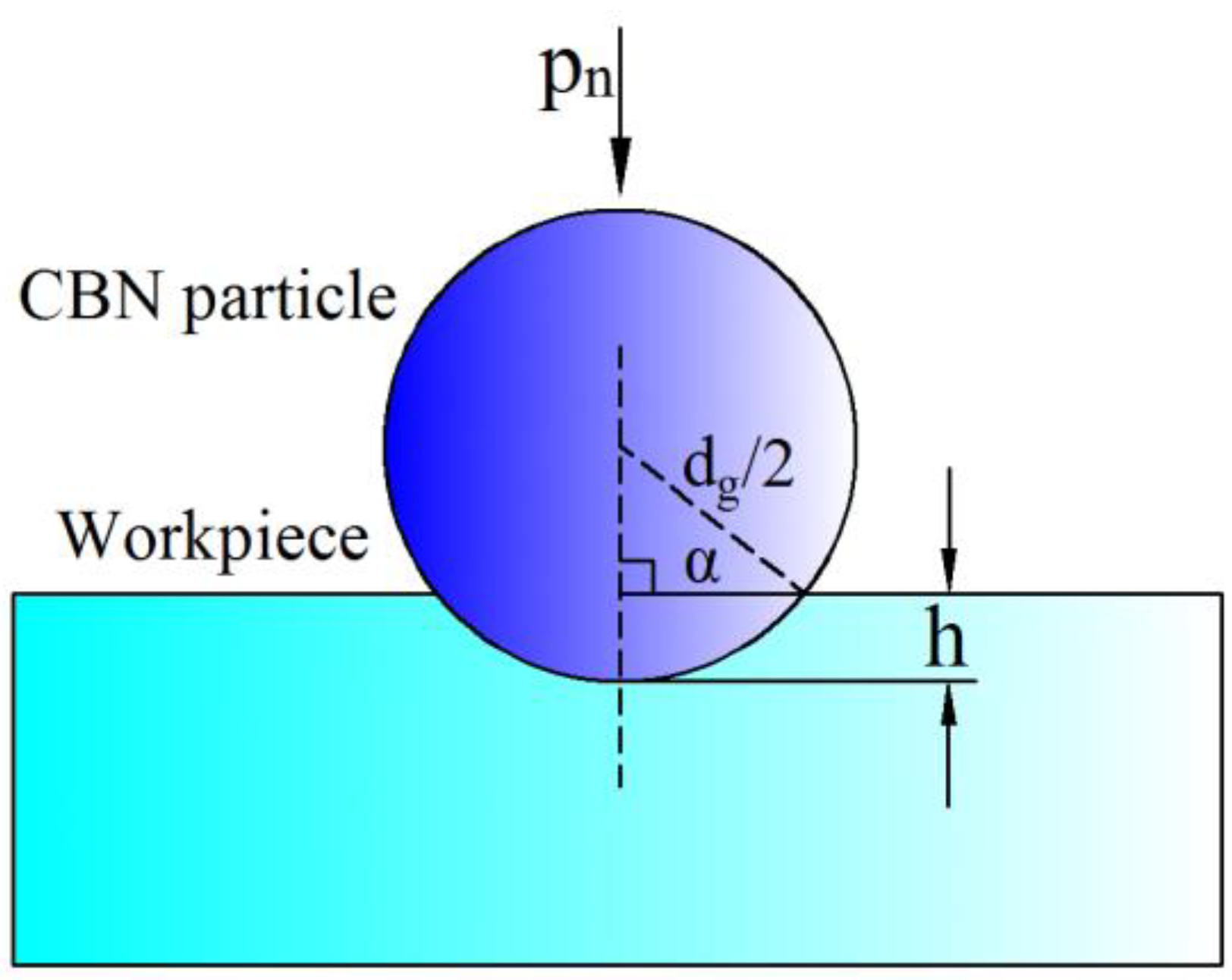
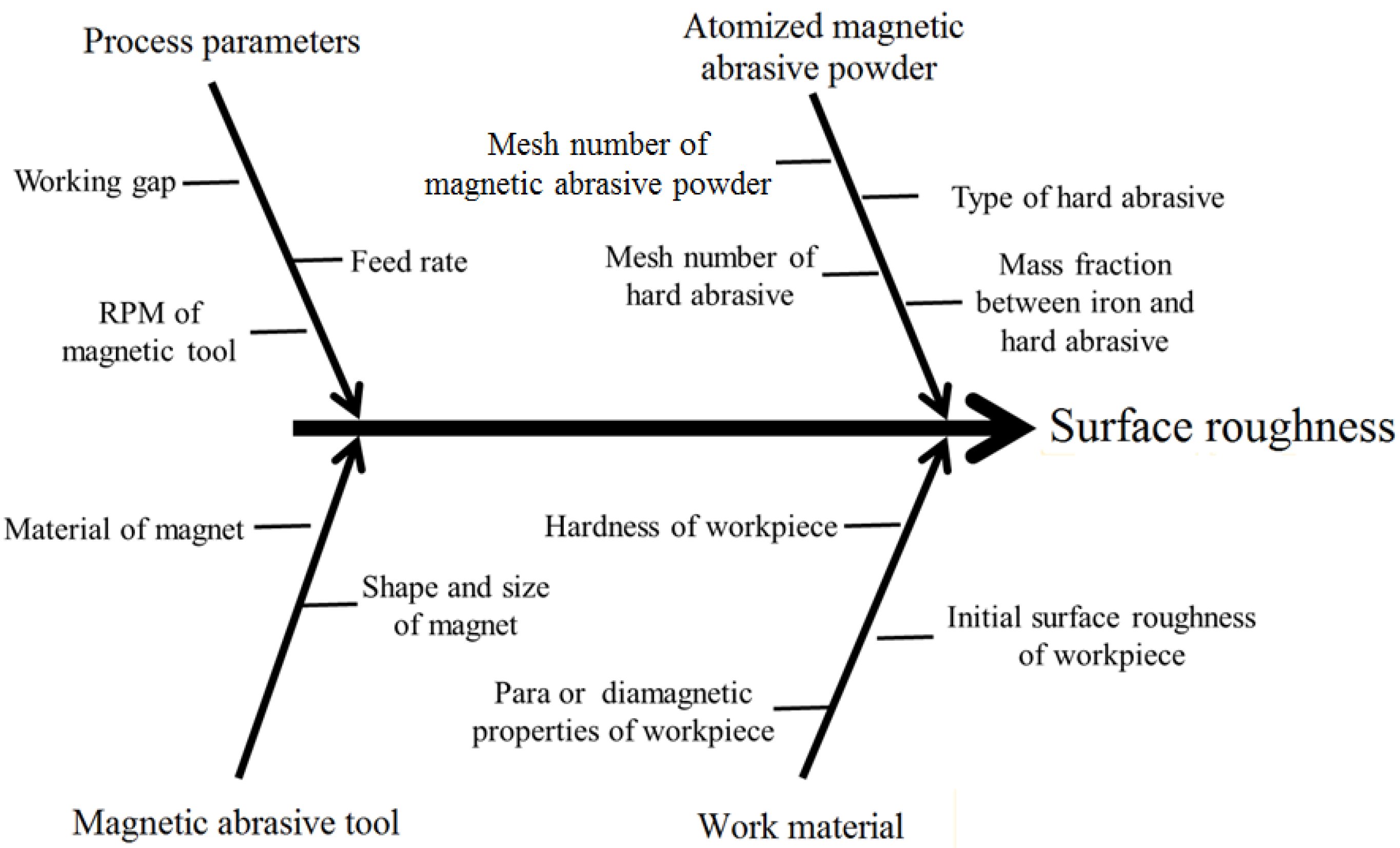




| Model | Dimension | Material | Relative Permeability |
|---|---|---|---|
| Handle | Φ15 mm × H30 mm | Stainless steel | 1 |
| Working gap | 50 mm × 30 mm × 2 mm | Air | 1.0000004 |
| Workpiece | 70 mm × 30 mm × 5 mm | Cemented carbide | M-H curve |
| Magnetic pole | Φ30 mm × H20 mm | N45 | 1.2 |
| Groove openings | Width: 1–3 mm Depth: 1–3 mm Number: 4–8 |
| Hardness | 864 Hv |
|---|---|
| Dimension | 15 mm × 15 mm × 5 mm |
| Alloying element | Wt.% |
| W | 64.45 |
| Ti | 14.55 |
| C | 9.16 |
| O | 5.32 |
| Co Cr | 3.88 2.64 |
| Factors | Parameter | Level | ||||
|---|---|---|---|---|---|---|
| −2 | −1 | 0 | 1 | 2 | ||
| X1 | Working gap (mm) | 1 | 1.5 | 2 | 2.5 | 3 |
| X2 | Rotational speed (rpm) | 1000 | 1250 | 1500 | 1750 | 2000 |
| X3 | Feed rate (mm/min) | 1 | 2 | 3 | 4 | 5 |
| X4 | Mesh number | 50 | 100 | 150 | 200 | 250 |
| Working Gap (mm) | Quality of MAP (g) |
|---|---|
| 1 | 2 |
| 1.5 | 2.5 |
| 2 | 3 |
| 2.5 | 3.5 |
| 3 | 4 |
| Run Order | Working Gap (mm) | RPM | Feed Rate (mm/min) | Mesh Number | Finished Roughness (nm) | |
|---|---|---|---|---|---|---|
| 1 | 2 | 1500 | 1 | 150 | 101.6 | 68.31 |
| 2 | 2 | 1500 | 3 | 150 | 122.2 | 63.86 |
| 3 | 2.5 | 1250 | 4 | 200 | 207.2 | 46.61 |
| 4 | 2.5 | 1750 | 2 | 100 | 165.7 | 54.96 |
| 5 | 1.5 | 1250 | 4 | 200 | 175.2 | 53.54 |
| 6 | 2 | 1000 | 3 | 150 | 155.8 | 55.23 |
| 7 | 2.5 | 1750 | 4 | 200 | 233.6 | 36.7 |
| 8 | 1.5 | 1250 | 2 | 100 | 118.5 | 63.54 |
| 9 | 1.5 | 1250 | 4 | 100 | 183 | 52.96 |
| 10 | 2.5 | 1750 | 4 | 100 | 198.6 | 45.15 |
| 11 | 2 | 2000 | 3 | 150 | 176.8 | 46.27 |
| 12 | 2.5 | 1250 | 2 | 200 | 237.8 | 37.26 |
| 13 | 2 | 1500 | 5 | 150 | 197.3 | 41.44 |
| 14 | 2 | 1500 | 3 | 250 | 198.5 | 48.57 |
| 15 | 1.5 | 1750 | 2 | 200 | 138.7 | 61.7 |
| 16 | 1.5 | 1250 | 2 | 200 | 168.7 | 57.39 |
| 17 | 2.5 | 1250 | 4 | 100 | 183 | 50.8 |
| 18 | 2.5 | 1750 | 2 | 200 | 173.1 | 51.24 |
| 19 | 1.5 | 1750 | 4 | 100 | 197.7 | 52.01 |
| 20 | 1 | 1500 | 3 | 150 | 119.5 | 64.43 |
| 21 | 2 | 1500 | 3 | 50 | 189.7 | 50.73 |
| 22 | 2.5 | 1250 | 3 | 150 | 209.4 | 46.57 |
| 23 | 3 | 1500 | 3 | 150 | 196.3 | 43.26 |
| 24 | 1.5 | 1750 | 2 | 100 | 150 | 61.15 |
| 25 | 1.5 | 1750 | 4 | 200 | 184.4 | 43.6 |
| Source | DF | Seq.SS | MS | F | p | R2 | |
|---|---|---|---|---|---|---|---|
| Regression | 12 | 1885.805 | 134.7 | 20.378 | 0.000 | 95.005% | Model is adequate and lack of fit is insignificant |
| Linear | 4 | 745.57 | |||||
| Square | 4 | 1181.078 | |||||
| Interaction | 4 | 217.623 | |||||
| Residual error | 15 | 99.15 | 6.61 | ||||
| Lack-of-fit | 10 | 89.665 | 2.31 | 0.0502 | |||
| Pure error | 5 | 9.486 | |||||
| Total | 27 | 1984.957 |
| Order | Process Parameters | Ra | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Original Ra (nm) | Finished Ra (nm) | Predicted Values | Experimental Observation | % Error | |||||
| 1 | 1 | 2000 | 1 | 200 | 348.2 | 140.3 | 64.05 | 59.7 | 7.28 |
| 2 | 1.5 | 1000 | 5 | 50 | 316.5 | 130 | 55.33 | 58.93 | 6.1 |
| 3 | 2 | 1750 | 4 | 100 | 365.7 | 205.8 | 47.33 | 43.72 | 8.25 |
| 4 | 2.5 | 1500 | 3 | 150 | 374 | 173.2 | 57.57 | 53.69 | 7.23 |
| 5 | 3 | 1250 | 2 | 250 | 355.3 | 156.5 | 59.95 | 55.95 | 7.15 |
| R1 | 1 | 1638 | 1 | 150 | 346.1 | 71 | 74.35 | 79.49 | 6.47 |
| R2 | 1 | 1638 | 1 | 150 | 325 | 93.8 | 74.35 | 71.14 | 4.5 |
| R3 | 1 | 1638 | 1 | 150 | 302.6 | 57.6 | 74.35 | 80.96 | 8.15 |
| Preparation Technology | MAPs | Workpiece Material | Original Ra | Finished Ra | |
|---|---|---|---|---|---|
| Mixing [3] | Fe/Al2O3 | Ti-6Al-4V | 1.121 μm | 0.046 μm | 95.9% |
| Sintering [23] | Fe/Al2O3 | Steel718 | 2.8 μm | 0.39 μm | 86% |
| Gel [16] | Fe/SiC | SKD11 | 0.65 μm | 0.110 μm | 83% |
| Plasma sprayed [24] | Fe/Al2O3 | SS316 | 0.299 μm | 0.068 μm | 77% |
| Alloy-Hardening [25] | CI-MAPs | Zr-alloy | 0.361 μm | 0.085 μm | 76.5% |
| Present work | Fe/CBN | cemented carbide | 0.3 μm | 0.0576 μm | 80.8% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, P.; Gao, Y.; Zhao, Y.; Zhao, G.; Zhang, G.; Zhang, H.; Song, Z. Polishing Characteristics of Cemented Carbide Using Cubic Boron Nitride Magnetic Abrasive Powders. Micromachines 2022, 13, 2167. https://doi.org/10.3390/mi13122167
Chen P, Gao Y, Zhao Y, Zhao G, Zhang G, Zhang H, Song Z. Polishing Characteristics of Cemented Carbide Using Cubic Boron Nitride Magnetic Abrasive Powders. Micromachines. 2022; 13(12):2167. https://doi.org/10.3390/mi13122167
Chicago/Turabian StyleChen, Pengfei, Yuewu Gao, Yugang Zhao, Guoyong Zhao, Guixiang Zhang, Haiyun Zhang, and Zhuang Song. 2022. "Polishing Characteristics of Cemented Carbide Using Cubic Boron Nitride Magnetic Abrasive Powders" Micromachines 13, no. 12: 2167. https://doi.org/10.3390/mi13122167
APA StyleChen, P., Gao, Y., Zhao, Y., Zhao, G., Zhang, G., Zhang, H., & Song, Z. (2022). Polishing Characteristics of Cemented Carbide Using Cubic Boron Nitride Magnetic Abrasive Powders. Micromachines, 13(12), 2167. https://doi.org/10.3390/mi13122167





