Dynamics of Tri-Hybrid Nanoparticles in the Rheology of Pseudo-Plastic Liquid with Dufour and Soret Effects
Abstract
:1. Introduction
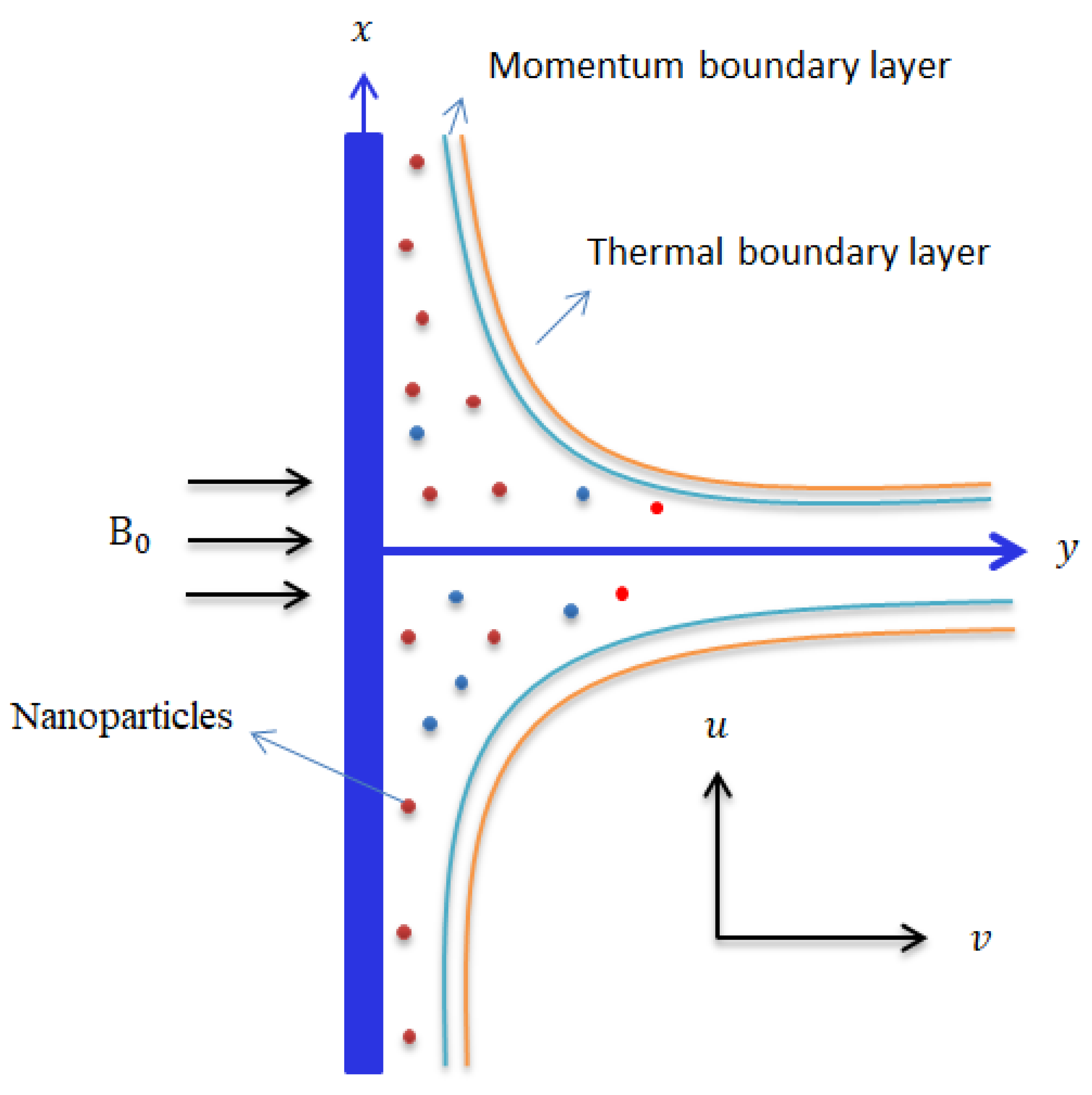
2. Description of Constructing Model
- Two-dimensional flow of the pseudo-plastic material is considered;
- Fourier’s law and Fick’s law are assumed;
- Chemical reaction and heat generation are addressed;
- Transfer of heat is characterized in the presence of the Dufour and Soret effects;
- Viscous dissipation is removed;
- Darcy–Forchheimer porous theory is analyzed, and the vertical surface is plotted in Figure 2.
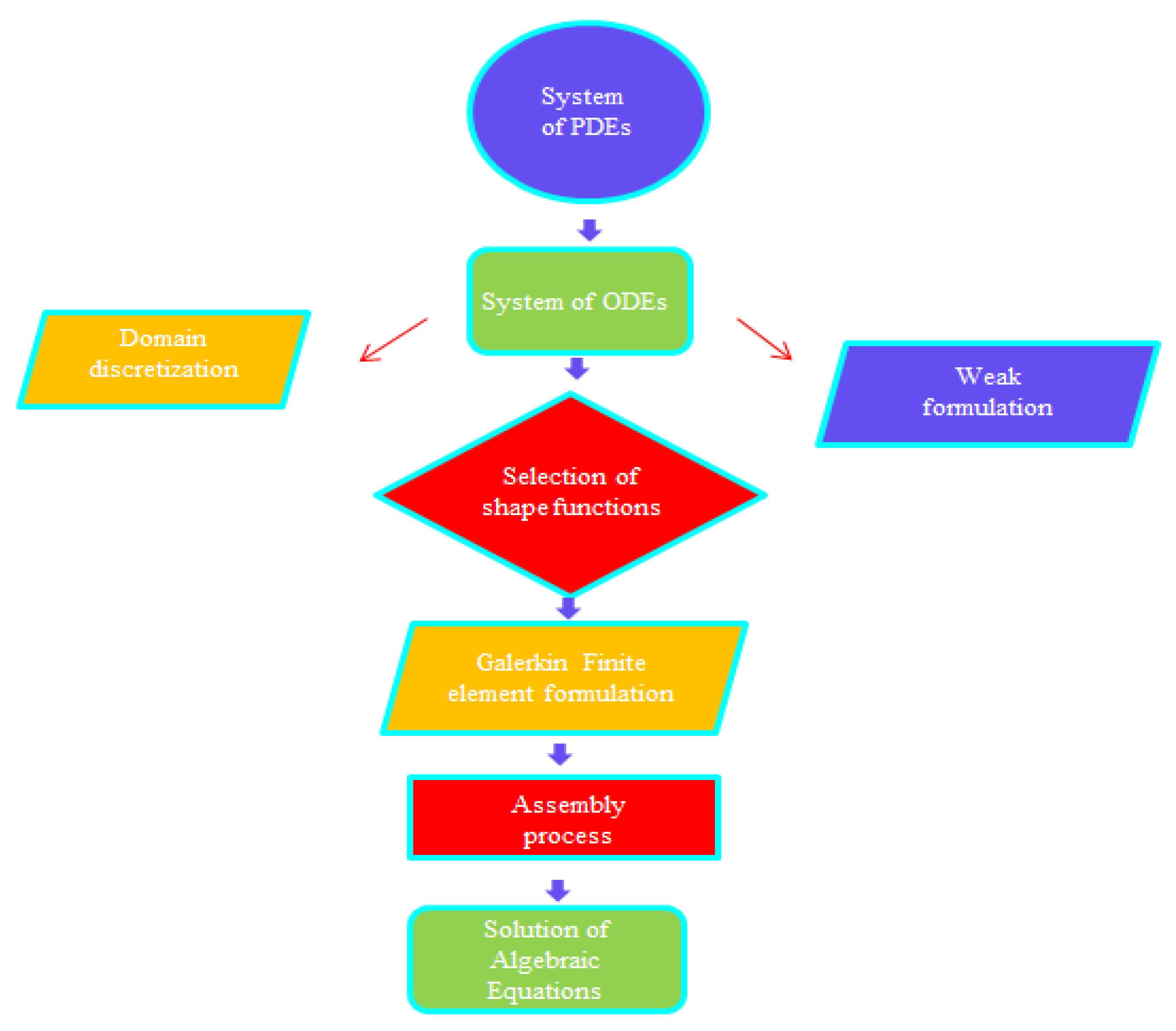
3. Numerical Scheme
- ⮚
- Complex type geometries are easily tackled using the finite element method;
- ⮚
- Physical problems in applied science are numerically solved by finite element formulation (FEM);
- ⮚
- FEM needs a low level of investment in view of time and resources;
- ⮚
- An important role of FEM is to simulate various types of boundary conditions;
- ⮚
- It has the ability to perform discretization regarding derivatives.
3.1. Domain Discretization
3.2. Choice of Shape Function
3.3. Residuals
3.4. Assembly Approach
3.5. Testing of Error Analysis and Mesh Free Analysis
3.6. Validation of Numerical Results
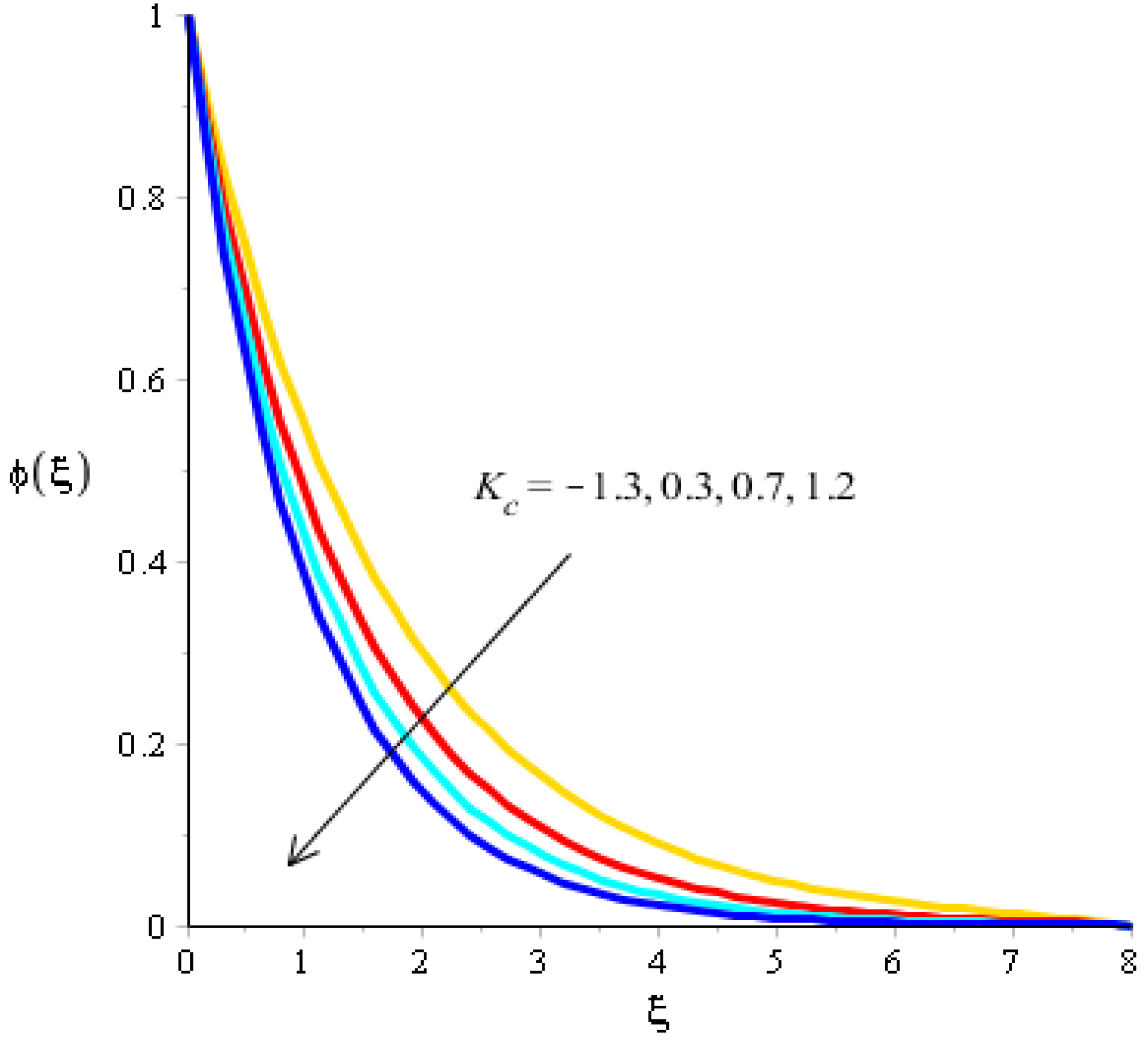
4. Results and Discussion
4.1. Analysis Related to Motion into Particles
4.2. Analysis Related to Thermal Energy into Particles
4.3. Analysis Related to Mass Species
5. Conclusions
- Confirmation of convergence analysis occurred at 270 elements.
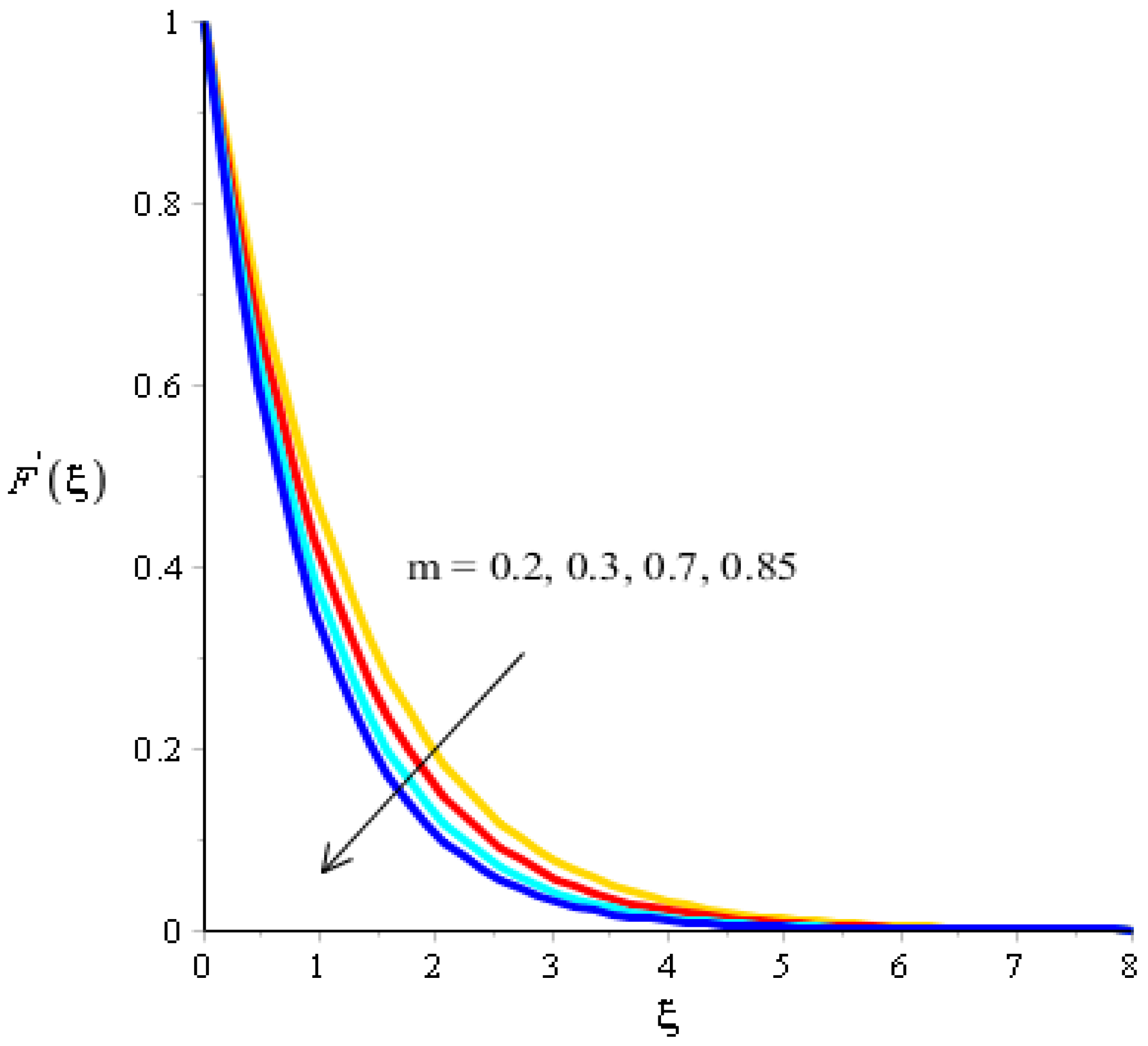
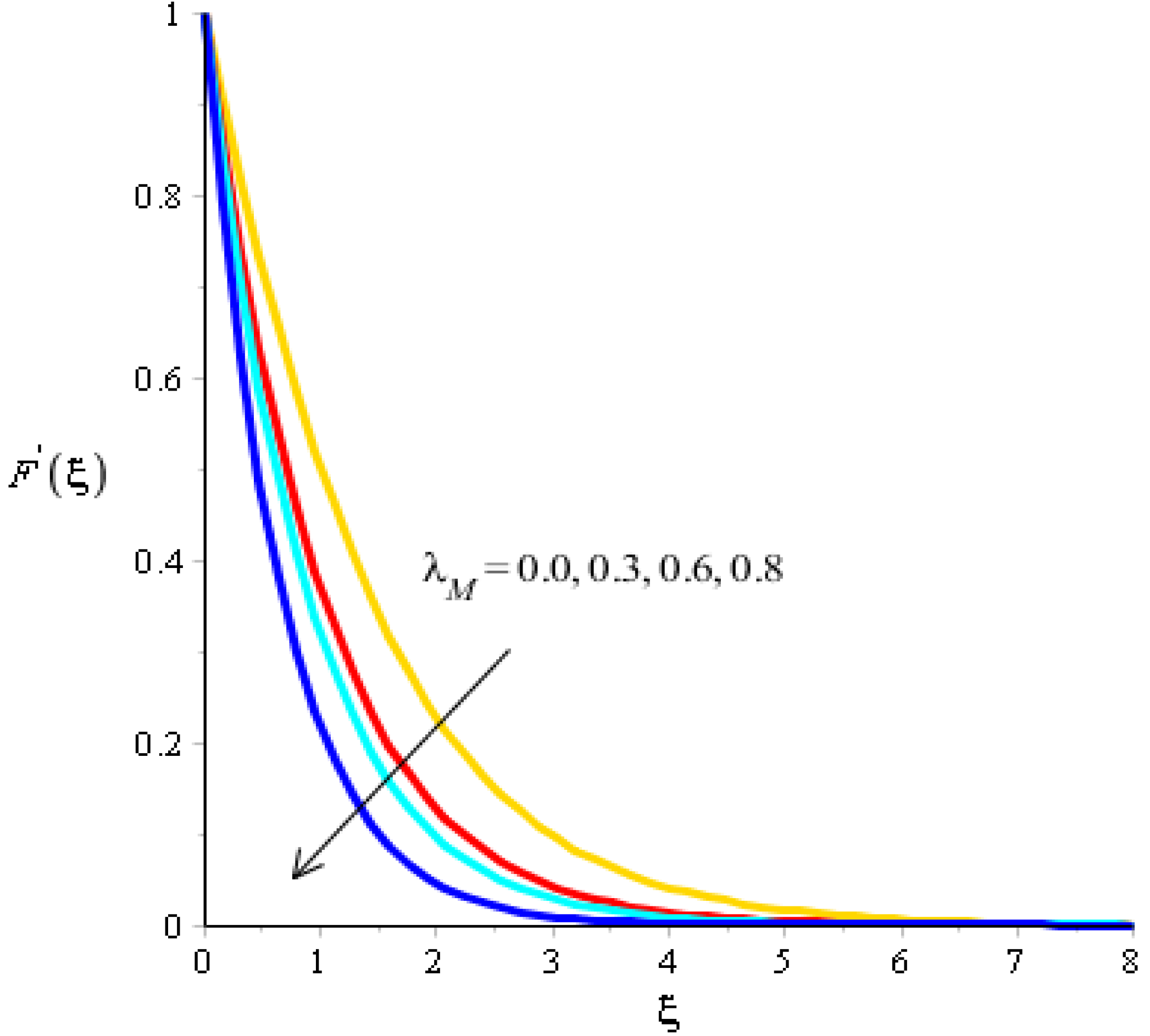
- Forchheimer number, power law number, and caused a decline in the thickness of the momentum boundary layer. However, an increment was investigated in the flow versus argument values of the bouncy parameter (
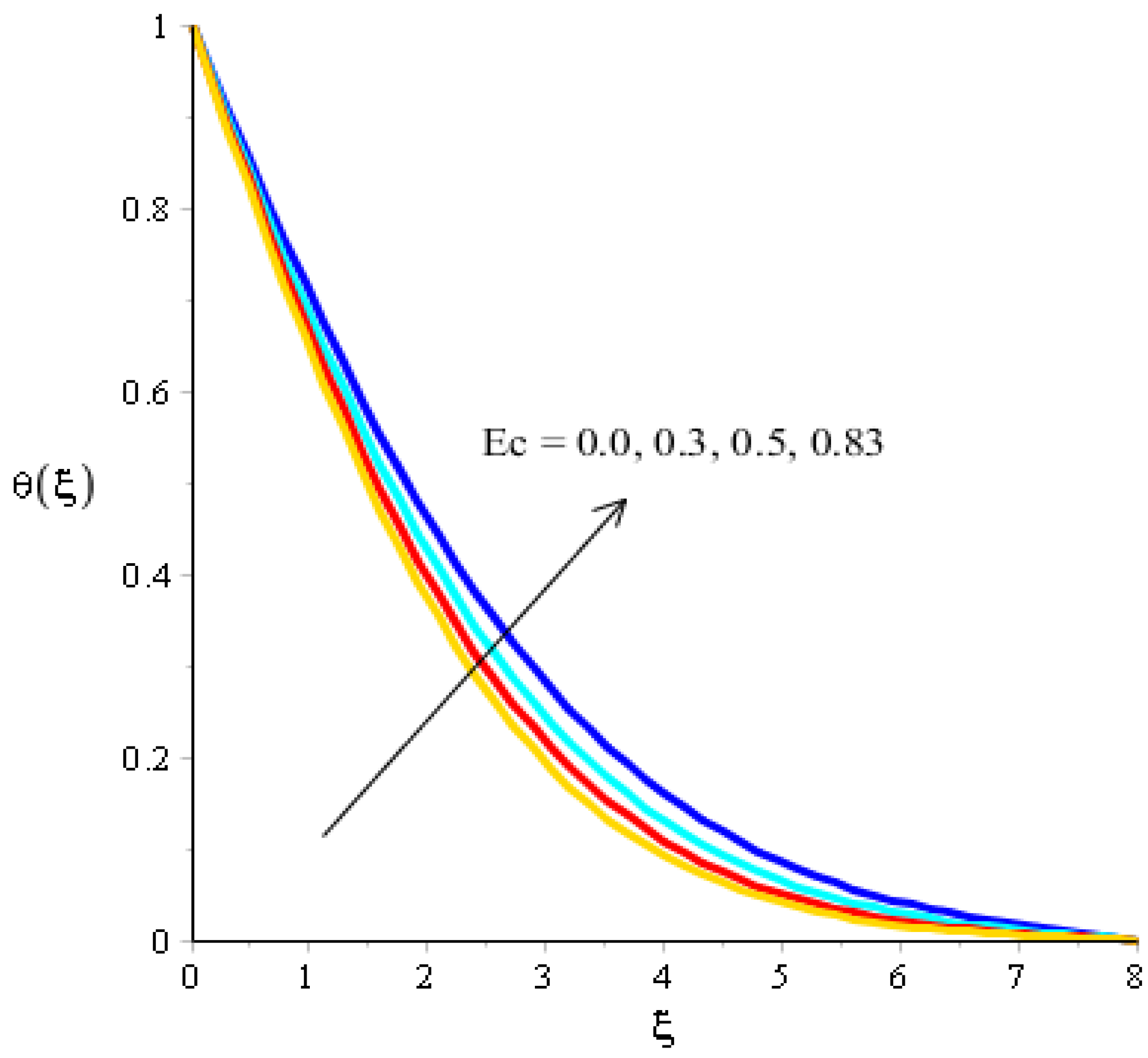
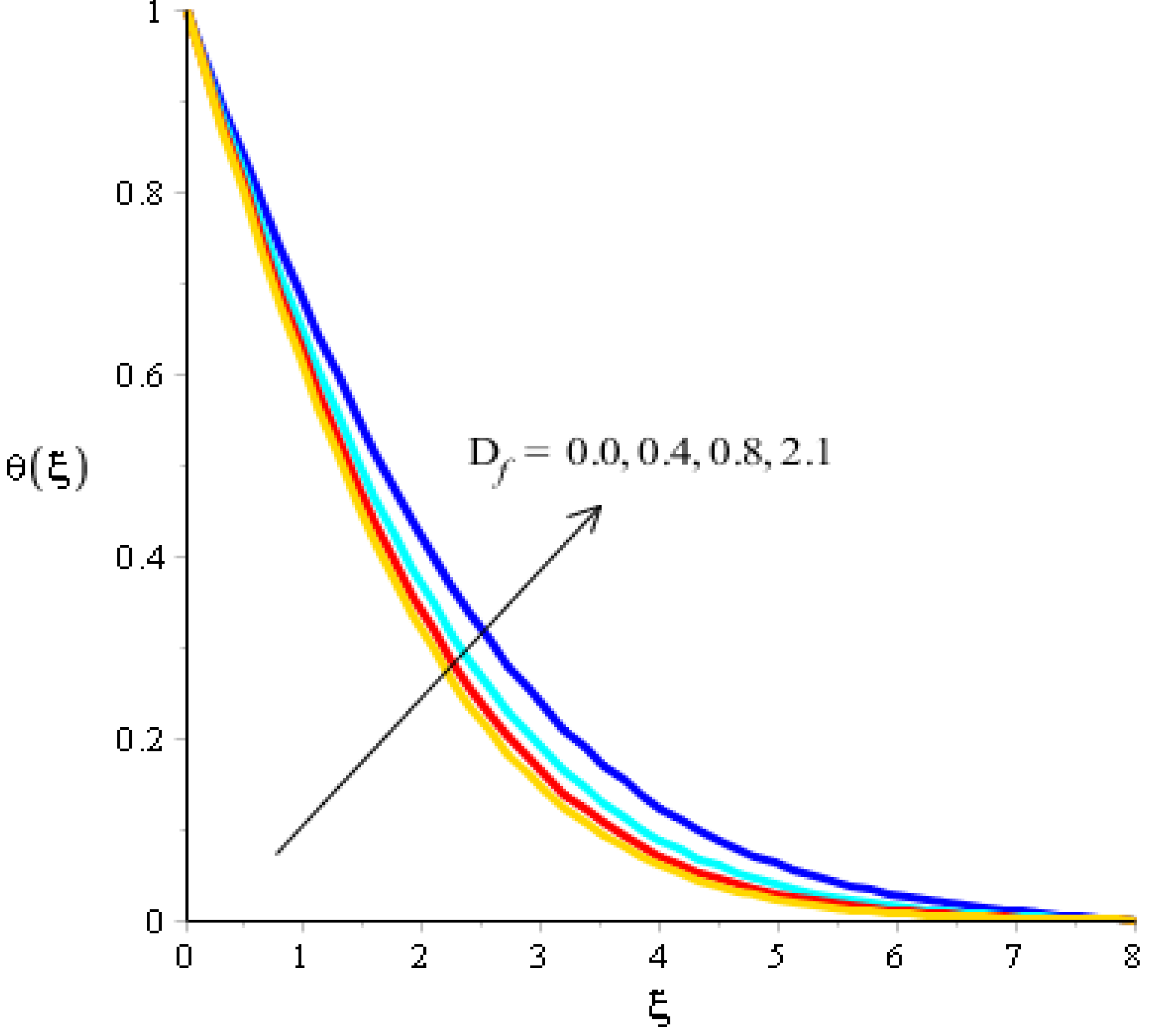
- Temperature distribution was maximized versus higher impacts of the Eckert number, heat generation, and the Dufour number while the thickness associated with thermal layers was increased.
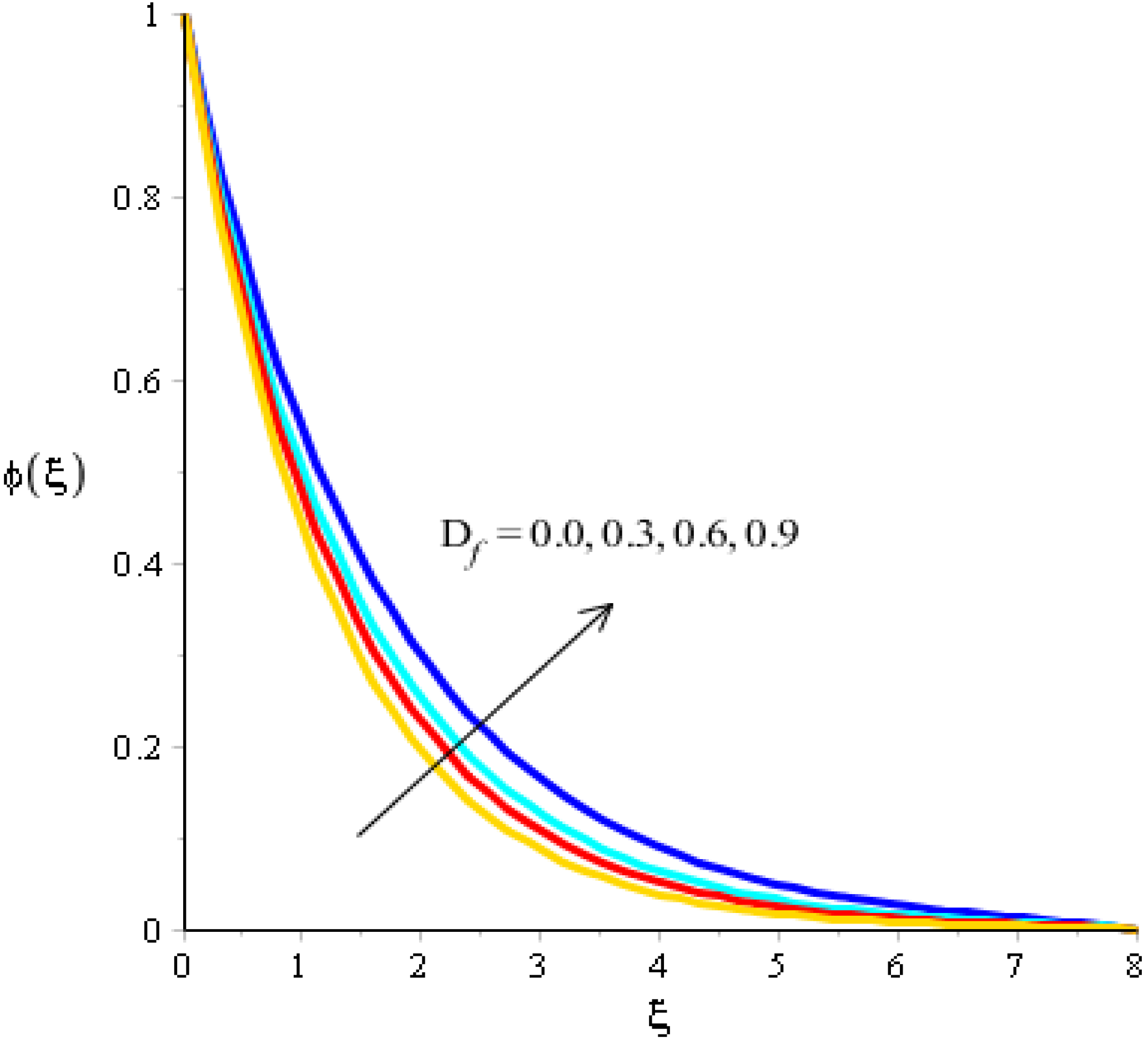
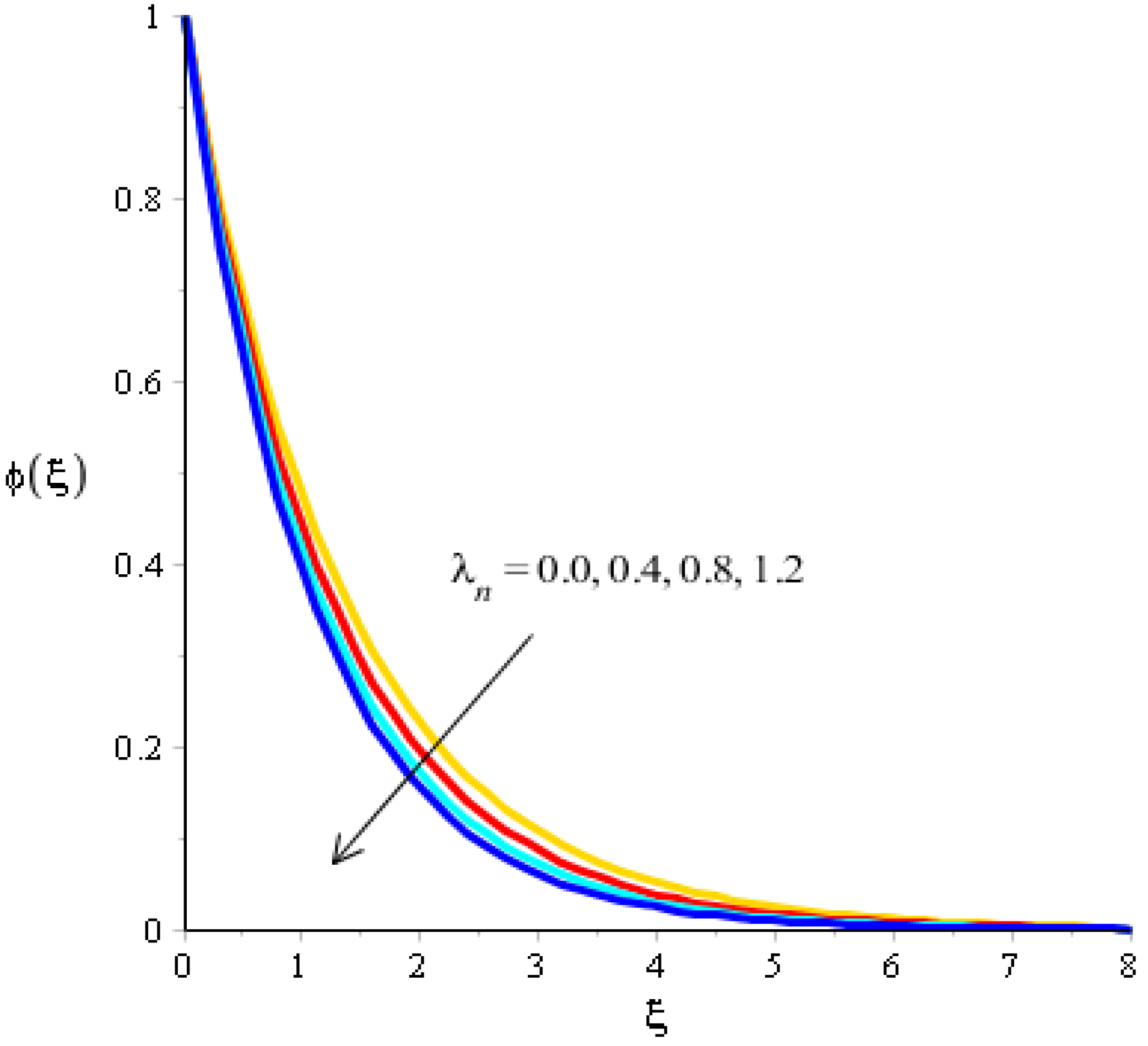
- The concentration field was decreased against the argument values of the Schmidt number, chemical reaction number, and bouncy number, whereas the concentration field was enhanced against higher values of the Dufour number.
- The approach of utilizing ternary hybrid nanoparticles was found to be a significant factor in obtaining maximum thermal energy.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Symbols | Used for | Symbols | Used for |
| Power law number | |||
| Gravitational acceleration | |||
| BCs | Boundary conditions | ||
| Independent variable | ODEs | Ordinary differential equations | |
| Dimensionless velocity | Dimensionless temperature | ||
| Dimensionless concentration | Volume fraction | ||
| Porosity number | Bouncy number | ||
| Bouncy number | Prandtl number | ||
| Eckert number | Dufour number | ||
| Heat generation number | Schmidt number | ||
| Chemical reaction number | Soret number | ||
| Skin friction coefficient | Nusselt number | ||
| Tri-hybrid nanoparticles | Reynolds number | ||
| CFD | Computational fluid dynamics | FES | Finite element approach |
| Infinity | |||
| Volume fractions | Residual function | ||
| Shape function | Heat flux | ||
| Consistency coefficient | Shear stress | ||
| Dimensionless velocity field | Hybrid nanofluid | ||
| Nanofluid | Tri-hybrid nanoparticles | ||
| Fluid | Subscripts regarding nanoparticles |
References
- Barnes, H.A.; Hutton, J.F.; Walters, K. An Introduction to Rheology, Rheology Series 3; Elsevier: Amsterdam, The Netherlands, 1989. [Google Scholar]
- Eberhard, U.; Seybold, H.J.; Floriancic, M.; Bertsch, P.; Jiménez-Martínez, J.; Andrade, J.S., Jr.; Holzner, M. Determination of the effective viscosity of non-Newtonian fluids flowing through porous media. Front. Phys. 2019, 7, 71. [Google Scholar] [CrossRef] [Green Version]
- Rosti, M.E.; Takagi, S. Shear-thinning and shear-thickening emulsions in shear flows. Phys. Fluids 2021, 33, 083319. [Google Scholar] [CrossRef]
- Gul, T.; Shah, R.A.; Islam, S.; Ullah, M.; Khan, M.A.; Zaman, A.; Haq, Z. Exact Solution of Two Thin Film Non-Newtonian Immiscible Fluids on a Vertical Belt. J. Basic Appl. Sci. Res. 2014, 4, 283–288. [Google Scholar]
- Hussain, F.; Hussain, A.; Nadeem, S. Thermophoresis and Brownian model of pseudo-plastic nanofluid flow over a vertical slender cylinder. Math. Probl. Eng. 2020, 2020, 8428762. [Google Scholar] [CrossRef]
- Abdelsalam, S.I.; Sohail, M. Numerical approach of variable thermophysical features of dissipated viscous nanofluid comprising gyrotactic micro-organisms. Pramana 2020, 94, 1–12. [Google Scholar] [CrossRef]
- Sohail, M.; Naz, R. Modified heat and mass transmission models in the magnetohydrodynamic flow of Sutterby nanofluid in stretching cylinder. Phys. A Stat. Mech. Its Appl. 2020, 549, 124088. [Google Scholar] [CrossRef]
- Chu, Y.M.; Nazir, U.; Sohail, M.; Selim, M.M.; Lee, J.R. Enhancement in thermal energy and solute particles using hybrid nanoparticles by engaging activation energy and chemical reaction over a parabolic surface via finite element approach. Fractal Fract. 2021, 5, 119. [Google Scholar] [CrossRef]
- Hina, S.; Hayat, T.; Mustafa, M.; Alsaedi, A. Peristaltic transport of pseudoplastic fluid in a curved channel with wall properties and slip conditions. Int. J. Biomath. 2014, 7, 1450015. [Google Scholar] [CrossRef]
- Salahuddin, T.; Malik, M.Y.; Hussain, A.; Bilal, S. Combined effects of variable thermal conductivity and MHD flow on pseudoplastic fluid over a stretching cylinder by using Keller box method. Inf. Sci. Lett. 2016, 5, 2. [Google Scholar] [CrossRef]
- Alam, M.K.; Siddiqui, A.M.; Rahim, M.T.; Islam, S.; Avital, E.J.; Williams, J.J.R. Thin film flow of magnetohydrodynamic (MHD) pseudo-plastic fluid on vertical wall. Appl. Math. Comput. 2014, 245, 544–556. [Google Scholar] [CrossRef]
- Hayat, T.; Iqbal, R.; Tanveer, A.; Alsaedi, A. Variable viscosity effect on MHD peristaltic flow of pseudoplastic fluid in a tapered asymmetric channel. J. Mech. 2018, 34, 363–374. [Google Scholar] [CrossRef]
- Sohail, M.; Ali, U.; Zohra, F.T.; Al-Kouz, W.; Chu, Y.M.; Thounthong, P. Utilization of updated version of heat flux model for the radiative flow of a non-Newtonian material under Joule heating: OHAM application. Open Phys. 2021, 19, 100–110. [Google Scholar] [CrossRef]
- Sohail, M.; Raza, R. Analysis of radiative magneto nano pseudo-plastic material over three dimensional nonlinear stretched surface with passive control of mass flux and chemically responsive species. Multidiscip. Modeling Mater. Struct. 2020, 16, 1061–1083. [Google Scholar] [CrossRef]
- Shafiq, A.; Khan, I.; Rasool, G.; Sherif, E.S.M.; Sheikh, A.H. Influence of single-and multi-wall carbon nanotubes on magnetohydrodynamic stagnation point nanofluid flow over variable thicker surface with concave and convex effects. Mathematics 2020, 8, 104. [Google Scholar] [CrossRef] [Green Version]
- Manjunatha, S.; Puneeth, V.; Gireesha, B.J.; Chamkha, A. Theoretical Study of Convective Heat Transfer in Ternary Nanofluid Flowing past a Stretching Sheet. J. Appl. Comput. Mech. 2021, 1–8. [Google Scholar] [CrossRef]
- Nazir, U.; Sohail, M.; Hafeez, M.B.; Krawczuk, M. Significant Production of Thermal Energy in Partially Ionized Hyperbolic Tangent Material Based on Ternary Hybrid Nanomaterials. Energies 2021, 14, 6911. [Google Scholar] [CrossRef]
- Chen, Z.; Yan, H.; Lyu, Q.; Niu, S.; Tang, C. Ternary hybrid nanoparticles of reduced graphene oxide/graphene-like MoS2/zirconia as lubricant additives for bismaleimide composites with improved mechanical and tribological properties. Compos. Part A Appl. Sci. Manuf. 2017, 101, 98–107. [Google Scholar] [CrossRef]
- Zayan, M.; Rasheed, A.K.; John, A.; Muniandi, S.; Faris, A. Synthesis and Characterization of Novel Ternary Hybrid Nanoparticles as Thermal Additives in H2O. ChemRxiv 2021. [Google Scholar] [CrossRef]
- Shafiq, A.; Mebarek-Oudina, F.; Sindhu, T.N.; Abidi, A. A study of dual stratification on stagnation point Walters’ B nanofluid flow via radiative Riga plate: A statistical approach. Eur. Phys. J. Plus 2021, 136, 1–24. [Google Scholar] [CrossRef]
- Swain, K.; Mebarek-Oudina, F.; Abo-Dahab, S.M. Influence of MWCNT/Fe3O4 hybrid nanoparticles on an exponentially porous shrinking sheet with chemical reaction and slip boundary conditions. J. Therm. Anal. Calorim. 2021, 147, 1561–1570. [Google Scholar] [CrossRef]
- Mebarek-Oudina, F.; Fares, R.; Aissa, A.; Lewis, R.W.; Abu-Hamdeh, N.H. Entropy and convection effect on magnetized hybrid nano-liquid flow inside a trapezoidal cavity with zigzagged wall. Int. Commun. Heat Mass Transf. 2021, 125, 105279. [Google Scholar] [CrossRef]
- Warke, A.S.; Ramesh, K.; Mebarek-Oudina, F.; Abidi, A. Numerical investigation of the stagnation point flow of radiative magnetomicropolar liquid past a heated porous stretching sheet. J. Therm. Anal. Calorim. 2021, 1–12. [Google Scholar] [CrossRef]
- Dadheech, P.K.; Agrawal, P.; Mebarek-Oudina, F.; Abu-Hamdeh, N.H.; Sharma, A. Comparative heat transfer analysis of MoS2/C2H6O2 and SiO2-MoS2/C2H6O2 nanofluids with natural convection and inclined magnetic field. J. Nanofluids 2020, 9, 161–167. [Google Scholar] [CrossRef]
- Marzougui, S.; Mebarek-Oudina, F.; Magherbi, M.; Mchirgui, A. Entropy generation and heat transport of Cu-water nanoliquid in porous lid-driven cavity through magnetic field. Int. J. Numer. Methods Heat Fluid Flow, 2021; ahead-of-print. [Google Scholar]
- Mebarek-Oudina, F. Convective heat transfer of Titania nanofluids of different base fluids in cylindrical annulus with discrete heat source. Heat Transf. Asian Res. 2019, 48, 135–147. [Google Scholar] [CrossRef] [Green Version]
- Dhif, K.; Mebarek-Oudina, F.; Chouf, S.; Vaidya, H.; Chamkha, A.J. Thermal Analysis of the Solar Collector Cum Storage System Using a Hybrid-Nanofluids. J. Nanofluids 2021, 10, 616–626. [Google Scholar] [CrossRef]
- Zamzari, F.; Mehrez, Z.; Cafsi, A.E.; Belghith, A.; Quéré, P.L. Entropy generation and mixed convection in a horizontal channel with an open cavity. Int. J. Exergy 2015, 17, 219–239. [Google Scholar] [CrossRef]
- Li, Y.X.; Al-Khaled, K.; Khan, S.U.; Sun, T.C.; Khan, M.I.; Malik, M.Y. Bio-convective Darcy-Forchheimer periodically accelerated flow of non-Newtonian nanofluid with Cattaneo–Christov and Prandtl effective approach. Case Stud. Therm. Eng. 2021, 26, 101102. [Google Scholar] [CrossRef]
- Mehrez, Z.; El Cafsi, A. Heat exchange enhancement of ferrofluid flow into rectangular channel in the presence of a magnetic field. Appl. Math. Comput. 2021, 391, 125634. [Google Scholar] [CrossRef]
- Khashi’ie, N.S.; Arifin, N.M.; Sheremet, M.; Pop, I. Shape factor effect of radiative Cu–Al2O3/H2O hybrid nanofluid flow towards an EMHD plate. Case Stud. Therm. Eng. 2021, 26, 101199. [Google Scholar] [CrossRef]
- Esfe, M.H.; Esfandeh, S.; Kamyab, M.H.; Toghraie, D. Analysis of rheological behavior of MWCNT-Al2O3 (10: 90)/5W50 hybrid non-Newtonian nanofluid with considering viscosity as a three-variable function. J. Mol. Liq. 2021, 341, 117375. [Google Scholar] [CrossRef]
- Mehrez, Z.; El Cafsi, A. Thermodynamic Analysis of Al2O3–Water Nanofluid Flow in an Open Cavity Under Pulsating Inlet Condition. Int. J. Appl. Comput. Math. 2017, 3, 489–510. [Google Scholar] [CrossRef]
- Algehyne, E.A.; El-Zahar, E.R.; Sohail, M.; Nazir, U.; AL-bonsrulah, H.A.Z.; Veeman, D.; Felemban, B.F.; Alharbi, F.M. Thermal Improvement in Pseudo-Plastic Material Using Ternary Hybrid Nanoparticles via Non-Fourier’s Law over Porous Heated Surface. Energies 2021, 14, 8115. [Google Scholar] [CrossRef]
- Sakiadis, B.C. Boundary-layer behavior on continuous solid surfaces: II. The boundary layer on a continuous flat surface. AiChE J. 1961, 7, 221–225. [Google Scholar] [CrossRef]
- Fox, V.G.; Erickson, L.E.; Fan, L.T. Methods for solving the boundary layer equations for moving continuous flat surfaces with suction and injection. AIChE J. 1968, 14, 726–736. [Google Scholar] [CrossRef]
- Chen, C.H. Forced convection over a continuous sheet with suction or injection moving in a flowing fluid. Acta Mech. 1999, 138, 1–11. [Google Scholar] [CrossRef]





| K (Thermal Conductivity) | σ (Electrical Conductivity) | ρ (Density) | |
|---|---|---|---|
| 0.253 | 1113.5 | ||
| 32.9 | 6310 | ||
| 8.953 | 4250 | ||
| 1.4013 | 2270 |
| Number of Elements | |||
|---|---|---|---|
| 30 | 0.01780117292 | 0.2638819583 | 0.1043559742 |
| 60 | 0.02008198265 | 0.2370957263 | 0.09636478785 |
| 90 | 0.02028786930 | 0.2277953868 | 0.09382548703 |
| 120 | 0.02030867335 | 0.2231793011 | 0.09257835690 |
| 150 | 0.02029805160 | 0.2204312762 | 0.09183716567 |
| 180 | 0.02028205279 | 0.2186104527 | 0.09134595367 |
| 210 | 0.02026649287 | 0.2173159996 | 0.09099653209 |
| 240 | 0.02025264615 | 0.2163487639 | 0.09073525408 |
| 270 | 0.02024062141 | 0.2155986751 | 0.09053255564 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hou, E.; Wang, F.; Nazir, U.; Sohail, M.; Jabbar, N.; Thounthong, P. Dynamics of Tri-Hybrid Nanoparticles in the Rheology of Pseudo-Plastic Liquid with Dufour and Soret Effects. Micromachines 2022, 13, 201. https://doi.org/10.3390/mi13020201
Hou E, Wang F, Nazir U, Sohail M, Jabbar N, Thounthong P. Dynamics of Tri-Hybrid Nanoparticles in the Rheology of Pseudo-Plastic Liquid with Dufour and Soret Effects. Micromachines. 2022; 13(2):201. https://doi.org/10.3390/mi13020201
Chicago/Turabian StyleHou, Enran, Fuzhang Wang, Umar Nazir, Muhammad Sohail, Noman Jabbar, and Phatiphat Thounthong. 2022. "Dynamics of Tri-Hybrid Nanoparticles in the Rheology of Pseudo-Plastic Liquid with Dufour and Soret Effects" Micromachines 13, no. 2: 201. https://doi.org/10.3390/mi13020201
APA StyleHou, E., Wang, F., Nazir, U., Sohail, M., Jabbar, N., & Thounthong, P. (2022). Dynamics of Tri-Hybrid Nanoparticles in the Rheology of Pseudo-Plastic Liquid with Dufour and Soret Effects. Micromachines, 13(2), 201. https://doi.org/10.3390/mi13020201









