Study on the Formation Mechanism of Cutting Dead Metal Zone for Turning AISI4340 with Different Chamfering Tools
Abstract
:1. Introduction
2. Numerical Simulation
2.1. Numerical Approach
2.2. Orthogonal Cutting Model with Chamfered Tool
2.3. Model of Johnson–Cook Material
3. Experimental Work
4. Results and Discussion
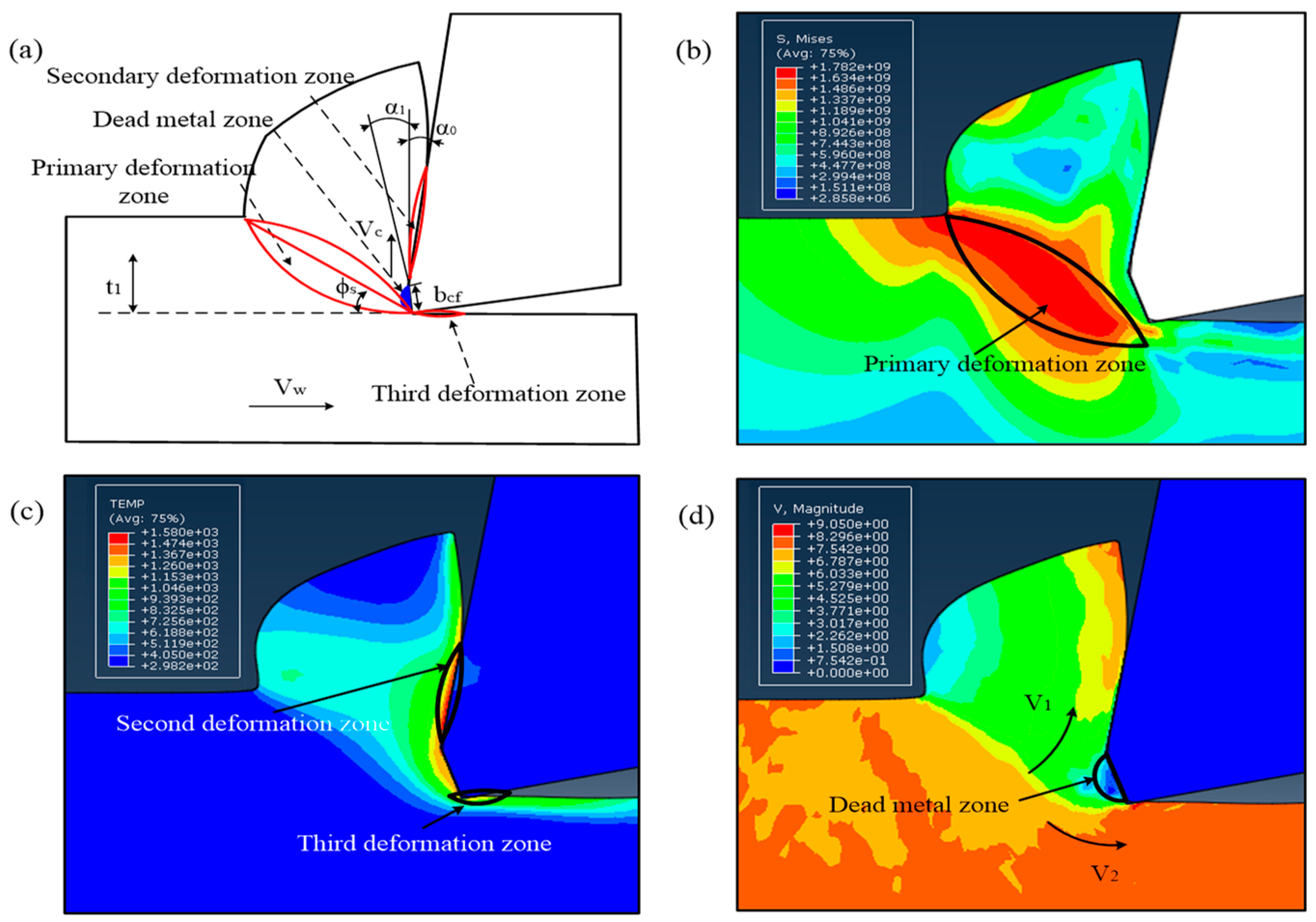
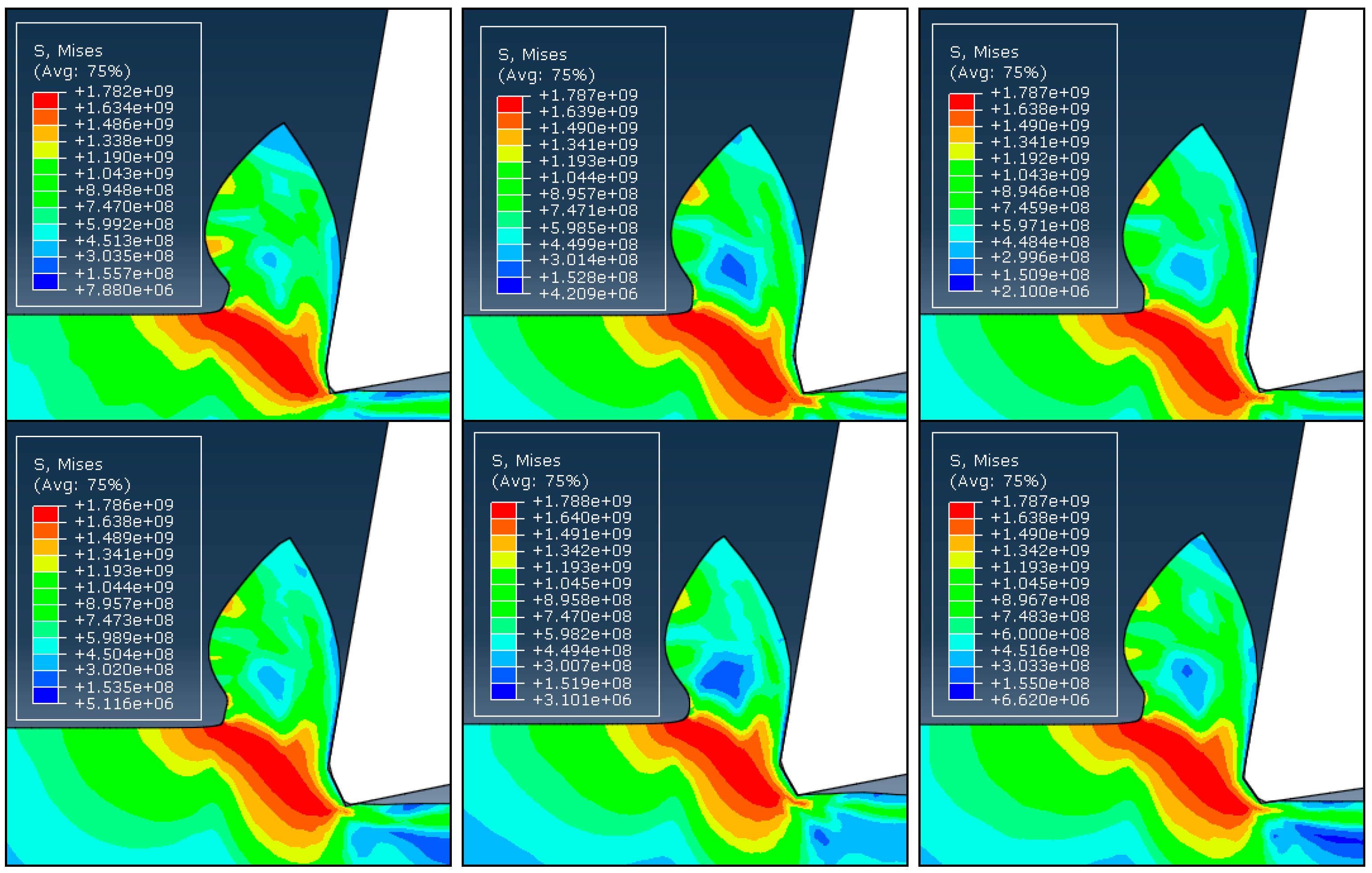
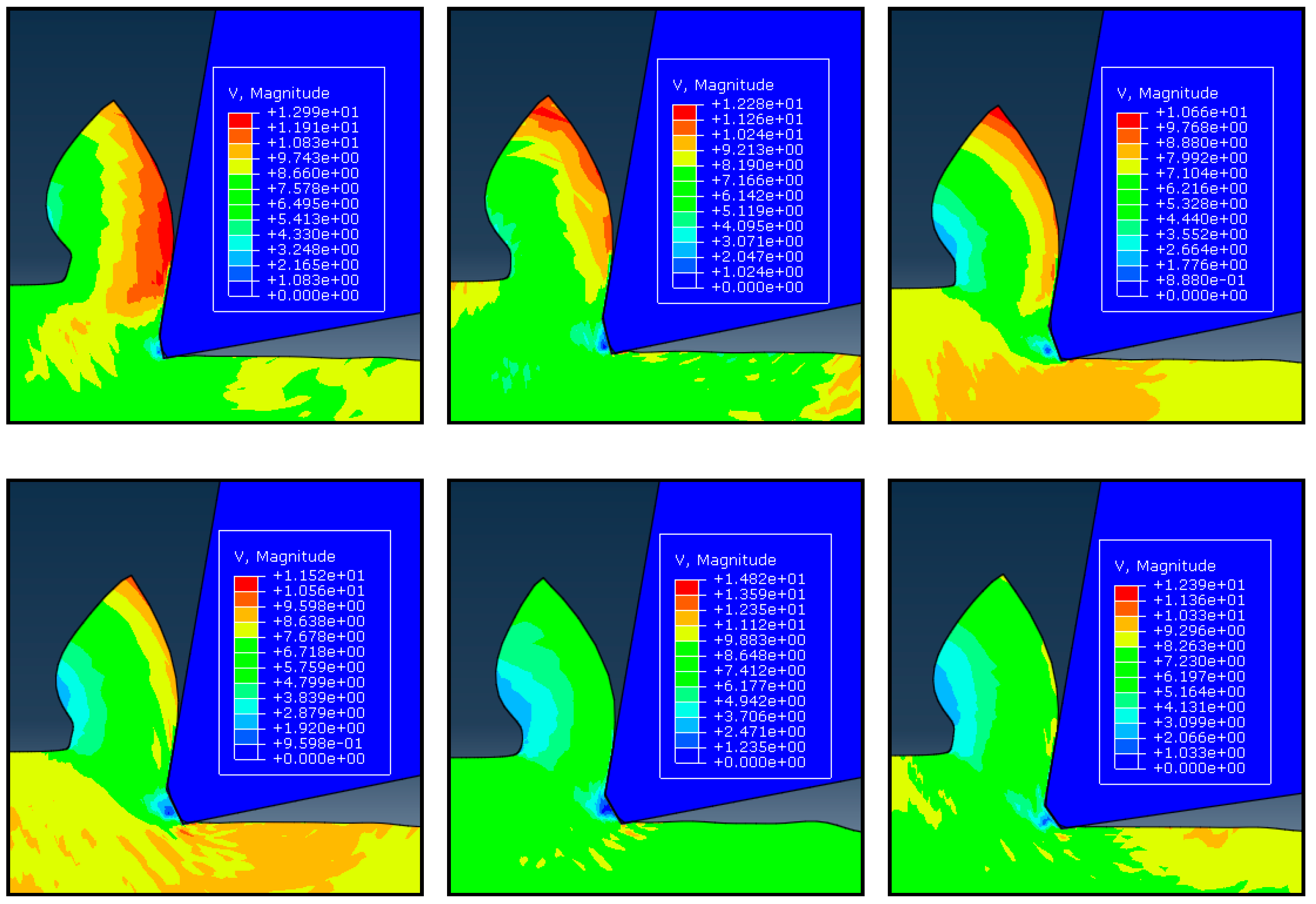
4.1. Influence of Chamfered Angle on Chip Formation Process
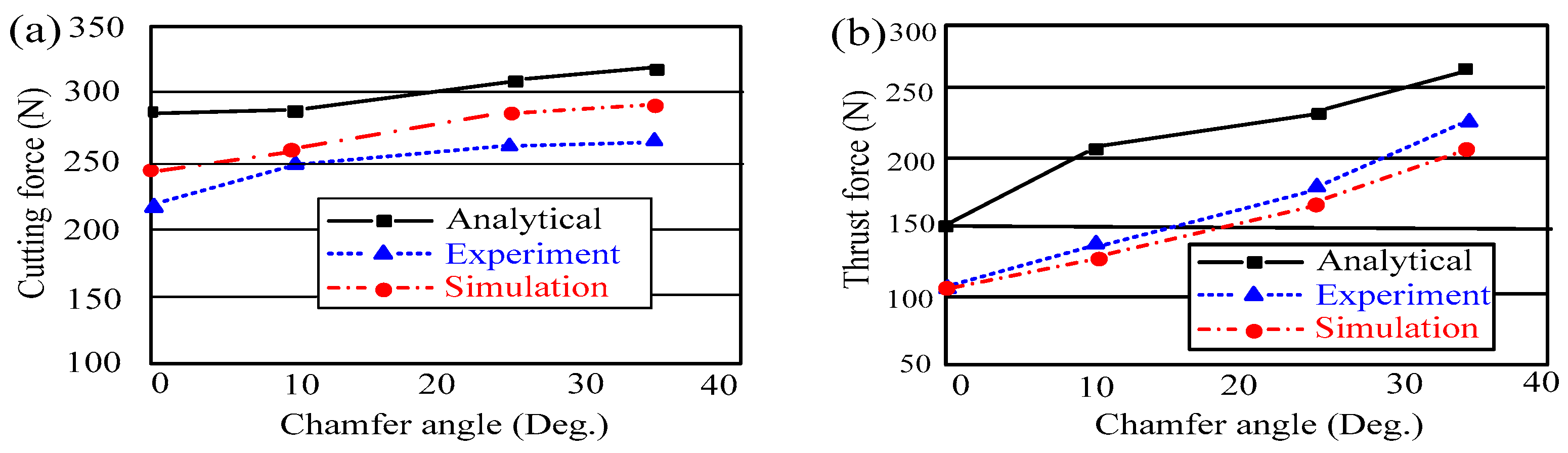
4.2. Effects of the Tool Geometry on Machining Forces
4.3. The Existence of the Dead Metal Zone
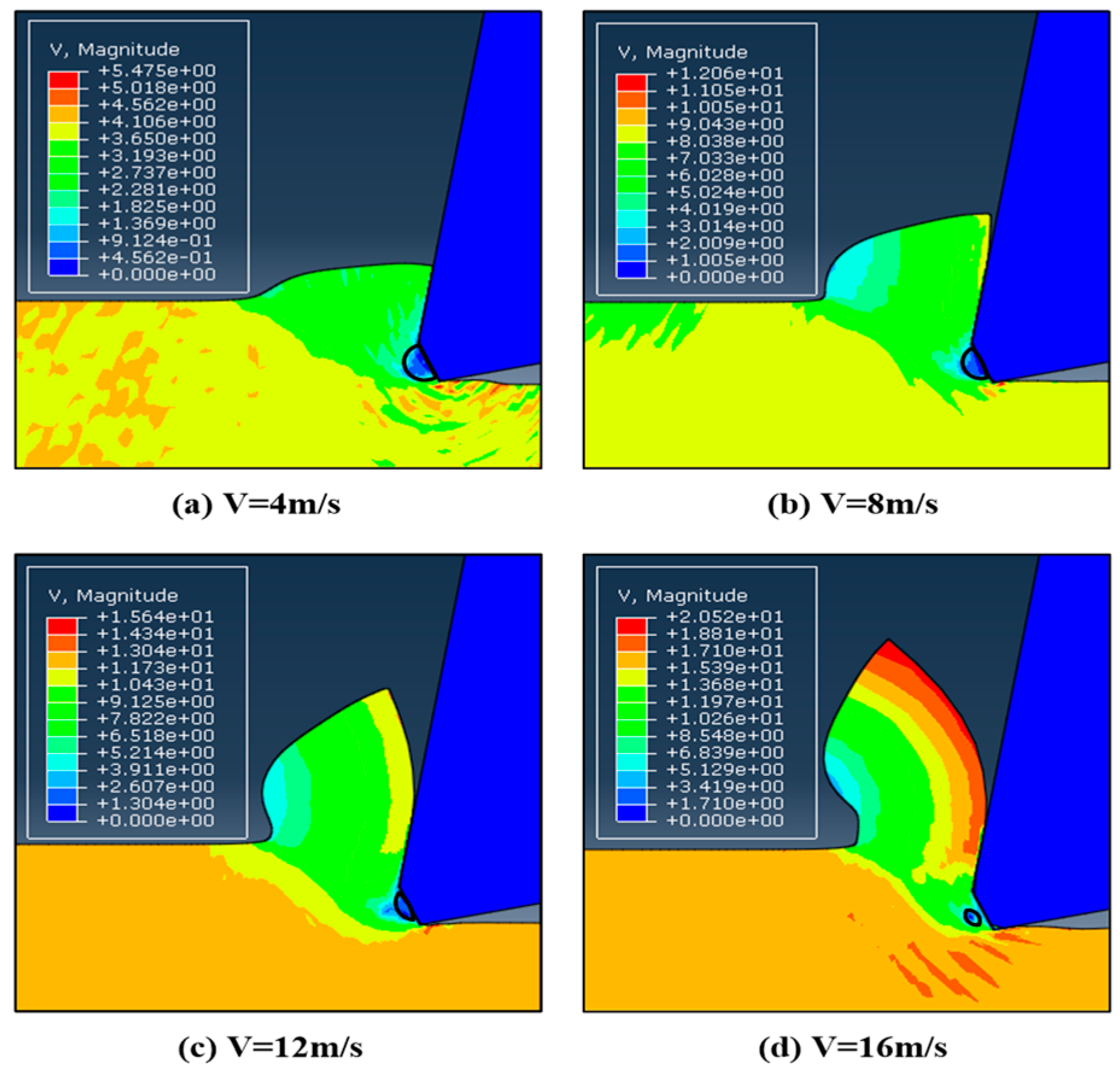
4.4. The Effect of Rotating Speed on Machining Forces
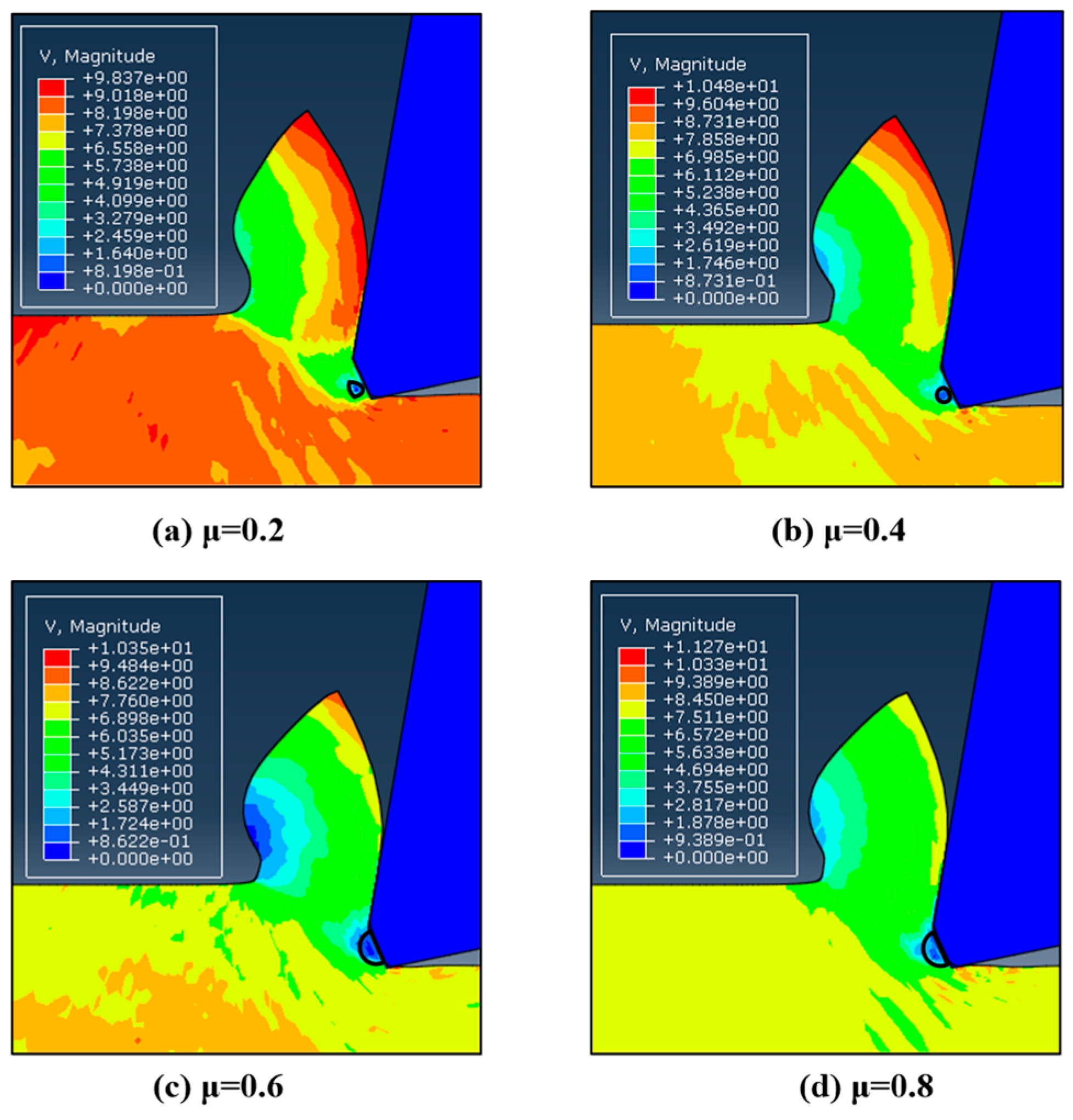
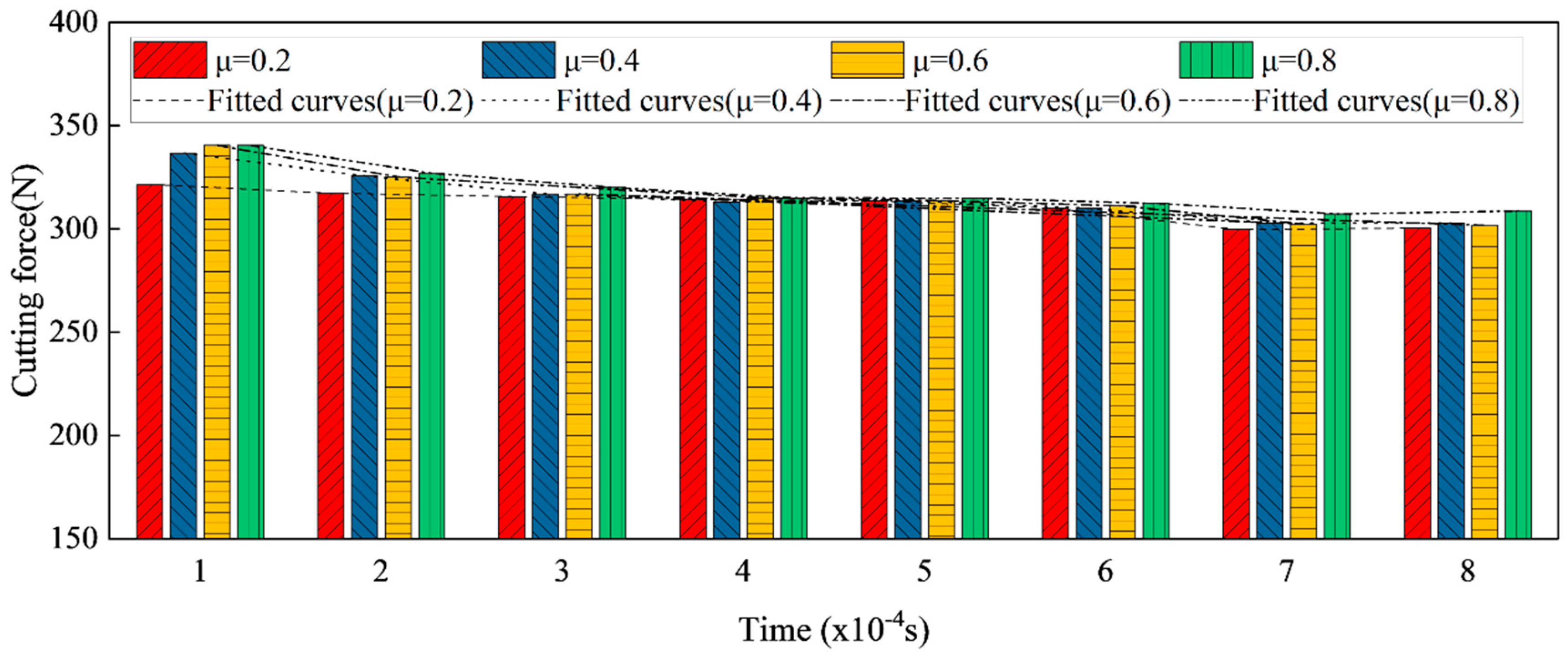
4.5. The Effect of Friction Coefficient on Machining Force
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
List of Symbols
| Chamfer angle | |
| α0 Front angle | |
| lengths | |
| Equivalent stress | |
| Initial yield stress | |
| Hardening modulus | |
| Strain rate dependency coefficient | |
| Plastic strain | |
| Strain rate | |
| Reference strain rate | |
| Operating temperature | |
| Room temperature | |
| Melting temperature | |
| Strain hardening exponent | |
| Thermal softening coefficient | |
| Coefficient of dynamic friction between tool and workpiece |
References
- Azaath, L.M.; Mohan, E.; Natarajan, U. Effect of rake angle and tool geometry during machining process of AISI 4340 steel in finite element approach. Mater. Today Proc. 2021, 37, 3731–3736. [Google Scholar] [CrossRef]
- Jiang, L.L.; Wang, D.Z. Finite-element-analysis of the effect of different wiper tool edge geometries during the hard turning of AISI 4340 steel. Simul. Model. Pract. Theory 2019, 94, 250–263. [Google Scholar] [CrossRef]
- Chatterjee, S.; Mahapatra, S.S.; Abhishek, K. Simulation and optimization of machining parameters in drilling of titanium alloys. Simul. Model. Pract. Theory 2016, 62, 31–48. [Google Scholar] [CrossRef]
- Mandal, N.; Doloi, B.; Mondal, B. Predictive modeling of surface roughness in high speed machining of AISI 4340 steel using yttria stabilized zirconia toughened alumina turning insert. Int. J. Refract. Met. Hard Mater. 2013, 38, 40–46. [Google Scholar] [CrossRef]
- Dong, G.; Wang, L.; Li, C.; Yu, Y. Investigation on ultrasonic elliptical vibration boring of deep holes with large depth- diameter ratio for high-strength steel 18Cr2Ni4WA. Int. J. Adv. Manuf. Technol. 2020, 108, 1527–1539. [Google Scholar] [CrossRef]
- Merchant, M.E. Mechanics of the metal cutting process. Plast. Cond. Orthogonal Cut. 1945, 16, 318–324. [Google Scholar]
- Oxley, P.L.B.; Hastings, W.F. Predicting the strain rate in the zone of intense shear in which the chip is formed in maching from the dynamic flow stress properties of the work material and the cutting conditions. Proc. R. Soc. Lond. 1977, 356, 395–410. [Google Scholar]
- Wardany, T.I.E.; Kishawy, H.A.; Elbestawi, M.A. Surface integrity of die material in high speed hard machining. J. Manuf. Sci. Eng. 2000, 122, 620–631. [Google Scholar] [CrossRef]
- Agrawal, S.; Joshi, S.S. Analytical modelling of residual stresses in orthogonal machining of AISI4340 steel. J. Manuf. Processes 2013, 15, 167–179. [Google Scholar] [CrossRef]
- Geetha, C.H.T.S.; Dash, A.K.; Kavya, B.; Amrita, M. Analysis of hybrid nanofluids in machining AISI 4340 using minimum quantity lubrication. Mater. Today Proc. 2021, 43, 579–586. [Google Scholar] [CrossRef]
- Das, A.; Pradhan, O.; Patel, S.K.; Das, S.R.; Biswal, B.B. Performance appraisal of various nanofluids during hard machining of AISI 4340 steel. J. Manuf. Processes 2019, 46, 248–270. [Google Scholar] [CrossRef]
- Zhang, J.J.; Wang, D.Z. Investigations of tangential ultrasonic vibration turning of Ti6Al4V using finite element method. Int. J. Mater. Form. 2019, 12, 257–267. [Google Scholar] [CrossRef]
- Wan, L.; Wang, D.Z. Numerical analysis of the formation of the dead metal zone with different tools in orthogonal cutting. Simul. Model. Pract. Theory 2015, 56, 1–15. [Google Scholar] [CrossRef]
- Wan, L.; Wang, D.Z.; Gao, Y.Y. The investigation of mechanism of serrated chip formation under different cutting speeds. Int. J. Adv. Manuf. Technol. 2016, 82, 951–959. [Google Scholar] [CrossRef]
- Dong, G.; Zhang, L. Investigation on grinding force and machining quality during rotary ultrasonic grinding deep-small hole of fluorophlogopite ceramics. Int. J. Adv. Manuf. Technol. 2019, 104, 2815–2825. [Google Scholar] [CrossRef]
- Wan, L.; Wang, D.Z.; Gao, Y.Y. Investigations on the effects of different tool edge geometries in the finite element simulation of machining. J. Mech. Eng. 2015, 61, 157–166. [Google Scholar] [CrossRef]
- Kapat, Y.; Özel, T. Analytical and thermal modeling of high-speed machining with chamfered tools. J. Manuf. Sci. Eng. 2008, 130, 1–15. [Google Scholar]
- Movaheddy, M.R.; Altintas, Y.; Gadala, M.S. Numerical analysis of metal cutting with chanfered and blunt tools. ASME J. Manuf. 2002, 122, 178–188. [Google Scholar] [CrossRef]
- Ozturk, S.; Altan, E. Design of a computer aided quick-stop device for study of dead metal zone formation. J. Braz. Soc. Mech. Sci. Eng. 2012, 34, 501–505. [Google Scholar] [CrossRef] [Green Version]
- Ozturk, S.; Uysal, A.; Altan, E. Metallographic analysis of the dead metal zone in metal cutting with a rounded-edge tool. Pract. Metallogr. 2013, 50, 318–330. [Google Scholar] [CrossRef]
- Karami, P.; Abrinia, K.; Saghafi, B. A new analytical definition of the dead material zone for forward extrusion of shaped sections. Meccanica 2014, 49, 295–304. [Google Scholar] [CrossRef]
- Uysal, A.; Ozturk, S.; Altan, E. An experimental study on dead metal zone in orthogonal cutting with worn rounded-edge cutting tools. Int. J. Mater. Prod. Technol. 2015, 51, 401–412. [Google Scholar] [CrossRef]
- Al-Athel, K.S.; Gadala, M.S. The use of volume of solid approach in simulating metal with chamfered and blunt tools. Int. J. Mech. Sci. 2011, 53, 23–30. [Google Scholar] [CrossRef]
- Long, Y.; Huang, Y. Force modeling under dead metal zone effect in orthogonal cutting with chamfered tools. Soc. Manuf. Eng. 2005, 33, 573–580. [Google Scholar]
- Ren, H.; Altintas, Y. Mechanics of machining with chamfered tools. J. Manuf. Sci. Eng. 2000, 122, 650–659. [Google Scholar] [CrossRef]
- Wang, D.Z.; Wu, S.J.; Lin, J.P.; Guo, G.Q.; Wang, P. Research on ultrasonic elliptical vibration micro-cutting inconel718 based on minimum quantity lubrication. J. Mech. Eng. 2021, 57, 264–271. (In Chinese) [Google Scholar]
- Chen, F.Y.; Wang, D.Z.; Wu, S.J. Influence of ultrasonic vibration-assisted cutting on ploughing effect in cutting Ti6Al4V. Arch. Civ. Mech. Eng. 2021, 21, 42. [Google Scholar] [CrossRef]
- Hirao, M.; Tlusty, R.; Sowerby, R.; Chandra, G. Chip formation with chamfered tools. ASME J. Ind. 1982, 104, 339–342. [Google Scholar] [CrossRef]
- Zhang, H.T.; Liu, P.D.; Hu, R.S. A three zone models of orthogonal cutting with application to single point diamond turning. Wear 1991, 14, 29–43. [Google Scholar] [CrossRef]
- Jacobson, S.; Wallén, P. A new classification system for dead metal zones in metal cutting. Int. J. Manuf. 1988, 28, 529–538. [Google Scholar] [CrossRef]
- Nalbant, M.; Altin, A.; GÖkkaya, H. The effect of cutting speed and cutting tool geometry on machinability properties of nickel-base Inconel 718 super alloys. Mater. Des. 2007, 28, 1334–1338. [Google Scholar] [CrossRef]
- Atlati, S.; Haddag, B.; Nouari, M.; Moufki, A. Effect of the local friction and contact nature on the built-up edge formation process in machining ductile metals. Tribol. Int. 2015, 90, 217–227. [Google Scholar] [CrossRef]




| Abaqus/Explicit | |||
|---|---|---|---|
| Material properties | Plasticity, Johnson-Cook law | A(MPa) | 1150 |
| B(MPa) | 739 | ||
| n | 0.26 | ||
| C | 0.014 | ||
| m | 1.03 | ||
| Inelastic heat fraction(β) | 0.9 | ||
| Density(ρ)(kg/m3) | Workpiece(AISI 4340) | 7850 | |
| Tool(Carbide) | 11500 | ||
| Elasticity | Workpiece | 2.1 × 1011 | |
| Tool | 5.3 × 1011 | ||
| Conductivity(k) (W/m·k) | Workpiece | 44.5 | |
| Tool | 120 | ||
| Specific heat(c) (J/kg·K) | Workpiece | 502 | |
| Tool | 343.3 | ||
| Expansion(K−1) | Workpiece | 1.23 × 10−0.005 | |
| Tool | 5.2 × 10−0.006 | ||
| Friction coefficient (μ) | 0.2, 0.4, 0.6, 0.8 | ||
| Process | Cutting speed (v) (m/s) | 0.5, 1, 1.5, 2, 2.5 | |
| Rake angle (γ) (°) | 10° | ||
| Clearance angle (α) (°) | 10° | ||
| Case | α0 | α1 | bcf |
|---|---|---|---|
| 1 | 10 | 0 | − |
| 2 | 10 | −10 | 0.0902 |
| 3 | 10 | −15 | 0.1177 |
| 4 | 10 | −20 | 0.1177 |
| 5 | 10 | −25 | 0.1177 |
| 6 | 10 | −30 | 0.1019 |
| 7 | 10 | −35 | 0.0981 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, S.; Wang, D.; Zhang, J.; Nadykto, A.B. Study on the Formation Mechanism of Cutting Dead Metal Zone for Turning AISI4340 with Different Chamfering Tools. Micromachines 2022, 13, 1156. https://doi.org/10.3390/mi13071156
Wu S, Wang D, Zhang J, Nadykto AB. Study on the Formation Mechanism of Cutting Dead Metal Zone for Turning AISI4340 with Different Chamfering Tools. Micromachines. 2022; 13(7):1156. https://doi.org/10.3390/mi13071156
Chicago/Turabian StyleWu, Shujing, Dazhong Wang, Jiajia Zhang, and Alexey B. Nadykto. 2022. "Study on the Formation Mechanism of Cutting Dead Metal Zone for Turning AISI4340 with Different Chamfering Tools" Micromachines 13, no. 7: 1156. https://doi.org/10.3390/mi13071156
APA StyleWu, S., Wang, D., Zhang, J., & Nadykto, A. B. (2022). Study on the Formation Mechanism of Cutting Dead Metal Zone for Turning AISI4340 with Different Chamfering Tools. Micromachines, 13(7), 1156. https://doi.org/10.3390/mi13071156






