Research on Vibration Energy Harvester Based on Two-Dimensional Acoustic Black Hole
Abstract
1. Introduction
2. Structural Design of 2-D ABH
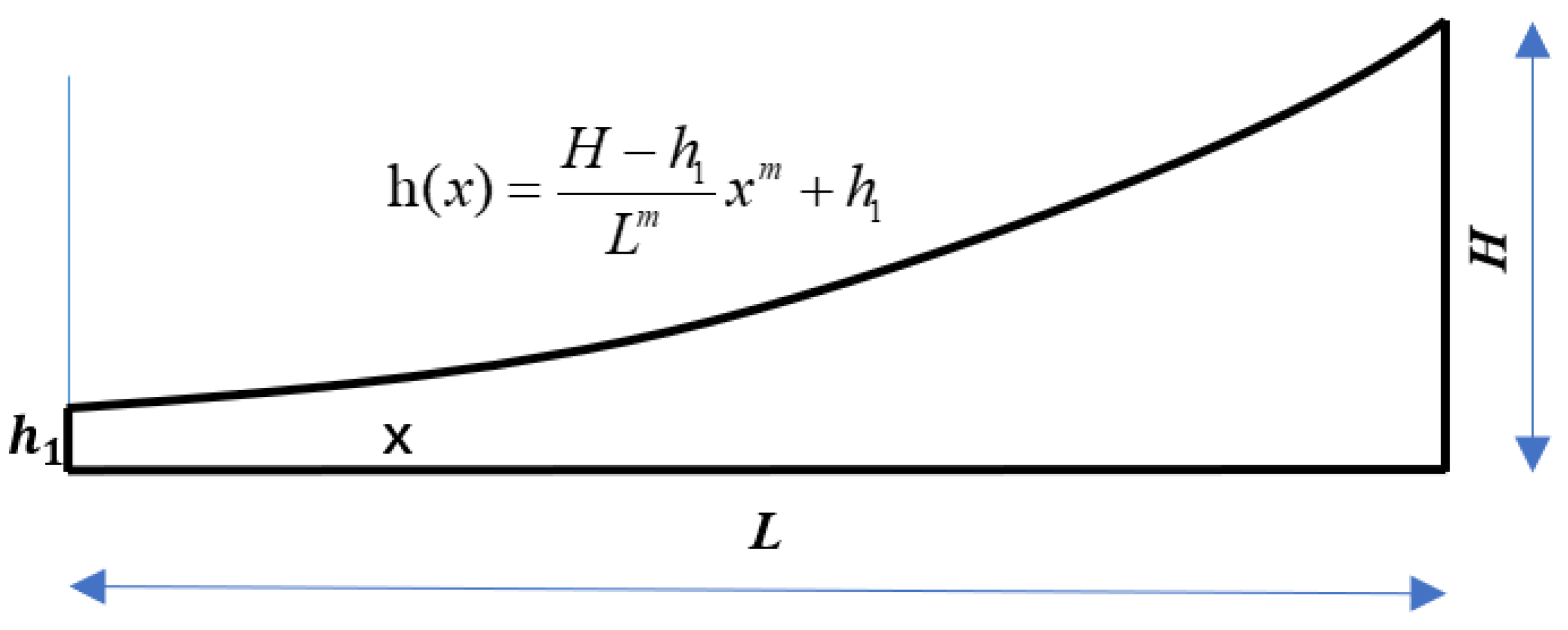
2.1. ABH Theory
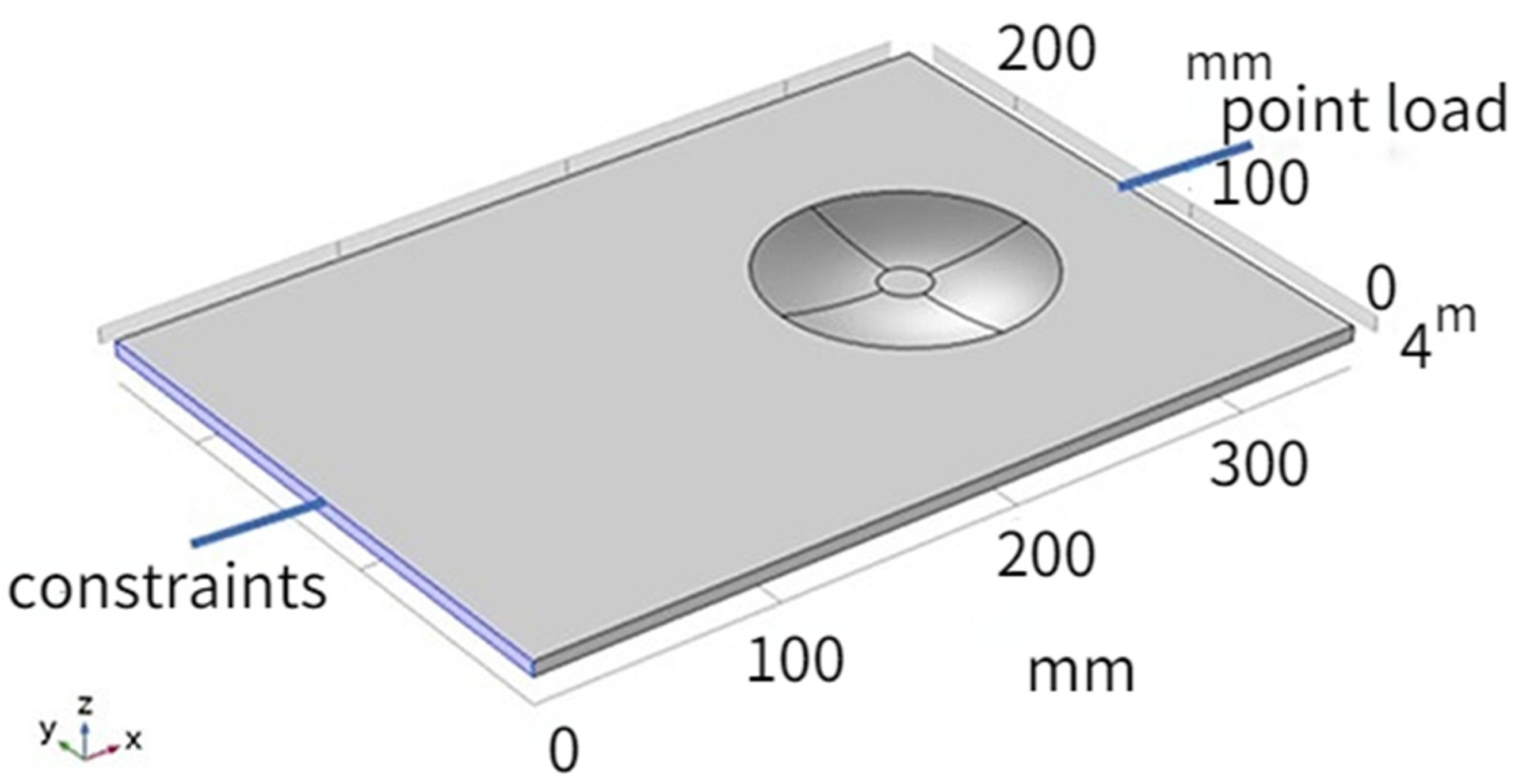
2.2. Structural Design of 2-D ABH
2.3. Structure Analyses of 2-D ABH
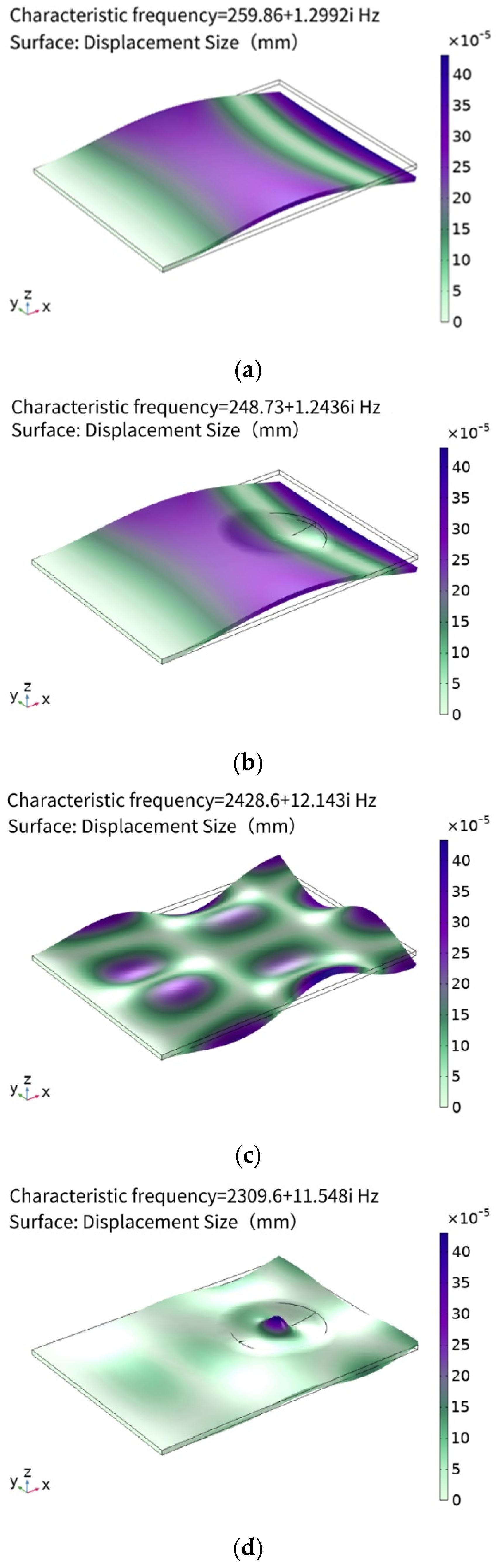
2.3.1. The Energy Concentration Effect of 2-D ABH
2.3.2. Influence of the ABH Power Index on Vibration
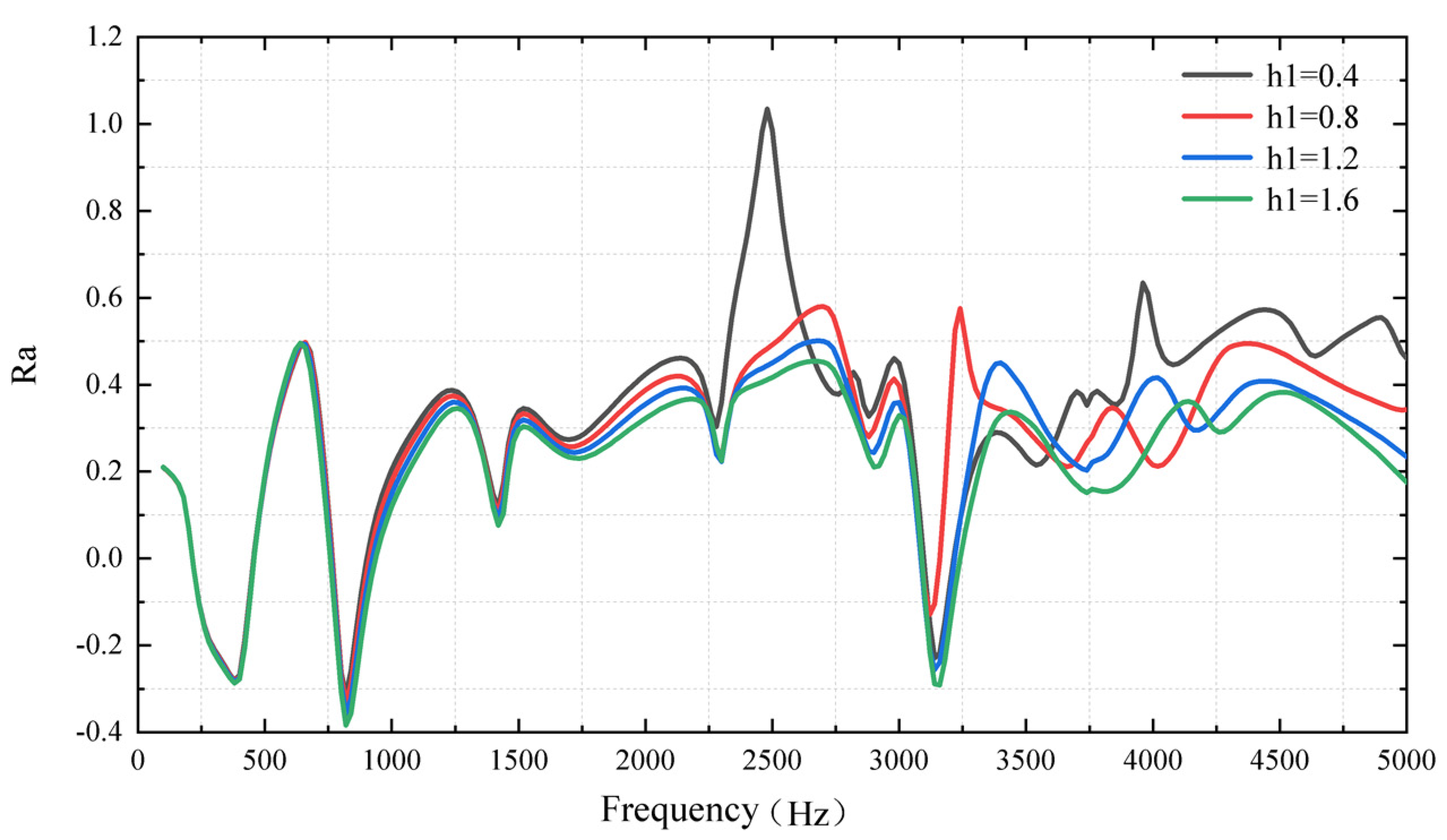
2.3.3. Influence of ABH Truncation Thickness on Vibration
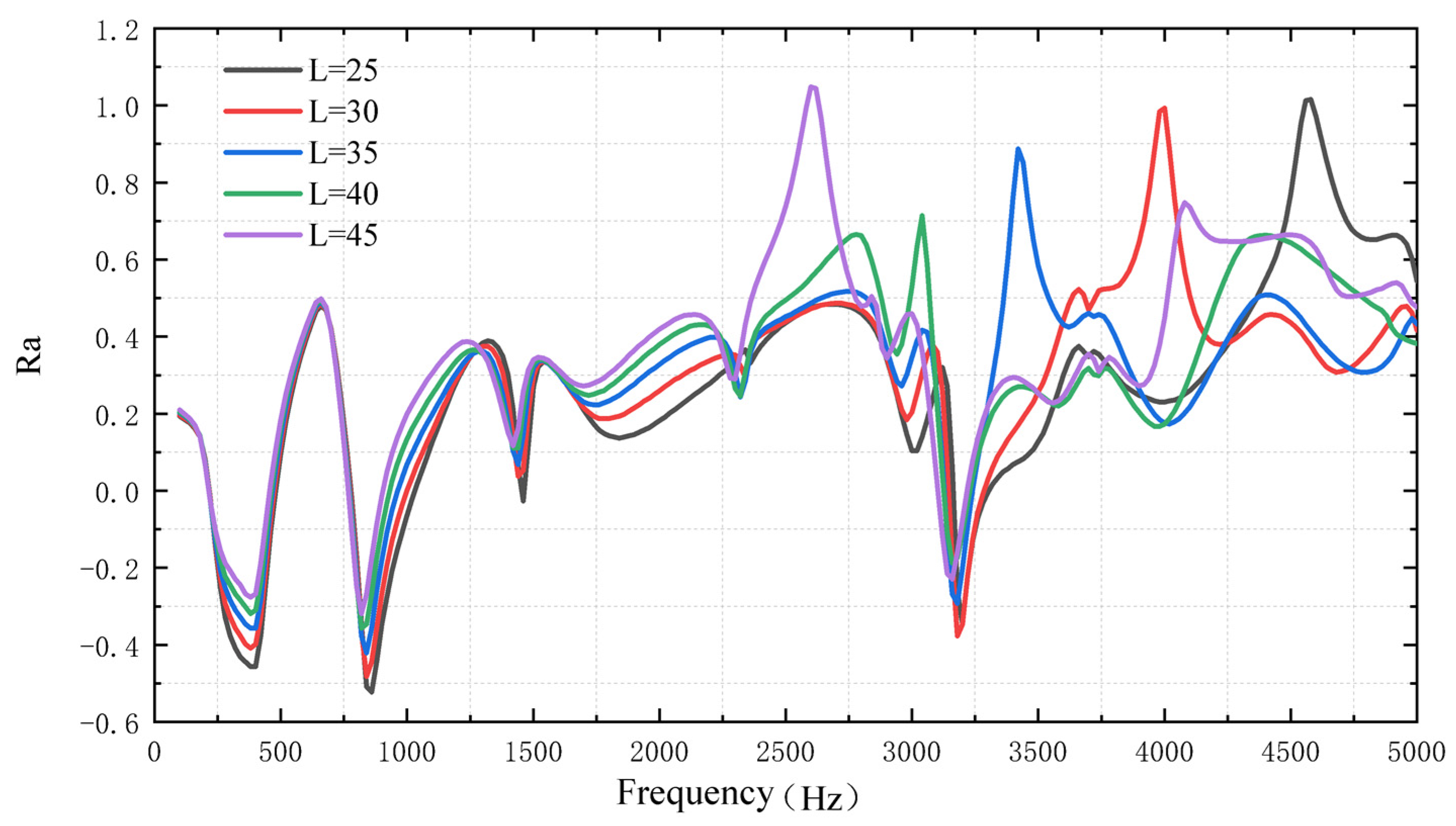
2.3.4. Influence of ABH Cross-Sectional Length on Vibration
3. Optimization and Analysis
3.1. Optimization and Analysis of ABH Size
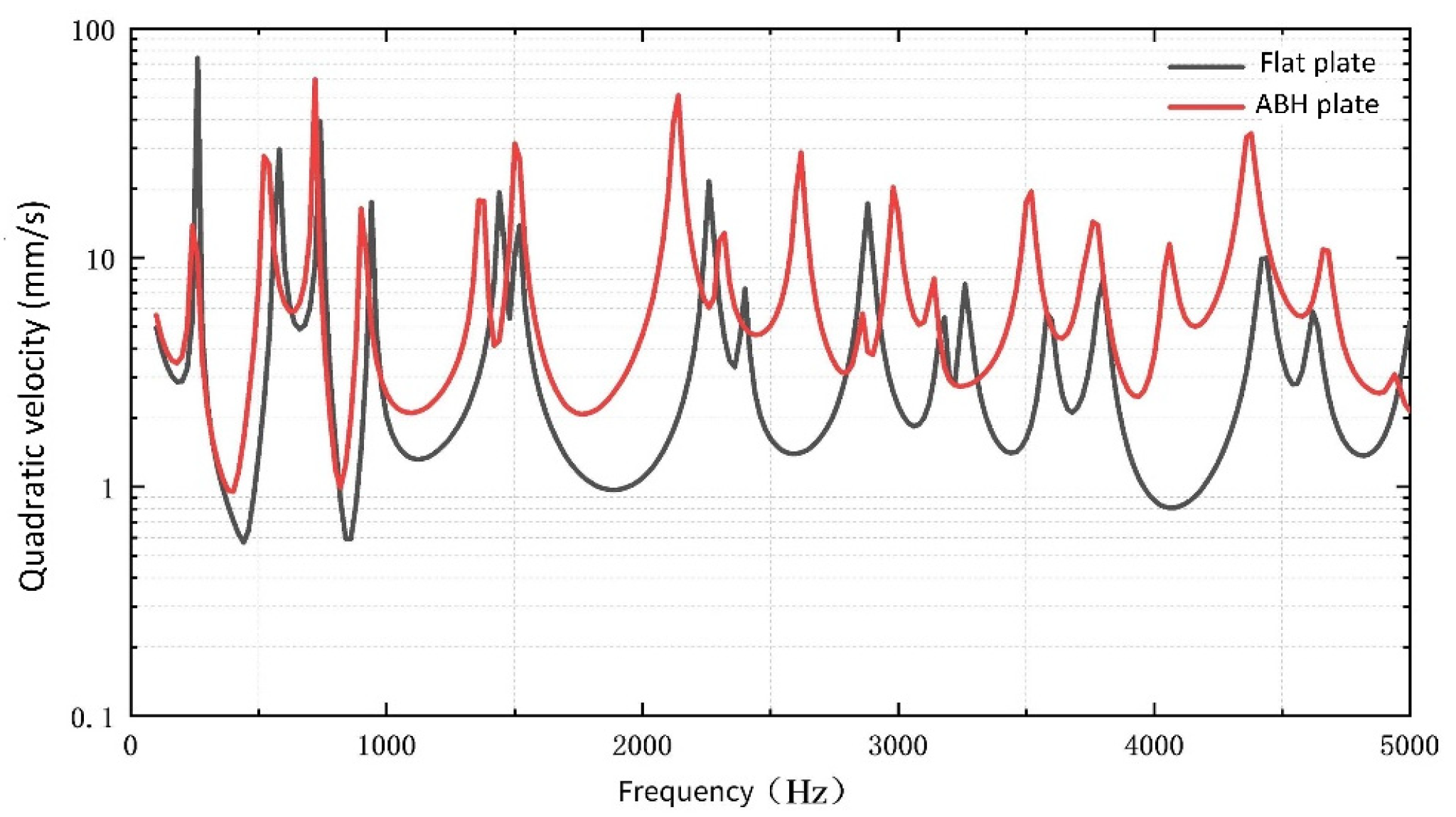
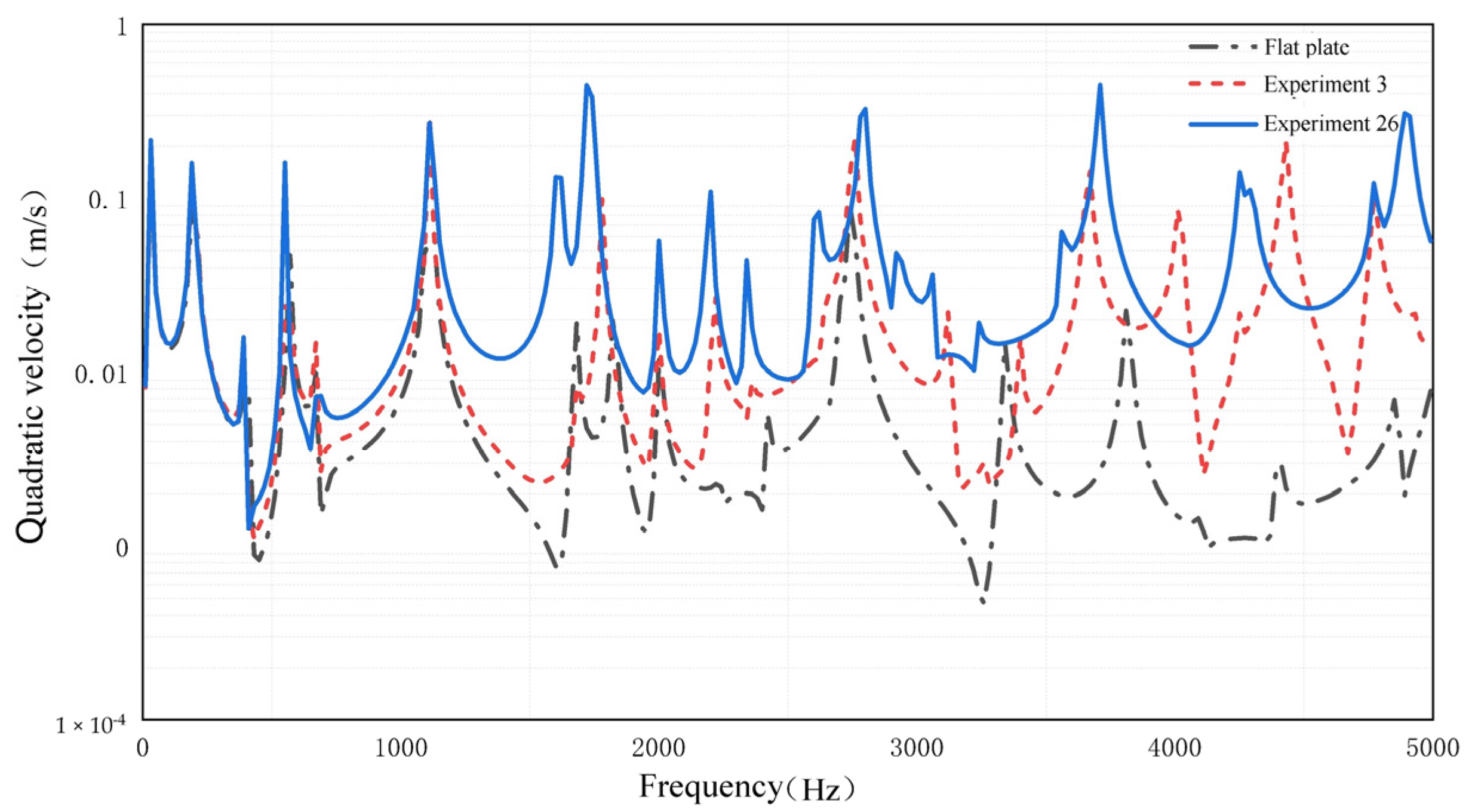
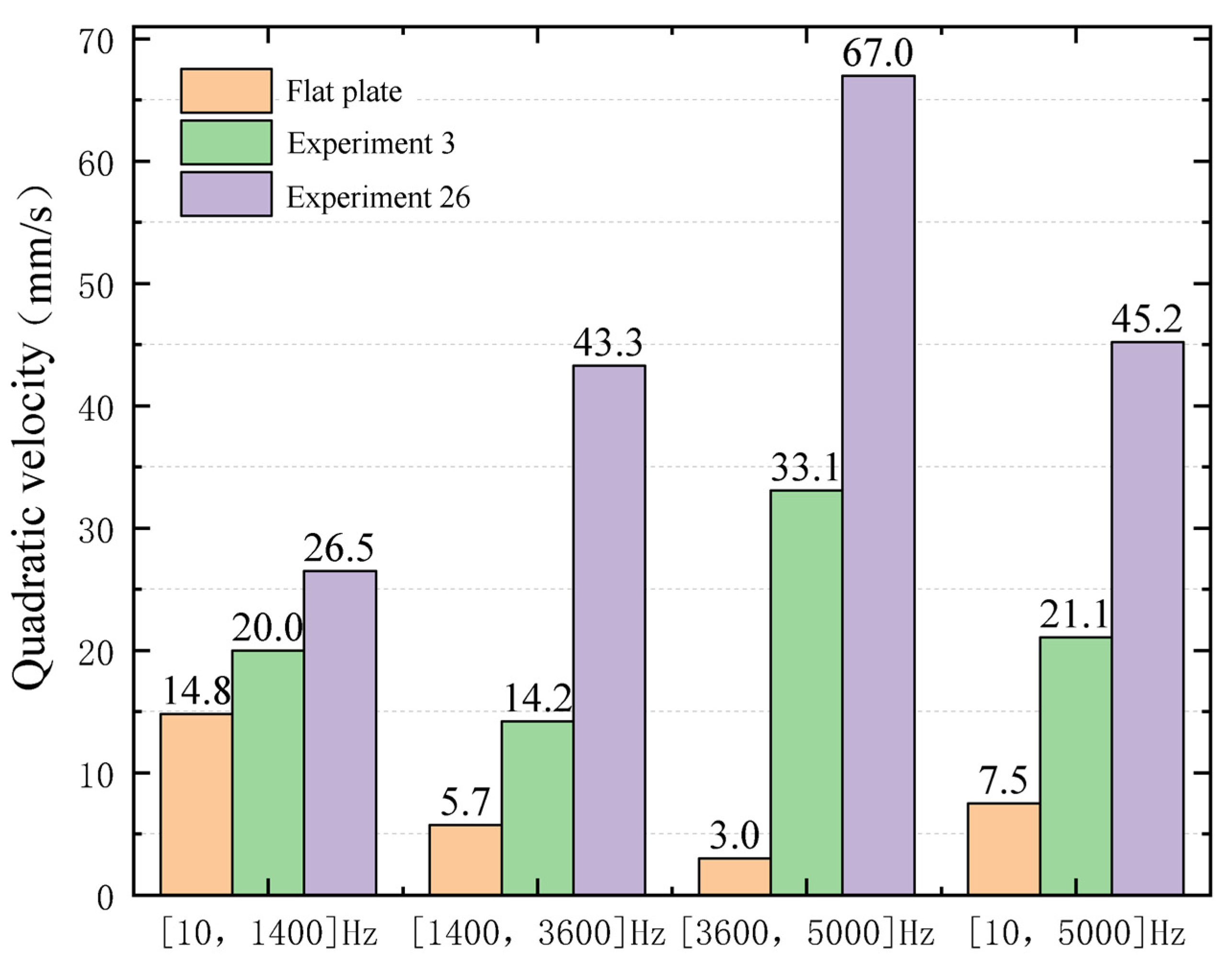
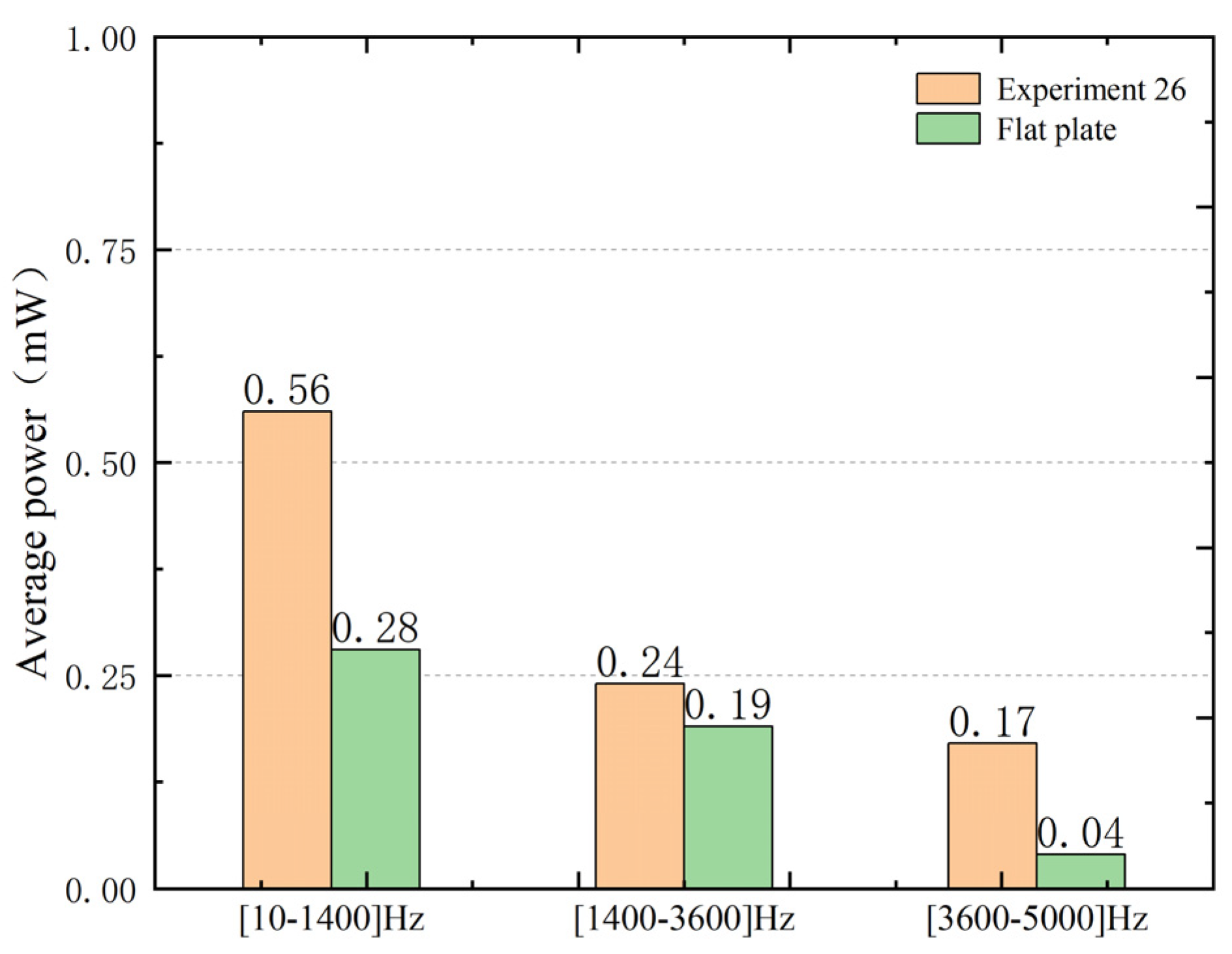
3.2. Frequency Domain Analysis of Different Experiments
3.3. Output Performance Simulation of VEH
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Wang, Z.; Du, Y.; Li, T.; Yan, Z.; Tan, T. A flute-inspired broadband piezoelectric vibration energy harvesting device with mechanical intelligent design. Appl. Energy 2021, 303, 117577. [Google Scholar] [CrossRef]
- Shi, T.; Hu, G.; Zou, L.; Song, J.; Kwok, K. Performance of an omnidirectional piezoelectric wind energy harvester. Wind Energy 2021, 24, 1167–1179. [Google Scholar] [CrossRef]
- Caetano, V.J.; Savi, M.A. Multimodal pizza-shaped piezoelectric vibration-based energy harvesters. J. Intell. Mater. Syst. Struct. 2021, 32, 2505–2528. [Google Scholar] [CrossRef]
- Qi, N.; Dai, K.; Wang, X.; You, Z. Optimization for piezoelectric energy harvesters with self-coupled structure: A double kill in bandwidth and power. Nano Energy 2022, 102, 107602. [Google Scholar] [CrossRef]
- Chen, K.; Gao, F.; Liu, Z.; Liao, W.H. A nonlinear M-shaped tri-directional piezoelectric energy harvester. Smart Mater. Struct. 2021, 30, 045017. [Google Scholar] [CrossRef]
- Pertin, O.; Guha, K.; Jakšić, O.; Jakšić, Z.; Iannacci, J. Investigation of Nonlinear Piezoelectric Energy Harvester for Low-Frequency and Wideband Applications. Micromachines 2022, 13, 1399. [Google Scholar] [CrossRef] [PubMed]
- Sneller, A.J.; Cette, P.; Mann, B.P. Experimental investigation of a post-buckled piezoelectric beam with an attached central mass used to harvest energy. Proc. Inst. Mech. Eng. Part I J. Syst. Control Eng. 2011, 225, 497–509. [Google Scholar] [CrossRef]
- Derakhshani, M.; Berfield, T.; Murphy, K.D. Dynamic Analysis of a Bi-stable Buckled Structure for Vibration Energy Harvester; Springer International: New York, NY, USA, 2018; Volume 1, pp. 199–208. [Google Scholar]
- Halim, M.A.; Kim, D.H.; Park, J.Y. Low Frequency Vibration Energy Harvester Using Stopper-Engaged Dynamic Magnifier for Increased Power and Wide Bandwidth. J. Electr. Eng. Technol. 2016, 11, 707–714. [Google Scholar] [CrossRef]
- Ambrożkiewicz, B.; Czyż, Z.; Karpiński, P.; Stączek, P.; Litak, G.; Grabowski, Ł. Ceramic-Based Piezoelectric Material for Energy Harvesting Using Hybrid Excitation. Materials 2021, 14, 5816. [Google Scholar] [CrossRef] [PubMed]
- Ambrożkiewicz, B.; Czyż, Z.; Stączek, P.; Tiseira, A.; García-Tíscar, J. Performance Analysis of a Piezoelectric Energy Harvesting System. Adv. Sci. Technol. Res. J. 2022, 16, 179–185. [Google Scholar] [CrossRef]






| Parameter | Numerical Value |
|---|---|
| Elastic Modulus (GPa) | 70 |
| Density (kg/m3) | 2700 |
| Poisson’s Ratio | 0.3 |
| Mechanical Damping | 0.005 |
| Length/mm | 400 |
| Thickness/mm | 300 |
| Width/mm | 6 |
| Parameter | Numerical Value |
|---|---|
| Power Exponent m | 2 |
| Truncation Thickness h1/mm | 0.4 |
| Section Length L/mm | 45 |
| Round Platform Diameter d/mm | 20 |
| Order | Flat Plate | ABH Plate |
|---|---|---|
| First order | 42.0 Hz | 43.7 Hz |
| Second order | 131.3 Hz | 127.4 Hz |
| Third order | 259.9 Hz | 248.7 Hz |
| Sixteenth order | 2397.8 Hz | 2288.1 Hz |
| Seventeenth order | 2428.6 Hz | 2309.6 Hz |
| Eighteenth order | 2604.4 Hz | 2540.5 Hz |
| Twenty-nine order | 4432.4 Hz | 3967.1 Hz |
| Thirty order | 4626.1 Hz | 4049.8 Hz |
| Thirty-one order | 4721.5 Hz | 4055.3 Hz |
| m | Fifth-Order | Sixth-Order | Seventh-Order | Eighth-Order |
|---|---|---|---|---|
| 2 | 720.21 Hz | 905.74 Hz | 908.66 Hz | 1298.7 Hz |
| 5 | 730.57 Hz | 896.69 Hz | 901.95 Hz | 984.06 Hz |
| 8 | 681.53 Hz | 753.68 Hz | 894.95 Hz | 900.05 Hz |
| 11 | 582.73 Hz | 747.72 Hz | 892.76 Hz | 898.76 Hz |
| 14 | 543.20 Hz | 748.26 Hz | 890.99 Hz | 897.36 Hz |
| 17 | 535.90 Hz | 749.05 Hz | 888.52 Hz | 895.52 Hz |
| 20 | 534.09 Hz | 749.73 Hz | 884.66 Hz | 892.29 Hz |
| Serial Number | 1 | 2 | 3 | 4 |
|---|---|---|---|---|
| Factor | Power Exponent m | Section Length L (mm) | Truncated Thickness h1 (mm) | Diameter of Cone d (mm) |
| Level 1 | 2 | 2 | 0.4 | 15 |
| Level 2 | 3 | 3 | 0.6 | 20 |
| Level 3 | 4 | 4 | 0.8 | 25 |
| Level 4 | 5 | 5 | 1.0 | 30 |
| Level 5 | 6 | 6 | 1.2 | 35 |
| Factor | Power Index | Truncation Thickness | Cross-Sectional Length | Round Table Diameter | Simulation Result |
|---|---|---|---|---|---|
| Experiment 1 | 1 | 1 | 1 | 1 | 1.000 |
| Experiment 2 | 1 | 2 | 2 | 2 | 1.684 |
| Experiment 3 | 1 | 3 | 3 | 3 | 0.871 |
| Experiment 4 | 1 | 4 | 4 | 4 | 0.740 |
| Experiment 5 | 1 | 5 | 5 | 5 | 0.633 |
| Experiment 6 | 2 | 1 | 2 | 3 | 1.145 |
| Experiment 7 | 2 | 2 | 3 | 4 | 0.882 |
| Experiment 8 | 2 | 3 | 4 | 5 | 0.979 |
| Experiment 9 | 2 | 4 | 5 | 1 | 0.175 |
| Experiment 10 | 2 | 5 | 1 | 2 | 3.448 |
| Experiment 11 | 3 | 1 | 3 | 5 | 1.547 |
| Experiment 12 | 3 | 2 | 4 | 1 | 0.547 |
| Experiment 13 | 3 | 3 | 5 | 2 | 0.335 |
| Experiment 14 | 3 | 4 | 1 | 3 | 3.000 |
| Experiment 15 | 3 | 5 | 2 | 4 | 1.158 |
| Experiment 16 | 4 | 1 | 4 | 2 | 0.493 |
| Experiment 17 | 4 | 2 | 5 | 3 | 0.418 |
| Experiment 18 | 4 | 3 | 1 | 4 | 3.445 |
| Experiment 19 | 4 | 4 | 2 | 5 | 1.345 |
| Experiment 20 | 4 | 5 | 3 | 1 | 0.416 |
| Experiment 21 | 5 | 1 | 5 | 4 | 0.469 |
| Experiment 22 | 5 | 2 | 1 | 5 | 0.563 |
| Experiment 23 | 5 | 3 | 2 | 1 | 2.155 |
| Experiment 24 | 5 | 4 | 3 | 2 | 0.930 |
| Experiment 25 | 5 | 5 | 4 | 3 | 0.450 |
| Mean value 1 | 0.986 | 0.931 | 2.291 | 0.859 | |
| Mean value 2 | 1.326 | 0.819 | 1.497 | 1.378 | |
| Mean value 3 | 1.317 | 1.557 | 0.929 | 1.177 | |
| Mean value 4 | 1.223 | 1.238 | 0.642 | 1.339 | |
| Mean value 5 | 0.913 | 1.221 | 0.406 | 1.013 | |
| Range | 0.413 | 0.738 | 1.885 | 0.519 | |
| Error square sum | 0.737 | 1.681 | 11.418 | 0.959 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, C.; Yuan, Y.; Wang, H.; Tang, Y.; Gui, J. Research on Vibration Energy Harvester Based on Two-Dimensional Acoustic Black Hole. Micromachines 2023, 14, 538. https://doi.org/10.3390/mi14030538
Yang C, Yuan Y, Wang H, Tang Y, Gui J. Research on Vibration Energy Harvester Based on Two-Dimensional Acoustic Black Hole. Micromachines. 2023; 14(3):538. https://doi.org/10.3390/mi14030538
Chicago/Turabian StyleYang, Chunlai, Yikai Yuan, Hai Wang, Ye Tang, and Jingsong Gui. 2023. "Research on Vibration Energy Harvester Based on Two-Dimensional Acoustic Black Hole" Micromachines 14, no. 3: 538. https://doi.org/10.3390/mi14030538
APA StyleYang, C., Yuan, Y., Wang, H., Tang, Y., & Gui, J. (2023). Research on Vibration Energy Harvester Based on Two-Dimensional Acoustic Black Hole. Micromachines, 14(3), 538. https://doi.org/10.3390/mi14030538








