Structural Engineering in Piezoresistive Micropressure Sensors: A Focused Review
Abstract
1. Introduction
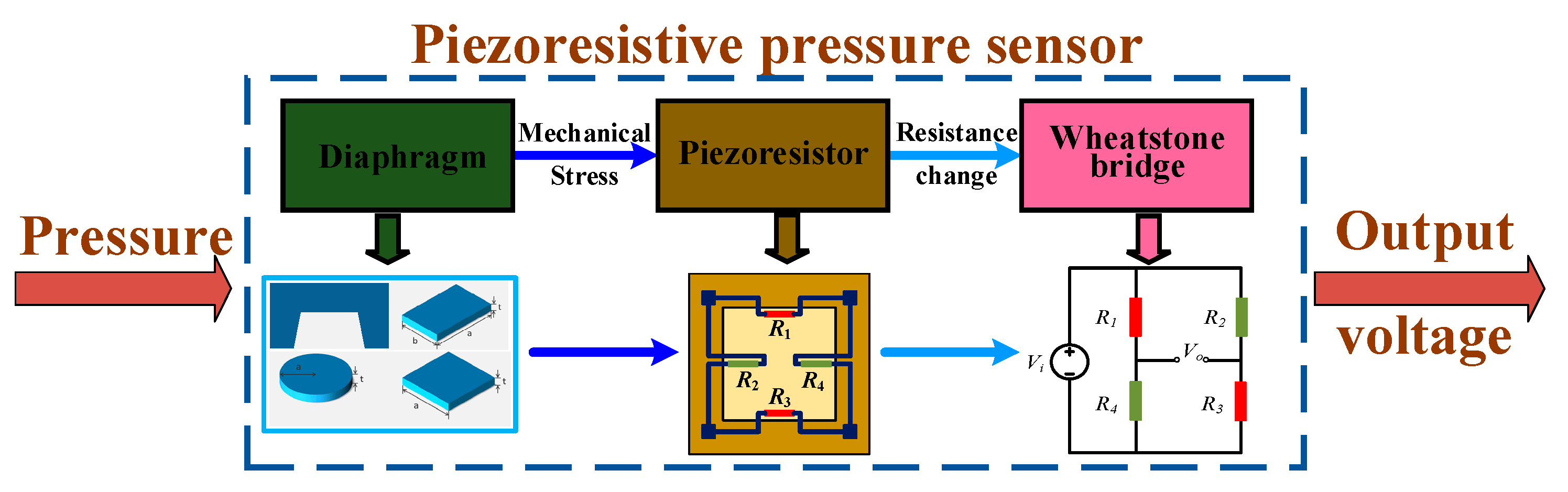
2. Fundamentals for Piezoresistive Micropressure Sensors
3. Structural Engineering in Diaphragms
3.1. Structural Engineering on the Top Layer
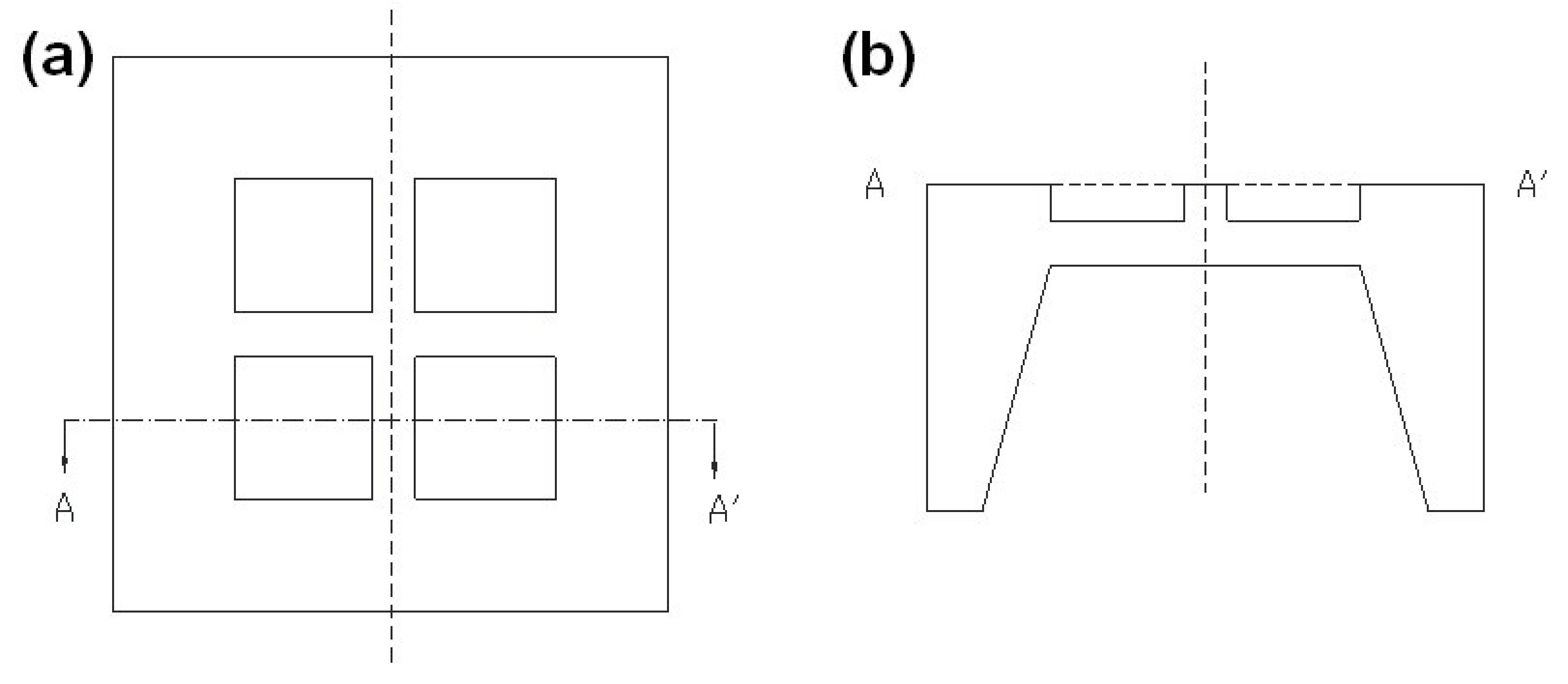
3.1.1. Beam–Membrane Structure
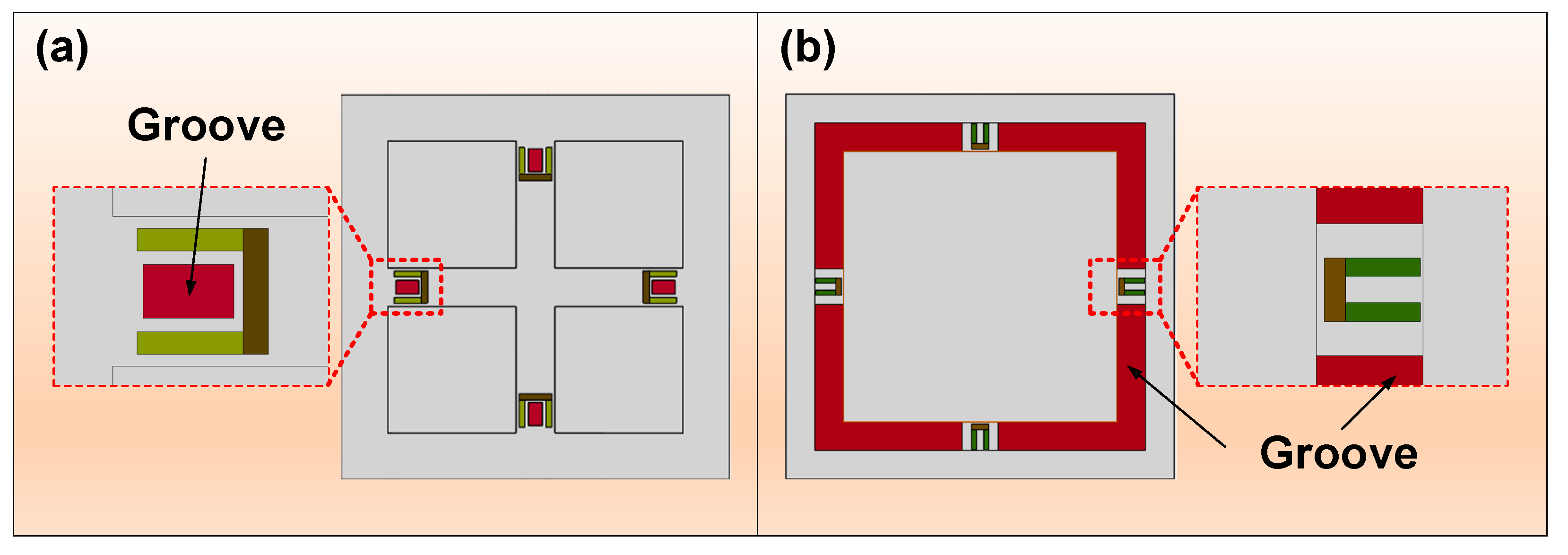
3.1.2. Groove Structure
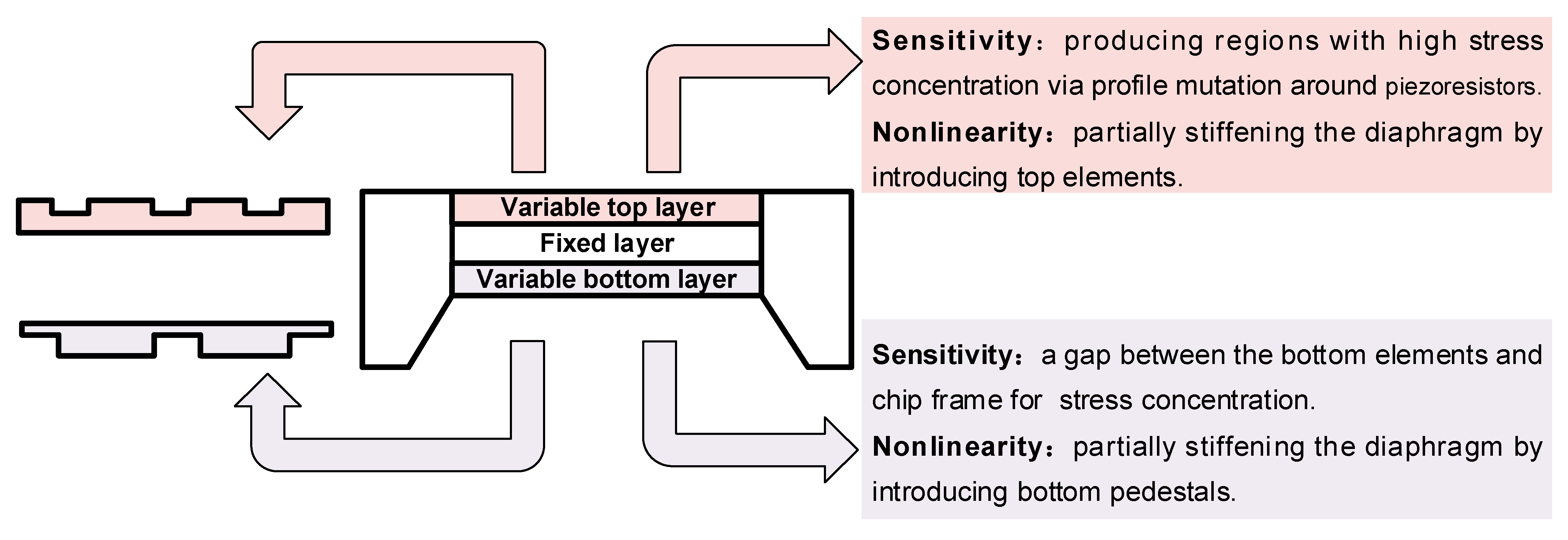
3.2. Structural Engineering on Both Layers
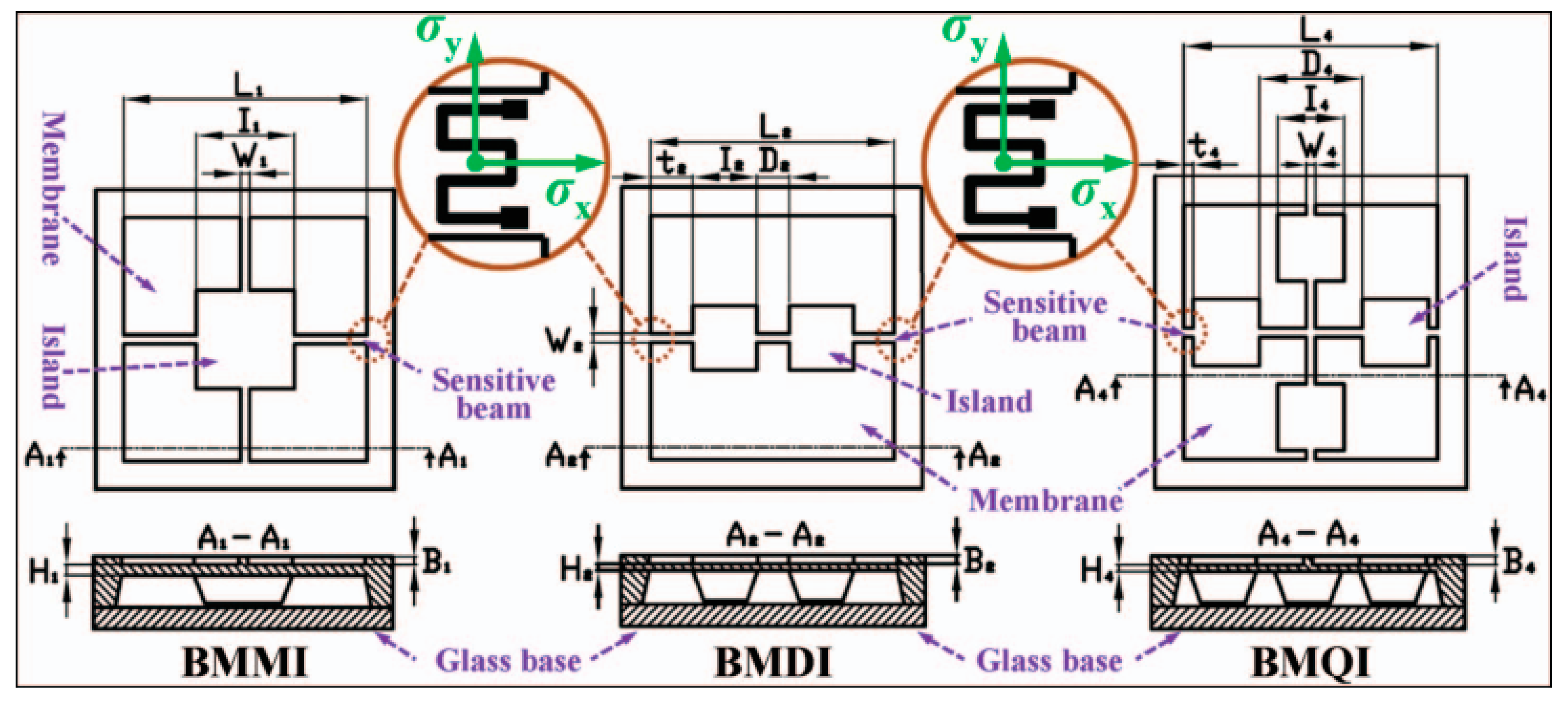
3.2.1. Available Elements for the Bottom Layer
3.2.2. Configurations for Both-Layer Scheme
3.3. Summaries for the Structural Engineering in Diaphragms
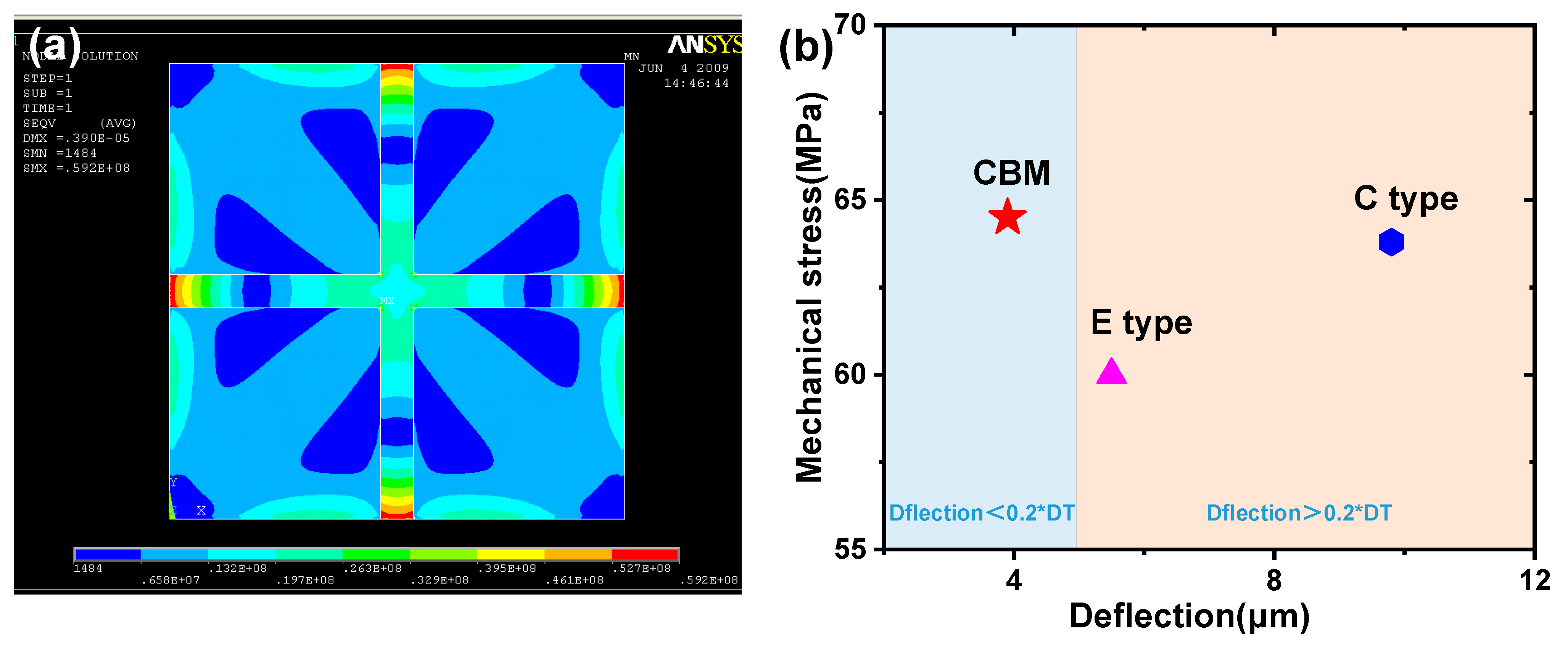
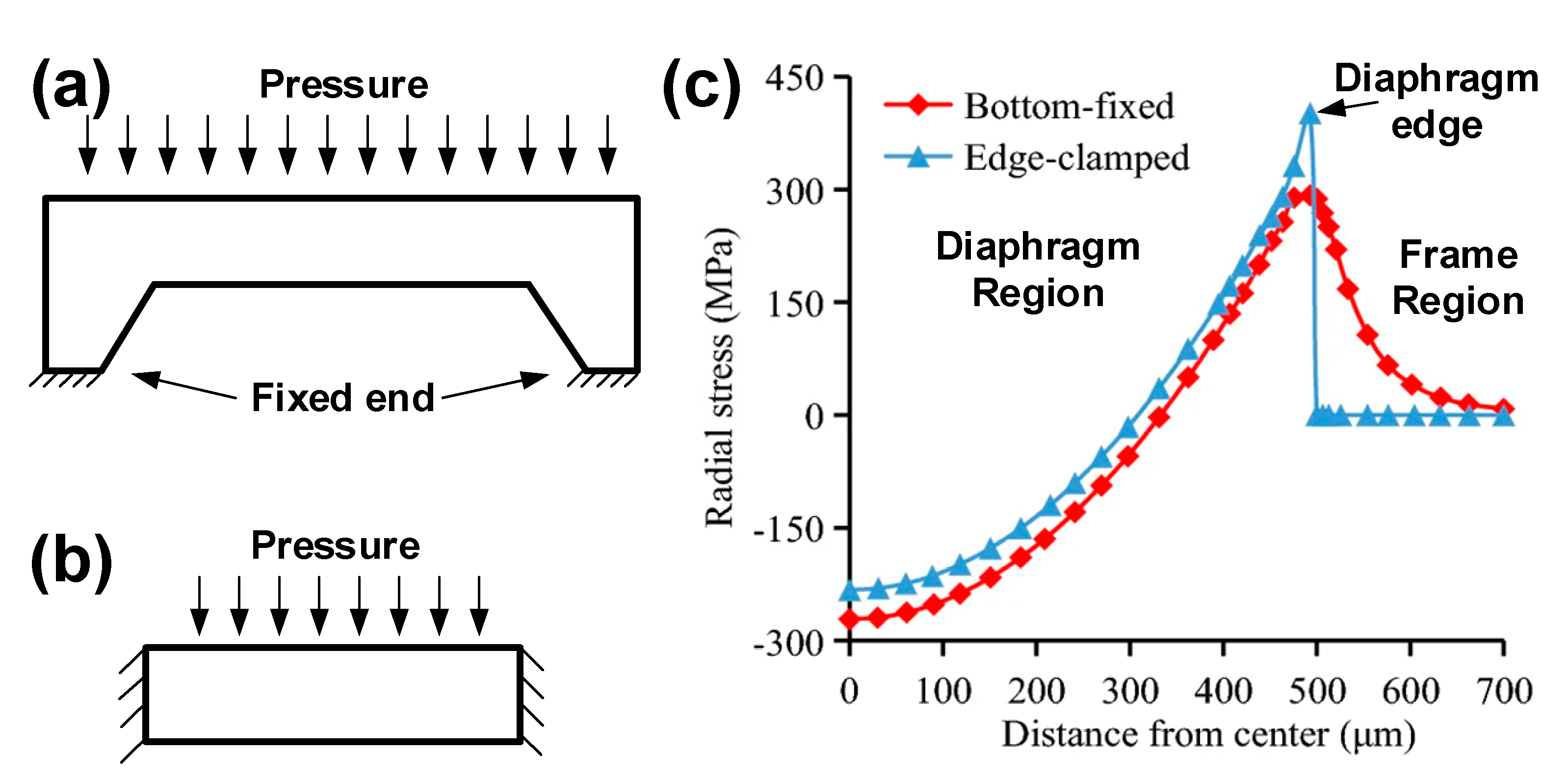
3.4. Modeling and Optimization of Diaphragms
4. Structural Engineering in Piezoresistors
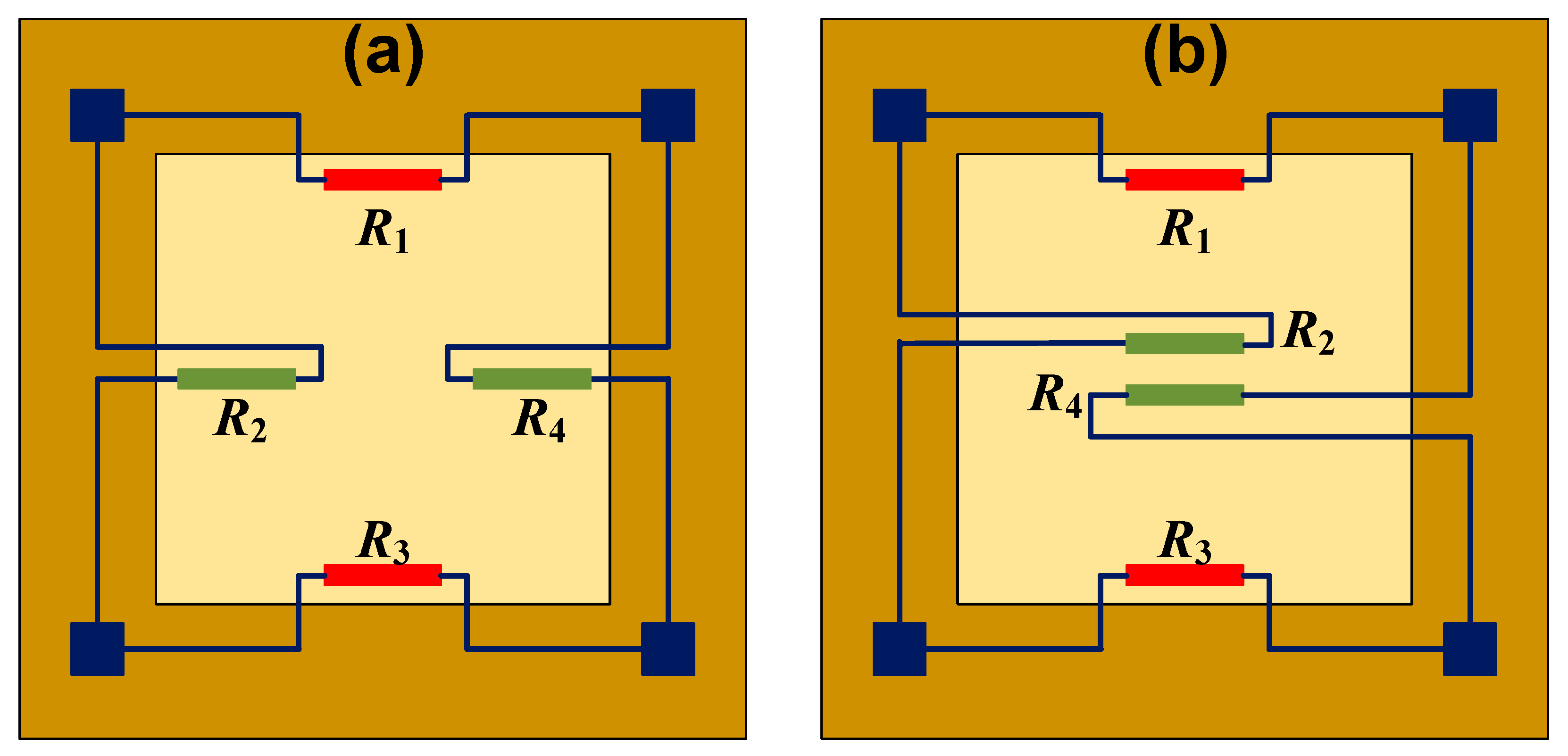
4.1. Arrangement of Piezoresistors
4.2. Shape of Piezoresistors
5. Contributions of New Materials
5.1. New Materials for Sensing Diaphragms
5.2. New Materials for Piezoresistors
5.3. Discussion on the Contributions of Flexible Conductive Materials
6. Future Trends
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Fiorillo, A.S.; Critello, C.D.; Pullano, S.A. Theory, technology and applications of piezoresistive sensors: A review. Sens. Actuators A Phys. 2018, 281, 156–175. [Google Scholar] [CrossRef]
- Li, X.; Wang, K.; Wang, Y.L.; Wang, K.C. Plantar pressure measurement system based on piezoelectric sensor: A review. Sens. Rev. 2022, 42, 241–249. [Google Scholar] [CrossRef]
- Tong, B.; Nguyen, T.-H.; Nguyen, H.-Q.; Nguyen, T.-K.; Nguyen, T.; Dinh, T.; Vo Ke Thanh, N.; Huu Ly, T.; Chi Cuong, N.; Ba Cuong, H.; et al. Highly sensitive and robust 3C-SiC/Si pressure sensor with stress amplification structure. Mater. Des. 2022, 224, 111297. [Google Scholar] [CrossRef]
- Hao, L.; Li, C.; Wang, L.; Bai, B.; Zhao, Y.; Luo, C. Design and Fabrication of a High-Temperature SOI Pressure Sensor with Optimized Crossbeam Membrane. Micromachines 2023, 14, 1045. [Google Scholar] [CrossRef]
- Li, C.; Cordovilla, F.; Jagdheesh, R.; Ocana, J.L. Design Optimization and Fabrication of a Novel Structural SOI Piezoresistive Pressure Sensor with High Accuracy. Sensors 2018, 18, 439. [Google Scholar] [CrossRef] [PubMed]
- Fang, X.; Liu, H. Measuring Micro-Friction Torque in MEMS Gas Bearings. Sensors 2016, 16, 726. [Google Scholar] [CrossRef] [PubMed]
- Liu, Y.; Zhao, Y.; Wang, W.; Sun, L.; Jiang, Z. A high-performance multi-beam microaccelerometer for vibration monitoring in intelligent manufacturing equipment. Sens. Actuators A Phys. 2013, 189, 8–16. [Google Scholar] [CrossRef]
- Chen, P.; Zhao, Y.; Tian, B.; Li, Y. Design and fluid–structure interaction analysis of a micromachined cantilever-based differential pressure flow sensor. Micro Nano Lett. 2014, 9, 650–654. [Google Scholar] [CrossRef]
- Wang, P.; Yang, Y.; Zhao, R.; Yang, F.; Wang, N.; Zhang, C.; Liang, Y. Research on Orthogonal Decagonal Ring 3-D Cutting Force Sensor. IEEE Sens. J. 2023, 23, 1042–1051. [Google Scholar] [CrossRef]
- Tian, B.; Zhao, Y.; Jiang, Z.; Zhang, L.; Liao, N.; Liu, Y.; Meng, C. Fabrication and Structural Design of Micro Pressure Sensors for Tire Pressure Measurement Systems (TPMS). Sensors 2009, 9, 1382–1393. [Google Scholar] [CrossRef]
- Meena, K.V.; Sankar, A.R. Biomedical Catheters With Integrated Miniature Piezoresistive Pressure Sensors: A Review. IEEE Sens. J. 2021, 21, 10241–10290. [Google Scholar] [CrossRef]
- Marco, S.; Samitier, J.; Ruiz, O.; Morante, J.R.; Esteve, J. High-performance piezoresistive pressure sensors for biomedical applications using very thin structured membranes. Meas. Sci. Technol. 1996, 7, 1195–1203. [Google Scholar] [CrossRef]
- Basov, M.; Prigodskiy, D.M. Investigation of High-Sensitivity Piezoresistive Pressure Sensors at Ultra-Low Differential Pressures. IEEE Sens. J. 2020, 20, 7646–7652. [Google Scholar] [CrossRef]
- Liu, Y.; Wang, H.; Zhao, W.; Qin, H.; Fang, X. Thermal-Performance Instability in Piezoresistive Sensors: Inducement and Improvement. Sensors 2016, 16, 1984. [Google Scholar] [CrossRef]
- Li, C.; Zhao, L.; Ocaña, J.L.; Cordovilla, F.; Yin, Z. Characterization and analysis of a novel structural SOI piezoresistive pressure sensor with high sensitivity and linearity. Microsyst. Technol. 2020, 26, 2955–2960. [Google Scholar] [CrossRef]
- Wu, X.-P. A new pressure sensor with innercompensation for nonlinearity and protection to overpressure. Sens. Actuators A Phys. 1990, 21, 65–69. [Google Scholar] [CrossRef]
- Kumar, S.S.; Pant, B.D. Design principles and considerations for the ‘ideal’ silicon piezoresistive pressure sensor: A focused review. Microsyst. Technol. 2014, 20, 1213–1247. [Google Scholar] [CrossRef]
- Song, P.; Ma, Z.; Ma, J.; Yang, L.; Wei, J.; Zhao, Y.; Zhang, M.; Yang, F.; Wang, X. Recent Progress of Miniature MEMS Pressure Sensors. Micromachines 2020, 11, 56. [Google Scholar] [CrossRef]
- Barlian, A.A.; Park, W.T.; Mallon, J.R., Jr.; Rastegar, A.J.; Pruitt, B.L. Review: Semiconductor Piezoresistance for Microsystems. Proc. IEEE Inst. Electr. Electron Eng. 2009, 97, 513–552. [Google Scholar] [CrossRef]
- Dong, P.; Li, X.; Yang, H.; Bao, H.; Zhou, W.; Li, S.; Feng, S. High-performance monolithic triaxial piezoresistive shock accelerometers. Sens. Actuators A Phys. 2008, 141, 339–346. [Google Scholar] [CrossRef]
- Li, T.; Shang, H.; Wang, W. Simulation and Nonlinearity Optimization of a High-Pressure Sensor. Sensors 2020, 20, 4419. [Google Scholar] [CrossRef] [PubMed]
- Yan, L.; Yulong, Z.; Sun, L. An improved structural design for accelerometers based on cantilever beam-mass structure. Sens. Rev. 2012, 32, 222–229. [Google Scholar] [CrossRef]
- Suzuki, K.; Ishihara, T.; Hirata, M.; Tanigawa, H. Nonlinear analysis of a CMOS integrated silicon pressure sensor. IEEE Trans. Electron Devices 1987, 34, 1360–1367. [Google Scholar] [CrossRef]
- Li, C.; Xie, J.; Cordovilla, F.; Zhou, J.; Jagdheesh, R.; Ocaña, J.L. Design, fabrication and characterization of an annularly grooved membrane combined with rood beam piezoresistive pressure sensor for low pressure measurements. Sens. Actuators A Phys. 2018, 279, 525–536. [Google Scholar] [CrossRef]
- Tian, B.; Zhao, Y.; Jiang, Z. The novel structural design for pressure sensors. Sens. Rev. 2010, 30, 305–313. [Google Scholar] [CrossRef]
- Tian, B.; Zhao, Y.; Jiang, Z.; Hu, B. The design and analysis of beam-membrane structure sensors for micro-pressure measurement. Rev. Sci. Instrum. 2012, 83, 045003. [Google Scholar] [CrossRef] [PubMed]
- Huang, X.; Zhang, D. A high sensitivity and high linearity pressure sensor based on a peninsula-structured diaphragm for low-pressure ranges. Sens. Actuators A Phys. 2014, 216, 176–189. [Google Scholar] [CrossRef]
- Tran, A.V.; Zhang, X.; Zhu, B. Effects of Temperature and Residual Stresses on the Output Characteristics of a Piezoresistive Pressure Sensor. IEEE Access 2019, 7, 27668–27676. [Google Scholar] [CrossRef]
- Tran, A.V.; Zhang, X.; Zhu, B. Mechanical Structural Design of a Piezoresistive Pressure Sensor for Low-Pressure Measurement: A Computational Analysis by Increases in the Sensor Sensitivity. Sensors 2018, 18, 2023. [Google Scholar] [CrossRef]
- Wu, J.; Zhao, X.; Liu, Y.; Wen, D. The simulation, fabrication technology and characteristic research of micro-pressure sensor with isosceles trapezoidal beam-membrane. Mod. Phys. Lett. B 2020, 34, 2050285. [Google Scholar] [CrossRef]
- Tran, A.V.; Zhang, X.; Zhu, B. The Development of a New Piezoresistive Pressure Sensor for Low Pressures. IEEE Trans. Ind. Electron. 2018, 65, 6487–6496. [Google Scholar] [CrossRef]
- Guan, T.; Yang, F.; Wang, W.; Huang, X.; Jiang, B.; Zhang, D. The Design and Analysis of Piezoresistive Shuriken-Structured Diaphragm Micro-Pressure Sensors. J. Microelectromechanical Syst. 2017, 26, 206–214. [Google Scholar] [CrossRef]
- Zhang, S.; Wang, T.; Lou, L.; Tsang, W.M.; Sawada, R.; Kwong, D.-L.; Lee, C. Annularly Grooved Diaphragm Pressure Sensor with Embedded Silicon Nanowires for Low Pressure Application. J. Microelectromechanical Syst. 2014, 23, 1396–1407. [Google Scholar] [CrossRef]
- Thawornsathit, P.; Juntasaro, E.; Rattanasonti, H.; Pengpad, P.; Saejok, K.; Leepattarapongpan, C.; Chaowicharat, E.; Jeamsaksiri, W. Enhancing Performance of a MEMS-Based Piezoresistive Pressure Sensor by Groove: Investigation of Groove Design Using Finite Element Method. Micromachines 2022, 13, 2247. [Google Scholar] [CrossRef]
- Gao, C.; Li, F.; Yang, F.; Zhang, D. A Novel 0–5 kPa Piezoresistive Pressure Sensor Based on Peninsula Structure Diaphragm. In Proceedings of the 2021 21st International Conference on Solid-State Sensors, Actuators and Microsystems (Transducers 2021), Virtual Conference, 20–25 June 2021; pp. 1323–1326. [Google Scholar]
- Ren, X.; Liu, X.; Su, X.; Jiang, X. Design and Optimization of a Pressure Sensor Based on Serpentine-Shaped Graphene Piezoresistors for Measuring Low Pressure. Sensors 2022, 22, 4937. [Google Scholar] [CrossRef]
- Tang, X.; Tian, J.; Zhao, J.; Jin, Z.; Liu, Y.; Liu, J.; Chen, T.; Li, J. Structure design and optimization of SOI high-temperature pressure sensor chip. Microelectron. J. 2021, 118, 105245. [Google Scholar] [CrossRef]
- Sandmaier, H.; Kuhl, K. A square-diaphragm piezoresistive pressure sensor with a rectangular central boss for low-pressure ranges. IEEE Trans. Electron Devices 1993, 40, 1754–1759. [Google Scholar] [CrossRef]
- Li, C.; Cordovilla, F.; Ocaña, J.L. Design optimization and fabrication of a novel structural piezoresistive pressure sensor for micro-pressure measurement. Solid-State Electron. 2018, 139, 39–47. [Google Scholar] [CrossRef]
- Yu, Z.; Meng, X.; Jiang, Z.; Zhao, Y.; Tian, B. Absolute micro pressure measurements based on a high-overload-resistance sensor. Micro Nano Lett. 2012, 7, 1180–1183. [Google Scholar] [CrossRef]
- Yu, Z.; Zhao, Y.; Li, L.; Li, C.; Liu, Y.; Tian, B. Realization of a micro pressure sensor with high sensitivity and overload by introducing beams and Islands. Microsyst. Technol. 2014, 21, 739–747. [Google Scholar] [CrossRef]
- Yu, Z.; Zhao, Y.; Li, L.; Li, C.; Meng, X.; Tian, B. Design optimization of a high-sensitive absolute micro-pressure sensor. Sens. Rev. 2014, 34, 312–318. [Google Scholar] [CrossRef]
- Yu, Z.; Zhao, Y.; Sun, L.; Tian, B.; Jiang, Z. Incorporation of beams into bossed diaphragm for a high sensitivity and overload micro pressure sensor. Rev. Sci. Instrum. 2013, 84, 015004. [Google Scholar] [CrossRef] [PubMed]
- Yu, Z.; Zhao, Y.; Li, L.; Tian, B.; Li, C. Geometry optimization for micro-pressure sensor considering dynamic interference. Rev. Sci. Instrum. 2014, 85, 095002. [Google Scholar] [CrossRef] [PubMed]
- Chao, D.; Chunjun, C.; Yuxiao, C. Investigation on Acceleration Effect Calibration Method of Piezoresistive Pressure Sensor Based on System Identification. Exp. Tech. 2022. [Google Scholar] [CrossRef]
- Yu, Z.; Zhao, Y.; Li, L.; Tian, B.; Cheng, R.; Li, C. Configuration improvement for micropressure sensor with vibration interference. Micro Nano Lett. 2014, 9, 680–685. [Google Scholar] [CrossRef]
- Xu, T.; Zhao, L.; Jiang, Z.; Guo, X.; Ding, J.; Xiang, W.; Zhao, Y. A high sensitive pressure sensor with the novel bossed diaphragm combined with peninsula-island structure. Sens. Actuators A Phys. 2016, 244, 66–76. [Google Scholar] [CrossRef]
- Zhao, L.; Xu, T.; Hebibul, R.; Jiang, Z.; Ding, J.; Peng, N.; Guo, X.; Xu, Y.; Wang, H.; Zhao, Y. A bossed diaphragm piezoresistive pressure sensor with a peninsula–island structure for the ultra-low-pressure range with high sensitivity. Meas. Sci. Technol. 2016, 27, 124012. [Google Scholar] [CrossRef]
- Xu, T.; Lu, D.; Zhao, L.; Jiang, Z.; Wang, H.; Guo, X.; Li, Z.; Zhou, X.; Zhao, Y. Application and Optimization of Stiffness Abruption Structures for Pressure Sensors with High Sensitivity and Anti-Overload Ability. Sensors 2017, 17, 1965. [Google Scholar] [CrossRef]
- Xu, T.; Wang, H.; Xia, Y.; Zhao, Z.; Huang, M.; Wang, J.; Zhao, L.; Zhao, Y.; Jiang, Z. Piezoresistive pressure sensor with high sensitivity for medical application using peninsula-island structure. Front. Mech. Eng. 2017, 12, 546–553. [Google Scholar] [CrossRef]
- Li, C.; Cordovilla, F.; Ocana, J.L. Annularly grooved membrane combined with rood beam piezoresistive pressure sensor for low pressure applications. Rev. Sci. Instrum. 2017, 88, 035002. [Google Scholar] [CrossRef]
- Chen, S.; Zhu, M.Q.; Ma, B.H.; Yuan, W.Z. Design and optimization of a micro piezoresistive pressure sensor. In Proceedings of the 2008 3rd IEEE International Conference on Nano/Micro Engineered and Molecular Systems, Sanya, China, 6–9 January 2008; pp. 351–356. [Google Scholar]
- Zou, H.; Wang, J.; Li, X. High-Performance Low-Range Differential Pressure Sensors Formed With a Thin-Film Under Bulk Micromachining Technology. J. Microelectromechanical Syst. 2017, 26, 879–885. [Google Scholar] [CrossRef]
- Gao, C.; Zhang, D. The Establishment and Verification of the Sensitivity Model of the Piezoresistive Pressure Sensor Based on the New Peninsula Structure. J. Microelectromechanical Syst. 2022, 31, 305–314. [Google Scholar] [CrossRef]
- Podder, I.; Fischl, T.; Bub, U. Artificial Intelligence Applications for MEMS-Based Sensors and Manufacturing Process Optimization. Telecom 2023, 4, 165–197. [Google Scholar] [CrossRef]
- Yigit, E.; Hayber, Ş.E.; Aydemir, U. ANN-based estimation of MEMS diaphragm response: An application for three leaf clover diaphragm based Fabry-Perot interferometer. Measurement 2022, 199, 111534. [Google Scholar] [CrossRef]
- Guo, R.; Sui, F.; Yue, W.; Wang, Z.; Pala, S.; Li, K.; Xu, R.; Lin, L. Deep learning for non-parameterized MEMS structural design. Microsyst. Nanoeng. 2022, 8, 91. [Google Scholar] [CrossRef]
- Niu, Z.; Zhao, Y.; Tian, B. Design optimization of high pressure and high temperature piezoresistive pressure sensor for high sensitivity. Rev. Sci. Instrum. 2014, 85, 015001. [Google Scholar] [CrossRef]
- Meng, Q.; Lu, Y.; Wang, J.; Chen, D.; Chen, J. A Piezoresistive Pressure Sensor with Optimized Positions and Thickness of Piezoresistors. Micromachines 2021, 12, 1095. [Google Scholar] [CrossRef]
- Jiao, D.; Ni, Z.; Wang, J.; Li, X. Ultra-small pressure sensors fabricated using a scar-free microhole inter-etch and sealing (MIS) process. J. Micromechanics Microengineering 2020, 30, 065012. [Google Scholar] [CrossRef]
- Tian, B.; Shang, H.; Zhao, L.; Wang, W. Performance optimization of SiC piezoresistive pressure sensor through suitable piezoresistor design. Microsyst. Technol. 2021, 27, 3083–3093. [Google Scholar] [CrossRef]
- Song, J.W.; Lee, J.S.; An, J.E.; Park, C.G. Design of a MEMS piezoresistive differential pressure sensor with small thermal hysteresis for air data modules. Rev. Sci. Instrum. 2015, 86, 065003. [Google Scholar] [CrossRef]
- Aryafar, M.; Hamedi, M.; Ganjeh, M.M. A novel temperature compensated piezoresistive pressure sensor. Measurement 2015, 63, 25–29. [Google Scholar] [CrossRef]
- Li, T.; Shang, H.; Xiao, B.; Wang, W. Influence of heavily doped connecting layers on the sensitivity of pressure sensors. Sens. Actuators A Phys. 2022, 338, 113493. [Google Scholar] [CrossRef]
- Li, T.; Xue, H.; Wang, W. A High-pressure Sensor with High Linearity with S-shaped Piezoresistors. IEEE Sens. J. 2022, 23, 1052–1059. [Google Scholar] [CrossRef]
- Phan, H.-P.; Dao, D.V.; Nakamura, K.; Dimitrijev, S.; Nguyen, N.-T. The Piezoresistive Effect of SiC for MEMS Sensors at High Temperatures: A Review. J. Microelectromechanical Syst. 2015, 24, 1663–1677. [Google Scholar] [CrossRef]
- Ser, C.T.; Mak, A.M.; Wejrzanowski, T.; Tan, T.L. Designing piezoresistive materials from first-principles: Dopant effects on 3C-SiC. Comput. Mater. Sci. 2021, 186, 110040. [Google Scholar] [CrossRef]
- Hrovat, M.; Belavič, D.; Benčan, A.; Bernard, J.; Holc, J.; Cilenšek, J.; Smetana, W.; Homolka, H.; Reicher, R.; Golonka, L.; et al. Thick-film resistors on various substrates as sensing elements for strain-gauge applications. Sens. Actuators A Phys. 2003, 107, 261–272. [Google Scholar] [CrossRef]
- Wu, J.; Zhao, X.; Ai, C.; Yu, Z.; Wen, D. The piezoresistive properties research of SiC thin films prepared by RF magnetron sputtering. Int. J. Mod. Phys. B 2019, 33, 1950152. [Google Scholar] [CrossRef]
- Li, Y.; Liang, T.; Lei, C.; Hong, Y.; Li, W.; Li, Z.; Ghaffar, A.; Li, Q.; Xiong, J. Quantitative Analysis of Piezoresistive Characteristic Based on a P-type 4H-SiC Epitaxial Layer. Micromachines 2019, 10, 629. [Google Scholar] [CrossRef]
- Peiner, E.; Tibrewala, A.; Bandorf, R.; Lüthje, H.; Doering, L.; Limmer, W. Diamond-like carbon for MEMS. J. Micromechanics Microengineering 2007, 17, S83–S90. [Google Scholar] [CrossRef]
- Biehl, S.; Rumposch, C.; Bräuer, G.; Hoffmeister, H.-W.; Luig, M. Development of a novel piezoresistive thin film sensor system based on hydrogenated carbon. Microsyst. Technol. 2014, 20, 989–993. [Google Scholar] [CrossRef]
- Xue, P.; Chen, C.; Diao, D. Ultra-sensitive flexible strain sensor based on graphene nanocrystallite carbon film with wrinkle structures. Carbon 2019, 147, 227–235. [Google Scholar] [CrossRef]
- Mehmood, Z.; Haneef, I.; Udrea, F. Material selection for optimum design of MEMS pressure sensors. Microsyst. Technol. 2019, 26, 2751–2766. [Google Scholar] [CrossRef]
- Wang, L.; Zhao, Y.; Yang, Y.; Pang, X.; Hao, L.; Zhao, Y. Piezoresistive 4H-SiC Pressure Sensor With Diaphragm Realized by Femtosecond Laser. IEEE Sens. J. 2022, 22, 11535–11542. [Google Scholar] [CrossRef]
- Wang, L.; Zhao, Y.; Yang, Y.; Pang, X.; Hao, L.; Zhao, Y.; Liu, J. Development of Laser-Micromachined 4H-SiC MEMS Piezoresistive Pressure Sensors for Corrosive Environments. IEEE Trans. Electron Devices 2022, 69, 2009–2014. [Google Scholar] [CrossRef]
- Zhao, Y.; Zhao, Y.; Wang, L.; Yang, Y.; Wang, Y. Femtosecond Laser Processing Assisted SiC High-Temperature Pressure Sensor Fabrication and Performance Test. Micromachines 2023, 14, 587. [Google Scholar] [CrossRef]
- Shang, H.; Tian, B.; Wang, D.; Liu, Y.; Wang, W. Development of All-SiC Absolute Pressure Sensor Based on Sealed Cavity Structure. IEEE Sens. J. 2021, 21, 27308–27314. [Google Scholar] [CrossRef]
- Wejrzanowski, T.; Tymicki, E.; Plocinski, T.; Bucki, J.J.; Tan, T.L. Design of SiC-Doped Piezoresistive Pressure Sensor for High-Temperature Applications. Sensors 2021, 21, 6066. [Google Scholar] [CrossRef]
- Smith, A.D.; Niklaus, F.; Paussa, A.; Vaziri, S.; Fischer, A.C.; Sterner, M.; Forsberg, F.; Delin, A.; Esseni, D.; Palestri, P.; et al. Electromechanical piezoresistive sensing in suspended graphene membranes. Nano Lett. 2013, 13, 3237–3242. [Google Scholar] [CrossRef]
- Li, M.; Wu, C.; Zhao, S.; Deng, T.; Wang, J.; Liu, Z.; Wang, L.; Wang, G. Pressure sensing element based on the BN–graphene–BN heterostructure. Appl. Phys. Lett. 2018, 112, 143502. [Google Scholar] [CrossRef]
- Janssens, S.D.; Drijkoningen, S.; Haenen, K. Ultra-thin nanocrystalline diamond membranes as pressure sensors for harsh environments. Appl. Phys. Lett. 2014, 104, 073107. [Google Scholar] [CrossRef]
- Ma, X.; Zhang, Q.; Guo, P.; Li, H.; Zhao, Y.; Wang, A. Self-Supporting Ultrathin DLC/Si3N4/SiO2 for Micro-Pressure Sensor. IEEE Sens. J. 2022, 22, 3937–3944. [Google Scholar] [CrossRef]
- Liu, Y.; Wang, H.; Zhao, W.; Zhang, M.; Qin, H.; Xie, Y. Flexible, Stretchable Sensors for Wearable Health Monitoring: Sensing Mechanisms, Materials, Fabrication Strategies and Features. Sensors 2018, 18, 645. [Google Scholar] [CrossRef]
- Balderrama, V.S.; Leon-Gil, J.A.; Fernandez-Benavides, D.A.; Ponce-Hernandez, J.; Bandala-Sanchez, M. MEMS Piezoresistive Pressure Sensor Based on Flexible PET Thin-Film for Applications in Gaseous-Environments. IEEE Sens. J. 2022, 22, 1939–1947. [Google Scholar] [CrossRef]
- Liu, M.; Zhang, Q.; Zhao, Y.; Liu, C.; Shao, Y.; Tong, X. A high sensitivity micro-pressure sensor based on 3D printing and screen-printing technologies. Meas. Sci. Technol. 2020, 31, 035106. [Google Scholar] [CrossRef]
- Glukhovskoy, A.; Prediger, M.S.; Schafer, J.; Ambrosius, N.; Vogt, A.; Santos, R.; Ostholt, R.; Wurz, M.C. Proof of Concept: Glass-Membrane Based Differential Pressure Sensor. In Proceedings of the 2021 IEEE 71st Electronic Components and Technology Conference, San Diego, CA, USA, 1–4 June 2021; pp. 1563–1570. [Google Scholar]
- Van Toan, N.; Tuoi, T.T.K.; Tsai, Y.C.; Lin, Y.C.; Ono, T. Micro-Fabricated Presure Sensor Using 50 nm-Thick of Pd-Based Metallic Glass Freestanding Membrane. Sci. Rep. 2020, 10, 10108. [Google Scholar] [CrossRef] [PubMed]
- Zhu, Z.; Wang, J.; Wu, C.; Chen, X.; Liu, X.; Li, M. A Wide Range and High Repeatability MEMS Pressure Sensor Based on Graphene. IEEE Sens. J. 2022, 22, 17737–17745. [Google Scholar] [CrossRef]
- Cai, M.-X.; Chang, J.-C.; Wang, W.-Y.; Chiu, Y.-H.; Yang, Y.-J. A Highly-Sensitive Pressure Sensor Based on Percolative Nanoparticle Arrays Formed by Dewetting Effect. In Proceedings of the 2022 IEEE 35th International Conference on Micro Electro Mechanical Systems Conference, Tokyo, Japan, 9–13 January 2022; pp. 798–801. [Google Scholar]
- Amjadi, M.; Kyung, K.-U.; Park, I.; Sitti, M. Stretchable, Skin-Mountable, and Wearable Strain Sensors and Their Potential Applications: A Review. Adv. Funct. Mater. 2016, 26, 1678–1698. [Google Scholar] [CrossRef]
- Hu, J.; Dun, G.; Geng, X.; Chen, J.; Wu, X.; Ren, T.L. Recent progress in flexible micro-pressure sensors for wearable health monitoring. Nanoscale Adv. 2023, 5, 3131–3145. [Google Scholar] [CrossRef]
- Kwak, Y.; Choi, M.; Nam, C. Design of Elastomer-Based Piezoresistive Sensors: Materials, Structural Aspects, and Prospects. ACS Appl. Electron. Mater. 2023, 5, 2912–2932. [Google Scholar] [CrossRef]
- Amjadi, M.; Pichitpajongkit, A.; Lee, S.; Ryu, S.; Park, I. Highly stretchable and sensitive strain sensor based on silver nanowire-elastomer nanocomposite. ACS Nano 2014, 8, 5154–5163. [Google Scholar] [CrossRef]
- Chen, T.; Xie, Y.; Wang, Z.; Lou, J.; Liu, D.; Xu, R.; Cui, Z.; Li, S.; Panahi-Sarmad, M.; Xiao, X. Recent Advances of Flexible Strain Sensors Based on Conductive Fillers and Thermoplastic Polyurethane Matrixes. ACS Appl. Polym. Mater. 2021, 3, 5317–5338. [Google Scholar] [CrossRef]
- Zhao, Z.; Li, Q.; Dong, Y.; Gong, J.; Li, Z.; Zhang, J. Washable Patches with Gold Nanowires/Textiles in Wearable Sensors for Health Monitoring. ACS Appl. Mater. Interfaces 2022, 14, 18884–18900. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Yue, Y.; Cheng, F.; Cheng, Y.; Ge, B.; Liu, N.; Gao, Y. Ti(3)C(2)T(x) MXene-Based Flexible Piezoresistive Physical Sensors. ACS Nano 2022, 16, 1734–1758. [Google Scholar] [CrossRef] [PubMed]
- Luo, R.; Cui, Y.; Li, H.; Wu, Y.; Du, B.; Zhou, S.; Hu, J. Fragmented Graphene Aerogel/Polydimethylsiloxane Sponges for Wearable Piezoresistive Pressure Sensors. ACS Appl. Nano Mater. 2023, 6, 7065–7076. [Google Scholar] [CrossRef]
- Xu, C.; Lu, H.; Liu, Z.; Luo, N.; Wei, A. Flexible piezoresistive sensors based on porous PDMS/CB composite materials prepared by the solvothermal method. J. Mater. Sci. Mater. Electron. 2023, 34, 906. [Google Scholar] [CrossRef]
- Liu, G.; Zhang, Z.; Li, Z.; Guo, L.; Ning, L. 0D to 2D carbon-based materials in flexible strain sensors: Recent advances and perspectives. 2D Mater. 2023, 10, 022002. [Google Scholar] [CrossRef]
- Bhakhar, S.A.; Tannarana, M.; Pataniya, P.M.; Patel, N.F.; Chauhan, B.; Solanki, G.K. Flexible paper-based piezo-resistive sensor functionalized by MoS2 nanosheets. J. Mater. Sci. Mater. Electron. 2023, 34, 994. [Google Scholar] [CrossRef]
- Ge, G.; Zhang, Y.Z.; Zhang, W.; Yuan, W.; El-Demellawi, J.K.; Zhang, P.; Di Fabrizio, E.; Dong, X.; Alshareef, H.N. Ti(3)C(2)T(x) MXene-Activated Fast Gelation of Stretchable and Self-Healing Hydrogels: A Molecular Approach. ACS Nano 2021, 15, 2698–2706. [Google Scholar] [CrossRef]
- Zhang, J.; Zhang, Q.; Liu, X.; Xia, S.; Gao, Y.; Gao, G. Flexible and wearable strain sensors based on conductive hydrogels. J. Polym. Sci. 2022, 60, 2663–2678. [Google Scholar] [CrossRef]
- Ge, G.; Mandal, K.; Haghniaz, R.; Li, M.; Xiao, X.; Carlson, L.; Jucaud, V.; Dokmeci, M.R.; Ho, G.W.; Khademhosseini, A. Deep Eutectic Solvents-based Ionogels with Ultrafast Gelation and High Adhesion in Harsh Environments. Adv. Funct. Mater. 2023, 33, 2207388. [Google Scholar] [CrossRef] [PubMed]
- Li, J.; Ding, Q.; Wang, H.; Wu, Z.; Gui, X.; Li, C.; Hu, N.; Tao, K.; Wu, J. Engineering Smart Composite Hydrogels for Wearable Disease Monitoring. Nanomicro Lett. 2023, 15, 105. [Google Scholar] [CrossRef] [PubMed]
- Ge, G.; Wang, Q.; Zhang, Y.Z.; Alshareef, H.N.; Dong, X. 3D Printing of Hydrogels for Stretchable Ionotronic Devices. Adv. Funct. Mater. 2021, 31, 2107437. [Google Scholar] [CrossRef]
- Yihan, C.; Siyu, L.; Yixin, L.; Zhiling, Y.; Yutong, D.; Philip, Y.S.; Lichao, S. A Comprehensive Survey of AI-Generated Content (AIGC): A History of Generative AI from GAN to ChatGPT. arxiv 2023, arXiv:2303.04226. [Google Scholar] [CrossRef]
- Zhao, X.; Zhao, S.; Zhang, X.; Su, Z. Recent progress in flexible pressure sensors based on multiple microstructures: From design to application. Nanoscale 2023, 15, 5111–5138. [Google Scholar] [CrossRef]
- Ling, Y.; Zhao, G.; Su, Y.; Wu, Q.; Xu, Y.; Chen, Z.; Arends, B.; Emeje, O.; Huang, G.; Xie, J.; et al. Skin-Inspired Porous Mesh Bioelectronics with Built-In Multifunctionality for Concurrently Monitoring Heart Electrical and Mechanical Functions. Adv. Funct. Mater. 2023. [Google Scholar] [CrossRef]




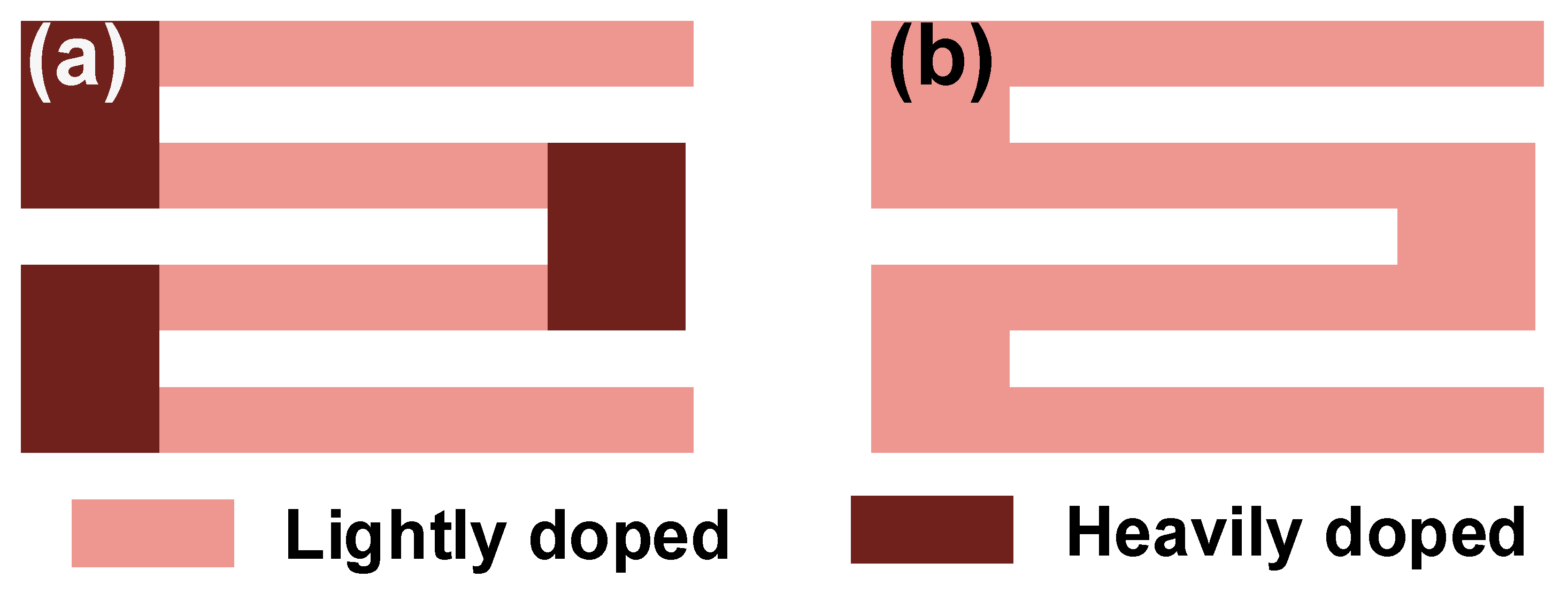

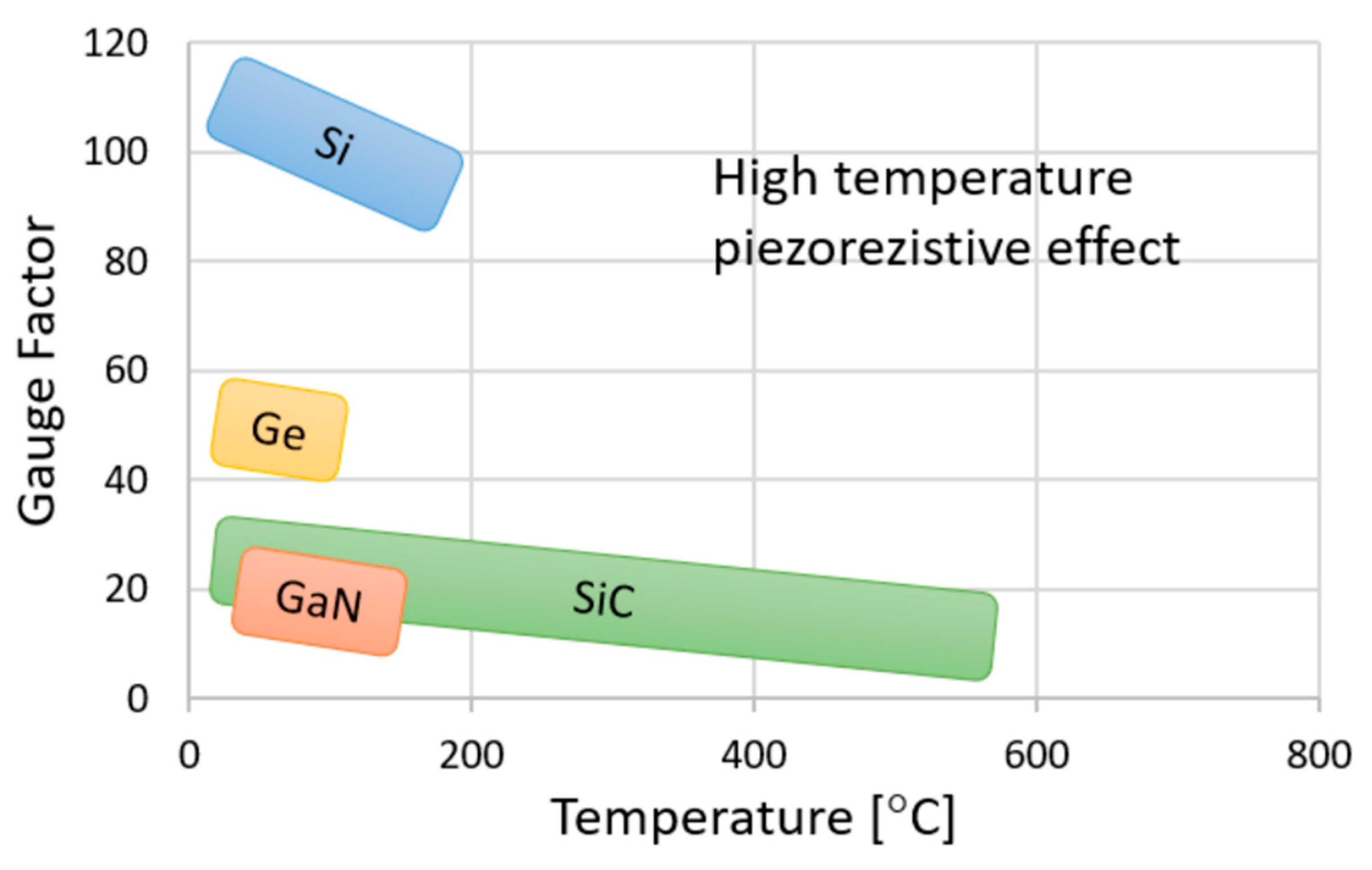
| Type | DC | DA (μm2) | Range (kPa) | SEN (mV/(V·kPa)) | NL (/%FS) | Ref. |
|---|---|---|---|---|---|---|
| Null | Flat | 1150 × 1150 | 100 | 0.23 | 0.58 | [52] |
| Only top layer | CBM | 2900 × 2900 | 10 | 1.61 | 0.19 | [25] |
| Peninsula-structured | 1900 × 1900 | 5 | 3.68 | 0.36 | [27] | |
| Shuriken-structured | 1900 × 1900 | 3 | 4.72 | 0.18 | [32] | |
| Modified beam–island | 950 μm a | 1.2 | 3.654 | 0.05 | [53] | |
| CBMP | 2900 × 2900 | 5 | 5.16 | 0.28 | [31] | |
| ITBM | 4000 × 4000 | 3 | 1.928 | 0.09 | [30] | |
| NPSD with groove | 1900 × 1900 | 5 | 4.54 | 0.11 | [54] | |
| Both top and bottom layers | Top groove and bottom peninsula | 3500 × 3500 | 0.5 | 65 | 0.33 | [47] |
| BMMI | 5600 × 5600 | 0.5 | 11.1 | 0.196 | [43] | |
| BMQI | 5600 × 5600 | 0.5 | 17.8 | 0.14 | [41] | |
| BMDI | 5600 × 5600 | 0.5 | 16.1 | 0.259 | [42] | |
| Top groove and bottom rood beam | 3600 × 3600 | 6.89 | 4.48 | 0.25 | [24] | |
| CBMP+ petal | 3100 × 3100 | 5 | 6.934 | 0.23 b | [29] |
| Property | Inorganic Semiconductor | Organic Composite | Relevant Sensing Parameter |
|---|---|---|---|
| Modulus | Low | High | Operating frequency |
| Allowable Temperature | Lower than 100 °C | Higher than 200 °C | Operating temperature |
| Durability | Medium | High | Performance stability under different conditions |
| Long-term stability | Medium | High | Longstanding stability for measuring capacity |
| Hysteresis | High | Low | Measurement accuracy |
| Creepage | High | Low | Measurement accuracy |
| Operation strain | High | Very low | Stretchability and linearity |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Y.; Jiang, X.; Yang, H.; Qin, H.; Wang, W. Structural Engineering in Piezoresistive Micropressure Sensors: A Focused Review. Micromachines 2023, 14, 1507. https://doi.org/10.3390/mi14081507
Liu Y, Jiang X, Yang H, Qin H, Wang W. Structural Engineering in Piezoresistive Micropressure Sensors: A Focused Review. Micromachines. 2023; 14(8):1507. https://doi.org/10.3390/mi14081507
Chicago/Turabian StyleLiu, Yan, Xin Jiang, Haotian Yang, Hongbo Qin, and Weidong Wang. 2023. "Structural Engineering in Piezoresistive Micropressure Sensors: A Focused Review" Micromachines 14, no. 8: 1507. https://doi.org/10.3390/mi14081507
APA StyleLiu, Y., Jiang, X., Yang, H., Qin, H., & Wang, W. (2023). Structural Engineering in Piezoresistive Micropressure Sensors: A Focused Review. Micromachines, 14(8), 1507. https://doi.org/10.3390/mi14081507







