Laser Metal Deposition of Rene 80—Microstructure and Solidification Behavior Modelling †
Abstract
1. Introduction
2. Analytical Model
3. Experimental Work
3.1. Material
3.2. Laser Metal Deposition
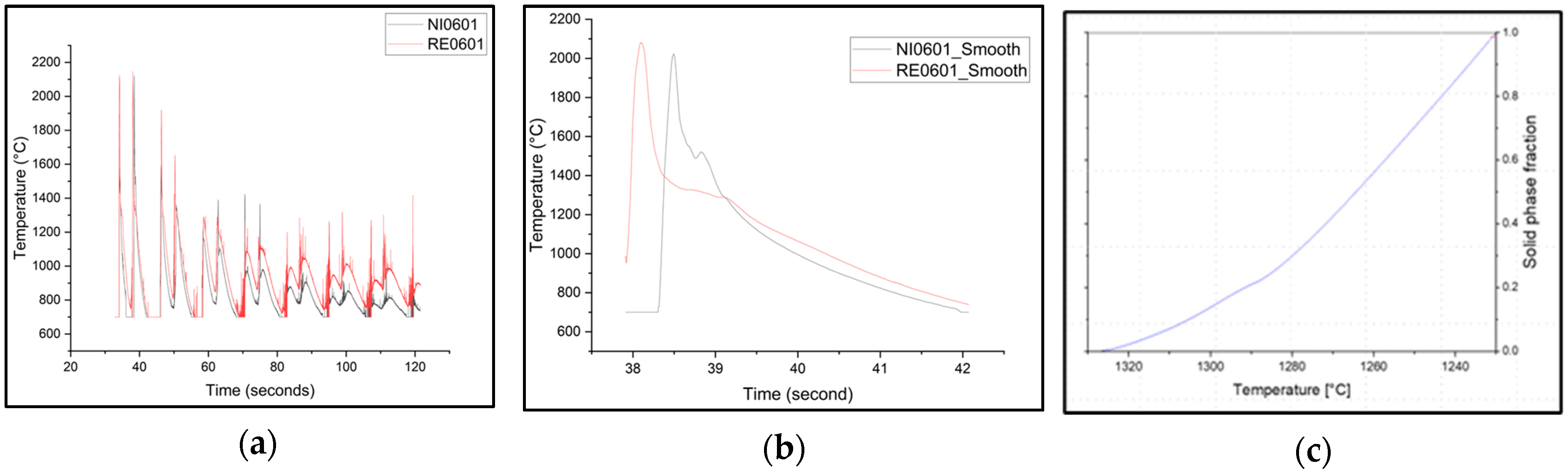
3.3. Temperature Measurement for LMD
3.4. Differential Scanning Calorimetry (DSC)
3.5. Thermodynamic Calculations
3.6. Microstructure Characterization
4. Results and Discussion
4.1. Thermo-Calc Calculations
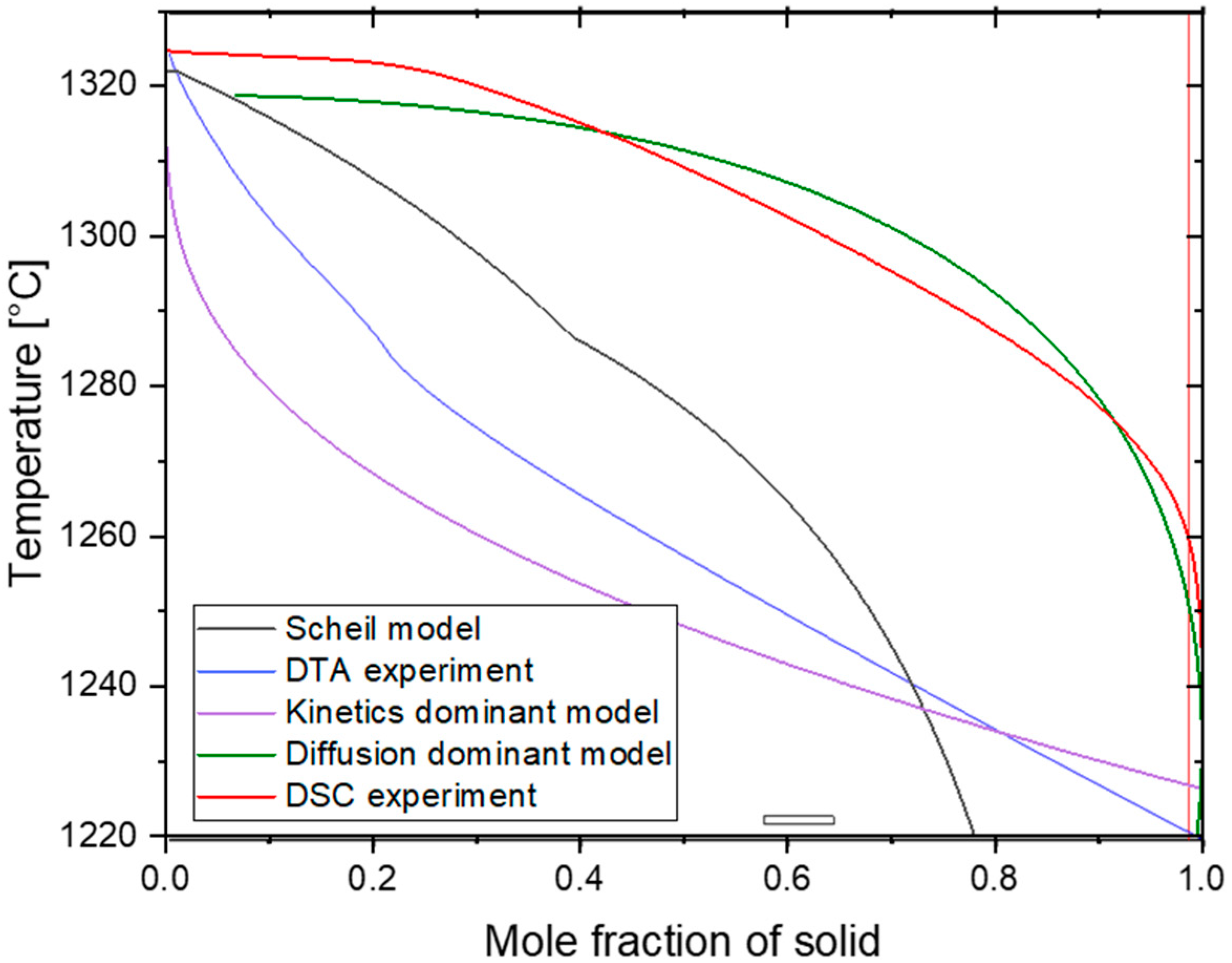
4.2. Differential Scanning Calorimetry Analysis
4.3. LMD Thermal Cycles and Differential Thermal Analysis (DTA)
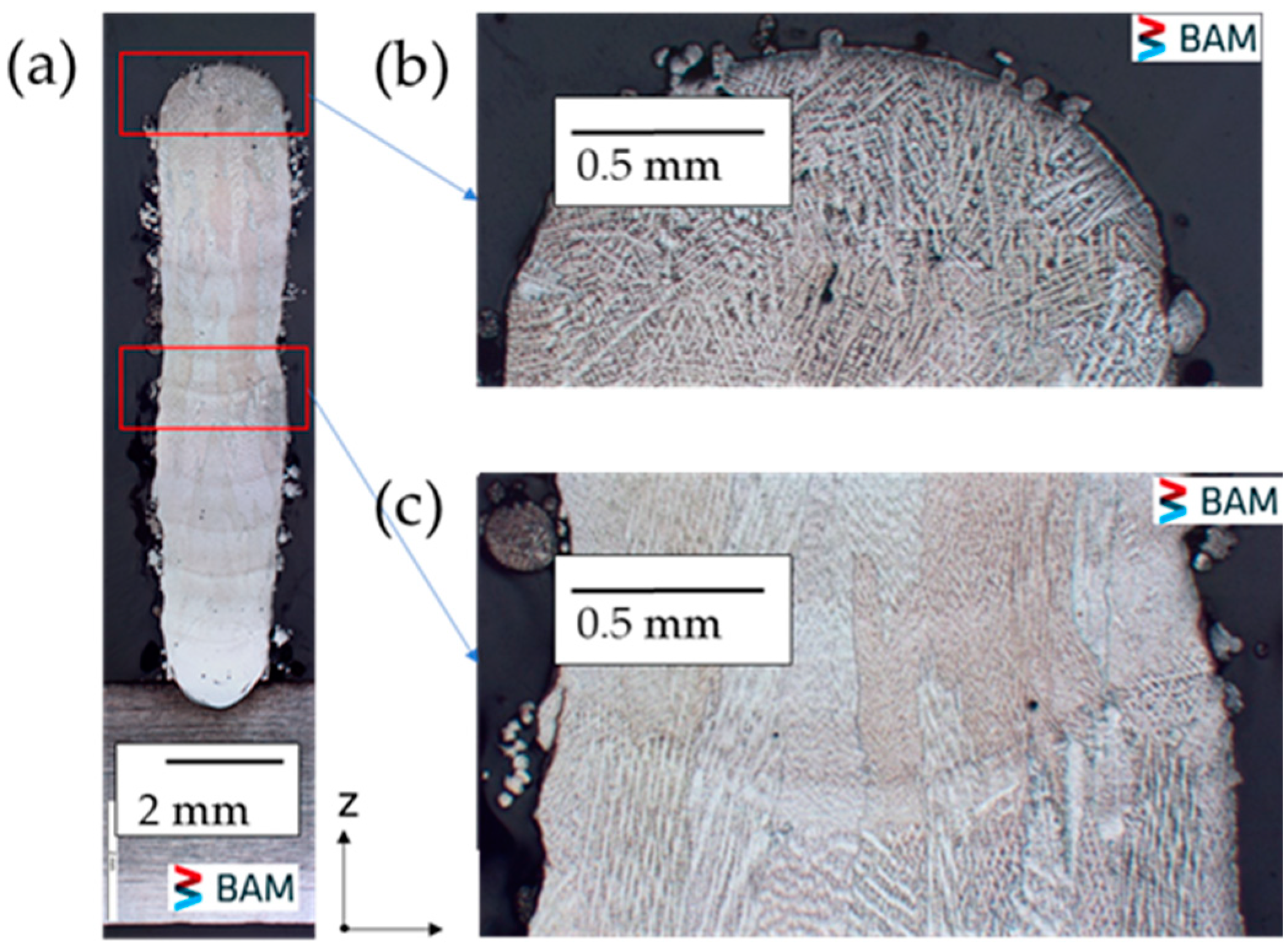
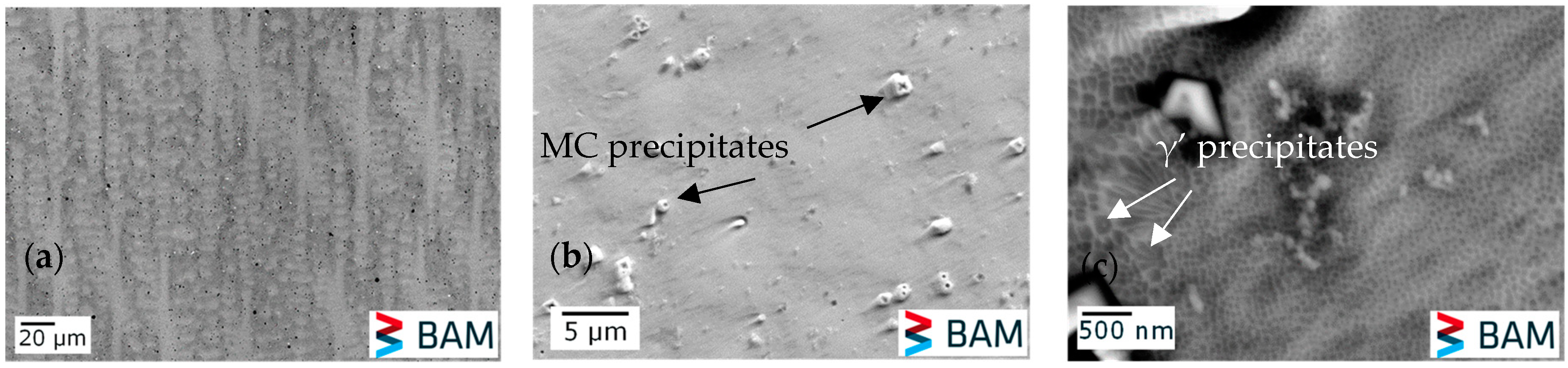
4.4. Microstructure Characterization
4.5. Validation of the Analytical Model
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
References
- Sun, C.; Wang, Y.; Mcmurtrey, M.; Jerred, N.; Liou, F.; Li, J. Additive Manufacturing for Energy: A review. Appl. Energy 2021, 282, 116041. [Google Scholar] [CrossRef]
- Siemens. Siemens and E.ON Reach Milestone with 3D-Printed Burner for SGT-700 Gas Turbine. (19 September 2018). Available online: https://press.siemens.com/global/en/pressrelease/siemens-and-eon-reach-milestone-3d-printed-burner-sgt-700-gas-turbine (accessed on 24 June 2023).
- DuPont, J.N.; Lippold, J.C. Welding metallurgy and weldability of nickel-base alloys. J. Chem. Inf. Model. 2009, 53, 456. [Google Scholar]
- Li, J.; Wang, H.M. Microstructure and mechanical properties of rapid directionally solidified Ni-base superalloy Rene’41 by laser melting deposition manufacturing. Mater. Sci. Eng. A 2010, 527, 4823–4829. [Google Scholar] [CrossRef]
- Carter, J.L.N.; Attallah, M.M.; Reed, R.C. Laser powder bed fabrication of nickel-base superalloys: Influence of parameters; characterisation, quantification and mitigation of cracking. In Proceedings of the Superalloys 2012: The 12th International Symposium on Superalloys, Champion, PA, USA, 9–13 September 2012; pp. 577–586. [Google Scholar]
- Mignanelli, M.; Jones, G.; Pickering, J.; Messe, M.; Rae, F.; Stone, J. Gamma-gamma prime-gamma double prime dual-superlattice superalloys. Scr. Mater. 2017, 136, 136–140. [Google Scholar] [CrossRef]
- Martelli, P.A.; Sivo, A.; Calignano, F.; Bassini, E.; Biamino, S.; Ugues, D. Parameters Optimization and Repeatability Study on Low-Weldable Nickel-Based Superalloy René 80 Processed via Laser Powder–Bed Fusion (L-PBF). Metals 2023, 13, 210. [Google Scholar] [CrossRef]
- Huarte-Mendicoa, L.; Penaranda, X.; Lamikiz, A.; Moralejo, S. Experimental Microstructure Evaluation of Rene 80 in Laser Cladding. Lasers Manuf. Mater. Process. 2019, 6, 317–331. [Google Scholar] [CrossRef]
- Kitt, A.L.; Mohr, L.; Kerwin, L.; Bhaduri, A.; Seyyedhosseinzadeh, H.; Dhakad, A.; Kmiotek, D.; Shen, C.; Huang, S.; Kiedrowski, A.; et al. Physics-based crack formation model for René 80 in laser blown directed energy deposition: Theory and experiment. Addit. Manuf. 2023, 73, 103675. [Google Scholar] [CrossRef]
- Yin, H.; Felicelli, S.D. Dendrite growth simulation during solidification in the LENS process. Acta Mater. 2010, 58, 1455–1465. [Google Scholar] [CrossRef]
- Nie, P.; Ojo, O.A.; Li, Z. Numerical modeling of microstructure evolution during laser additive manufacturing of a nickel-based superalloy. Acta Mater. 2014, 77, 85–95. [Google Scholar] [CrossRef]
- Keller, T.; Lindwall, G.; Ghosh, S.; Ma, L.; Lane, B.M.; Zhang, F.; Kattner, U.R.; Lass, E.A.; Heigel, J.C.; Idell, Y.; et al. Application of finite element, phase-field, and calphad-based methods to additive manufacturing of Ni-based superalloys. Acta Mater. 2017, 139, 244–253. [Google Scholar] [CrossRef] [PubMed]
- Wang, X.; Liu, P.W.; Ji, Y.; Liu, Y.; Horstemeyer, M.H.; Chen, L. Investigation on microsegregation of IN718 alloy during additive manufacturing via integrated phase-field and finite-element modeling. J. Mater. Eng. Perform 2018, 28, 657–665. [Google Scholar] [CrossRef]
- Ghosh, S.; Ma, L.; Ofori-Opoku, N.; Guyer, J.E. On the primary spacing and microsegregation of cellular dendrites in laser deposited Ni–Nb alloys. Model. Simul. Mater. Sci. Eng. 2017, 25, 065002. [Google Scholar] [CrossRef]
- Xiao, W.; Li, S.; Wang, C.; Shi, Y.; Mazumder, J.; Xing, H.; Song, L. Multi-scale simulation of dendrite growth for direct energy deposition of nickel-based superalloys. Mater. Des. 2019, 164, 107553. [Google Scholar] [CrossRef]
- Kumara, C.; Segerstark, A.; Hanning, F.; Dixit, N.; Joshi, S.; Moverare, J.; Nylen, P. Microstructure modelling of laser metal powder directed energy deposition of alloy 718. Addit. Manuf. 2019, 25, 357–364. [Google Scholar] [CrossRef]
- Chen, S.-L.; Yang, Y.; Chen, S.-W.; Lu, X.-G.; Chang, Y.A. Solidification Simulation Using Scheil Model in Multicomponent Systems. J. Phase Equilibria Diffus. 2009, 30, 429–434. [Google Scholar] [CrossRef]
- Aziz, M.J. Model for solute redistribution during rapid solidification. J. Appl. Phys. 1982, 53, 1158–1168. [Google Scholar] [CrossRef]
- Srinivasan, K.; Gumenyuk, A.; Rethmeier, M. Laser Metal Deposition of Rene 80—Microstructure and Solidification Behaviour Modelling. In Proceedings of the Laser in Manufacturing Conference, Munich, Germany, 26–29 June 2023. [Google Scholar]
- Pelton, A.D. Equilibrium and Scheil-Gulliver Solidification. In Phase Diagrams and Thermodynamic Modeling of Solutions; Elsevier: Amsterdam, The Netherlands, 2019; pp. 133–148. [Google Scholar]
- Park, J.U.; Jun, S.Y.; Lee, B.H.; Jang, J.H.; Lee, B.S.; Lee, H.J.; Lee, J.H.; Hong, H.U. Alloy design of Ni-based superalloy with high γ′ volume fraction suitable for additive manufacturing and its deformation behavior. Addit. Manuf. 2022, 52, 102680. [Google Scholar] [CrossRef]
- Carslaw, H.S.; Jaeger, J.C. Conduction of Heat in Solids; Oxford University: Oxford, UK, 1959; p. 202. [Google Scholar]






| Parameter | Value |
|---|---|
| Diffusion coefficient, D | 5 × 10−5 cm2/s |
| Kinetic constant, K | 0.026 cm/s |
| DAS, 2 a | 6.5 µm |
| Initial concentration, C0 | 0.05 |
| Material | Cr | Co | Ti | W | Mo | Al | Ni |
|---|---|---|---|---|---|---|---|
| Rene 80 in wt.-% | 14.2 | 9.6 | 5.1 | 4.1 | 4.1 | 3 | Bal. |
| Pure Ni in wt.-% | - | - | - | - | - | - | 99.8 |
| Number | Power P (W) | Scan Speed v (mm/min) | Feed Rate m (g/min) | Spot Diameter s (mm) |
|---|---|---|---|---|
| RE0601 | 800 | 600 | 15 | 1.6 |
| NI0601 | 800 | 600 | 15 | 1.6 |
| RE0602 | 1000 | 600 | 15 | 1.6 |
| RE0809 | 800 | 800 | 21 | 2.0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Srinivasan, K.; Gumenyuk, A.; Rethmeier, M. Laser Metal Deposition of Rene 80—Microstructure and Solidification Behavior Modelling. Micromachines 2024, 15, 1234. https://doi.org/10.3390/mi15101234
Srinivasan K, Gumenyuk A, Rethmeier M. Laser Metal Deposition of Rene 80—Microstructure and Solidification Behavior Modelling. Micromachines. 2024; 15(10):1234. https://doi.org/10.3390/mi15101234
Chicago/Turabian StyleSrinivasan, Krishnanand, Andrey Gumenyuk, and Michael Rethmeier. 2024. "Laser Metal Deposition of Rene 80—Microstructure and Solidification Behavior Modelling" Micromachines 15, no. 10: 1234. https://doi.org/10.3390/mi15101234
APA StyleSrinivasan, K., Gumenyuk, A., & Rethmeier, M. (2024). Laser Metal Deposition of Rene 80—Microstructure and Solidification Behavior Modelling. Micromachines, 15(10), 1234. https://doi.org/10.3390/mi15101234







