A Low-Frequency Multi-Band Piezoelectric MEMS Acoustic Sensor Inspired by Ormia ochracea
Abstract
1. Introduction
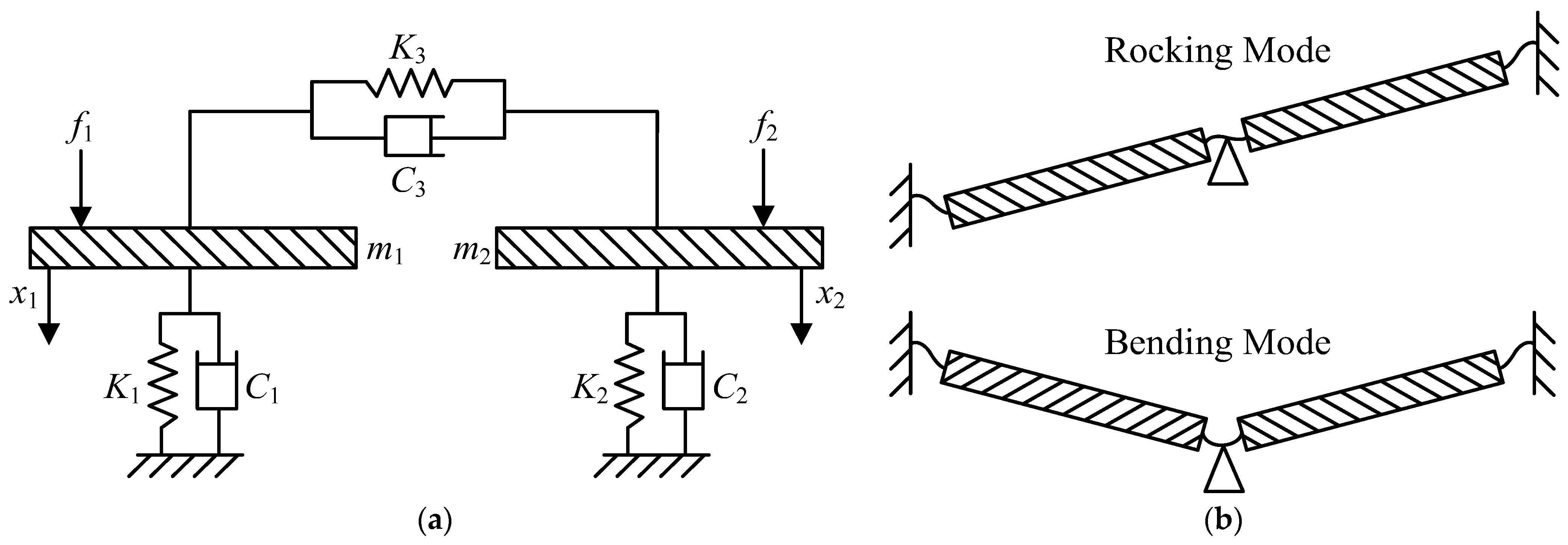
- A mechanical analysis is conducted to reveal the coupled vibration characteristics of the Ormia ochracea auditory system, demonstrating that the rocking mode is driven by the interaural pressure difference, while the bending mode is driven by the total pressure.
- An improved inter-tympanal bridge coupling structure is proposed to enable low-frequency detection in a miniaturized design.
- An asymmetric wing design is introduced to enhance the rocking mode response, while a coupled structure of two diaphragm pairs enables multi-band operation.
- AlN is adopted as the piezoelectric material in the d₃₃ mode, and a branched comb interdigitated electrode structure is designed to improve the SNR.
- Simulation results confirm that the sensor’s four eigenfrequencies are evenly distributed below 2000 Hz and exhibit a consistent cosine directional response to the incident sound source at all eigenfrequencies.
2. Mechanical Analysis of the Ormia ochracea Auditory System
2.1. Mechanical Model Development
2.2. Relationship Between Tympanic Membrane Response and Sound Source Direction
3. Improved Coupling Structure Design
4. Multi-Band Response Design
4.1. Asymmetric Design for Enhanced Rocking Mode Response
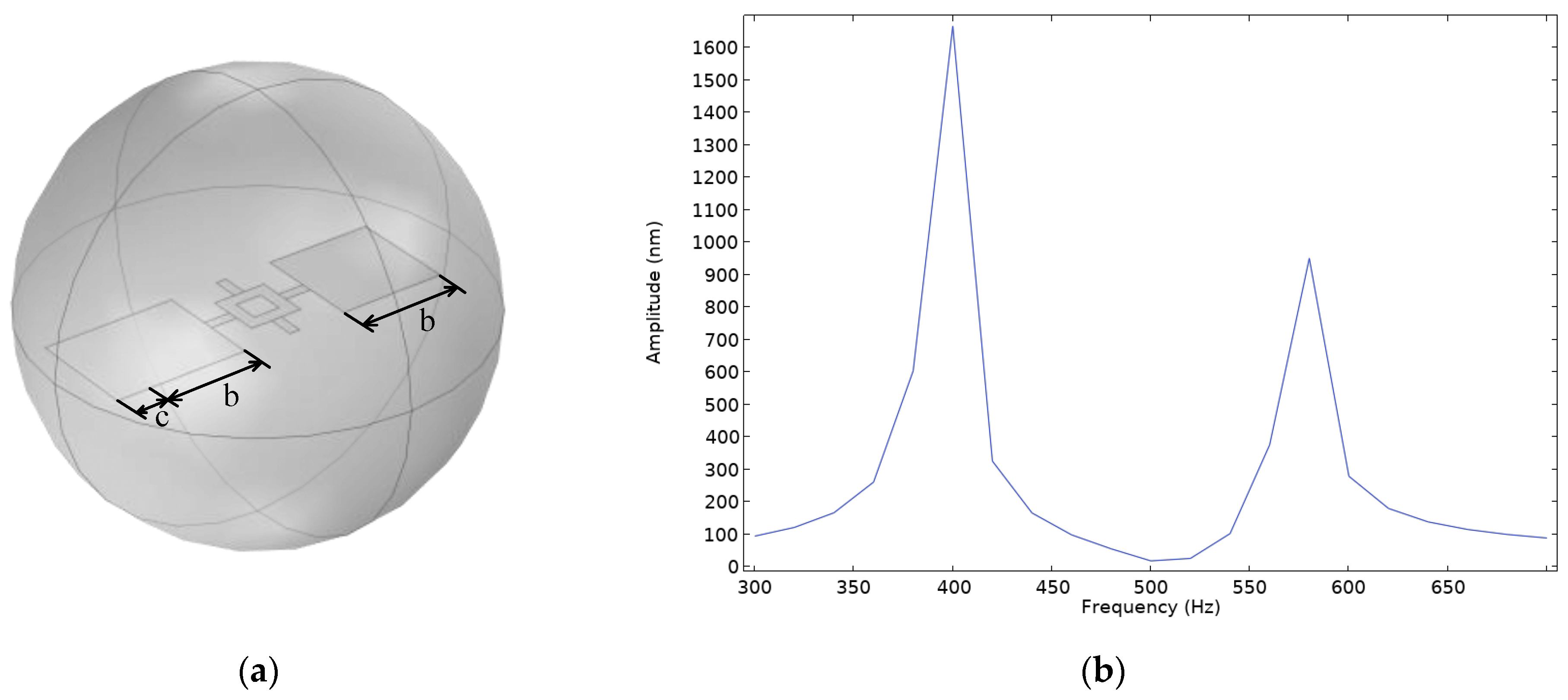
4.2. Coupled Design of Inner and Outer Diaphragms
- Coupled structure A where both the inner and outer diaphragms adopt Model A;
- Coupled structure B where both the inner and outer diaphragms adopt Model D;
- Coupled structure C where the inner diaphragm adopts Model A, while the outer diaphragm adopts Model D;
- Coupled structure D where the inner diaphragm adopts Model D, while the outer diaphragm adopts Model A.
- Coupled structure A where the eigenfrequencies of the outer diaphragm in the rocking and bending modes are 636.0 Hz and 956.7 Hz, respectively, while those of the inner diaphragm are 2894.4 Hz and 8458.2 Hz, respectively;
- Coupled structure B where the eigenfrequencies of the outer diaphragm in the rocking and bending modes are 186.5 Hz and 232.3 Hz, respectively, while those of the inner diaphragm are 1719.7 Hz and 2664.6 Hz, respectively;
- Coupled structure C where the eigenfrequencies of the outer diaphragm in the rocking and bending modes are 189.6 Hz and 246.9 Hz, respectively, while those of the inner diaphragm are 2958.9 Hz and 8763.4 Hz, respectively;
- Coupled structure D where the eigenfrequencies of the outer diaphragm in the rocking and bending modes are 451.6 Hz and 870.8 Hz, respectively, while those of the inner diaphragm are 1728.5 Hz and 2016.0 Hz, respectively.
- Even distribution of eigenfrequencies where the four eigenfrequencies are evenly distributed across the low- and mid-frequency ranges;
- Suitability for low-frequency detection where all eigenfrequencies are below 2000 Hz, making it well-suited for low-frequency signal detection;
- Minimization of interfering modes where compared to other coupled configurations, coupled structure D exhibits purer eigenmodes within the target detection frequency range, reducing the likelihood of interference. For example, coupled structures A and B contain additional interfering modes, as illustrated in Figure 10, with eigenfrequencies of 2939.2 Hz and 1210.5 Hz, respectively, which may affect detection performance.
5. Piezoelectric Sensing Design
5.1. Selection of Piezoelectric Material and Operating Mode
- In the d₃₁ mode, the direction of stress applied to the piezoelectric thin film is perpendicular to the direction in which the electric charge is generated. As a result, the electrodes must be placed on the top and bottom surfaces of the piezoelectric thin film, and the output signal amplitude is proportional to the film thickness. However, due to the thin nature of MEMS-fabricated piezoelectric thin films, the output in this mode is significantly limited.
- In contrast, the d₃₃ mode aligns the stress direction with the charge generation di-rection, requiring only IDTs on the top surface of the film. In this mode, the output signal strength can be optimized by adjusting the electrode spacing, making it independent of the film thickness.
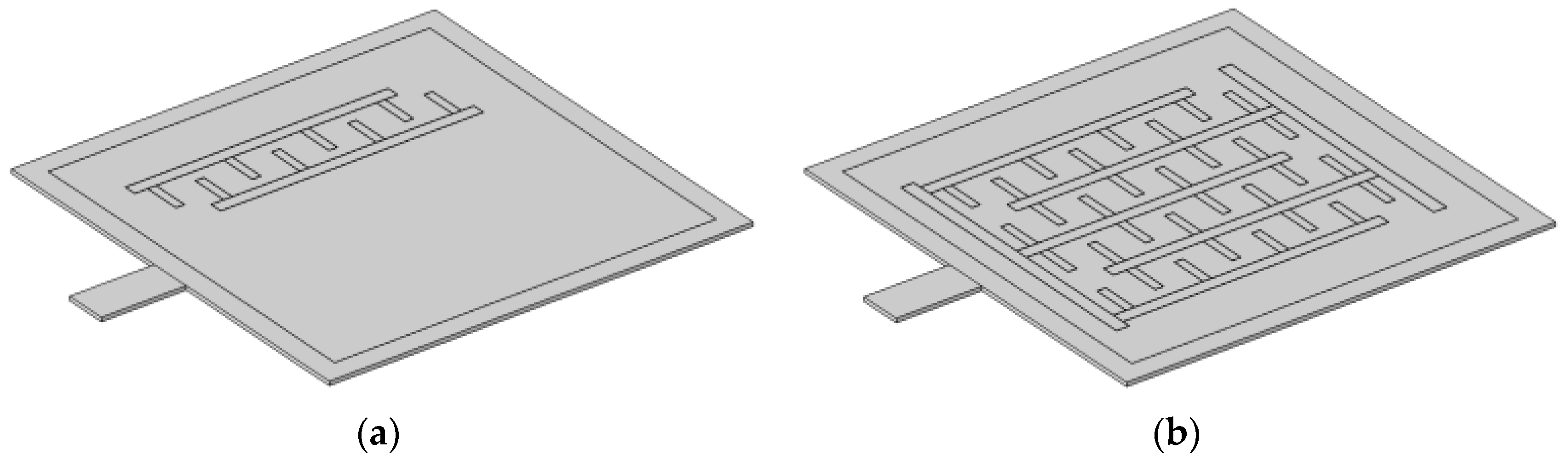
5.2. Design of Branched Comb-Shaped Interdigitated Electrodes
6. Simulation Validation
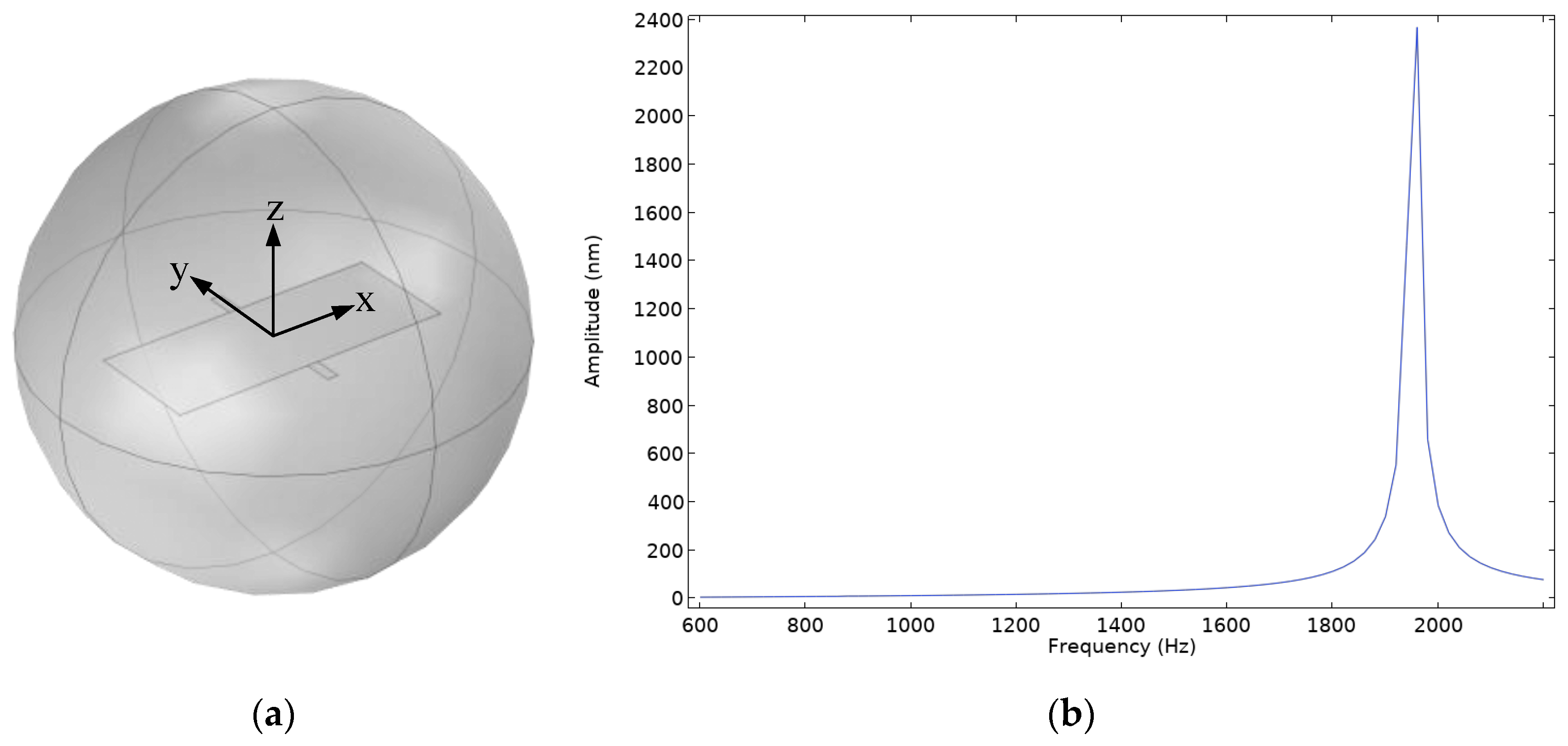
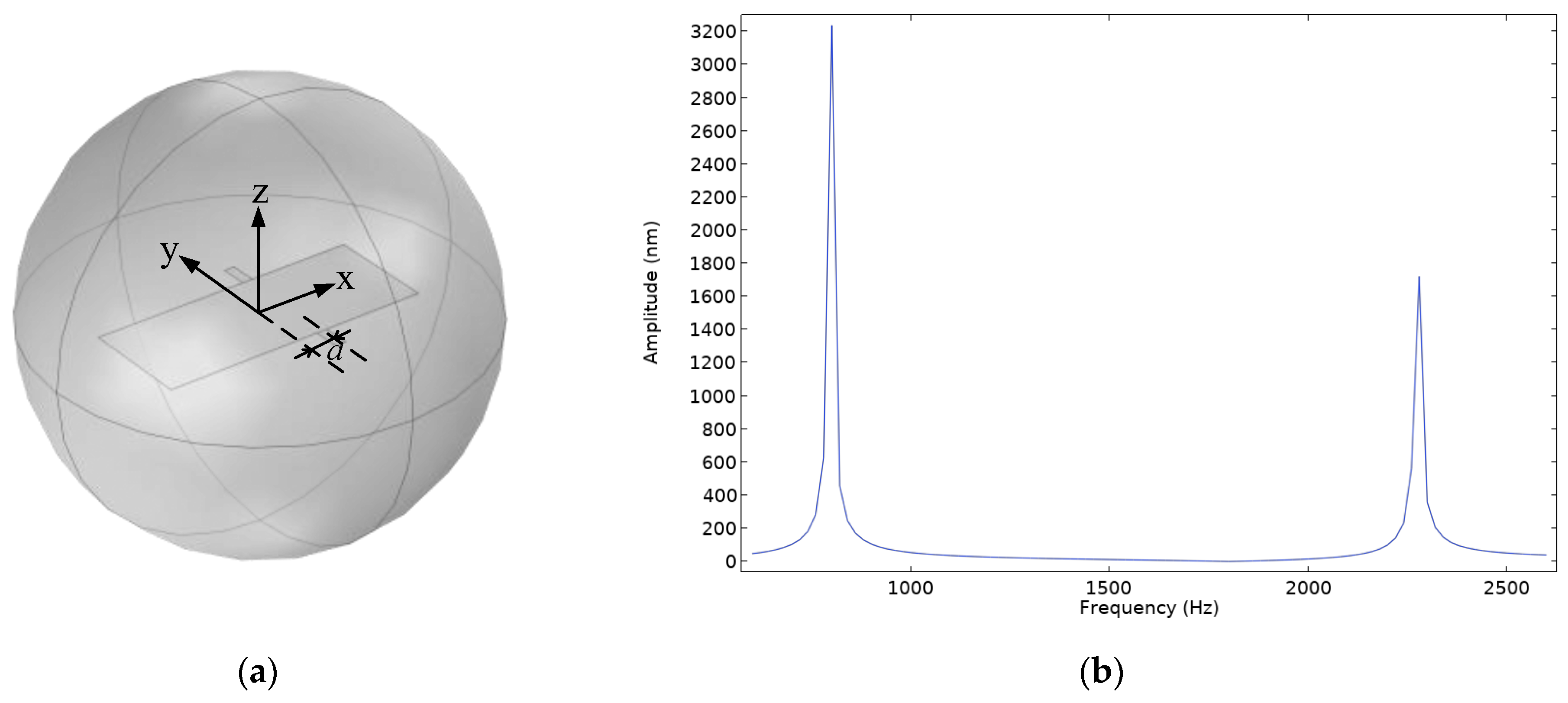
6.1. Frequency Response
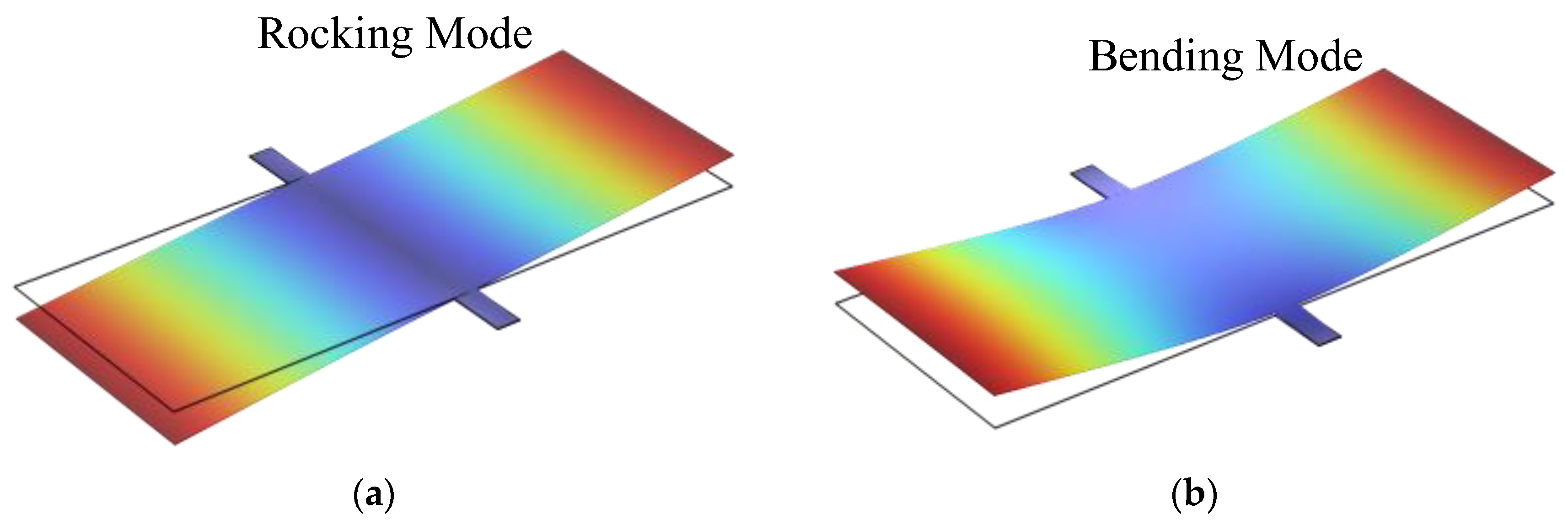
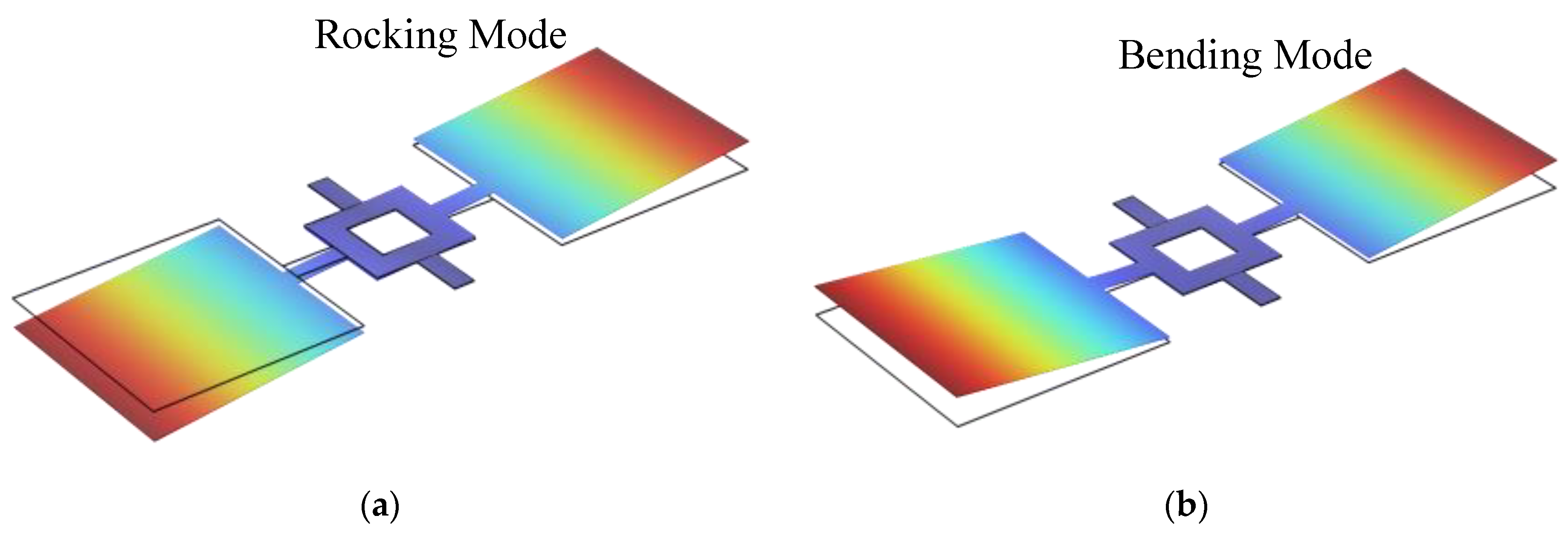
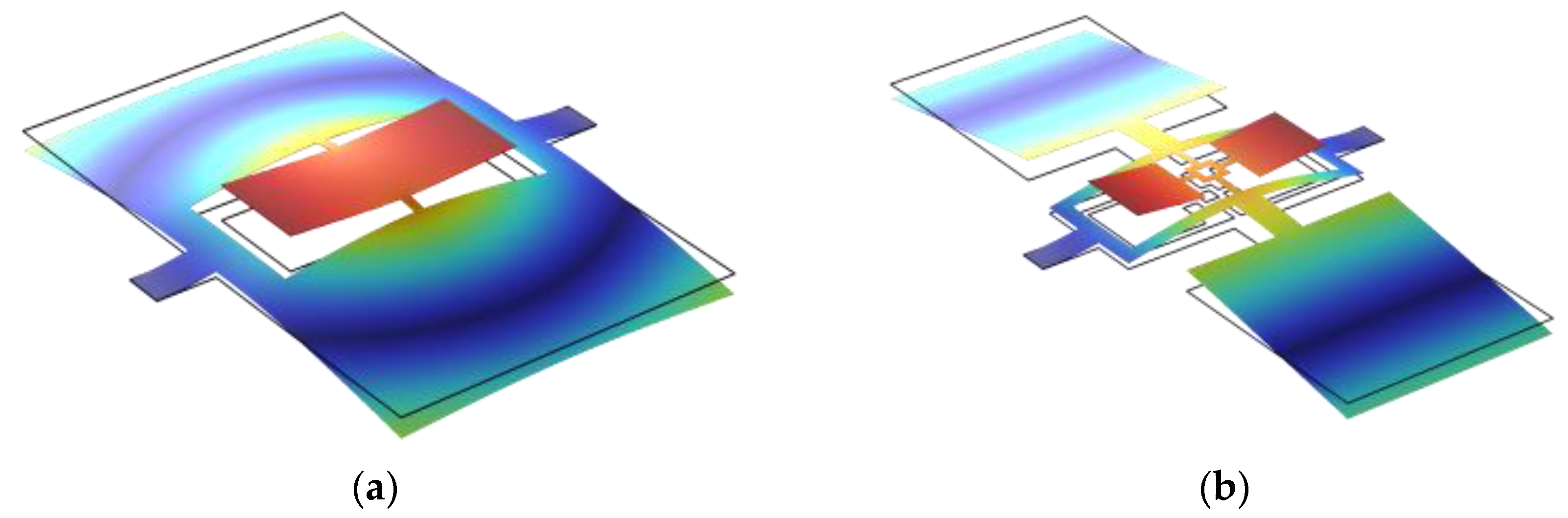
- The first eigenmode corresponds to the rocking mode of the outer diaphragm, with primary vibrations concentrated on the larger wing of the outer diaphragm.
- The second eigenmode corresponds to the bending mode of the outer diaphragm, with primary vibrations concentrated on the smaller wing of the outer diaphragm.
- The third eigenmode corresponds to the rocking mode of the inner diaphragm, with primary vibrations concentrated on the larger wing of the inner diaphragm.
- The fourth eigenmode corresponds to the bending mode of the inner diaphragm, with primary vibrations concentrated on the smaller wing of the inner diaphragm.
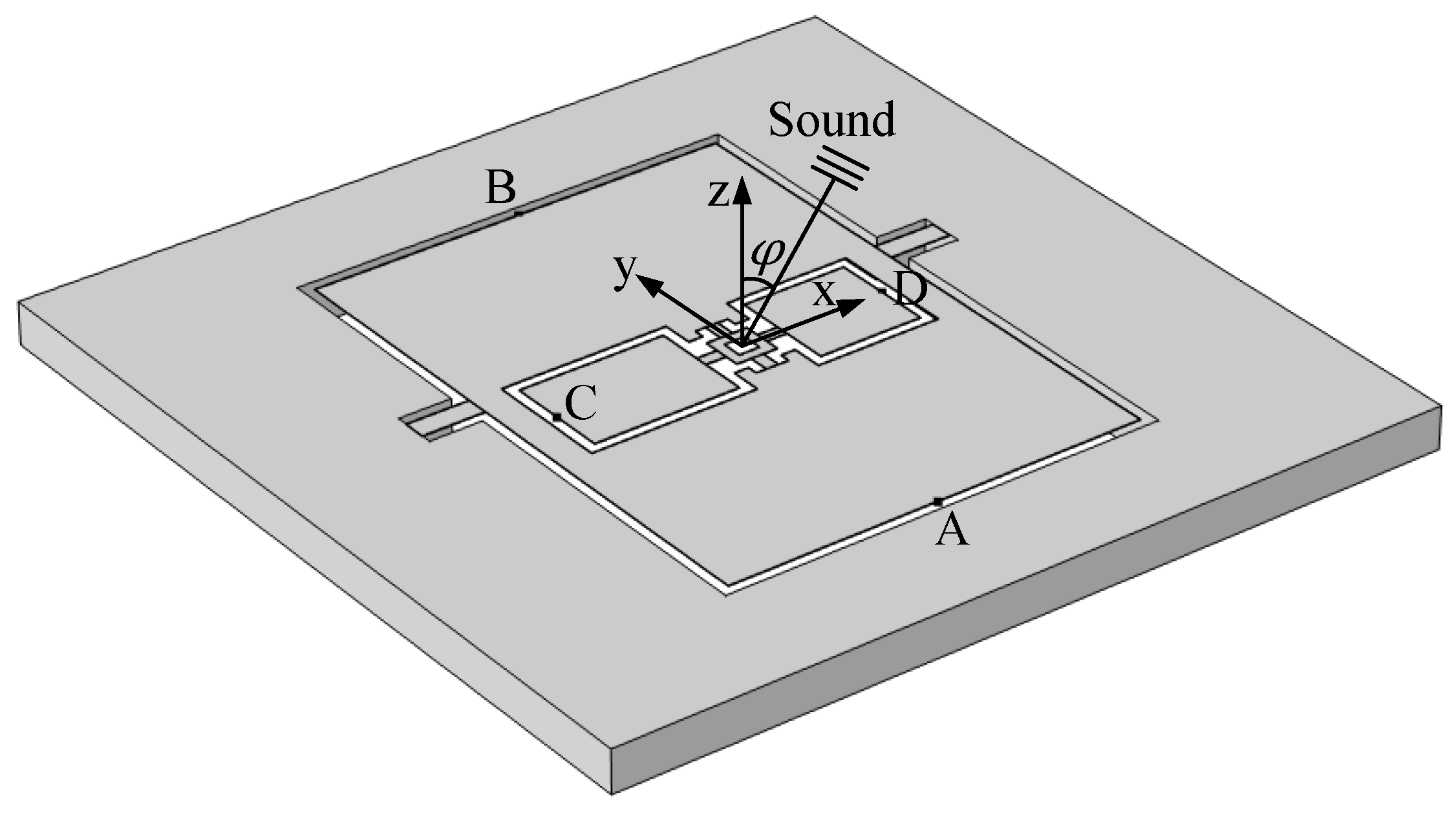
- Point A represents the center of the edge of the larger wing of the outer diaphragm;
- Point B represents the center of the edge of the smaller wing of the outer diaphragm;
- Point C represents the center of the edge of the larger wing of the inner diaphragm;
- Point D represents the center of the edge of the smaller wing of the inner diaphragm.
6.2. Directional Response
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| MEMS | Micro-Electro-Mechanical systems |
| AlN | Aluminum nitride |
| SNR | Signal-to-noise ratio |
| TDOA | Time difference of arrival |
| ILD | Interaural level difference |
| ITD | Interaural time difference |
| PZT | Lead zirconate titanate |
| CMOS | Complementary metal-oxide-semiconductor |
| IDT | Interdigitated electrode |
| 2-DOF | Two-degree-of-freedom |
| PVDF | Polyvinylidene fluoride |
| Al | Aluminum |
| SOI | Silicon-on-insulator |
| BOX | Buried oxide |
| Cr | Chromium |
References
- Rahaman, A.; Kim, B. Fly-inspired mems directional acoustic sensor for sound source direction. In Proceedings of the 2019 20th International Conference on Solid-State Sensors, Actuators and Microsystems & Eurosensors XXXIII (TRANSDUCERS & EUROSENSORS XXXIII), Berlin, Germany, 23–27 June 2019. [Google Scholar]
- Rahaman, A.; Kim, B.; Park, D. Design and characterizations of a multi–sound receiver using fly Ormia ochracea’s ears–inspired MEMS directional microphone array. Appl. Acoust. 2025, 228, 110359. [Google Scholar] [CrossRef]
- Zhang, Y.S.; Windmill, J.F.C.; Uttamchandani, D. Biomimetic MEMS directional microphone structures for multi-band operation. In Proceedings of the SENSORS, 2014 IEEE, Valencia, Spain, 2–5 November 2014. [Google Scholar]
- Miles, R.N.; Robert, D.; Hoy, R.R. Mechanically coupled ears for directional hearing in the parasitoid fly Ormia ochracea. J. Acoust. Soc. Am. 1995, 98, 3059–3070. [Google Scholar] [CrossRef] [PubMed]
- Robert, D.; Miles, R.N.; Hoy, R.R. Directional hearing by mechanical coupling in the parasitoid fly Ormia ochracea. J. Comp. Physiol. A 1996, 179, 29–44. [Google Scholar] [CrossRef] [PubMed]
- Ishfaque, A.; Kim, B. Squeeze film damping analysis of biomimetic micromachined microphone for sound source localization. Sens. Actuators A Phys. 2016, 250, 60–70. [Google Scholar] [CrossRef]
- Liu, H.J.; Yu, M.; Zhang, X.M. Biomimetic optical directional microphone with structurally coupled diaphragms. Appl. Phys. Lett. 2008, 93, 243902. [Google Scholar] [CrossRef]
- Alves, F.; Rabelo, R.; Karunasiri, G. Dual band MEMS directional acoustic sensor for near resonance operation. Sensors 2022, 22, 5635. [Google Scholar] [CrossRef] [PubMed]
- Wilmott, D.; Alves, F.; Karunasiri, G. Bio-inspired miniature direction finding acoustic sensor. Sci. Rep. 2016, 6, 29957. [Google Scholar] [CrossRef] [PubMed]
- Rabelo, R.C.; Alves, F.D.; Karunasiri, G. Electronic phase shift measurement for the determination of acoustic wave DOA using single MEMS biomimetic sensor. Sci. Rep. 2020, 10, 12714. [Google Scholar] [CrossRef] [PubMed]
- Ishfaque, A.; Rahaman, A.; Kim, B. Bioinspired low noise circular-shaped MEMS directional microphone. J. Micro/Nanolithogr. MEMS MOEMS 2019, 18, 010501. [Google Scholar] [CrossRef]
- Rahaman, A.; Ishfaque, A.; Jung, H.; Kim, B. Bio-inspired rectangular shaped piezoelectric MEMS directional microphone. IEEE Sens. J. 2018, 19, 88–96. [Google Scholar] [CrossRef]
- Rahaman, A.; Kim, B. An mm-sized biomimetic directional microphone array for sound source localization in three dimensions. Microsyst. Nanoeng. 2022, 8, 66. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.S.; Bauer, R.; Jackson, J.C.; Whitmer, W.M.; Windmill, J.F.C.; Uttamchandani, D. A low-frequency dual-band operational microphone mimicking the hearing property of Ormia ochracea. J. Microelectromech. Syst. 2018, 27, 667–676. [Google Scholar] [CrossRef]
- Miles, R.N.; Cui, W.L.; Su, Q.T.; Homentcovschi, Q. A MEMS low-noise sound pressure gradient microphone with capacitive sensing. J. Microelectromech. Syst. 2014, 24, 241–248. [Google Scholar] [CrossRef]
- Seo, Y.; Corona, D.; Hall, N.A. On the theoretical maximum achievable signal-to-noise ratio (SNR) of piezoelectric microphones. Sens. Actuators A Phys. 2017, 264, 341–346. [Google Scholar] [CrossRef] [PubMed]
- Rahaman, A.; Jung, H.; Kim, B. Coupled D33 mode-based high performing bio-inspired piezoelectric MEMS directional microphone. Appl. Sci. 2021, 11, 1305. [Google Scholar] [CrossRef]
- Zhang, Y.S.; Bauer, R.; Windmill, J.F.C.; Uttamchandani, D. Multi-band asymmetric piezoelectric MEMS microphone inspired by the Ormia ochracea. In Proceedings of the 2016 IEEE 29th International Conference on Micro Electro Mechanical Systems (MEMS), Shanghai, China, 24–28 January 2016. [Google Scholar]
- Bauer, R.; Zhang, Y.S.; Jackson, J.C.; Whitmer, W.M.; Brimijoin, W.O.; Akeroyd, M.; Uttamchandani, D.; Windmill, J.F.C. Housing influence on multi-band directional MEMS microphones inspired by Ormia ochracea. In Proceedings of the 2016 IEEE SENSORS, Orlando, FL, USA, 30 October–3 November 2016. [Google Scholar]
- Zhang, Y.S. Development of Biomimetic MEMS Microphones Inspired by Ormia Ochracea Working in Audio Range. Ph.D. Thesis, University of Strathclyde, Glasgow, UK, 2019. [Google Scholar]
- Downey, R.H.; Karunasiri, G. Reduced residual stress curvature and branched comb fingers increase sensitivity of MEMS acoustic sensor. J. Microelectromech. Syst. 2013, 23, 417–423. [Google Scholar] [CrossRef]



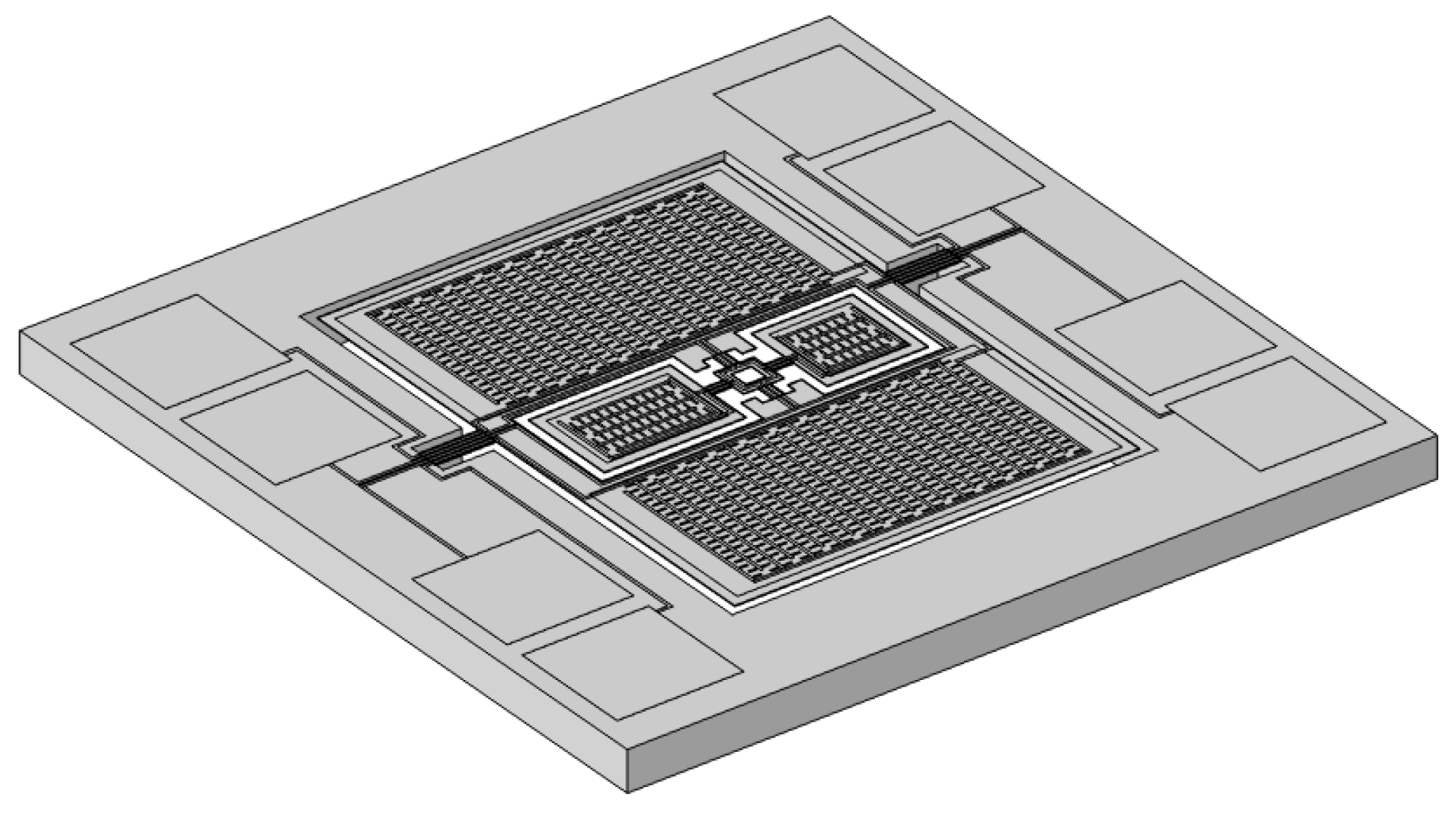




| Structure Type | Rocking Mode Eigenfrequency (Hz) | Bending Mode Eigenfrequency (Hz) | Reduction in Rocking Mode Eigenfrequency Compared to Model A (%) | Reduction in Bending Mode Eigenfrequency Compared to Model A (%) |
|---|---|---|---|---|
| Model A | 840.2 | 1902.7 | 0.0 | 0.0 |
| Model B | 832.1 | 1600.9 | 1.0 | 15.9 |
| Model C | 811.7 | 1350.3 | 3.4 | 29.0 |
| Model D | 490.8 | 631.4 | 41.6 | 66.8 |
| Coupled Structure | Eigenfrequency of Outer Diaphragm Rocking Mode (Hz) | Eigenfrequency of Outer Diaphragm Bending Mode (Hz) | Eigenfrequency of Inner Diaphragm Rocking Mode (Hz) | Eigenfrequency of Inner Diaphragm Bending Mode (Hz) |
|---|---|---|---|---|
| Coupled Structure A | 636.0 | 956.7 | 2894.4 | 8458.2 |
| Coupled Structure B | 186.5 | 232.3 | 1719.7 | 2664.6 |
| Coupled Structure C | 189.6 | 246.9 | 2958.9 | 8763.4 |
| Coupled Structure D | 451.6 | 870.8 | 1728.5 | 2016.0 |
| Layer | Material | Thickness (μm) |
|---|---|---|
| Electrode | Al + Cr | 1 + 0.01 |
| Piezoelectric | AlN | 0.5 |
| Thermal oxide | SiO2 | 0.2 |
| Top silicon | Si | 10 |
| BOX | SiO2 | 1 |
| Bottom silicon | Si | 400 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Y.; Zhao, L.; Ding, X. A Low-Frequency Multi-Band Piezoelectric MEMS Acoustic Sensor Inspired by Ormia ochracea. Micromachines 2025, 16, 451. https://doi.org/10.3390/mi16040451
Liu Y, Zhao L, Ding X. A Low-Frequency Multi-Band Piezoelectric MEMS Acoustic Sensor Inspired by Ormia ochracea. Micromachines. 2025; 16(4):451. https://doi.org/10.3390/mi16040451
Chicago/Turabian StyleLiu, Yi, Liye Zhao, and Xukai Ding. 2025. "A Low-Frequency Multi-Band Piezoelectric MEMS Acoustic Sensor Inspired by Ormia ochracea" Micromachines 16, no. 4: 451. https://doi.org/10.3390/mi16040451
APA StyleLiu, Y., Zhao, L., & Ding, X. (2025). A Low-Frequency Multi-Band Piezoelectric MEMS Acoustic Sensor Inspired by Ormia ochracea. Micromachines, 16(4), 451. https://doi.org/10.3390/mi16040451






