Research and Experimentation on Acoustic Monitoring Technology for Laser Drilling Penetration
Abstract
1. Introduction
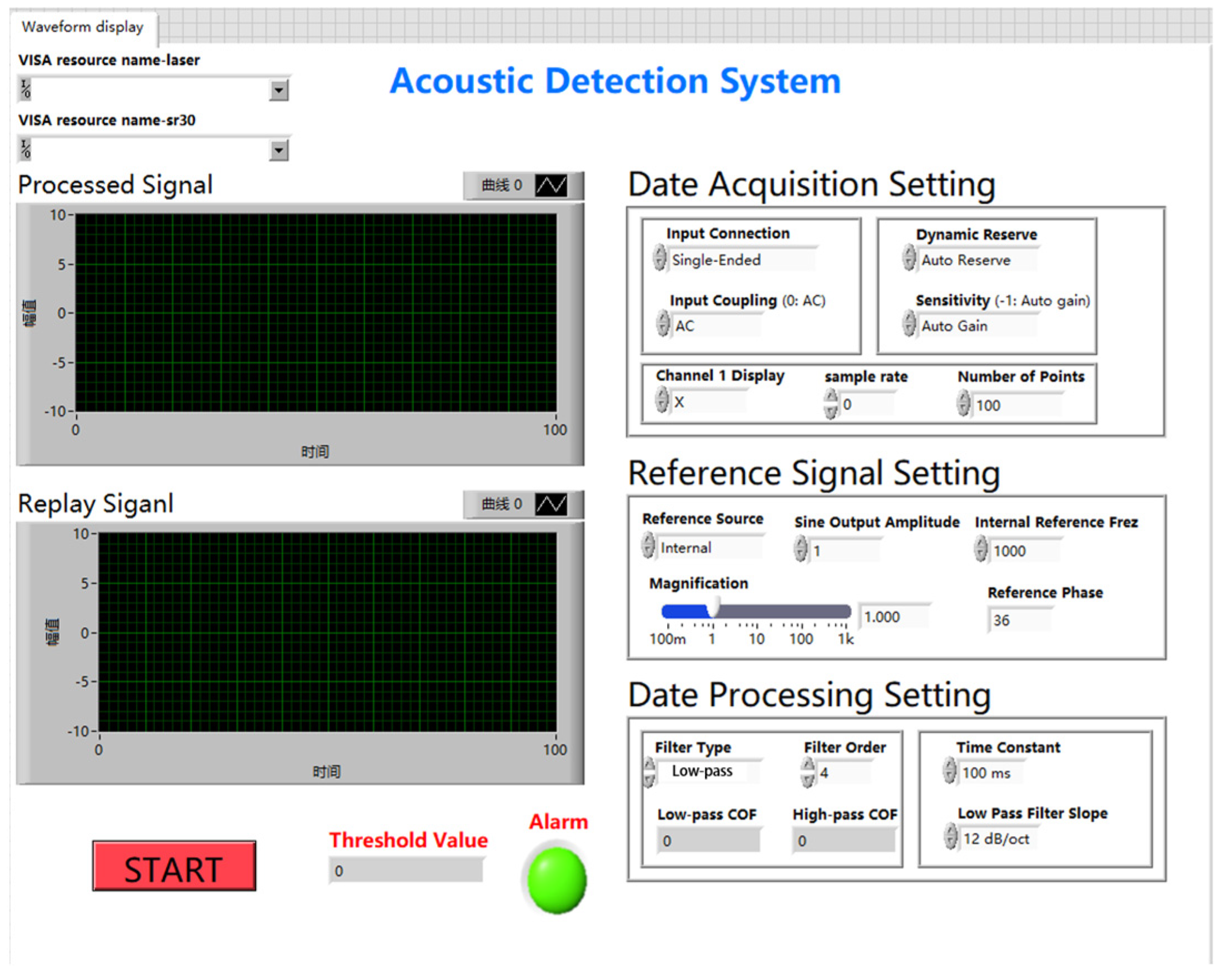
2. Principle and Simulation of Acoustic Monitoring System for Laser Machining
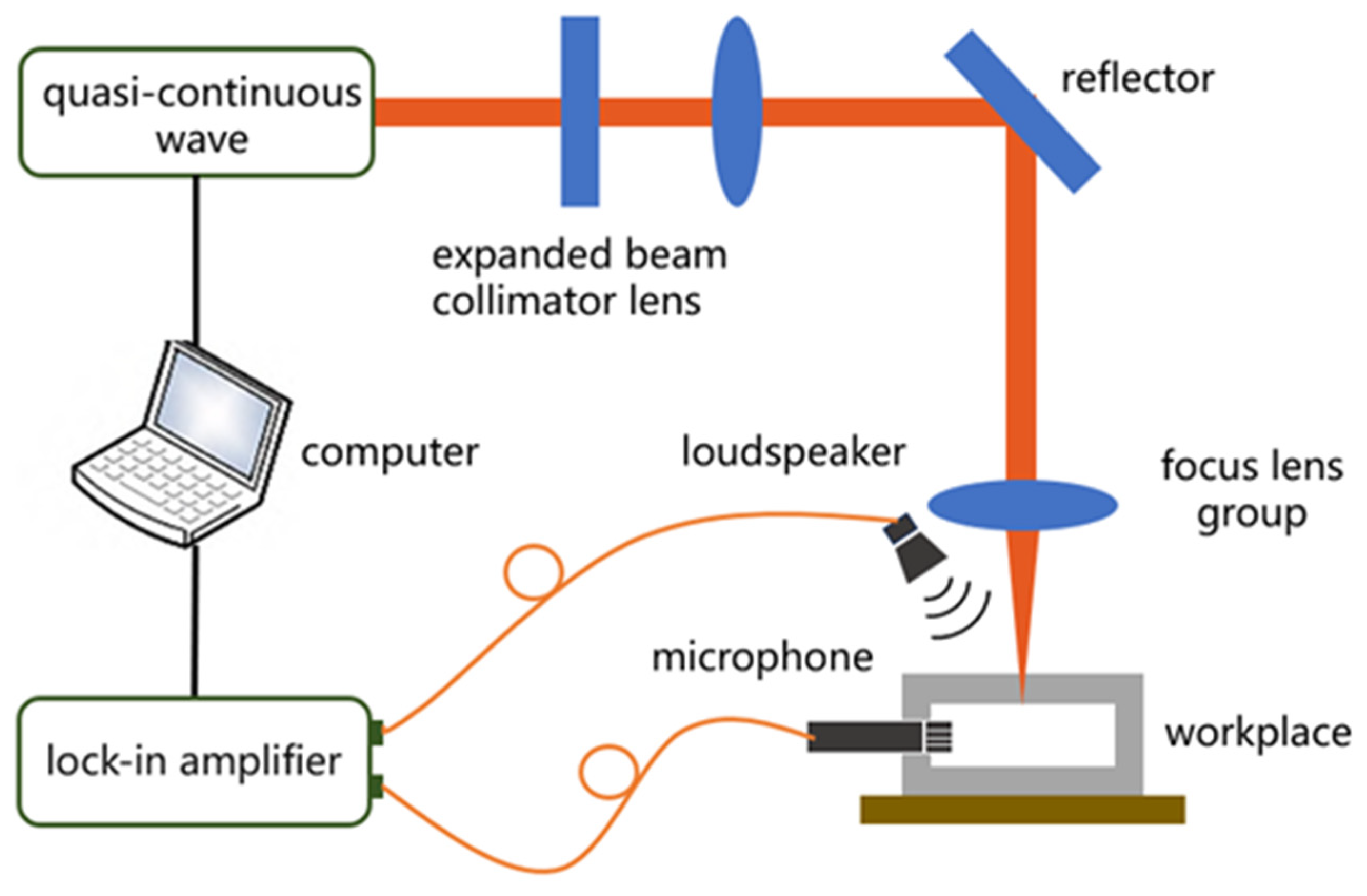
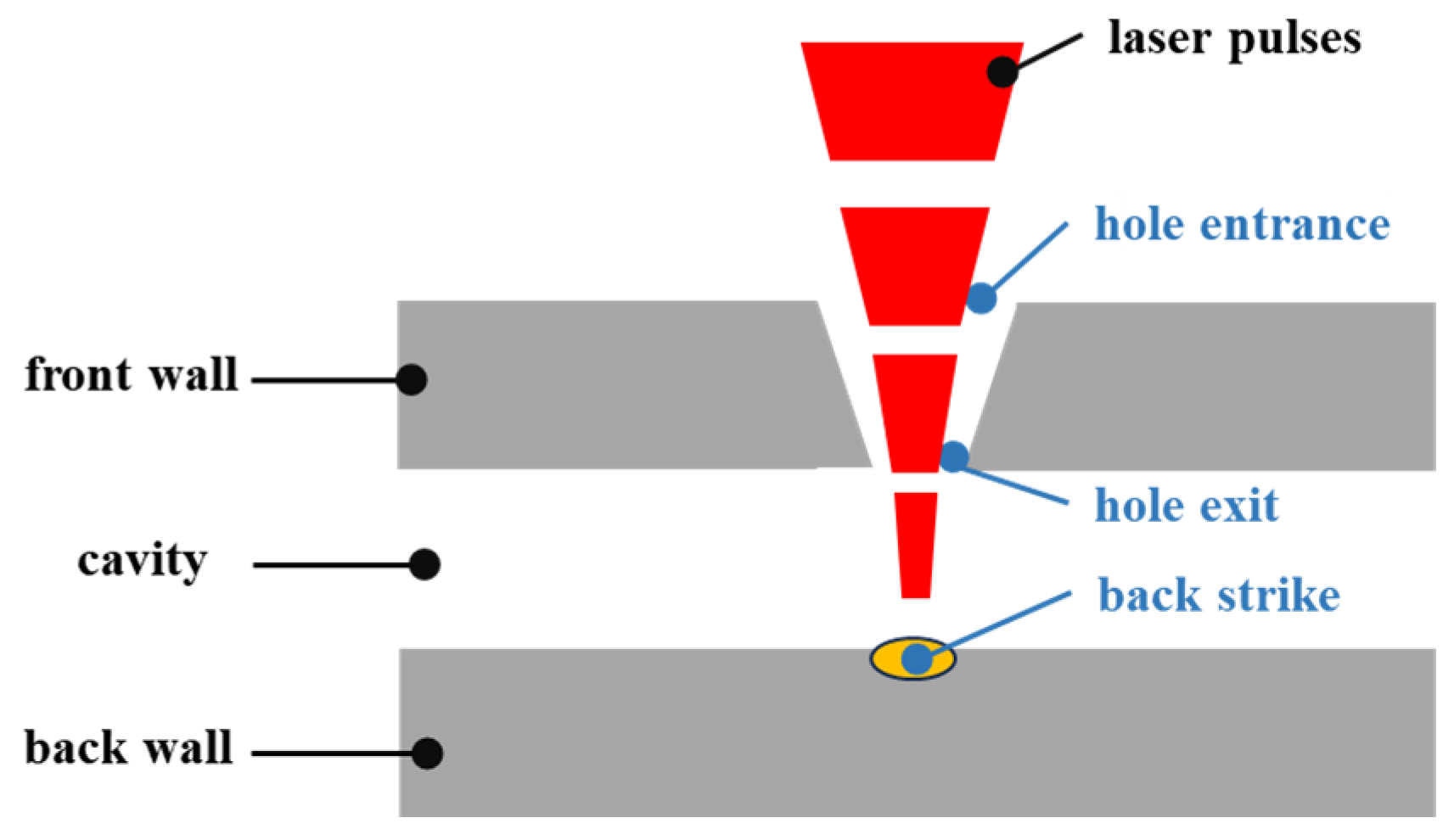
2.1. Principle of the Acoustic Monitoring System for Laser Machining
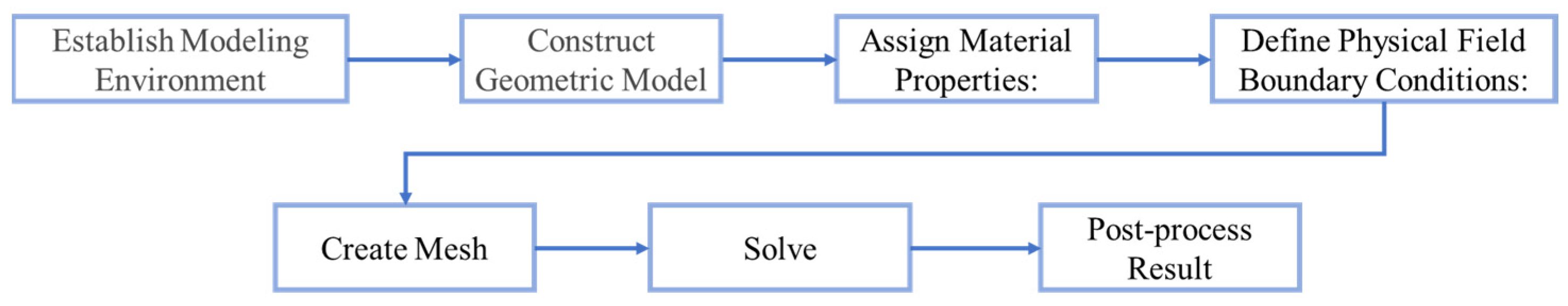
2.2. Acoustic System Simulation
- is the density,
- c is the speed of sound,
- ω is the angular frequency.
- μ is the dynamic viscosity,
- g is the gravitational acceleration.
- p0 represents the applied external pressure,
- i is the imaginary unit.
3. Acoustic Monitoring Experiment for Hole Penetration
3.1. Experimental Material and Parameters
3.1.1. Experimental Materials
3.1.2. Experimental Parameter Settings
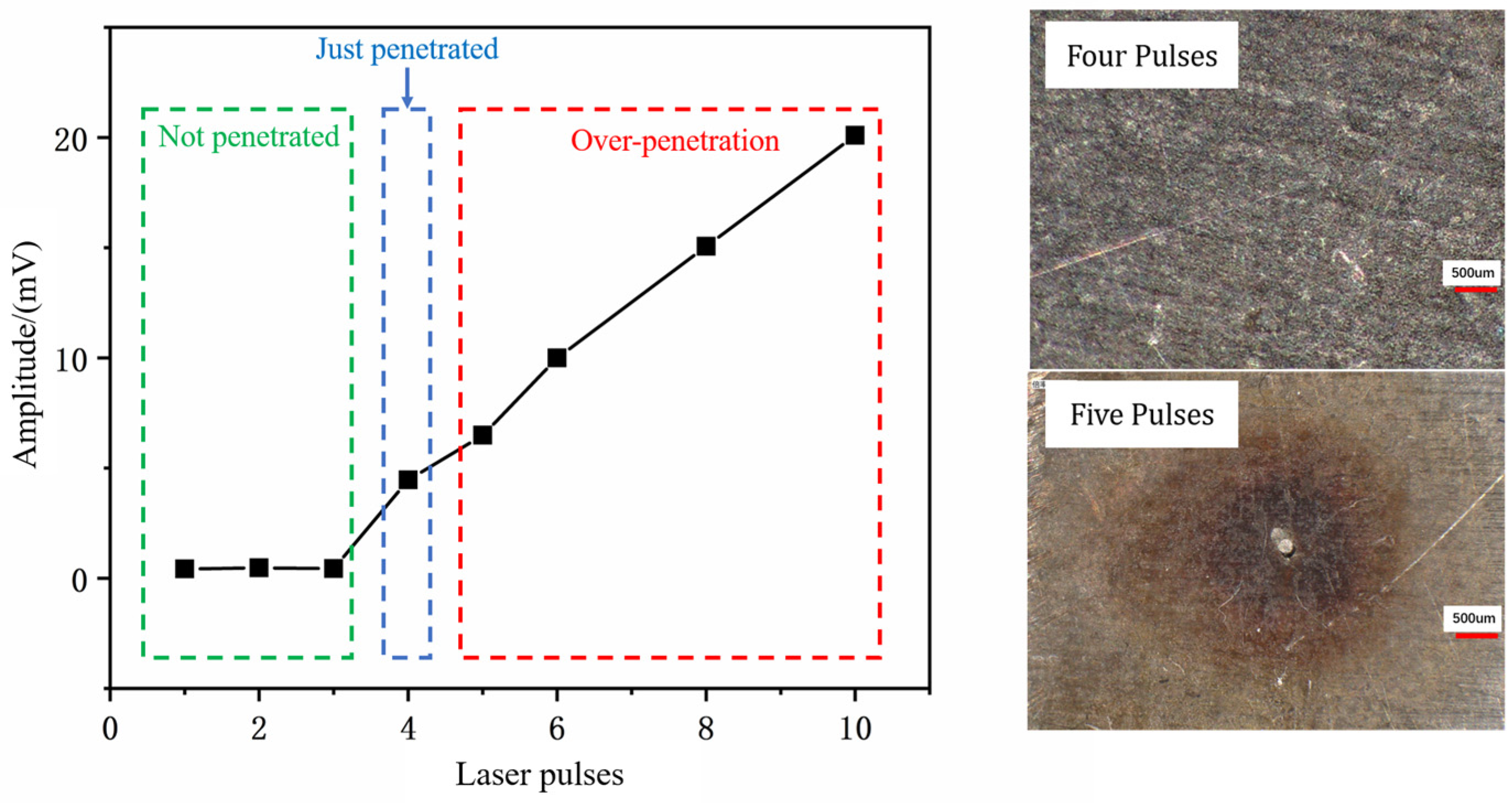
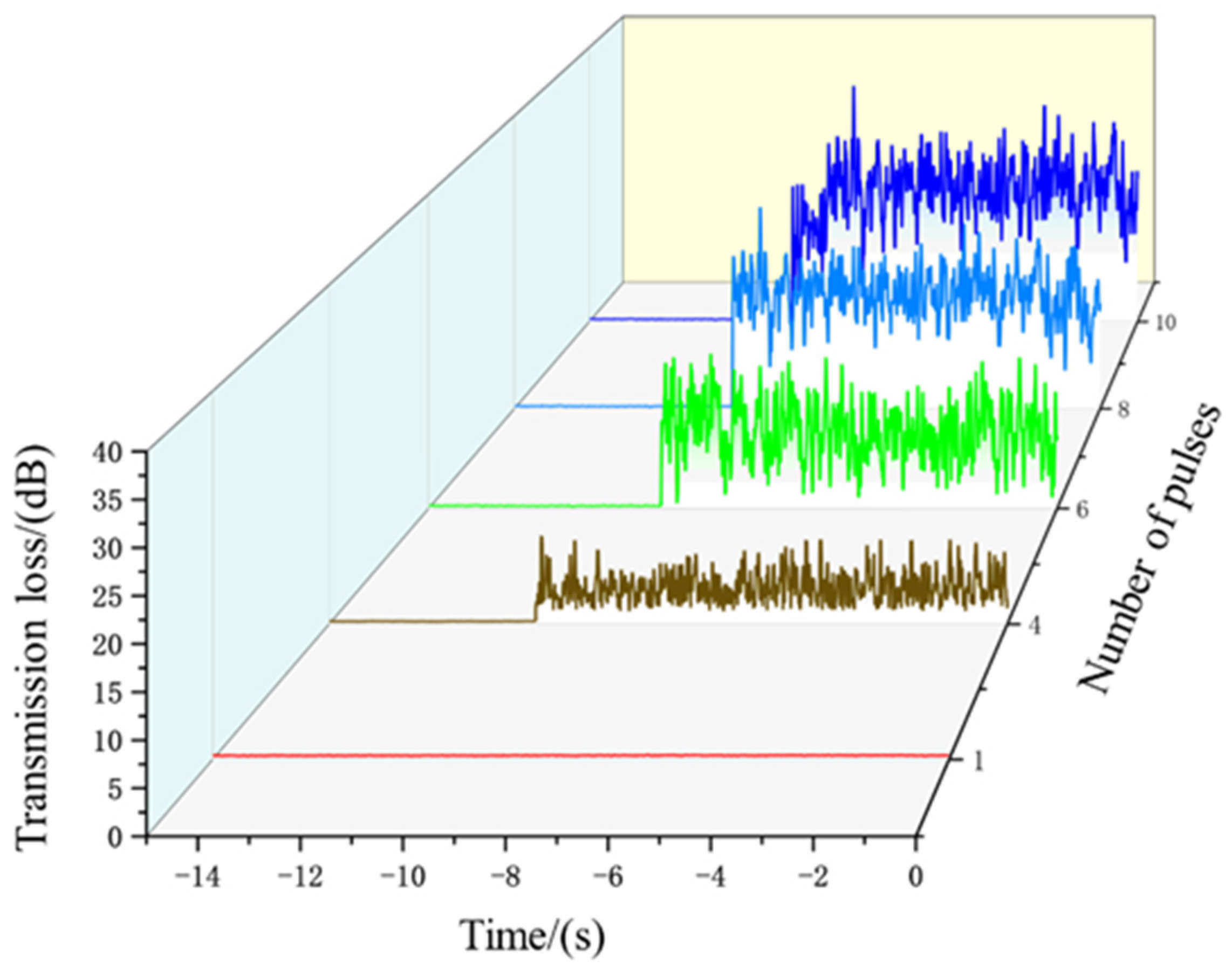
3.2. Determination of Hole Penetration Threshold
- When the number of pulses was three or fewer, the upper workpiece remained unpenetrated.
- At four pulses, the upper workpiece was fully penetrated, and no damage was observed on the backwall of the lower workpiece.
- When the number of pulses exceeded four, the upper workpiece was penetrated, and varying degrees of damage were observed on the backwall of the lower workpiece.
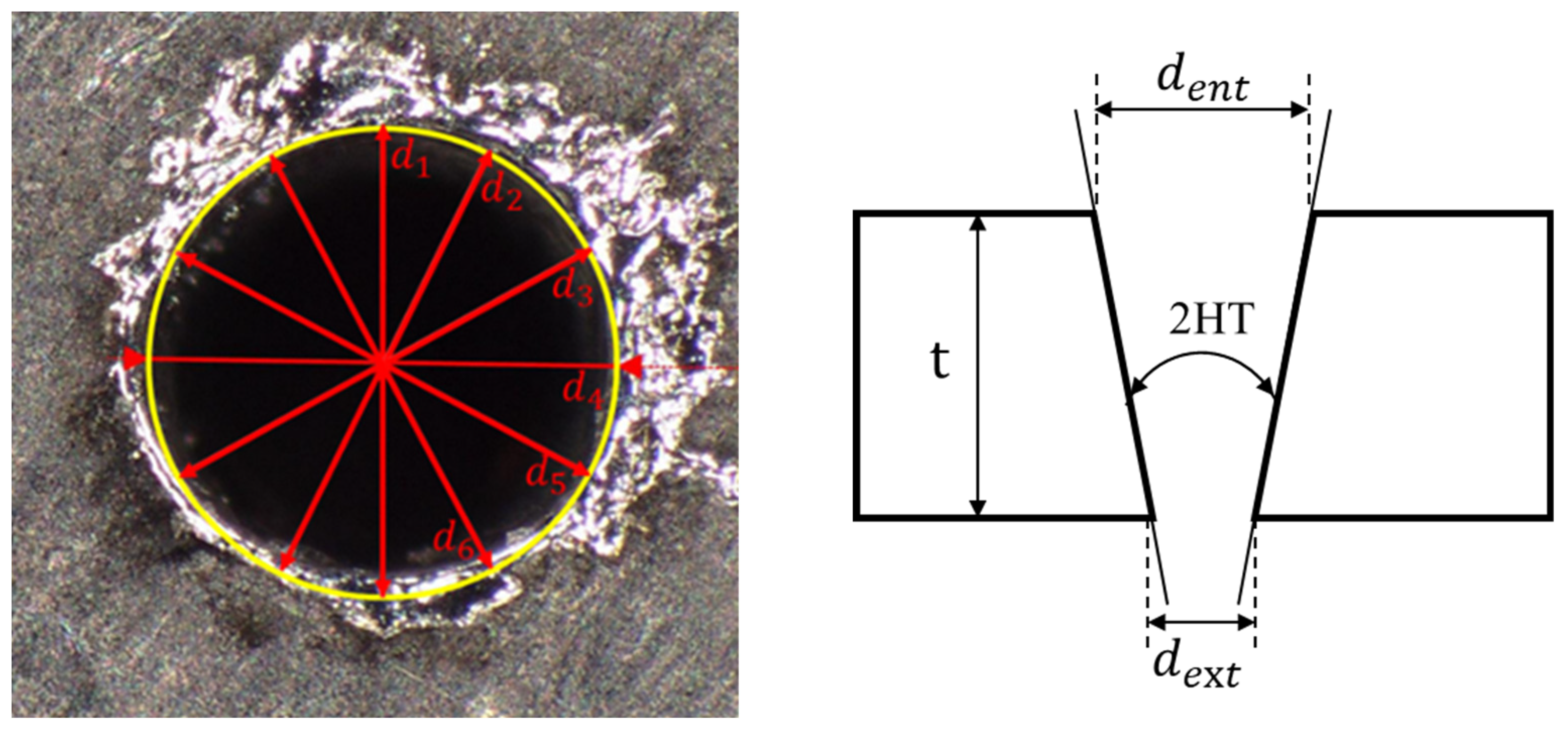
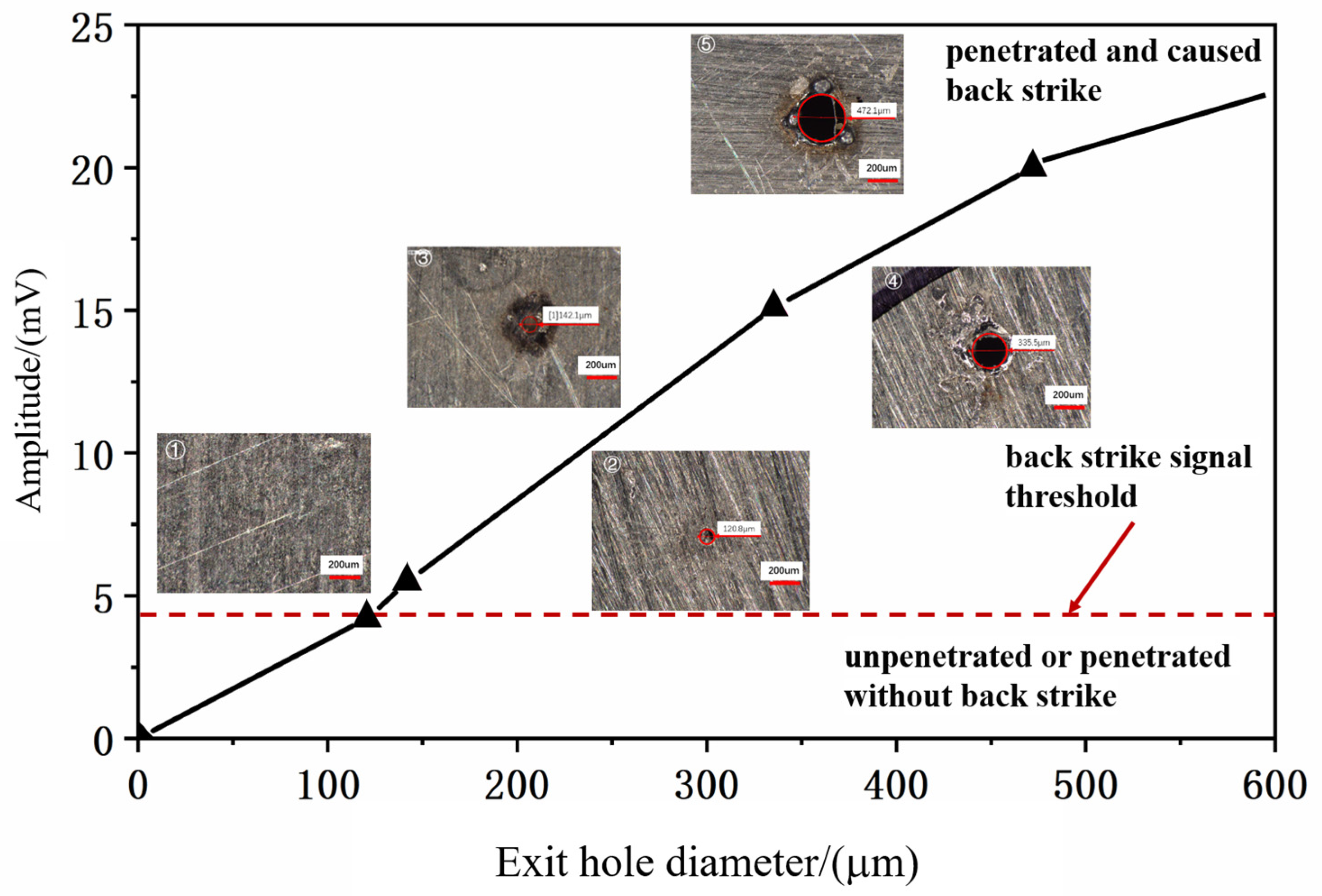
3.3. Investigation of the Relationship Between Signal Intensity and Hole Diameter
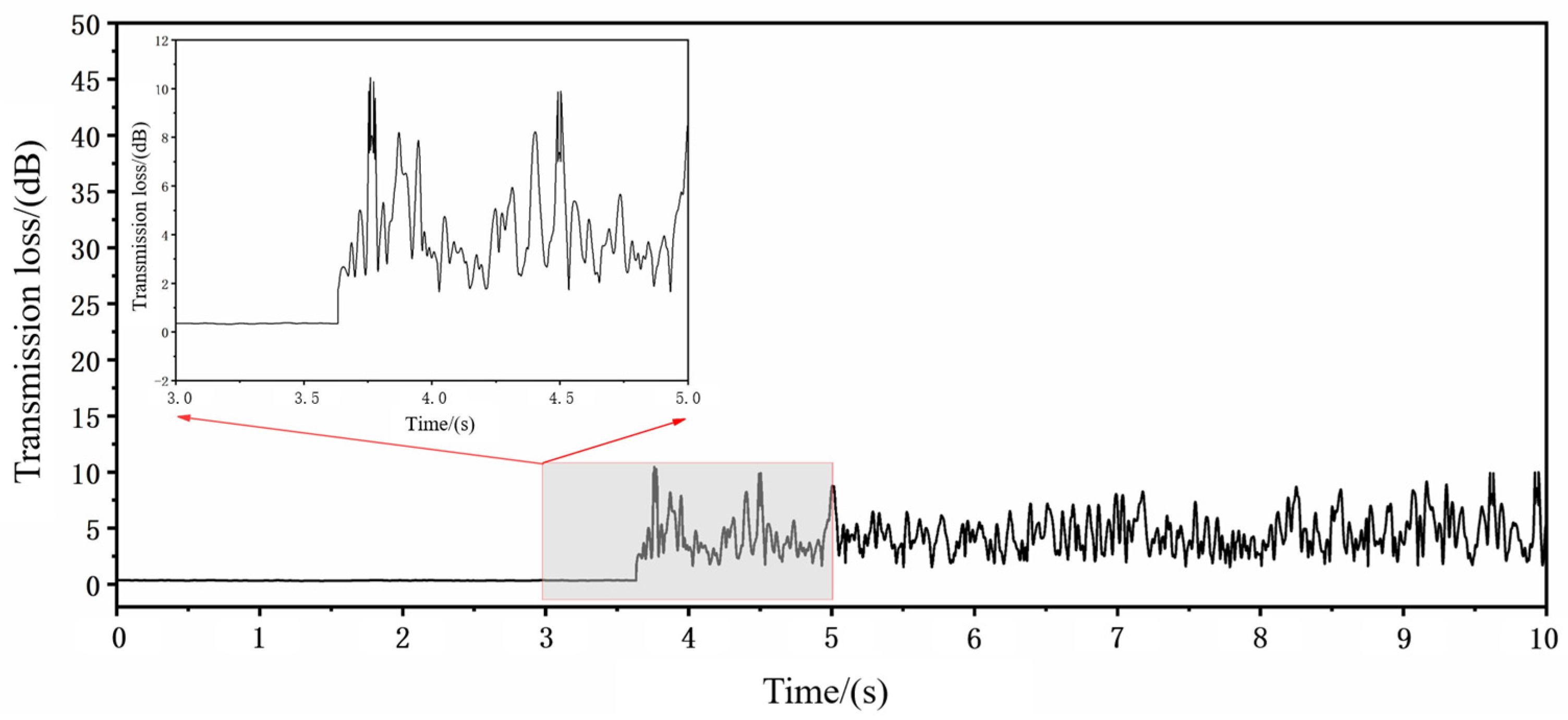
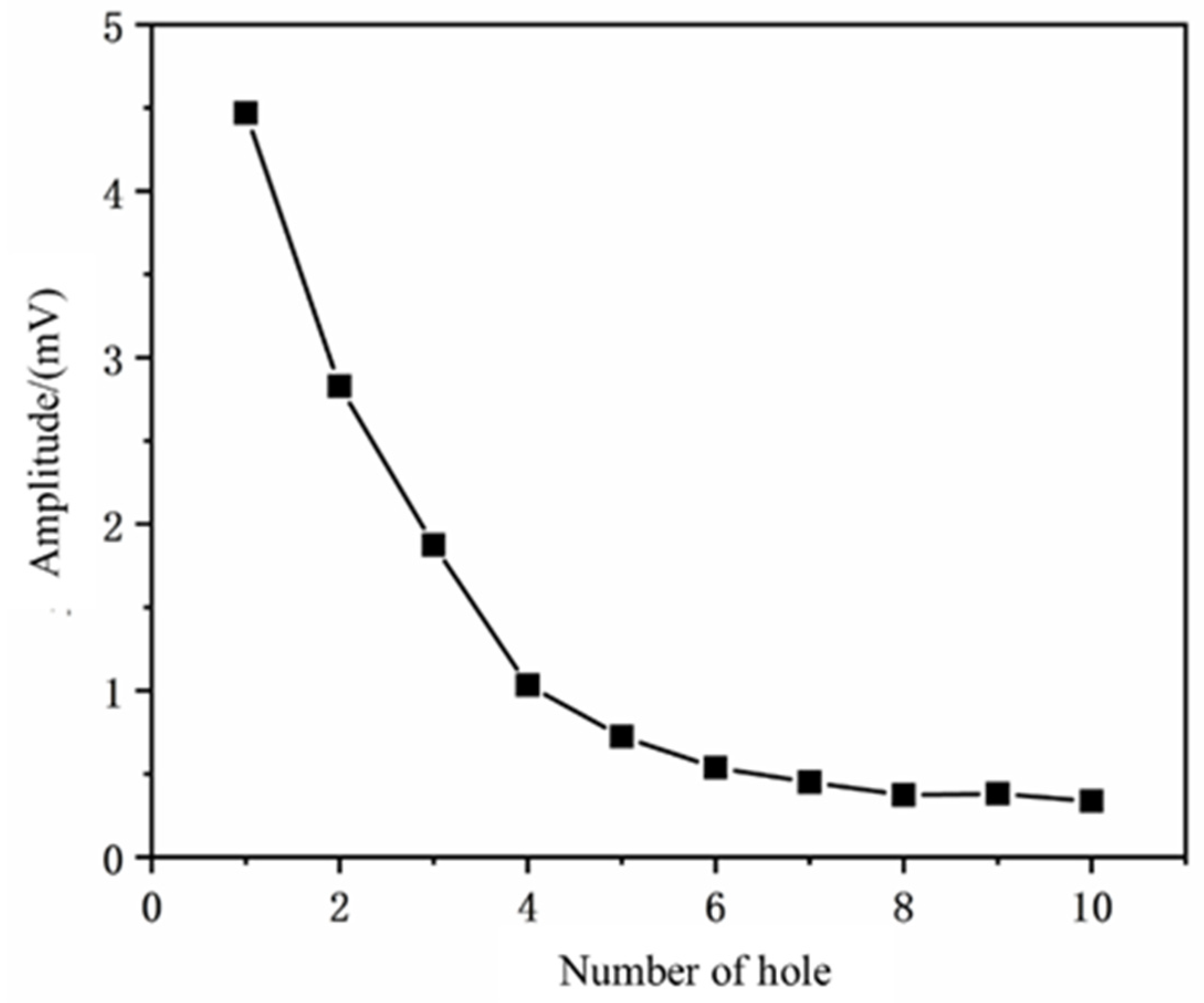
3.4. Investigation of Signal Variation Patterns During Multi-Hole Drilling
4. Discussion
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A


| Peak Power (W) | Inlet Diameter (μm) | Outlet Diameter (μm) | Roundness | Taper (°) | ||
|---|---|---|---|---|---|---|
| Dmax | Dmin | Dmax | Dmin | |||
| 5000 | 415.56 | 405.17 | 208.45 | 194.32 | 0.974998 | 3.168315 |
| 6000 | 419.05 | 410.24 | 257.39 | 234.54 | 0.978976 | 2.642521 |
| 7000 | 424.69 | 412.27 | 282.61 | 230.31 | 0.970755 | 2.783823 |
| 8000 | 426.85 | 418.42 | 365.17 | 304.16 | 0.980251 | 1.757319 |
| 9000 | 426.69 | 406.66 | 385.91 | 343.24 | 0.953057 | 1.195296 |
| 10,000 | 426.52 | 411.06 | 409.65 | 341.29 | 0.963753 | 1.220800 |

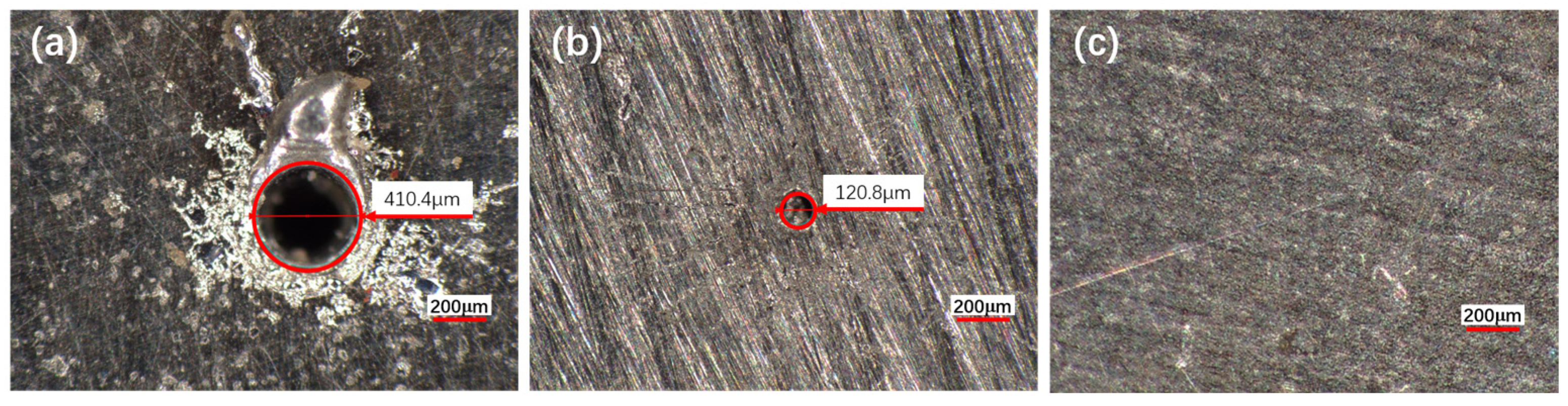

| Number of Pulses | Aperture (μm) | Average Signal Strength (mV) |
|---|---|---|
| 1 | 0 | 0.428 |
| 4 | 120.8 | 4.469 |
| 6 | 207.8 | 9.997 |
| 8 | 335.5 | 15.067 |
| 10 | 472.1 | 20.106 |
| Experiment Number | Number of Machined Holes | Average Signal Strength/(mV) |
|---|---|---|
| 1 | 1 | 4.469 |
| 2 | 2 | 2.828 |
| 3 | 3 | 1.874 |
| 4 | 4 | 1.033 |
| 5 | 5 | 0.725 |
| 6 | 6 | 0.537 |
| 7 | 7 | 0.45 |
| 8 | 8 | 0.374 |
| 9 | 9 | 0.381 |
| 10 | 10 | 0.337 |
References
- Hu, X.; Li, Y.; Bai, S. Research Progress of Laser Application in Material Removal. Laser Optoelectron. Prog. 2021, 58, 0500008-1–0500008-9. [Google Scholar]
- Sebastian, M.; Dirk, B. Analysis of the laser drilling process for pilot holes in complex shaped components. In Proceedings of the 10th CIRP Conference on Photonic Technologies (LANE), Furth, Germany, 3–6 September 2018. [Google Scholar]
- Wang, J.; Yin, Y.; Li, Y. Overview of quasi-CW laser drilling process. Laser Infrared 2022, 52, 1112–1118. [Google Scholar]
- Li, X.L.; Han, D.D.; Zhang, Y.C.; Zhou, H.; Zhang, T.T.; Wang, L.; Zhang, Y.L. Direct Laser Scribing of All-Solid-State In-Plane Proton Microsupercapacitors on Ionic Covalent Organic Framework Films. Adv. Funct. Mater. 2025, 2423854. [Google Scholar] [CrossRef]
- Heise, G.; Konrad, J.; Sarrach, S. Directly induced ablation of metal thin films by ultra short laser pulses. In Proceedings of the Conference on Frontiers in Ultrafast Optics—Biomedical, Scientific, and Industrial Applications XI, San Francisco, CA, USA, 23–26 January 2011. [Google Scholar]
- Tashev, T. Signal measuring instrument lock-in amplifier. In Proceedings of the 7th WSEAS International Conference on Circuits, Systems, Electronics, Control & Signal Processing, Puerto de la Cruz, Spain, 15–17 December 2008. [Google Scholar]
- Naeem, M. Advances in drilling with fiber lasers. In Proceedings of the 4th Industrial Laser Applications Symposium (ILAS), Kenilworth, UK, 17–18 March 2015. [Google Scholar]
- Lu, Y.; Feng, A.; Dai, F. Mechanism study on acoustic signal emission during pulse laser drilling. Laser Infrared 2014, 44, 1331–1334. [Google Scholar]
- Zhang, F.; Wang, J.; Wang, X. Experimental study of nickel-based superalloy IN792 with femtosecond laser drilling method. Opt. Laser Technol. 2021, 143, 107335. [Google Scholar] [CrossRef]
- Wei, T.; Sun, S.; Zhang, F. A review on laser drilling optimization technique: Parameters, methods, and physical-field assistance. Int. J. Adv. Manuf. Technol. 2024, 131, 5691–5710. [Google Scholar] [CrossRef]
- Turner, S.C. Laser Barrier Material for Laser Drilling Hollow Component. US5767482-A, 16 June 1998; EP753373-A1; EP753373-B1; DE69603920-E [P/OL]. <Go to ISI>://DIIDW:1997079223.
- Corfe, A.G.; Stroud, D. Laser barrier material used in laser machining. GB2235893-A, 19 May 1993; US5049722-A, 17 September 1991; GB2235893-B. [P/OL]. <Go to ISI>://DIIDW:1991082611.
- Ma, K.; Pinder, J.T. Laser Drilling Through Hollow Component Wall with Thixotropic Medium in Component to Disperse Penetrating Beam Energy. US4873414-A, 10 October 1989; EP347053-A; JP2037984-A; US5011626-A; EP347053-B1; DE68907527-E [P/OL]. <Go to ISI>://DIIDW:1990029517.
- Wang, B.; Liu, Y.; Wang, Y. Back Strike and Its Protection of Laser Machining of Microholes on Thin-Walled Cavity. Chin. J. Lasers 2021, 48, 1002201. [Google Scholar]
- Tian, D.P.; He, B.; Li, P. Investigation of gas film holes machining with no damage on inner surface of cavity by femtosecond laser. Aviat. Precis. Manuf. Technol. 2018, 54, 6–9. [Google Scholar]
- Sun, T.; Mei, X.; Sun, X. Real-time monitoring and control of the breakthrough stage in ultrafast laser drilling based on sequential three-way decision. IEEE Trans. Ind. Inform. 2022, 19, 5422–5432. [Google Scholar] [CrossRef]
- Sun, T.; Zhao, W.; Fan, Z. In-situ monitoring of hole evolution process in ultrafast laser drilling using optical coherence tomography. J. Manuf. Process. 2025, 133, 1290–1299. [Google Scholar] [CrossRef]
- Stournaras, A.; Chryssolouris, G. On acoustic emissions in percussion laser drilling. Int. J. Adv. Manuf. Technol. 2010, 46, 611–620. [Google Scholar] [CrossRef]
- Yan, Q.; Peng, B.; Wang, L. Research on Laser Cold Machining Hole Penetration Spectroscopy Detection Technology. Acta Photonica Sin. 2023, 52, 0352119. [Google Scholar]
- Stournaras, A.; Salonitis, K.; Chryssolouris, G. Optical emissions for monitoring of the percussion laser drilling process. Int. J. Adv. Manuf. Technol. 2010, 46, 589–603. [Google Scholar] [CrossRef]
- Ruutiainen, M.; Roozbahani, H.; Alizadeh, M.; Handroos, H.; Salminen, A. Real-time monitoring and control of ultra-fast laser engraving process utilizing spectrometer. IEEE Access 2022, 10, 27113–27120. [Google Scholar] [CrossRef]
- Drissi-Daoudi, R.; Masinelli, G.; de Formanoir, C.; Wasmer, K.; Jhabvala, J.; Logé, R.E. Acoustic emission for the prediction of processing regimes in Laser Powder Bed Fusion, and the generation of processing maps. Addit. Manuf. 2023, 67, 103484. [Google Scholar] [CrossRef]
- Liu, L.; Sun, B.; Li, J. Time-frequency analysis and recognition for UAVs based on acoustic signals collected by low-frequency acoustic-electric sensor. IEEE Sens. J. 2024, 24, 19601–19613. [Google Scholar] [CrossRef]
- Zhang, Q.; Jeong, W.; Kang, D.J. Lock-in amplifiers as a platform for weak signal measurements: Development and applications. Curr. Appl. Phys. 2024, 66, 95–109. [Google Scholar] [CrossRef]
- Qu, W. A high accuracy method for long-time evolution of acoustic wave equation. Appl. Math. Lett. 2019, 98, 135–141. [Google Scholar] [CrossRef]
- Andras, A.; Popescu, F.D.; Radu, S.M. Numerical simulation and modeling of mechano–electro–thermal behavior of electrical contact using comsol Multiphysics. Appl. Sci. 2024, 14, 4026. [Google Scholar] [CrossRef]
- Su, Y. Measurement of sound power of steady-state sound sources: Comsol simulation study. J. Phys. Conf. Ser. 2025, 1, 012012. [Google Scholar] [CrossRef]
- Guler, M.T.; Inal, M.; Bilican, I. CO2 laser machining for microfluidics mold fabrication from PMMA with applications on viscoelastic focusing, electrospun nanofiber production, and droplet generation. J. Ind. Eng. Chem. 2021, 98, 340–349. [Google Scholar] [CrossRef]
- Bilican, I.; Guler, M.T. Assessment of PMMA and polystyrene based microfluidic chips fabricated using CO2 laser machining. Appl. Surf. Sci. 2020, 534, 147642. [Google Scholar] [CrossRef]
- Wang, X.; Guo, W.; Shi, L. Characteristics and mechanisms of multiple pulsed laser drilling of blind-holes in Inconel 718 alloy. J. Laser Appl. 2025, 37, 012018. [Google Scholar] [CrossRef]
- Xia, K.; Wang, H.; Ren, N. Laser drilling in nickel super-alloy sheets with and without ultrasonic assistance characterized by transient in-process detection with indirect characterization after hole-drilling. Opt. Laser Technol. 2021, 134, 106559. [Google Scholar] [CrossRef]




| Element | Cu | Mn | Co | Mo | Nb | Cr | Ni | Ti | Al |
|---|---|---|---|---|---|---|---|---|---|
| Mass Fraction/% | ≤0.3 | ≤0.35 | ≤1 | 2.8~3.3 | 4.75~5.5 | 17.0~19.0 | 50.0~55.0 | 0.75~1.15 | 0.2~0.6 |
| Parameters | Numerical Values |
|---|---|
| Maximum value | 10.45488886 mV |
| Median | 4.208818585 mV |
| Minimum value | 1.520186749 mV |
| Average value | 4.468757777 mV |
| Initial average value | 0.428234525 mV |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lian, B.; Pan, K.; Wang, L.; Shi, L.; Yao, J.; Guo, W. Research and Experimentation on Acoustic Monitoring Technology for Laser Drilling Penetration. Micromachines 2025, 16, 475. https://doi.org/10.3390/mi16040475
Lian B, Pan K, Wang L, Shi L, Yao J, Guo W. Research and Experimentation on Acoustic Monitoring Technology for Laser Drilling Penetration. Micromachines. 2025; 16(4):475. https://doi.org/10.3390/mi16040475
Chicago/Turabian StyleLian, Bowen, Kewen Pan, Liqun Wang, Liwu Shi, Jianhua Yao, and Wei Guo. 2025. "Research and Experimentation on Acoustic Monitoring Technology for Laser Drilling Penetration" Micromachines 16, no. 4: 475. https://doi.org/10.3390/mi16040475
APA StyleLian, B., Pan, K., Wang, L., Shi, L., Yao, J., & Guo, W. (2025). Research and Experimentation on Acoustic Monitoring Technology for Laser Drilling Penetration. Micromachines, 16(4), 475. https://doi.org/10.3390/mi16040475






