Tuning Photocatalytic Performance of Multilayer ZnO for Water Splitting by Biaxial Strain Composites
Abstract
1. Introduction
2. Results and Discussions
2.1. Geometric Structures and Electronic Properties of Multilayer ZnO
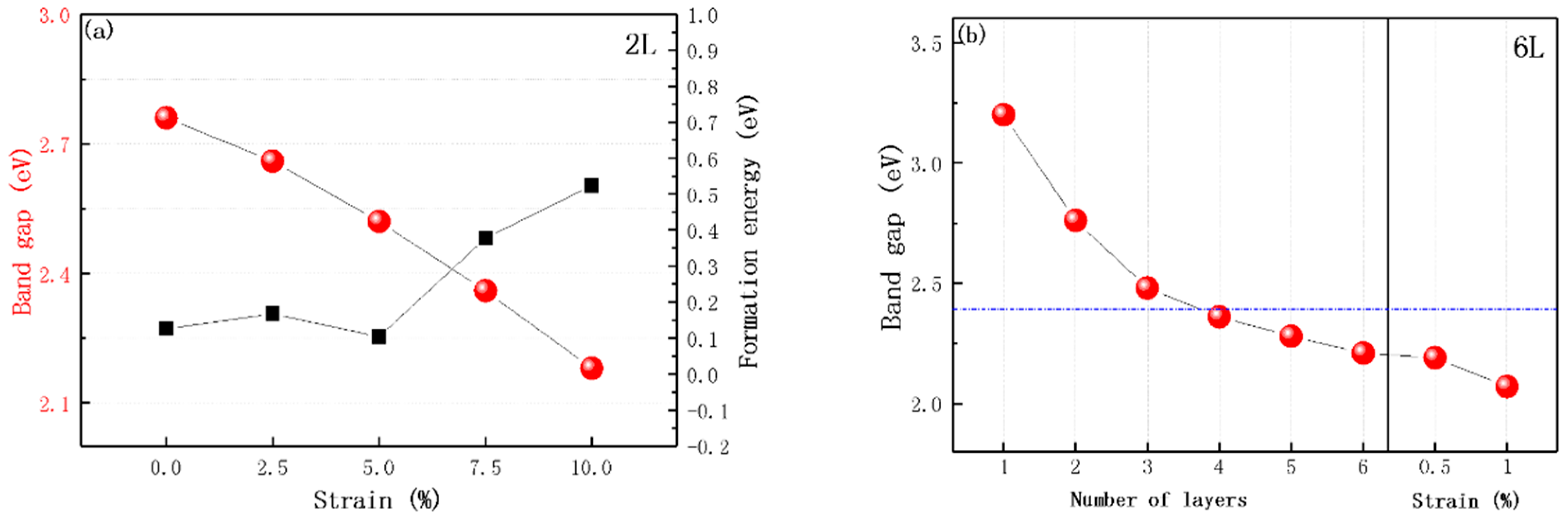
2.2. Effect of the Biaxial Strain
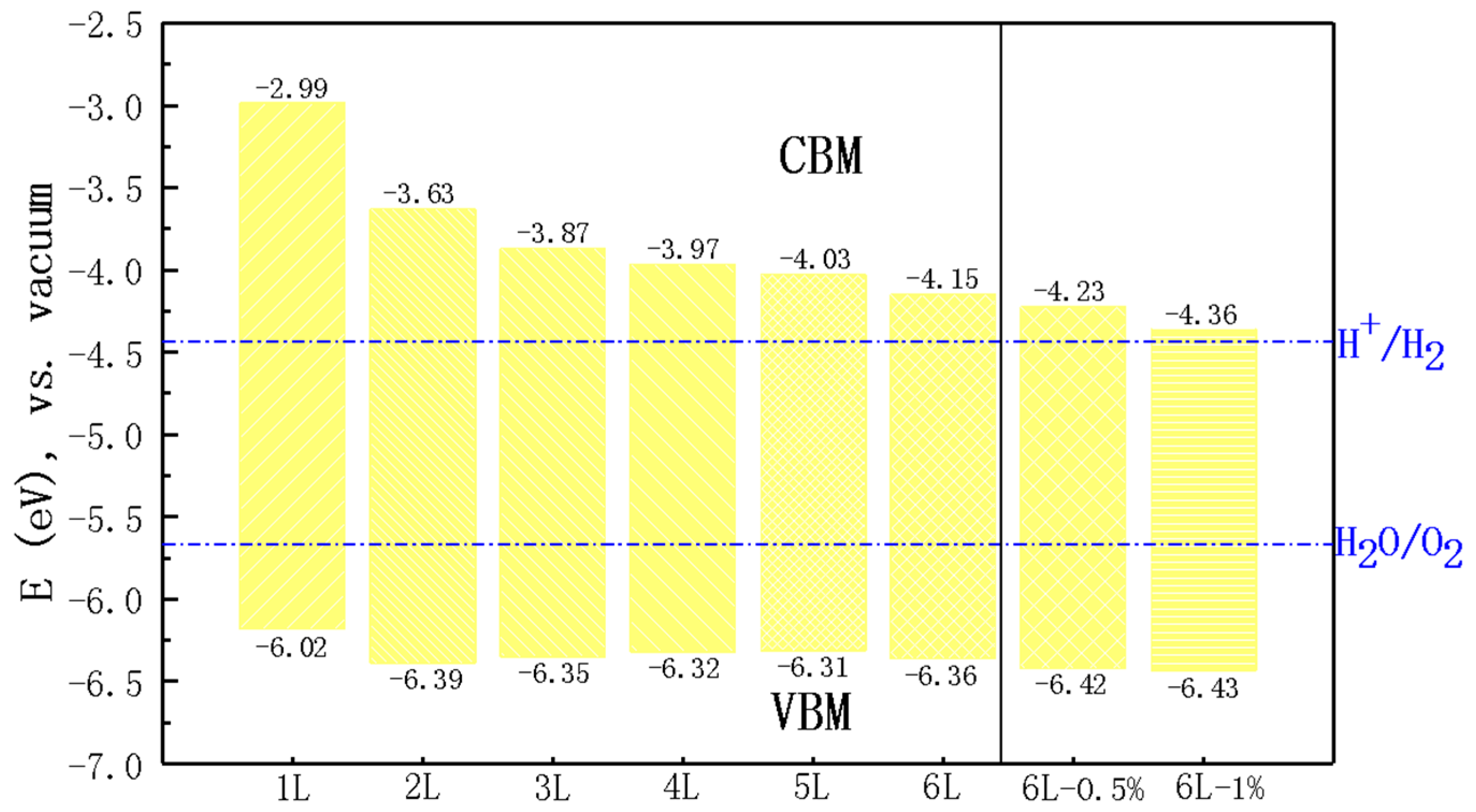
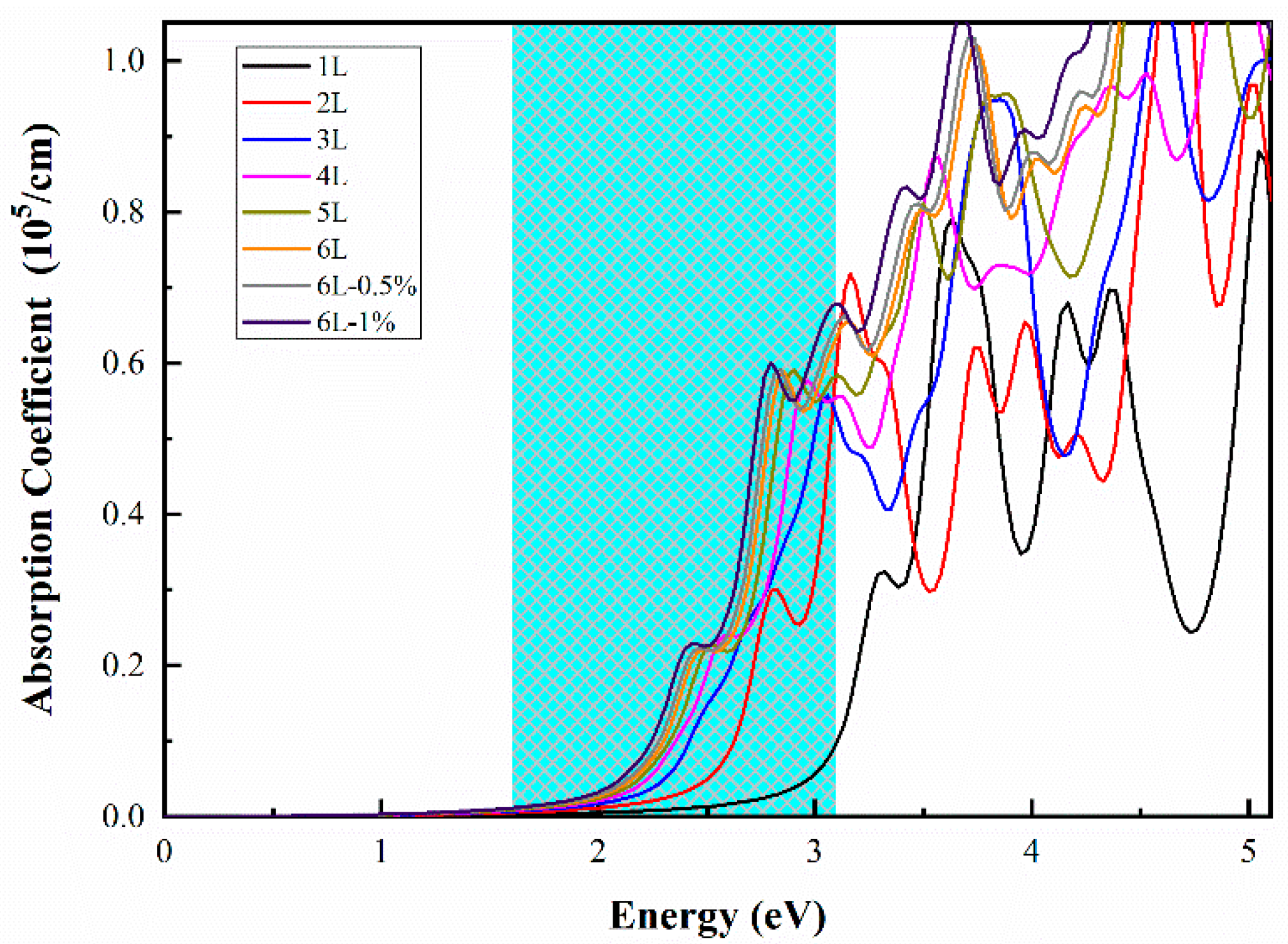
2.3. Photocatalytic Water Splitting
3. Computational Methods
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Christoforidis, K.C.; Fornasiero, P. Photocatalytic Hydrogen Production: A Rift into the Future Energy Supply. ChemCatChem 2017, 9, 1523–1544. [Google Scholar] [CrossRef]
- Hoffmann, M.R.; Martin, S.T.; Choi, W.; Bahnemann, D.W. Environmental Applications of Semiconductor Photocatalysis. Chem. Rev. 1995, 95, 69–96. [Google Scholar] [CrossRef]
- Chen, S.; Wang, L.-W. Thermodynamic Oxidation and Reduction Potentials of Photocatalytic Semiconductors in Aqueous Solution. Chem. Mater. 2012, 24, 3659–3666. [Google Scholar] [CrossRef]
- Hamid, S.B.A.; Teh, S.J.; Lai, C.W. Photocatalytic Water Oxidation on ZnO: A Review. Catalysts 2017, 7, 93. [Google Scholar] [CrossRef]
- Özgür, Ü.; Alivov, Y.I.; Liu, C.; Teke, A.; Reshchikov, M.A.; Doğan, S.; Avrutin, V.; Cho, S.J.; Morkoç, H. A comprehensive review of ZnO materials and devices. J. Appl. Phys. 2005, 98, 041301. [Google Scholar] [CrossRef]
- Hoffman, R.L.; Norris, B.J.; Wager, J.F. ZnO-based transparent thin-film transistors. Appl. Phys. Lett. 2003, 82, 733–735. [Google Scholar] [CrossRef]
- Qiu, Y.; Yan, K.; Deng, H.; Yang, S. Secondary Branching and Nitrogen Doping of ZnO Nanotetrapods: Building a Highly Active Network for Photoelectrochemical Water Splitting. Nano Lett. 2011, 12, 407–413. [Google Scholar] [CrossRef]
- Kalantar-Zadeh, K.; Ou, J.Z.; Daeneke, T.; Mitchell, A.; Sasaki, T.; Fuhrer, M.S. Two dimensional and layered transition metal oxides. Appl. Mater. Today 2016, 5, 73–89. [Google Scholar] [CrossRef]
- Freeman, C.L.; Claeyssens, F.; Allan, N.L.; Harding, J.H. Graphitic Nanofilms as Precursors to Wurtzite Films: Theory. Phys. Rev. Lett. 2006, 96, 066102. [Google Scholar] [CrossRef]
- Tusche, C.; Meyerheim, H.L.; Kirschner, J. Observation of depolarized ZnO(0001) mono-layers: Formation of unreconstructed planar sheets. Phys. Rev. Lett. 2007, 99, 026102. [Google Scholar] [CrossRef]
- Wang, G.; Li, D.; Sun, Q.; Dang, S.; Zhong, M.-M.; Xiao, S.; Liu, G. Hybrid Density Functional Study on the Photocatalytic Properties of Two-dimensional g-ZnO Based Heterostructures. Nanomaterials 2018, 8, 374. [Google Scholar] [CrossRef]
- Peng, R.; Ma, Y.; Huang, B.; Dai, Y. Two-dimensional Janus PtSSe for photocatalytic water splitting under the visible or infrared light. J. Mater. Chem. A 2019, 7, 603–610. [Google Scholar] [CrossRef]
- Drmosh, Q.; Hezam, A.; Hossain, M.; Qamar, M.; Yamani, Z.; Byrappa, K. A novel Cs2O–Bi2O3–TiO2–ZnO heterostructure with direct Z-Scheme for efficient photocatalytic water splitting. Ceram. Int. 2019, 45, 23756–23764. [Google Scholar] [CrossRef]
- Ren, K.; Yu, J.; Tang, W. Two-dimensional ZnO/BSe van der waals heterostructure used as a promising photocatalyst for water splitting: A DFT study. J. Alloys Compd. 2020, 812, 152049. [Google Scholar] [CrossRef]
- Wang, S.; Ren, C.; Tian, H.; Yu, J.; Sun, M. MoS2/ZnO van der Waals heterostructure as a high-efficiency water splitting photocatalyst: A first-principles study. Phys. Chem. Chem. Phys. 2018, 20, 13394–13399. [Google Scholar] [CrossRef]
- Ren, K.; Luo, Y.; Yu, J.; Ren, K. Theoretical prediction of two-dimensional ZnO/GaN van der Waals heterostructure as a photocatalyst for water splitting. Chem. Phys. 2020, 528, 110539. [Google Scholar] [CrossRef]
- Deng, D.; Novoselov, K.S.; Fu, Q.; Zheng, N.; Tian, Z.; Bao, X. Catalysis with two-dimensional materials and their heterostructures. Nat. Nanotechnol. 2016, 11, 218–230. [Google Scholar] [CrossRef] [PubMed]
- Xu, L.; Huang, W.; Hu, W.; Yang, K.; Zhou, B.-X.; Pan, A.; Huang, G. Two-Dimensional MoS2-Graphene-Based Multilayer van der Waals Heterostructures: Enhanced Charge Transfer and Optical Absorption, and Electric-Field Tunable Dirac Point and Band Gap. Chem. Mater. 2017, 29, 5504–5512. [Google Scholar] [CrossRef]
- Yu, W.; Wang, X.; Li, C. Tuning the Electronic Structure of ZnO Bilayer: A First-Principles Study. J. Nanosci. Nanotechnol. 2019, 19, 2839–2843. [Google Scholar] [CrossRef]
- Gao, X.; Shen, Y.; Ma, Y.; Wu, S.; Zhou, Z. ZnO/g-GeC van der Waals heterostructure: Novel photocatalyst for small molecule splitting. J. Mater. Chem. C 2019, 7, 4791–4799. [Google Scholar] [CrossRef]
- Wang, C.; Wang, W.-H.; Lu, F.; Cheng, Y.; Ren, L.; Wang, W.; Liu, H. Pure and Oxidized Ag Substrate Influence on the Phase Transformation and Semiconducting Behaviors of Layered ZnO: A First-Principles Study. J. Phys. Chem. C 2015, 119, 4891–4897. [Google Scholar] [CrossRef]
- Tom, K.B.; Lin, S.; Wan, L.F.; Wang, J.; Ahlm, N.; N’Diaye, A.T.; Bustillo, K.C.; Huang, J.; Liu, Y.; Lou, S.; et al. Solution-Based, Template-Assisted Realization of Large-Scale Graphitic ZnO. ACS Nano 2018, 12, 7554–7561. [Google Scholar] [CrossRef]
- Niemelä, J.-P.; Aghaee, M.; Kessels, W.E.; Creatore, M.; Verheijen, M.A. Transition in layer structure of atomic/molecular layer deposited ZnO-zincone multilayers. J. Vac. Sci. Technol. A 2019, 37, 040602. [Google Scholar] [CrossRef]
- Quang, H.T.; Bachmatiuk, A.; Dianat, A.; Ortmann, F.; Zhao, J.; Warner, J.H.; Eckert, J.; Cuniberti, G.; Rümmeli, M.H. In Situ Observations of Free-Standing Graphene-like Mono- and Bilayer ZnO Membranes. ACS Nano 2015, 9, 11408–11413. [Google Scholar] [CrossRef] [PubMed]
- Khan, M.; Bhatti, K.; Qindeel, R.; Alonizan, N.; Althobaiti, H.S. Characterizations of multilayer ZnO thin films deposited by sol-gel spin coating technique. Results Phys. 2017, 7, 651–655. [Google Scholar] [CrossRef]
- Wu, D.; Lagally, M.G.; Liu, F. Stabilizing Graphitic Thin Films of Wurtzite Materials by Epitaxial Strain. Phys. Rev. Lett. 2011, 107, 236101. [Google Scholar] [CrossRef] [PubMed]
- Lee, J.; Sorescu, D.C.; Deng, X. Tunable Lattice Constant and Band Gap of Single- and Few-Layer ZnO. J. Phys. Chem. Lett. 2016, 7, 1335–1340. [Google Scholar] [CrossRef]
- Guan, Z.; Ni, S.; Hu, S. Tunable Electronic and Optical Properties of Monolayer and Multilayer Janus MoSSe as a Photocatalyst for Solar Water Splitting: A First-Principles Study. J. Phys. Chem. C 2018, 122, 6209–6216. [Google Scholar] [CrossRef]
- Zhao, Y.; Liu, N.; Zhou, S.; Zhao, J. Two-dimensional ZnO for the selective photoreduction of CO2. J. Mater. Chem. A 2019, 7, 16294–16303. [Google Scholar] [CrossRef]
- Behera, H.; Mukhopadhyay, G. Strain-tunable band parameters of ZnO mono-layer in graphene-like honeycomb structure. Phys. Lett. A 2012, 376, 3287–3289. [Google Scholar] [CrossRef]
- Zhuang, H.L.; Hennig, R.G. Single-Layer Group-III Monochalcogenide Photocatalysts for Water Splitting. Chem. Mater. 2013, 25, 3232–3238. [Google Scholar] [CrossRef]
- Zhang, X.; Li, B.; Wang, J.; Yuan, Y.; Zhang, Q.; Gao, Z.; Liu, L.-M.; Chen, L. The stabilities and electronic structures of single-layer bismuth oxyhalides for photocatalytic water splitting. Phys. Chem. Chem. Phys. 2014, 16, 25854–25861. [Google Scholar] [CrossRef] [PubMed]
- Kaewmaraya, T.; De Sarkar, A.; Sa, B.; Sun, Z.; Ahuja, R. Strain-induced tunability of optical and photocatalytic properties of ZnO mono-layer nanosheet. Comput. Mater. Sci. 2014, 91, 38–42. [Google Scholar] [CrossRef]
- Chen, H.F.; Tan, C.L.; Zhang, K.; Zhao, W.B.; Tian, X.H.; Huang, Y.W. Enhanced photocatalytic performance of ZnO mono-layer for water splitting via biaxial strain and external electric field. Appl. Surf. Sci. 2019, 481, 1064–1071. [Google Scholar] [CrossRef]
- Tu, Z. First-Principles Study on Physical Properties of a Single ZnO Monolayer with Graphene-Like Structure. J. Comput. Theor. Nanosci. 2010, 7, 1182–1186. [Google Scholar] [CrossRef]
- Trasatti, S. The absolute electrode potential: An explanatory note (Recommendations 1986). Pure Appl. Chem. 1986, 58, 955–966. [Google Scholar] [CrossRef]
- Kanan, D.K.; Carter, E.A. Band Gap Engineering of MnO via ZnO Alloying: A Potential New Visible-Light Photocatalyst. J. Phys. Chem. C 2012, 116, 9876–9887. [Google Scholar] [CrossRef]
- Kresse, G.; Hafner, J. Abinitio molecular dynamics for liquid metals. Phys. Rev. B 1993, 47, 13115–13118. [Google Scholar] [CrossRef]
- Ernzerhof, M.; Scuseria, G.E. Assessment of the Perdew–Burke–Ernzerhof exchange-correlation functional. J. Chem. Phys. 1999, 110, 5029–5036. [Google Scholar] [CrossRef]
- White, J.A.; Bird, D. Implementation of gradient-corrected exchange-correlation potentials in Car-Parrinello total-energy calculations. Phys. Rev. B 1994, 50, 4954–4957. [Google Scholar] [CrossRef]
- Grimme, S. Semiempirical GGA-type density functional constructed with a long-range dispersion correction. J. Comput. Chem. 2006, 27, 1787–1799. [Google Scholar] [CrossRef] [PubMed]
- Jurecka, P.; Cerny, J.; Hobza, P.; Salahub, D.R. Density functional theory augmented with an empirical dispersion term. Interaction energies and geometries of 80 noncovalent complexes compared with ab initio quantum mechanics calculations. J. Comp. Chem. 2007, 28, 555–569. [Google Scholar] [CrossRef] [PubMed]
- Hu, W.; Yang, J. First-principles study of two-dimensional van der Waals heterojunctions. Comput. Mater. Sci. 2016, 112, 518–526. [Google Scholar] [CrossRef]
- Heyd, J.; Scuseria, G.E.; Ernzerhof, M. Hybrid functionals based on a screened Coulomb potential. J. Chem. Phys. 2003, 118, 8207–8215. [Google Scholar] [CrossRef]
- Chowdhury, C.; Karmakar, S.; Datta, A. Monolayer Group IV–VI Monochalcogenides: Low-Dimensional Materials for Photocatalytic Water Splitting. J. Phys. Chem. C 2017, 121, 7615–7624. [Google Scholar] [CrossRef]

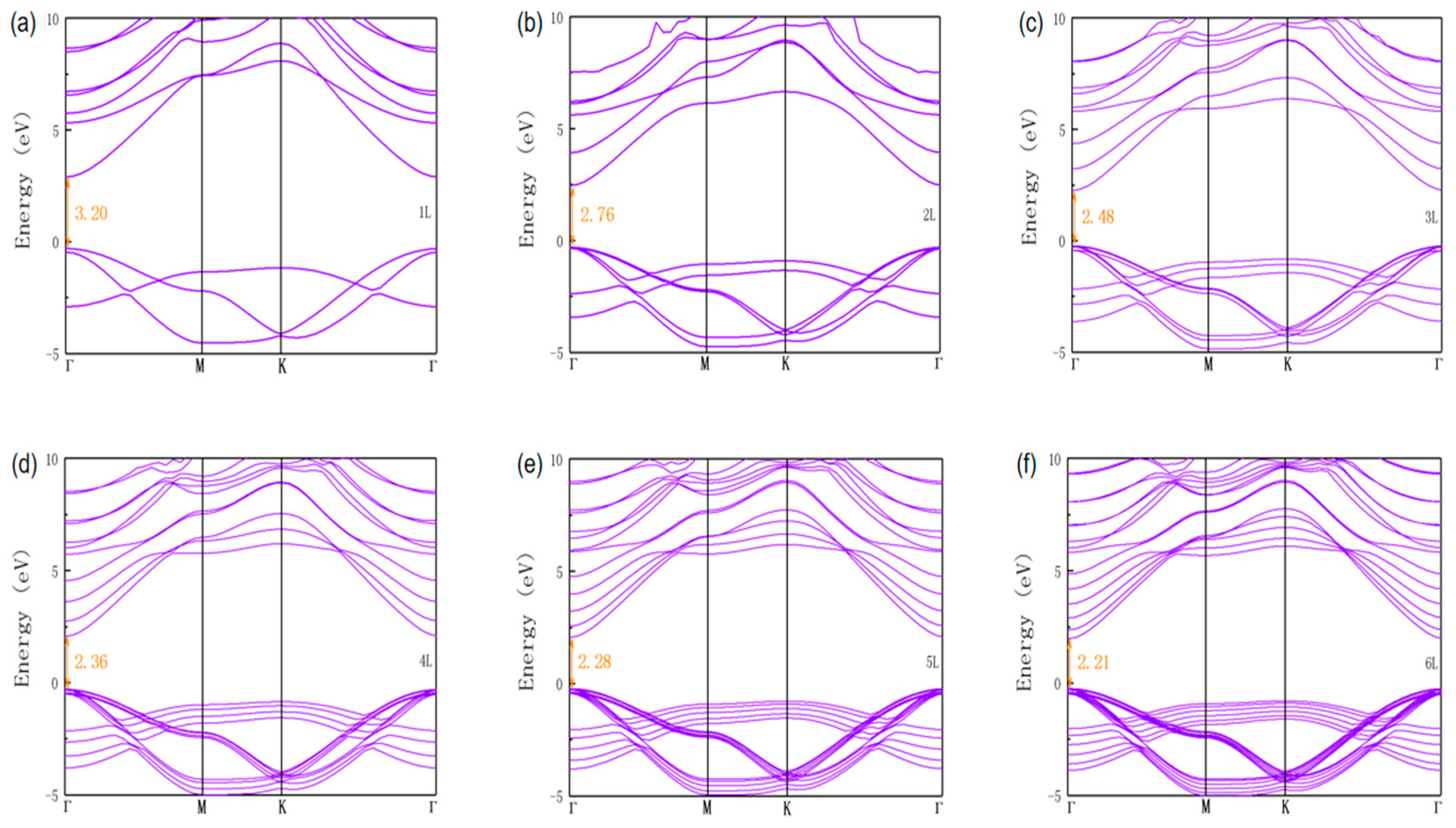
| ZnO | Eg (eV) | Et (eV) | Ef (eV) | Eb (eV) |
|---|---|---|---|---|
| 1L | 3.20 | −10.898 | 0.235 | - |
| 2L | 2.76 | −22.238 | 0.123 | −0.443 |
| 3L | 2.48 | −33.420 | 0.115 | −0.726 |
| 4L | 2.36 | −44.605 | 0.110 | −1.014 |
| 5L | 2.28 | −55.793 | 0.105 | −1.304 |
| 6L | 2.21 | −66.975 | 0.103 | −1.588 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cai, X.; Huang, Y.; Hu, J.; Zhu, S.; Tian, X.; Zhang, K.; Ji, G.; Zhang, Y.; Fu, Z.; Tan, C. Tuning Photocatalytic Performance of Multilayer ZnO for Water Splitting by Biaxial Strain Composites. Catalysts 2020, 10, 1208. https://doi.org/10.3390/catal10101208
Cai X, Huang Y, Hu J, Zhu S, Tian X, Zhang K, Ji G, Zhang Y, Fu Z, Tan C. Tuning Photocatalytic Performance of Multilayer ZnO for Water Splitting by Biaxial Strain Composites. Catalysts. 2020; 10(10):1208. https://doi.org/10.3390/catal10101208
Chicago/Turabian StyleCai, Xiaofan, Yuewu Huang, Jinzhi Hu, Shiwei Zhu, Xiaohua Tian, Kun Zhang, Guangju Ji, Yunxiao Zhang, Zhendong Fu, and Changlong Tan. 2020. "Tuning Photocatalytic Performance of Multilayer ZnO for Water Splitting by Biaxial Strain Composites" Catalysts 10, no. 10: 1208. https://doi.org/10.3390/catal10101208
APA StyleCai, X., Huang, Y., Hu, J., Zhu, S., Tian, X., Zhang, K., Ji, G., Zhang, Y., Fu, Z., & Tan, C. (2020). Tuning Photocatalytic Performance of Multilayer ZnO for Water Splitting by Biaxial Strain Composites. Catalysts, 10(10), 1208. https://doi.org/10.3390/catal10101208








