Enhancing Nickel-Iron Gas Diffusion Electrodes for Oxygen Evolution in Alkaline Water Electrolysis
Abstract
:1. Introduction
2. Results and Discussion
2.1. Physical Characterization
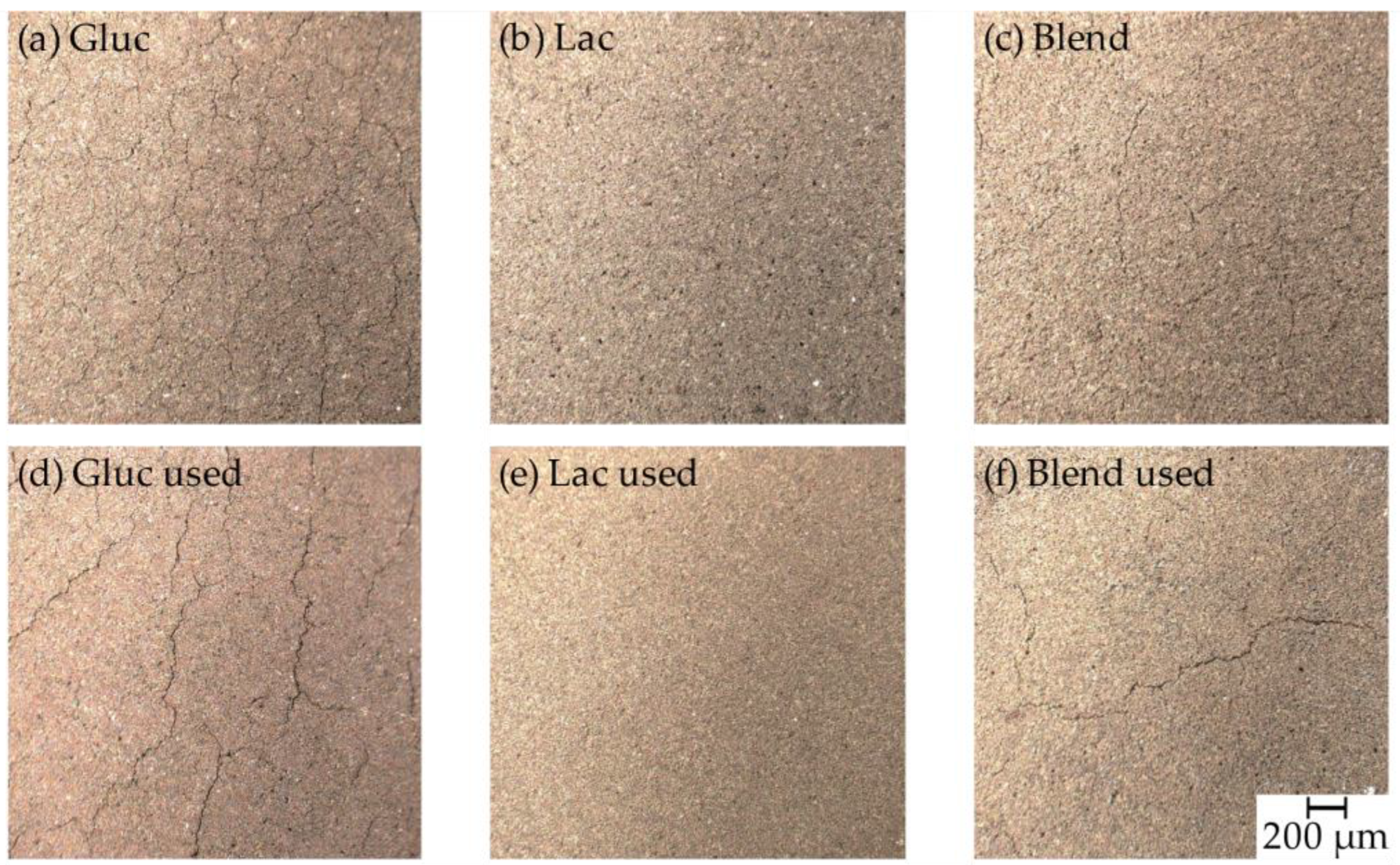
2.1.1. Optical Microscope
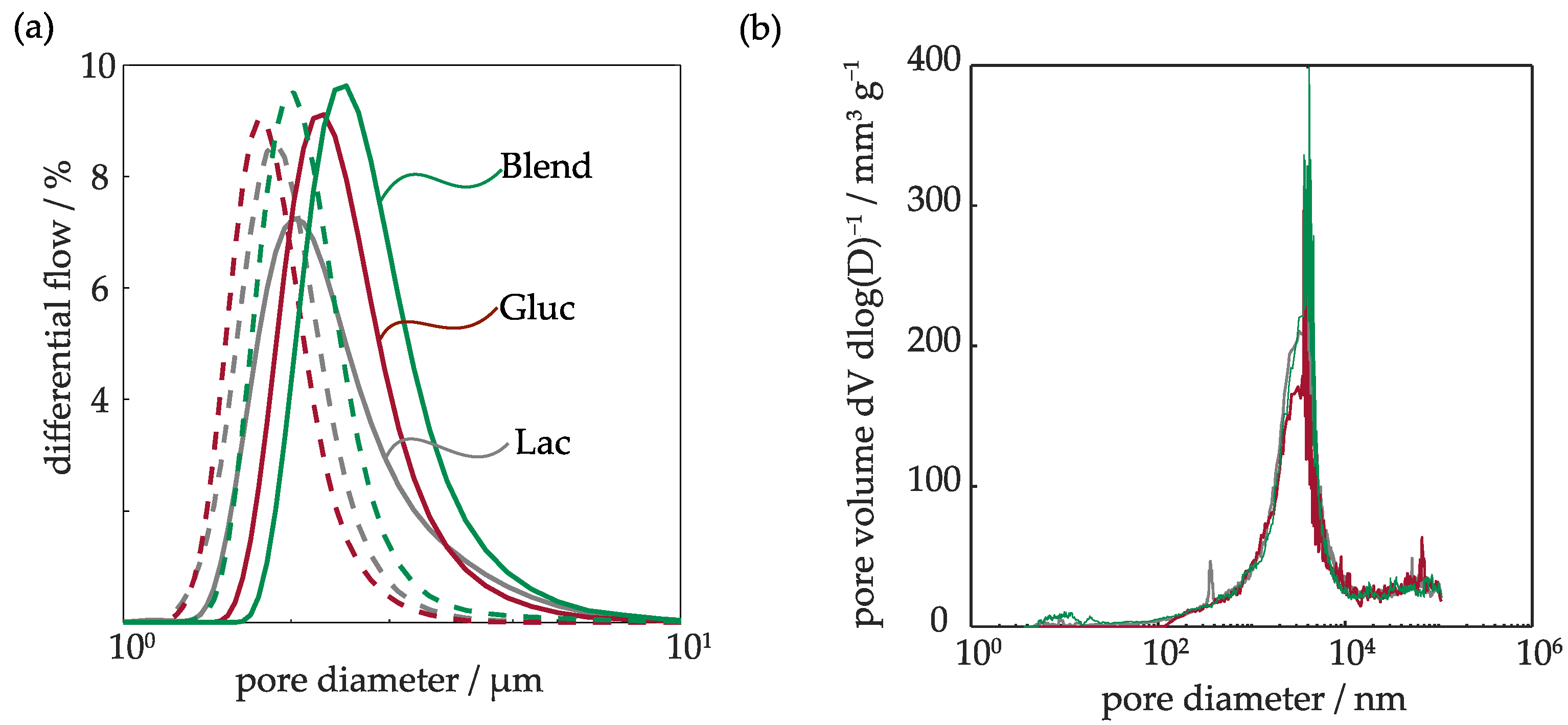
2.1.2. Pore Diameter and Surface Area
2.2. Electrochemical Results
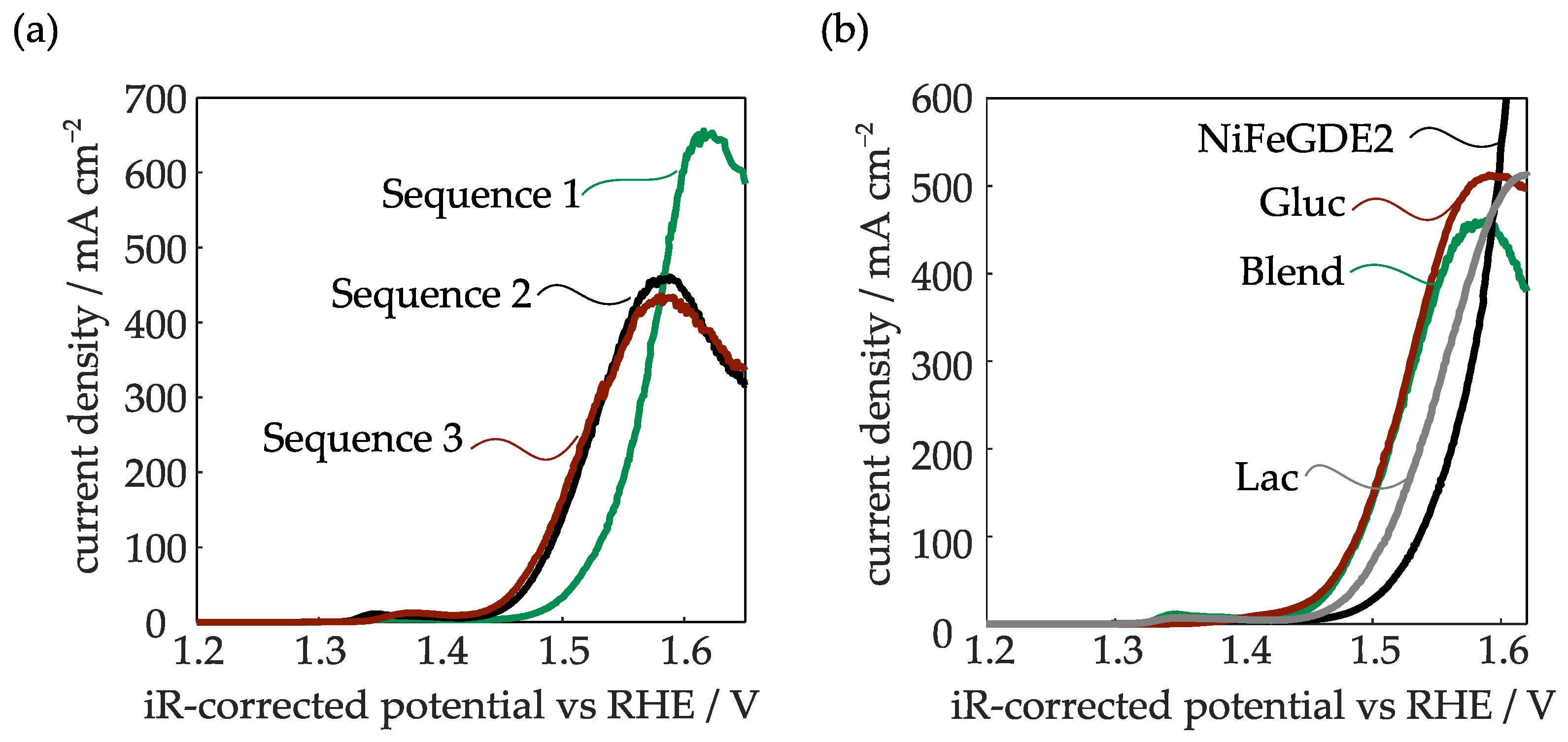
2.2.1. Linear Sweep Voltammetry
2.2.2. Tafel and EIS Analysis
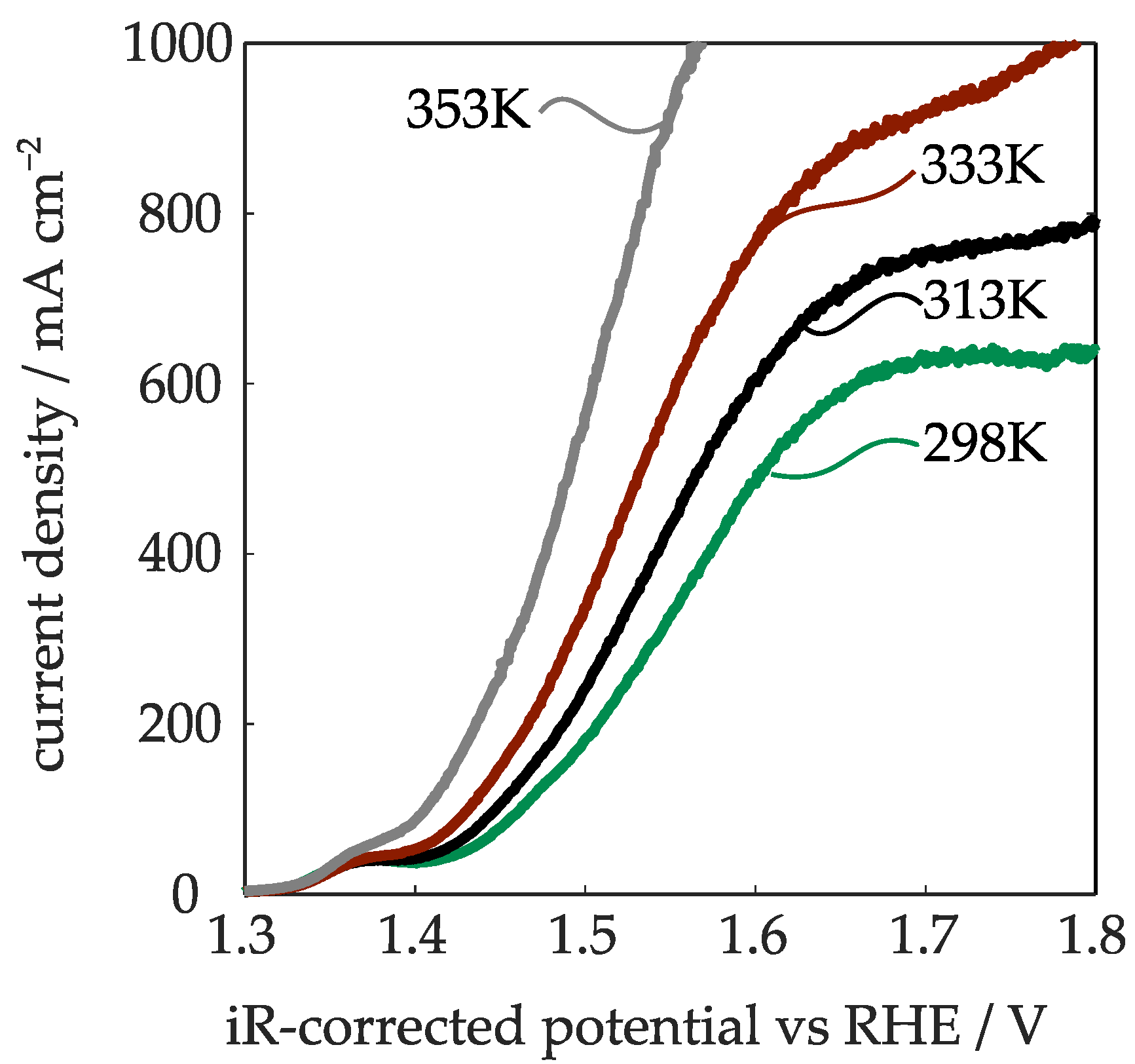
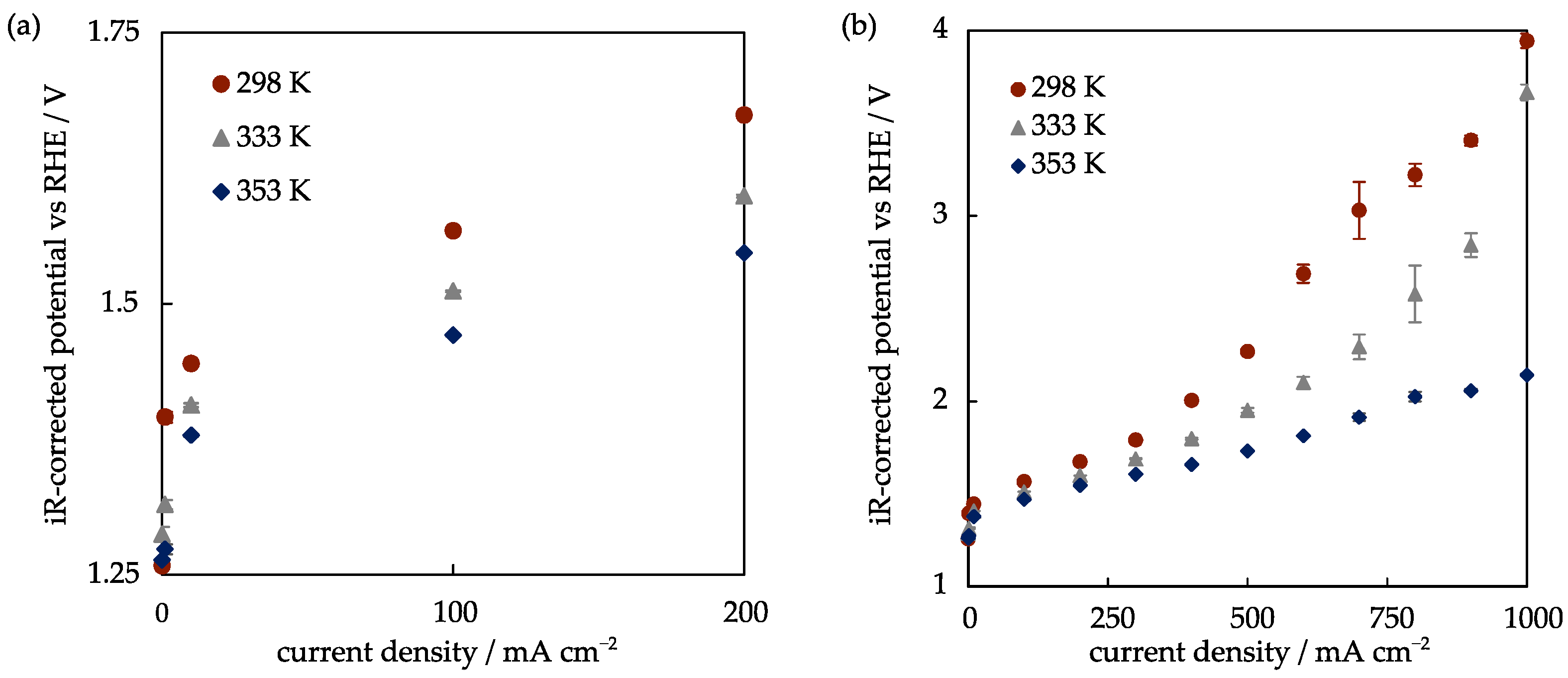
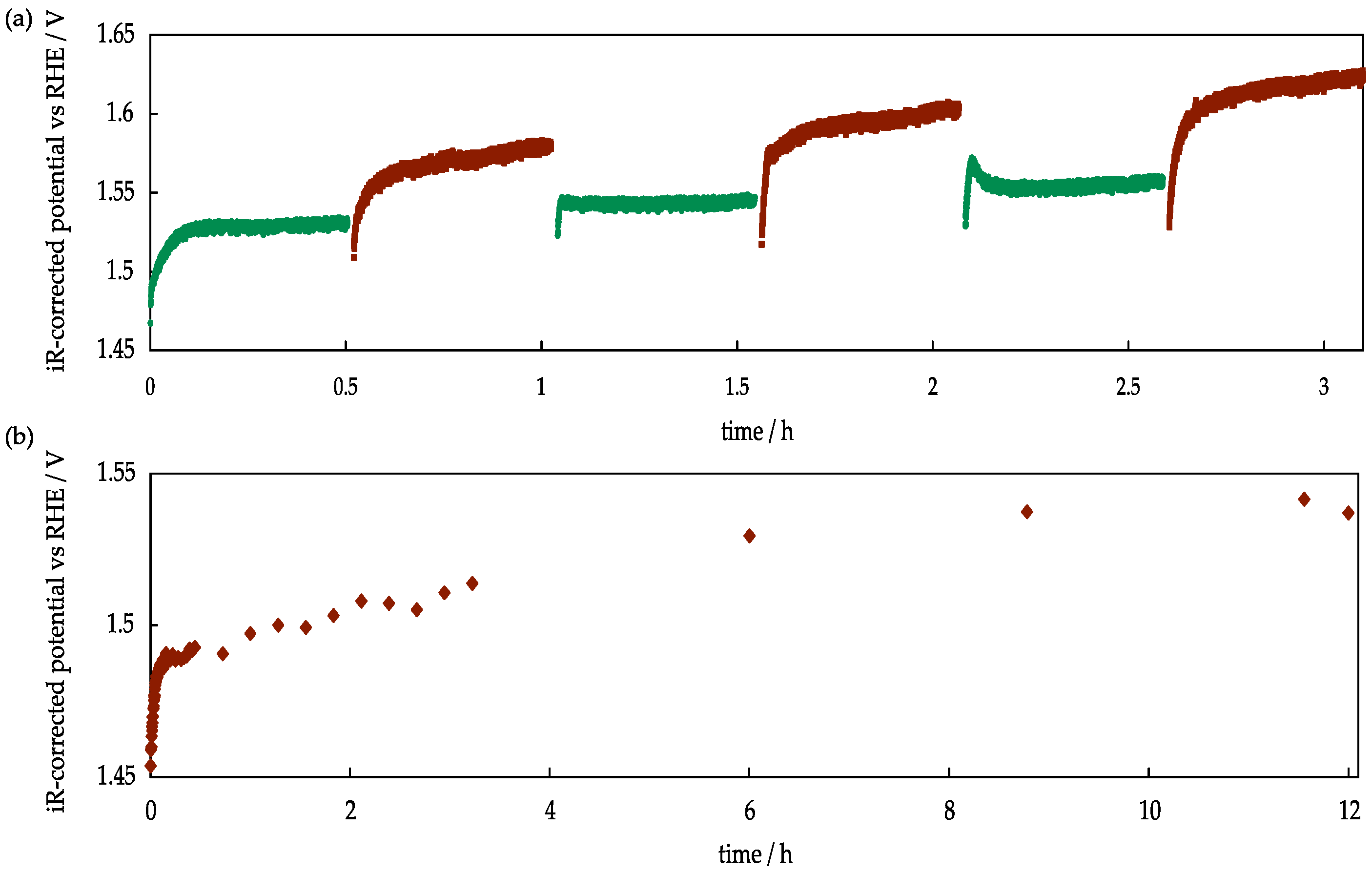
2.2.3. Temperature Influence
3. Materials and Methods
3.1. GDE Manufacture
3.2. Physical Characterization
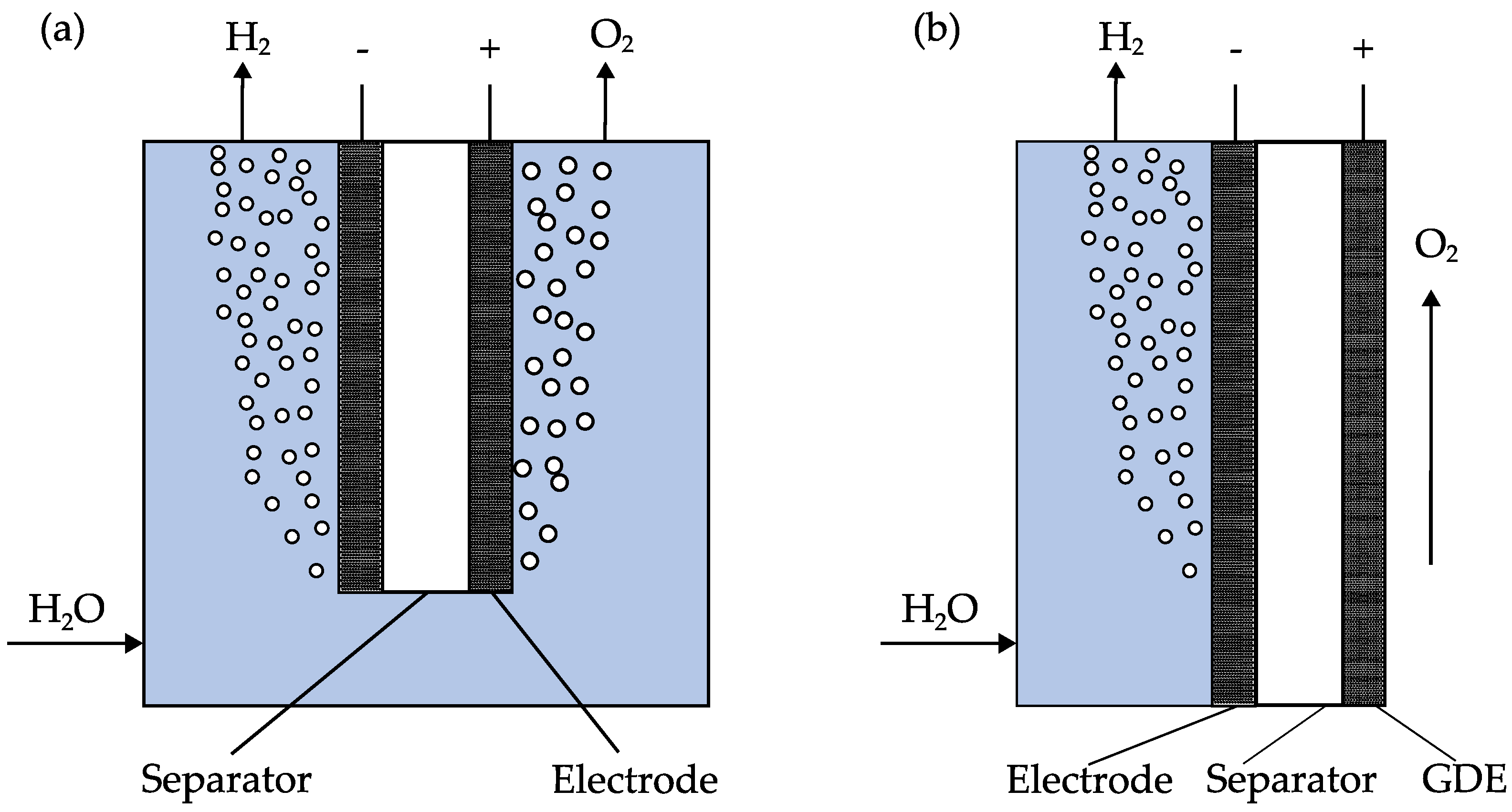
3.3. Electrochemical Cell
3.4. Electrochemical Techniques
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- IEA—International Energy Agency. Global Hydrogen REVIEW 2021. Available online: https://iea.blob.core.windows.net/assets/5bd46d7b-906a-4429-abda-e9c507a62341/GlobalHydrogenReview2021.pdf (accessed on 16 August 2023).
- McKinsey & Company. Hydrogen for Net-Zero: A Critical Cost-Competitive Energy Vector. Available online: https://hydrogencouncil.com/wp-content/uploads/2021/11/Hydrogen-for-Net-Zero.pdf (accessed on 25 August 2023).
- McKinsey & Company. Hydrogen Insights: A Perspective on Hydrogen Investment Market Development and Cost Competitiveness. Available online: https://hydrogencouncil.com/wp-content/uploads/2021/02/Hydrogen-Insights-2021.pdf (accessed on 24 August 2023).
- Moberg, J.; Bartlett, B. The Mirage of Blue Hydrogen is Fading. Available online: https://gh2.org/blog/mirage-blue-hydrogen-fading (accessed on 18 August 2023).
- Zhang, H.; Guan, D.; Hu, Z.; Huang, Y.-C.; Wu, X.; Dai, J.; Dong, C.-L.; Xu, X.; Lin, H.-J.; Chen, C.-T.; et al. Exceptional lattice-oxygen participation on artificially controllable electrochemistry-induced crystalline-amorphous phase to boost oxygen-evolving performance. Appl. Catal. B Environ. 2021, 297, 120484. [Google Scholar] [CrossRef]
- Guan, D.; Xu, H.; Zhang, Q.; Huang, Y.-C.; Shi, C.; Chang, Y.-C.; Xu, X.; Tang, J.; Gu, Y.; Pao, C.-W.; et al. Identifying A Universal Activity Descriptor and a Unifying Mechanism Concept on Perovskite Oxides for Green Hydrogen Production. Adv. Mater. 2023, e2305074. [Google Scholar] [CrossRef] [PubMed]
- Etzold, B.J.; Krewer, U.; Thiele, S.; Dreizler, A.; Klemm, E.; Turek, T. Understanding the activity transport nexus in water and CO2 electrolysis: State of the art, challenges and perspectives. Chem. Eng. J. 2021, 424, 130501. [Google Scholar] [CrossRef]
- David, M.; Ocampo-Martínez, C.; Sánchez-Peña, R. Advances in alkaline water electrolyzers: A review. J. Energy Storage 2019, 23, 392–403. [Google Scholar] [CrossRef]
- Chatenet, M.; Pollet, B.G.; Dekel, D.R.; Dionigi, F.; Deseure, J.; Millet, P.; Braatz, R.D.; Bazant, M.Z.; Eikerling, M.; Staffell, I.; et al. Water electrolysis: From textbook knowledge to the latest scientific strategies and industrial developments. Chem. Soc. Rev. 2022, 51, 4583–4762. [Google Scholar] [CrossRef] [PubMed]
- Schmidt, O.; Gambhir, A.; Staffell, I.; Hawkes, A.; Nelson, J.; Few, S. Future cost and performance of water electrolysis: An expert elicitation study. Int. J. Hydrogen Energy 2017, 42, 30470–30492. [Google Scholar] [CrossRef]
- Buttler, A.; Spliethoff, H. Current status of water electrolysis for energy storage, grid balancing and sector coupling via power-to-gas and power-to-liquids: A review. Renew. Sustain. Energy Rev. 2018, 82, 2440–2454. [Google Scholar] [CrossRef]
- Brauns, J.; Turek, T. Alkaline Water Electrolysis Powered by Renewable Energy: A Review. Processes 2020, 8, 248. [Google Scholar] [CrossRef]
- Haug, P.; Koj, M.; Turek, T. Influence of process conditions on gas purity in alkaline water electrolysis. Int. J. Hydrogen Energy 2017, 42, 9406–9418. [Google Scholar] [CrossRef]
- Perry, S.C.; Ponce de León, C.; Walsh, F.C. Review—The Design, Performance and Continuing Development of Electrochemical Reactors for Clean Electrosynthesis. J. Electrochem. Soc. 2020, 167, 155525. [Google Scholar] [CrossRef]
- Koj, M.; Qian, J.; Turek, T. Novel alkaline water electrolysis with nickel-iron gas diffusion electrode for oxygen evolution. Int. J. Hydrogen Energy 2019, 44, 29862–29875. [Google Scholar] [CrossRef]
- Koj, M.; Gimpel, T.; Schade, W.; Turek, T. Laser structured nickel-iron electrodes for oxygen evolution in alkaline water electrolysis. Int. J. Hydrogen Energy 2019, 44, 12671–12684. [Google Scholar] [CrossRef]
- Gilliam, R.; Graydon, J.; Kirk, D.; Thorpe, S. A review of specific conductivities of potassium hydroxide solutions for various concentrations and temperatures. Int. J. Hydrogen Energy 2007, 32, 359–364. [Google Scholar] [CrossRef]
- Becker, H.; Murawski, J.; Shinde, D.V.; Stephens, I.E.L.; Hinds, G.; Smith, G. Impact of impurities on water electrolysis: A review. Sustain. Energy Fuels 2023, 7, 1565–1603. [Google Scholar] [CrossRef]
- Haug, P.; Kreitz, B.; Koj, M.; Turek, T. Process modelling of an alkaline water electrolyzer. Int. J. Hydrogen Energy 2017, 42, 15689–15707. [Google Scholar] [CrossRef]
- Trinke, P.; Haug, P.; Brauns, J.; Bensmann, B.; Hanke-Rauschenbach, R.; Turek, T. Hydrogen Crossover in PEM and Alkaline Water Electrolysis: Mechanisms, Direct Comparison and Mitigation Strategies. J. Electrochem. Soc. 2018, 165, F502–F513. [Google Scholar] [CrossRef]
- Song, F.; Bai, L.; Moysiadou, A.; Lee, S.; Hu, C.; Liardet, L.; Hu, X. Transition Metal Oxides as Electrocatalysts for the Oxygen Evolution Reaction in Alkaline Solutions: An Application-Inspired Renaissance. J. Am. Chem. Soc. 2018, 140, 7748–7759. [Google Scholar] [CrossRef]
- Schmidt, V.M. Elektrochemische Verfahrenstechnik: Grundlagen, Reaktionstechnik, Prozeßoptimierung; Wiley-VCH: Weinheim, Germany, 2004; ISBN 3527602143. [Google Scholar]
- Louie, M.W.; Bell, A.T. An investigation of thin-film Ni-Fe oxide catalysts for the electrochemical evolution of oxygen. J. Am. Chem. Soc. 2013, 135, 12329–12337. [Google Scholar] [CrossRef]
- Guo, B.; Ding, Y.; Huo, H.; Wen, X.; Ren, X.; Xu, P.; Li, S. Recent Advances of Transition Metal Basic Salts for Electrocatalytic Oxygen Evolution Reaction and Overall Water Electrolysis. Nano-Micro Lett. 2023, 15, 57. [Google Scholar] [CrossRef]
- Franzen, D.; Ellendorff, B.; Paulisch, M.C.; Hilger, A.; Osenberg, M.; Manke, I.; Turek, T. Influence of binder content in silver-based gas diffusion electrodes on pore system and electrochemical performance. J. Appl. Electrochem. 2019, 49, 705–713. [Google Scholar] [CrossRef]
- Bienen, F.; Paulisch, M.C.; Mager, T.; Osiewacz, J.; Nazari, M.; Osenberg, M.; Ellendorff, B.; Turek, T.; Nieken, U.; Manke, I.; et al. Investigating the electrowetting of silver-based gas-diffusion electrodes during oxygen reduction reaction with electrochemical and optical methods. Electrochem. Sci. Adv. 2023, 3, 494. [Google Scholar] [CrossRef]
- Zhang, G.-R.; Shen, L.-L.; Schmatz, P.; Krois, K.; Etzold, B.J. Cathodic activated stainless steel mesh as a highly active electrocatalyst for the oxygen evolution reaction with self-healing possibility. J. Energy Chem. 2020, 49, 153–160. [Google Scholar] [CrossRef]
- Chen, H.; Li, J.; Shen, Y.; Jiao, W.; Wang, J.; Zou, Y.; Zou, X. Room temperature, fast fabrication of square meter-sized oxygen evolution electrode toward industrial alkaline electrolyzer. Appl. Catal. B Environ. 2022, 316, 121605. [Google Scholar] [CrossRef]
- Sequeira, C.A.C.; Santos, D.M.F.; Šljukić, B.; Amaral, L. Physics of Electrolytic Gas Evolution. Braz. J. Phys. 2013, 43, 199–208. [Google Scholar] [CrossRef]
- Marini, S.; Salvi, P.; Nelli, P.; Pesenti, R.; Villa, M.; Kiros, Y. Oxygen evolution in alkali with gas diffusion electrodes. Int. J. Hydrogen Energy 2013, 38, 11496–11506. [Google Scholar] [CrossRef]
- Graedel, T.E.; Harper, E.M.; Nassar, N.T.; Nuss, P.; Reck, B.K. Criticality of metals and metalloids. Proc. Natl. Acad. Sci. USA 2015, 112, 4257–4262. [Google Scholar] [CrossRef]
- Seh, Z.W.; Kibsgaard, J.; Dickens, C.F.; Chorkendorff, I.; Nørskov, J.K.; Jaramillo, T.F. Combining theory and experiment in electrocatalysis: Insights into materials design. Science 2017, 355, aad4998. [Google Scholar] [CrossRef]
- Sigma-Aldrich Chemie GmbH. Eisen(II)-Acetat 95% | Sigma-Aldrich. Available online: https://www.sigmaaldrich.com/DE/de/product/aldrich/339199 (accessed on 25 August 2023).
- Sigma-Aldrich Chemie GmbH. Eisen(II)-Lactat Hydrat ≥98.0% (Dried Material) | Sigma-Aldrich. Available online: https://www.sigmaaldrich.com/DE/de/product/aldrich/44953 (accessed on 25 August 2023).
- Sigma-Aldrich Chemie GmbH. Eisen(II)-D-gluconat Dihydrat 98% | Sigma-Aldrich. Available online: https://www.sigmaaldrich.com/DE/de/product/aldrich/344427 (accessed on 25 August 2023).
- Arenas, L.F.; Hadjigeorgiou, G.; Jones, S.; van Dijk, N.; Hodgson, D.; Cruden, A.; Ponce de León, C. Effect of airbrush type on sprayed platinum and platinum-cobalt catalyst inks: Benchmarking as PEMFC and performance in an electrochemical hydrogen pump. Int. J. Hydrogen Energy 2020, 45, 27392–27403. [Google Scholar] [CrossRef]
- Kreysa, G.; Ota, K.-I.; Savinell, R.F. (Eds.) Encyclopedia of Applied Electrochemistry: Chapter 3 Three-Dimensional Electrodes; The Electrosynthesis Company Inc.: New York, NY, USA, 1992; ISBN 978-1-4419-6995-8. [Google Scholar]
- Kong, Y.; Liu, M.; Hu, H.; Hou, Y.; Vesztergom, S.; Gálvez-Vázquez, M.d.J.; Zelocualtecatl Montiel, I.; Kolivoška, V.; Broekmann, P. Cracks as Efficient Tools to Mitigate Flooding in Gas Diffusion Electrodes Used for the Electrochemical Reduction of Carbon Dioxide. Small Methods 2022, 6, e2200369. [Google Scholar] [CrossRef]
- Fabbri, E.; Habereder, A.; Waltar, K.; Kötz, R.; Schmidt, T.J. Developments and perspectives of oxide-based catalysts for the oxygen evolution reaction. Catal. Sci. Technol. 2014, 4, 3800–3821. [Google Scholar] [CrossRef]
- Trotochaud, L.; Young, S.L.; Ranney, J.K.; Boettcher, S.W. Nickel-iron oxyhydroxide oxygen-evolution electrocatalysts: The role of intentional and incidental iron incorporation. J. Am. Chem. Soc. 2014, 136, 6744–6753. [Google Scholar] [CrossRef] [PubMed]
- Zou, S.; Burke, M.S.; Kast, M.G.; Fan, J.; Danilovic, N.; Boettcher, S.W. Fe (Oxy)hydroxide Oxygen Evolution Reaction Electrocatalysis: Intrinsic Activity and the Roles of Electrical Conductivity, Substrate, and Dissolution. Chem. Mater. 2015, 27, 8011–8020. [Google Scholar] [CrossRef]
- Alobaid, A.; Wang, C.; Adomaitis, R.A. Mechanism and Kinetics of HER and OER on NiFe LDH Films in an Alkaline Electrolyte. J. Electrochem. Soc. 2018, 165, J3395–J3404. [Google Scholar] [CrossRef]
- Li, G.; Anderson, L.; Chen, Y.; Pan, M.; Abel Chuang, P.-Y. New insights into evaluating catalyst activity and stability for oxygen evolution reactions in alkaline media. Sustain. Energy Fuels 2018, 2, 237–251. [Google Scholar] [CrossRef]
- Márquez, R.A.; Kawashima, K.; Son, Y.J.; Castelino, G.; Miller, N.; Smith, L.A.; Chukwuneke, C.E.; Mullins, C.B. Getting the Basics Right: Preparing Alkaline Electrolytes for Electrochemical Applications. ACS Energy Lett. 2023, 8, 1141–1146. [Google Scholar] [CrossRef]
- Hodges, A.; Renz, S.; Lohmann-Richters, F.; Al-Musawi, A.; Jupke, A.; Lehnert, W.; Swiegers, G.F.; Wallace, G.G. Critical Analysis of Published Physical Property Data for Aqueous Potassium Hydroxide. Collation into Detailed Models for Alkaline Electrolysis. J. Chem. Eng. Data 2023, 68, 1485–1506. [Google Scholar] [CrossRef]
- Schalenbach, M.; Lueke, W.; Stolten, D. Hydrogen Diffusivity and Electrolyte Permeability of the Zirfon PERL Separator for Alkaline Water Electrolysis. J. Electrochem. Soc. 2016, 163, F1480–F1488. [Google Scholar] [CrossRef]
- Bubble-Point Test Method, 2001. Available online: https://www.sae.org/standards/content/arp901a/ (accessed on 30 August 2023).
- Koj, M. Entwicklung und Charakterisierung von Elektroden für die Sauerstoffentwicklung in der alkalischen Wasserelektrolyse. Ph.D. Thesis, TU Clausthal, Clausthal-Zellerfeld, Germany, 2021. [Google Scholar]
- Ende, D.; Mangold, K.-M. Impedanzspektroskopie. Chem. Unserer Zeit 1993, 27, 134–140. [Google Scholar] [CrossRef]
- Shinagawa, T.; Garcia-Esparza, A.T.; Takanabe, K. Insight on Tafel slopes from a microkinetic analysis of aqueous electrocatalysis for energy conversion. Sci. Rep. 2015, 5, 13801. [Google Scholar] [CrossRef]
- Kubisztal, J.; Budniok, A. Study of the oxygen evolution reaction on nickel-based composite coatings in alkaline media. Int. J. Hydrogen Energy 2008, 33, 4488–4494. [Google Scholar] [CrossRef]
| SBET/m2 g−1 | Mercury Porosimetry Pore Characteristics | Porometer Flow-through Pore Characteristics | |||
|---|---|---|---|---|---|
| Total Pore volume/mm3 g−1 | Mean Pore Diameter/µm | Mean Flow-through Pore Diameter/µm | Bubble Point/Bar | ||
| Lac | 0.52 | 153.3 ± 6.2 | 1.4 | 1.83 | 0.22 |
| Gluc | 0.78 | 131.1 ± 4.5 | 1.91 | 2.01 | 0.24 |
| Blend | 0.48 | 159.8 ± 1.2 | 0.32 | 2.4 | 0.18 |
| NiFeGDE2 [15] | 2.08 | 120.6 | 3.78 | 1.16 | 0.22 |
| Tafel Slope/mV dec−1 | Exchange Current Density/ A cm−2 | Overpotential (10/100 mA cm−2)/mV | |
|---|---|---|---|
| Lac | 56 | 9.2∙10−7 | 218/277 |
| Gluc | 64 | 1.9∙10−6 | 191/261 |
| Blend | 51 | 6.8∙10−7 | 204/260 |
| NiFeGDE2 [8] | 57 | 7.8∙10−7 | 233/294 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kaiser, M.; Gäde, F.; Brauns, J.; Turek, T. Enhancing Nickel-Iron Gas Diffusion Electrodes for Oxygen Evolution in Alkaline Water Electrolysis. Catalysts 2023, 13, 1266. https://doi.org/10.3390/catal13091266
Kaiser M, Gäde F, Brauns J, Turek T. Enhancing Nickel-Iron Gas Diffusion Electrodes for Oxygen Evolution in Alkaline Water Electrolysis. Catalysts. 2023; 13(9):1266. https://doi.org/10.3390/catal13091266
Chicago/Turabian StyleKaiser, Marcel, Felix Gäde, Jörn Brauns, and Thomas Turek. 2023. "Enhancing Nickel-Iron Gas Diffusion Electrodes for Oxygen Evolution in Alkaline Water Electrolysis" Catalysts 13, no. 9: 1266. https://doi.org/10.3390/catal13091266
APA StyleKaiser, M., Gäde, F., Brauns, J., & Turek, T. (2023). Enhancing Nickel-Iron Gas Diffusion Electrodes for Oxygen Evolution in Alkaline Water Electrolysis. Catalysts, 13(9), 1266. https://doi.org/10.3390/catal13091266








