Parameter Estimation of the Stochastic Primary Nucleation Kinetics by Stochastic Integrals Using Focused-Beam Reflectance Measurements
Abstract
:1. Introduction
2. Materials and Methods
2.1. Substances and Devices
2.2. Experimental Procedure
2.3. Mathematical Model
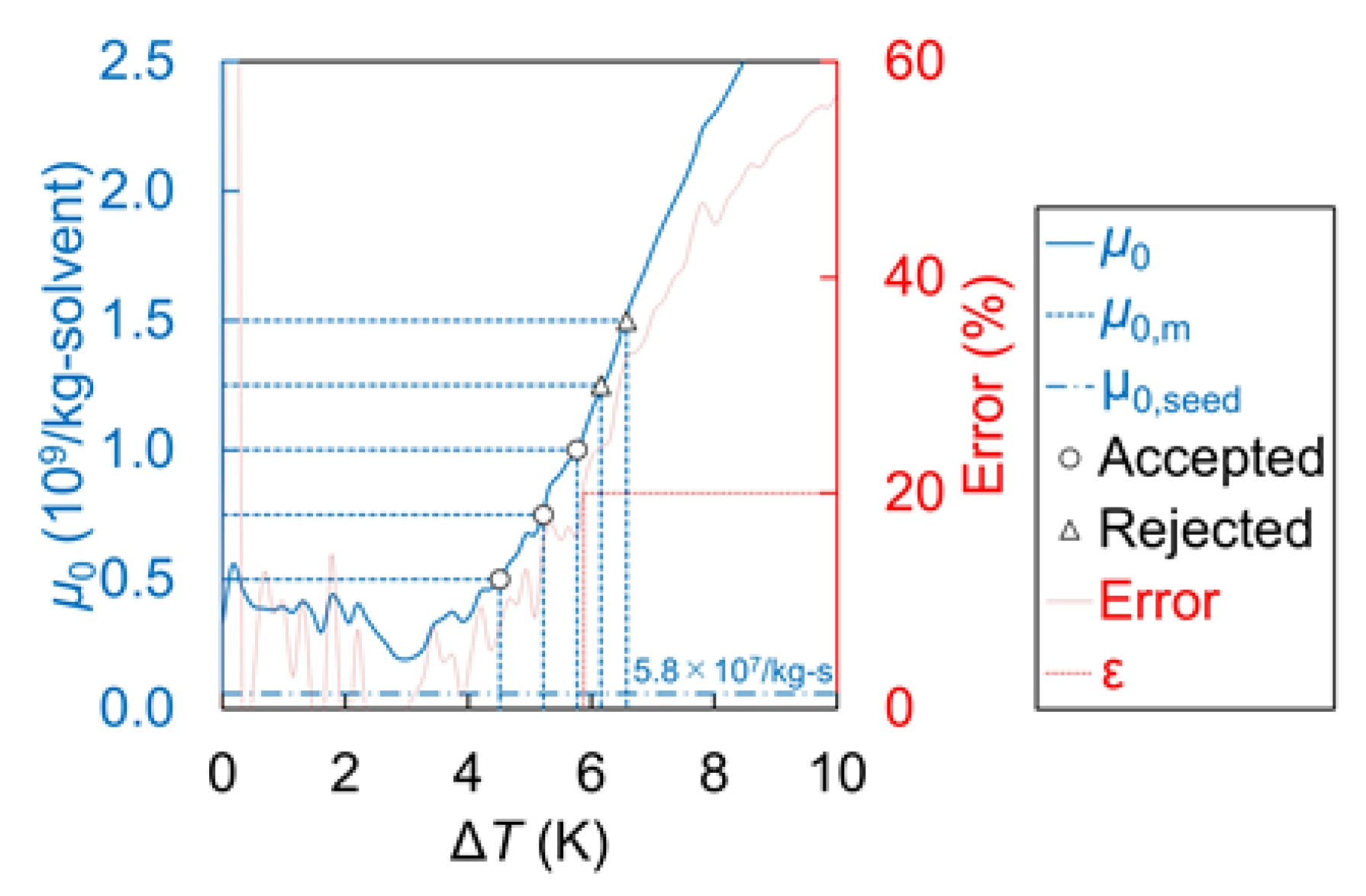
2.4. Parameter Estimation
2.5. Validation
3. Results and Discussion
3.1. Parameter Estimation
3.2. Validation
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Weng, J.; Huang, Y.; Hao, D.; Ji, Y. Recent advances of pharmaceutical crystallization theories. Chin. J. Chem. Eng. in press. [CrossRef]
- El-Zhry El-Yafi, A.K.; El-Zein, H. Technical crystallization for application in pharmaceutical material engineering: Review article. Asian J. Pharm. Sci. (Amsterdam, Neth.) 2014, 10, 283–291. [Google Scholar] [CrossRef] [Green Version]
- Tung, H.-H. Industrial perspectives of pharmaceutical crystallization. Org. Process Res. Dev. 2013, 17, 445–454. [Google Scholar] [CrossRef]
- Artusio, F.; Pisano, R. Surface-induced crystallization of pharmaceuticals and biopharmaceuticals: A review. Int. J. Pharm. (Amsterdam, Neth.) 2018, 547, 190–208. [Google Scholar] [CrossRef]
- Sleutel, M.; Van Driessche, A.E.S. Nucleation of protein crystals-a nanoscopic perspective. Nanoscale 2018, 10, 12256–12267. [Google Scholar] [CrossRef]
- Sear, R. What do crystals nucleate on? What is the microscopic mechanism? How can we model nucleation? MRS Bull. 2016, 41, 363–368. [Google Scholar] [CrossRef]
- Kulkarni, S.A.; Kadam, S.S.; Meekes, H.; Stankiewicz, A.I.; Ter Horst, J.H. Crystal nucleation kinetics from induction times and metastable zone widths. Cryst. Growth Des. 2013, 13, 2435–2440. [Google Scholar] [CrossRef]
- Jiang, S.; Ter Horst, J.H. Crystal nucleation rates from probability distributions of induction times. Cryst. Growth Des. 2011, 11, 256–261. [Google Scholar] [CrossRef]
- Maggioni, G.M.; Mazzotti, M. A Stochastic Population Balance Equation Model for Nucleation and Growth of Crystals with Multiple Polymorphs. Cryst. Growth Des. 2019, 19, 4698–4709. [Google Scholar] [CrossRef]
- Maggioni, G.M.; Bezinge, L.; Mazzotti, M. Stochastic nucleation of polymorphs: Experimental evidence and mathematical modeling. Cryst. Growth Des. 2017, 17, 6703–6711. [Google Scholar] [CrossRef]
- Kadam, S.S.; Kulkarni, S.A.; Coloma Ribera, R.; Stankiewicz, A.I.; ter Horst, J.H.; Kramer, H.J.M. A new view on the metastable zone width during cooling crystallization. Chem. Eng. Sci. 2012, 72, 10–19. [Google Scholar] [CrossRef]
- Kubota, N. An attempt to explain the waiting time distribution for primary nucleation of potassium sulphate from aqueous solution by a stochastic model. J. Cryst. Growth 1983, 62, 161–166. [Google Scholar] [CrossRef]
- Thijssen, H.A.C.; Vorstman, M.A.G.; Roels, J.A. Heterogeneous primary nucleation of ice in water and aqueous solutions. J. Cryst. Growth 1968, 3–4, 355–359. [Google Scholar] [CrossRef]
- Kubota, N. Analysis of the effect of volume on induction time and metastable zone width using a stochastic model. J. Cryst. Growth 2015, 418, 15–24. [Google Scholar] [CrossRef]
- Kubota, N.; Kubota, K. Secondary nucleation of magnesium sulphate from single seed crystal by fluid shear in agitated supersaturated aqueous solution. J. Cryst. Growth 1986, 76, 69–74. [Google Scholar] [CrossRef]
- Kubota, N.; Karasawa, H.; Kawakami, T. On estimation of critical supercooling from waiting times measured at constant supercooling — A secondary nucleation from a single seed crystal of potash alum suspended in agitated solution. J. Chem. Eng. Jpn. 1978, 11, 290–295. [Google Scholar] [CrossRef] [Green Version]
- Unno, J.; Kawase, H.; Kaneshige, R.; Hirasawa, I. Estimation of Kinetics for Batch Cooling Crystallization by Focused-Beam Reflectance Measurements. Chem. Eng. Technol. 2019, 42, 1428–1434. [Google Scholar] [CrossRef]
- Unno, J.; Umeda, R.; Hirasawa, I. Computing Crystal Size Distribution by Focused-Beam Reflectance Measurement when Aspect Ratio Varies. Chem. Eng. Technol. 2018, 41, 1147–1151. [Google Scholar] [CrossRef]
- Trampuž, M.; Teslić, D.; Likozar, B. Crystallization of fesoterodine fumarate active pharmaceutical ingredient: Modelling of thermodynamic equilibrium, nucleation, growth, agglomeration and dissolution kinetics and temperature cycling. Chem. Eng. Sci. 2019, 201, 97–111. [Google Scholar] [CrossRef]
- Pandit, A.; Katkar, V.; Ranade, V.; Bhambure, R. Real-Time Monitoring of Biopharmaceutical Crystallization: Chord Length Distribution to Crystal Size Distribution for Lysozyme, rHu Insulin, and Vitamin B12. Ind. Eng. Chem. Res. 2019, 58, 7607–7619. [Google Scholar] [CrossRef]
- Kim, W.-S.; Koo, K.-K. Crystallization of Azilsartan with Acidification of Azilsartan Disodium Salt. Cryst. Growth Des. 2019, 19, 1797–1804. [Google Scholar] [CrossRef]
- Ruf, A.; Worlitschek, J.; Mazzotti, M. Modeling and experimental analysis of PSD measurements through FBRM. Part. Part. Syst. Charact. 2000, 17, 167–179. [Google Scholar] [CrossRef]
- Kail, N.; Briesen, H.; Marquardt, W. Advanced geometrical modeling of focused beam reflectance measurements (FBRM). Part. Part. Syst. Charact. 2007, 24, 184–192. [Google Scholar] [CrossRef]
- Unno, J.; Hirasawa, I. Transformation of CSD When Crystal Shape Changes with Crystal Size into CLD from FBRM by Using Monte Carlo Analysis. Adv. Chem. Eng. Sci. 2017, 7, 91–107. [Google Scholar] [CrossRef] [Green Version]
- Creating and Controlling a Random Number Stream - MATLAB & Simulink - MathWorks. Available online: https://jp.mathworks.com/help/matlab/math/creating-and-controlling-a-random-number-stream.html?lang=en (accessed on 26 April 2020).
- Matsumoto, M.; Nishimura, T. Mersenne Twister: A 623-Dimensionally Equidistributed Uniform Pseudo-Random Number Generator. ACM Trans. Model. Comput. Simul. 1998, 8, 3–30. [Google Scholar] [CrossRef] [Green Version]
- Uniformly distributed random rotations - MATLAB randrot — MathWorks. Available online: https://jp.mathworks.com/help/robotics/ref/randrot.html (accessed on 26 April 2020).
- Zhang, F.; Liu, T.; Wang, X.Z.; Liu, J.; Jiang, X. Comparative study on ATR-FTIR calibration models for monitoring solution concentration in cooling crystallization. J. Cryst. Growth 2017, 459, 50–55. [Google Scholar] [CrossRef]
- Nicoud, L.; Licordari, F.; Myerson, A.S. Polymorph control in batch seeded crystallizers. A case study with paracetamol. CrystEngComm 2019, 21, 2105–2118. [Google Scholar] [CrossRef] [Green Version]
- Soto, R.; Verma, V.; Rasmuson, Å.C. Crystal Growth Kinetics of a Metastable Polymorph of Tolbutamide in Organic Solvents. Cryst. Growth Des. 2020, 20, 1985–1996. [Google Scholar] [CrossRef]
- Mullin, J.W. Crystallization, 4th ed.; Butterworth-Heinemann: Oxford, UK, 2001; pp. 181–288. [Google Scholar]
- Kubota, N. A new interpretation of metastable zone widths measured for unseeded solutions. J. Cryst. Growth 2008, 310, 629–634. [Google Scholar] [CrossRef]
- Kubota, N. A unified interpretation of metastable zone widths and induction times measured for seeded solutions. J. Cryst. Growth 2010, 312, 548–554. [Google Scholar] [CrossRef]
- Solve nonstiff differential equations — medium order method - MATLAB ode45 – MathWorks. Available online: https://jp.mathworks.com/help/matlab/ref/ode45.html?lang=en (accessed on 26 April 2020).
- Shampine, L.F.; Reichelt, M.W. The MATLAB ode suite. SIAM J. Sci. Comput. 1997, 18, 1–22. [Google Scholar] [CrossRef] [Green Version]
- Random numbers from Poisson distribution - MATLAB poissrnd - MathWorks. Available online: https://jp.mathworks.com/help/stats/poissrnd.html?lang=en (accessed on 26 April 2020).
- Randolph, A.D.; Larson, M.A. Transient and steady state size distributions in continuous mixed suspension crystallizers. AIChE J. 1962, 8, 639–645. [Google Scholar] [CrossRef]
- Hulburt, H.M.; Katz, S. Some problems in particle technology. A statistical mechanical formulation. Chem. Eng. Sci. 1964, 19, 555–574. [Google Scholar] [CrossRef]
- Gridded data interpolation - MATLAB – MathWorks. Available online: https://jp.mathworks.com/help/matlab/ref/griddedinterpolant.html?lang=en (accessed on 26 April 2020).
- Solve nonlinear least-squares (nonlinear data-fitting) problems - MATLAB lsqnonlin – MathWorks. Available online: https://jp.mathworks.com/help/optim/ug/lsqnonlin.html?lang=en (accessed on 26 April 2020).
- Coleman, T.F.; Li, Y. An interior trust region approach for nonlinear minimization subject to bounds. SIAM J. Optim. 1996, 6, 418–445. [Google Scholar] [CrossRef] [Green Version]
- Coleman, T.F.; Li, Y. On the convergence of interior-reflective Newton methods for nonlinear minimization subject to bounds. Math. Program. 1994, 67, 189–224. [Google Scholar] [CrossRef]
- Empirical cumulative distribution function - MATLAB ecdf - MathWorks. Available online: https://jp.mathworks.com/help/stats/ecdf.html?lang=en (accessed on 26 April 2020).
- Marsaglia, G.; Tsang, W.W.; Wang, J. Evaluating Kolmogorov’s distribution. J. Stat. Softw. 2003, 8, 1–4. [Google Scholar] [CrossRef]
- Two-sample Kolmogorov-Smirnov test - MATLAB kstest2 - MathWorks. Available online: https://jp.mathworks.com/help/stats/kstest2.html?lang=en (accessed on 26 April 2020).
- Passalacqua, A.; Laurent, F.; Madadi-Kandjani, E.; Heylmun, J.C.; Fox, R.O. An open-source quadrature-based population balance solver for OpenFOAM. Chem. Eng. Sci. 2018, 176, 306–318. [Google Scholar] [CrossRef] [Green Version]
- Vanni, M. Approximate population balance equations for aggregation-breakage processes. J. Colloid Interface Sci. 2000, 221, 143–160. [Google Scholar] [CrossRef]








| Name | Symbol | Unit | Value |
|---|---|---|---|
| Density | ρc | (kg/m3) | 1324 |
| Volume shape factor | kv | (−) | 1/9 2 |
| MWhydrate/MWanhydrate 1 | Rh | (−) | 1.207 |
| Nucleated crystal size | L0 | (μm) | 8.15 2 |
| Coefficients of Equation (4) | αsat | (10−6 g/g−solvent/°C 2) | 182 2 |
| βsat | (10−3 g/g−solvent/°C) | −1.37 2 | |
| γsat | (g/g−solvent) | 0.102 2 |
| Name | Preliminary | Main |
|---|---|---|
| Crystal shape | Square prism 1 | |
| Aspect ratio | 3 1 | |
| Number of trials 2 | 50,000,000 | |
| Size of S | 90 × 90 | 100 × 50 |
| Model | M500 | S400A |
| Measuring interval | 10 s | 15 s |
| Axis | Logarithmic | Linear |
| Range | 1−1000 μm | 0−1000 μm |
| Number of channels | 90 | 100 |
| Name | Preliminary | Main |
|---|---|---|
| Wavenumber | 1439−1380 cm−1 * | |
| Model | ReactIR 45 m | ReactIR 15 |
| Measuring interval | 10 s | 15 s |
| Name | Unit | Secondary Nucleation | Growth | Main |
|---|---|---|---|---|
| Saturated temperature | (°C) | 40 | 45 | |
| Initial temperature | (°C) | 45 | 50 | |
| Final temperature | (°C) | 20 | 15 | |
| Mass of seed crystals | (mg) | 50 | 0 | |
| Cooling rate | (K/min) | 0.2, 0.4, 0.6, 0.8, 1.0, 1.2, 1.4, 1.6, 1.8, 2.0 | 0.2, 0.3, 0.4, 0.5, 0.6 | 35/60 ≈ 0.583 (×25 runs) |
| Name | Symbol | Unit | Value |
|---|---|---|---|
| Primary nucleation coefficient | kb1 | (/kg−solvent/s) | 69.7 |
| Primary nucleation order | b1 | (−) | 2.28 |
| Secondary nucleation coefficient | kb2 | (1010/s/m3/Kb2) | 1.65 |
| Secondary nucleation order | b2 | (−) | 2.67 |
| Growth coefficient | kg | (μm/s) | 4.15 |
| Growth order | g | (−) | 2.32 |
| Name | j’ = 0 | j’ = 1 | j’ = 2 | j’ = 3 |
|---|---|---|---|---|
| Threshold (105) | 3 | 6 | 15 | 30 |
| Fraction of max. (%) | 1 | 2 | 5 | 10 |
| Mean (s) | 1454 | 1524 | 1571 | 1708 |
| Standard deviation (s) | 133 | 71.1 | 75.4 | 296 |
| Accepted or rejected | Rejected | Accepted | Accepted | Rejected |
| p-value for stochastic B2 | 7.95 × 10−4 | 0.198 | 0.235 | 1.78 × 10−4 |
| p-value for deterministic B2 | 1.48 × 10−3 | 0.152 | 0.166 | 1.78 × 10−4 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Unno, J.; Hirasawa, I. Parameter Estimation of the Stochastic Primary Nucleation Kinetics by Stochastic Integrals Using Focused-Beam Reflectance Measurements. Crystals 2020, 10, 380. https://doi.org/10.3390/cryst10050380
Unno J, Hirasawa I. Parameter Estimation of the Stochastic Primary Nucleation Kinetics by Stochastic Integrals Using Focused-Beam Reflectance Measurements. Crystals. 2020; 10(5):380. https://doi.org/10.3390/cryst10050380
Chicago/Turabian StyleUnno, Joi, and Izumi Hirasawa. 2020. "Parameter Estimation of the Stochastic Primary Nucleation Kinetics by Stochastic Integrals Using Focused-Beam Reflectance Measurements" Crystals 10, no. 5: 380. https://doi.org/10.3390/cryst10050380
APA StyleUnno, J., & Hirasawa, I. (2020). Parameter Estimation of the Stochastic Primary Nucleation Kinetics by Stochastic Integrals Using Focused-Beam Reflectance Measurements. Crystals, 10(5), 380. https://doi.org/10.3390/cryst10050380





