1. Introduction
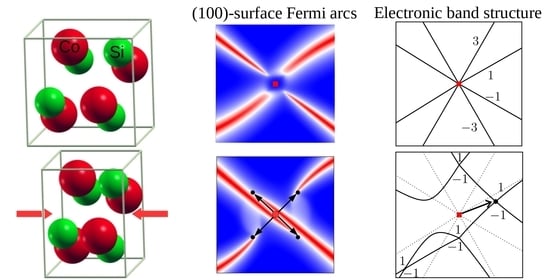
Cobalt monosilicide crystallizes in the cubic noncentrosymmetric space group #198 (P2
3). The unit cell and the Brillouin zone of CoSi are shown in
Figure 1a,b. The band structure, magnetic, optical, transport and, in particular, thermoelectric properties of CoSi have been extensively studied [
1,
2,
3,
4,
5,
6,
7,
8,
9,
10,
11,
12,
13,
14,
15]. Initially, the interpretation of experimental results was based on a simple semimetallic band structure model with small energy overlap of parabolic valence and conduction bands [
1,
2]. With the development of first-principle density functional theory (DFT) methods, a more realistic CoSi band structure has emerged [
3,
4,
5,
6,
7,
8]. Band structures calculated with and without the account of spin-orbit coupling (SOC) are plotted in
Figure 1c,d, respectively. Earlier calculations [
3,
4] without the account of SOC revealed the presence of multiple band crossings at the
and
R points of the Brillouin zone, but they did not consider the topology of the band structure. The symmetry analysis allowed to predict the existence of chiral fermions and multifold band crossings with high topological charges in crystals belonging to several space groups (including space group #198) in the presence of time-reversal symmetry [
16,
17]. In CoSi, multifold linear band crossing and spin texture was initially investigated around the
point, based on first-principle fully-relativistic calculations [
5]. Later, detailed studies of band structure topology were made for CoSi [
6,
8] and for isostructural RhSi [
7]. Effective
Hamiltonians around the time-reversal invariant momentum (TRIM) points were written down in Ref. [
17] for
R point and in Ref. [
8] for
point. It was shown [
6,
8] that the topological charges at the
and
R points are equal to
and there are four surface Fermi arcs, connecting projections of these points on the surface Brillouin zone. Because spin-orbit coupling in CoSi is not strong, the Chern numbers were also calculated without SOC [
6,
18]. It was shown that multifold nodes have large topological charges of
even without SOC (see
Figure 1d for illustration). The existence of multifold fermions and surface Fermi arcs in CoSi was recently confirmed by angle resolved photoemission spectroscopy (ARPES) [
18,
19,
20].
The theoretical study of the band structure beyond DFT, taking into account dynamic on-site correlations of d-electrons, revealed that, in contrast to FeSi, the electronic states in CoSi are only moderately influenced by electronic correlations [
21]. Band broadening in CoSi is small in the range of ±0.3 eV near the Fermi level and decreases with the temperature. Thus, DFT description of CoSi band structure should give quite accurate results, that is confirmed by ARPES experiments [
18,
19,
20] and by the better agreement of calculated lattice constants and elastic modules with experimental results for CoSi, compared to other monosilicides of the elements of the 4th period [
22].
New information on the band structure of CoSi prompted to study the manifestation of its non-trivial topology and provided a base for correct interpretation of experimental results on conventional transport properties of the compound. For example, the account of real band structure and energy dependent relaxation time allowed to adequately explain the concentration dependencies of thermoelectric and galvanomagnetic properties of Co
Fe
Si and Co
Ni
Si alloys [
10,
11,
12]. Recently, the effects of nonstoichiometry of CoSi-based materials on thermoelectric [
9] and magnetic [
14] properties were studied. In particular, in samples with the excess of Co, magnetically ordered states with helical and skyrmionic spin structures were observed near room temperature [
14]. Quantum oscillations of thermopower with a beating pattern were observed in high-quality CoSi crystals [
13]. They were successfully interpreted by the coexistence of two close Fermi surfaces in agreement with DFT results for the band structure. The influence of chiral fermions and charge density waves on magnetic field dependent electrical transport was studied in [
23]. The experimental and theoretical investigation of optical conductivity of CoSi revealed various exotic multifold quasiparticles [
15]. Moreover, low-frequency part of optical conductivity spectrum confirmed the existence of previously experimentally unobserved four-fold spin-3/2 node at the
point [
15].
As the features of the band structure topology are due to particular crystal symmetry of CoSi, it is interesting to investigate the evolution of these properties when the symmetry changes. Such changes can appear due to mechanical stress, for example, in thin film devices or experimental setups, and can be important for device operation or interpretation of experimental data. In addition to the change of symmetry, mechanical deformation, in principle, can lead to the opening of a gap in the energy spectrum and the disappearance of the topological nodes. The possibility of using CMOS-compatible CoSi thin films for thermoelectric and sensor applications were considered recently in Ref. [
24]. The stability of CoSi under hydrostatic pressure was theoretically investigated in Ref. [
25], where it was predicted that the transition to CsCl structure (
) take place at hight pressure of 270 GPa. In the present work, we theoreticaly investigate another possibility—the change of band structure under uniaxial strain. In contrast to isotropic strain, uniaxial deformation changes the crystal symmetry even at low pressure. We considered deformation in [100], [110] and [111] directions. Based on symmetry analysis, the
Hamiltonian, taking into account deformation, was constructed for both the
and
R points. Combining ab initio calculations, analytical model and symmetry considerations, the band splitting at the
and
R points, the types of nodes arising from multifold band crossings and their energy and
k-space positions were carefully studied both with and without SOC.
2. Method of Calculation
DFT calculations were performed in an integrated suite of Open-Source computer codes for electronic-structure calculations—Quantum ESPRESSO (QE) [
26], using fully relativistic optimized normconserving Vanderbilt pseudopotentials (ONCV) [
27]. The plane wave cut-off energy was 80 eV. The calculations were performed on
Monkhorst–Pack(MP) grid with the optimized lattice parameter
Å. Four atomic positions of each of atomic species in the unit cell of undeformed CoSi are
,
,
, and
. Their optimization gives
,
.
Under uniaxial deformation, we set the unit cell parameters based on corresponding strain tensor and performed the relaxation of atomic positions, that allowed to determine the space group of deformed crystal.
For detailed study of the band structure, we performed Wannier interpolation using Wannier90 [
28]. The position of nodes, topological charges and Fermi arcs were calculated using WannierTools [
29] software package.
In order to analyze low-energy excitations around the nodes at
and
R points, we constructed
Hamiltonian
in the presence of deformation from symmetry considerations. This allowed to independently verify the position of nodes and topological charges. As the effects of strain was assumed to be small, we considered only zeroth order in
k terms in Hamiltonian proportional to strain tensor [
30,
31]:
, where
are strain tensor components and
are deformation potential parameters. The independent terms can be identified, applying symmetry operations of the considered space group (P2
3, #198), as it was made for the construction of Hamiltonian without stain [
8,
16,
17]. We took into account that
is transformed under symmetry operations as a product of wave vector components
, and used irreducible representations of space (double space) groups from Bilbao Crystallographic Server [
32] for the case without (with) spin-orbit coupling. Since the spin-orbit coupling is also small in CoSi, we did not consider terms in
that depend on both strain and spin-orbit interaction. As will be seen from what follows, this approximation is sufficient.
The form of obtained Hamiltonians and their parameters are given in
Appendix A. In the equations we used eV as units of energy. The wave vector components
(in crystal coordinates) are measured in fractions of the reciprocal lattice vectors. The wave vector components
(in Cartesian coordinates) are measured in the units of
Å
. If not stated otherwise, the latter units were used in band structure plots.
The deformation potential parameters were obtained using shifts of energy levels from ab initio calculations of undeformed and deformed crystal. The deformation potential parameters at the diagonal elements of strain tensor
in
Hamiltonian determine the absolute shift of energy levels upon deformation. For CoSi, as metallic material, the absolute shift of energy level
due to deformation can be calculated as
, where
are energy levels in deformed (undeformed) crystal, and
are corresponding Fermi levels (see
Appendix B for details).
3. Results without SOC
The band structure of CoSi without SOC features a triply-degenerate energy level at the
point close to the Fermi level. It is plotted in the inset of the
Figure 1d and in the
Figure 2 with dotted lines. The wave functions are transformed according to the three-dimensional single-valued representation
of the little group of the
point of P
(#198). The low-energy excitations around this point can be considered as effective spin-1 quasiparticles [
33]. The topological charges of lower and upper linear branches are
and 2 respectively, while nearly flat band has zero charge.
Let us consider the simplest deformation of a crystal along the crystallographic direction
. When stretched along this direction, the spatial symmetry of group P
(#198) is lowered to P2
2
2
(#19), and essential remaining symmetry elements are:
,
,
. At the
point, this group has only one-dimensional irreducible representations and, using character theory, we can expect that the three-dimensional representation
of the space group P
(#198) splits into three one-dimensional representations of P
(#19) as:
As
are real single-valued representations, they are not combined due to time-reversal symmetry (TRS) [
32].
Thus, without taking into account the spin-orbit interaction, the triply-degenerate level at the
point is split into three with different energies. The low-energy band structure around the
point in CoSi under 1% uniaxial deformation in [100] direction is shown in the
Figure 2 with solid lines for compressive strain and with dashed lines for tensile strain.
As the time-reversal symmetry is preserved in the considered cases, the whole energy spectrum around the TRIM point is symmetric with respect to the change of the sign of the wave vector
. In addition, our
Hamiltonian is linear both in
and in components of deformation tensor
. Hence, the low-energy band structure for stretched crystal can be obtained from the band structure of compressed crystal by changing the sign of energy
, that can be seen by comparing solid and dashed curves in the
Figure 2. It should be noted that this conclusion applies to all considered cased with the exception of eight-band Hamiltonian around the
R point including SOC, as the latter contains terms independent of both wave vector and deformation tensor.
In compressed crystal there are nodes shifted in
and
directions upwards and downwards in energy relative to unstrained case, as shown in
Figure 2. The topological charges of both nodes are equal to
. In stretched crystal, the sign of the energy changes, and the nodes swap. In both cases, we have two doubly degenerate (spinless) nodes at the same energy at the positions
with the total topological charge of
and similar nodes at positions
. In the case, when only
is not zero, the node positions for small deformation can be obtained from eigenvalues of
Hamiltonian, and are equal to
and
. In these expressions
v is the Fermi velocity at the
point in unstrained crystal and
are the deformation potential parameters, defined in
Appendix A after Equations (
A1) and (
A2).
It can be seen also, that the dispersion around doubly degenerate nodal points is tilted. As was shown in the Ref. [
34], the general form of the Hamiltonian for Weyl point is the following:
, where
is a
matrix of coefficients and
are the
unit matrix and the three Pauli matrices for
and
respectively. The spectrum can be written as
, where
and
. The nodal point is of the type II if there is a direction, in which
. In the present case, it can be shown that in linear approximation
in
or
directions independently of the magnitude of strain
e. Thus, under strain the nodal points are at the border of transition from type I to type II nodal points. However, it should be emphasized that, in contrast to ordinary type-II Weyl fermions, fermion states discussed here are spin degenerate.
The shift of nodes in reciprocal space implies the modification of the shape of surface Fermi arcs, that should emanate from the projections of the nodal points on the (100) surface Brillouin zone. In CoSi, it appeared that due to large extension of the Fermi arcs between the projections of the
and
R point, their general shape changes quite moderately (at the scale of the full Brillouin zone) compared to undeformed case, presented in Refs. [
6,
8]. Therefore here we illustrate their variation around the
point only for several selected cases. The
Figure 3 shows the Fermi arcs in the (100) surface Brillouin zone for the case of compressive deformation in the [100] direction for
. For better visualisation, Fermi level was shifted to the energy of nodal points. The position of nodes, obtained by
calculations, are plotted in the figure by black asterisks. It can be seen, that two nodes are shifted along
direction, and their positions correlate with the sources of two surface Fermi arcs.
When deforming in
direction, off-diagonal elements of the strain tensor begin to play a role. The spatial symmetry of deformed cobalt monosilicide is described by the space group P2
(#4) with the only symmetry element
(except lattice translations). Therefore, without taking into account the spin-orbit interaction, the three-dimensional representation
of the group
splits into three one-dimensional representations of
as:
. In this way, the degeneracy at the
point is completely lifted, as in the case of the deformation along the main unit cell directions. In the most simple case, compatible with considered symmetry, where the only non-zero components of stain tensor are
, two effective spin-1/2 nodes symmetrically diverge from
point along [110] ([1
0]) crystallographic direction and shift to lower energies in the case of compressive (tensile) strain
(
). Similar nodes appear at higher energies but they are shifted along [1
0] ([110]) directions for
(
). In more general case, when
, that corresponds to the absence of deformation in directions normal to [110], the nodes split along the line, that is rotated by a small angle (about
at
) from [110] ([1
0]) axis. Let’s denote these directions by
(
). The low-energy band structure for these directions and topological charges are given in the
Figure 4. The situation is somewhat similar to [100] case (see,
Figure 2), but the shift of nodes in both energy and k-space are larger. In this case we obtain again effective spin-1/2 nodes with the tilt intermediate between tilts of the type-I and type-II nodes. The total topological charge of each pair of the nodes is
.
The deformation along the
crystallographic direction needs special consideration for CoSi. When deformed in [111] direction, the symmetry of cobalt monosilicide is reduced to
space group with the single 3-fold rotation axis. Character theory suggests that without SOC, the three-dimensional representation
of
space group splits into three one-dimensional representations of
as:
. Since the representations
and
are mutually conjugate, they should be combined due to time reversal symmetry. The triply degenerate level is split into two (nondegenerate and doubly degenerate) levels, in contrast to other types of deformation in which the degeneracy is completely lifted at the
point. In this case, strain tensor was taken in the form
for all
. In deformed crystal, one unusual node is located at the
point and two other nodes are displaced along the [111] direction at the positions
with
(see the
Figure 5). In this case we obtained one node with topological charge
at the
point and two nodes with charges
at
points. The spectrum for compressive and tensile strains are again can be obtained by the energy sign change. Thus the number of nodes between the two upper bands depends on the sign of deformation
e. Another way to see this result is to plot Fermi arcs in the (001) surface Brillouin zone for compressive and tensile strain (see
Figure 6). Consider a compressed crystal. Below the Fermi level, there are two nodes shifted in [111] direction. Their projections on (001) plane are sources of two Fermi arcs (left panel). The starting points of the arcs are shifted towards the projections of the nodes (black asterisks) but do not coincide with them exactly, since the Fermi level is located above these nodes. In the case of expansion, the node with topological charge of 2 is below the Fermi level, and both Fermi arcs start from the
point (right panel).
The fermions around the node with topological charge of 2 at the
point are similar to that of quadratic double-Weyl fermions in SrSi
, described in Ref. [
35], but they arise for another reason. In SrSi
without SOC, on the four-fold rotation axes of the crystal, there are two-fold band crossings with linear dispersion. The bands are spin degenerate. The account of spin-orbit coupling adds terms independent of wave vector to the Hamiltonian that leads to a partial lifting of the degeneracy and to a change of linear dispersion to quadratic in certain directions. In CoSi, the k-independent terms in
appear due to strain even without SOC. Counting energy relative to the charge-2 node in the
Figure 5, the dispersion in [111] direction remains linear:
. While, in perpendicular direction, it becomes quadratic,
or flat. Thus, in CoSi under [111] deformation, there is a strain induced transition from spin-1 quasiparticles to quadratic effective-spin-1/2 fermions.
Let us now consider low-energy band structure around the
R point without spin-orbit coupling. In this case in unstrained CoSi, the energy level at the
R point is four-fold degenerate not considering spin (see
Figure 1d). Low energy excitations around the
R point are double spin-1/2 fermions [
33] with the Chern number −2 [
6]. The wave functions are transformed according to the direct sum of single-valued mutually conjugated complex two-dimensional representations
and
, combined due to TRS. The switching to
(#19) space group under [100] uniaxial deformation does not lead to the energy level splitting, because the representation
is transformed into the direct sum of the pseudoreal representations
of
. Thus the node at the
R point remains intact.
Under uniaxial [110] stress, the space group
is reduced to
group and the
R point goes into the
E point, which is also located at the vertex of the deformed Brillouin zone. The representation
is transformed into
. The
and
representations of
are one-dimensional and mutually conjugated, therefore they are combined due to TRS. Thus, four-fold degenerate energy level splits into two doubly degenerated levels (see
Figure 7). The nodes are split along
direction and are situated at
, where deformation potential constants are given in
Appendix A after Equation (
A3). The topological charges of these two nodes are
. Two crossing points at the
R point (
E point of P2
2
2
space group) in the
Figure 7 are not nodes as the states are degenerate on the
surface of the deformed Brillouin zone.
In the case of [111] deformation, the four-fold degenerate level at the
R point splits into three levels (two nondegenerate, and one doubly degenerate) at the corner (
T point) of deformed Brillouin zone (see
Figure 8). Representations transform according to the expression
. All representations at the
T point are one-dimensional, but
and
are mutually conjugated and should be combined due to TRS. Similarly to the case of
point, doubly degenerate node at the
T point has topological charge of
and has quadratic dispersion in the direction perpendicular to [111]. There are also nodes with tilted dispersion, shifted in the [111] direction by
. Their topological charge is
.
4. Results with SOC
Taking into account the spin-orbital coupling in the crystal without deformation, the 6-fold degenerate level at the
point splits into a doublet and 4-fold degenerate levels. Their wave functions are transformed according to the
irreducible representation and mutually conjugated
and
irreducible representations, combined due to time-reversal symmetry [
8]. The low-energy excitations around the 4-fold degenerate node at the
point are spin-3/2 fermions [
6,
33] with topological charge of 4. Its dispersion is shown in the
Figure 1c, and is also plotted with dotted lines in the
Figure 9. The doublet does not move from the
point due to deformation, so we consider in more details the evolution of fourfold node at the
point under the influence of deformation along the main crystallographic directions.
Under the deformation along [100] the fourfold degenerate level splits into two twofold degenerate levels, both corresponding to
representation of the P
(#19) group, as this two-dimensional representation is time-reversal invariant. The low-energy Hamiltonian for these levels is given by Equations (
A4) and (
A5) of
Appendix A. When the only non-zero component of deformation is
, the gap between two levels at the
point is equal to
. In general case, it is equal to
, where
is the Poisson ratio. The low-energy spectrum is shown in the
Figure 9. The doublets at the
point form Weyl nodes with unit topological charge. Four nodes are shifted from the
point along the diagonals of the
plane and each of them has a unit topological charge. In addition, two Weyl nodes shifted from the
point in the
directions appear between two lower branches of the spectrum, and two similar Weyl nodes shifted in the
directions (not shown in
Figure 9) appear between two upper branches of the spectrum. The tensile and compressive strain spectra differ from each other by a change in the sign of the energy.
Without deformation, there are four surface Fermi arcs, emanating from the projection of the nodal point at
, as the topological charge is 4. The shift of the node positions for [100] deformation (
) is accompanied by a change in the surface Fermi arcs, shown in the
Figure 10, for the case when the Fermi level coincides with the nodes located along the diagonals of the
plane. The projections of the nodes are marked with asterisks. Their positions, calculated by the
method, quite well coincide with the sources of four Fermi arcs.
Considering the deformation along [110] axis, we found similar splitting of four-fold degenerate level at the
point into 2 doublets:
, where irreducible representations
of the space group
are one-dimensional and combined together due to TRS. The energy gap at the
point is equal to
. When the only non-zero components of stain tensor are
, the four-fold degenerate node splits into 4 nodes, moving along crystallographic directions [100], [
], [010], [0
] by the distance
. Due to distortion, small deviation from corresponding Cartesian axis appears by an angle of
, which is equal, for example, to 0.3
at
. In the case of the absence of deformations in the directions, normal to [110], when
, the shift of energy levels due to volume change leads to additional deviation of nodes from Cartesian axes, which is about
at
. Similar to the case of [100] deformations, there are also two Weyl nodes below and two Weyl nodes above the node in unstrained crystal, but here they are shifted close to the diagonals of
plane. To within a change of the directions of node shifts, the low-energy spectrum around the
point for this case is qualitatively very similar to the case of [100] deformations (see the
Figure 9).
When the stress is applied along [111] axis, we obtain similar 4-fold level slitting into two doublets at the
point with
, where all irreducible representations
of the space group
(#146) are one-dimensional.
is real and it is doubled due to TRS, while
are complex conjugated and they are combined together due to TRS. These two band crossings at the
point are shown in the
Figure 11. The lower one is a Weyl node. The node at higher energy has linear dispersion in the [111] direction and nonlinear dispersion in the perpendicular plane (this branch is not shown in the figure). It looks like the triple-Weyl node [
36,
37,
38] with topological charge ± 3, but it is located at the TRIM point. In addition, near the
point, there are two groups of nodes connected by time-reversal symmetry. Each of these groups consist of 4 nodes. One of them
is shifted from the
point along the [111] axis by a distance
, which is about
at
(see
Figure 11). In the case of compressive (tensile) strain the energy of the node shifts downwards (upwards) relative to the node at the
point in unstrained crystal. More detailed calculations showed that there are another 3 nodes in each group: one of them
is shifted from
into
direction and positions of two other nodes
can be obtained using
rotation around [111] axis. The distance between nodes is rather small, about 0.0003 at
. The calculation of topological charge showed that three equivalent nodes
have topological charge of 1 each, and the node
has a charge of −1. Thus total topological charge of each group is 2, giving 4 for both groups together. The dispersion around the nodes is rather complex and is given in the
Figure 12. It can be seen that the electronic velocities are very different in different directions, and the nodes are tilted. In addition to these groups of nodes, there are also two tilted Weyl nodes in the directions [111] and
. The lowest band crossing in
Figure 11 is one of these node.
Let us now consider the effect of deformation on the low-energy spectrum around the
R point in the presence of spin-orbit coupling. In unstrained crystal, the 8-fold degenerate level at the
R point splits into 6-fold degenerate level
(double spin-1 quasiparticles) and doublet
due to SOC. The doublet is not split by the strain, so we will consider only 6-fold degenerate level (see the
Figure 1c). In the case of [100] deformation, it is split into 3 doublets with the wave functions, transforming according to the representations
,
and
of the P
(#19) group. The change of the dispersion around the
R point is shown in the
Figure 13. Two nodes at lower energy are shifted along positive and negative
(
) directions under compressive (tensile) strain. There is a crossing of four energy branches at each of these nodal points. They are a tilted double spin-1/2 nodes [
33] with Chern numbers of
. In the case of eight-band Hamiltonian, the equality
does not hold exactly. But in the case of [100] deformation the spectrum for six considered bands approximately follows this rule. This implies that there are the two additional double spin-1/2 nodes in
(
) directions for compressive (tensile) strain (see
Figure 13).
The deformation in [110] direction again leads to the splitting of 6-fold degenerate level into 3 doublets at the vertex of deformed Brillouin zone (
E point). Wave functions of two doublets are transformed according to the
representation and one doublet according to the
representation, where both
are real one-dimensional. These doublets do not form Weyl nodes, as the energy branches, starting from them are degenerate at the edges of the Brillouin zone parallel to (001) plane. Four simple Weyl nodes are formed near the
E point. They shifted mainly into
direction to the points with coordinates
, where
at
(see
Figure 14). In the case of tensile strain the shift appeared to be almost the same. As the degeneracy of the bands is completely lifted under this deformation, the topological charge of each of the 4 nodes is
.
The case of [111] deformation is similar to the two previously considered cases in the sense that one obtains 3 doublets instead of 6-fold degenerate level at the vertex of the Brillouin zone (2
, where
is real one-dimensional representations,
and
, are complex conjugated one dimensional representations of the little group of the
T point of the
space group). Each of these doublets corresponds to a Weyl node. The two band crossings at the
T point are the conventional Weyl nodes with Chern number of
. The third band crossing looks like the triple-Weyl node with the topological charge of
. It is similar to triple-Weyl node at the
point. In addition, near the
T point, there are two groups of nodes connected by time-reversal symmetry, as in the case of the
point. Two of them are simple Weyl nodes shifted into [111] and
directions, but they have topological charge 1. Around each of them, there are three conventional Weyl nodes with topological charges −1. The total charge of these eight nodes is −4. For example, in the case of compressive strain with
, one of the nodes at the [111] direction have coordinates
relative to the
T point. One of its satellites have coordinates
, and the coordinates of another two satellites
can be obtained by cyclic permutations. The dispersion around the
T point towards nodes
and
is plotted in the
Figure 15. It is almost linear. At the same time, the dispersion along the line connecting the central node with one of its satellites (
Figure 16) looks like a result of the crossing of two nonlinear bands.