Isotope Effect in Thermal Conductivity of Polycrystalline CVD-Diamond: Experiment and Theory

Abstract

We measured the thermal conductivity $\kappa (T)$ of polycrystalline diamond with natural (${}^{\mathrm{nat}}$C) and isotopically enriched (${}^{12}$C content up to 99.96 at.%) compositions over a broad temperature T range, from 5 to 410 K. The high quality polycrystalline diamond wafers were produced by microwave plasma chemical vapor deposition in CH${}_4$-H${}_2$ mixtures. The thermal conductivity of ${}^{12}$C diamond along the wafer, as precisely determined using a steady-state longitudinal heat flow method, exceeds much that of the ${}^{\mathrm{nat}}$C sample at $T>60$ K. The enriched sample demonstrates the value of $\kappa (298\mathrm{K})=25.1\pm 0.5$ W cm${}^{-1}$ K${}^{-1}$ that is higher than the ever reported conductivity of natural and synthetic single crystalline diamonds with natural isotopic composition. A phenomenological theoretical model based on the full version of Callaway theory of thermal conductivity is developed which provides a good approximation of the experimental data. The role of different resistive scattering processes, including due to minor isotope ${}^{13}$C atoms, defects, and grain boundaries, is estimated from the data analysis. The model predicts about a 37% increase of thermal conductivity for impurity and dislocation free polycrystalline chemical vapor deposition (CVD)-diamond with the ${}^{12}$C-enriched isotopic composition at room temperature.

1. Introduction

The very high thermal conductivity of diamond and the wide band-gap semiconductor makes it a very attractive material for applications in technology, electronics, and optics. In dielectric crystalline materials, including diamond, the heat is carried out almost exclusively by phonons at temperatures near and below room temperature. A significant potential for increasing the thermal conductivity of diamond lies in the isotopic purification of carbon. Despite the fact that in the natural composition of carbon isotopes ${}^{\mathrm{nat}}$C, the content of the “impurity” isotope ${}^{13}$C is only 1.07 at.% (the remaining 98.93 at.% is ${}^{12}$C), their random distribution over the lattice sites causes strong scattering of thermal phonons participating in heat conduction. As a result, the thermal conductivity of a single crystal diamond enriched to 99.93% ${}^{12}$C surpasses by about 50% that for ${}^{\mathrm{nat}}$C at room temperature, reaching 33.2 W cm${}^{-1}$ K${}^{-1}$ [1,2,3,4]. With decreasing temperature, the isotope effect increases and at $T=104$ K, the minimum temperature at which the measurements were carried out, the thermal conductivity of a diamond containing $>99.9$% ${}^{12}$C is about 410 W cm${}^{-1}$ K${}^{-1}$ [4], which is the highest value of any material at temperatures above the temperature of liquid nitrogen.

The large isotope effect in diamond at room temperature indicated a high rate of phonon scattering in normal (N) phonon-phonon processes, in which the total momentum of interacting quasiparticles is preserved [5,6,7,8,9]. The isotope effect was explained [4,10,11] in the framework of the Callaway theory [12] of thermal conductivity by a significant contribution into the total thermal conductivity $\kappa$ of the drift (Ziman) term ${\kappa }_2$, which is due to the specific role of the N-processes, in comparison with the kinetic (Debye) term ${\kappa }_1$. Note that the large isotope effect was also obtained within the framework of theoretical approaches based on the exact solution of the Boltzmann transport equation without using the relaxation time approximation [13,14,15].

The previous experiments [1,2,3,4] were performed on diamonds grown by the high-pressure and high-temperature (HPHT) technique. Another widely-used method for diamond synthesis is chemical vapor deposition (CVD), which allows for the production of larger-area polycrystalline diamond plates with columnar crystallites directed along the growth direction, with a typical average lateral size (“diameter”) of the order of several tens of microns [16]. The columnar structure leads to anisotropy of properties: The in-plane thermal conductivity ${\kappa }_{\Vert }$ is up to 10% lower than the thermal conductivity ${\kappa }_{\perp }$ in the direction perpendicular to the plate at room temperature for high-quality CVD diamonds. In this case, ${\kappa }_{\perp }\approx 22$ W cm${}^{-1}$ K${}^{-1}$, i.e., close to the thermal conductivity of the highest quality natural and synthetic single crystals [16,17,18].

The first attempt to detect the isotope effect in the thermal conductivity of CVD diamonds was unsuccessful due to the unsatisfactory quality of the samples investigated [19]. Apparently, the high concentration of crystal lattice defects severely limited the value of $\kappa$ and hid the isotopic effect. For the first time, a significant increase in the thermal conductivity of a polycrystalline CVD diamond enriched above 99.945% ${}^{12}$C compared to the ${}^{\mathrm{nat}}$C sample was observed by Graebner et al. [20]. The increase was 27% for ${\kappa }_{\Vert }$ and 40% for ${\kappa }_{\perp }$ at room temperature. Such a large anisotropy of the isotope effect is unclear. At low temperatures, the $\kappa (T)$ of the enriched sample turned out to be lower than for the ${}^{\mathrm{nat}}$C sample. This indicates that structural defects (with a dimension higher than zero) in the ${}^{12}$C sample created a significantly higher thermal resistance than in the ${}^{\mathrm{nat}}$C specimen. That is, structurally, the enriched diamond turned out to be clearly worse than its “natural” counterpart. Later, we reported on the isotope effect of 34% for ${\kappa }_{\Vert }$ measured for polycrystalline CVD diamond ${}^{12}$C at room temperature, and up to 67% at ≈150 K [21]. However, the enriched plate consisted of two layers: ${}^{\mathrm{nat}}$C on the nucleation side and the enriched ${}^{12}$C on the growth side. This two-layer structure complicated the data analysis. For an accurate experimental determination of the isotope effect, it is necessary that the compared samples with different isotopic compositions should be close in terms of chemical composition, crystal structure defects, and crystal structure. It is also important that the concentration of defects is low and the processes of phonon scattering corresponding to these defects are insignificant in comparison with scattering by isotopes.

Over the past decade, significant progress has been achieved in the technology of CVD diamond synthesis. Here, we present the results of a study of the isotope effect in the thermal conductivity of high quality polycrystalline CVD diamond with natural and enriched ${}^{12}$C isotope compositions. The experimental dependencies $\kappa (T)$ have been precisely measured in a broad temperature range, and well fitted with a Callaway theory taking into account different phonon scattering sources.

2. Materials and Methods

The polycrystalline diamond wafers were grown on silicon substrates, 57 mm in diameter in a microwave plasma CVD reactor (ARDIS-100, Optosystems) in CH${}_4$-H${}_2$ gas mixtures. The growth parameters were nominally identical: Total gas flow of 1000 sccm, CH${}_4$ content of 1.2%, pressure of 87 Torr, substrate temperature of 820 ${}^{\circ }$C, microwave power of 4.4 kW, and a growth rate of ≈1.5 $\mathsf{\mu }$m/h. The hydrogen and methane purity was 99.9999% and 99.999%, respectively. The isotopically-enriched methane ${}^{12}$CH${}_4$ containing 99.96% ${}^{12}$C was obtained by conversion of isotopically-enriched carbon monoxide ${}^{12}$CO followed by cryogenic rectification to remove chemical impurities [22].

Raman spectra (UV LabRAM HR spectrometer, excitation with Ar+ laser at wavelength of 514.5 nm) of the samples revealed only a strong narrow peak of diamond at 1332 cm${}^{-1}$ without any sign of amorphous carbon inclusions. The concentration of bound hydrogen (CH${}_x$ groups) $[{\mathrm{CH}}_x]\approx 100$ ppm ($1\mathrm{ppm}=1.76\times {10}^{17}$ cm${}^{-3}$ for a diamond crystal) was estimated from IR absorption spectra in the range 2800–3100 cm${}^{-1}$ [23]. The concentration of single substitutional nitrogen N${}_s^0$ in the neutral charge state $[{\mathrm{N}}_s^0]\approx 0.4$ ppm in the ${}^{\mathrm{nat}}$C diamond was determined with optical absorption spectroscopy from intensity of the absorption line near 270 nm wavelength, according to the procedure described in [23]. The nitrogen content in the ${}^{12}$ C diamond was higher ($[{\mathrm{N}}_s^0]\approx 1.9$ ppm) presumably due to a higher level of impurity nitrogen with the ${}^{12}$CH${}_4$ gas.

Samples for measurements ${\kappa }_{\Vert }(T)$ in the form of rectangular parallelepiped were cut from the plates using laser cutting. The sample of diamond with natural isotopic content ${}^{\mathrm{nat}}$C had a cross section of $2.00\times 0.435$ mm, and the cross section of the isotopically enriched sample ${}^{12}$C was $2.00\times 0.328$ mm. The samples were about 14 mm long. The wide faces of ${}^{\mathrm{nat}}$C plate were mechanically polished, and a heavily defective layer about 50 $\mathsf{\mu }$m thick was removed from the substrate side. From the substrate side of the ${}^{12}$C sample, ≈130 $\mathsf{\mu }$m was removed by laser ablation, and the growth side was left untreated. The grains of polycrystalline CVD diamond have a columnar form, their mean diameter increases with a distance from a nucleation side of the plate. For our samples, the mean grain size was ∼20 $\mathsf{\mu }$m on the polished nucleation side, and ∼80 $\mathsf{\mu }$m on the growth side [24].

Measurements of ${\kappa }_{\Vert }(T)$ in the range $5<T<410$ K were performed by the method of stationary heat flux. The heat flux $\mathbf{Q}$ (W/cm${}^2$) along the long axis of the sample was created using a heater H glued to the free end of the sample; the opposite end was attached to the heat sink S (see the left-hand inset in Figure 1). To determine the temperature gradient, a pair of resistive thermometers Cernox CX-1050-SD (Lake Shore Cryotronics, Inc., Westerville, OH, USA), T1 and T2, was attached to the sample using mechanical clamps. The distance $\Delta L$ between the thermometers was ≈$8.5$ mm; the temperature difference $\Delta T$ between them was set at <0.03 T. The value of thermal conductivity is determined as $\kappa =Q\Delta L/\Delta T$. The measurements were carried out in vacuum, and to minimize the error caused by radiation heat losses, the sample was placed inside a multilayered radiation shield for measurements above 95 K. The error in determining the absolute value of $\kappa$ did not exceed 3% and was mainly due to a systematic error in distance between thermometers.

3. Results

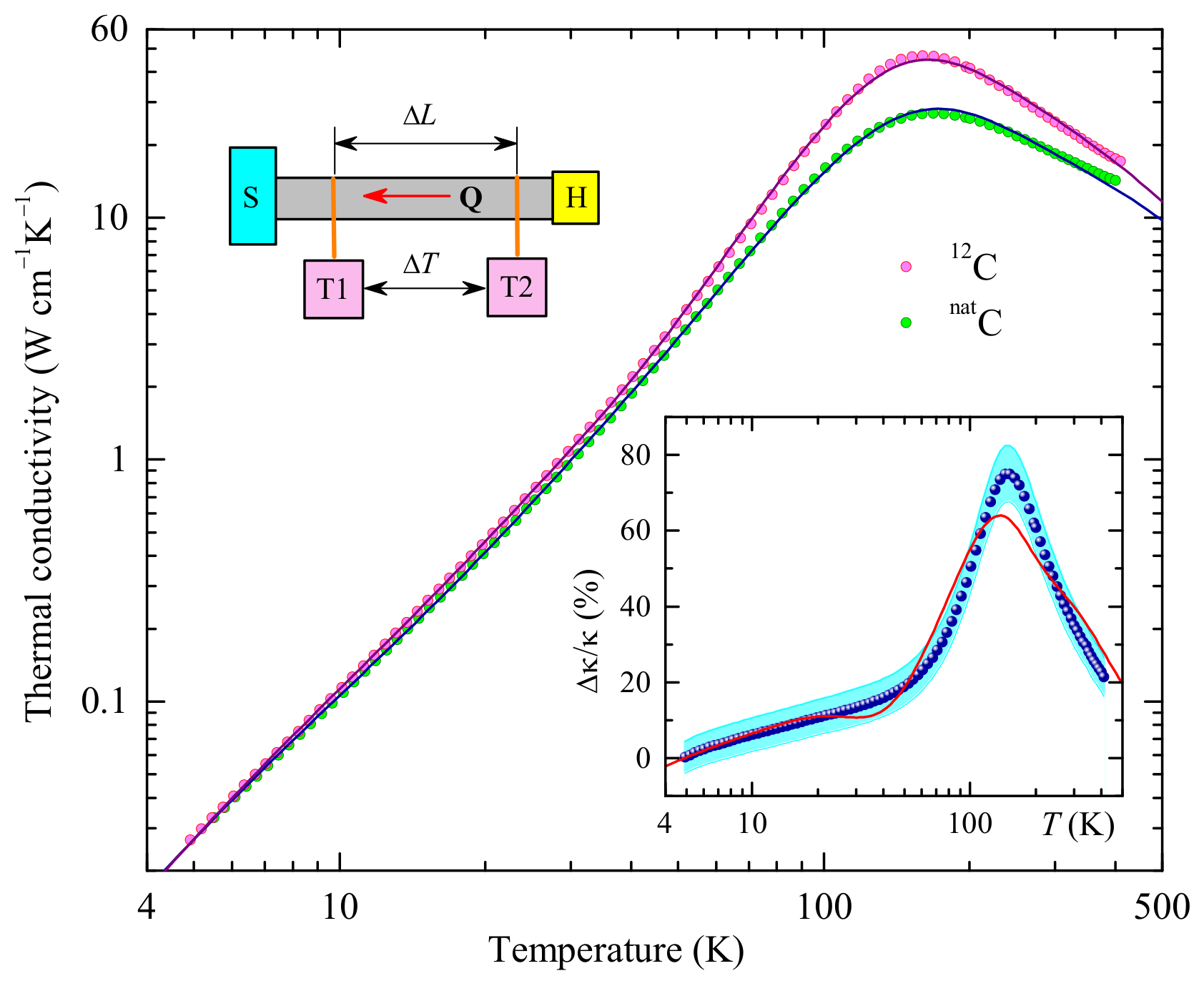
3.1. Thermal Conductivity: Experimental Data

The experimental data on the thermal conductivity ${\kappa }_{\Vert }(T)$ of CVD diamond samples over the entire temperature range studied are shown in Figure 1 (hereinafter, for simplicity, we omit the ‖ index).

Sample ${}^{\mathrm{nat}}$C demonstrates the $\kappa (T)$ typical for high quality polycrystalline CVD diamonds. In the investigated temperature range, it is close to $\kappa (T)$ for a sample of CVD diamond with the natural isotopic composition (no. RDH54b) from [20]. At temperatures from 30 to 50 K, a characteristic feature of CVD diamonds is observed in the form of a “dip” in $\kappa (T)$. The maximum thermal conductivity of the ${}^{\mathrm{nat}}$C sample is realized at $T_{\max }\approx 168$ K and at a lower temperature of 161 K for ${}^{12}$C. At room temperature (298 K), the thermal conductivity of the ${}^{\mathrm{nat}}$C sample is 18.6 W cm${}^{-1}$ K${}^{-1}$, and the ${}^{12}$C sample is 25.1 W cm${}^{-1}$ K${}^{-1}$, i.e., is 35% higher. Note that this isotopically purified polycrystalline CVD diamond exhibits thermal conductivity, which is higher than that of most perfect natural and synthetic single crystals with a natural abundance of ${}^{13}$C.

The inset to Figure 1 shows the relative difference in thermal conductivity of the ${}^{12}$C and ${}^{\mathrm{nat}}$ C samples, i.e., $\Delta \kappa /\kappa =\kappa {(}^{12}\mathrm{C})/\kappa {(}^{\mathrm{nat}}\mathrm{C})-1$ as a function of temperature. As seen, the $\Delta \kappa /\kappa$ increases with decreasing temperature, reaching a maximum of 75% at 150 K. At lower temperatures, $\Delta \kappa /\kappa$ decreases relatively rapidly to temperatures of about 60 K, and practically vanishes below 10 K.

3.2. Modeling

To analyze the experimental data we use a theoretical model proposed for heat conduction in a polycrystalline CVD diamond [25], which is based on the Callaway’s theory [12]. Neglecting the differences in phonon polarizations, and using the Debye approximation, the thermal conductivity can be presented in the following form [12]:

$$\kappa (T)={\kappa }_1+{\kappa }_2,$$

(1)

where

$$\begin{array}{ccc}\hfill {\kappa }_1& =& G{T}^3\langle {\tau }_C\rangle ,\hfill \end{array}$$

(2)

$$\begin{array}{ccc}\hfill {\kappa }_2& =& G{T}^3\frac{{\langle {\tau }_C/{\tau }_N\rangle }^2}{\langle {\tau }_C/({\tau }_N{\tau }_R)\rangle },\hfill \end{array}$$

(3)

$$\begin{array}{ccc}\hfill G& =& \frac{{k_B}^4}{2{\pi }^2{\hslash }^{3}v}.\hfill \end{array}$$

(4)

Here v is a mean sound velocity, ${\tau }_R^{-1}={\sum }_j{\tau }_j^{-1}$ is the total rate of all resistive phonon scattering processes with rates ${\tau }_j^{-1}$ discussed below, ${\tau }_N^{-1}$ is the rate of the N-processes, and ${\tau }_C^{-1}={\tau }_R^{-1}+{\tau }_N^{-1}$ is the combined scattering rate. The angle brackets $\langle y\rangle$ denote the following:

$$\langle y\rangle ={\int }_0^{T_D/T}\frac{x^4{e}^x}{{(e^x-1)}^2}y(x)dx,$$

(5)

where $x=\hslash \omega /k_{B}T$ and $\omega$ is the phonon frequency. In the case when resistive processes dominate in the phonon scattering, the $\kappa$ is determined mainly by the Debye term ${\kappa }_1$. In the opposite case, when N-processes dominate, $\kappa \approx {\kappa }_2$.

For the scattering rates in the three-phonon processes we use the expressions:

$$\begin{array}{ccc}\hfill {\tau }_N^{-1}& =& {\tilde{A}}_N\omega T^4=A_{N}x{T}^5,\hfill \end{array}$$

(6)

$$\begin{array}{ccc}\hfill {\tau }_U^{-1}& =& {\tilde{A}}_U\omega T^2{e}^{-T_D/\alpha T}=A_{U}x{T}^3{e}^{-T_D/\alpha T},\hfill \end{array}$$

(7)

where $T_D=2230$ K is the Debye temperature for diamond. The values of parameters $A_N=3.353\times {10}^{-3}{\mathrm{s}}^{-1}{\mathrm{K}}^{-5}$, $A_U=1272{\mathrm{s}}^{-1}{\mathrm{K}}^{-3}$, and $T_D/\alpha =560$ K were found to yield the best fitting of the theoretical model to experimental data on $\kappa (T)$ for a highly pure single crystal diamond [26]. In addition to the U-processes, resistive scattering processes due to phonon interactions with point defects, dislocations, charge carriers bound to dopants, intergrain regions, and external boundaries of the sample were taken into account. Such a set of resistive processes makes it possible to obtain a good approximation of the experimental data in nearly the entire temperature range of investigation.

3.2.1. External Boundaries

The rate ${\tau }_b^{-1}$ of phonon scattering by the external boundaries of the sample is represented by the following expression:

$${\tau }_b^{-1}=v/l_b,$$

(8)

where $l_b$ is the corresponding phonon free path. In the case of diffuse scattering $l_b=l_C$, $l_C$ is the Casimir length determined by the sample geometry. $l_C$ is equal to the diameter of the sample with a circular cross section. For a sample with a rectangular section of $d_1\times d_2$ length $l_C=r\times 1.115{(d_1{d}_2)}^{1/2}$, where $r⩽1$ (see, for example, [27]). When $d_1\approx d_2$, then $r\approx 1$; if $d_1$ is very different from $d_2$, then $r<1$. For the samples studied in this work, the calculated values of $l_C$ are given below in

Table 1. Parameters of the model that yield the best fit to the experimental data for chemical vapor deposition (CVD) diamond samples. The standard error, including the systematic one, is indicated in parentheses. $N_{\mathrm{ac}}$ is the concentration of acceptors in 1 cm${}^3$.

	${\mathit{N}}_{\mathbf{ac}}{({\mathit{D}}_{{\mathit{u}}^{\prime }}^{\mathit{a}})}^4$,	${\mathit{A}}_{\mathbf{pd}}$,	${\mathit{A}}_{\mathbf{disl}}$,	${\mathit{l}}_{\mathit{g}}$,	${\mathit{t}}_{\mathbf{gg}}$,	f,	${\mathit{l}}_{\mathit{C}}$,
	${10}^{21}$ cm${}^{-3}$ eV${}^4$	${10}^{-3}$ s${}^{-1}$K${}^{-4}$	${10}^6$ s${}^{-1}$K${}^{-1}$	$\mathsf{\mu }$m	nm	%	mm
${}^{\mathrm{nat}}$C	6.4 (2.5)	7.8 (0.3)	2.2 (0.1)	25 (3)	1.7 (0.2)	5.3 (0.6)	0.91
${}^{12}$C	17 (3)	2.3 (0.1)	1.1 (0.1)	31 (2)	1.9 (0.2)	9.8 (0.8)	0.75

. We have found from calculations of $\kappa (T)$ that the phonon scattering by the external boundaries with these values of $l_C$ contributes negligible to the conductivity values for our polycrystalline samples in the temperature range under consideration.

3.2.2. Point Defects and ${}^{13}$C Isotopes

For low-frequency phonons with a linear dispersion law, the scattering rate by point defects has the Rayleigh frequency dependence [28]:

$${\tau }_{\mathrm{pd}}^{-1}(\omega )={\tilde{A}}_{\mathrm{pd}}{\omega }^4=A_{\mathrm{pd}}{x}^4{T}^4,$$

(9)

here the factor $A_{\mathrm{pd}}$ is determined by the concentration and properties of the impurity. In the case of an isotopic impurity in long-wave approximation [29]:

$${\tilde{A}}_{\mathrm{pd}}={\tilde{A}}_{\mathrm{iso}}=g_2\frac{V_0}{4\pi v^3},$$

(10)

where $V_0$ is the atomic volume (5.6736 Å${}^3$ here), and the mass variance parameter $g_2$ is given by:

$$g_2=\sum _i{c}_i{\left(\Delta M_i/M\right)}^2.$$

(11)

Here $c_i$ is the relative concentration of the i-th isotope, the mass of which $M_i$ differs from the average mass $M={\sum }_i{c}_i{M}_i$ by $\Delta M_i=M_i-M$. For the ${}^{\mathrm{nat}}$C diamond, the parameter $g_2^{(\mathrm{nat})}=7.39\times {10}^{-5}$, and $A_{\mathrm{iso}}^{(\mathrm{nat})}=4.109\times {10}^{-3}$ s${}^{-1}$K${}^{-4}$ (we used the value of Debye velocity $v_D=13.36\times {10}^5$ cm s${}^{-1}$ for the mean phonon velocity in our calculations). For the ${}^{12}$C sample: $g_2^{(12)}=2.8\times {10}^{-6}$, i.e., 26 times less than $g_2^{(\mathrm{nat})}$, and $A_{\mathrm{iso}}^{(12)}=0.153\times {10}^{-3}$ s${}^{-1}$K${}^{-4}$. More accurate calculations of the isotope scattering in the coherent potential approximation, carried out in [7] for diamond, showed that Equations (9) and (10) give a fairly accurate scattering rate at temperatures below 410 K.

3.2.3. Dislocations

To quantify the effect of phonon-dislocation interactions on thermal conductivity we used the phonon scattering from the static strain field surrounding dislocations derived by Carruthers [28]. The rate of this scattering from an array of independent randomly oriented edge and screw dislocations is given approximately by:

$${\tau }_{\mathrm{disl}}^{-1}(\omega )={\tilde{A}}_{\mathrm{disl}}\omega =A_{\mathrm{disl}}xT,$$

(12)

where

$${\tilde{A}}_{\mathrm{disl}}={\rho }_d{b}^2{\gamma }^2.$$

(13)

Here, ${\rho }_d$ is the density of dislocations per unit area, b is the Burgers vector of the dislocation, and $\gamma$ is the Grüneisen constant. Equations (12) and (13) give semiquantitative prediction for dislocation scattering (see, e.g., [30,31,32]).

3.2.4. Charge Carriers Bound to Dopant Centers

Recently, Inyushkin et al. [33] evidenced a strong effect of the phonon interaction with charge carriers bound to the dopant centers on the low temperature thermal conductivity of single crystal diamonds with a low concentration of nitrogen atoms as low as a few ppm. Such scattering by neutral donors in the diamond was discussed briefly by Graebner [34]. In the case of acceptors, Suzuki and Mikoshiba [35,36] have derived analytical expressions for scattering rates of longitudinal and transverse phonons. In this work we used the expression similar to those presented in [37,38] for the elastic (resonant) phonon scattering by holes:

$${\tau }_{\mathrm{ac}}^{-1}(\omega )=N_{\mathrm{ac}}{(D_{u^{\prime }}^a)}^4{\omega }^{4}G(\omega ),$$

(14)

where $N_{\mathrm{ac}}$ is the concentration of neutral acceptors, $D_{u^{\prime }}^a$ is the deformation potentials for $T_2$-type lattice distortion, and $G(\omega )$ is a rather complex function of the phonon frequency, which depends on the effective radius of the acceptor center and splitting of the ground state levels of the bound holes. The rate ${\tau }_{\mathrm{ac}}^{-1}\sim {\omega }^4$ for low enough $\omega$, and can be orders of magnitude stronger than the mass-difference Rayleigh scattering from the dopant. The scattering processes due to phonon interactions with neutral acceptor and donor centers produce nearly the same effect on the $\kappa (T)$ if the centers have nearly the same values of effective radii and energy splitting. The scattering rate cuts off sharply at high frequencies. In diamonds with nitrogen impurities, this scattering disappears at temperatures above 190 K [33].

3.2.5. Grain Boundaries and Intergrain Regions

It has been shown [25] that the thermal conductivity of polycrystalline CVD diamond at low temperatures is largely determined by phonon scattering by intergrain regions. According to Klemens’ theory [39], the intergrain region with a thickness $t_{\mathrm{gg}}$, inside which the sound velocity is much lower than in grains (crystallites), i.e., relative change in velocity $\Delta v/v$∼1, can strongly scatter thermal phonons. The rate of such scattering ${\tau }_{\mathrm{gg}}^{-1}$ has the form:

$${\tau }_{\mathrm{gg}}^{-1}=\frac{4}{3}\frac{v}{d_g}{\left(\frac{\Delta v}{v}\right)}^2\left(1-cos\frac{2\omega }{{\omega }_c}\right),$$

(15)

where $d_g$ is the characteristic crystallite size, and the crossover frequency is ${\omega }_c=v/t_{\mathrm{gg}}$. At low temperatures, when the frequency of thermal phonons is $\omega \ll {\omega }_c$, the rate ${\tau }_{\mathrm{gg}}^{-1}$ is proportional to $t_{\mathrm{gg}}^2$ and ${\omega }^2$. At high temperatures ($\omega \gg {\omega }_c$), the scattering rate does not depend on $t_{\mathrm{gg}}$ and $\omega$, and is determined by the square of $\Delta v/v$. Since the thicknesses of the intergrain regions in the sample can be different, then in the first approximation we take this circumstance into account, assuming the presence of two types of regions: “thick”, the relative concentration of which is f, and “normal” with the concentration $1-f$. For thick regions, the crossover frequency is very low, and they scatter thermal phonons regardless of their frequency over the entire temperature range studied. Normal contacts scatter phonons according to the Equation (15). Then the scattering rate of phonons by intergrain regions can be represented as:

$${\tau }_{\mathrm{gg}}^{-1}=\frac{v}{l_g}\times \left\{\begin{array}{cc}f+(1-f)\frac{1}{2}\left(1-cos\frac{2\omega }{{\omega }_c}\right),\hfill & \frac{\omega }{{\omega }_c}<\frac{\pi }{2},\hfill \\ 1,\hfill & \frac{\omega }{{\omega }_c}⩾\frac{\pi }{2},\hfill \end{array}\right.$$

(16)

where $l_g$ is the phonon free path due to scattering:

$$l_g=\frac{3}{8}{d}_g{\left(\frac{v}{\Delta v}\right)}^2.$$

(17)

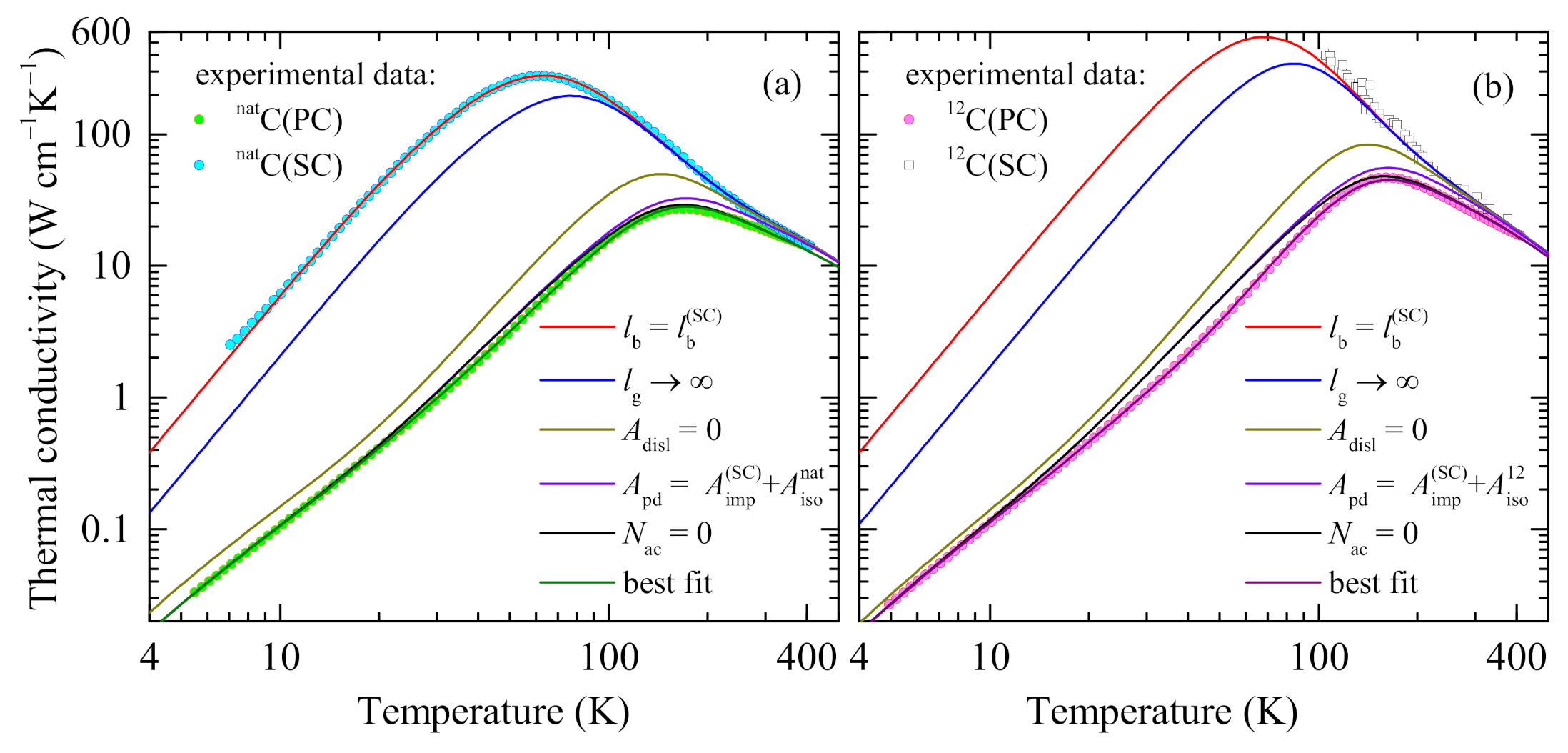
3.3. Fitting Results

The values of the model parameters were determined by fitting the theoretical $\kappa (T)$, calculated by Equation (1), to the experimental data. The fit was performed using the least squares method, with $A_{\mathrm{pd}}$, $A_{\mathrm{d}}$, $N_{\mathrm{ac}}{(D_{u^{\prime }}^a)}^4$, $t_{\mathrm{gg}}$, $l_g$, and f being free parameters. The parameter values are listed in Table 1.

The calculated dependencies $\kappa (T)$ are shown by solid lines in Figure 1 and Figure 2 for the range of relatively high temperatures. Evidently, the theoretical model approximates well the experimental data for both ${}^{\mathrm{nat}}$C and ${}^{12}$C samples over the entire temperature range. The calculated relative difference $\Delta \kappa /\kappa$ is in satisfactory agreement with experimental data as seen in Figure 1, the solid red line in the inset.

4. Discussion

4.1. Scattering by Point Defects and Holes Bound to Acceptors

From Table 1, it can be deduced that for the ${}^{\mathrm{nat}}$C sample, the $A_{\mathrm{pd}}$ parameter is about twice as large as the theoretical value $A_{\mathrm{iso}}^{(\mathrm{nat})}$, i.e., nonisotopic defects make almost the same contribution to the phonon scattering as isotopes do. In the ${}^{12}$C sample, the scattering rate by point defects is ≈15 times higher than that by the residual impurity ${}^{13}$C atoms. Notable also is that $A_{\mathrm{pd}}$ for the ${}^{12}$C sample is less than $A_{\mathrm{iso}}^{(\mathrm{nat})}$, which indicates that isotope scattering is reduced substantially in this isotopically purified sample. Representing $A_{\mathrm{pd}}$ as a sum $A_{\mathrm{imp}}+A_{\mathrm{iso}}$, where $A_{\mathrm{imp}}$ is the contribution of nonisotopic point defects, and calculating the thermal conductivity of polycrystal ${}^{\mathrm{nat}}$C with $A_{\mathrm{imp}}=0$ we obtain $\kappa (298\mathrm{K})=21.9$ W cm${}^{-1}$ K${}^{-1}$, which is 18% higher than the observed one (18.6 W cm${}^{-1}$ K${}^{-1}$). This result indicates that the point defects limit substantially the thermal conductivity of our CVD diamond as compared to the highest quality poly- and single-crystalline diamonds of a natural isotopic composition. Assuming that the dominant defects are single nitrogen atoms in substitutional positions, we can estimate their concentration $[{\mathrm{N}}_s^0]$ from Equations (9)–(11) using the following expression [40]:

$${\tilde{A}}_{\mathrm{imp}}={\tilde{A}}_{\mathrm{pd}}-{\tilde{A}}_{\mathrm{iso}}=\frac{V_0}{4\pi v^3}c{\left(\frac{\Delta M}{M_{\mathrm{C}}}+2\gamma \beta \right)}^2.$$

(18)

Here c is the concentration of impurity ($[{\mathrm{N}}_s^0]=c$ in this case), $\Delta M=M_{\mathrm{N}}-M_{\mathrm{C}}$ is the atomic mass difference of nitrogen and carbon, $\beta$ is the relative difference in atomic volumes between nitrogen and carbon in the diamond lattice, and $\gamma$ is the Grüneisen constant ($\gamma =1.1$ for diamond [41]). Lang and Pang [42] have found experimentally that $\beta \approx 0.35$. From Equation (18) and the values of $A_{\mathrm{pd}}$ presented in Table 1, we obtain that $[{\mathrm{N}}_s^0]\approx 75$ ppm and ≈ 43 ppm for the samples ${}^{\mathrm{nat}}$C and ${}^{12}$C, respectively. These concentrations are more than an order of magnitude higher than the nitrogen content in the samples determined with optical absorption spectroscopy in the UV region. Note also that the parameter $A_{\mathrm{imp}}$ for the ${}^{12}$C sample is about 1.7 times less than that for the ${}^{\mathrm{nat}}$C sample, and hence the nitrogen concentration is to be correspondingly lower. The optical spectroscopy data show an inverse. These results suggests that nitrogen impurity is not the main source of thermal resistance in the samples under investigation.

As known, the crystal lattice vacancies are very effective phonon scatterers among point defects. Within perturbation theory Ratsifaritana and Klemens [43] have shown that the expression in parentheses of Equation (18) is $-3$ in the case of single vacancies. Katcho et al. [44] have found from the first-principal study of vacancy defect in diamonds that this number is $-6$. Therefore, the scattering rate from one vacancy is 41 times as large as the rate from one single substitutional nitrogen atom in diamond lattice. Assuming that the extra point defect scattering is due solely to vacancy scattering and using the later result of Katcho et al., the vacancy concentration is estimated to be approximately 1.8 ppm and 1.1 ppm for ${}^{\mathrm{nat}}$C and ${}^{12}$C samples, respectively. These values agree by order of magnitude with the experimental data on positron annihilation [45,46] for synthetic diamonds. Thus, the scattering of phonons by vacancies can indeed dominate over other processes of scattering by point defects in pure polycrystalline CVD diamonds. This conclusion is in agreement with results of several works [25,47,48]. For instance, Wörner et al. [48] have estimated a vacancy concentration of ≈4 ppm for pure (the nitrogen content below 2.3 ppm) polycrystalline microwave plasma CVD diamond from a magnitude of a point-defect scattering rate. We evaluated the phonon scattering rate by point defects for this sample using our theoretical model, and obtained $A_{\mathrm{pd}}\approx 7.3\times {10}^{-3}$ s${}^{-1}$K${}^{-4}$. From this the concentration of vacancies is estimated to be 1.6 ppm, which is much closer to our ${}^{\mathrm{nat}}$C sample.

The phonon scattering rate from holes bound to acceptors, which is characterized by $N_{\mathrm{ac}}{(D_{u^{\prime }}^a)}^4$ in our model, is about 3 times higher in the ${}^{12}$C sample than in ${}^{\mathrm{nat}}$C one. This rate correlates directly with the nitrogen concentration in the samples. We assume that these acceptor centers relate to nitrogen impurity defects in our samples.

4.2. Scattering by Grain Boundaries and Intergrain Regions

From the values of $l_g$ listed in Table 1 the mean diameters of grains can be estimated from Equation (17) as $\lesssim 65$ and $\lesssim 82$ $\mathsf{\mu }$m for the ${}^{\mathrm{nat}}$C and ${}^{12}$C samples, respectively ($\Delta v/v$ was taken equal to 1). These values are close to those estimated from visual observation of the samples. The larger value for the ${}^{12}$C sample is in accord with expectations because the substantially thicker layer of material of the virgin diamond plate has been removed from the nucleation side during sample preparation. The thickness of the intergrain regions $t_{\mathrm{gg}}$, which is less than 2 nm, is approximately the same in both samples. These regions scatter diffusely the high frequency phonons, but become “transparent” for low frequency phonons with a wavelength much larger than the regions thickness [25]. There are, however, relatively thick regions (with the fraction f) in the samples, which scatter diffusely all phonons within the temperature interval under investigations. As seen from Table 1, the parameter f for the ${}^{12}$C sample is almost twice as that for the ${}^{\mathrm{nat}}$C sample. We attribute this difference to some variations of growth parameters of the ${}^{12}$C plate from the optimal one.

4.3. Dislocation Scattering

As to the dislocation scattering, its parameter $A_{\mathrm{disl}}$, obtained from the best fit, for the ${}^{\mathrm{nat}}$C sample is two times that for the ${}^{12}$C sample. Using Equations (12) and (13) one can estimate the concentration of dislocations from the values of $A_{\mathrm{disl}}$. Taking $\gamma \approx 1.1$ [41] and $b=\sqrt{2}/3a$, $a=3.567$ Å is the diamond lattice constant, and considering that Carruthers’ theory underestimates the rate of dislocation scattering by 4–6 times [28,31], we infer that ${\rho }_d\sim 1\times {10}^{10}$ cm${}^{-2}$ for the ${}^{\mathrm{nat}}$C sample and ∼$5\times {10}^9$ cm${}^{-2}$ for the ${}^{12}$C sample.

It is worthy to note here that Sood et al. [49] have observed strongly suppressed thermal transport near grain boundaries by nearly ∼60% as compared to the peak conductivity inside a grain in polycrystalline CVD diamond with an average grain size of ∼23 $\mathsf{\mu }$m. This reduction in local thermal conductivity is measured up to ∼10 $\mathsf{\mu }$m away from a grain boundary. In addition, recently, Ralchenko et al. [50] have measured the thermal resistivity across a low-angle interface between two single crystalline blocks in a mosaic CVD diamond. The additional thermal resistance was attributed to the strong phonon scattering by point defects and dislocations accumulated in a layer, ∼20 $\mathsf{\mu }$m in thickness next to the interface. These findings might suggest that a layer of ∼10 $\mathsf{\mu }$m in thickness contains a higher concentration of the structural defects (dislocations, vacancies, and their complexes) than an interior of grain in the chemically pure polycrystalline CVD diamond, in accordance with results of Spiteri et al. [51] and Anaya et al. [52]. If this is the case, then the polycrystal with a larger grain size would contain a lesser concentration of point and extended defects as compared with the fine-gain polycrystal under the identical growth conditions. This circumstance can explain why our ${}^{12}$C sample with its larger grain size exhibits lower scattering rates by dislocations and point defects ($A_{\mathrm{imp}}$) than the ${}^{\mathrm{nat}}$C sample.

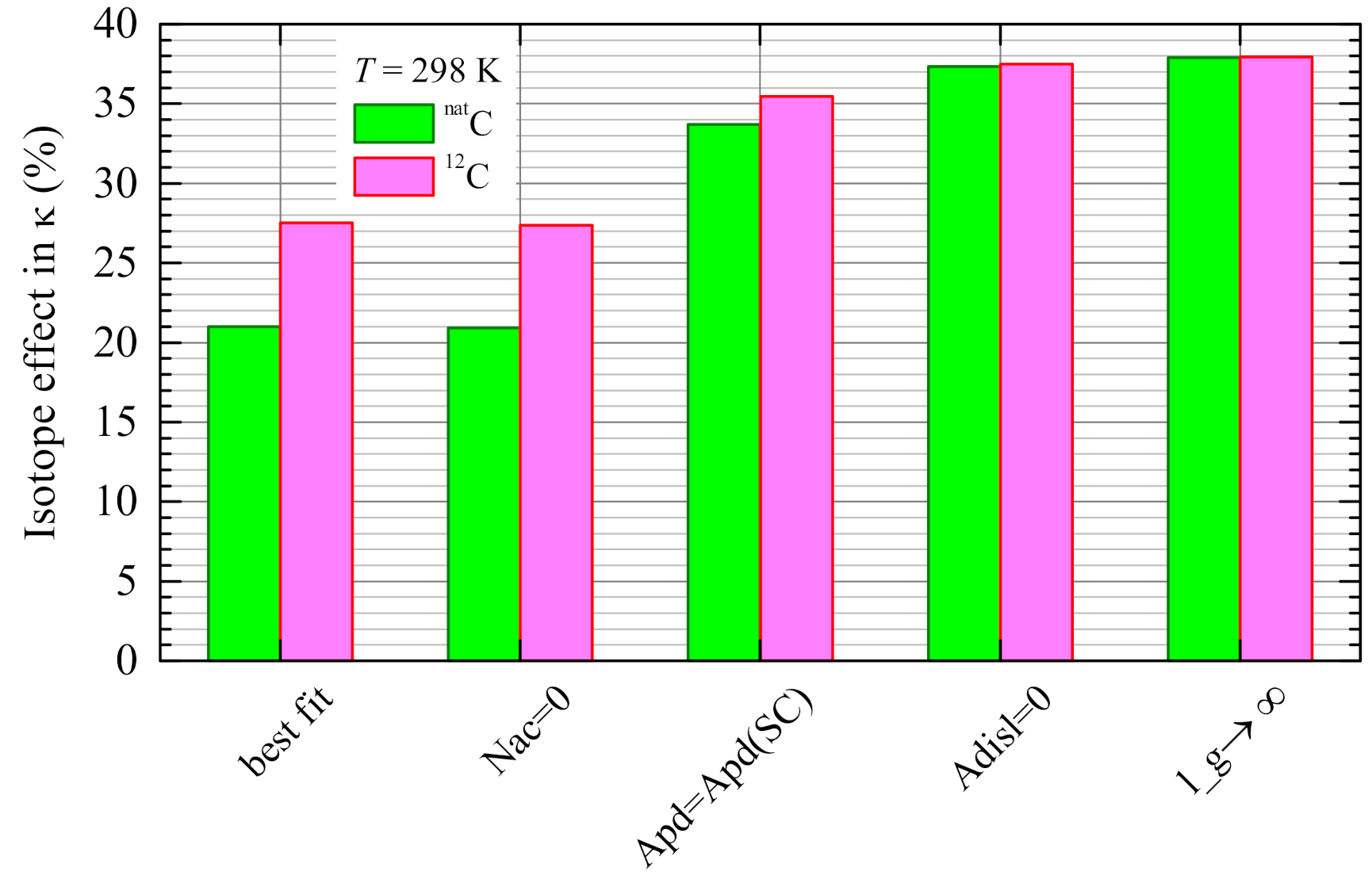
4.4. Contributions of Different Scattering Processes

To get insight into the contribution of different resistive scattering processes to the thermal conductivity of polycrystalline diamond we analyzed the evolution of the calculated $\kappa (T)$, excluding them out of operation, and in these calculations the model parameters values listed in Table 1 were used. The calculated dependencies are presented in Figure 3 for ${}^{\mathrm{nat}}$C, panel (a), and ${}^{12}$C, panel (b). The experimental data for the very pure, nearly nitrogen-free, single crystal ${}^{\mathrm{nat}}$C (no. NE6) [26] and isotopically-enriched single crystal ${}^{12}$C [4] are shown for comparison reasons. The parameters $A_{\mathrm{pd}}^{(\mathrm{SC})}=4.88\times {10}^{-3}$ s${}^{-1}$K${}^{-4}$ and $l_b^{(\mathrm{SC})}=2.62$ mm were obtained for the single crystal ${}^{\mathrm{nat}}$C in [26]. Taking $A_{\mathrm{pd}}^{(\mathrm{SC})}=A_{\mathrm{imp}}^{(\mathrm{SC})}+A_{\mathrm{iso}}^{(\mathrm{nat})}$, we get $A_{\mathrm{imp}}^{(\mathrm{SC})}=0.77\times {10}^{-3}$ s${}^{-1}$K${}^{-4}$, this value is considered as characteristic for scattering from lattice imperfections in high purity synthetic diamond.

Solid lines located from the bottom to the top of the plots represent $\kappa (T)$ of the best fitting to experimental data, and then for the cases with successive turning off the scattering by holes bound to acceptors ($N_{\mathrm{ac}}=0$), reduction of point-defect scattering to the strength found in highly pure single crystal ${}^{\mathrm{nat}}$C ($A_{\mathrm{pd}}=A_{\mathrm{imp}}^{(\mathrm{SC})}+A_{\mathrm{iso}}^{(\mathrm{nat})}$), turning off the scattering by dislocations ($A_{\mathrm{disl}}=0$), excluding scattering by grain boundaries and intergrain regions ($l_g\to \infty$), and setting the phonon free-path in the scattering by external sample boundaries at the value observed for the single crystal ${}^{\mathrm{nat}}$C ($l_b=l_b^{(\mathrm{SC})}$). It is seen from Figure 3a that the phonon scattering from bound charge produces only a minor reduction of $\kappa (T)$ within temperatures from about 20 K to 200 K, being most effective near 40–50 K. Point defects contribute sizably to the resistivity near and above the peak in conductivity. Dislocation scattering reduces essentially the conductivity almost at all temperatures under examination, but above the room temperature it becomes inessential. The scattering by grain boundaries and intergrain regions is the dominant process that determines the $\kappa (T)$ at temperatures below approximately 250 K. The scattering from external sample boundaries becomes essential below ≈120 K. A similar changing of $\kappa (T)$ for the isotopically-enriched material is seen in Figure 3b. In the ${}^{12}$C sample, however, the effect of the phonon scattering from bound charge is stronger due to an apparently higher concentration of acceptors. This scattering is negligible above 200 K as in the case of the ${}^{\mathrm{nat}}$C sample. Both Figure 3a,b demonstrate how $\kappa (T)$ for polycrystalline sample evolves to that for a single crystal.

As the cryogenic applications of diamond still are of limited demand it is interesting, for practical purposes, to reveal the contribution of different scattering processes to the thermal conductivity of polycrystalline diamond at room and higher temperatures. Figure 4 illustrates the variation of the reduced thermal conductivity $\kappa /{\kappa }_0$ for ${}^{\mathrm{nat}}$C and ${}^{12}$C samples with the exclusion and reduction of scattering processes (in the same fashion as described above) at 298 K (a) and 404 K (b). ${\kappa }_0$ is the conductivity for the defect-free single crystal with only isotope and external boundary scattering as extrinsic processes; ${\kappa }_0(298\mathrm{K})=24.20$ W cm${}^{-1}$ K${}^{-1}$ and 35.39 W cm${}^{-1}$ K${}^{-1}$ are obtained for ${}^{\mathrm{nat}}$C and ${}^{12}$C samples, respectively. At 404 K ${\kappa }_0=15.55$ W cm${}^{-1}$ K${}^{-1}$ for ${}^{\mathrm{nat}}$C and $19.56$ W cm${}^{-1}$ K${}^{-1}$ for ${}^{12}$C.

This figures clearly demonstrates that at 298 K, point defects and dislocations are the most important extrinsic scatterers, the grain boundaries and intergrain regions contribute substantially less; the other extrinsic resistive processes are negligible for both samples. At 404 K, the contributions of dislocations and grains are less important yet, among extrinsic processes only scattering by point defects plays an important role. Within the framework of the proposed theoretical model, the scattering of phonons by grains and intergrain regions reduces the parallel thermal conductivity by only about 2%. This 2% is the upper estimate for the thermal conductivity anisotropy of CVD diamonds. This value is much less than experimentally determined in [20], in which, apparently, there is a large systematic error.

4.5. Evaluation of the Isotope Effect

In order to obtain an accurate value of the isotope effect on thermal transport one has to compare magnitudes of thermal conductivity for two samples identical in all characteristics but the isotopic composition. Our samples ${}^{\mathrm{nat}}$C and ${}^{12}$C differ in a number of parameters important for thermal conductivity. Among them are impurity content (nitrogen and hydrogen-related defects, vacancies and their complexes), extended crystal lattice defects, sizes of grains (crystallites), thickness, and elastic properties of the intergrain regions. Until now the growth of entirely identical diamond crystals is a complicated task even if the process parameters are kept nominally the same. For this reason it is useful to compare the results of model calculations to obtain accurate information on the net isotope effect on thermal conductivity. We computed ${\kappa }^{(\mathrm{nat})}(T)$ and ${\kappa }^{(12)}(T)$ with the isotope scattering parameter $A_{\mathrm{iso}}^{(\mathrm{nat})}$ and $A_{\mathrm{iso}}^{(12)}$, respectively, for the set of model parameters that gives the best fit to the measured data for the sample ${}^{\mathrm{nat}}$C. The isotope effect is defined as ${\kappa }^{(12)}(T)/{\kappa }^{(\mathrm{nat})}(T)-1$. Following that the set of parameters was changed cumulatively as it was described above and the isotope effect for each such set was calculated. The data obtained (in percent) are shown in Figure 5.

The “raw” isotope effect determined from the measured thermal conductivity for the ${}^{12}$C and ${}^{\mathrm{nat}}$C samples is shown on this figure (blue circles) for comparison purposes. Additionally shown by the red curve is the “best fit” isotope effect determined from the model best fitting ${\kappa }_{\mathrm{bf}}{(}^{12}\mathrm{C})$ and ${\kappa }_{\mathrm{bf}}{(}^{\mathrm{nat}}\mathrm{C})$ to experimental data for our ${}^{12}$C and ${}^{\mathrm{nat}}$C samples, respectively. The net isotope effect calculated with a full set of parameters of the best fit (the solid green curve for the ${}^{\mathrm{nat}}$C sample is much weaker than the raw effect determined from experimental data. Only at near 400 K do these quantities become close to each other. The turning off the phonon scattering from charges bound to dopants results in very minor changes in the isotope effect (short dotted curves). Therefore, this scattering influences negligible the isotope effect in our case. The reduction of the strength of point defect scattering increases substantially the isotope effect at temperatures above ≈100 K (short dash lines in Figure 5): The isotope effect becomes practically equal to the relative difference of experimental $\kappa (T)$ for our samples at room temperature and above. Excluding dislocation, scattering also yields a substantial increase in the isotope effect at temperatures from about 40 K up to room temperature (dot lines labeled with $A_{\mathrm{disl}}=0$). Next, additional switching off the scattering from the grain boundaries and intergrain regions, which converts our model polycrystalline sample to a single crystal, rises the isotope effect at a lower temperature interval from ∼15 to ∼200 K (dash lines, label $l_g\to \infty$). The crystallinity changes the isotope effect less than by 5% above 200 K. Similar calculations were done using the adjustable parameters of the best fitting to the experimental data for the ${}^{12}$C sample. The results obtained are similar to those presented in Figure 5.

From this consideration it becomes evident that the relatively large difference in the reduced $\kappa (T)$ for our samples originates from the difference in contributions of resistive scattering processes due to point defects, dislocations, grain boundaries, and intergrain regions in our specimens. The relative contributions of these processes change with temperature: At low temperatures (below ∼120 K) the most essential are scattering processes due to dislocations and crystallite structure of samples, the scattering by dislocations and point-defect prevail at temperatures between 120 and 250 K, and point defect scattering dominate above 250 K.

Figure 6 demonstrates the evolution of the net isotope effect at 298 K calculated from fitting parameters for our samples ${}^{\mathrm{nat}}$C (green columns) and ${}^{12}$C (magenta columns) with turning off or decreasing the scattering processes as depicted on the x-axis from left to right.

It is seen that the magnitude of the net isotope effect is from 21 to 28% for our polycrystalline samples. The weakening of point defect scattering to a strength characteristic of the reference single crystal increases the isotope effect to 34–36%, the turning off the dislocation scattering pulls up the effect to 37.5%, and eliminating grain scattering adds only 0.5% to the isotope effect. From this consideration we can conclude that crystal lattice defects, those relate to the vacancies and dislocations, reduce substantially the isotope effect in polycrystalline diamonds as compared with pure single crystals. These defects are inherent for polycrystalline diamonds and accumulated near grain boundaries. The increase of grain sizes will reduce the boundary area and decrease the phonon scattering from defects associated with grain boundaries at room temperature. This in turn will increase the magnitude of the isotope effect around and above room temperature.

5. Conclusions

We experimentally demonstrated that the ${}^{12}$C polycrystalline CVD diamond isotopically enriched to 99.96% at a good quality had a significantly higher parallel thermal conductivity compared to a diamond of natural isotopic composition ${}^{\mathrm{nat}}$C. The measured thermal conductivity of the isotopically purified diamond was 25.1 W cm${}^{-1}$ K${}^{-1}$ at room temperature, exceeding the conductivity of the best single crystalline diamonds of a natural isotopic mixture.

Within the phenomenological model, we found that the scattering by grain boundaries and intergrain regions was the most important process that determined the behavior of $\kappa (T)$ below ≈250 K. The phonon scattering from the static strain field surrounding dislocations reduced substantially the conductivity at temperatures below room temperature. The point defects scattering plays an important role near and above the peak in conductivity. We assumed that vacancies yielded the major contribution to this scattering. The enhancement in room-temperature thermal conductivity upon elimination of the natural abundance of ${}^{13}$C (1.07 at.%) was estimated to be 21–28% at room temperature for polycrystalline CVD diamond plates under investigation. The magnitude of the isotope effect could be increased up to 37% if point defects, other than isotopes, and dislocations were eliminated from the samples crystal lattice.