Statistical Damage Constitutive Model for High-Strength Concrete Based on Dissipation Energy Density
Abstract
:1. Introduction
2. Conventional Triaxial Compression Tests of C60 and C70 High-Strength Concrete
2.1. Test Materials and Equipment
2.2. Analysis of Test Results
3. Energy Analysis of High-Strength Concrete during Compression
3.1. Energy Analysis
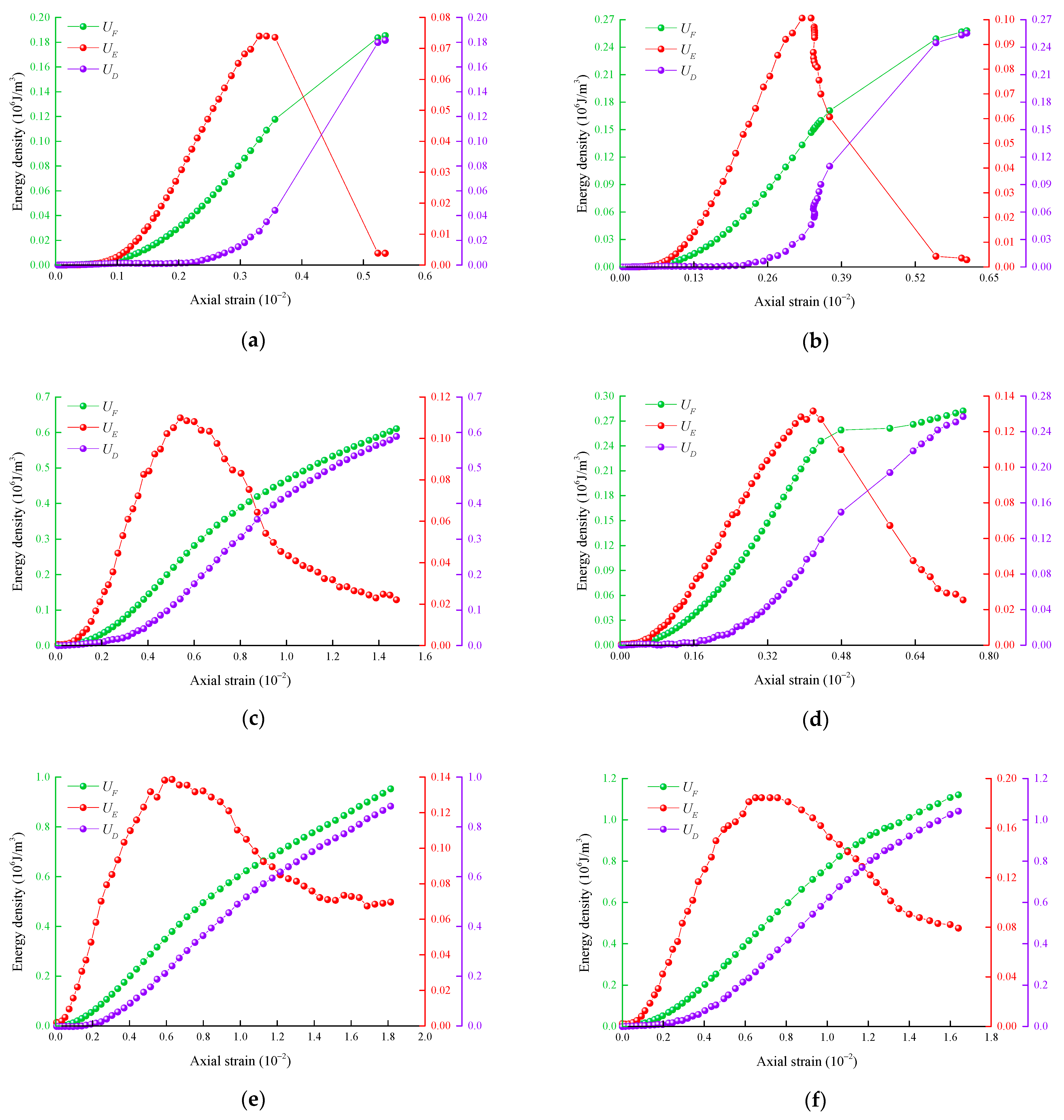
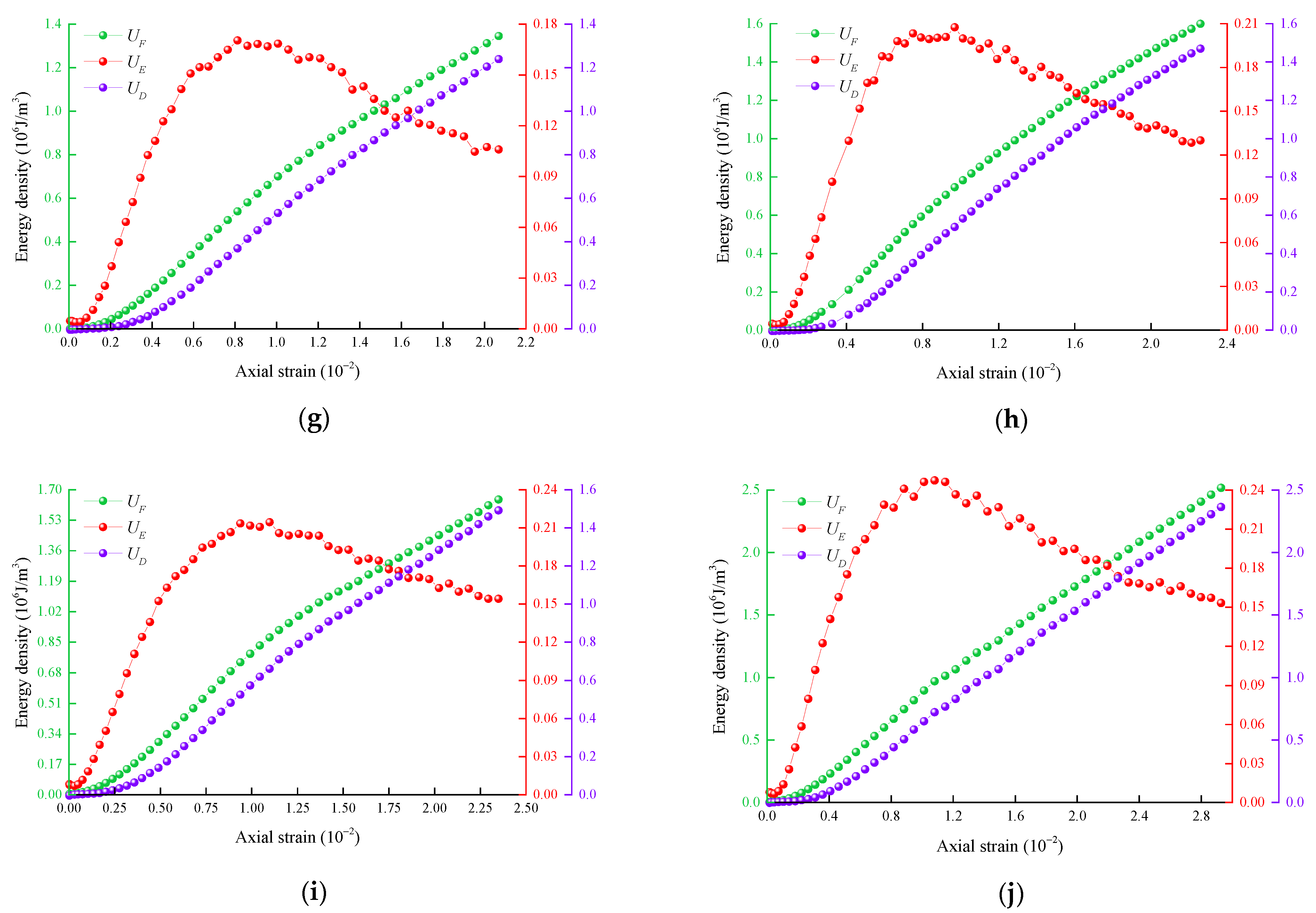
3.2. Relationship between Energy Density and Axial Strain
3.3. Relationship between Energy Density Corresponding to Peak Stress and Confining Pressure
3.4. Relationship between Final Energy Density and Confining Pressure
4. Statistical Damage Constitutive Model of High-Strength Concrete
4.1. Establishment of Constitutive Model
4.2. Verification of Constitutive Model
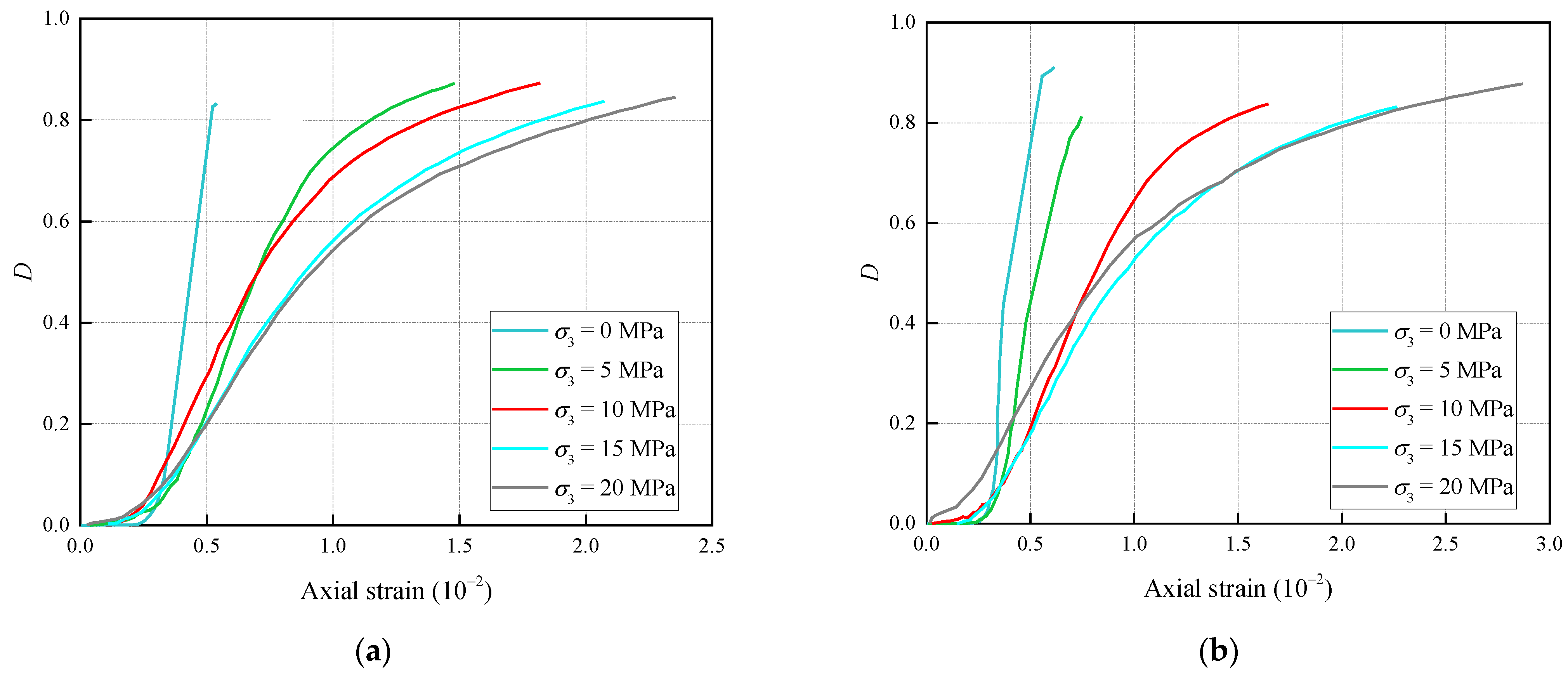
4.3. Damage Analysis of High-Strength Concrete
5. Conclusions
- (1)
- At different confining pressures, the input energy density and dissipation energy density of the C60 and C70 high-strength concrete samples increased as a function of the axial strain, while the elastic strain energy density increased first and then decreased as a function of the axial strain, and reached the maximum value at the peak stress. Before the concrete sample entered the plastic stage, the dissipated energy density increased slowly as a function of the axial strain, and the input energy was basically transformed into elastic-strain energy. After the specimen was destroyed, the elastic-strain energy was released rapidly and the input energy was basically transformed into dissipation energy.
- (2)
- The input, elastic, and the dissipated energy densities corresponding to the peak stresses of the C60 and C70 high-strength concrete increased as a function of the increase of confining pressure, and the elastic-strain energy density corresponding to the peak stress increased linearly as a function of the confining pressure. As the elastic strain energy density of the concrete sample at the moment of failure increased, the energy release at high-confining pressures became more rapid and abrupt compared with those at low-confining pressures.
- (3)
- At both the pre-peak elastic strain energy storage stage and the post-peak elastic strain energy release stage, the statistical damage constitutive model curves of C60 and C70 high-strength concrete at different confining pressures were in good agreement with the experimental curves, and the average relative standard deviations were only 3.64% and 3.99%. Conversely, this constitutive model overcame the defect of low correlation between the concrete constitutive model and the test results in the post-peak stage and improved the accuracy of the model. It was also verified that the statistical damage constitutive model established from the energy theory and statistical damage theory was suitable in describing the constitutive behavior of high-strength concrete.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Li, W.S.; Wu, J.Y. A consistent and efficient localized damage model for concrete. Int. J. Damage Mech. 2018, 27, 541–567. [Google Scholar] [CrossRef]
- Li, R.T.; Li, X.K. A coupled chemo-elastoplastic-damage constitutive model for plain concrete subjected to high temperature. Int. J. Damage Mech. 2010, 19, 971–1000. [Google Scholar]
- Nishiyama, T.; Chen, Y.; Kusuda, H.; Ito, T.; Kita, H. The examination of fracturing process subjected to triaxial compression test in Inada granite. Eng. Geol. 2002, 66, 257–269. [Google Scholar] [CrossRef]
- McSaveney, M.J.; Davies, T.R. Surface energy is not one of the energy losses in rock comminution. Eng. Geol. 2009, 109, 109–113. [Google Scholar] [CrossRef]
- Krajcinovic, D.; Silva, M.A.G. Statistical aspects of the continuous damage theory. Int. J. Solids Struct. 1982, 18, 551–562. [Google Scholar] [CrossRef]
- Yang, X.; Liang, N.H.; Liu, X.R.; Zhong, Z. A study of test and statistical damage constitutive model of multi-size polypropylene fiber concrete under impact load. Int. J. Damage Mech. 2019, 28, 973–989. [Google Scholar] [CrossRef]
- Bai, W.F.; Chen, J.Y.; Fan, S.L.; Lin, G. The statistical damage constitutive model for concrete materials under uniaxial compression. J. Harbin Inst. Technol. (New Ser.) 2010, 17, 338–344. [Google Scholar]
- Liang, H.; Zou, R.H.; Peng, G.; Tian, W.; Chen, X.Q. Rate-dependent constitutive model of concrete based on the statistical theory of Weibull. J. Yangtze River Sci. Res. Inst. 2016, 33, 111–114. [Google Scholar]
- Cervera, M.; Oliver, J.; Manzoli, O. A rate-dependent isotropic damage model for the seismic analysis of concrete dams. Earthq. Eng. Struct. Dyn. 1996, 25, 987–1010. [Google Scholar] [CrossRef]
- Zhou, W.; Feng, P.; Lin, H.W. Constitutive relations of coral aggregate concrete under uniaxial and triaxial compression. Constr. Build. Mater. 2020, 251, 118957. [Google Scholar] [CrossRef]
- Wu, J.Y.; Li, J.; Faria, R. An energy release rate-based plastic-damage model for concrete. Int. J. Solids Struct. 2006, 43, 583–612. [Google Scholar] [CrossRef] [Green Version]
- Zhang, H.M.; Lei, L.N.; Yang, G.S. Characteristic and representative model of rock damage process under constant confining stress. J. China Univ. Min. Technol. 2015, 44, 59–63. [Google Scholar] [CrossRef]
- Golewski, G.L. The benefificial effect of the addition of fly ash on reduction of the size of microcracks in the ITZ of concrete composites under dynamic loading. Energies 2021, 14, 668. [Google Scholar] [CrossRef]
- Chen, W.; Konietzky, H.; Tan, X.; Thomas, F. Pre-failure damage analysis for brittle rocks under triaxial compression. Comput. Geotech. 2016, 74, 45–55. [Google Scholar] [CrossRef]
- You, M.Q.; Hua, A.Z. Energy analysis of failure process of rock specimens. Chin. J. Rock Mech. Eng. 2002, 21, 778–781. [Google Scholar]
- Zhao, Z.H.; Xie, H.P. Energy transfer and energy dissipation in rock deformation and fracture. J. Sichuan Univ. (Eng. Sci. Ed.) 2008, 40, 26–31. [Google Scholar]
- Xie, H.P.; Li, L.Y.; Peng, R.D.; Yang, J. Energy analysis and criteria for structural failure of rocks. J. Rock Mech. Geotech. Eng. 2009, 1, 11–20. [Google Scholar] [CrossRef] [Green Version]
- Zuo, J.P.; Huang, Y.M.; Xiong, G.J.; Liu, J.; Li, M.M. Study of energy-drop coefficient of brittle rock failure. Rock Soil Mech. 2014, 35, 321–327. [Google Scholar]
- Gong, F.Q.; Luo, S.; Li, X.B.; Yan, J. Linear energy storage and dissipation rule of red sandstone materials during the tensile failure process. Chin. J. Rock Mech. Eng. 2018, 37, 352–363. [Google Scholar]
- Lemaitre, J. Evaluation of dissipation and damage in metals submitted to dynamic loading. In Proceedings of the 1st International Conference on Mechanical Behavior of Materials, Kyoto, Japan, 15–20 August 1971; pp. 540–549. [Google Scholar]
- Deng, J.; Gu, D.S. 2011 On a statistical damage constitutive model for rock materials. Comput. Geosci. 2011, 37, 122–128. [Google Scholar] [CrossRef]
- Fu, Q.; Xie, Y.J.; Song, H.; Zhou, H. Model for mechanical properties of cement and asphalt mortar. J. Chin. Ceram. Soc. 2014, 42, 1396–1403. [Google Scholar]
- Wang, Z.L.; Li, Y.C.; Wang, J.G. A damage-softening statistical constitutive model considering rock residual strength. Comput. Geosci. 2007, 33, 1–9. [Google Scholar] [CrossRef]
- Guo, J.Q.; Liu, X.R.; Zhao, Q. Theoretical research on rock unloading mechanical characteristics. Rock Soil Mech. 2017, 38, 123–130. [Google Scholar]







| Strength Grade | Cement (kg/m3) | Admixture (kg/m3) | Cementing Materials (kg/m3) | Sand (kg/m3) | Gravel (kg/m3) | Water (kg/m3) | Sand Rate | Water Cement Ratio |
|---|---|---|---|---|---|---|---|---|
| C60 | 415 | 135 | 550 | 620 | 1105 | 175 | 0.36 | 0.42 |
| C70 | 425 | 145 | 570 | 615 | 1095 | 170 | 0.36 | 0.4 |
| Project | Technical Parameters |
|---|---|
| Maximum axial test force | 2000 kN |
| Test force accuracy | ±1% |
| Test force resolution | 1/180,000 |
| Maximum displacement of piston | 120 mm |
| Displacement accuracy | ±0.5% FS |
| Displacement resolution | 5 μm |
| Deformation measurement accuracy | ±1% |
| Deformation resolution | 1/180,000 |
| Maximum confining pressure | 50 MPa |
| Confining pressure accuracy | ±1% |
| Confining pressure resolution | 1/180,000 |
| Minimum control temperature | −20 °C |
| Temperature control accuracy | ±0.5 °C |
| Elastic Modulus (GPa) | Poisson’s Ratio | |||||
|---|---|---|---|---|---|---|
| C60 | C70 | C60 | C70 | C60 | C70 | |
| 0 | 65.38 | 84.41 | 28 | 31 | 0.31 | 0.29 |
| 5 | 81.96 | 100.22 | 29 | 32 | 0.28 | 0.26 |
| 10 | 98.26 | 115.98 | 31 | 33 | 0.29 | 0.26 |
| 15 | 114.01 | 128.22 | 33 | 35 | 0.28 | 0.28 |
| 20 | 129.12 | 141.67 | 34 | 36 | 0.26 | 0.26 |
| Confining Pressure (MPa) | Weibull Distribution Parameters | R2 | ||||
|---|---|---|---|---|---|---|
| C60 | C70 | C60 | C70 | |||
| m | k | m | k | |||
| 0 | 3.576 | 1.538 | 3.311 | 2.747 | 0.962 | 0.978 |
| 5 | 2.493 | 1.225 | 1.973 | 2.146 | 0.984 | 0.988 |
| 10 | 1.778 | 0.997 | 1.766 | 1.056 | 0.991 | 0.982 |
| 15 | 1.718 | 0.947 | 1.453 | 0.916 | 0.994 | 0.992 |
| 20 | 1.146 | 0.919 | 1.233 | 0.762 | 0.989 | 0.985 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, L.; Cheng, H.; Wang, X.; Liu, J.; Guo, L. Statistical Damage Constitutive Model for High-Strength Concrete Based on Dissipation Energy Density. Crystals 2021, 11, 800. https://doi.org/10.3390/cryst11070800
Zhang L, Cheng H, Wang X, Liu J, Guo L. Statistical Damage Constitutive Model for High-Strength Concrete Based on Dissipation Energy Density. Crystals. 2021; 11(7):800. https://doi.org/10.3390/cryst11070800
Chicago/Turabian StyleZhang, Liangliang, Hua Cheng, Xiaojian Wang, Jimin Liu, and Longhui Guo. 2021. "Statistical Damage Constitutive Model for High-Strength Concrete Based on Dissipation Energy Density" Crystals 11, no. 7: 800. https://doi.org/10.3390/cryst11070800
APA StyleZhang, L., Cheng, H., Wang, X., Liu, J., & Guo, L. (2021). Statistical Damage Constitutive Model for High-Strength Concrete Based on Dissipation Energy Density. Crystals, 11(7), 800. https://doi.org/10.3390/cryst11070800







