Quantifying Computed Tomography of Basalt Fiber-Reinforced Concrete under Unconfined Compression
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Methods
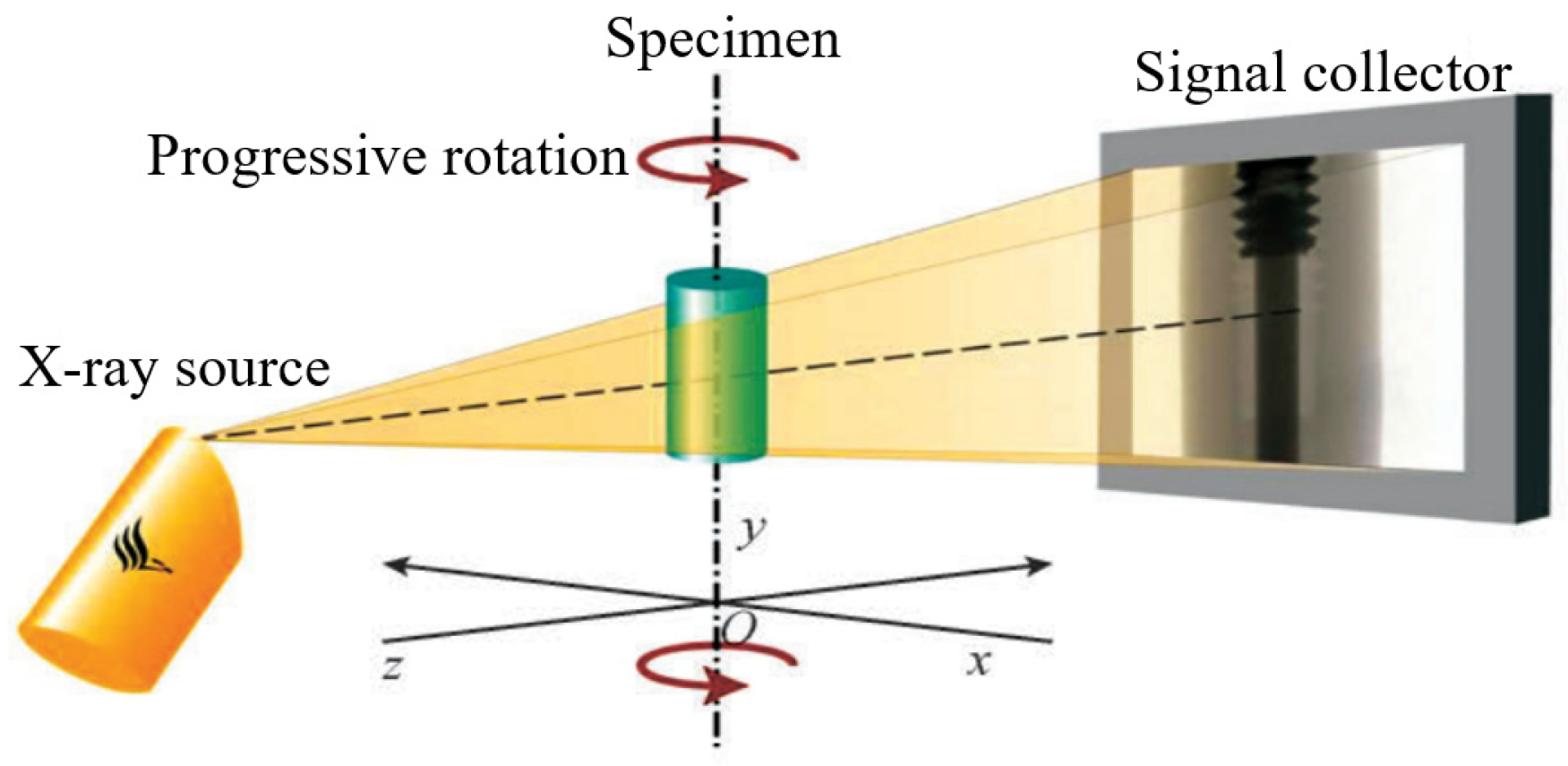
2.2.1. Instrument Introduction
2.2.2. CT Scanning Principle
2.2.3. Gaussian Filter
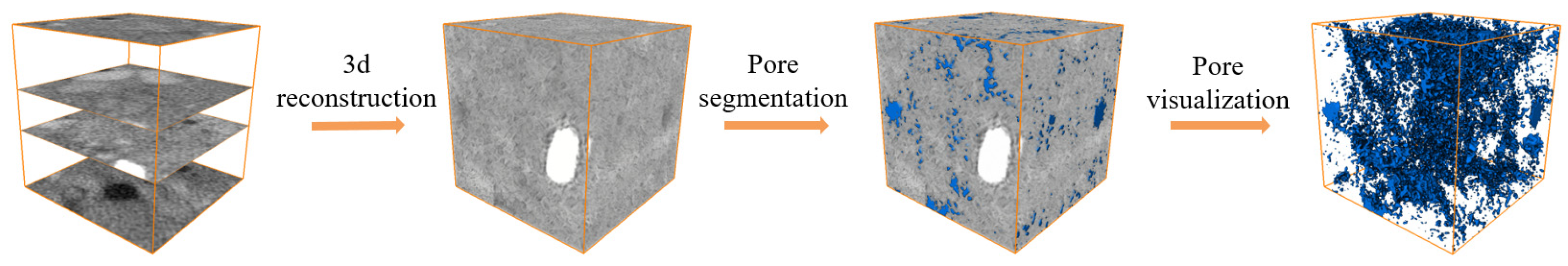
2.2.4. Interactive Segmentation Algorithm
3. Results
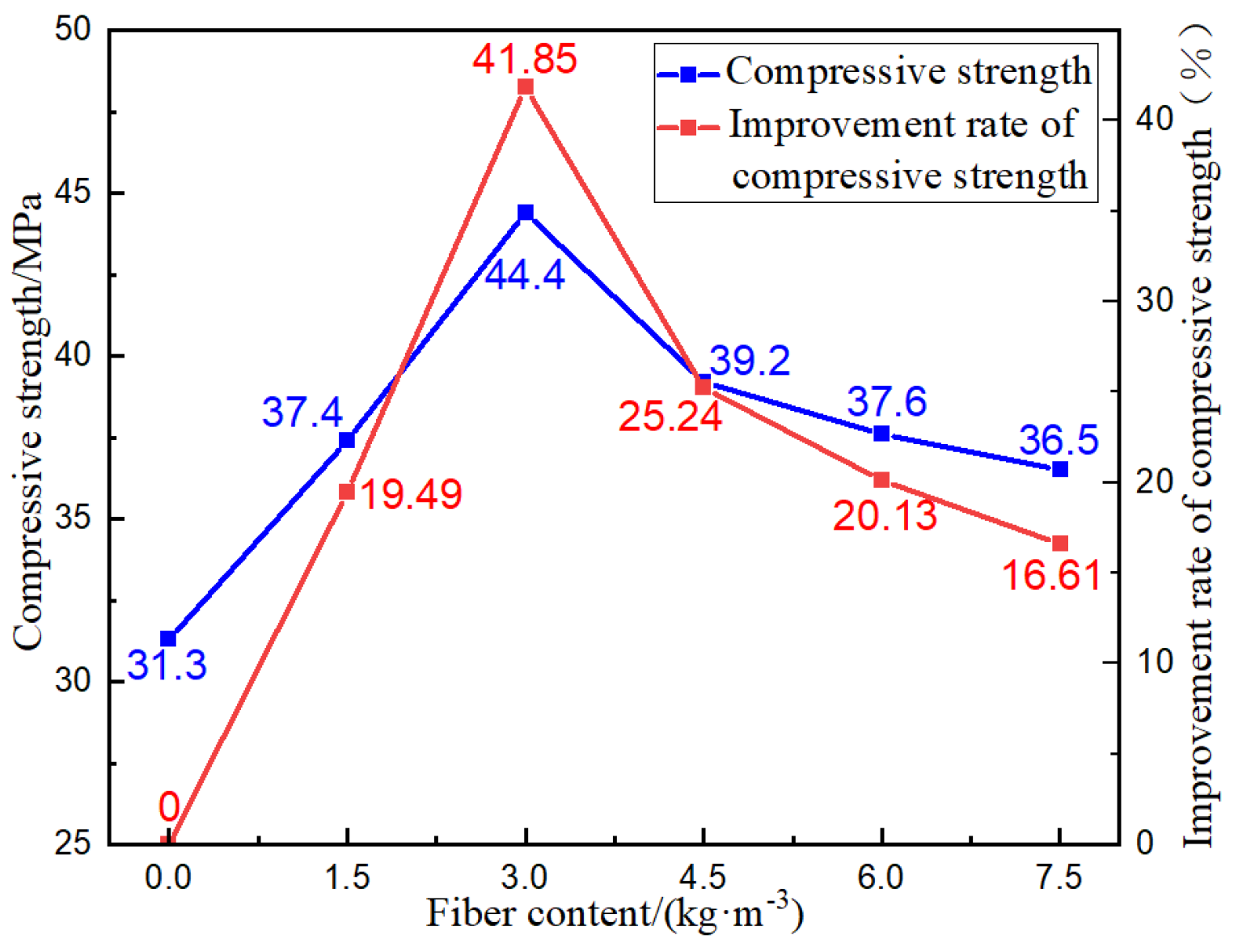
3.1. Unconfined Compression Test
3.2. Equivalent Pore Network Model
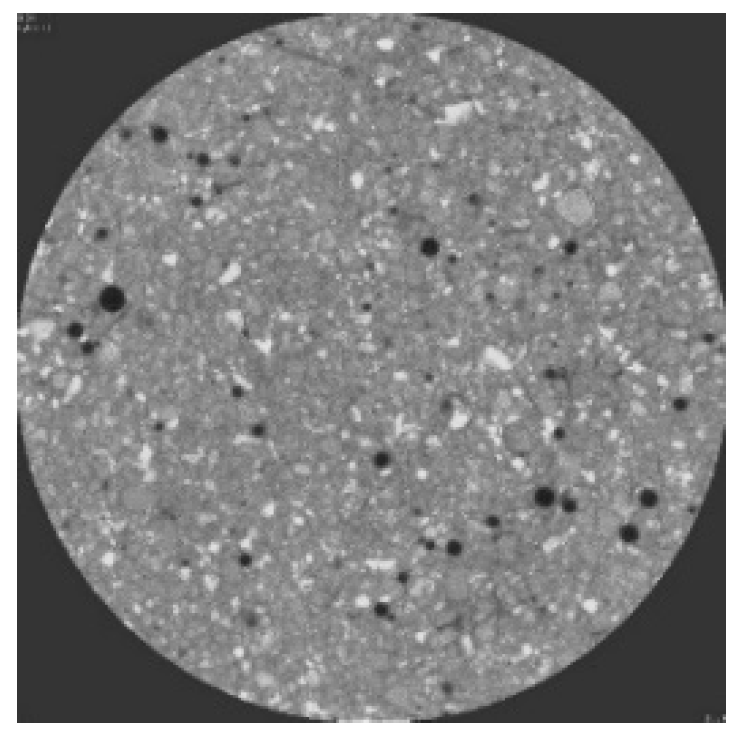
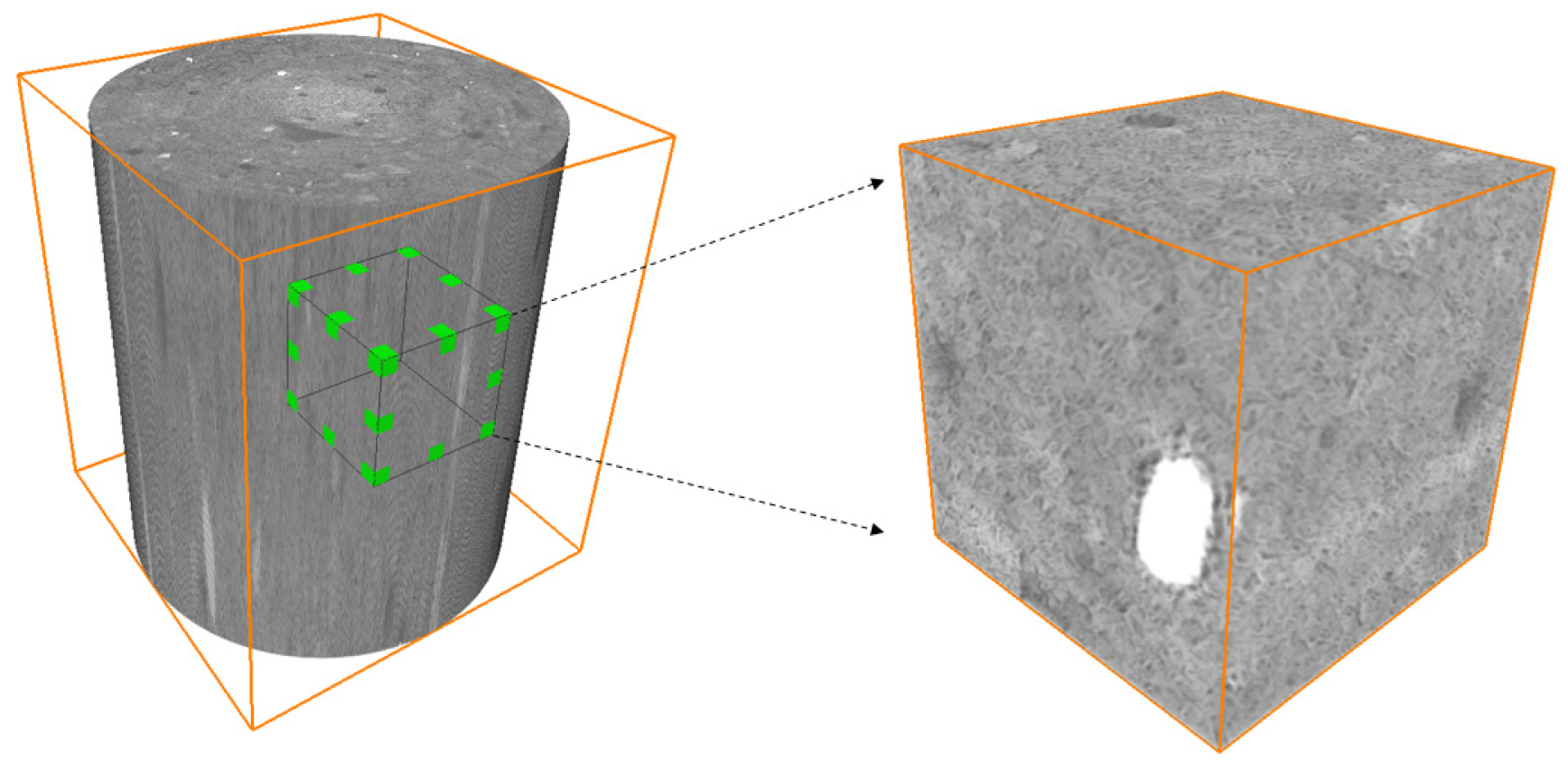
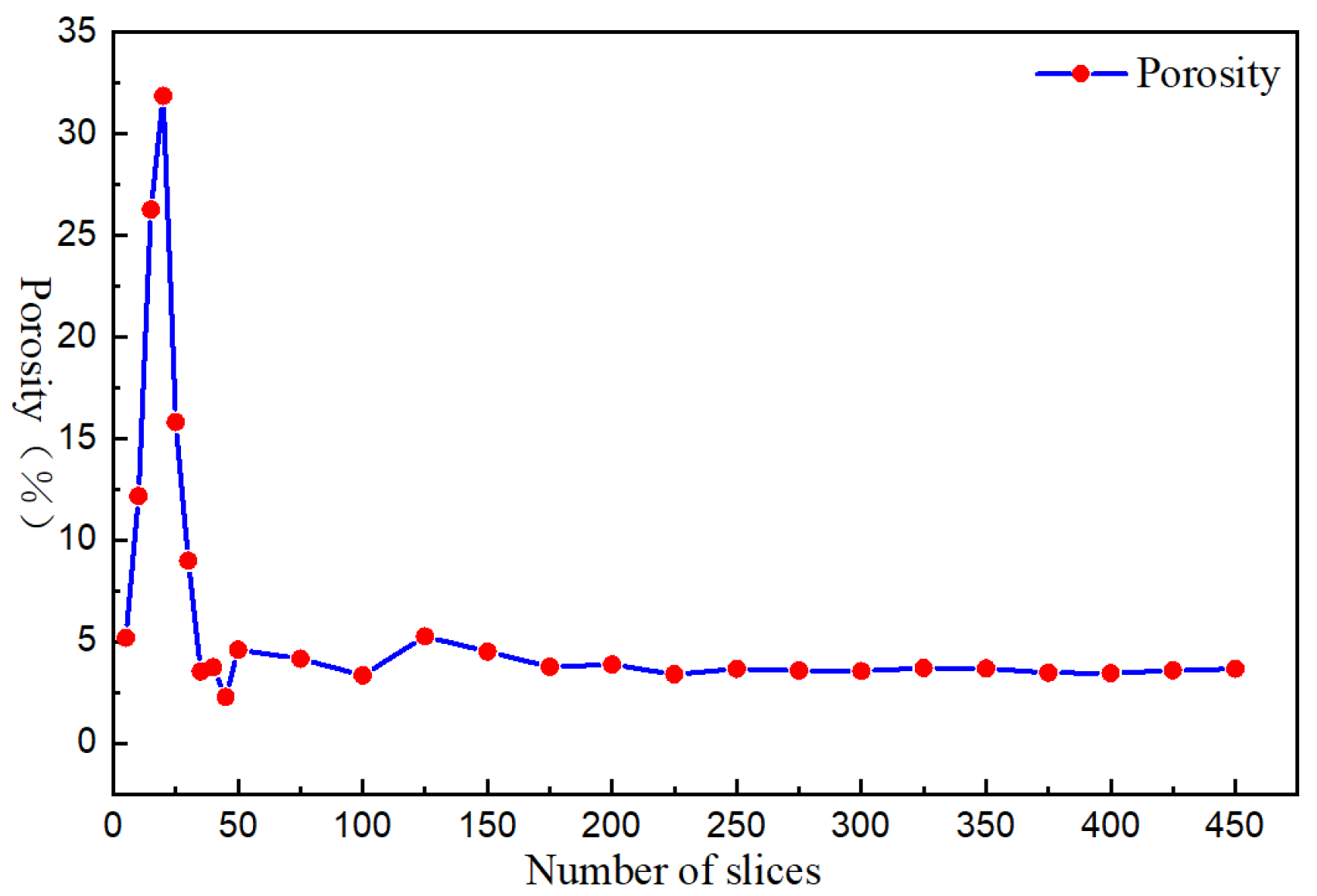
3.2.1. Determination of Characterization Unit
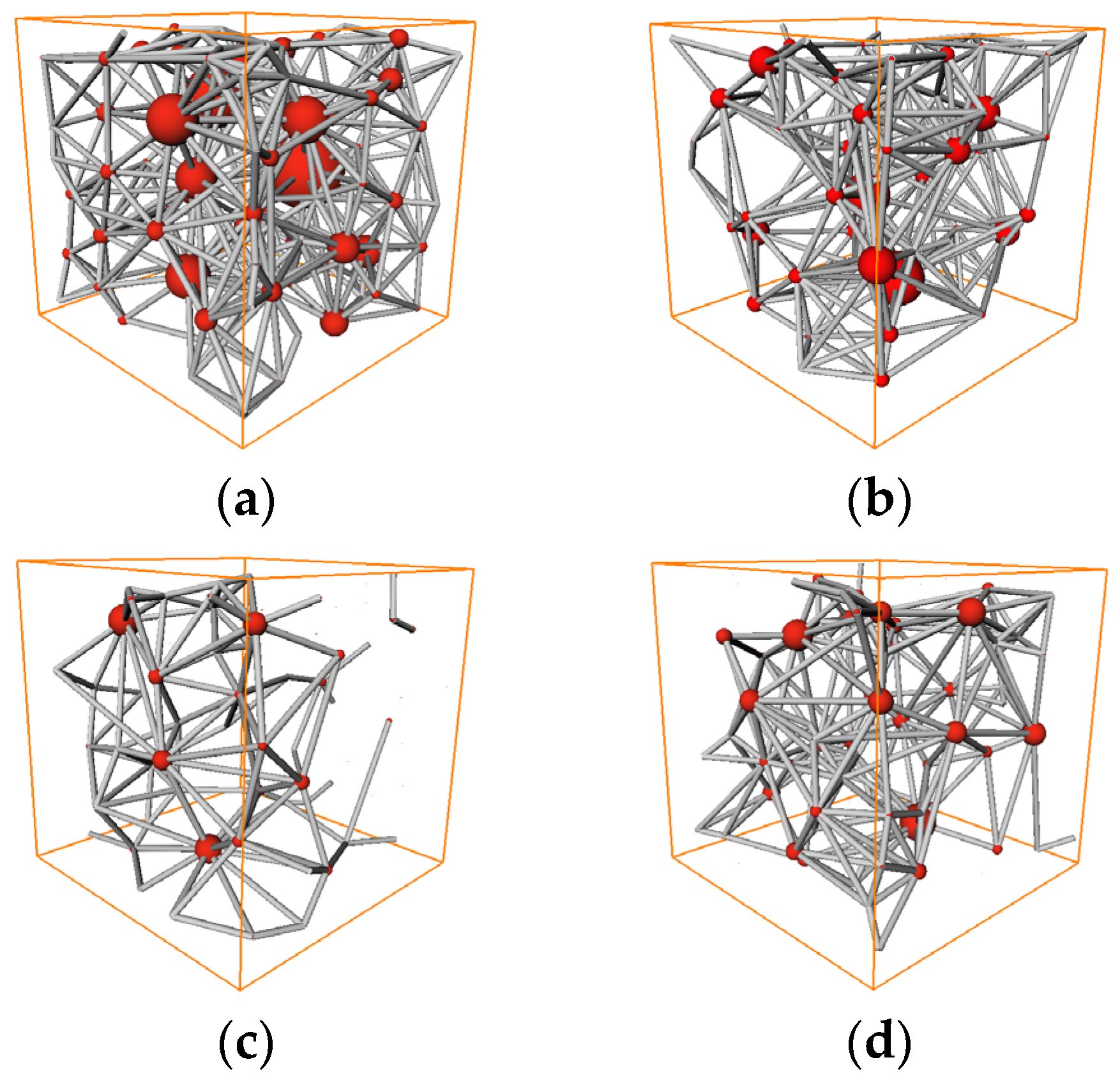
3.2.2. Pore Network Model
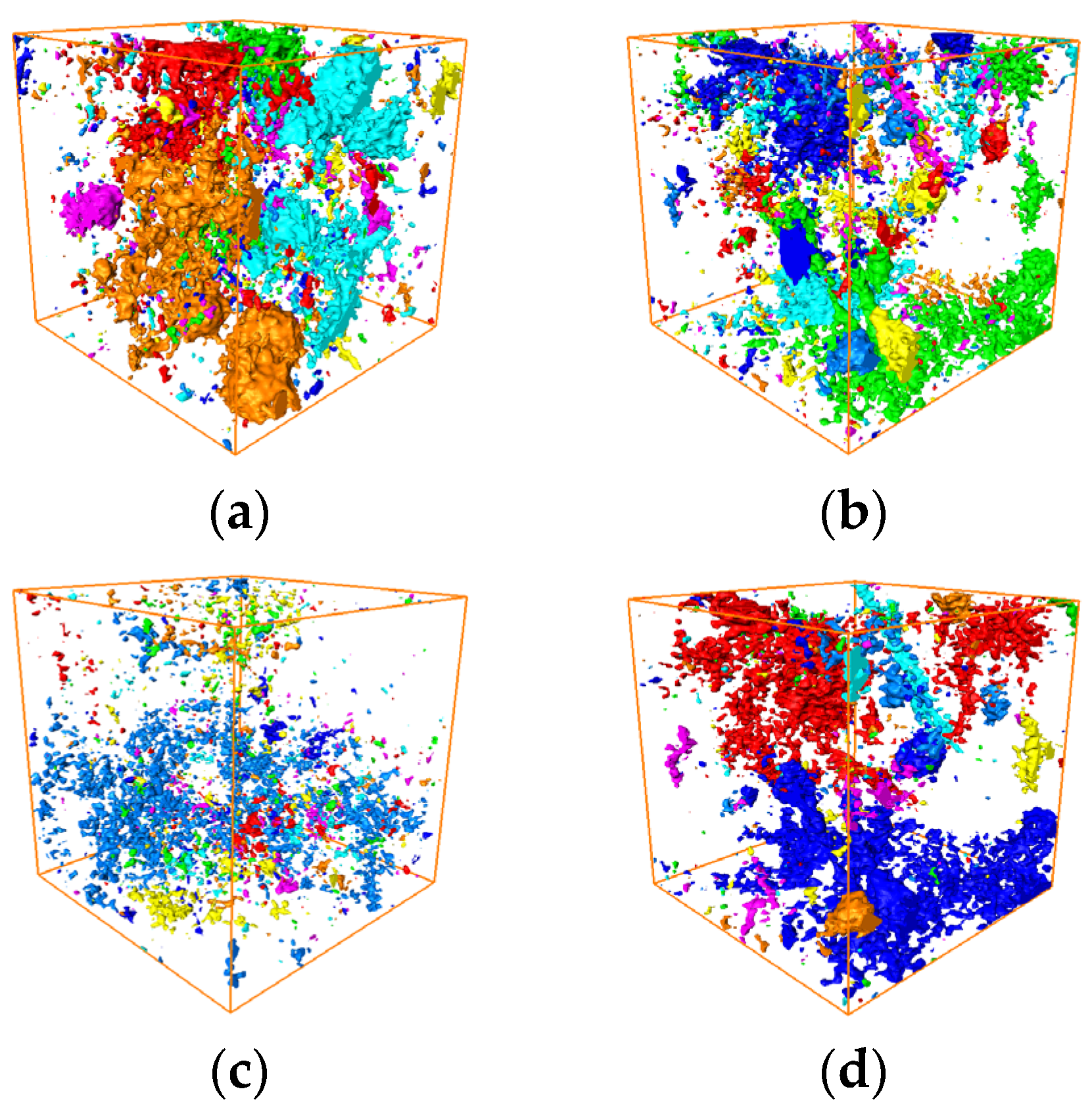
3.2.3. Ball-and-Stick Network Model
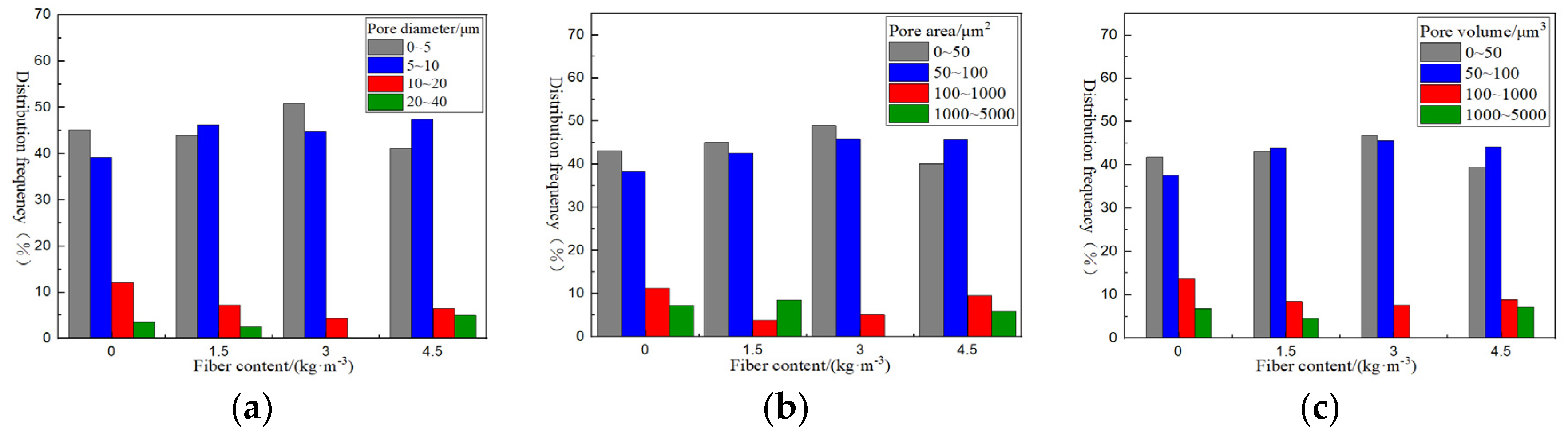
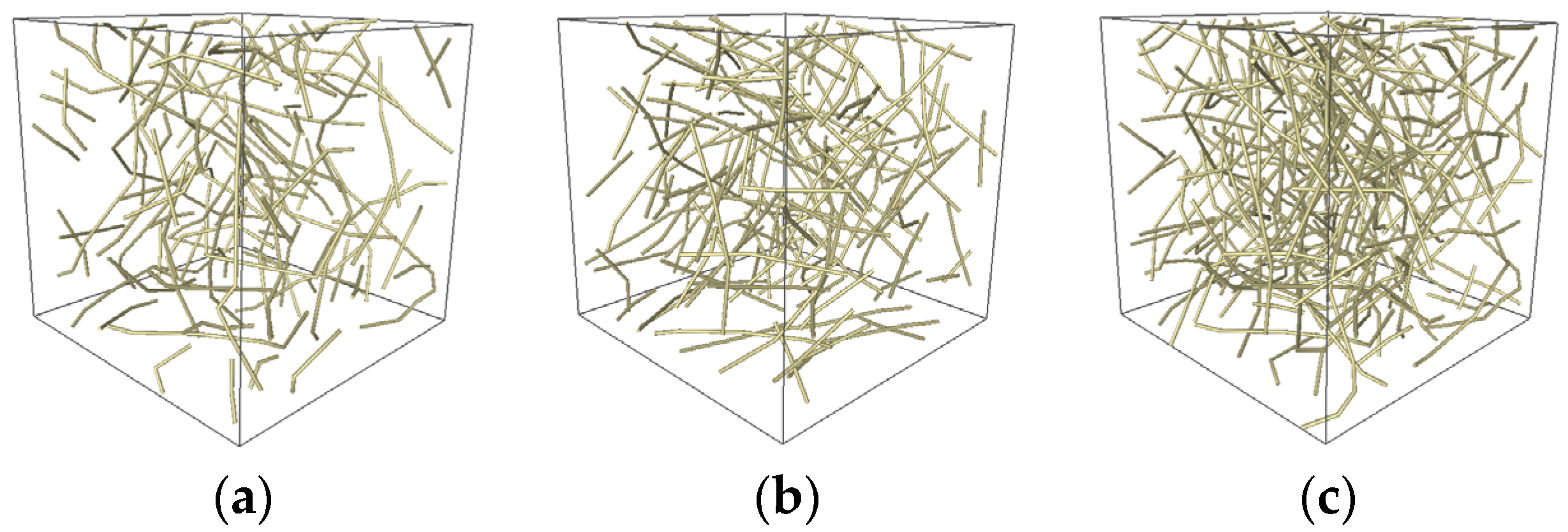
3.3. Fiber Distribution
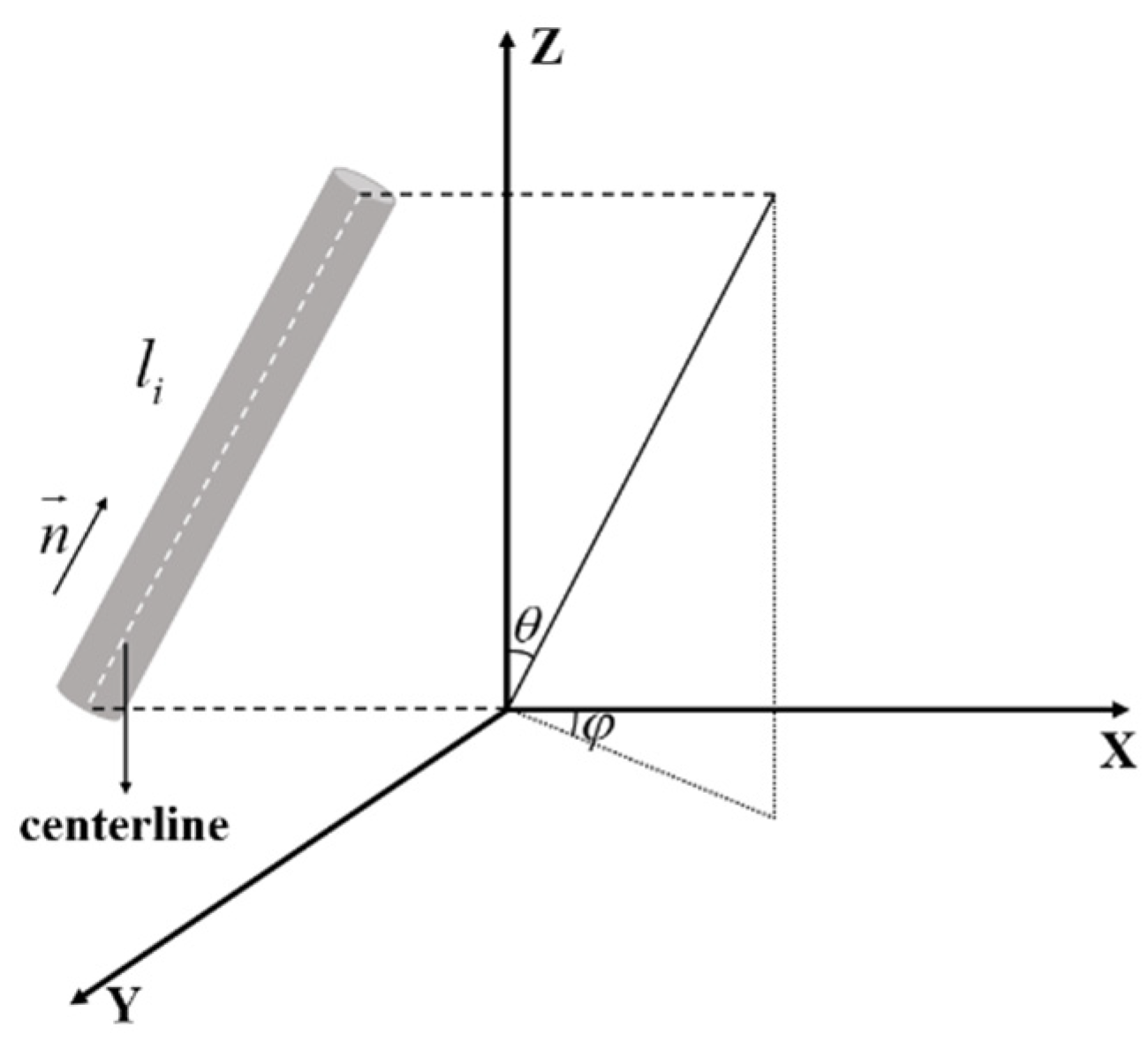
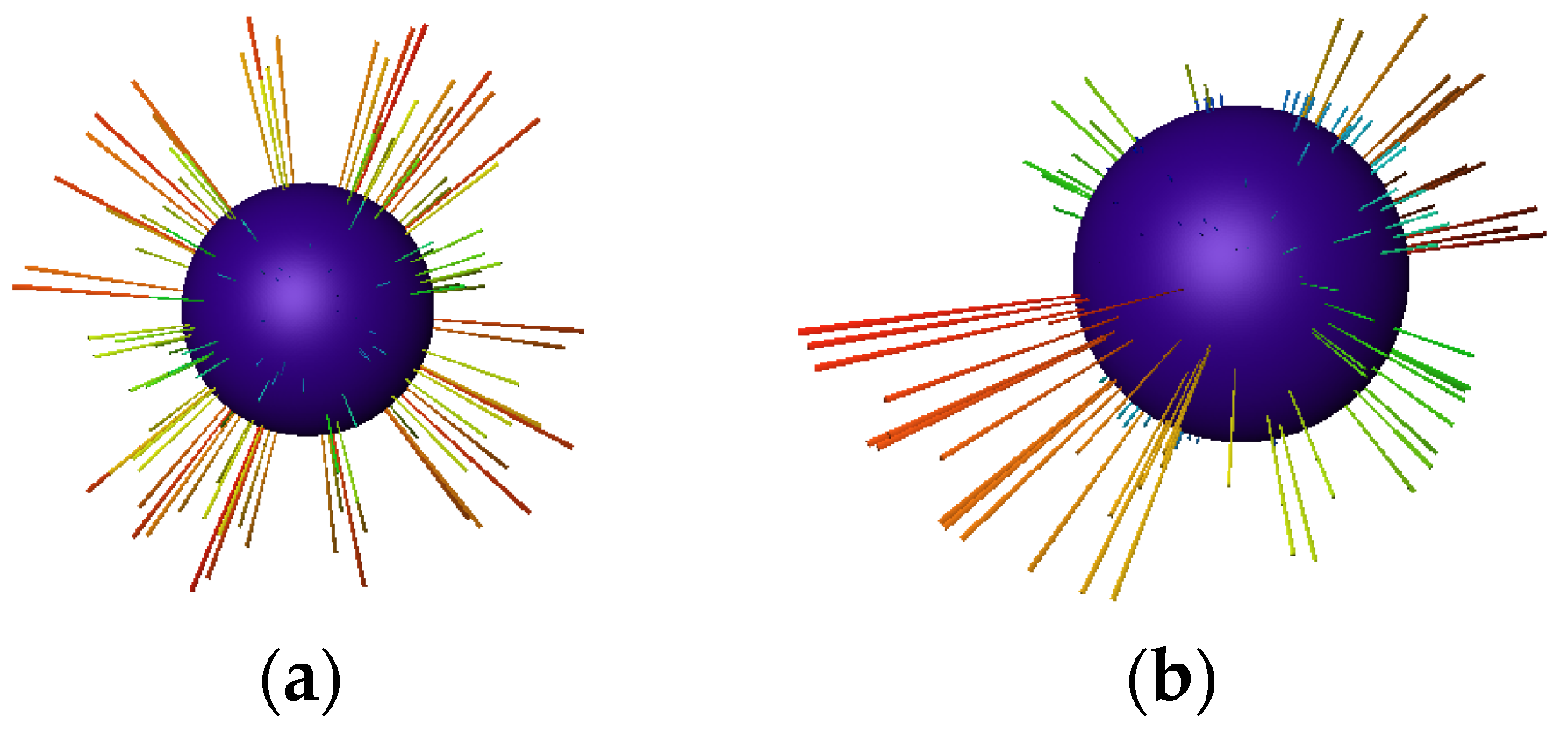
3.3.1. Search Cone Algorithm
3.3.2. Fiber Orientation
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Li, F.Y.; Li, L.Y.; Dang, Y.; Wu, P.F. Study of the Effect of Fibre Orientation on Artificially Directed Steel Fibre-Reinforced Concrete. Adv. Mater. Sci. Eng. 2018, 2018, 8657083. [Google Scholar] [CrossRef] [Green Version]
- Yan, L.B.; Chouw, N. A comparative study of steel reinforced concrete and flax fibre reinforced polymer tube confined coconut fibre reinforced concrete beams. J. Reinf. Plast. Compos. 2013, 32, 1155–1164. [Google Scholar] [CrossRef]
- Yuan, H.Q.; Chen, J.T.; Zhu, J.D. Mechanical Properties of Layered Hybrid Fiber Reinforced Concrete. J. Wuhan Univ. Technol.-Mater. Sci. 2003, 18, 68–70. [Google Scholar] [CrossRef]
- Liu, J.L.; Jia, Y.M.; Wang, J. Experimental Study on Mechanical and Durability Properties of Glass and Polypropylene Fiber Reinforced Concrete. Fibers Polym. 2019, 20, 1900–1908. [Google Scholar] [CrossRef]
- Mastali, M.; Dalvand, A. The impact resistance and mechanical properties of self-compacting concrete reinforced with recycled CFRP pieces. Compos. Part B Eng. 2016, 92, 360–376. [Google Scholar] [CrossRef]
- Sun, Z.Y.; Yang, Y.; Qin, W.H.; Ren, S.T.; Wu, G. Experimental study on flexural behavior of concrete beams reinforced by steel-fiber reinforced polymer composite bars. J. Reinf. Plast. Compos. 2013, 31, 1737–1745. [Google Scholar] [CrossRef]
- Wang, D.Y.; Qi, J.S.; Cui, G.Y.; Yang, Y.L.; Chang, J. Model Test on Bearing Characteristics of Basalt Fiber-Reinforced Concrete Lining. Adv. Mater. Sci. Eng. 2020, 2020, 3891343. [Google Scholar] [CrossRef] [Green Version]
- Monaldo, E.; Nerilli, F.; Vairo, G. Basalt-based fiber-reinforced materials and structural applications in civil engineering. Compos. Struct. 2019, 214, 246–263. [Google Scholar] [CrossRef]
- Zhou, H.; Jia, B.; Huang, H.; Mou, Y.L. Experimental Study on Basic Mechanical Properties of Basalt Fiber Reinforced Concrete. Materials 2020, 13, 1362. [Google Scholar] [CrossRef] [Green Version]
- Jalasutram, S.; Sahoo, D.R.; Matsagar, V. Experimental investigation of the mechanical properties of basalt fiber-reinforced concrete. Struct. Concr. 2017, 18, 292–302. [Google Scholar] [CrossRef]
- Alaskar, A.; Albidah, A.; Alqarni, A.S.; Alyousef, R.; Mohammadhosseini, H. Performance evaluation of high-strength concrete reinforced with basalt fibers exposed to elevated temperatures. J. Build. Eng. 2021, 35, 102108. [Google Scholar] [CrossRef]
- Chen, F.B.; Xu, B.; Jiao, H.Z.; Chen, X.M.; Shi, Y.L.; Wang, J.X.; Li, Z. Triaxial mechanical properties and microstructure visualization of BFRC. Constr. Build. Mater. 2021, 278, 122275. [Google Scholar] [CrossRef]
- Li, X. Research on Micro and Meso Void Structure and Preferential Flow Characteristics of Loess Based on CT. Ph.D. Thesis, Chang’an University, Xi′an, China, 2020. [Google Scholar]
- Song, D.Y.; He, K.K.; Ji, X.F.; Li, Y.B.; Zhao, H.T. Fine characterization of pores and fractures in coal based on a CT scan. Nat. Gas Ind. 2018, 38, 41–49. [Google Scholar] [CrossRef]
- Liu, X.; Sun, Q.H.; Yuan, Y.; Taerwe, L. Comparison of the structural behavior of reinforced concrete tunnel segments with steel fiber and synthetic fiber addition. Tunn. Undergr. Space Technol. 2020, 103, 103506. [Google Scholar] [CrossRef]
- Wang, T.; Zhang, J.H.; Bai, W.F.; Hao, S.M. Forming process and mechanical properties of fibers-reinforced polymer concrete. J. Reinf. Plast. Compos. 2013, 32, 907–911. [Google Scholar] [CrossRef]
- Jiao, H.Z.; Han, Z.Y.; Chen, X.M.; Yang, Y.X.; Wang, Y.F. Flexural toughness evolution of of basalt fiber reinforced shotcrete based on NMR technology. J. China Coal Soc. 2019, 44, 2990–2998. [Google Scholar]
- Jiao, H.Z.; Chen, W.L.; Wu, A.X.; Yu, Y.; Ruan, Z.E.; Honaker, R.; Chen, X.M.; Yu, J.X. Flocculated unclassified tailings settling efficiency improvement by particle collision optimization in the feedwell. Int. J. Miner. Metall. Mater. 2022, 44, 553. [Google Scholar] [CrossRef]
- Jiao, H.Z.; Wu, Y.C.; Wang, H.; Chen, X.M.; Li, Z.; Wang, Y.; Zhang, B.; Liu, J. Micro-scale mechanism of sealed water seepage and thickening from tailings bed in rake shearing thickener. Miner. Eng. 2021, 173, 107043. [Google Scholar] [CrossRef]
- Zhu, H.G.; Xie, H.P.; Yi, C.; Liu, Z.; Liu, H.X.; Wang, H.-T. CT Identification of Microcracks Evolution for Rock Materials. Chin. J. Rock Mech. Eng. 2011, 30, 1230–1238. [Google Scholar]
- Wang, D.K.; Zhang, H.; Wei, J.P.; Wu, Y.; Zhang, H.T.; Yao, B.H.; Fu, J.H.; Zhao, H.; Li, Z. Dynamic evolution characteristics of fractures in gas-bearing coal under the influence of gas pressure using industrial CT scanning technology. J. China Coal Soc. 2021, 46, 3550–3564. [Google Scholar]
- Zhong, Y.R.; Guo, D.W.; Huang, M.F. Application of Gauss filtering and information entropy principle in verification of arithmetic mean deviation. Comput. Eng. Appl. 2009, 45, 230–234. [Google Scholar]
- Yang, J.; Wu, Q.Y.; Tian, J.; Yang, H. The Implementation and Evaluation of Several CT Image Segmentation Algorithms. Trans. Beijing Inst. Technol. 2000, 20, 720–724. [Google Scholar]
- Gao, Z.; Cao, P.; Sun, X.J.; Zhao, Y.W. Compressive strength analysis and microscopic characterization of basalt fiber reinforced concrete. J. Hydroelectr. Eng. 2018, 37, 111–120. [Google Scholar]
- Zheng, D.; Song, W.D.; Fu, J.X.; Xue, G.L.; Li, J.J.; Cao, S. Research on mechanical characteristics, fractal dimension and internal structure of fiber reinforced concrete under uniaxial compression. Constr. Build. Mater. 2020, 258, 120351. [Google Scholar] [CrossRef]
- Rios, J.D.; Minguez, J.; Martinez-De La Concha, A.; Vicente, M.A.; Cifuentes, H. Microstructural analyses of the addition of PP fibres on the fracture properties of high-strength self-compacting concrete by X-ray computed tomography. Constr. Build. Mater. 2020, 261, 120499. [Google Scholar] [CrossRef]
- Tan, X.X. Research on Microstructure and Seepage Characteristics of Compacted Granite Weathered Soil. Master’s Thesis, Guilin University of Technology, Guilin, China, 2021. [Google Scholar]
- Li, N.; Zhao, Y.R. Research Progress of Concrete Internal Damage Based on X-ray CT Technology. Mater. Rep. 2021, 35, 21169–21177. [Google Scholar]
- Li, J.S.; Wang, D.; Kang, T.H. Algorithmic study on rock pore structure based on micro-CT experiment. Chin. J. Geotech. Eng. 2010, 32, 1703–1708. [Google Scholar]
- Lv, B.M. Research on Three-Dimensional Characterization and Permeation of Coal-Based Porous Carbon. Master’s Thesis, China University of Mining and Technology, Xuzhou, China, 2019. [Google Scholar]
- Wei, H.L. Research on Meso-Pore Structure and Clogging Laws of Ceramic Permeable Pavement Brick. Master’s Thesis, Northwest A&F University, Xianyang, China, 2021. [Google Scholar]
- Yang, Q.; Yu, Y.B.; Cui, W.T.; Gao, C.W.; Zhang, X.; Shen, J.L. Fracture evolution of coal under uniaxial compression based on X-ray microscopic imaging. Coal Sci. Technol. 2022. [Google Scholar] [CrossRef]
- Liu, T.T. Pore Characteristics Analysis and Seepage Simulation of Cast-In-Situ Frost-Resistant Vegetation Concrete. Master’s Thesis, Shandong Agricultural University, Shandong, China, 2020. [Google Scholar]
- Chen, F.B.; Xu, B.; Jiao, H.Z.; Ruan, Z.E.; Liu, J.H.; Chen, X.M.; Yang, L.H.; Li, Z. Characterization of Internal Defects and Fiber Distribution of BFRC Based on the Digital Image Processing Technology. Crystals 2021, 11, 847. [Google Scholar] [CrossRef]
- Liu, Z.X.; Dong, S.N.; Nan, S.H.; Zhang, D.-Y.; Liu, D. Micro-CT analysis of void characteristics at the top of middle ordovician limestone in Hanxing mining area. J. Min. Saf. Eng. 2021, 38, 343–352. [Google Scholar]
- Jin, Z.M.; Zhou, H.W.; Xue, D.J. Measurement of porosity and specific surface area of coal rock using CT images. J. Xi’an Univ. Sci. Technol. 2020, 40, 133–140. [Google Scholar]
- Jing, H. Characterization for Micro-structure and Compressional Behaviour of General Fibrous Assemblies. Ph.D. Thesis, Donghua University, Shanghai, China, 2018. [Google Scholar]
- Chen, X.M.; Shi, Y.L.; Jiao, H.Z.; Jin, X.F.; Wu, Y.C.; Tan, Y. Fiber Distribution Characteristics Based on Search Cone Algorithm and Enhancement Mechanism of BFRC. Mater. Rep. 2021, 35, 4061–4066. [Google Scholar]
- Mu, R.; Ma, Y.F.; Li, H.; Wang, X.W.; Zhang, P. Analysis of the distribution of steel fiber in aligned steel fiber reinforced concrete using digital X-ray CT scanning. J. Chin. Electron Microsc. Soc. 2015, 34, 487–491. [Google Scholar]
- Li, H. Numerical Investigation on Mechanism of Effect of Fiber Orientation on Properties of Cement-Based Composites at Meso- and Micro-Scale. Ph.D. Thesis, Southeast University, Nanjing, China, 2019. [Google Scholar]
- Yu, R.; Fan, D.Q.; Sun, M.J.; Zhang, J.J.; Shuai, Z.H.; Hou, D.S.; Wang, D.S.; Wang, X.P.; Feng, Y. Effects of Steel Fibre Content and 3D Network on Performance of Ultra-High Performance Concrete. J. Chin. Ceram. Soc. 2021, 49, 2313–2321. [Google Scholar]
- Zhao, J.Z.; Shi, Y.L.; Li, X.L.; Xu, Y. Study on the Influence of Fiber Distribution on Mechanical Properties of Polymer Cement Concrete. China Concr. Cem. Prod. 2021, 11, 55–58+72. Available online: https://cf.cnki.net/kcms/detail/detail.aspx?filename=HNTW202111015amp;dbcode=XWCJamp;dbname=XWCTLKCJFDLAST2021amp;v= (accessed on 29 January 2022).
- Ponikiewski, T.; Golaszewski, J.; Rudzki, M.; Bugdol, M. Determination of steel fibres distribution in self-compacting concrete beams using X-ray computed tomography. Arch. Civ. Mech. Eng. 2015, 15, 558–568. [Google Scholar] [CrossRef]
- Zarzycki, P.K.; Katzer, J.; Domski, J. Fast classification of fibres for concrete based on multivariate statistics. Comput. Concr. An Int. J. 2017, 20, 23–29. [Google Scholar]








| Strength Grade | Apparent Density | Bulk Density | Setting Time (h) | Flexural Strength (MPa) | Compressive Strength (MPa) | |||
|---|---|---|---|---|---|---|---|---|
| 42.5 | 3.1 | 1.47 | Initial Setting | Final Coagulation | 3d | 28d | 3d | 28d |
| 2 | 7.5 | 3.5 | 6.5 | 17.0 | 42.5 | |||
| Density | Diameter (μm) | Tensile Strength (MPa) | Modulus of Elasticity (GPa) | Elongation at Break (%) | Maximum Operating Temperature (°C) |
|---|---|---|---|---|---|
| 2.7~2.8 | 13 | 3000~4000 | 80~100 | 2.7~3.4 | 600~700 |
| Fiber Dosage/kg·m−3 | Model Size/μm | Number of Spheres | Number of Sicks | Porosity |
|---|---|---|---|---|
| 0 | 200 × 200 × 200 | 534 | 218 | 18.59 |
| 1.5 | 512 | 176 | 14.36 | |
| 3 | 486 | 152 | 11.28 | |
| 4.5 | 508 | 182 | 15.29 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jiao, H.; Chen, X.; Yang, X.; Wang, Y.; Yang, Y.; Chen, X.; Yang, L.; Yu, J. Quantifying Computed Tomography of Basalt Fiber-Reinforced Concrete under Unconfined Compression. Crystals 2022, 12, 360. https://doi.org/10.3390/cryst12030360
Jiao H, Chen X, Yang X, Wang Y, Yang Y, Chen X, Yang L, Yu J. Quantifying Computed Tomography of Basalt Fiber-Reinforced Concrete under Unconfined Compression. Crystals. 2022; 12(3):360. https://doi.org/10.3390/cryst12030360
Chicago/Turabian StyleJiao, Huazhe, Xi Chen, Xiaolin Yang, Yunfei Wang, Yixuan Yang, Xinming Chen, Liuhua Yang, and Jianxin Yu. 2022. "Quantifying Computed Tomography of Basalt Fiber-Reinforced Concrete under Unconfined Compression" Crystals 12, no. 3: 360. https://doi.org/10.3390/cryst12030360
APA StyleJiao, H., Chen, X., Yang, X., Wang, Y., Yang, Y., Chen, X., Yang, L., & Yu, J. (2022). Quantifying Computed Tomography of Basalt Fiber-Reinforced Concrete under Unconfined Compression. Crystals, 12(3), 360. https://doi.org/10.3390/cryst12030360







