Understanding the Plastic Deformation of Gradient Interstitial Free (IF) Steel under Uniaxial Loading Using a Dislocation-Based Multiscale Approach †
Abstract
:1. Introduction
2. Materials and Methods
2.1. CDD-VPSC Model
2.2. Back-Stress
2.3. Slip Transmission
2.4. Implementation
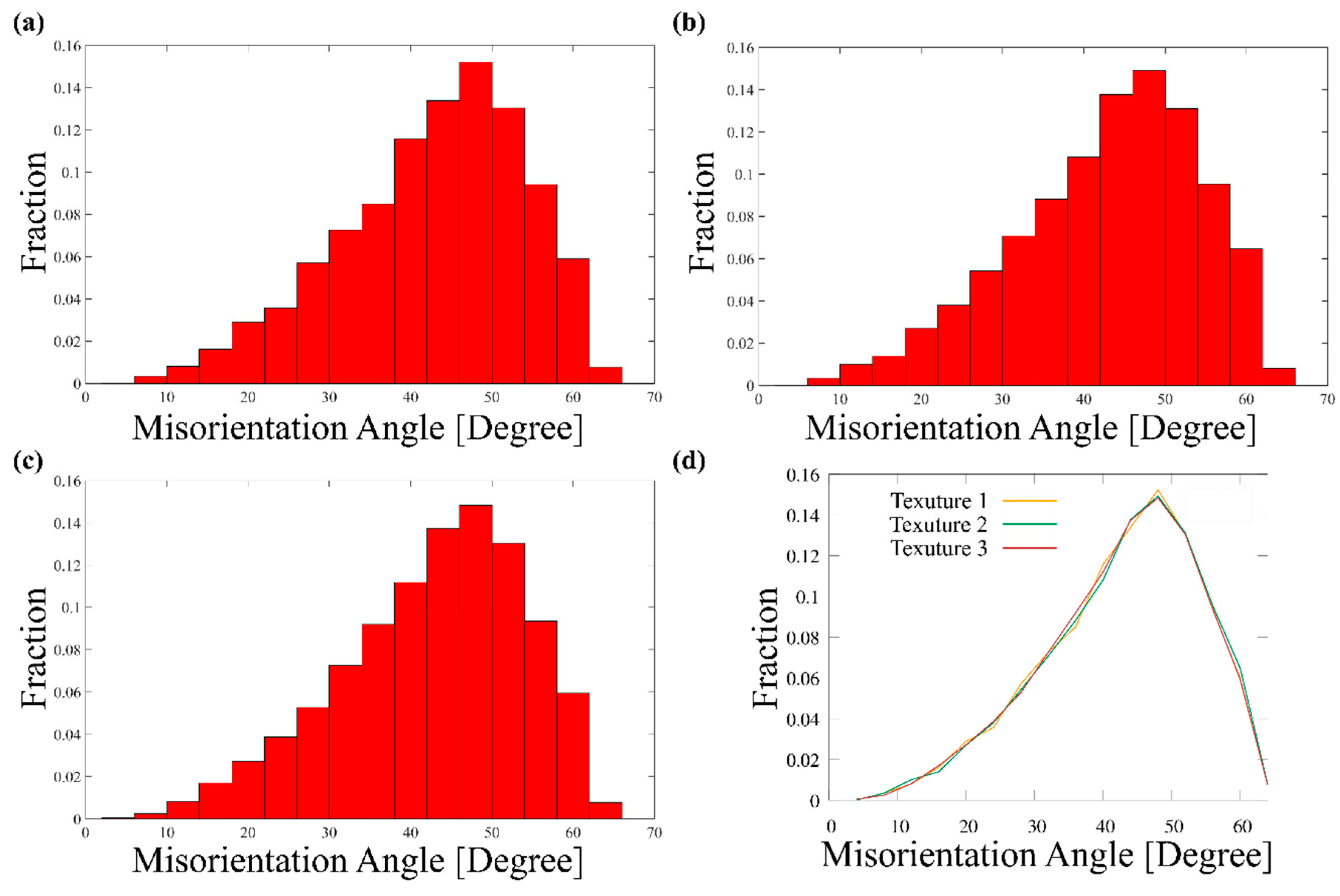
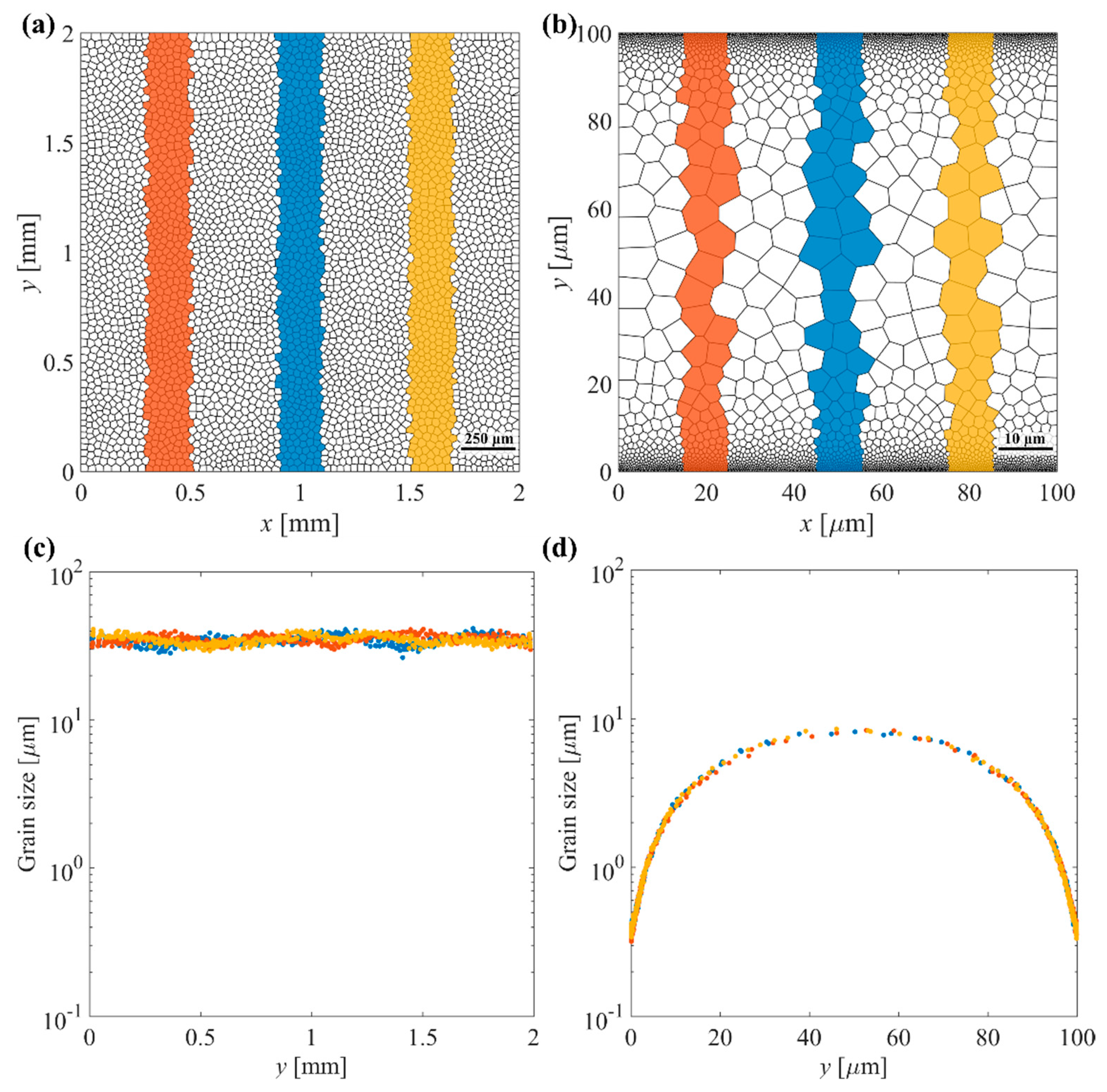
2.5. Samples Generation
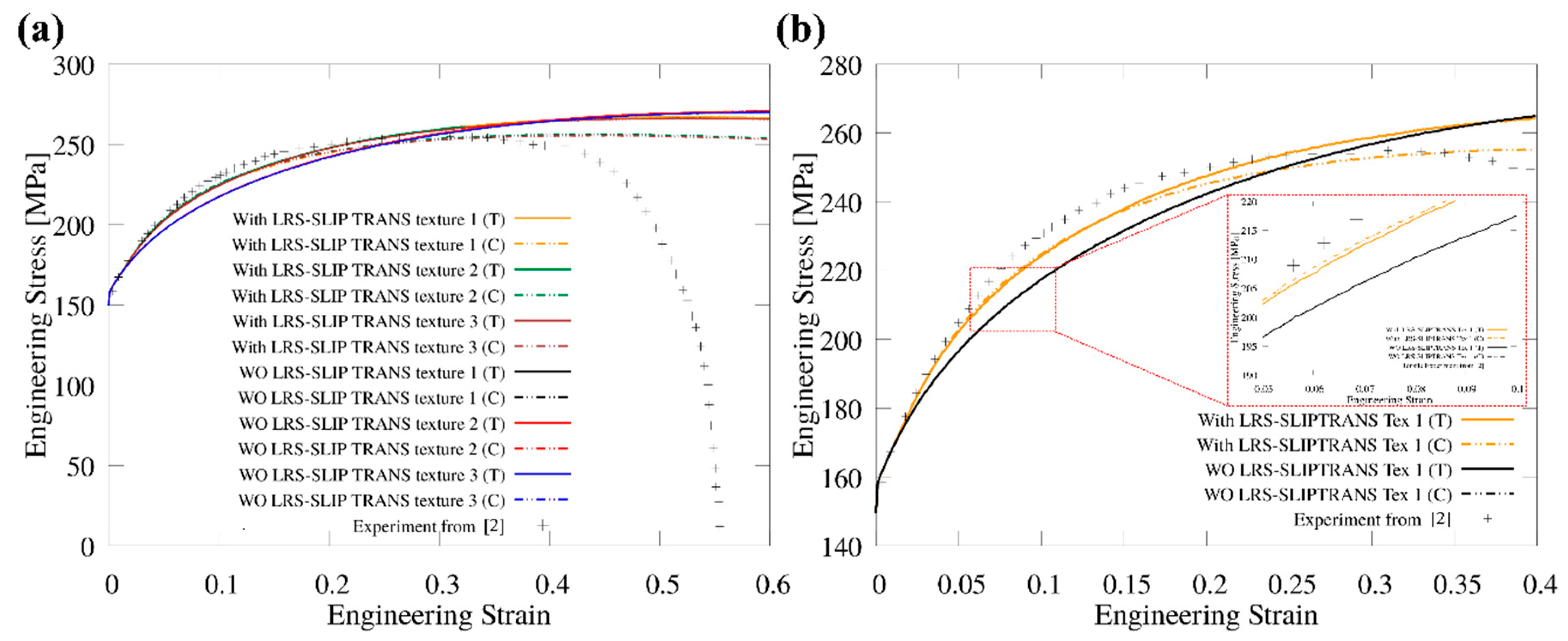
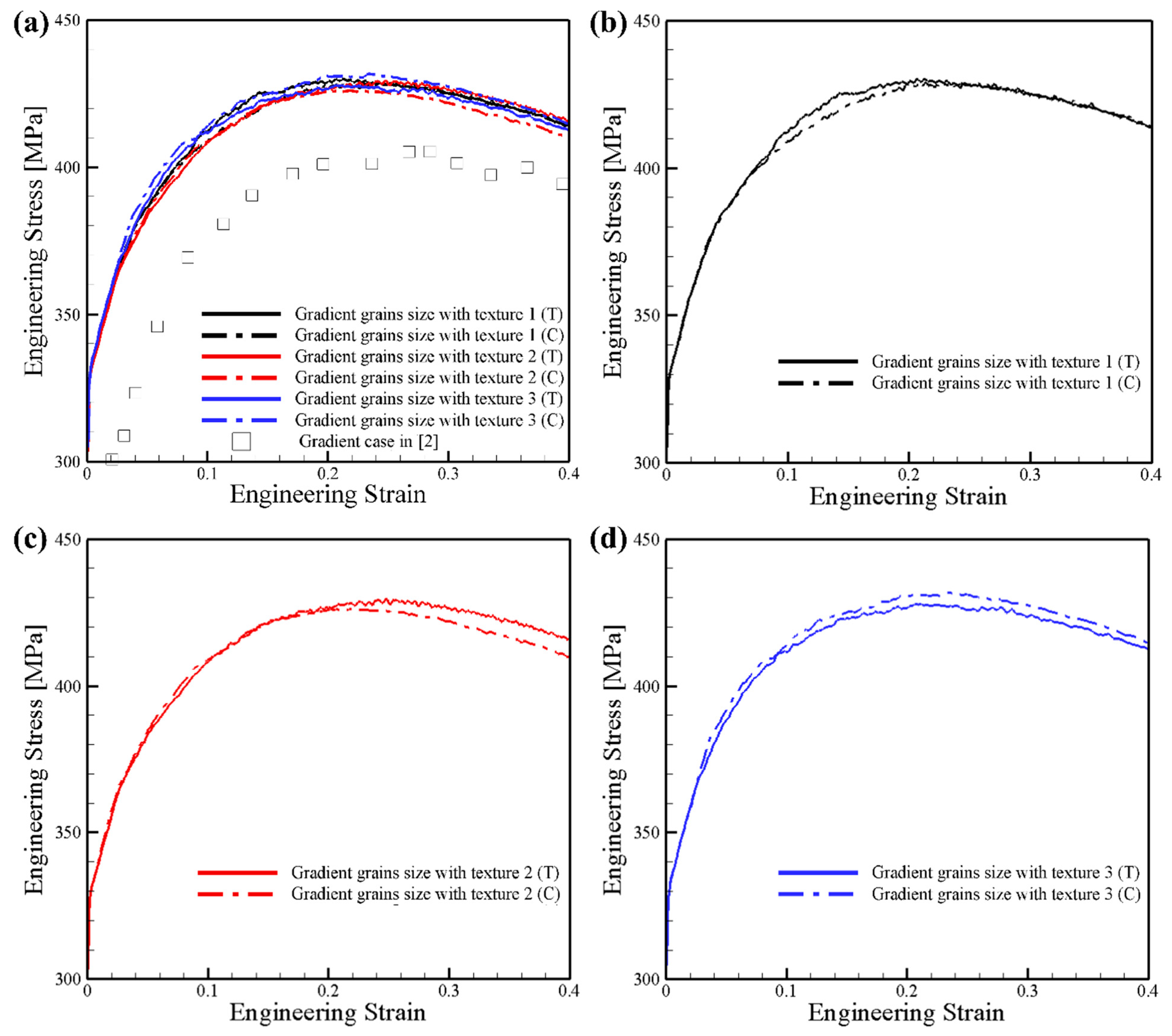
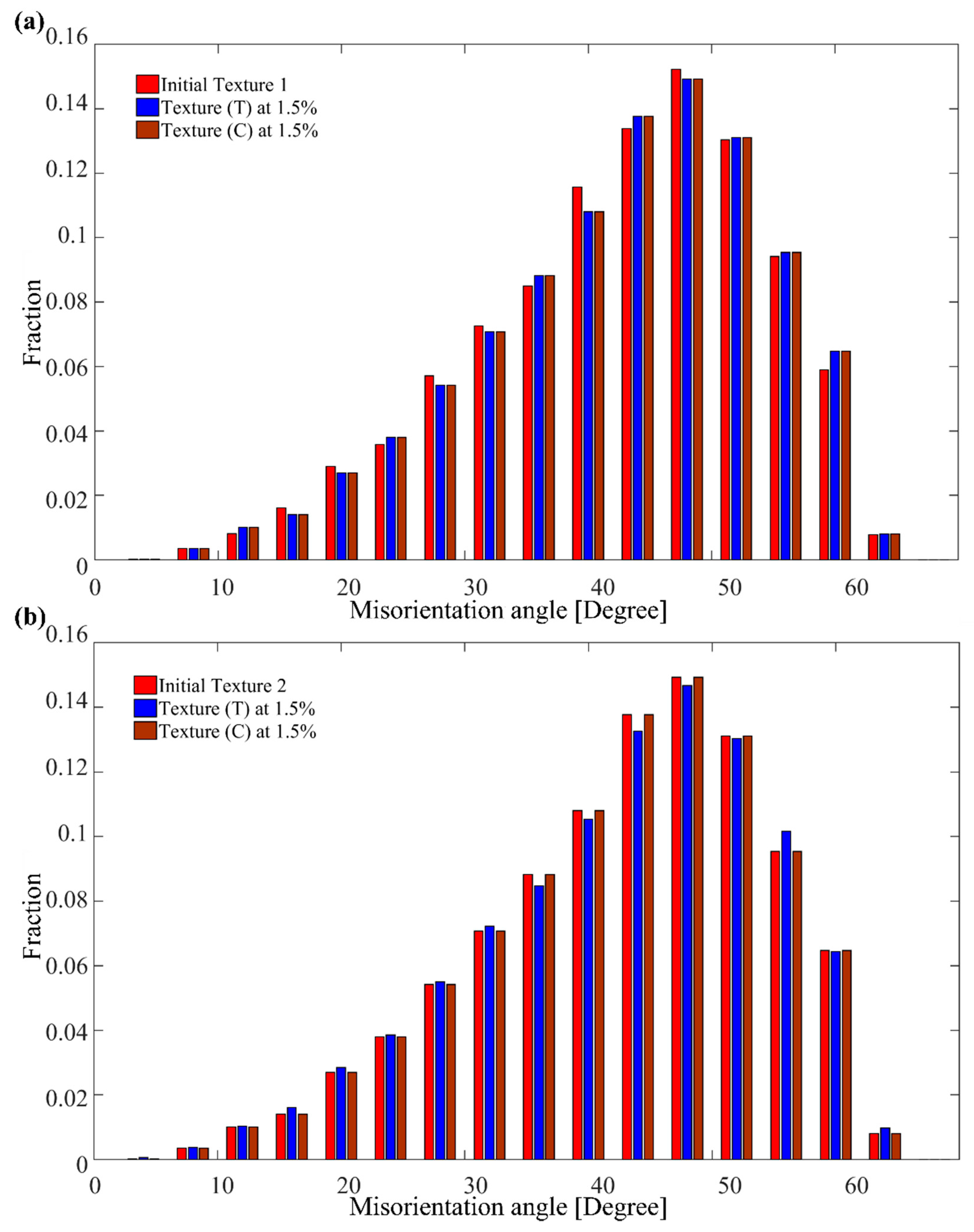
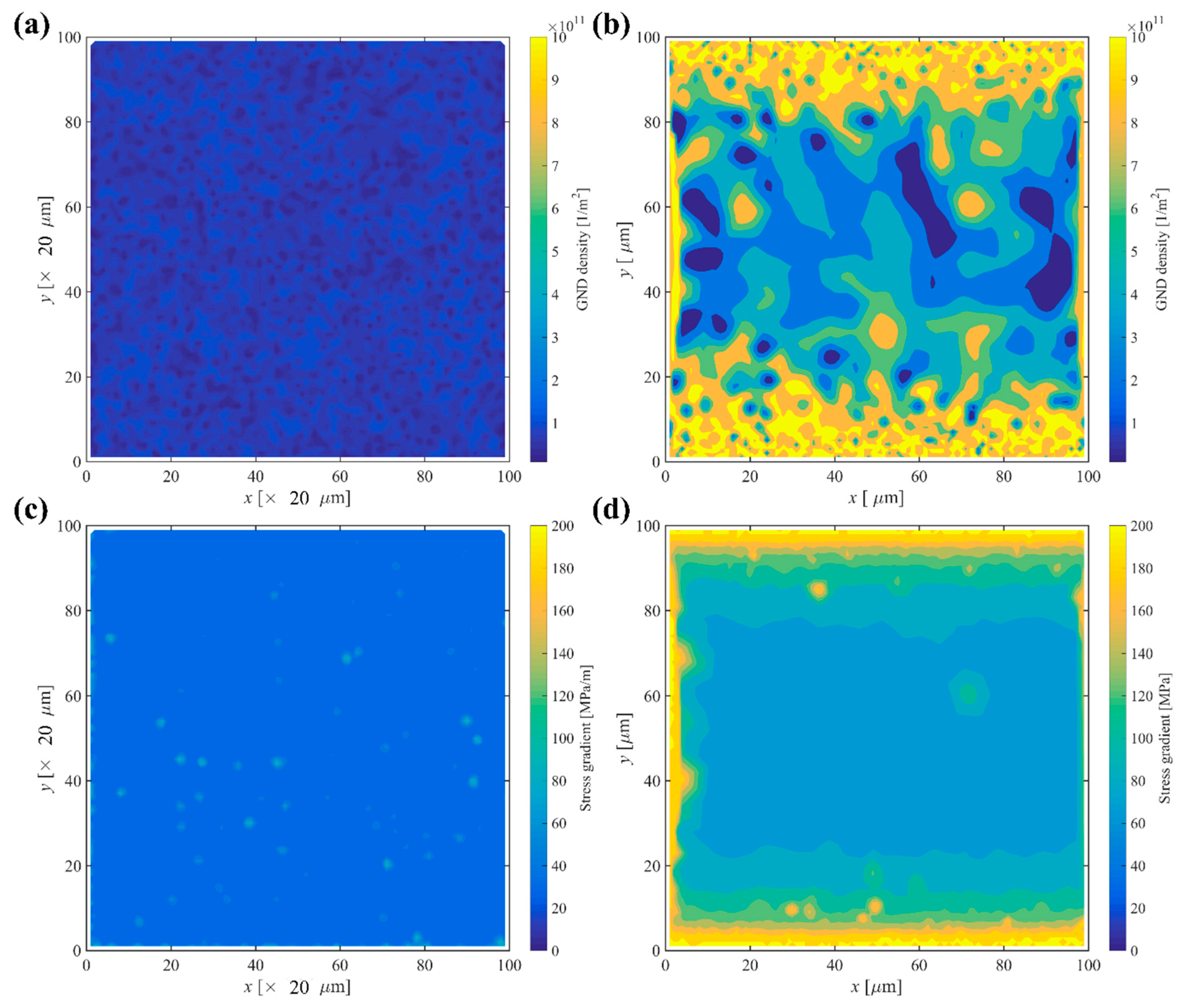
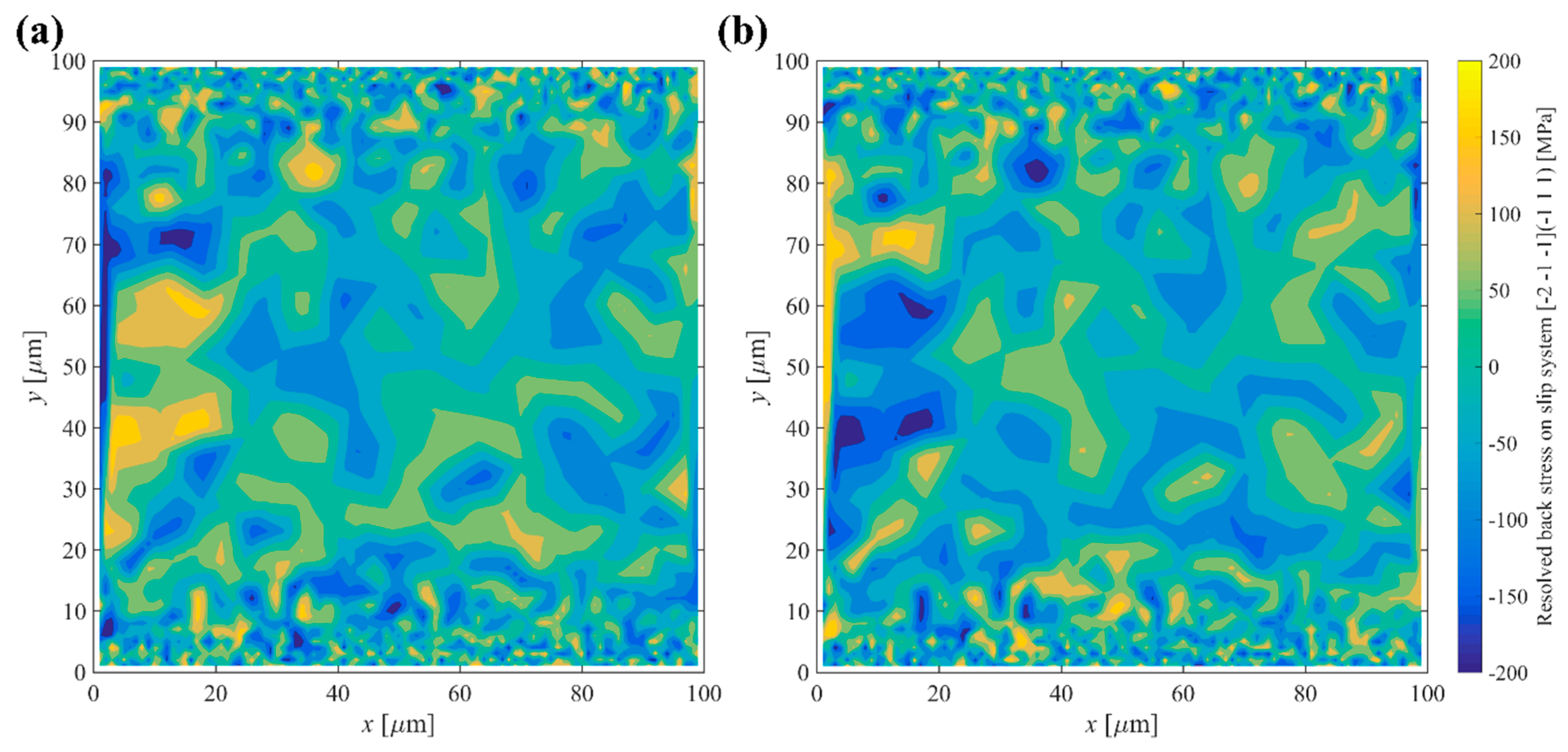
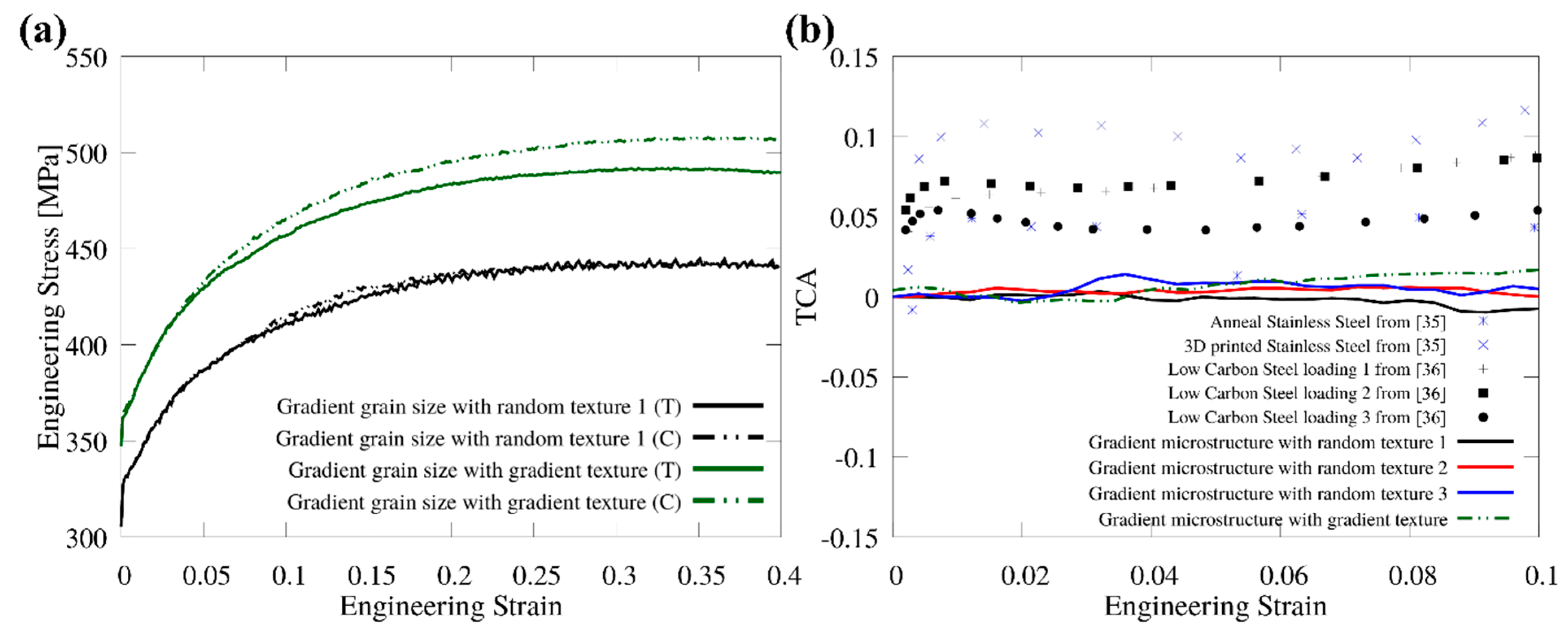
3. Results
4. Discussion
4.1. Homogeneous vs. Gradient Structures
4.2. Slip Transmission and Relation to Tensile Strength and Ductility
4.3. Slip Transmission in Homogeneous vs. Gradient Structures
4.4. Slip Transmission in Compression vs. Tension
4.5. Tension-Compression Asymmetry (TCA)
5. Conclusions
- (1)
- Specimens with gradient texture cause deformation gradient along the grain size gradient direction. Plastic deformation occurs first in the coarse grains in the center region. Then it gradually expands to the transient and the nano region. Local inhomogeneous deformations were more easily induced for microstructures with a bi-or multi-modal grain size distribution and gradient size distribution along the y-direction.
- (2)
- There is no clear correlation between slip transmission and tensile strength. More slip transmission in the nano region could explain the delay in the onset of instability.
- (3)
- The back-stress quantity which arises from dislocations and slip transmission causes tension-compression asymmetry (TCA) behavior. TCA exists in specimens with homogeneous microstructure and with gradient microstructure. The simulations show that the predicted TCA values are lower than recorded experimentally. The initial texture of the specimens plays a predominant role in TCA.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
Appendix B
Appendix C
References
- Lu, K. Making strong nanomaterials ductile with gradients: Microstructures that increase metal crystallite size from nanoscale with surface depth are both strong and ductile. Science 2014, 345, 1455–1456. [Google Scholar] [CrossRef] [PubMed]
- Wu, X.; Jiang, P.; Chen, L.; Yuan, F.; Zhu, Y.T. Extraordinary strain hardening by gradient structure. Proc. Natl. Acad. Sci. USA 2014, 111, 7197–7201. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Shao, C.W.; Zhang, P.; Zhu, Y.K.; Zhang, Z.J.; Tian, Y.Z.; Zhang, Z.F. Simultaneous improvement of strength and plasticity: Additional work-hardening from gradient microstructure. Acta Mater. 2018, 145, 413–428. [Google Scholar] [CrossRef]
- Wu, X.L.; Yang, M.X.; Yuan, F.P.; Chen, L.; Zhu, Y.T. Combining gradient structure and TRIP effect to produce austenite stainless steel with high strength and ductility. Acta Mater. 2016, 112, 337–346. [Google Scholar] [CrossRef] [Green Version]
- Wu, Y.; Guelorget, B.; Sun, Z.; Déturche, R.; Retraint, D. Characterization of gradient properties generated by SMAT for a biomedical grade 316L stainless steel. Mater. Charact. 2019, 155, 109788. [Google Scholar] [CrossRef]
- Wang, L.; Li, B.; Shi, Y.; Huang, G.; Song, W.; Li, S. Optimizing mechanical properties of gradient-structured low-carbon steel by manipulating grain size distribution. Mater. Sci. Eng. A 2019, 743, 309–313. [Google Scholar] [CrossRef]
- Shi, Y.; Li, B.; Gao, F.; Wang, L.; Qin, F.; Liu, H.; Li, S. An outstanding synergy of high strength and ductility in gradient structured low-carbon steel. Materialia 2019, 5, 100181. [Google Scholar] [CrossRef]
- Jamalian, M.; Hamid, M.; De Vincentis, N.; Buck, Q.; Field, D.P.; Zbib, H.M. Creation of heterogeneous microstructures in copper using high-pressure torsion to enhance mechanical properties. Mater. Sci. Eng. A 2019, 756, 142–148. [Google Scholar] [CrossRef]
- Kang, J.Y.; Kim, J.G.; Park, H.W.; Kim, H.S. Multiscale architectured materials with composition and grain size gradients manufactured using high-pressure torsion. Sci. Rep. 2016, 6, 26590. [Google Scholar] [CrossRef]
- Ma, E.; Zhu, T. Towards strength–ductility synergy through the design of heterogeneous nanostructures in metals. Mater. Today 2017, 20, 323–331. [Google Scholar] [CrossRef]
- Li, J.; Cao, Y.; Gao, B.; Li, Y.; Zhu, Y. Superior strength and ductility of 316L stainless steel with heterogeneous lamella structure. J. Mater. Sci. 2018, 53, 10442–10456. [Google Scholar] [CrossRef]
- Li, J.; Gao, B.; Huang, Z.; Zhou, H.; Mao, Q.; Li, Y. Design for strength-ductility synergy of 316L stainless steel with heterogeneous lamella structure through medium cold rolling and annealing. Vacuum 2018, 157, 128–135. [Google Scholar] [CrossRef]
- Wang, P.; Xiang, Y.; Wang, X.; Liu, Z.; Qu, S.; Zhuang, Z. New insight for mechanical properties of metals processed by severe plastic deformation. Int. J. Plast. 2019, 123, 22–37. [Google Scholar] [CrossRef]
- Wang, Y.F.; Wang, M.S.; Fang, X.T.; Guo, F.J.; Liu, H.Q.; Scattergood, R.O.; Huang, C.X.; Zhu, Y.T. Extra strengthening in a coarse/ultrafine grained laminate: Role of gradient interfaces. Int. J. Plast. 2019, 123, 196–207. [Google Scholar] [CrossRef]
- Roland, T.; Retraint, D.; Lu, K.; Lu, J. Fatigue life improvement through surface nanostructuring of stainless steel by means of surface mechanical attrition treatment. Scr. Mater. 2006, 54, 1949–1954. [Google Scholar] [CrossRef]
- Yang, M.; Pan, Y.; Yuan, F.; Zhu, Y.; Wu, X. Back stress strengthening and strain hardening in gradient structure. Mater. Res. Lett. 2016, 4, 145–151. [Google Scholar] [CrossRef]
- Yang, X.; Ma, X.; Moering, J.; Zhou, H.; Wang, W.; Gong, Y.; Tao, J.; Zhu, Y.; Zhu, X. Influence of gradient structure volume fraction on the mechanical properties of pure copper. Mater. Sci. Eng. A 2015, 645, 280–285. [Google Scholar] [CrossRef]
- Fang, T.H.; Li, W.L.; Tao, N.R.; Lu, K. Revealing extraordinary intrinsic tensile plasticity in gradient nano-grained copper. Science 2011, 331, 1587–1590. [Google Scholar] [CrossRef] [Green Version]
- Yan, X.; Meng, X.; Luo, L.; Jing, Y.; Yi, G.; Lu, J.; Liu, Y. Mechanical behaviour of AZ31 magnesium alloy with the laminate and gradient structure. Philos. Mag. 2019, 99, 3059–3077. [Google Scholar] [CrossRef]
- Kale, C.; Turnage, S.; Avery, D.Z.; El Kadiri, H.; Jordon, J.B.; Solanki, K.N. Towards dynamic tension-compression asymmetry and relative deformation mechanisms in magnesium. Materialia 2020, 9, 100543. [Google Scholar] [CrossRef]
- Ning, J.L.; Xu, B.; Feng, Y.L.; Li, X.D.; Li, X.K.; Tong, W.P. Tension–Compression Yield Asymmetry Influenced by the Variable Deformation Modes in Gradient Structure Mg Alloys. Acta Metall. Sin. (Engl. Lett.) 2020, 33, 252–266. [Google Scholar] [CrossRef] [Green Version]
- Lin, J.B.; Ren, W.J.; Wang, X.Y.; Ma, L.F. Tension–compression asymmetry in yield strength and hardening behaviour of as-extruded AZ31 alloy. Mater. Sci. Technol. 2016, 32, 1855–1860. [Google Scholar] [CrossRef]
- Habib, S.A.; Khan, A.S.; Gnäupel-Herold, T.; Lloyd, J.T.; Schoenfeld, S.E. Anisotropy, tension-compression asymmetry and texture evolution of a rare-earth-containing magnesium alloy sheet, ZEK100, at different strain rates and temperatures: Experiments and modeling. Int. J. Plast. 2017, 95, 163–190. [Google Scholar] [CrossRef]
- Kim, J.Y.; Greer, J.R. Tensile and compressive behavior of gold and molybdenum single crystals at the nano-scale. Acta Mater. 2009, 57, 5245–5253. [Google Scholar] [CrossRef]
- Alkan, S.; Sehitoglu, H. Non-Schmid response of Fe3Al: The twin-antitwin slip asymmetry and non-glide shear stress effects. Acta Mater. 2017, 125, 550–566. [Google Scholar] [CrossRef] [Green Version]
- Xing, Z.; Fan, H.; Tang, J.; Wang, B.; Kang, G. Molecular dynamics simulation on the cyclic deformation of magnesium single crystals. Comput. Mater. Sci. 2021, 186, 110003. [Google Scholar] [CrossRef]
- Kurukuri, S.; Worswick, M.J.; Tari, D.G.; Mishra, R.K.; Carter, J.T. Rate sensitivity and tension-compression asymmetry in AZ31B magnesium alloy sheet. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2014, 372, 20130216. [Google Scholar] [CrossRef]
- Ahmadikia, B.; Kumar, M.A.; Beyerlein, I.J. Effect of neighboring grain orientation on strain localization in slip bands in HCP materials. Int. J. Plast. 2021, 144, 103026. [Google Scholar] [CrossRef]
- Wang, Z.Q.; Beyerlein, I.J. An atomistically-informed dislocation dynamics model for the plastic anisotropy and tension-compression asymmetry of BCC metals. Int. J. Plast. 2011, 27, 1471–1484. [Google Scholar] [CrossRef]
- Cho, H.; Bronkhorst, C.A.; Mourad, H.M.; Mayeur, J.R.; Luscher, D.J. Anomalous plasticity of body-centered-cubic crystals with non-Schmid effect. Int. J. Solids Struct. 2018, 139–140, 138–149. [Google Scholar] [CrossRef] [Green Version]
- Gröger, R.; Vitek, V. Impact of non-Schmid stress components present in the yield criterion for bcc metals on the activity of {110}<111> slip systems. Comput. Mater. Sci. 2019, 159, 297–305. [Google Scholar] [CrossRef]
- Long, J.; Pan, Q.; Tao, N.; Lu, L. Residual stress induced tension-compression asymmetry of gradient nanograined copper. Mater. Res. Lett. 2018, 6, 456–461. [Google Scholar] [CrossRef]
- Maeda, T.; Noma, N.; Kuwabara, T.; Barlat, F.; Korkolis, Y.P. Experimental Verification of the Tension-Compression Asymmetry of the Flow Stresses of a High Strength Steel Sheet. Procedia Eng. 2017, 207, 1976–1981. [Google Scholar] [CrossRef]
- Chen, W.; Voisin, T.; Zhang, Y.; Florien, J.B.; Spadaccini, C.M.; McDowell, D.L.; Zhu, T.; Wang, Y.M. Microscale residual stresses in additively manufactured stainless steel. Nat. Commun. 2019, 10, 4338. [Google Scholar] [CrossRef] [Green Version]
- Koizumi, T.; Kuroda, M. Evaluation of tension-compression asymmetry of a low-carbon steel sheet using a modified classical compression test method. J. Phys. Conf. Ser. 2018, 1063, 012167. [Google Scholar] [CrossRef] [Green Version]
- Lehto, P.; Remes, H.; Saukkonen, T.; Hänninen, H.; Romanoff, J. Influence of grain size distribution on the Hall-Petch relationship of welded structural steel. Mater. Sci. Eng. A 2014, 592, 28–39. [Google Scholar] [CrossRef] [Green Version]
- Li, J.; Weng, G.J.; Chen, S.; Wu, X. On strain hardening mechanism in gradient nanostructures. Int. J. Plast. 2017, 88, 89–107. [Google Scholar] [CrossRef] [Green Version]
- Lyu, H.; Hamid, M.; Ruimi, A.; Zbib, H.M. Stress/strain gradient plasticity model for size effects in heterogeneous nano-microstructures. Int. J. Plast. 2017, 97, 46–63. [Google Scholar] [CrossRef]
- Ma, A.; Roters, F.; Raabe, D. A dislocation density based constitutive model for crystal plasticity FEM including geometrically necessary dislocations. Acta Mater. 2006, 54, 2169–2179. [Google Scholar] [CrossRef]
- Zhao, J.; Lu, X.; Yuan, F.; Kan, Q.; Qu, S.; Kang, G.; Zhang, X. Multiple mechanism based constitutive modeling of gradient nanograined material. Int. J. Plast. 2020, 125, 314–330. [Google Scholar] [CrossRef]
- Xiao, X.; Chen, L.; Yu, L.; Duan, H. Modelling nano-indentation of ion-irradiated FCC single crystals by strain-gradient crystal plasticity theory. Int. J. Plast. 2019, 116, 216–231. [Google Scholar] [CrossRef]
- Fleck, N.A.; Muller, G.M.; Ashby, M.F.; Hutchinson, J.W. Strain gradient plasticity: Theory and experiment. Acta Metall. Mater. 1994, 42, 475–487. [Google Scholar] [CrossRef]
- Hirth, J.P. Dislocation pileups in the presence of stress gradients. Philos. Mag. 2006, 86, 3959–3963. [Google Scholar] [CrossRef]
- Chakravarthy, S.S.; Curtin, W.A. Stress-gradient plasticity. Proc. Natl. Acad. Sci. USA 2011, 108, 15716–15720. [Google Scholar] [CrossRef] [Green Version]
- Liu, D.; He, Y.; Zhang, B.; Shen, L. A continuum theory of stress gradient plasticity based on the dislocation pile-up model. Acta Mater. 2014, 80, 350–364. [Google Scholar] [CrossRef]
- Taheri-Nassaj, N.; Zbib, H.M. On dislocation pileups and stress-gradient dependent plastic flow. Int. J. Plast. 2015, 74, 1–16. [Google Scholar] [CrossRef]
- Lyu, H.; Ruimi, A.; Zbib, H.M. A dislocation-based model for deformation and size effect in multi-phase steels. Int. J. Plast. 2015, 72, 44–59. [Google Scholar] [CrossRef]
- Lyu, H.; Taheri-Nassaj, N.; Zbib, H.M. A multiscale gradient-dependent plasticity model for size effects. Philos. Mag. 2016, 96, 1883–1908. [Google Scholar] [CrossRef]
- Akarapu, S. Dislocation Interactions with Interfaces; Washington State University: Washington, DC, USA, 2009. [Google Scholar]
- Lim, L.C.; Raj, R. Continuity of slip screw and mixed crystal dislocations across bicrystals of nickel at 573 K. Acta Metall. 1985, 33, 1577–1583. [Google Scholar] [CrossRef]
- Zaefferer, S.; Kuo, J.C.; Zhao, Z.; Winning, M.; Raabe, D. On the influence of the grain boundary misorientation on the plastic deformation of aluminum bicrystals. Acta Mater. 2003, 51, 4719–4735. [Google Scholar] [CrossRef]
- Priester, L. Grain Boundaries: From Theory to Engineering; Springer: Berlin/Heidelberg, Germany, 2013; Volume 172, ISBN 978-94-007-4968-9. [Google Scholar]
- Kacher, J.; Eftink, B.P.; Cui, B.; Robertson, I.M. Dislocation interactions with grain boundaries. Curr. Opin. Solid State Mater. Sci. 2014, 18, 227–243. [Google Scholar] [CrossRef]
- Hamid, M.; Lyu, H.; Schuessler, B.J.; Wo, P.C.; Zbib, H.M. Modeling and characterization of grain boundaries and slip transmission in dislocation density-based crystal plasticity. Crystals 2017, 7, 152. [Google Scholar] [CrossRef] [Green Version]
- Akarapu, S.; Zbib, H. Dislocation interactions with tilt walls. Int. J. Mech. Mater. Des. 2008, 4, 399–406. [Google Scholar] [CrossRef]
- Akarapu, S.; Zbib, H.; Hirth, J.P. Modeling and analysis of disconnections in tilt walls. Scr. Mater. 2008, 59, 265–267. [Google Scholar] [CrossRef]
- Lebensohn, R. Modelling the role of local correlations in polycrystal plasticity using viscoplastic self-consistent schemes. Model. Simul. Mater. Sci. Eng. 1999, 7, 739–746. [Google Scholar] [CrossRef]
- Lebensohn, R.A.; Tomé, C.N.; Maudlin, P.J. A selfconsistent formulation for the prediction of the anisotropic behavior of viscoplastic polycrystals with voids. J. Mech. Phys. Solids 2004, 52, 249–278. [Google Scholar] [CrossRef]
- Orowan, E. Problems of plastic gliding. Proc. Phys. Soc. 1940, 52, 8–22. [Google Scholar] [CrossRef]
- Hochrainer, T.; Sandfeld, S.; Zaiser, M.; Gumbsch, P. Continuum dislocation dynamics: Towards a physical theory of crystal plasticity. J. Mech. Phys. Solids 2014, 63, 167–178. [Google Scholar] [CrossRef]
- Starkey, K.; Winther, G.; El-Azab, A. Theoretical development of continuum dislocation dynamics for finite-deformation crystal plasticity at the mesoscale. J. Mech. Phys. Solids 2020, 139, 103926. [Google Scholar] [CrossRef]
- Li, D.; Zbib, H.; Sun, X.; Khaleel, M. Predicting plastic flow and irradiation hardening of iron single crystal with mechanism-based continuum dislocation dynamics. Int. J. Plast. 2014, 52, 3–17. [Google Scholar] [CrossRef]
- Mura, T. Theory of Inclusions and Inhomogeneities. In Micromechanics of Defects in Solids; Springer: Dordrecht, The Netherlands, 1987; Volume 3, pp. 1–27. [Google Scholar]
- Luster, J.; Morris, M.A. Compatibility of deformation in two-phase Ti-Al alloys: Dependence on microstructure and orientation relationships. Metall. Mater. Trans. A 1995, 26, 1745–1756. [Google Scholar] [CrossRef]
- Bieler, T.R.; Eisenlohr, P.; Zhang, C.; Phukan, H.J.; Crimp, M.A. Grain boundaries and interfaces in slip transfer. Curr. Opin. Solid State Mater. Sci. 2014, 18, 212–226. [Google Scholar] [CrossRef] [Green Version]
- Shi, J.; Zikry, M.A. Modeling of grain boundary transmission, emission, absorption and overall crystalline behavior in ω1, ω3, and ω17b bicrystals. J. Mater. Res. 2011, 26, 1676–1687. [Google Scholar] [CrossRef]
- Lyu, H.; Ruimi, A.; Field, D.P.; Zbib, H.M. Plasticity in Materials with Heterogeneous Microstructures. Metall. Mater. Trans. A Phys. Metall. Mater. Sci. 2016, 47, 6608–6620. [Google Scholar] [CrossRef]
- Quey, R.; Dawson, P.R.; Barbe, F. Large-scale 3D random polycrystals for the finite element method: Generation, meshing and remeshing. Comput. Methods Appl. Mech. Eng. 2011, 200, 1729–1745. [Google Scholar] [CrossRef] [Green Version]
- Krill, C.E.; Chen, L.Q. Computer simulation of 3-D grain growth using a phase-field model. Acta Mater. 2002, 50, 3057–3073. [Google Scholar] [CrossRef]
- Wakai, F.; Enomoto, N.; Ogawa, H. Three-dimensional microstructural evolution in ideal grain growth general statistics. Acta Mater. 2000, 48, 1297–1311. [Google Scholar] [CrossRef]
- He, G.; Liu, F.; Huang, L.; Huang, Z.; Jiang, L. Controlling grain size via dynamic recrystallization in an advanced polycrystalline nickel base superalloy. J. Alloys Compd. 2017, 701, 909–919. [Google Scholar] [CrossRef]
- Moering, J.; Ma, X.; Chen, G.; Miao, P.; Li, G.; Qian, G.; Mathaudhu, S.; Zhu, Y. The role of shear strain on texture and microstructural gradients in low carbon steel processed by Surface Mechanical Attrition Treatment. Scr. Mater. 2015, 108, 100–103. [Google Scholar] [CrossRef] [Green Version]
- Nye, J.F. Some geometrical relations in dislocated crystals. Acta Metall. 1953, 1, 153–162. [Google Scholar] [CrossRef]
- Shizawa, K.; Zbib, H.M. A thermodynamical theory of gradient elastoplasticity with dislocation density tensor. I: Fundamentals. Int. J. Plast. 1999, 15, 899–938. [Google Scholar] [CrossRef]
- Shizawa, K.; Zbib, H.M. A Thermodynamical theory of plastic spin and internal stress with dislocation density tensor. J. Eng. Mater. Technol. Trans. ASME 1999, 121, 247–253. [Google Scholar] [CrossRef]
- Das, S.; Hofmann, F.; Tarleton, E. Consistent determination of geometrically necessary dislocation density from simulations and experiments. Int. J. Plast. 2018, 109, 18–42. [Google Scholar] [CrossRef]
- Rhee, M.; Zbib, H.M.; Hirth, J.P.; Huang, H.; De La Rubia, T. Models for long-/short-range interactions and cross slip in 3D dislocation simulation of BCC single crystals. Model. Simul. Mater. Sci. Eng. 1998, 6, 467–492. [Google Scholar] [CrossRef]




| Symbol | IF Steel (Unit) |
|---|---|
| c* (Bailey–Hirsh hardening coefficient) | 0.4 |
| τ0 (Internal friction) on [1 1 2] | 11 MPa |
| τ0 (Internal friction) on [1 1 0] | 27.5 MPa |
| τ0 (Internal friction) on [1 2 3] | 25 MPa |
| C11 (Elasticity constant) | 242 GPa |
| C12 (Elasticity constant) | 150 GPa |
| C44 (Elasticity constant) | 112 GPa |
| μ (Shear modulus) | 80 GPa |
| K (Hall–Petch constant) | 0.18 MPa/mm−1/2 |
| v0 (Reference strain rate) | 1 × 10−5 m/s |
| m (Strain rate sensitivity) | 0.05 |
| b (Magnitude of burger vector) | 2.54 Å |
| Rc (Critical radius for annihilation coefficient) | 15 b |
| q1 q2 q3 q4 q5 q6 q7 | 0.02 1.0 0.002 0.002 0.018 0.001 0.1 |
| Ωij (i = 1,48; j = 1,48) (Interaction matrix) | 0.5 |
| Measure 1 (Tensile Strength in MPa/Ductility) | Measure 2 (Tensile Strength in MPa/Ductility) | Measure 3 (Tensile Strength in MPa/Ductility) | Average Value (Tensile Strength in MPa/Ductility) | ||
|---|---|---|---|---|---|
| Texture 1 | T | 428/0.25 | 427/0.26 | 427/0.25 | 427/0.25 |
| C | 430/0.22 | 429/0.22 | 429/0.21 | 429/0.22 | |
| Texture 2 | T | 428/0.25 | 428/0.26 | 429/0.26 | 428/0.26 |
| C | 425/0.22 | 426/0.21 | 425/0.21 | 425/0.21 | |
| Texture 3 | T | 427/0.24 | 427/0.24 | 427/0.24 | 427/0.24 |
| C | 430/0.24 | 431/0.23 | 431/0.23 | 431/0.23 |
| Number of Slip Transmission in the Entire Sample | Number of Slip Transmission in the Transient Region (y > 80 µm and y < 20 µm) | Number of Slip Transmission Which Occurred in the Nano Region (y > 90 µm and y < 10 µm) | Tensile Strength (MPa) | Ductility | |||
|---|---|---|---|---|---|---|---|
| Texture 1 | Homogeneous | T | 82,336 | 31,599 | 15,638 | 266 | 0.55 |
| C homogeneous structure | 100,840 | 38,866 | 19,234 | 255 | 0.45 | ||
| Gradient | T | 1564 | 435 | 19 | 427 | 0.25 | |
| C homogeneous structure | 1736 | 287 | 0 | 429 | 0.22 | ||
| Texture 2 | Homogeneous | T | 79,896 | 31,193 | 15,754 | 266 | 0.55 |
| C homogeneous structure | 110,350 | 43,863 | 23,049 | 255 | 0.45 | ||
| Gradient | T | 1490 | 448 | 13 | 428 | 0.26 | |
| C homogeneous structure | 2036 | 583 | 3 | 425 | 0.21 | ||
| Texture 3 | Homogeneous | T | 80,524 | 32,788 | 16,716 | 266 | 0.55 |
| C homogeneous structure | 101,996 | 41,455 | 22,417 | 255 | 0.45 | ||
| Gradient | T | 1978 | 464 | 55 | 427 | 0.24 | |
| C homogeneous structure | 2196 | 568 | 8 | 431 | 0.23 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lyu, H.; Ruimi, A. Understanding the Plastic Deformation of Gradient Interstitial Free (IF) Steel under Uniaxial Loading Using a Dislocation-Based Multiscale Approach. Crystals 2022, 12, 889. https://doi.org/10.3390/cryst12070889
Lyu H, Ruimi A. Understanding the Plastic Deformation of Gradient Interstitial Free (IF) Steel under Uniaxial Loading Using a Dislocation-Based Multiscale Approach. Crystals. 2022; 12(7):889. https://doi.org/10.3390/cryst12070889
Chicago/Turabian StyleLyu, Hao, and Annie Ruimi. 2022. "Understanding the Plastic Deformation of Gradient Interstitial Free (IF) Steel under Uniaxial Loading Using a Dislocation-Based Multiscale Approach" Crystals 12, no. 7: 889. https://doi.org/10.3390/cryst12070889
APA StyleLyu, H., & Ruimi, A. (2022). Understanding the Plastic Deformation of Gradient Interstitial Free (IF) Steel under Uniaxial Loading Using a Dislocation-Based Multiscale Approach. Crystals, 12(7), 889. https://doi.org/10.3390/cryst12070889





