Optical, Dielectric, and Electrical Properties of Tungsten-Based Materials with the Formula Li(2−x)NaxWO4 (x = 0, 0.5, and 1.5)
Abstract
:1. Introduction
2. Materials and Methods
2.1. Synthesis
2.2. Equipment
3. Results
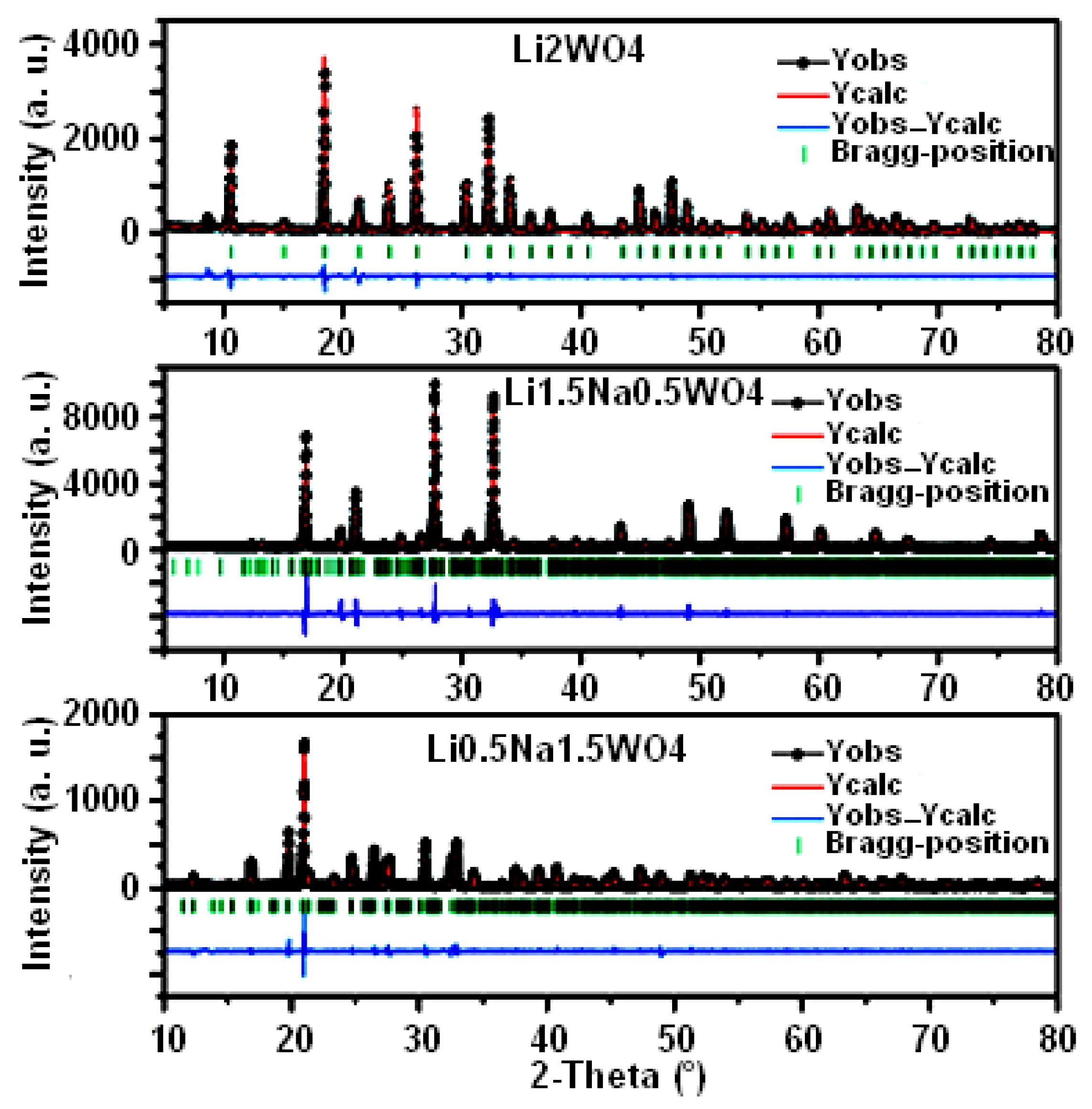
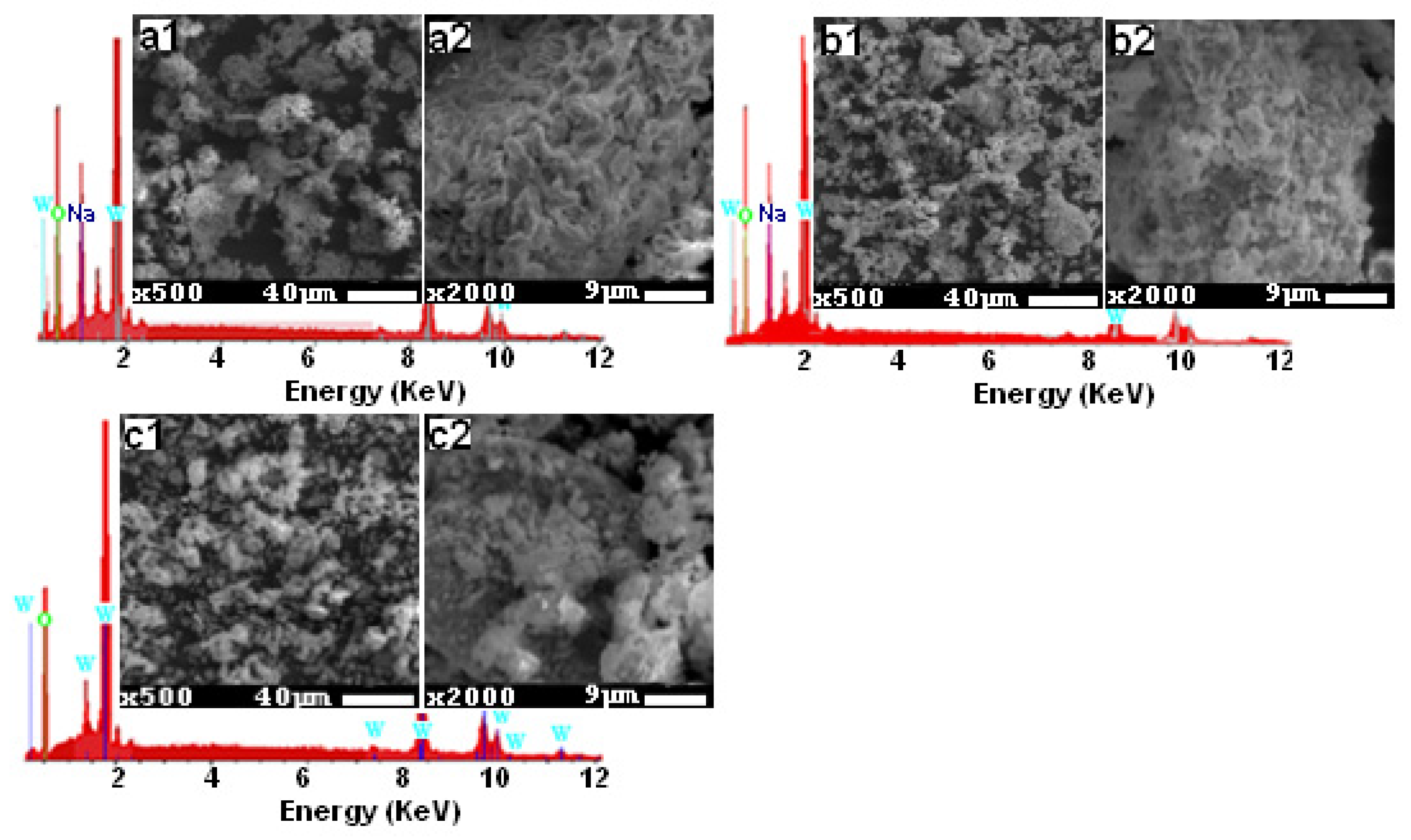
3.1. Structural and Morphological Studies
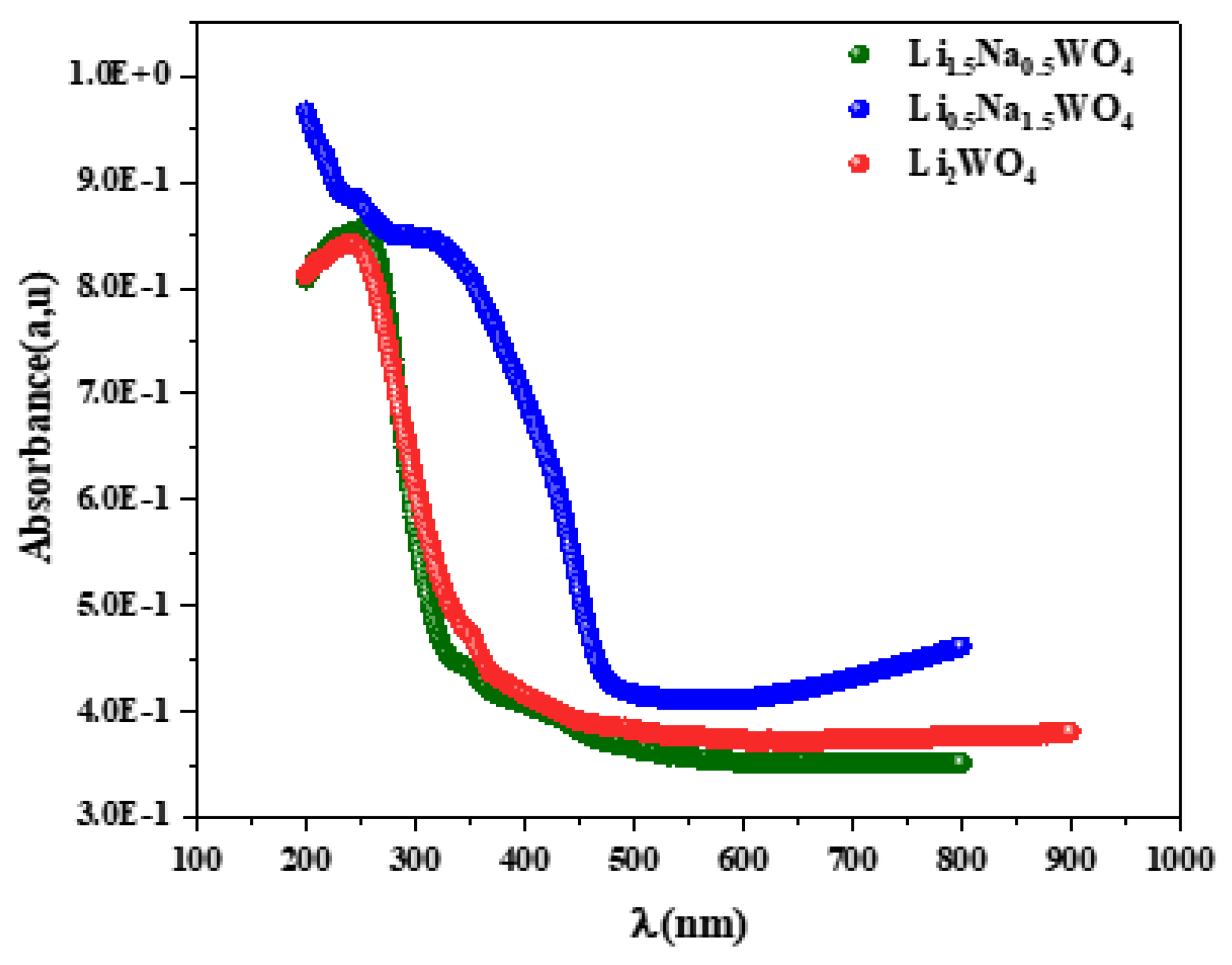
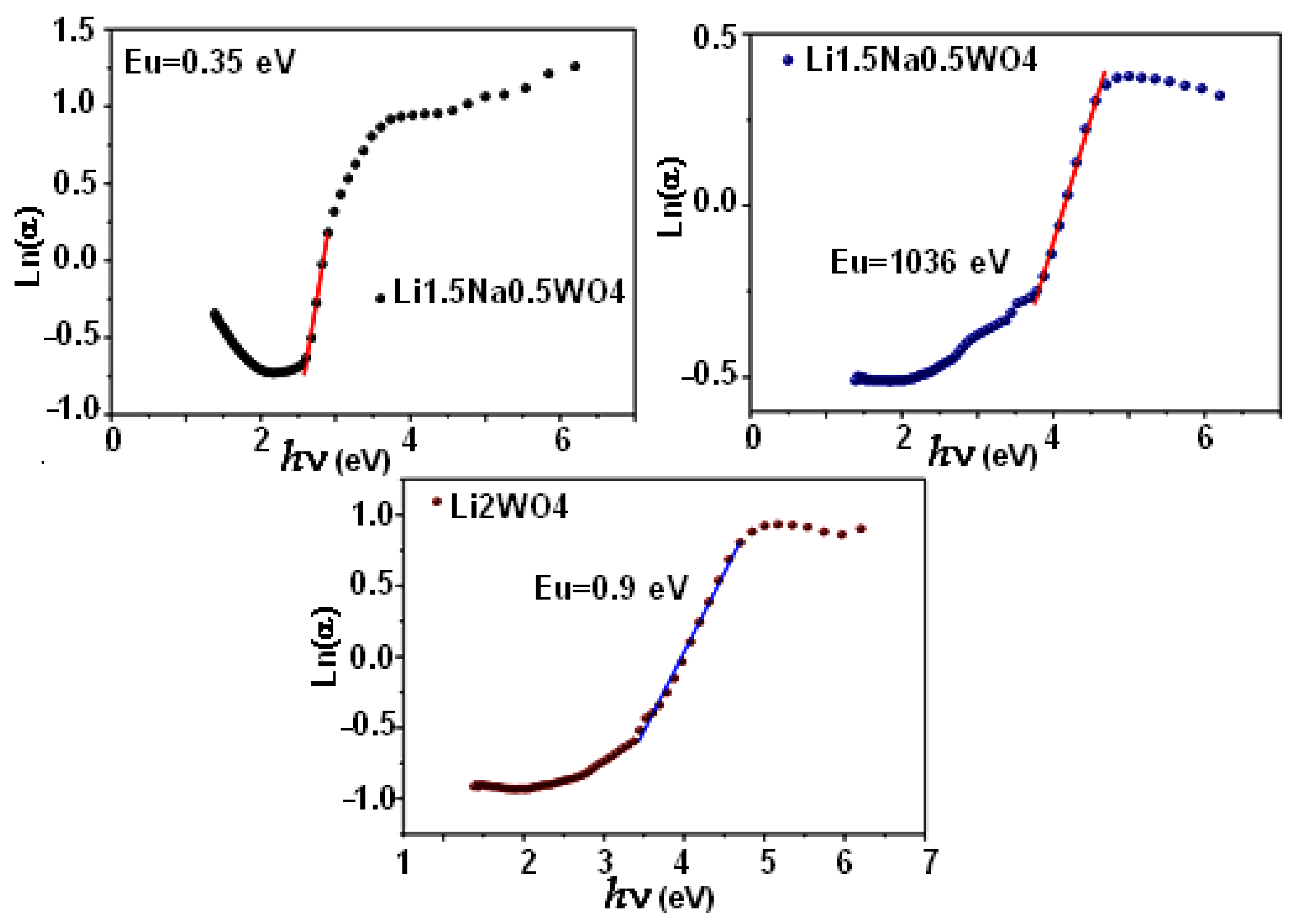
3.2. Optical Characterization
3.3. Thermal Analysis
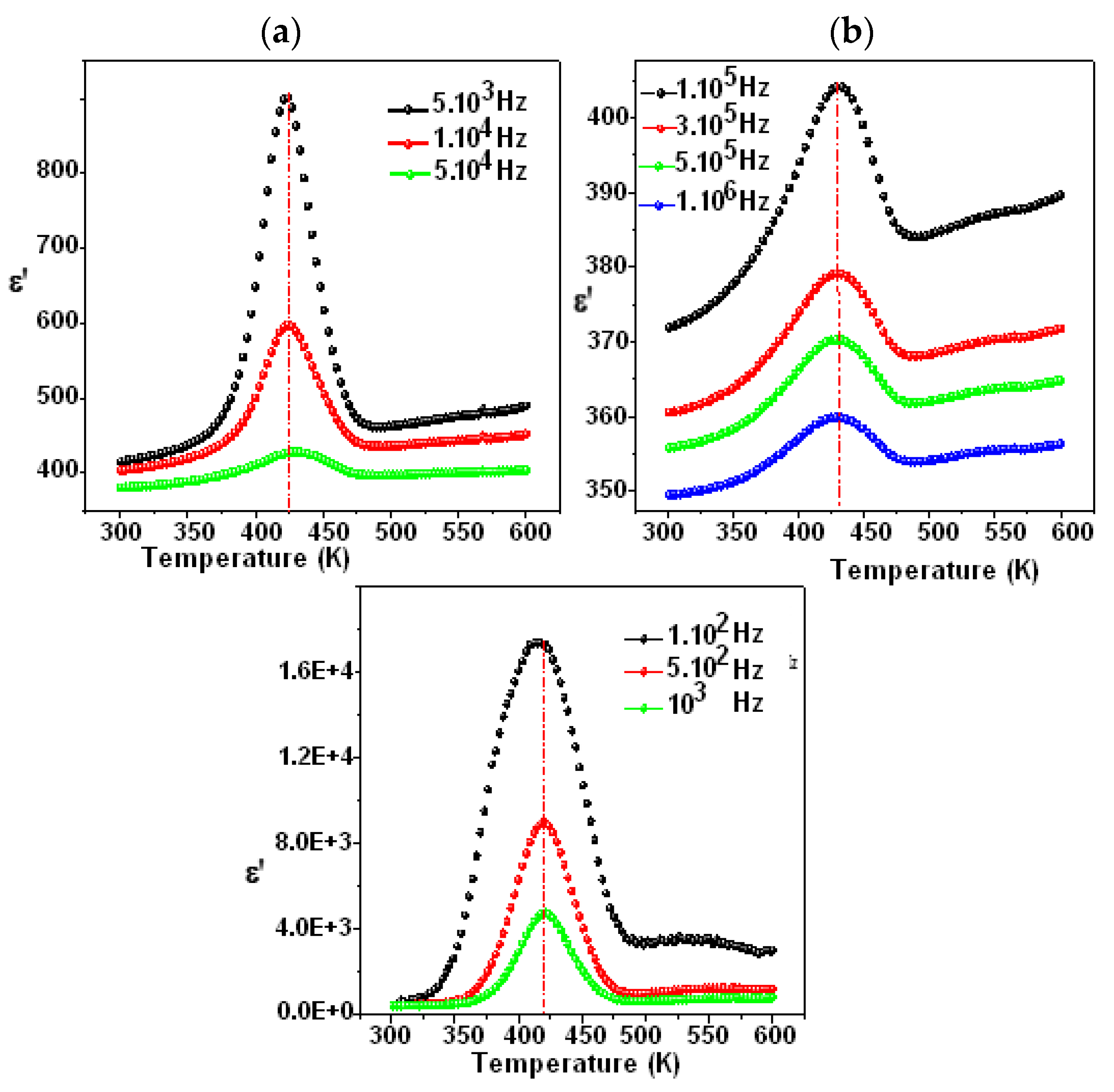
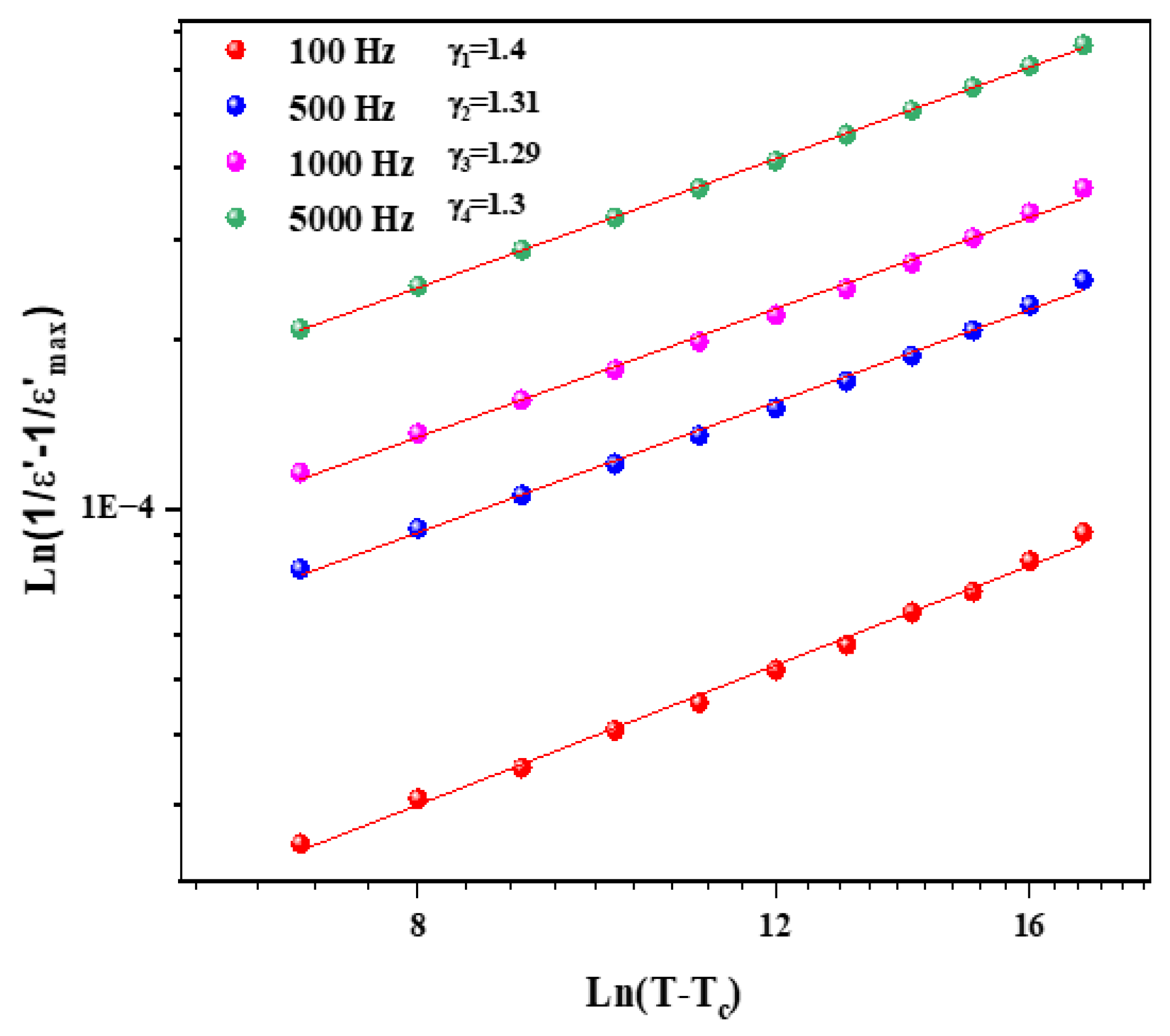
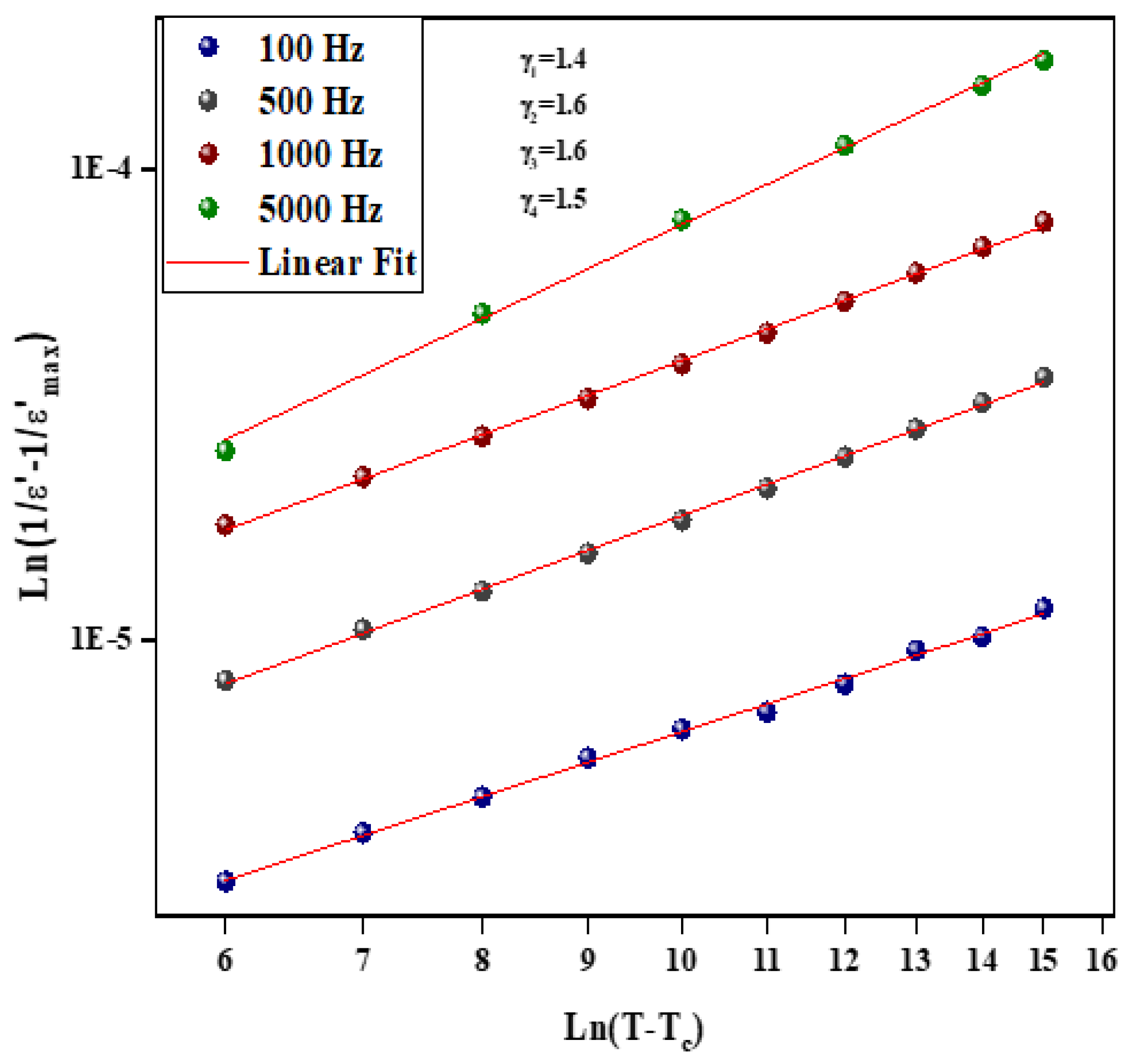
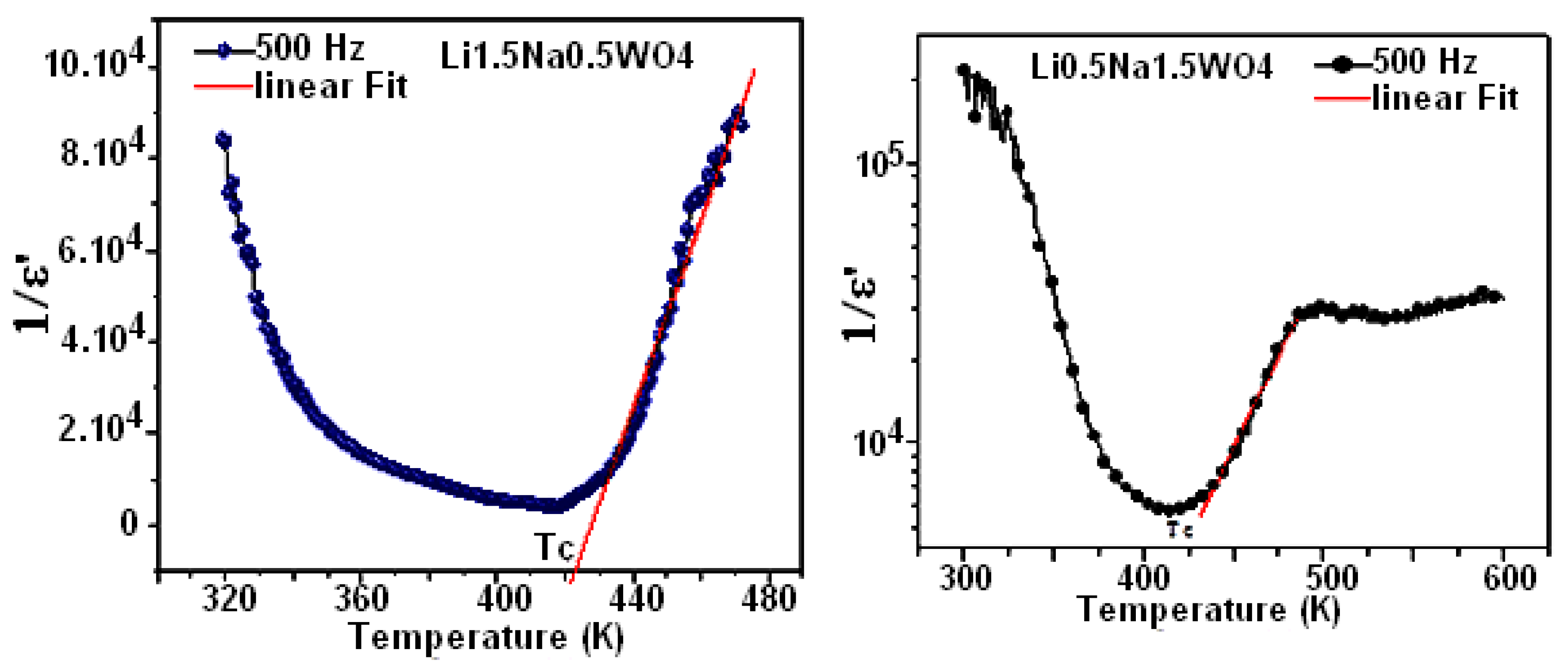
3.4. Dielectric Studies
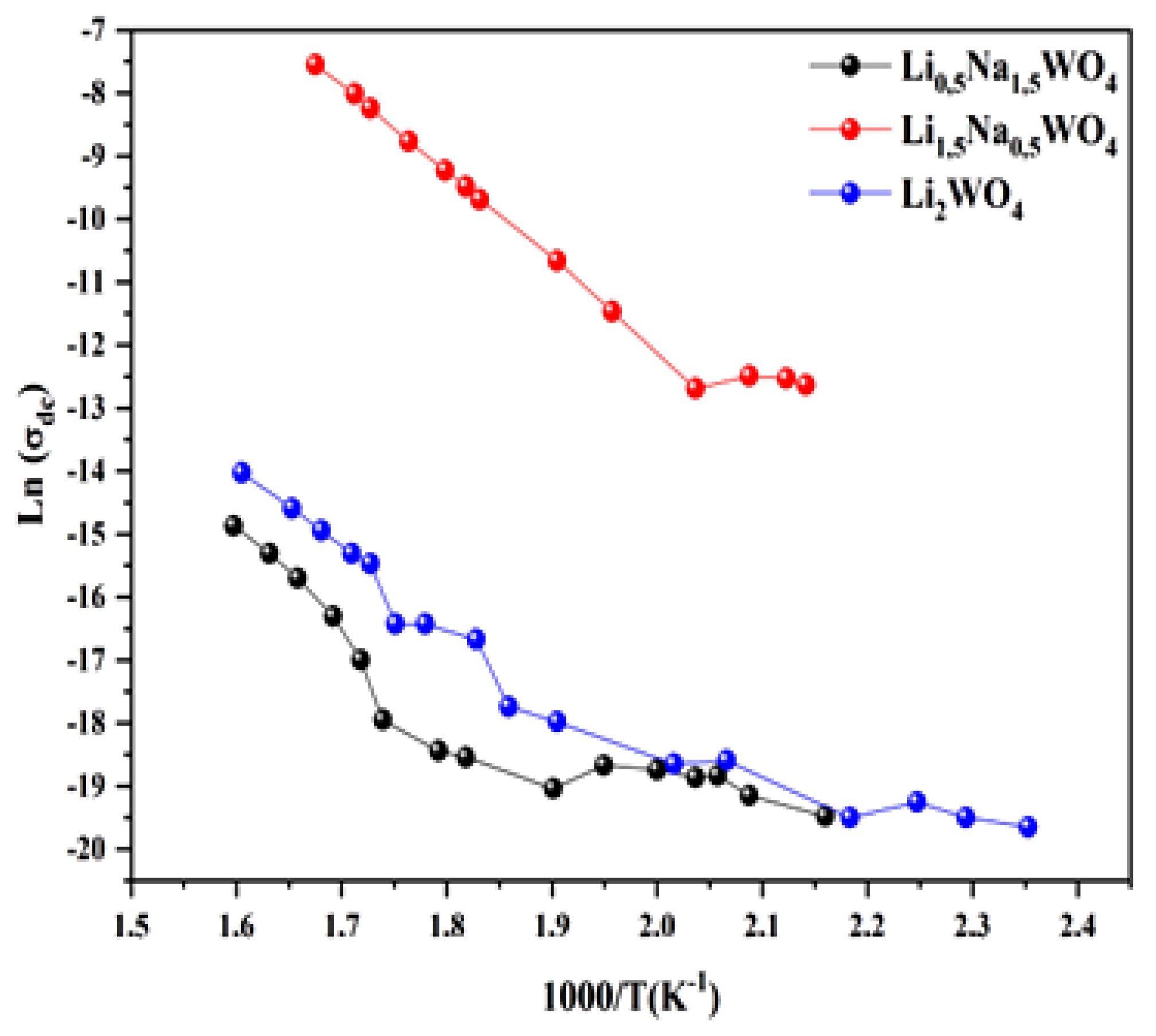
3.5. Conductivity Analysis
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Deb, K.K. Pyroelectric characteristics of (Pb0.9Sm0.1)TiO3 ceramics. Ferroelectrics 1988, 82, 45–53. [Google Scholar] [CrossRef]
- Tandon, R.P.; Singh, R.; Singh, V.; Swani, N.H.; Hans, V.K. Ferroelectric properties of lead titanate/polymer composite and its application in hydrophones. J. Mater. Sci. Lett. 1992, 11, 883–885. [Google Scholar] [CrossRef]
- Alvarez-Vega, M.; Rodriguez-Carvajal, J.; Reyes-Cardenas, J.G.; Fuentes, A.F.; Amador, U. A Topological Analysis of Void Spaces in Tungstate Frameworks: Assessing Storage Properties for the Environmentally Important Guest Molecules and Ions: CO2, UO2, PuO2, U, Pu, Sr2+, Cs+, CH4, and H2. J. Chem. Mater. 2001, 13, 3871–3875. [Google Scholar]
- Wong, C.P.P.; Lai, C.W.; Lee, K.M. Tungsten-Based Materials for Supercapacitors. In Book Inorganic Nanomaterials for Supercapacitor Design, 1st ed.; CRC Press: Boca Raton, FL, USA, 2020; Volume 11. [Google Scholar] [CrossRef]
- Isupov, V.A. Ferroelectric and Ferroelastic Phase Transitions in Molybdates and Tungstates of Monovalent and Bivalent Elements. Ferroelectrics 2005, 322, 83–114. [Google Scholar] [CrossRef]
- Sharma, S.; Choudhary, R.N.P. Phase transition in Li2WO4. Ferroelectrics 1999, 4, 129–137. [Google Scholar] [CrossRef]
- Besozzi, E.; Dellasega, D.; Pezzoli, A.; Conti, C.; Passoni, M.; Beghi, M.G. Amorphous, ultra-nano- and nano-crystalline tungsten-based coatings grown by Pulsed Laser Deposition: Mechanical characterization by Surface Brillouin Spectroscopy. Mater. Des. 2016, 106, 14–21. [Google Scholar] [CrossRef]
- Sharma, S.; Choudhary, R.N.P.; Shanigrahi, S.R. Structural and electrical properties of Na2WO4 ceramics. J. Mater. Lett. 1999, 40, 134–139. [Google Scholar] [CrossRef]
- Bazarova, Z.G.; Arkhincheyeva, S.I.; Batuyeva, I.S.; Bazarov, B.G.; Tushinova, Y.I.; Bazarova, S.T.; Fyodorov, K.N. Complex Oxide Compounds of Polyvalent Metals: Synthesis, Structure and Properties. Chem. Sustain. Dev. 2000, 8, 135–139. [Google Scholar]
- Nagasaki, T.; Kok, K.; Yahaya, A.H.; Igawa, N.; Noda, K.; Ohno, H. Phase identification and electrical conductivity of Li2WO4 Reviewed. Solid State Ion. 1997, 96, 61–74. [Google Scholar] [CrossRef]
- Busey, R.H.; Keller, O.L., Jr. Structure of the Aqueous Pertechnetate Ion by Raman and Infrared Spectroscopy. Raman and Infrared Spectra of Crystalline KTcO4, KReO4, Na2MoO4, Na2WO4, Na2MoO4·2H2O, and Na2WO4·2H2O. J. Chem. Phys. 1964, 41, 215–225. [Google Scholar] [CrossRef]
- Pal, I.; Agarwal, A.; Sanghi, S.; Shearan, A.; Ahlawat, N. Conductivity and dielectric relaxation in sodium borosulfate glasses. J. Alloys Compd. 2009, 472, 40–45. [Google Scholar] [CrossRef]
- Dyre, J.C. The random free-energy barrier model for ac conduction in disordered solids. J. Appl. Phys. 1988, 64, 2456–2468. [Google Scholar] [CrossRef]
- Karoui, K.; Rhaiem, A.B.; Jomni, F.; Moneger, J.L.; Bulou, A.; Guidara, K. Characterization of phase transitions of [N(CH3)4]2ZnCl2Br2 mixed crystals. J. Mol. Struct. 2013, 1048, 287–294. [Google Scholar] [CrossRef]
- Huebner, J.S.; Dillenburg, R.G. Impedance spectra of hot, dry silicate minerals and rock: Qualitative interpretation of spectra. J. Am. Mineral. 1995, 80, 46–64. [Google Scholar] [CrossRef]
- Imran, M.M.A.; Lafi, O.A. Electrical conductivity, density of states and optical band gap in Se90Te6Sn4 glassy semiconductor. Physica B 2013, 410, 201–205. [Google Scholar] [CrossRef]
- Bhowmik, R.N.; Vijayasri, G. Study of microstructure and semiconductor to metallic conductivity transition in solid state sintered Li0.5Mn0.5Fe2O4−δ spinel ferrite. J. Appl. Phys. 2013, 114, 223701. [Google Scholar] [CrossRef]
- Bhowmik, R.N.; Aneesh Kumar, K.S. Role of pH value during material synthesis and grain-grain boundary contribution on the observed semiconductor to metal like conductivity transition in Ni1.5Fe1.5O4 spinel ferrite. Mater. Chem. Phys. 2016, 177, 417–428. [Google Scholar] [CrossRef]
- Saraswat, V.K.; Singh, K.; Saxena, N.S.; Kishore, V.; Sharma, T.P.; Saraswat, P.K. Composition dependence of the electrical conductivity of Se85−xTe15Sbx (x = 2, 4, 6, 8 and 10) glass at room temperature. Curr. Appl. Phys. 2006, 6, 14–18. [Google Scholar] [CrossRef]
- Pradhan, S.K.; Kalidoss, J.; Barik, R.; Sivaiah, B.; Dhar, A.; Bajpai, S. Development of high density tungsten based scandate by Spark Plasma Sintering for the application in microwave tube devices. Int. J. Refract. Metals Hard Mater. 2016, 61, 215–224. [Google Scholar] [CrossRef]
- Namikawa, H. Characterization of the diffusion process in oxide glasses based on the correlation between electric conduction and dielectric relaxation. J. Non-Cryst. Solids 1975, 18, 173–195. [Google Scholar] [CrossRef]
- Gudmundsson, J.T.; Svavarsson, H.G.; Gudjonsson, S.; Gislason, H.P. Frequency-dependent conductivity in lithium-diffused and annealed GaAs. Phys. B Condens. Matter 2003, 340–342, 324–328. [Google Scholar] [CrossRef]
- van den Berg, A.J.; Tuinstra, F.; Warczewski, J. Modulated structures of some alkali molybdates and tungstates. Acta Cryst. B 1973, 29, 586–589. [Google Scholar] [CrossRef]
- Mollah, S.; Som, K.K.; Chaudri, K.B. AC conductivity in Bi4Sr3Ca3CuyOx (y=0–5) and Bi4Sr3Ca3−zLizCu4Ox (z=0.1–1.0) semiconducting oxide glasses. J. Appl. Phys. 1993, 74, 931–937. [Google Scholar] [CrossRef]
- Hayashi, T.; Okada, J.; Toda, E.; Kuzuo, R.; Matsuda, Y.; Kuwata, N.; Kawamura, J. Electrochemical effect of lithium tungsten oxide modification on LiCoO2 thin film electrode. J. Power Sources 2015, 285, 559–567. [Google Scholar] [CrossRef]
- Radzikhovskaya, M.A.; Garkushin, I.K.; Danilushikina, E.G. Ternary systems LiBr-Li2MoO4-Li2WO4 and LiF-Li2MoO4-Li2WO4. Russ. J. Inorg Chem. 2012, 57, 1616–1620. [Google Scholar] [CrossRef]
- Barinova, O.; Sadovskiy, A.; Ermochenkov, I.; Kirsanova, S.; Khomyakov, A.; Zykova, M.; Kuchuk, Z.; Avetissov, I. Solid solution Li2MoO4 – Li2WO4 crystal growth and characterization. Cryst. Growth 2017, 468, 365–368. [Google Scholar] [CrossRef]
- Dkhilalli, F.; Megdiche, S.; Guidara, K.; Rasheed, M.; Barillé, R.; Megdiche, M. AC conductivity evolution in bulk and grain boundary response of sodium tungstate Na2WO4. Ionics 2017, 24, 169–180. [Google Scholar] [CrossRef]
- Johan, M.R.; Han, T.K.; Arof, A.K. Growth and sintering effects of hydrated polycrystalline Li2WO4. Ionics 2010, 16, 323–333. [Google Scholar] [CrossRef]
- Luz Lima, C.; Saraiva, G.D.; Freire, P.T.C.; Maczka, M.; Paraguassu, W.; de Sousa, F.F.; Filho, J.M. Temperature-induced phase transformations in Na2WO4 and Na2MoO4 crystals. Raman Spectrosc. 2011, 42, 799–802. [Google Scholar] [CrossRef]
- Zhou, D.; Randall, C.A.; Pang, L.-X.; Wang, H.; Guo, J.; Zhang, G.-Q.; Wu, X.-G.; Shui, L.; Yao, X. Microwave Dielectric Properties of Li2WO4 Ceramic with Ultra-Low Sintering Temperature. J. Am. Ceram. Soc. 2011, 94, 348–350. [Google Scholar] [CrossRef]
- Bárbara, F.; Joséa, E. Antiferromagnetic and Ferroelectric Phase Transitions and Instabilities in PFW-PT Multiferroic Solid Solution Characterized by Anelastic Measurement. Ferroelectrics 2013, 448, 86–95. [Google Scholar]
- Urusova, M.A.; Valyashko, V.M. High-temperature equilibria and critical phenomena in the Na2CO3-NaCl-H2O and Na2CO3-Na2WO4-H2O systems. Russ. J. Inorg. Chem. 2011, 56, 430–441. [Google Scholar] [CrossRef]
- Krimi, M.; Karoui, K.; Sunol, J.J.; Rhaiem, A.B. Phase transition, impedance spectroscopy and conduction mechanism of Li0.5Na1.5WO4 material. Phys. E Low-Dimens. Syst. Nanostruct. 2018, 102, 137–145. [Google Scholar] [CrossRef]
- Krimi, M.; Karoui, K.; Suñol, J.J.; Rhaiem, A.B. Optical and electrical properties of Li2WO4 compound. Phase Trans. 2019, 92, 737–754. [Google Scholar] [CrossRef]
- Mallah, A.; Al-Thuwayb, F.; Khitouni, M.; Alsawi, A.; Suñol, J.J.; Greneche, J.-M.; Almoneef, M.M. Synthesis, Structural and Magnetic Characterization of Superparamagnetic Ni0.3Zn0.7Cr2−xFexO4 Oxides Obtained by Sol-Gel Method. Crystals 2023, 13, 894. [Google Scholar] [CrossRef]
- Warren, B.E. X-ray Diffraction; Dover: New York, NY, USA, 1990; pp. 251–275. [Google Scholar]
- Williamson, G.K.; Hall, W.H. X-ray line broadening from filed aluminium and wolfram. Acta Metall. 1953, 1, 22–31. [Google Scholar] [CrossRef]
- Kuschke, W.M.; Keller, R.M.; Grahle, P.; Mason, R.; Arzt, E. Mechanisms of Powder Milling Investigated by X-ray Diffraction and Quantitative Metallography. Z. Metallkd. 1995, 86, 804–813. [Google Scholar] [CrossRef]
- Aleksandrov, I.V.; Valiev, R.Z. Studies of Nanocrystalline Materials by X-ray Diffraction Techniques. Phys. Met. Metallogr. 1994, 77, 623–629. [Google Scholar]
- Halder, N.C.; Wagner, C.N. Analysis of the Broadening of Powder Pattern Peaks Using Variance, Integral Breadth, and Fourier Coefficients of the Line Profile. Adv. X-ray Anal. 1966, 9, 91–102. [Google Scholar]
- Daly, R.; Khitouni, M.; Kolsi, A.W.; Njah, N. The studies of crystallite size and microstrains in aluminum powder prepared by mechanical milling. Phys. Stat. Solidi C 2006, 3, 3325–3331. [Google Scholar] [CrossRef]
- Khitouni, M.; Kolsi, A.W.; Njah, N. The effects of boron additions on the disordering and crystallite refinement of NI3AI powders during mechanical milling. Ann. Chim. Sci. Matériaux 2003, 28, 17–29. [Google Scholar] [CrossRef]
- Barhoumi, A.; Leroy, G.; Duponchel, B.; Gest, J.; Yang, L.; Waldhoff, N.; Guermazi, S. Aluminum doped ZnO thin films deposited by direct current sputtering: Structural and optical properties. Superlattices Microstruct. 2015, 82, 483–498. [Google Scholar] [CrossRef]
- Kubelka, P.; Munk, F. An article on optics of paint layers. Z. Tech. Phys. 1931, 12, 259–274. [Google Scholar]
- Bougrine, A.; El Hichou, A.; Addou, M.; Ebothé, J.; Kachouane, A.; Troyon, M. Structural, optical and cathodoluminescence characteristics of undoped and tin-doped ZnO thin films prepared by spray pyrolysis. J. Mater. Chem. Phys. 2003, 80, 438–445. [Google Scholar] [CrossRef]
- Enneffati, M.; Maaloul, N.K.; Louati, B.; Guidara, K. Synthesis, vibrational and UV–visible studies of sodium cadmium orthophosphate. Opt. Quantum Electron. 2017, 49, 331. [Google Scholar] [CrossRef]
- Ben Nasr, W.; Karoui, K.; Bulou, A.; Ben Rhaiem, A. Li1.5Rb0.5MoO4: Ferroelectric properties and characterization of phase transitions by Raman spectroscopy. Phys. E Low-Dimens. Syst. Nanostruct. 2017, 93, 339–344. [Google Scholar] [CrossRef]
- Uchino, K.; Nomura, S. Critical Exponents of the Dielectric Constants in Diffused-Phase-Transition Crystals. Ferroelectr. Lett. Sect. 1982, 44, 55–61. [Google Scholar] [CrossRef]
- Viehland, D.; Wuttig, M.; Cross, L.E. The glassy behavior of relaxor ferroelectrics. Ferroelectrics 2011, 120, 71–77. [Google Scholar] [CrossRef]
- Tan, Y.-Q.; Yuan, Y.; Hao, Y.-M.; Dong, S.-Y.; Yang, Y.-W. Structure and dielectric properties of Ba5NdCu1.5Nb8.5O30−δ tungsten bronze ceramics. Mater. Res. Bull. 2013, 48, 1934–1938. [Google Scholar] [CrossRef]
- Rhaiem, A.; Jomni, F.; Karoui, K.; Guidara, K. Ferroelectric properties of the [N(CH3)4]2CoCl2Br2 compound. J. Mol. Struct. 2013, 1035, 140–144. [Google Scholar] [CrossRef]
- Hajji, R.; Oueslati, A.; Hajlaoui, F.; Bulou, A.; Hlel, F. Structural characterization, thermal, ac conductivity and dielectric properties of (C7H12N2)2[SnCl6]Cl2·1.5H2O. Phase Trans. 2016, 89, 523–542. [Google Scholar] [CrossRef]
- Long, A.R. Frequency-Dependent Loss in Amorphous Semiconductors. J. Adv. Phys. 1982, 31, 553–637. [Google Scholar] [CrossRef]
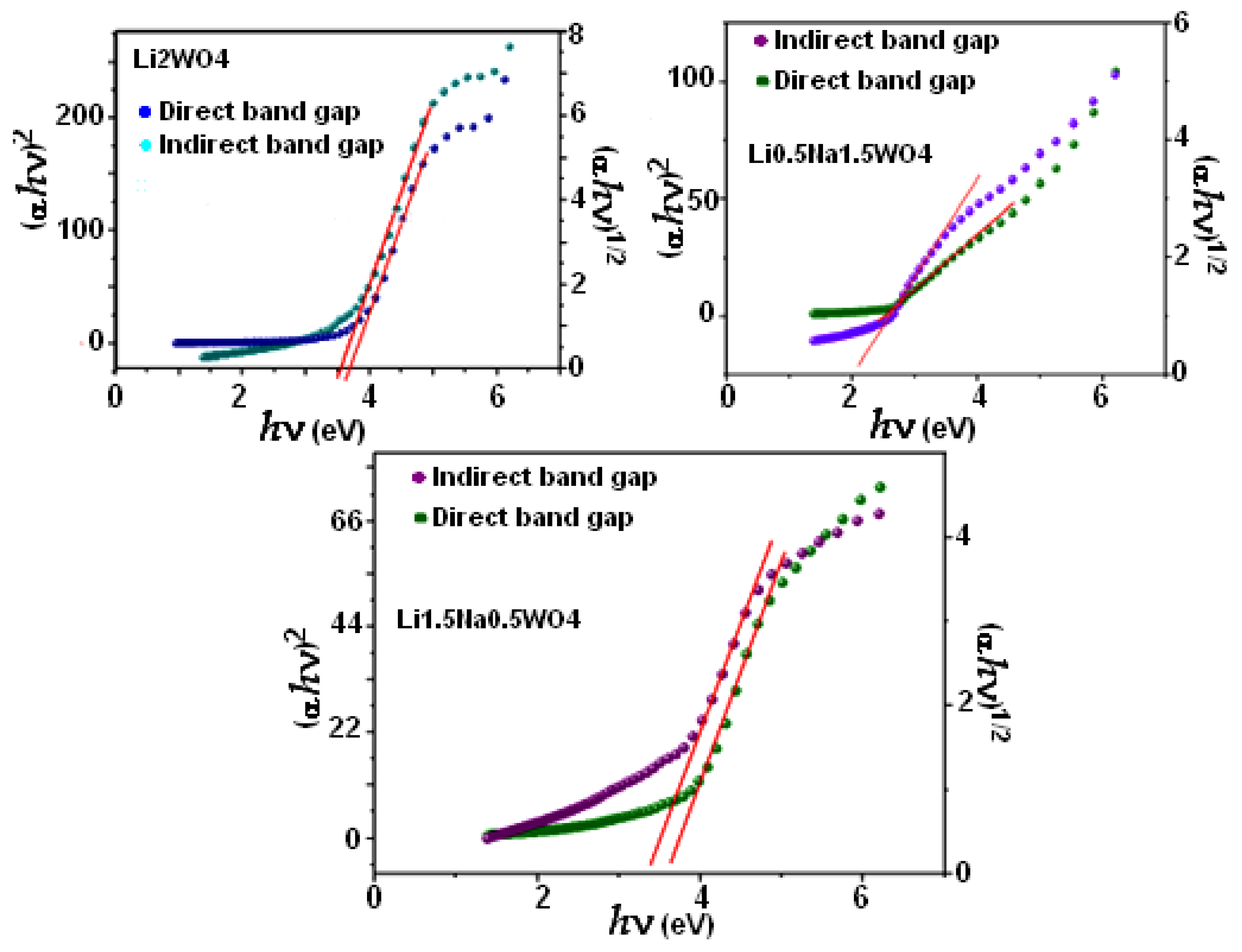

| Sample | Direct Gap (eV) | Indirect Gap (eV) |
|---|---|---|
| Li2WO4 | 4.1 | 3.5 |
| Li1.5Na0.5WO4 | 3.71 | 3.4 |
| Li0.5Na1.5WO4 | 2.6 | 2.1 |
| Sample | Li0.5Na1.5WO4 | Li1.5Na0.5WO4 | Li2WO4 |
|---|---|---|---|
| Density of localized states | 0.6 × 1020–6 × 1020 | 1.1022–2.1024 | 1.1022–9.1023 |
| Value of Urbach energy | 0.35 (eV) | 1.36 (eV) | 0.9 (eV) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Krimi, M.; Al-Harbi, M.H.; Alsulami, A.H.; Karoui, K.; Khitouni, M.; Ben Rhaiem, A. Optical, Dielectric, and Electrical Properties of Tungsten-Based Materials with the Formula Li(2−x)NaxWO4 (x = 0, 0.5, and 1.5). Crystals 2023, 13, 1649. https://doi.org/10.3390/cryst13121649
Krimi M, Al-Harbi MH, Alsulami AH, Karoui K, Khitouni M, Ben Rhaiem A. Optical, Dielectric, and Electrical Properties of Tungsten-Based Materials with the Formula Li(2−x)NaxWO4 (x = 0, 0.5, and 1.5). Crystals. 2023; 13(12):1649. https://doi.org/10.3390/cryst13121649
Chicago/Turabian StyleKrimi, Moufida, Mohammed H. Al-Harbi, Abdulelah H. Alsulami, Karim Karoui, Mohamed Khitouni, and Abdallah Ben Rhaiem. 2023. "Optical, Dielectric, and Electrical Properties of Tungsten-Based Materials with the Formula Li(2−x)NaxWO4 (x = 0, 0.5, and 1.5)" Crystals 13, no. 12: 1649. https://doi.org/10.3390/cryst13121649
APA StyleKrimi, M., Al-Harbi, M. H., Alsulami, A. H., Karoui, K., Khitouni, M., & Ben Rhaiem, A. (2023). Optical, Dielectric, and Electrical Properties of Tungsten-Based Materials with the Formula Li(2−x)NaxWO4 (x = 0, 0.5, and 1.5). Crystals, 13(12), 1649. https://doi.org/10.3390/cryst13121649









