Structural and Improper Ferroelectric Properties of TbInO3 Single Crystal Grown by Laser Floating Zone
Abstract
:1. Introduction
2. Experimental
2.1. Single Crystal Growth
2.2. Characterization
3. Results and Discussion
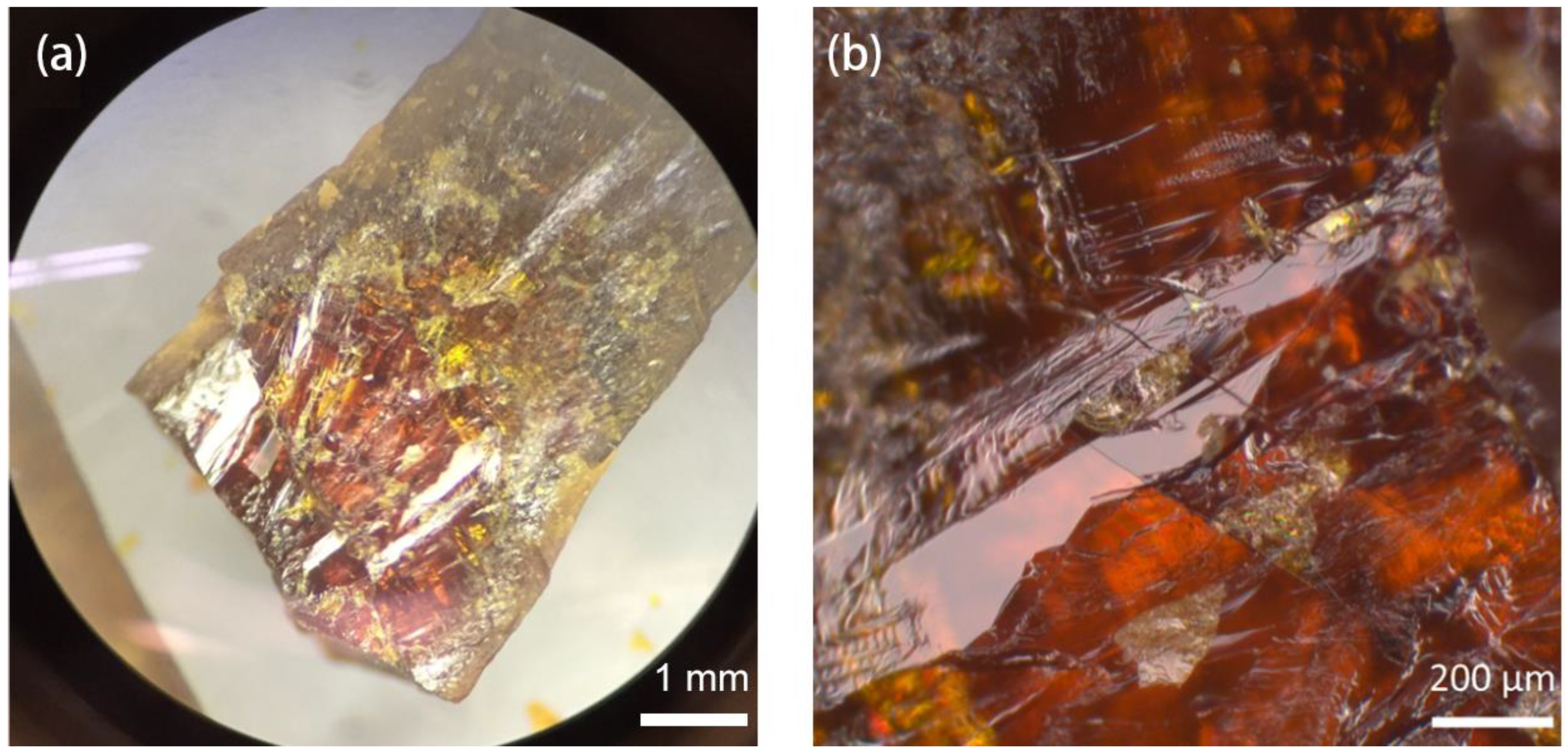
3.1. Crystal Growth
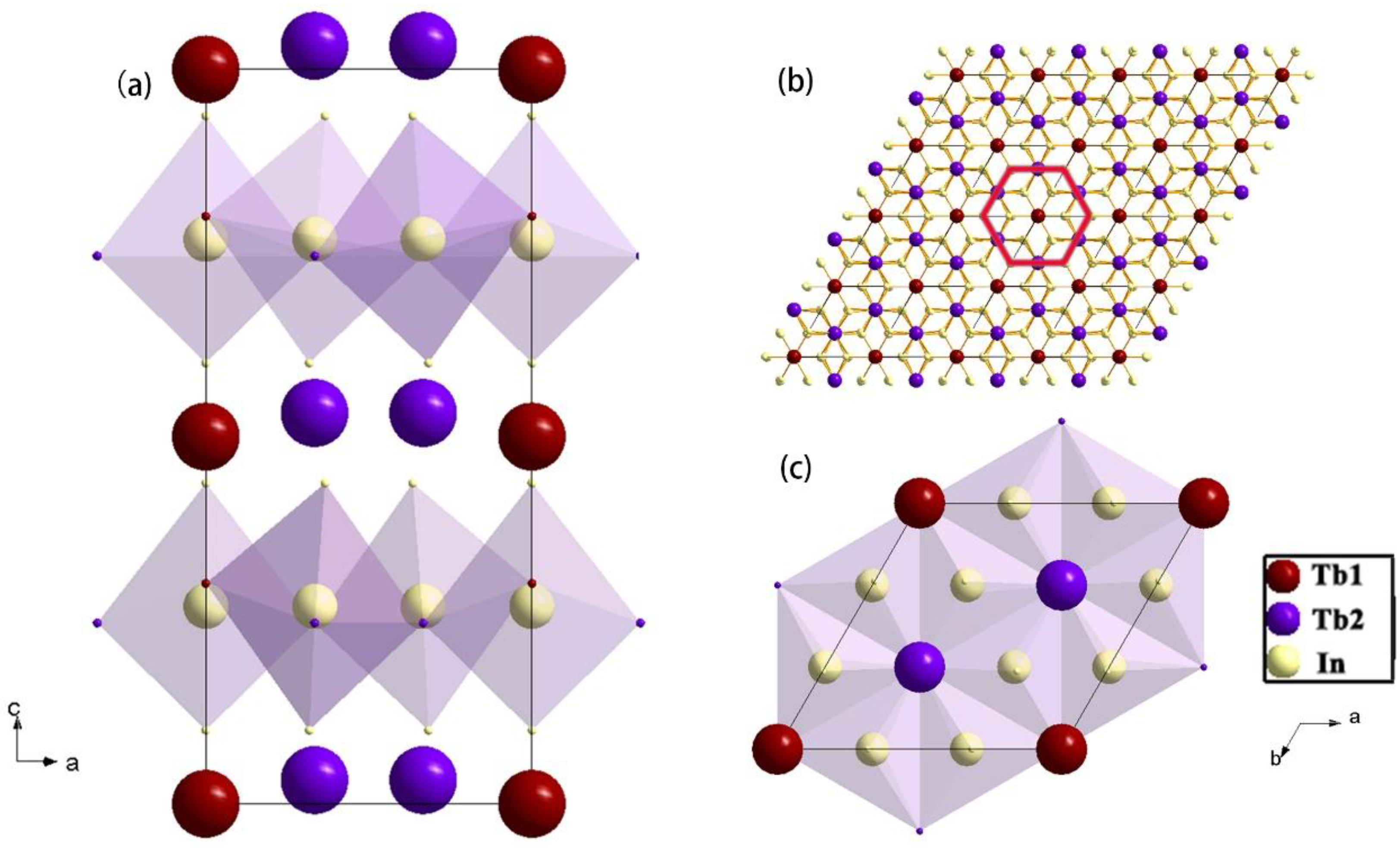
3.2. Crystal Structure and Laue Measurements
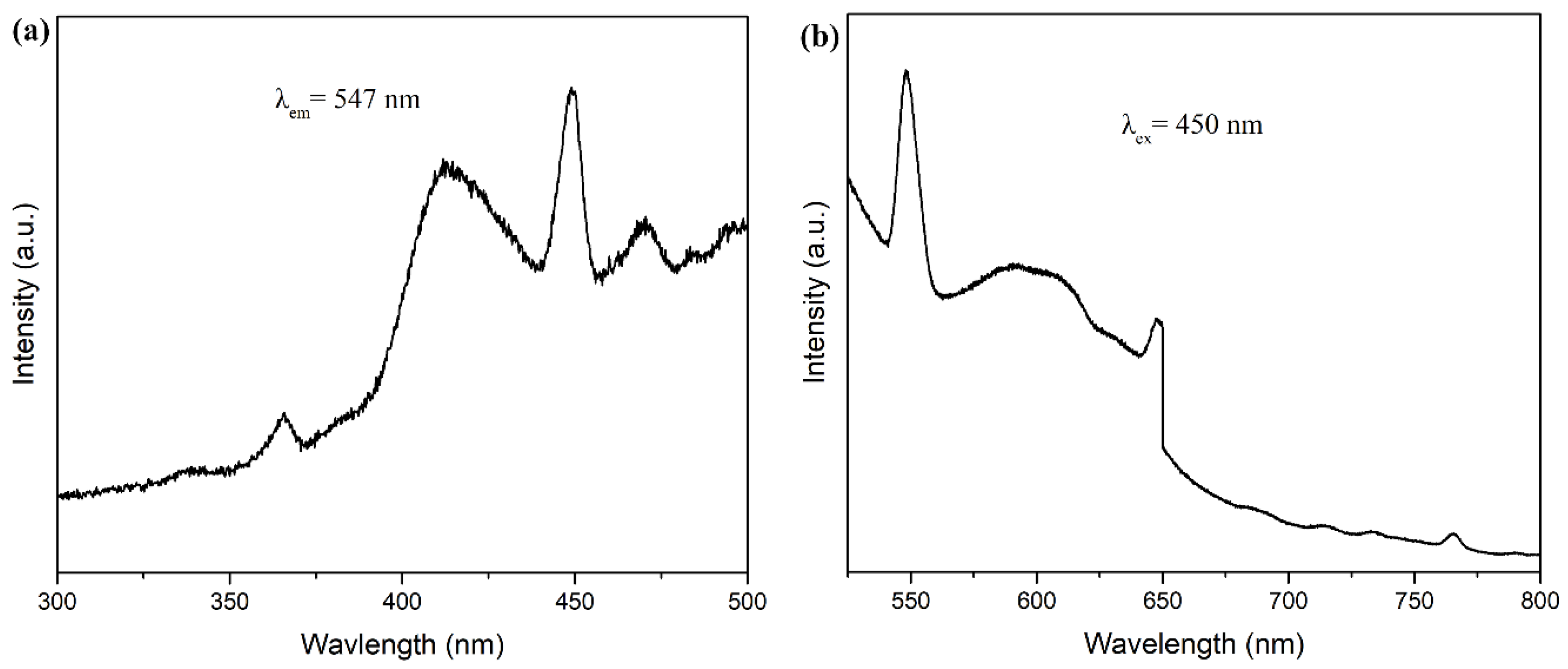
3.3. PL and PLE Spectra
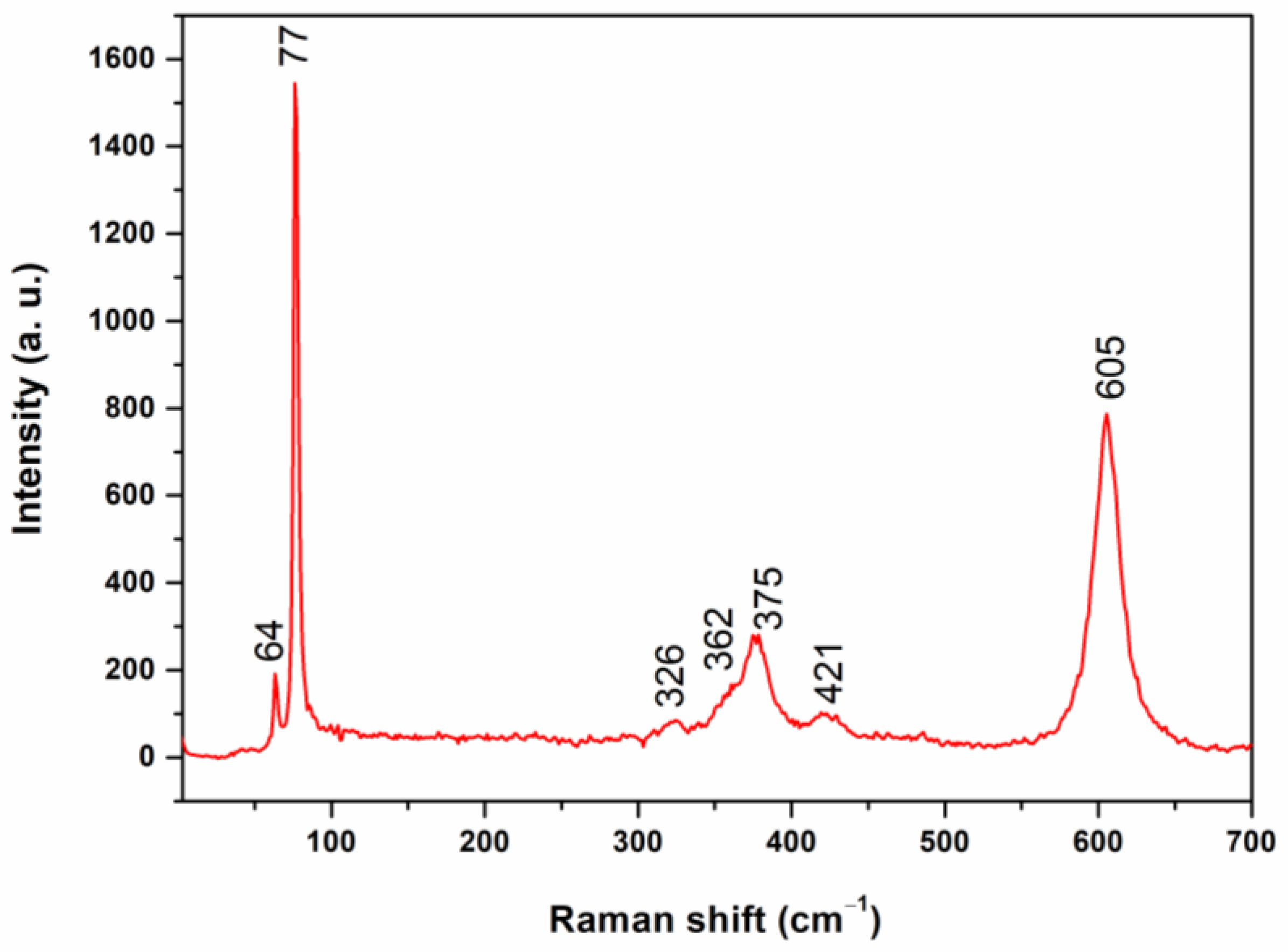
3.4. Raman Spectrum
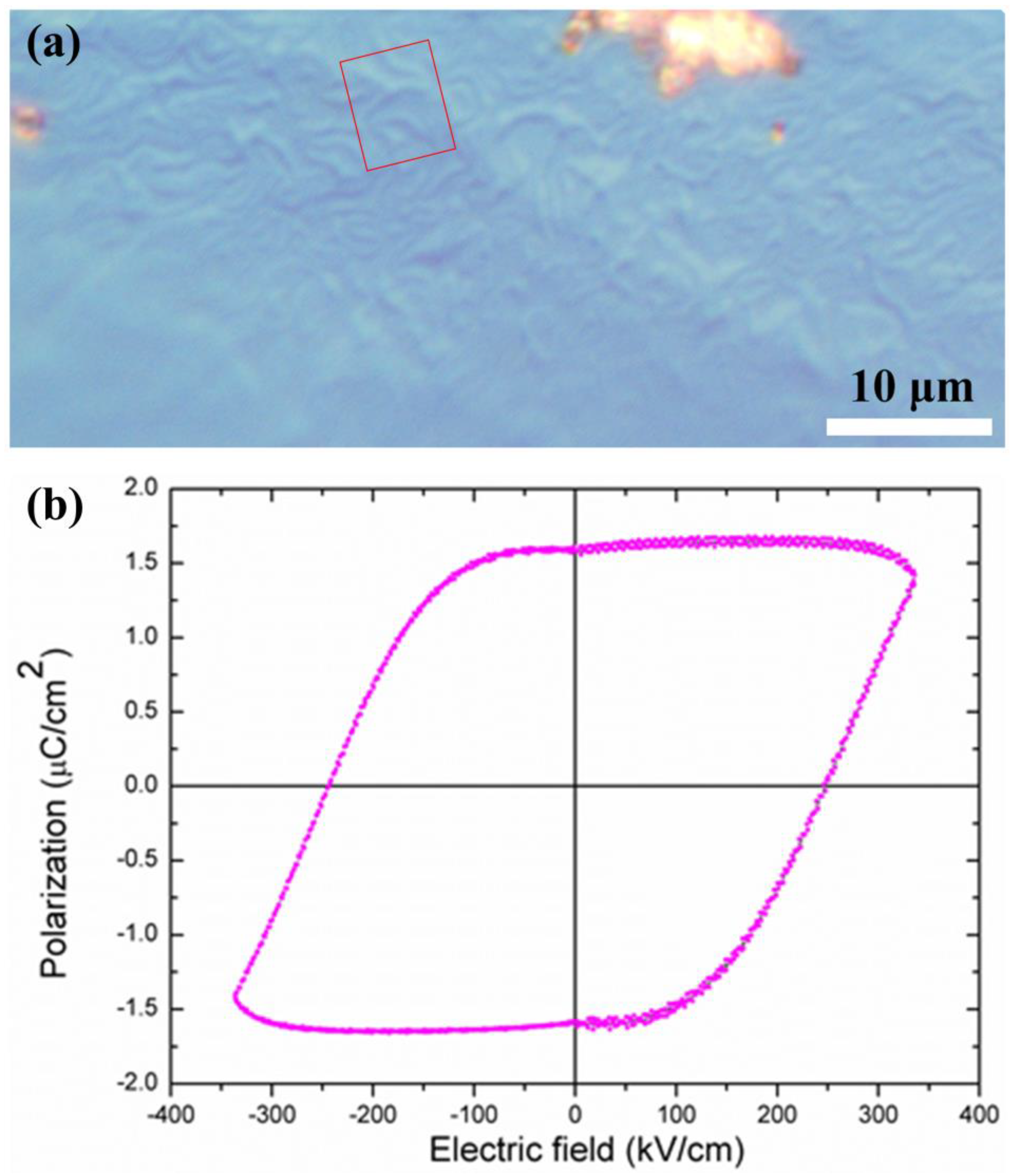
3.5. Ferroelectric Properties
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Stein, J.; Biesenkamp, S.; Cronert, T.; Frohlich, T.; Leist, J.; Schmalzl, K.; Komarek, A.C.; Braden, M. Combined Arrhenius-Merz Law Describing Domain Relaxation in Type-II Multiferroics. Phys. Rev. Lett. 2021, 127, 097601. [Google Scholar] [CrossRef] [PubMed]
- Kim, J.; Wang, X.; Huang, F.T.; Wang, Y.; Fang, X.; Luo, X.; Li, Y.; Wu, M.; Mori, S.; Kwok, D.; et al. Spin Liquid State and Topological Structural Defects in Hexagonal TbInO3. Phys. Rev. X 2019, 9, 031005. [Google Scholar] [CrossRef]
- Manz, S.; Matsubara, M.; Lottermoser, T.; Büchi, J.; Iyama, A.; Kimura, T.; Meier, D.; Fiebig, M. Reversible Optical Switching of Antiferromagnetism in TbMnO3. Nat. Photonics 2016, 10, 653–656. [Google Scholar] [CrossRef]
- Fiebig, M.; Lottermoser, T.; Meier, D.; Trassin, M. The Evolution of Multiferroics. Nat. Rev. Mater. 2016, 1, 16046. [Google Scholar] [CrossRef]
- Lilienblum, M.; Lottermoser, T.; Manz, S.; Selbach, S.M.; Cano, A.; Fiebig, M. Ferroelectricity in the Multiferroic Hexagonal manganites. Nat. Phys. 2015, 11, 1070–1073. [Google Scholar] [CrossRef]
- Bednorz, J.G.; Müller, K.A. Perovskite-Type Oxides—The New Approach to High-Tcsuperconductivity. Rev. Mod. Phys. 1988, 60, 585–600. [Google Scholar] [CrossRef]
- Moritomo, Y.; Asamitsu, A.; Kuwahara, H.; Tokura, Y. Giant Magnetoresistance of Manganese Oxides with a Layered Perovskite Structure. Nature 1996, 380, 141–144. [Google Scholar] [CrossRef]
- Cohen, R.E. Origin of Ferroelectricity in Perovskite Oxides. Nature 1992, 358, 136–138. [Google Scholar] [CrossRef]
- Cheong, S.-W.; Mostovoy, M. Multiferroics a Magnetic Twist for Ferroelectricity. Nat. Mater. 2007, 6, 13–20. [Google Scholar] [CrossRef]
- Zhang, Y.; Shimada, T.; Kitamura, T.; Wang, J. Ferroelectricity in Ruddlesden-Popper Chalcogenide Perovskites for Photovoltaic Application: The Role of Tolerance Factor. J. Phys. Chem. Lett. 2017, 8, 5834–5839. [Google Scholar] [CrossRef]
- Panwar, N.; Singh, K.; Kanwar, K.; Bitla, Y.; Kumar, S.; Puli, V.S. Low-Temperature Magnetic and Magnetocaloric Properties of Manganese-Substituted Gd0.5Er0.5CrO3 Orthochromites. Crystals 2022, 12, 263. [Google Scholar] [CrossRef]
- Grover, V.; Shukla, R.; Jain, D.; Deshpande, S.K.; Arya, A.; Pillai, C.G.S.; Tyagi, A.K. Complex GdSc1–xInxO3 Oxides: Synthesis and Structure Driven Tunable Electrical Properties. Chem. Mater. 2012, 24, 2186–2196. [Google Scholar] [CrossRef]
- Van Aken, B.B.; Palstra, T.T.; Filippetti, A.; Spaldin, N.A. The Origin of Ferroelectricity in Magnetoelectric YMnO3. Nat. Mater. 2004, 3, 164–170. [Google Scholar] [CrossRef]
- Katsufuji, T.; Masaki, M.; Machida, A.; Moritomo, M.; Kato, K.; Nishibori, E.; Takata, M.; Sakata, M.; Ohoyama, K.; Kitazawa, K.; et al. Crystal Structure and Magnetic Properties of Hexagonal RMnO3 (R = Y, Lu, and Sc) and the Effect of Doping. Phys. Rev. B 2002, 66, 134434. [Google Scholar] [CrossRef]
- Li, J.; Sleight, A.W.; Subramanian, M.A. Determination of the Local Environment of Mn3+ and In3+ in the YInO3–YMnO3 Solid Solution, Which Exhibits an Intense Blue Color. Chem. Mater. 2016, 28, 6050–6053. [Google Scholar] [CrossRef]
- Kim, M.G.; Winn, B.; Chi, S.; Savici, A.T.; Rodriguez-Rivera, J.A.; Chen, W.C.; Xu, X.; Li, Y.; Kim, J.W.; Cheong, S.W.; et al. Spin-Liquid-Like State in Pure and Mn-Doped TbInO3 with a Nearly Triangular Lattice. Phys. Rev. B 2019, 100, 024405. [Google Scholar] [CrossRef]
- Clark, L.; Sala, G.; Maharaj, D.D.; Stone, M.B.; Knight, K.S.; Telling, M.T.; Wang, X.; Xu, X.; Kim, J.; Li, Y.; et al. Two-Dimensional Spin Liquid Behaviour in the Triangular-Honeycomb Antiferromagnet TbInO3. Nat. Phys. 2019, 15, 262–268. [Google Scholar] [CrossRef]
- Fan, F.; Gao, Z.; Ponet, L.; Wang, J.; Huang, H.; Artyukhin, S.; Hong, J.; Cheong, S.-W.; Wang, X. Visualization of Large-Scale Charged Domain Walls in Hexagonal Manganites. Appl. Phys. Lett. 2021, 118, 072901. [Google Scholar] [CrossRef]
- Wang, X.; Yang, D.; Zhang, H.-M.; Song, C.; Wang, J.; Tan, G.; Zheng, R.; Dong, S.; Cheong, S.-W.; Zhang, J. Anisotropic Resistance Switching in Hexagonal Manganites. Phys. Rev. B 2019, 99, 054106. [Google Scholar] [CrossRef]
- Lin, S.-Z.; Wang, X.; Kamiya, Y.; Chern, G.-W.; Fan, F.; Fan, D.; Casas, B.; Liu, Y.; Kiryukhin, V.; Zurek, W.H.; et al. Topological Defects as Relics of Emergent Continuous Symmetry and Higgs Condensation of disorder in Ferroelectrics. Nat. Phys. 2014, 10, 970–977. [Google Scholar] [CrossRef] [Green Version]
- Geng, Y.; Das, H.; Wysocki, A.L.; Wang, X.; Cheong, S.W.; Mostovoy, M.; Fennie, C.J.; Wu, W. Direct Visualization of Magnetoelectric Domains. Nat. Mater. 2014, 13, 163–167. [Google Scholar] [CrossRef] [PubMed]
- Cichy, K.; Świerczek, K. Influence of Doping on the Transport Properties of Y1−xlnxMnO3+Δ (Ln: Pr, Nd). Crystals 2021, 11, 510. [Google Scholar] [CrossRef]
- Dong, C.; Chen, R.; Liu, Y.; Liu, C.; Zhu, H.; Ke, J.; Liu, W.; Yang, M.; Wang, J. Field-Induced Magnetic Phase Transitions and Rich Phase Diagram of HoMnO3 Single Crystal. Crystals 2019, 9, 419. [Google Scholar] [CrossRef]
- Balli, M.; Roberge, B.; Fournier, P.; Jandl, S. Review of the Magnetocaloric Effect in RMnO3 and RMn2O5 Multiferroic Crystals. Crystals 2017, 7, 44. [Google Scholar] [CrossRef]
- Yin, X.; Li, Y.; Wang, G.; Hu, J.; Xu, C.; Lu, Q.; Zhong, Y.; Zhao, J.; Zhao, X.; Zhang, Y.; et al. Magnetic Properties of the Quasi Two-Dimensional Centered Honeycomb Antiferromagnet GdInO3. Phys. Rev. B 2021, 104, 134432. [Google Scholar] [CrossRef]
- Kaiwart, R.; Dwivedi, A.; Shukla, R.; Velaga, S.; Grover, V.; Poswal, H.K. High Pressure Structural Evolution of Cubic Solid Solution YbInO3. J. Appl. Phys. 2021, 130, 035902. [Google Scholar] [CrossRef]
- Hartley, P.; Egdell, R.G.; Zhang, K.H.L.; Hohmann, M.V.; Piper, L.F.J.; Morgan, D.J.; Scanlon, D.O.; Williamson, B.A.D.; Regoutz, A. Experimental and Theoretical Study of the Electronic Structures of Lanthanide Indium Perovskites LnInO3. J. Phys. Chem. C Nanomater. Interfaces 2021, 125, 6387–6400. [Google Scholar] [CrossRef]
- Yu, Y.; Lin, N.; Wang, H.; Xu, R.; Ren, H.; Zhao, X. First Principles Study of Ferroelectric Hexagonal Compounds RInO3 (R = Dy, Er, and Ho): Electronic Structure, Optical and Dielectric Properties. RSC Adv. 2020, 10, 4080–4086. [Google Scholar] [CrossRef]
- Romanov, A.N.; Haula, E.V.; Shashkin, D.P.; Korchak, V.N. Broadband near-Ir Photoluminescence of Trigonal-Bipyramidal Coordinated Cu2+ Impurity Center in YGaO3, YInO3 and GdInO3 Hexagonal Phases. J. Lumin. 2020, 228, 117652. [Google Scholar] [CrossRef]
- Öztürk, E.; Karacaoglu, E. The Effect of Eu3+-Doping on the Photoluminescent Properties of ReInO3 (Re = Er, Sm) Type Phosphors. Mater. Today Commun. 2020, 25, 101556. [Google Scholar] [CrossRef]
- Ye, M.; Kung, H.H.; Rosa, P.F.S.; Rosenberg, E.; Kim, J.; Xu, X.H.; Bauer, E.D.; Fisk, Z.; Fisher, I.R.; Cheong, S.W.; et al. Crystal-Field Excitations and Quadrupolar Fluctuations of 4f-Electron Systems Studied by Polarized Light Scattering. J. Phys. Conf. Ser. 2022, 2164, 012054. [Google Scholar] [CrossRef]
- Tohei, T.; Moriwake, H.; Murata, H.; Kuwabara, A.; Hashimoto, R.; Yamamoto, T.; Tanaka, I. Geometric Ferroelectricity in Rare-Earth Compounds RGaO3 and RInO3. Phys. Rev. B 2009, 79, 144125. [Google Scholar] [CrossRef]
- Shukla, R.; Grover, V.; Deshpande, S.K.; Jain, D.; Tyagi, A.K. Synthesis and Structural and Electrical Investigations of a Hexagonal Y1−XGdXInO3 (0.0 ≤ x ≤ 1.0) System Obtained Via Metastable C-Type Intermediates. Inorg. Chem. 2013, 52, 13179–13187. [Google Scholar] [CrossRef]
- Shukla, R.; Sayed, F.N.; Grover, V.; Deshpande, S.K.; Guleria, A.; Tyagi, A.K. Quest for Lead Free Relaxors in YIn1−XFeXO3 (0.0 ≤ x ≤ 1.0) System: Role of Synthesis and Structure. Inorg. Chem. 2014, 53, 10101–10111. [Google Scholar] [CrossRef]
- Shukla, R.; Grover, V.; Srinivasu, K.; Paul, B.; Roy, A.; Gupta, R.; Tyagi, A.K. Rare Earth Indates (Re: La–Yb): Influence of the Synthesis Route and Heat Treatment on the Crystal Structure. Dalton Trans. 2018, 47, 6787–6799. [Google Scholar] [CrossRef]
- Li, Y.; Wang, Y.; Tan, W.; Wang, W.; Zhang, J.; Kim, J.W.; Cheong, S.-W.; Tao, X. Laser Floating Zone Growth of Improper Geometric Ferroelectric GdInO3 Single Crystals with Z6 Topological Defects. J. Mater. Chem. C 2018, 6, 7024–7029. [Google Scholar] [CrossRef]
- Wu, Y.; Li, Y.; Zhou, C.; Chen, H.; Cheong, S.-W.; Li, Y.; Tao, X.; Zhang, L. Novel Geometric Ferroelectric EuInO3 Single Crystals with Topological Vortex Domains. Cryst. Growth Des. 2023. [Google Scholar] [CrossRef]
- Savary, L.; Balents, L. Quantum Spin Liquids: A Review. Rep. Prog. Phys. 2017, 80, 016502. [Google Scholar] [CrossRef]
- Zhang, J.; Zhang, Z.; Zhang, W.; Zheng, Q.; Sun, Y.; Zhang, C.; Tao, X. Polymorphism of BaTeMo2O9: A New Polar Polymorph and the Phase Transformation. Chem. Mater. 2011, 23, 3752–3761. [Google Scholar] [CrossRef]
- Chai, Y.S.; Oh, Y.S.; Wang, L.J.; Manivannan, N.; Feng, S.M.; Yang, Y.S.; Yan, L.Q.; Jin, C.Q.; Kim, K.H. Intrinsic Ferroelectric Polarization of Orthorhombic Manganites Withe-Type Spin Order. Phys. Rev. B 2012, 85, 184406. [Google Scholar]
- Feng, S.M.; Chai, Y.S.; Zhu, J.L.; Manivannan, N.; Oh, Y.S.; Wang, L.J.; Yang, Y.S.; Jin, C.Q.; Kim, K.H. Determination of the Intrinsic Ferroelectric Polarization in Orthorhombic HoMnO3. New J. Phys. 2010, 12, 073006. [Google Scholar] [CrossRef] [Green Version]
- Scott, J.F.; Kammerdiner, L.; Parris, M.; Traynor, S.; Ottenbacher, V.; Shawabkeh, A.; Oliver, W. Switching Kinetics of Lead Zirconate Titanate Submicron Thin-Film Memories. J. Appl. Phys. 1988, 64, 787–792. [Google Scholar] [CrossRef]
- Ederer, C.; Spaldin, N.A. A New Route to Magnetic Ferroelectrics. Nat. Mater. 2004, 3, 849–851. [Google Scholar] [CrossRef] [PubMed]
- Zhao, S.; Yu, T.; Wang, Z.; Wang, S.; Wei, L.; Chen, X.; Wang, S. Flux Method Growth of Large Size Group IV–V 2d Gep Single Crystals and Photoresponse Application. Crystals 2021, 11, 235. [Google Scholar] [CrossRef]
- Paul, B.; Chatterjee, S.; Gop, S.; Roy, A.; Grover, V.; Shukla, R.; Tyagi, A.K. Evolution of Lattice Dynamics in Ferroelectric Hexagonal ReInO3 (Re = Ho, Dy, Tb, Gd, Eu, Sm) Perovskites. Mater. Res. Express 2016, 3, 075703. [Google Scholar] [CrossRef]
- Chae, S.C.; Horibe, Y.; Jeong, D.Y.; Rodan, S.; Lee, N.; Cheong, S.W. Self-Organization, Condensation, and Annihilation of Topological Vortices and Antivortices in a Multiferroic. Proc. Natl. Acad. Sci. USA 2010, 107, 21366–21370. [Google Scholar] [CrossRef] [Green Version]


| Formula | TbInO3 |
|---|---|
| FW (g·mol−1) | 321.74 |
| radiation wavelength | Mo Kα 0.71073 Å |
| crystal system | hexagonal |
| space group, Z | P63cm, 6 |
| a (Å) | 6.3088 (1) Å |
| c (Å) | 12.3067 (4) Å |
| V (Å3) | 424.20 (2) Å3 |
| ρcalc (g·cm−3) | 7.557 |
| absorp coeff (mm−1) | 32.71 |
| R(int) | 0.081 |
| GOF on F2 | 1.257 |
| R1 (I > 2σI) a | 0.0415 |
| wR2 (I > 2σI) a | 0.0847 |
| R1 (all data) a | 0.0449 |
| wR2 (all data) a | 0.0885 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Min, C.; Wu, Y.; Li, Y.; Zhou, C.; Cheong, S.-W.; Lu, X.; Zhou, T.; Zhang, L. Structural and Improper Ferroelectric Properties of TbInO3 Single Crystal Grown by Laser Floating Zone. Crystals 2023, 13, 287. https://doi.org/10.3390/cryst13020287
Min C, Wu Y, Li Y, Zhou C, Cheong S-W, Lu X, Zhou T, Zhang L. Structural and Improper Ferroelectric Properties of TbInO3 Single Crystal Grown by Laser Floating Zone. Crystals. 2023; 13(2):287. https://doi.org/10.3390/cryst13020287
Chicago/Turabian StyleMin, Chang, You Wu, Yanbin Li, Chunming Zhou, Sang-Wook Cheong, Xiaosong Lu, Tianyuan Zhou, and Le Zhang. 2023. "Structural and Improper Ferroelectric Properties of TbInO3 Single Crystal Grown by Laser Floating Zone" Crystals 13, no. 2: 287. https://doi.org/10.3390/cryst13020287
APA StyleMin, C., Wu, Y., Li, Y., Zhou, C., Cheong, S.-W., Lu, X., Zhou, T., & Zhang, L. (2023). Structural and Improper Ferroelectric Properties of TbInO3 Single Crystal Grown by Laser Floating Zone. Crystals, 13(2), 287. https://doi.org/10.3390/cryst13020287






