Energy-Composition Relations in Ni3(Al1−xXx) Phases
Abstract
:1. Introduction
2. Methods
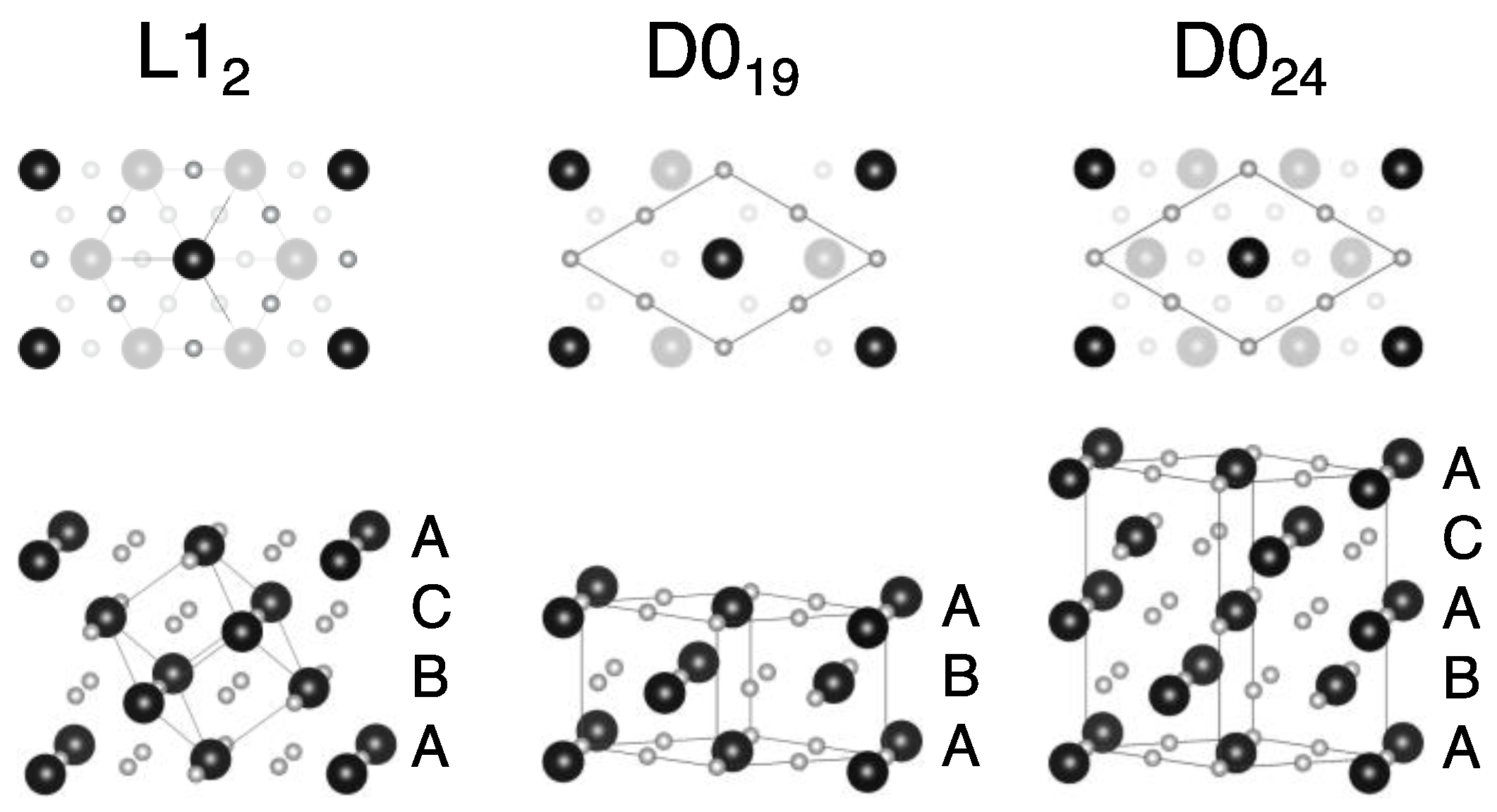
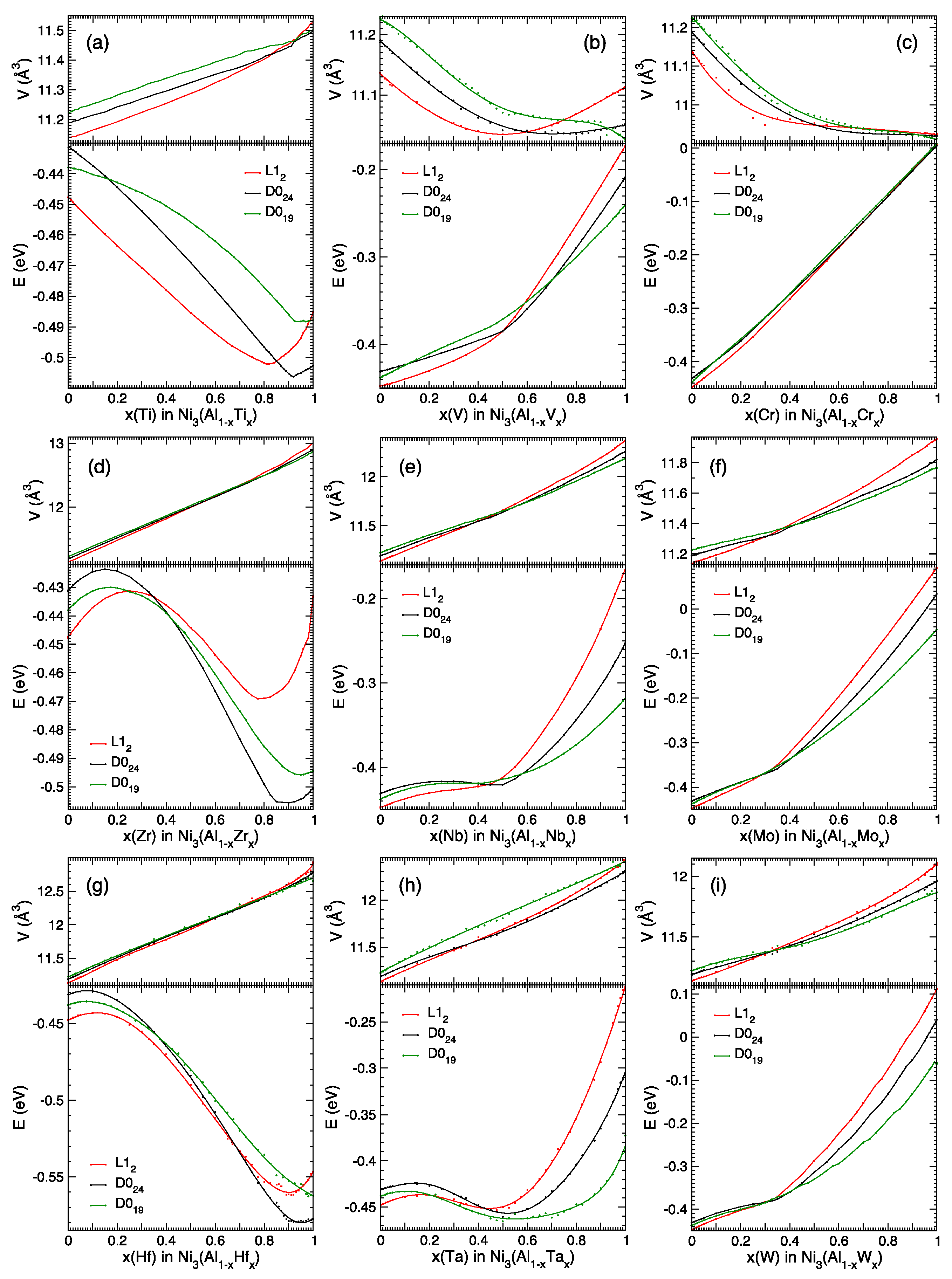
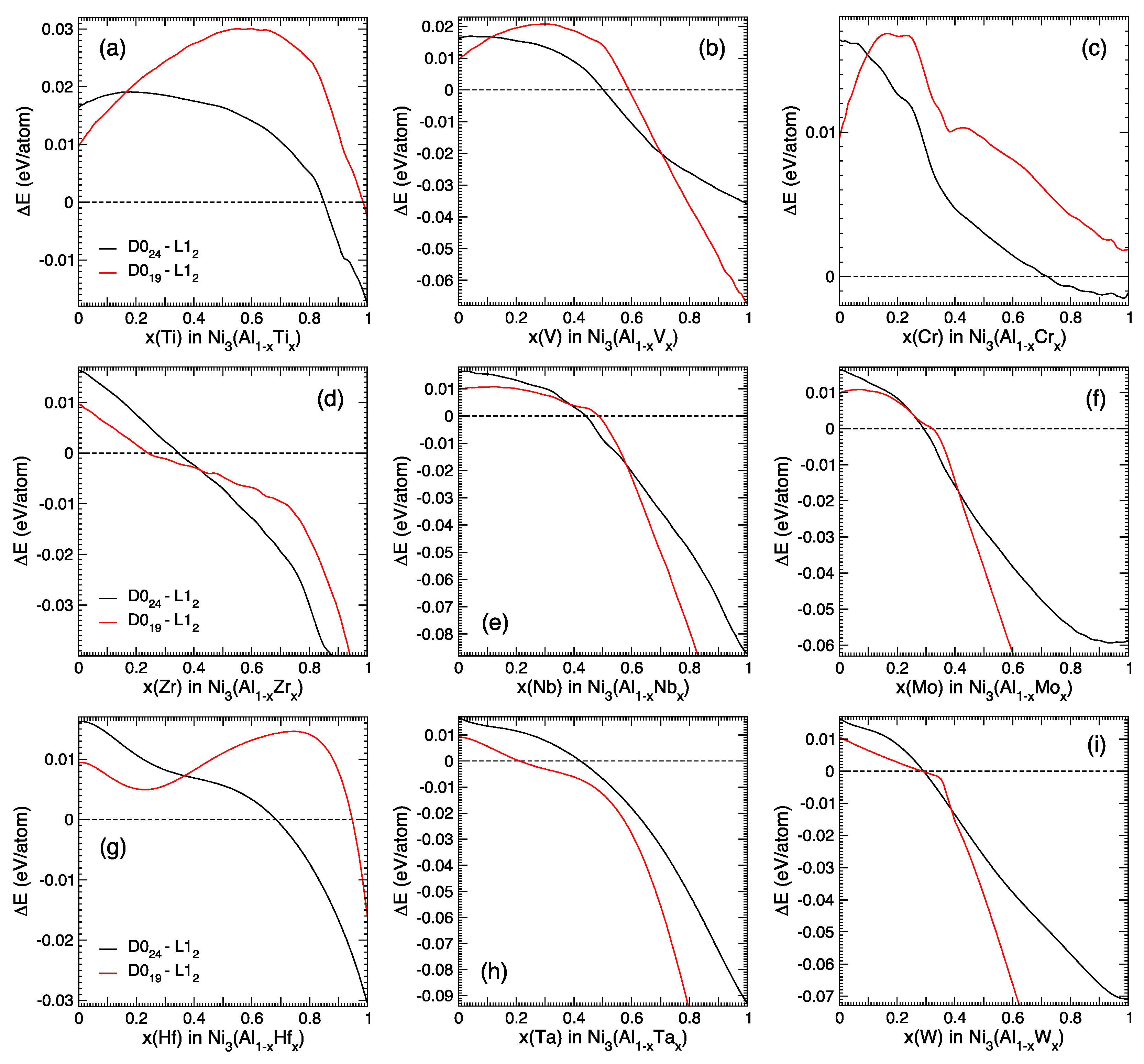
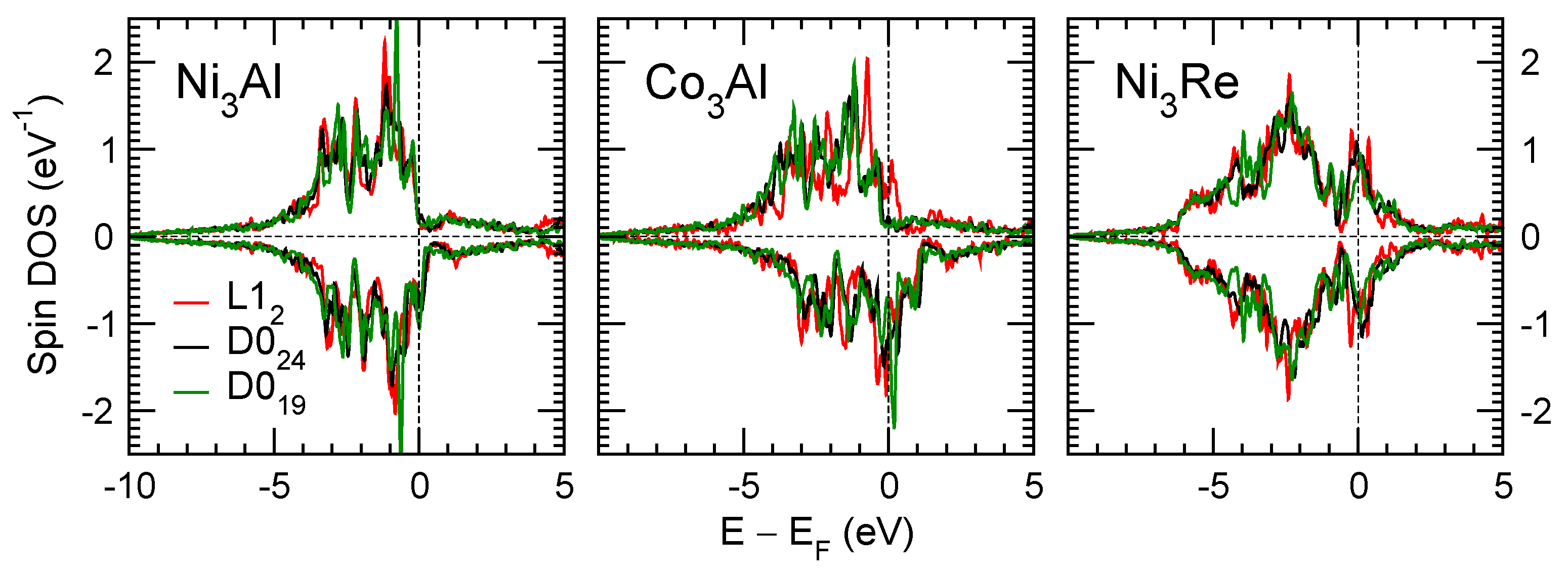
3. Results
4. Discussion
5. Summary
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Jena, A.K.; Chaturvedi, M.C. The role of alloying elements in the design of nickel-base superalloys. J. Mater. Sci. 1984, 19, 3121–3139. [Google Scholar] [CrossRef]
- Reed, R.C. The Superalloys: Fundamentals and Applications; Cambridge University Press: New York, NY, USA, 2006. [Google Scholar]
- Smith, T.M.; Zarkevich, N.A.; Egan, A.J.; Stuckner, J.; Gabb, T.P.; Lawson, J.W.; Mills, M.J. Utilizing local phase transformation strengthening for nickel-base superalloys. Commun. Mater. 2021, 2, 106. [Google Scholar] [CrossRef]
- Smith, T.M.; Esser, B.D.; Antolin, N.; Viswanathan, G.B.; Hanlon, T.; Wessman, A.; Mourer, D.; Windl, W.; McComb, D.W.; Mills, M.J.; et al. Segregation and η phase formation along stacking faults during creep at intermediate temperatures in a Ni-based superalloy. Acta Mater. 2015, 100, 19–31. [Google Scholar] [CrossRef]
- Smith, T.M.; Esser, B.D.; Antolin, N.; Carlsson, A.; Williams, R.E.A.; Wessman, A.; Hanlon, T.; Fraser, H.L.; Windl, W.; McComb, D.W.; et al. Phase transformation strengthening of high-temperature superalloys. Nat. Commun. 2016, 7, 13434. [Google Scholar] [CrossRef] [Green Version]
- Zarkevich, N.A. Theoretical and computational methods for accelerated materials discovery. Mod. Phys. Lett. B 2021, 35, 2130003. [Google Scholar] [CrossRef]
- Johnson, D.D.; Smirnov, A.V.; Khan, S.N. MECCA: Multiple-Scattering Electronic-Structure Calculations for Complex Alloys; KKR-CPA Program; Iowa State University and Ames Laboratory: Ames, Iowa, 2015. [Google Scholar]
- Kresse, G.; Hafner, J. Ab initio molecular dynamics for liquid metals. Phys. Rev. B 1993, 47, 558–561. [Google Scholar] [CrossRef]
- Perdew, J.P.; Ruzsinszky, A.; Csonka, G.I.; Vydrov, O.A.; Scuseria, G.E.; Constantin, L.A.; Zhou, X.; Burke, K. Restoring the Density-Gradient Expansion for Exchange in Solids and Surfaces. Phys. Rev. Lett. 2008, 100, 136406. [Google Scholar] [CrossRef] [Green Version]
- Zarkevich, N.A. Structural database for reducing cost in materials design and complexity of multiscale computations. Complexity 2006, 11, 36–42. [Google Scholar] [CrossRef]
- Johnson, D.D.; Nicholson, D.M.; Pinski, F.J.; Gyorffy, B.L.; Stocks, G.M. Density-Functional Theory for Random Alloys: Total Energy within the Coherent-Potential Approximation. Phys. Rev. Lett. 1986, 56, 2088–2091. [Google Scholar] [CrossRef]
- Korringa, J. On the calculation of the energy of a Bloch wave in a metal. Physica 1947, 13, 392–400. [Google Scholar] [CrossRef]
- Kohn, W.; Rostoker, N. Solution of the Schrödinger Equation in Periodic Lattices with an Application to Metallic Lithium. Phys. Rev. 1954, 94, 1111–1120. [Google Scholar] [CrossRef]
- Birch, F. Finite Elastic Strain of Cubic Crystals. Phys. Rev. 1947, 71, 809–824. [Google Scholar] [CrossRef]
- Murnaghan, F.D. The Compressibility of Media under Extreme Pressures. Proc. Natl. Acad. Sci. USA 1944, 30, 244–247. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zarkevich, N.A.; Johnson, D.D. Nudged-elastic band method with two climbing images: Finding transition states in complex energy landscapes. J. Chem. Phys. 2015, 142, 024106. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zarkevich, N.A. C2-NEB Source Code. Available online: https://lib.dr.iastate.edu/ameslab_software/1/ (accessed on 6 June 2023).
- Monkhorst, H.J.; Pack, J.D. Special points for Brillouin-zone integrations. Phys. Rev. B 1976, 13, 5188–5192. [Google Scholar] [CrossRef]
- Kohn, W.; Sham, L.J. Self-Consistent Equations Including Exchange and Correlation Effects. Phys. Rev. 1965, 140, A1133–A1138. [Google Scholar] [CrossRef] [Green Version]
- Martin, R.M. Electronic Structure: Basic Theory and Practical Methods; Cambridge University Press: Cambridge, UK, 2004. [Google Scholar]
- Martin, R.M.; Reining, L.; Ceperley, D.M. Interacting Electrons: Theory and Computational Approaches; Cambridge University Press: New York, NY, USA, 2016. [Google Scholar]
- Faulkner, J.S.; Stocks, G.M.; Wang, Y. Multiple Scattering Theory. In Electronic Structure of Solids; IOP Publishing: Bristol, UK, 2018. [Google Scholar] [CrossRef]
- Yibole, H.; Pathak, A.K.; Mudryk, Y.; Guillou, F.; Zarkevich, N.; Gupta, S.; Balema, V.; Pecharsky, V.K. Manipulating the stability of crystallographic and magnetic sub-lattices: A first-order magnetoelastic transformation in transition metal based Laves phase. Acta Mater. 2018, 154, 365–374. [Google Scholar] [CrossRef] [Green Version]
- Mignanelli, P.M.; Jones, N.G.; Pickering, E.J.; Messé, O.M.D.M.; Rae, C.M.F.; Hardy, M.C.; Stone, H.J. Gamma-gamma prime-gamma double prime dual-superlattice superalloys. Scr. Mater. 2017, 136, 136–140. [Google Scholar] [CrossRef]
- Kosorukova, T.; Firstov, G.; Noel, H.; Ivanckenko, V. Crystal structure changes in the Ni3Ta intermetallic compound. Chem. Met. Alloy. 2013, 6, 196–199. [Google Scholar] [CrossRef]
- Massalski, T.B. Binary Alloy Phase Diagrams, 2nd ed.; Massalski, T.B., Okamoto, H., Subramanian, P.R., Kacprzak, L., Eds.; ASM International: Materials Park, OH, USA, 1990. [Google Scholar]
- Nash, A.; Nash, P. The Ni−Ta (Nickel−Tantalum) system. Bull. Alloy Phase Diagr. 1984, 5, 259–265. [Google Scholar] [CrossRef]
- Yasuda, H.; Takasugi, T.; Koiwa, M. Elasticity of Ni-based L12-type intermetallic compounds. Acta Metall. Mater. 1992, 40, 381–387. [Google Scholar] [CrossRef]
- Prikhodko, S.V.; Yang, H.; Ardell, A.J.; Carnes, J.D.; Isaak, D.G. Temperature and composition dependence of the elastic constants of Ni3Al. Metall. Mater. Trans. A 1999, 30, 2403–2408. [Google Scholar] [CrossRef]
- Pearson, W.B.; Raynor, G.V. A Handbook of Lattice Spacings and Structures of Metals and Alloys; Elsevier: Amsterdam, The Netherlands, 2013. [Google Scholar] [CrossRef]
- Zarkevich, N.A.; Smith, T.M.; Baum, E.N.; Lawson, J.W. Compositional Glass: A State with Inherent Chemical Disorder, Exemplified by Ti-rich Ni3(Al,Ti)1 D024 Phase. Crystals 2022, 12, 1049. [Google Scholar] [CrossRef]
- Casselton, R.E.W.; Hume-Rothery, W. The equilibrium diagram of the system molybdenum-nickel. J. Less Common Met. 1964, 7, 212–221. [Google Scholar] [CrossRef]
- Wang, Y.; Woodward, C.; Zhou, S.H.; Liu, Z.K.; Chen, L.Q. Structural stability of Ni–Mo compounds from first-principles calculations. Scr. Mater. 2005, 52, 17–20. [Google Scholar] [CrossRef]
- Arapova, L.P. Crystalline structure of the intermetallic phases of WNi3 and MoNi3. Sov. Phys. J. 1973, 16, 1173–1175. [Google Scholar] [CrossRef]
- Isomäki, I.; Hämäläinen, M.; Braga, M.H.; Gasik, M. First principles, thermal stability and thermodynamic assessment of the binary Ni–W system. Int. J. Mater. Res. 2017, 108, 1025–1035. [Google Scholar] [CrossRef]
- Liu, X.; Pilling, J.; Heckel, R.W.; Lee, J.K. Influence of carbon on bond strength betvveen nickel and Ni–W alloys. Mater. Sci. Technol. 1991, 7, 228–238. [Google Scholar] [CrossRef]
- Cury, R.; Joubert, J.M.; Tusseau-Nenez, S.; Leroy, E.; Allavena-Valette, A. On the existence and the crystal structure of Ni4W, NiW and NiW2 compounds. Intermetallics 2009, 17, 174–178. [Google Scholar] [CrossRef]
- Nash, P. The Cr−Ni (Chromium-Nickel) system. Bull. Alloy Phase Diagr. 1986, 7, 466–476. [Google Scholar] [CrossRef]
- Zarkevich, N.A.; Tan, T.L.; Johnson, D.D. First-principles prediction of phase-segregating alloy phase diagrams and a rapid design estimate of their transition temperatures. Phys. Rev. B 2007, 75, 104203. [Google Scholar] [CrossRef]
- Lyu, F.; Liu, F.; Hu, X.; Yang, X.; Huang, C.; Shi, D. The δ Phase Precipitation of an Inconel 718 Superalloy Fabricated by Electromagnetic Stirring Assisted Laser Solid Forming. Materials 2019, 12, 2604. [Google Scholar] [CrossRef] [Green Version]
- Zarkevich, N.A.; Johnson, D.D. Reliable First-Principles Alloy Thermodynamics via Truncated Cluster Expansions. Phys. Rev. Lett. 2004, 92, 255702. [Google Scholar] [CrossRef] [Green Version]
- Zarkevich, N.A.; Tan, T.L.; Wang, L.L.; Johnson, D.D. Low-energy antiphase boundaries, degenerate superstructures, and phase stability in frustrated fcc Ising model and Ag-Au alloys. Phys. Rev. B 2008, 77, 144208. [Google Scholar] [CrossRef] [Green Version]
- Mydosh, J.A. Spin Glasses: An Experimental Introduction, 1st ed.; CRC Press: London, UK, 1993. [Google Scholar] [CrossRef]
- Rao, Y.; Smith, T.M.; Mills, M.J.; Ghazisaeidi, M. Segregation of alloying elements to planar faults in γ’-Ni3Al. Acta Mater. 2018, 148, 173–184. [Google Scholar] [CrossRef]
- Schoeck, G.; Kohlhammer, S.; Fahnle, M. Planar dissociations and recombination energy of [110] superdislocations in Ni3Al: Generalized Peierls model in combination with ab initioelectron theory. Philos. Mag. Lett. 1999, 79, 849–857. [Google Scholar] [CrossRef]
- Mryasov, O.N.; Gornostyrev, Y.N.; van Schilfgaarde, M.; Freeman, A.J. Superdislocation core structure in L12 Ni3Al, Ni3Ge and Fe3Ge: Peierls–Nabarro analysis starting from ab-initio GSF energetics calculations. Acta Mater. 2002, 50, 4545–4554. [Google Scholar] [CrossRef]
- Wen, Y.-F.; Sun, J.; Huang, J. First—Principles study of stacking fault energies in Ni3Al intermetallic alloys. Trans. Nonferr. Met. Soc. China 2012, 22, 661–664. [Google Scholar] [CrossRef]
- Yu, X.-X.; Wang, C.-Y. The effects of alloying elements on generalized stacking fault energies, strength and ductility of γ′-Ni3Al. Mater. Sci. Eng. A 2012, 539, 38–41. [Google Scholar] [CrossRef]
- Liu, L.-L.; Wu, X.-Z.; Wang, R.; Li, W.-G.; Liu, Q. Stacking fault energy, yield stress anomaly, and twinnability of Ni3Al: A first principles study*. Chin. Phys. B 2015, 24, 077102. [Google Scholar] [CrossRef]
- Hasan, H.; Mlkvik, P.; Haynes, P.D.; Vorontsov, V.A. Generalised stacking fault energy of Ni-Al and Co-Al-W superalloys: Density-functional theory calculations. Materialia 2020, 9, 100555. [Google Scholar] [CrossRef]
- Shang, S.-L.; Shimanek, J.; Qin, S.; Wang, Y.; Beese, A.M.; Liu, Z.-K. Unveiling dislocation characteristics in Ni3Al from stacking fault energy and ideal strength: A first-principles study via pure alias shear deformation. Phys. Rev. B 2020, 101, 024102. [Google Scholar] [CrossRef]
- Sims, C.T.; Hagel, W. (Eds.) Superalloys; Wiley: New York, NY, USA, 1972. [Google Scholar]
- Sims, C.T.; Stoloff, N.S.; Hagel, W.C. (Eds.) Superalloys II: High-Temperature Materials for Aerospace and Industrial Power; Wiley: New York, NY, USA, 1987. [Google Scholar]
- Caballero, F.G. (Ed.) Encyclopedia of Materials: Metals and Alloys; Elsevier: Amsterdam, The Netherlands, 2022. [Google Scholar]
- Smith, T.M.; Kantzos, C.A.; Zarkevich, N.A.; Harder, B.J.; Heczko, M.; Gradl, P.R.; Thompson, A.C.; Mills, M.J.; Gabb, T.P.; Lawson, J.W. A 3D Printable Alloy Designed for Extreme Environments. Nature 2023, 617, 513–518. [Google Scholar] [CrossRef] [PubMed]
- Reed, R.C.; Rae, C.M.F. 22-Physical Metallurgy of the Nickel-Based Superalloys. In Physical Metallurgy, 4th ed.; Laughlin, D.E., Hono, K., Eds.; Elsevier: Oxford, UK, 2014; pp. 2215–2290. [Google Scholar] [CrossRef]
- Sabol, G.P.; Stickler, R. Microstructure of Nickel-Based Superalloys. Phys. Status Solidi B 1969, 35, 11–52. [Google Scholar] [CrossRef]
- Borovikov, V.V.; Mendelev, M.I.; Smith, T.M.; Lawson, J.W. Dislocation-assisted diffusion-mediated atomic reshuffling in the Kolbe mechanism for micro-twinning in Ni-based superalloys from molecular dynamics simulation. Scr. Mater. 2023, 232, 115475. [Google Scholar] [CrossRef]
- Suzuki, H. Chemical interaction of solute atoms with dislocations. Sci. Rep. Res. Inst. Tohoku Univ. A 1952, 4, 455–463. [Google Scholar] [CrossRef]
- Borovikov, V.V.; Mendelev, M.I.; Smith, T.M.; Lawson, J.W. Molecular dynamics simulation of twin nucleation and growth in Ni-based superalloys. Int. J. Plast. 2023, 166, 103645. [Google Scholar] [CrossRef]
- Alabort, E.; Barba, D.; Sulzer, S.; Lißner, M.; Petrinic, N.; Reed, R.C. Grain boundary properties of a nickel-based superalloy: Characterisation and modelling. Acta Mater. 2018, 151, 377–394. [Google Scholar] [CrossRef]
- Zarkevich, N.A.; Johnson, D.D.; Pecharsky, V.K. High-throughput search for caloric materials: The CaloriCool approach. J. Phys. D Appl. Phys. 2017, 51, 024002. [Google Scholar] [CrossRef] [Green Version]

| Ni3X | E (eV/Atom) | V (Å3/Atom) | ||||
|---|---|---|---|---|---|---|
| L12 | D024 | D019 | L12 | D024 | D019 | |
| Ni3Al | −0.4477 | −0.4313 | −0.4381 | 10.90 | 10.91 | 10.92 |
| Ni3Ti | −0.4852 | −0.5028 | −0.4878 | 11.25 | 11.24 | 11.25 |
| Ni3Zr | −0.4332 | −0.5007 | −0.4943 | 12.63 | 12.62 | 12.62 |
| Ni3Hf | −0.5465 | −0.5774 | −0.5621 | 12.37 | 12.34 | 12.35 |
| Ni3V | −0.1729 | −0.2088 | −0.2404 | 10.81 | 10.77 | 10.73 |
| Ni3Nb | −0.1658 | −0.2531 | −0.3184 | 11.99 | 11.92 | 11.88 |
| Ni3Ta | −0.2155 | −0.3054 | −0.3727 | 11.96 | 11.88 | 11.83 |
| Ni3Cr | +0.0062 | +0.0051 | +0.0081 | 10.66 | 10.64 | 10.60 |
| Ni3Mo | +0.0924 | +0.0338 | −0.0460 | 11.59 | 11.49 | 11.46 |
| Ni3W | +0.1124 | +0.0403 | −0.0593 | 11.62 | 11.53 | 11.49 |
| Ni3X | B0 (GPa) | |||||
|---|---|---|---|---|---|---|
| L12 | D024 | D019 | L12 | D024 | D019 | |
| Ni3Al | 193.1 | 193.8 | 193.7 | 4.46 | 4.42 | 4.62 |
| Ni3Ti | 203.1 | 204.7 | 204.6 | 4.51 | 4.47 | 4.36 |
| Ni3Zr | 172.5 | 177.8 | 178.1 | 4.32 | 4.15 | 4.11 |
| Ni3Hf | 182.8 | 175.0 | 192.5 | 4.36 | 4.27 | 4.23 |
| Ni3V | 220.6 | 226.0 | 228.3 | 4.55 | 4.72 | 4.53 |
| Ni3Nb | 207.0 | 213.1 | 217.7 | 4.32 | 4.42 | 4.49 |
| Ni3Ta | 217.8 | 224.4 | 225.1 | 4.33 | 4.44 | ~5 |
| Ni3Cr | 214.6 | 216.4 | 217.8 | 5.02 | 4.96 | 5.04 |
| Ni3Mo | 226.7 | 229.0 | ~238 | 4.42 | 4.41 | 4.47 |
| Ni3W | 237.5 | 248.7 | ~270 | 4.56 | 4.20 | ~4.2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zarkevich, N.A.; Smith, T.M.; Lawson, J.W. Energy-Composition Relations in Ni3(Al1−xXx) Phases. Crystals 2023, 13, 943. https://doi.org/10.3390/cryst13060943
Zarkevich NA, Smith TM, Lawson JW. Energy-Composition Relations in Ni3(Al1−xXx) Phases. Crystals. 2023; 13(6):943. https://doi.org/10.3390/cryst13060943
Chicago/Turabian StyleZarkevich, Nikolai A., Timothy M. Smith, and John W. Lawson. 2023. "Energy-Composition Relations in Ni3(Al1−xXx) Phases" Crystals 13, no. 6: 943. https://doi.org/10.3390/cryst13060943
APA StyleZarkevich, N. A., Smith, T. M., & Lawson, J. W. (2023). Energy-Composition Relations in Ni3(Al1−xXx) Phases. Crystals, 13(6), 943. https://doi.org/10.3390/cryst13060943





