Analysis of the Effect of Simultaneous Melt Shearing and Cooling on Grain Formation and Rheology of A356 Aluminum Alloy
Abstract
:1. Introduction
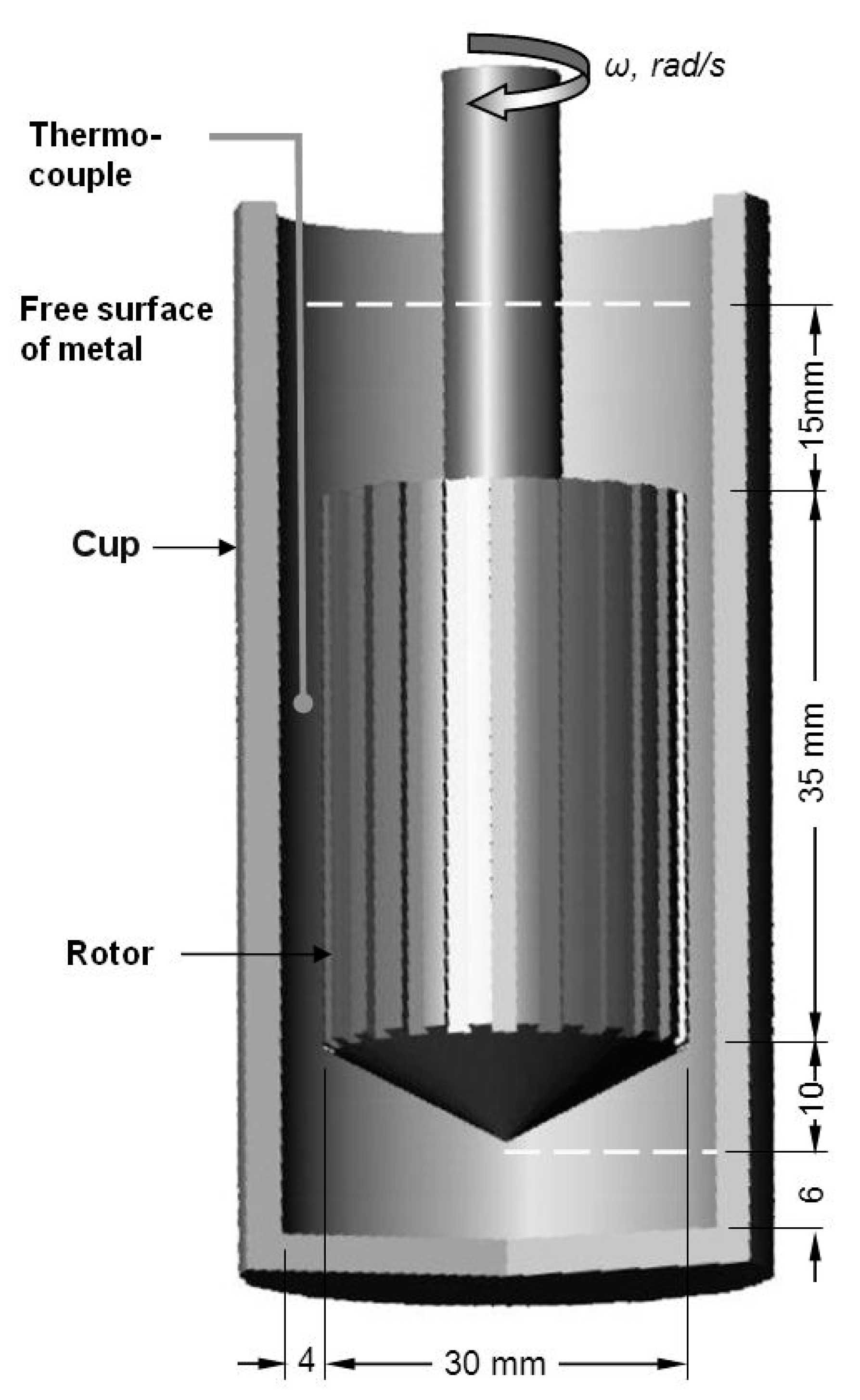
2. Methodology
3. Results
4. Analysis of the Results
5. Conclusions
- (1)
- Morphological evolution:
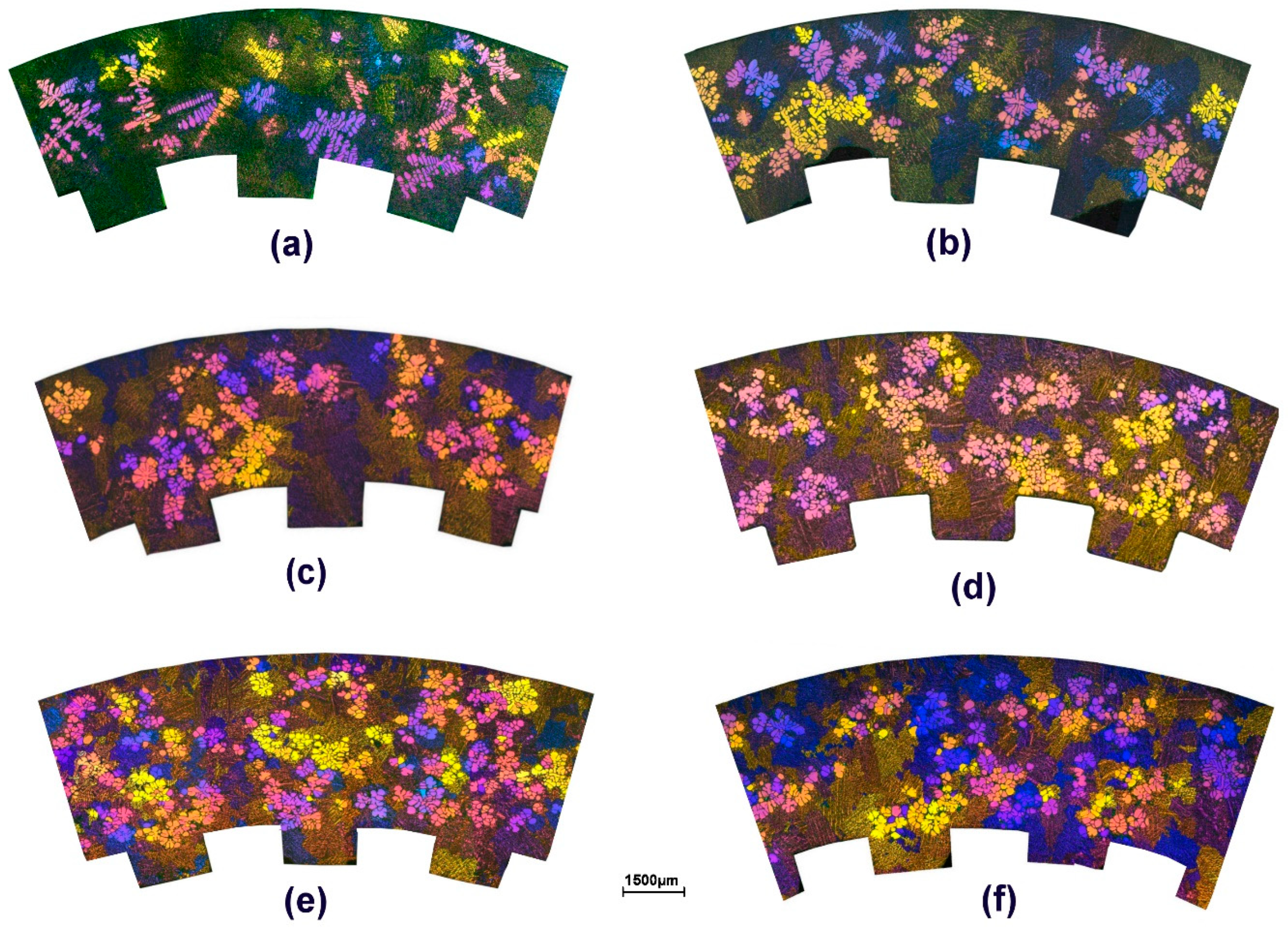
- The alloy A356 undergoes several morphological evolutions under shearing. Low shear rates provided a mixture of dispersed dendrites, cramped dendrites, and rosette-like grains. At higher shear rates, fine individual globular particles replaced the dendrites.
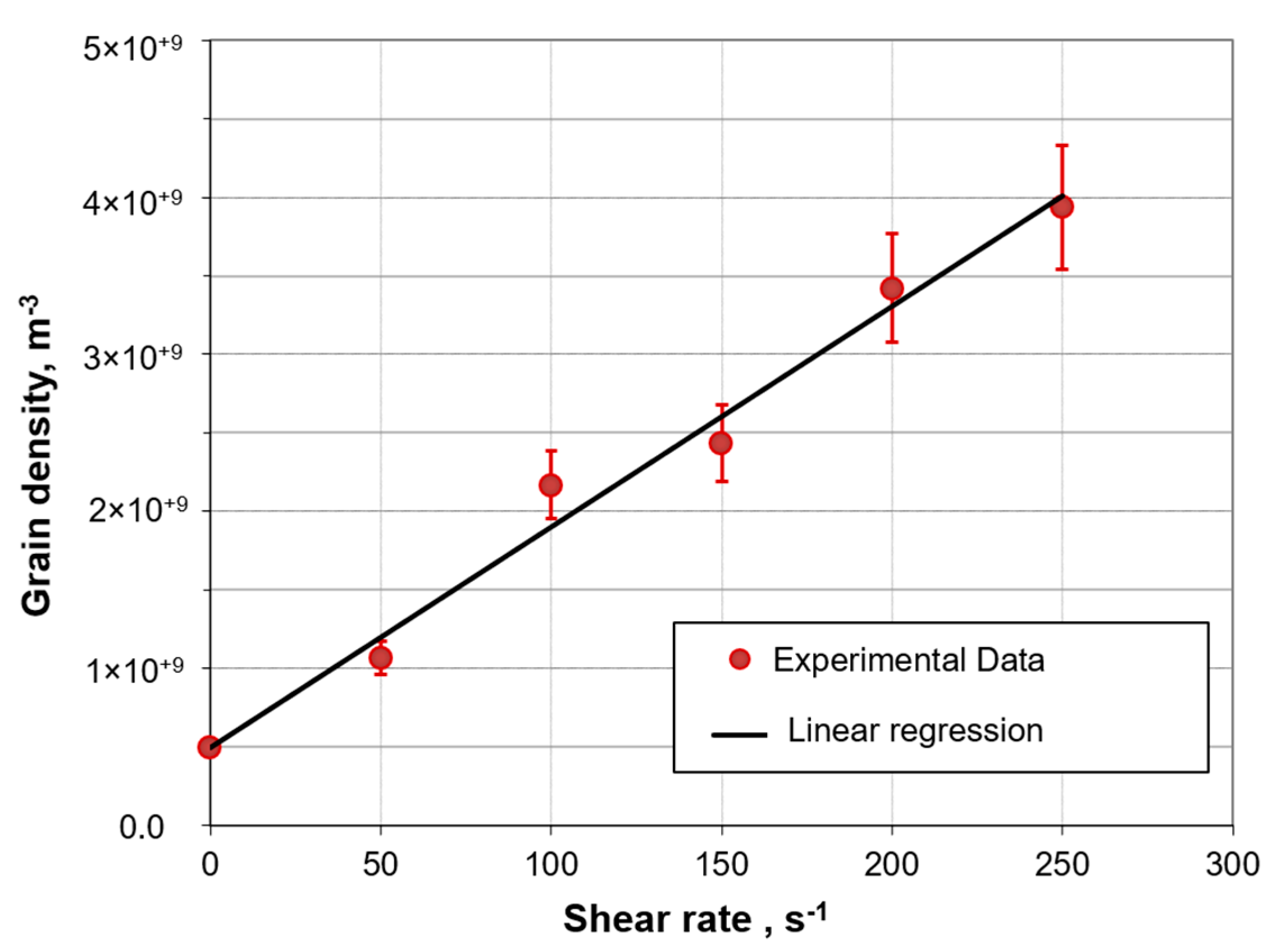
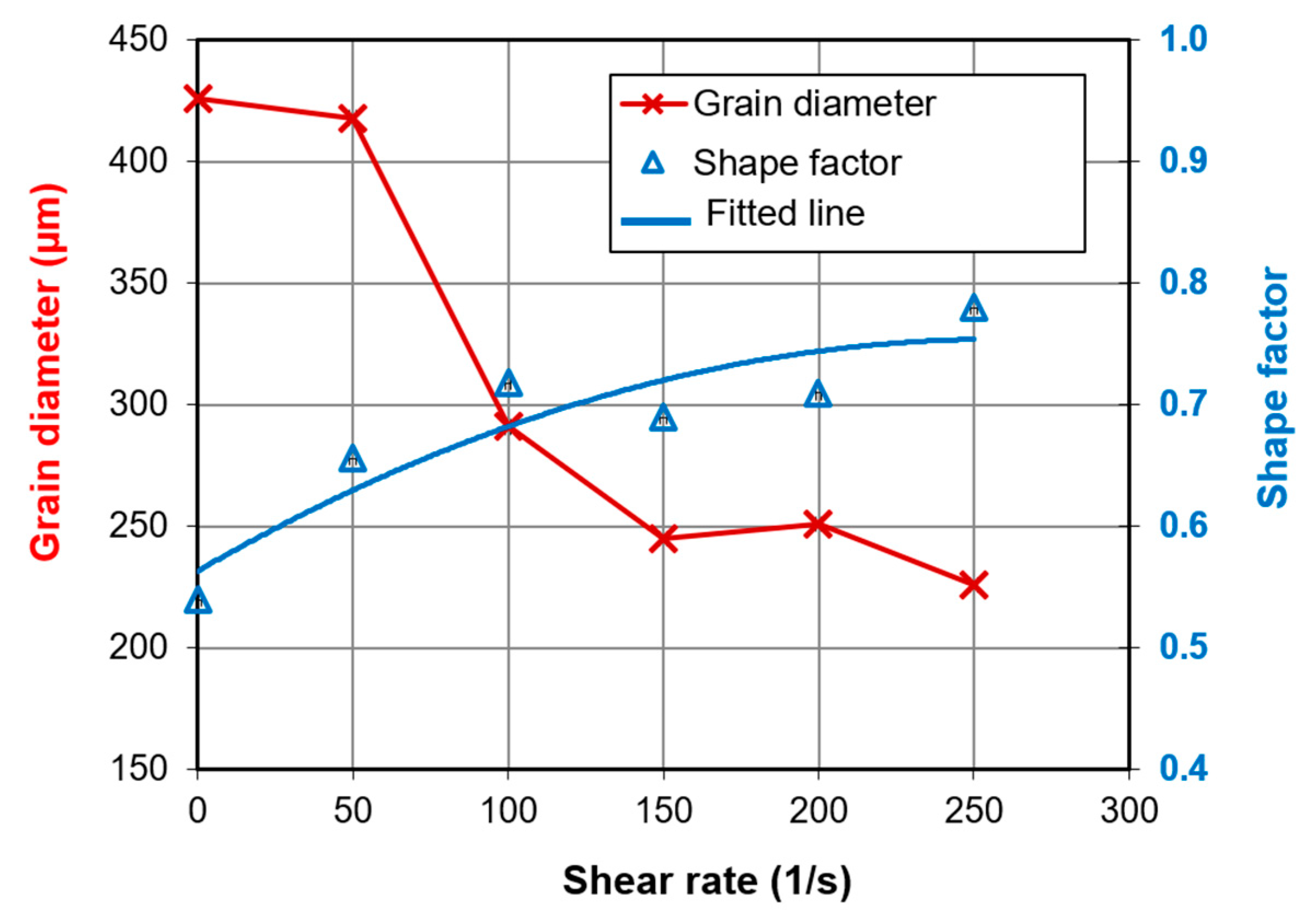
- Experiments showed an increasing grain number density, grain size refinement, and improved shape factor by increasing the shear rate.
- An empirical formula, , correlated to the applied shear rate and the undercooling-dependent grain density in the absence of shearing.
- A grain multiplication factor, , was suggested to account for the grains produced due to a shear rate increment. It would be very helpful in predicting grain density for when alloy mixture is transported between regions of different shear rates.
- (2)
- Rheology:
- The alloy exhibited a shear-thinning behavior where the viscosity obeyed the power law, .
- The measured viscosity showed a best fitting mixture viscosity model Hirai et al. [41]. Therefore, it was recommended for use in predicting the viscosity of semisolid slurries.
- (3)
- Outlook: Experiments can be expanded to cover higher solid fractions and shear rates in a trial to achieve a more comprehensive model. In situ observation of morphological evolutions during shearing of undercooled melts is highly recommended to accurately specify the involved mechanisms, which may lead to progress in modeling solidification under convective fields.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
References
- Meyer, I.L.; Durand, F. Modeling of Casting and Welding Processes-II; Metallurgical Society of AIME: Warrendale, PA, USA, 1984; pp. 179–191. [Google Scholar]
- Richards, R.S.; Rostoker, W. The influence of vibration on the solidification of an aluminum alloy. Trans. Am. Soc. Met. 1956, 48, 884–903. [Google Scholar]
- Li, M.; Tamura, T.; Miwa, K. Controlling microstructures of AZ31 magnesium alloys by an electromagnetic vibration technique during solidification: From experimental observation to theoretical understanding. Acta Mater. 2007, 55, 4635–4643. [Google Scholar] [CrossRef]
- Seifert, J.; Fischer, F. Horizontal continuous casting of metals with mould excited by ultrasonic waves. Ultrasonics 1977, 15, 154–158. [Google Scholar] [CrossRef]
- Spencer, D.B.; Mehrabian, R.; Flemings, M.C. Rheological behavior of Sn-15 pct Pb in the crystallization range. Metall. Trans. 1972, 3, 1925–1932. [Google Scholar] [CrossRef]
- Flemings, M.C.; Riek, R.G.; Young, K.P. Rheocasting. Mater. Sci. Eng. 1976, 25, 103–117. [Google Scholar] [CrossRef]
- Flemings, M.C. Behavior of metal alloys in the semisolid state. Metall. Trans. A 1991, 22A, 957–969. [Google Scholar] [CrossRef]
- Paradies, C.; Smith, R.; Glicksman, M. The influence of convection during solidification on fragmentation of the mushy zone of a model alloy. Metall. Mater. Trans. A 1997, 28A, 875–883. [Google Scholar] [CrossRef]
- Pilling, J.; Hellawell, A. Mechanical deformation of dendrites by fluid flow. Metall. Mater. Trans. A 1996, 27A, 229–232. [Google Scholar] [CrossRef]
- Ji, S.; Fan, Z. Solidification behavior of Sn-15 wt pct Pb alloy under a high shear rate and high intensity of turbulence during semisolid processing. Metall. Mater. Trans. A 2002, 33, 3511–3520. [Google Scholar] [CrossRef]
- Hitchcock, M.; Wang, Y.; Fan, Z. Secondary solidification behaviour of the Al–Si–Mg alloy prepared by the rheo-diecasting process. Acta Mater. 2007, 55, 1589–1598. [Google Scholar] [CrossRef]
- Fan, Z.; Liu, G.; Hitchcock, M. Solidification behaviour under intensive forced convection. Mater. Sci. Eng. A 2005, 413–414, 229–235. [Google Scholar] [CrossRef]
- Hitchcock, M.; Fan, Z. Solidification Behaviour of 357 Al-Alloy under Intensive Forced Convection. Mater. Sci. Forum 2006, 519–521, 1747–1752. [Google Scholar] [CrossRef]
- Fan, Z.; Wang, Y.; Xia, M.; Arumuganathar, S. Enhanced heterogeneous nucleation in AZ91D alloy by intensive melt shearing. Acta Mater. 2009, 57, 4891–4901. [Google Scholar] [CrossRef]
- Men, H.; Jiang, B.; Fan, Z. Mechanisms of grain refinement by intensive shearing of AZ91 alloy melt. Acta Mater. 2010, 58, 6526–6534. [Google Scholar] [CrossRef]
- Li, H.-T.; Wang, Y.; Fan, Z. Mechanisms of enhanced heterogeneous nucleation during solidification in binary Al–Mg alloys. Acta Mater. 2012, 60, 1528–1537. [Google Scholar] [CrossRef]
- Zhou, B.; Qiu, Z.; Chen, K.; Xu, C.; Wang, Z. Microstructure, Properties, and Numerical Simulation of Semi-Solid Aluminum Alloy under Planetary Stirring Process. Materials 2022, 15, 3009. [Google Scholar] [CrossRef]
- Yang, W.; Fredriksson, H.; Ji, S. Halo formation of Zn-Al alloys under conventional solidification and intensive convection solidification. J. Alloys Compd. 2017, 696, 460–469. [Google Scholar] [CrossRef]
- Fan, Z.; Gao, F.; Jiang, B.; Que, Z. Impeding Nucleation for More Significant Grain Refinement. Sci. Rep. 2020, 10, 9448. [Google Scholar] [CrossRef]
- Kumar, P.; Martin, C.L.; Brown, S. Constitutive modeling and characterization of the flow behavior of semi-solid metal alloys slurries—I. The flow response. Acta Metall. Mater. 1994, 42, 3595–3602. [Google Scholar] [CrossRef]
- Martin, C.L.; Kumar, P.; Brown, S. Constitutive modeling and characterization of the flow behavior of semi-solid metal alloy slurries—II. Structural evolution under shear deformation. Acta Metall. Mater. 1994, 42, 3603–3614. [Google Scholar] [CrossRef]
- Chen, J.Y.; Fan, Z. Modelling of rheological behaviour of semisolid metal slurries Part 1—Theory. Mater. Sci. Technol. 2002, 18, 237–242. [Google Scholar] [CrossRef]
- Fan, Z.; Chen, J.Y. Modelling of rheological behaviour of semisolid metal slurries Part 2—Steady state behaviour. Mater. Sci. Technol. 2002, 18, 243–249. [Google Scholar] [CrossRef]
- Fan, Z.; Chen, J.Y. Modelling of rheological behaviour of semisolid metal slurries Part 3—Transient state behaviour. Mater. Sci. Technol. 2002, 18, 250–257. [Google Scholar]
- Fan, Z.; Chen, J.Y. Modelling of rheological behaviour of semisolid metal slurries Part 4—Effects of particle morphology. Mater. Sci. Technol. 2002, 18, 258–266. [Google Scholar] [CrossRef]
- Li, L.; Zhou, X.W.; Chen, J.Y. A Rheological Model of Semisolid Magnesium Alloy Slurries. Mater. Sci. Forum 2005, 488–489, 333–336. [Google Scholar] [CrossRef]
- Molenaar, J.M.M.; Kool, W.H.; Smeulders, R.J. Coarsening during stircasting. J. Mater. Sci. 1987, 22, 1057–1060. [Google Scholar] [CrossRef]
- Mullis, A.M. Growth induced dendritic bending and rosette formation during solidification in a shearing flow. Acta Mater. 1999, 47, 1783–1789. [Google Scholar] [CrossRef]
- Rappaz, M.; Gandin, C.-A. Probabilistic modelling of microstructure formation in solidification processes. Acta Metall. Mater. 1993, 41, 345–360. [Google Scholar] [CrossRef]
- Liu, D.-R.; Guo, E.J.; Wang, L.P.; Kang, F.W.; Lan, Q. Modelling of dendritic growth under forced convection in solidification of Al–7Si alloy. Int. J. Cast. Met. Res. 2007, 20, 254–264. [Google Scholar] [CrossRef]
- Ahmadein, M.; Pustal, B.; Berger, R.; Subašić, E.; Bührig-Polaczek, A. Grain Nucleation Parameters for Aluminum Alloys: Experimental Determination and Model Validation. Metall. Mater. Trans. A 2009, 40, 646–683. [Google Scholar] [CrossRef]
- Atkinson, H.V. Modelling the semisolid processing of metallic alloys. Prog. Mater. Sci. 2005, 50, 341–412. [Google Scholar] [CrossRef]
- Dinsdale, A.T.; Quested, P.N. The viscosity of aluminium and its alloys—A review of data and models. J. Mater. Sci. 2004, 39, 7221–7228. [Google Scholar] [CrossRef]
- Zhang, Z.; Reddy, R.G.; Viswanathan, S. Viscosities of semisolid aluminum alloys and composites. In Proceedings of the Merton, C. Flemings Symposium on Solidification and Materials Processing; The Minerals, Metals & Materials Society, TMS: Pittsburgh, PA, USA, 2001; pp. 267–271. [Google Scholar]
- Barman, N.; Dutta, P. Rheology of A356 Alloy During Solidification Under Stirring. Trans. Indian Inst. Met. 2014, 67, 101–104. [Google Scholar] [CrossRef]
- Martin-Raya, O.; Menargues, S.; Martin, E.; Baile, M.T.; Picas, J.A. Rheological Behavior of the A356 Alloy in the Semisolid State at Low Shear Rates. Materials 2023, 16, 2280. [Google Scholar] [CrossRef] [PubMed]
- Jeng, S.-C.; Chen, S.-W. The solidification characteristics of 6061 and A356 aluminum alloys and their ceramic reinforced composites. Acta Mater. 1997, 45, 4887–4899. [Google Scholar] [CrossRef]
- Kim, N.S.; Kang, C.G. An investigation of flow characteristics considering the effect of viscosity variation in the thixoforming process. J. Mater. Process. Technol. 2000, 103, 237–246. [Google Scholar] [CrossRef]
- Brabazon, D.; Browne, D.; Carr, A. Experimental investigation of the transient and steady state rheological behaviour of Al-Si alloys in the mushy state. Mater. Sci. Eng. A 2003, 356, 69–80. [Google Scholar] [CrossRef]
- Moon, H.-K. Rheological Behavior and Microstructure of Ceramic Particulate/Aluminum Alloy Composites. Ph.D. Thesis, Massachusetts Institute of Technology, Cambridge, MA, USA, 1990; p. 209. [Google Scholar]
- Hirai, M.; Takebayashi, K.; Yoshikawa, Y.; Yamaguchi, R. Apparent Viscosity of Al-10mass%Cu Semi-solid Alloys. ISIJ Int. 1993, 33, 405–412. [Google Scholar] [CrossRef]
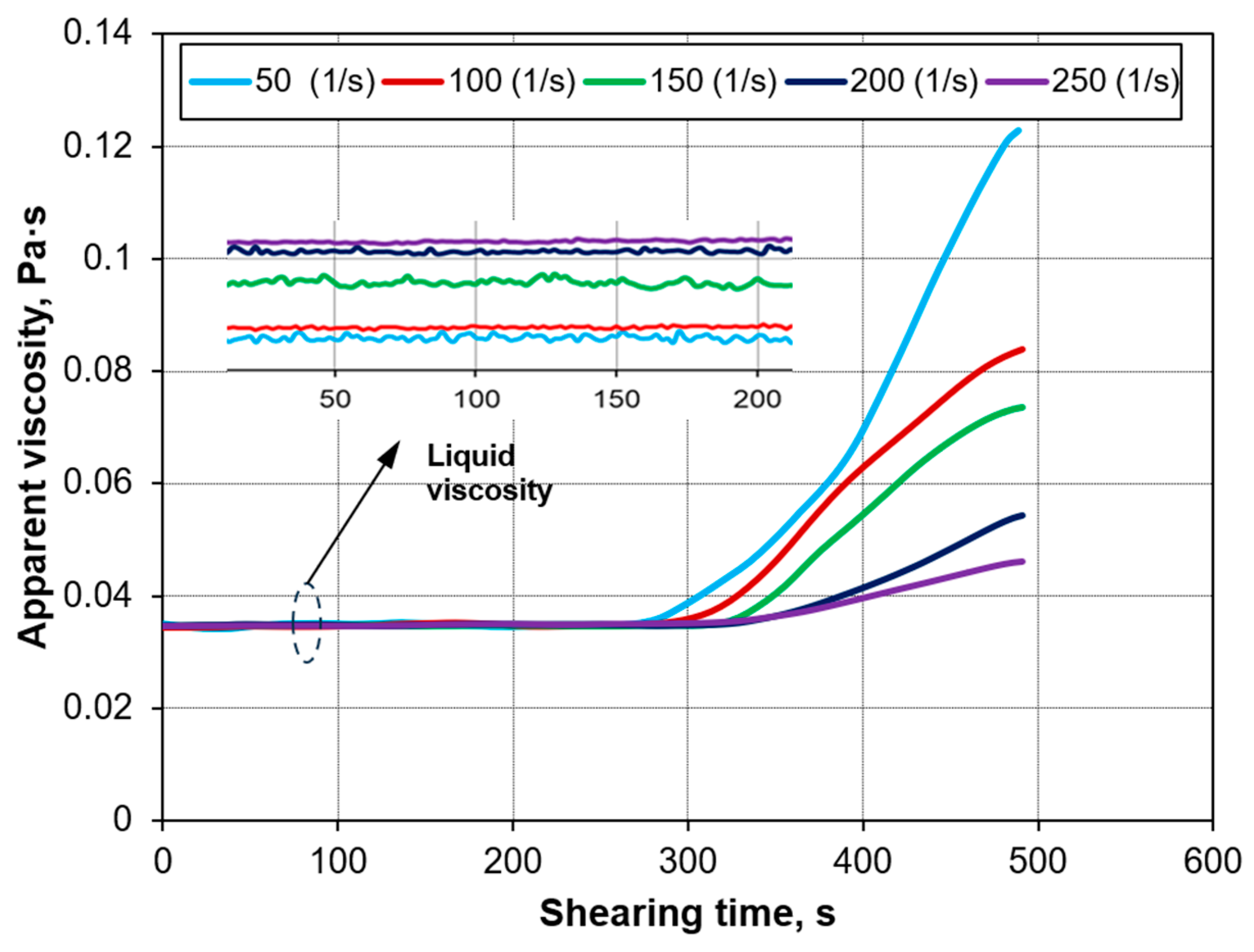

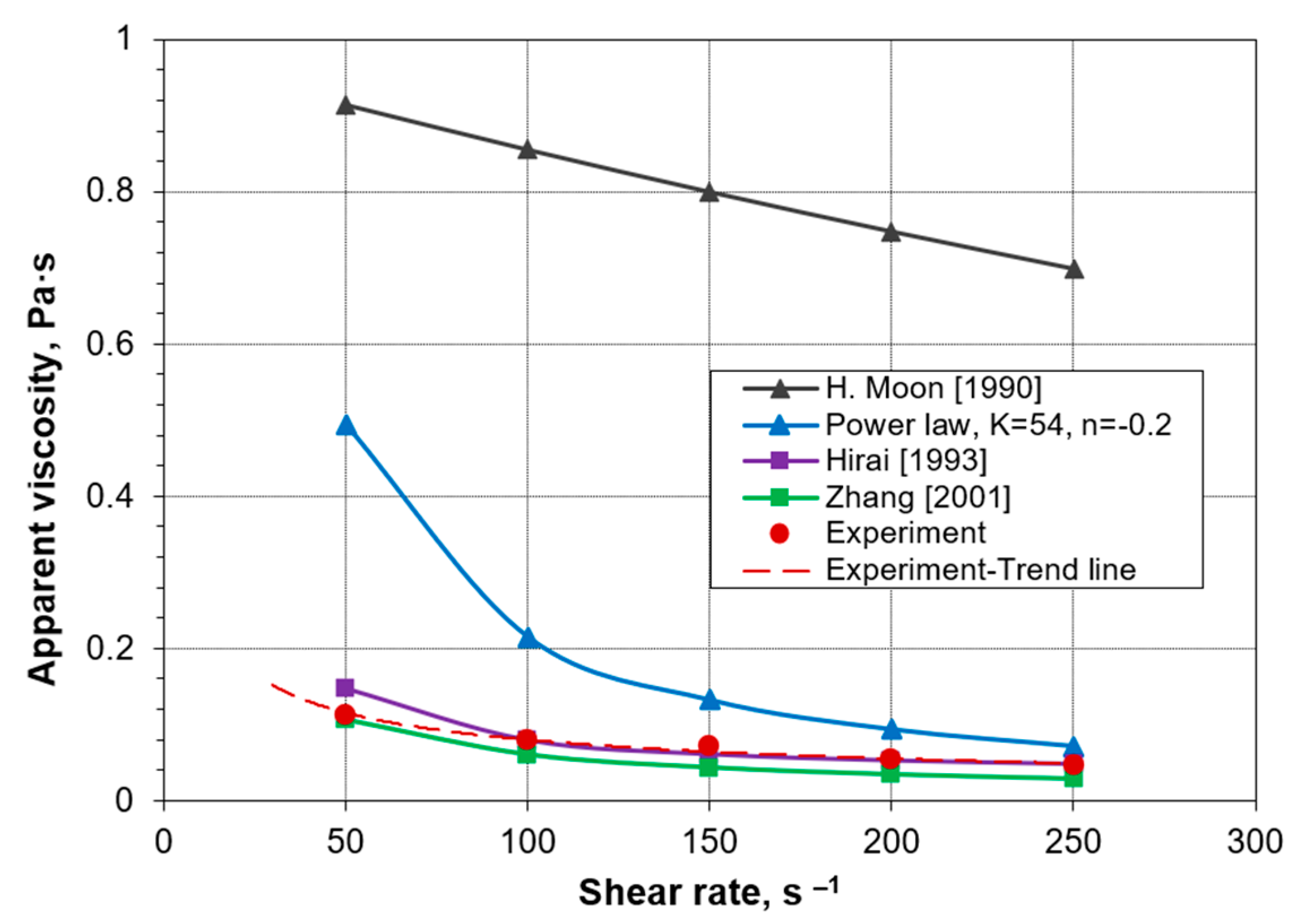
| Element | Si | Fe | Cu | Mg | Zn | Ti | Sr | Al |
|---|---|---|---|---|---|---|---|---|
| (wt.%) | 7.12 | 0.148 | 0.014 | 0.358 | 0.012 | 0.082 | 0.035 | Rest |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ahmadein, M.; Alsaleh, N.A.; Elsayed, M.A.; Ataya, S. Analysis of the Effect of Simultaneous Melt Shearing and Cooling on Grain Formation and Rheology of A356 Aluminum Alloy. Crystals 2023, 13, 1345. https://doi.org/10.3390/cryst13091345
Ahmadein M, Alsaleh NA, Elsayed MA, Ataya S. Analysis of the Effect of Simultaneous Melt Shearing and Cooling on Grain Formation and Rheology of A356 Aluminum Alloy. Crystals. 2023; 13(9):1345. https://doi.org/10.3390/cryst13091345
Chicago/Turabian StyleAhmadein, Mahmoud, Naser A. Alsaleh, Mahmoud Ahmed Elsayed, and Sabbah Ataya. 2023. "Analysis of the Effect of Simultaneous Melt Shearing and Cooling on Grain Formation and Rheology of A356 Aluminum Alloy" Crystals 13, no. 9: 1345. https://doi.org/10.3390/cryst13091345
APA StyleAhmadein, M., Alsaleh, N. A., Elsayed, M. A., & Ataya, S. (2023). Analysis of the Effect of Simultaneous Melt Shearing and Cooling on Grain Formation and Rheology of A356 Aluminum Alloy. Crystals, 13(9), 1345. https://doi.org/10.3390/cryst13091345










