High Mechanical Property and Texture Degree of Hot-Extruded Bi0.905Sb0.095
Abstract
1. Introduction
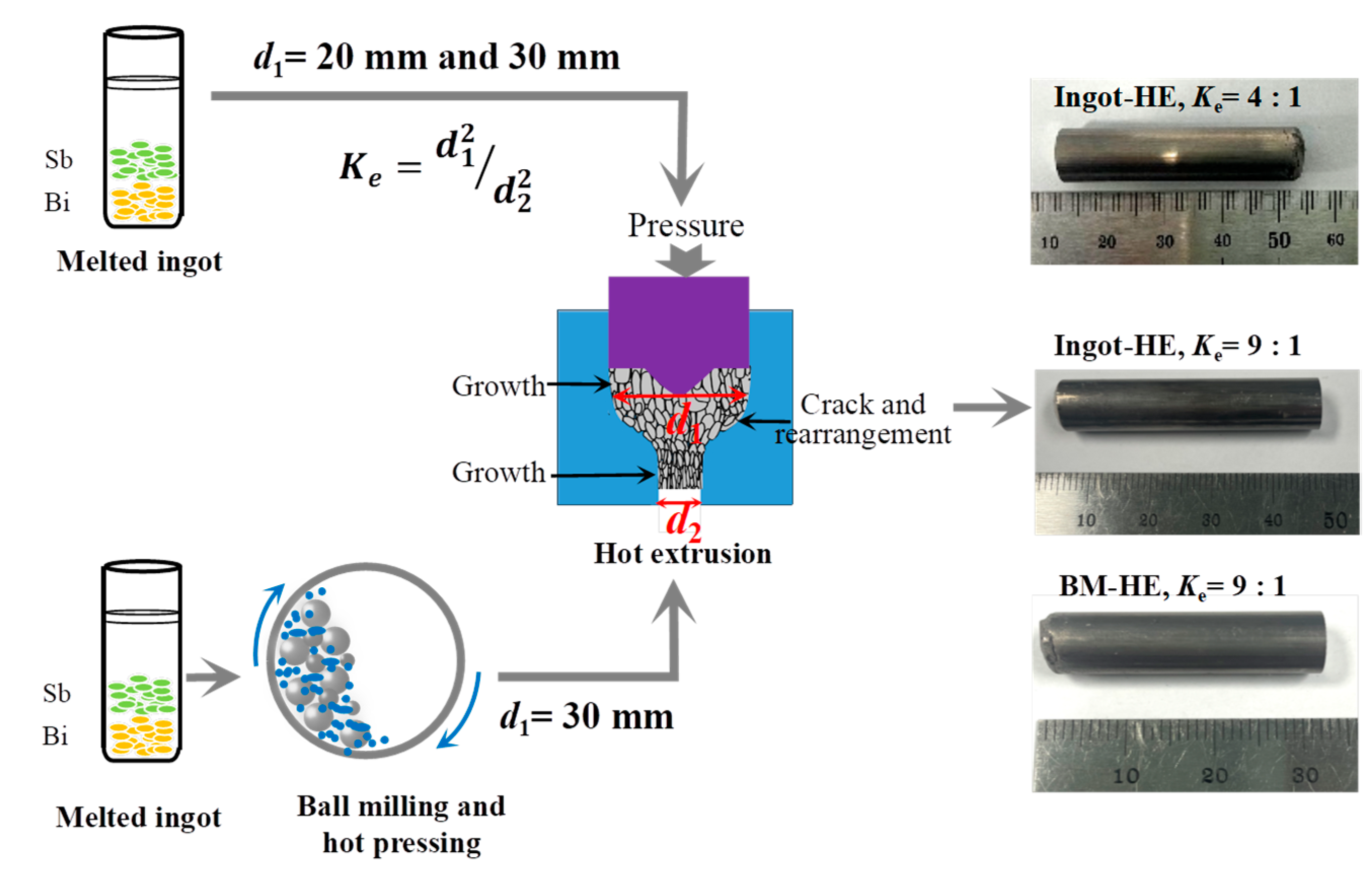
2. Materials and Methods
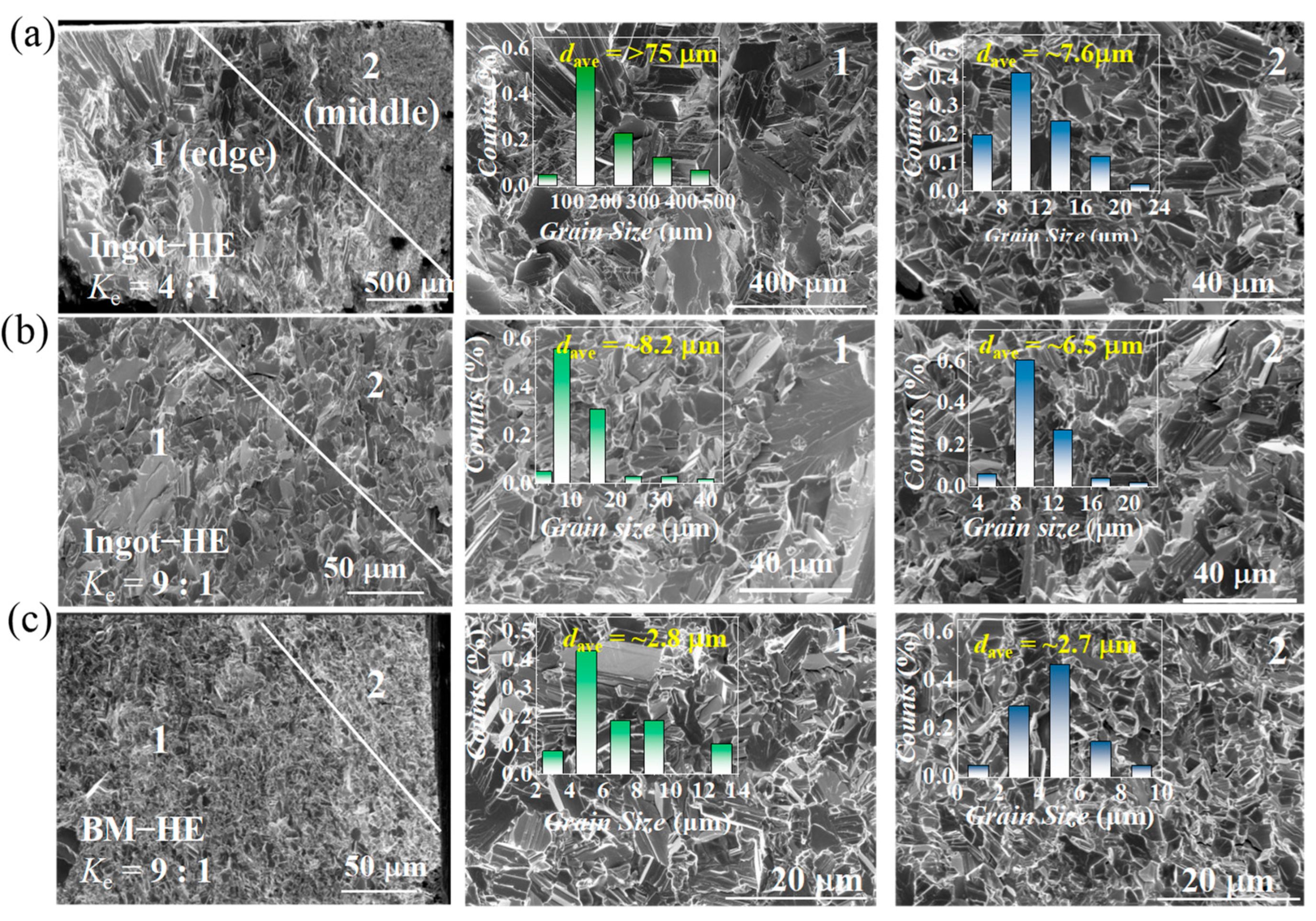
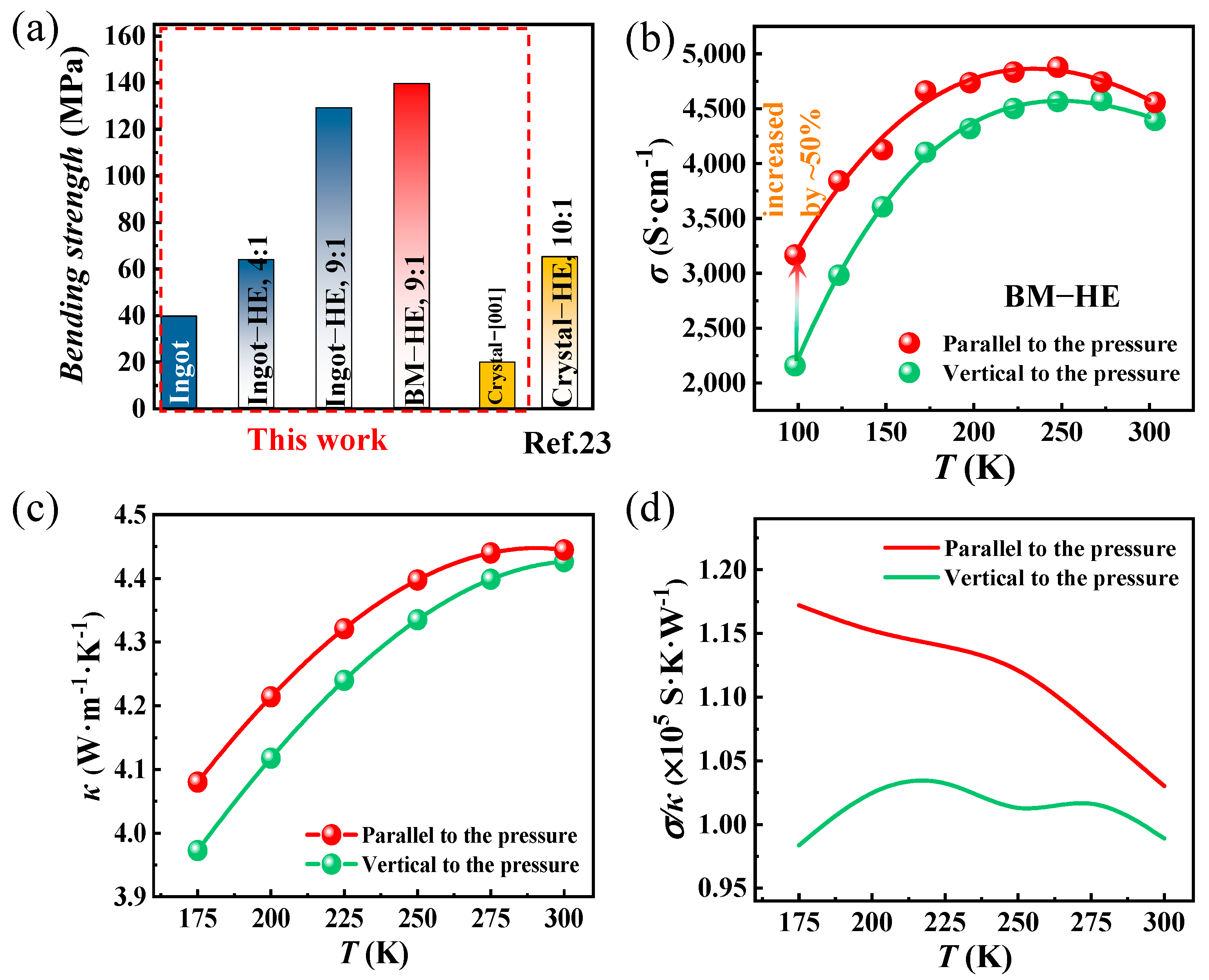
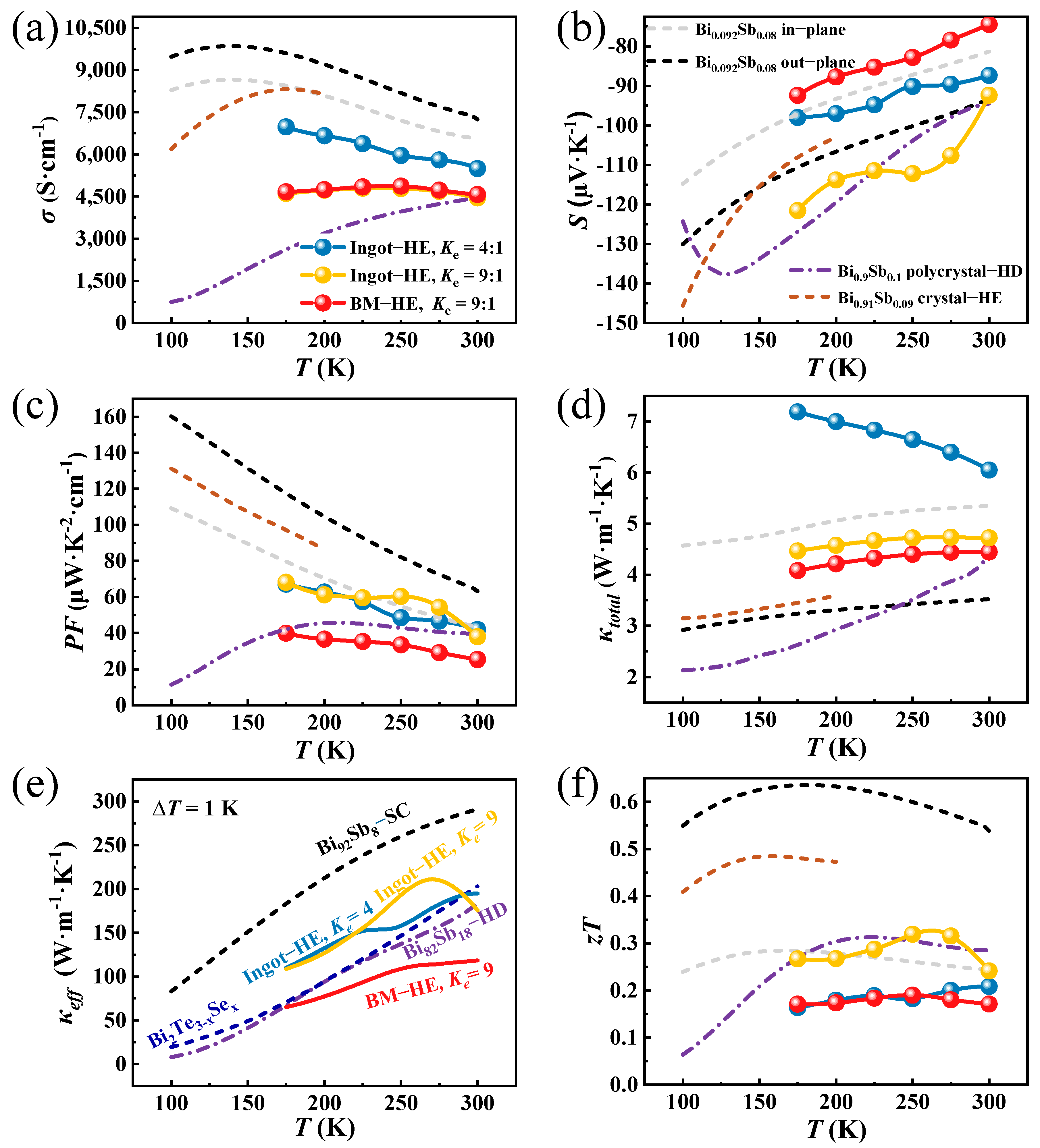
3. Results and Discussion
Characterization
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Feng, J.; Li, J.; Liu, R. Low-temperature thermoelectric materials and applications. Nano Energy 2024, 126, 109651. [Google Scholar] [CrossRef]
- Ling, Y.F.; Min, E.R.; Dong, G.Y.; Zhao, L.H.; Feng, J.H.; Li, J.; Zhang, P.; Liu, R.H.; Sun, R. Precise temperature control of electronic devices under ultra-high thermal shock via thermoelectric transient pulse cooling. Appl. Energ. 2023, 351, 121870. [Google Scholar]
- Chen, W.Y.; Shi, X.L.; Zou, J.; Chen, Z.G. Thermoelectric Coolers: Progress, Challenges, and Opportunities. Small Methods 2022, 6, 2101235. [Google Scholar] [CrossRef]
- Rogalski, A. History of infrared detectors. Opto-Electron. Rev. 2012, 20, 279–308. [Google Scholar] [CrossRef]
- Putra, N.; Sukyono, A.W.; Johansen, D.; Iskandar, F.N. The characterization of a cascade thermoelectric cooler in a cryosurgery device. Cryogenics 2010, 50, 759–764. [Google Scholar] [CrossRef]
- Khalatpour, A.; Paulsen, A.K.; Deimert, C.; Wasilewski, Z.R.; Hu, Q. High-power portable terahertz laser systems. Nat. Photonics 2021, 15, 16–20. [Google Scholar] [CrossRef]
- Rowe, D.M. Thermoelectrics Handbook Macro to Nano; CRC Press Taylor & Francis Group: Boca Raton, FL, USA, 2006. [Google Scholar]
- Zhou, J.; Feng, J.H.; Li, H.; Liu, D.; Qiu, G.J.; Qiu, F.; Li, J.; Luo, Z.Z.; Zou, Z.G.; Sun, R.; et al. Modulation of Vacancy Defects and Texture for High Performance n-Type Bi2Te3 via High Energy Refinement. Small 2023, 19, 2300654. [Google Scholar] [CrossRef]
- Huebener, R.P.; Tsuei, C.C. Prospects for Peltier cooling of superconducting electronics. Cryogenics 1998, 38, 325–328. [Google Scholar] [CrossRef]
- Li, H.; Feng, J.; Zhao, L.; Min, E.; Zhang, H.; Li, A.; Li, J.; Liu, R. Hierarchical Low-Temperature n-Type Bi2Te3 with High Thermoelectric Performances. ACS Appl. Mater. Inter. 2024, 16, 22147–22154. [Google Scholar] [CrossRef] [PubMed]
- Mao, J.; Chen, G.; Ren, Z.F. Thermoelectric cooling materials. Nat. Mater. 2021, 20, 454–461. [Google Scholar] [CrossRef] [PubMed]
- Boydston, R.W. Thermo-electric Effect in Single-Crystal Bismuth. Phys. Rev. 1927, 30, 911–921. [Google Scholar] [CrossRef]
- Gallo, C.F.; Chandrasekhar, B.S.; Sutter, P.H. Transport Properties of Bismuth Single Crystals. J. Appl. Phys. 1963, 34, 144–152. [Google Scholar] [CrossRef]
- Yim, W.M.; Amith, A. Bi-Sb Alloys for Magneto-Thermoelectric and Thermomagnetic Cooling. Solid State Electron. 1972, 15, 1141–1165. [Google Scholar] [CrossRef]
- Chandrasekhar, B.S. The seebeck coefficient of bismuth single crystals. J. Phys. Chem. Solids 1959, 11, 268–273. [Google Scholar] [CrossRef]
- Mao, J.; Zhu, H.T.; Ding, Z.W.; Liu, Z.H.; Gamage, G.A.; Chen, G.; Ren, Z.F. High thermoelectric cooling performance of n-type Mg3Bi2-based materials. Science 2019, 365, 495–498. [Google Scholar] [CrossRef]
- Schneider, G.; Herrmann, R.; Christ, B. Crystal growth and electron microprobe analysis of bismuth-antimony alloys (Bi100−xSbx). J. Cryst. Growth 1981, 52, 485–492. [Google Scholar] [CrossRef]
- Lenoir, B.; Dauscher, A.; Cassart, M.; Ravich, Y.I.; Scherrer, H. Effect of antimony content on the thermoelectric figure of merit of Bi1−xSbx alloys. J. Phys. Chem. Solids 1998, 59, 129–134. [Google Scholar] [CrossRef]
- Matsuo, T.; Suzuki, H. Phonon Scattering by Lattice-Defects in Deformed Bismuth Crystals. J. Phys. Soc. Jpn. 1977, 43, 1974–1981. [Google Scholar] [CrossRef]
- Matsuo, T.; Suzuki, H. Effect of Plastic-Deformation on Thermal-Conductivity of Bismuth Crystals. J. Phys. Soc. Jpn. 1976, 41, 1692–1698. [Google Scholar] [CrossRef]
- Tokumoto, Y.; Fujiwara, R.; Edagawa, K. High-Density Well-Aligned Dislocations Introduced by Plastic Deformation in Bi1−xSbx Topological Insulator Single Crystals. Crystals 2019, 9, 317. [Google Scholar] [CrossRef]
- Combe, E.; Funahashi, R.; Takeuchi, T.; Barbier, T.; Yubuta, K. Thermal deformation effects on thermoelectric properties for Bi0.82Sb0.18 alloys. J. Alloy Compd. 2017, 692, 563–568. [Google Scholar] [CrossRef]
- Sidorenko, N.; Parashchuk, T.; Maksymuk, M.; Dashevsky, Z. Development of cryogenic cooler based on n-type Bi-Sb thermoelectric and HTSC. Cryogenics 2020, 112, 103197. [Google Scholar] [CrossRef]
- Lu, T.B.; Wang, B.Y.; Li, G.D.; Yang, J.W.; Zhang, X.F.; Chen, N.; Liu, T.H.; Yang, R.G.; Niu, P.J.; Kan, Z.X.; et al. Synergistically enhanced thermoelectric and mechanical performance of Bi2Te3 via industrial scalable hot extrusion method for cooling and power generation applications. Mater. Today Phys. 2023, 32, 101035. [Google Scholar] [CrossRef]
- Liu, X.S.; Xing, T.; Qiu, P.F.; Deng, T.T.; Li, P.; Li, X.W.; Li, X.Y.; Shi, X. Suppressing the donor-like effect via fast extrusion engineering for high thermoelectric performance of polycrystalline Bi2Te2.79Se0.21. J. Materiomics 2023, 9, 345–352. [Google Scholar] [CrossRef]
- El-Asfoury, M.S.; Nasr, M.N.A.; Nakamura, K.; Abdel-Moneim, A. Structural and Thermoelectric Properties of Bi85Sb15 Prepared by Non-equal Channel Angular Extrusion. J. Electron. Mater. 2018, 47, 242–250. [Google Scholar] [CrossRef]
- El-Asfoury, M.S.; Abdou, S.M.; Nassef, A. Boosting Thermoelectric-Mechanical Properties of BiSb-Based Material by SiC Nanocomposites. JOM 2021, 73, 2808–2818. [Google Scholar] [CrossRef]
- Norizan, M.N.; Ohishi, Y.; Kurosaki, K.; Muta, H. Fabrication and Thermoelectric Property of Bi0.88Sb0.12/InSb Eutectic Alloy by Melt Spinning and Spark Plasma Sintering. Mater. Trans. 2019, 60, 1072–1077. [Google Scholar] [CrossRef]
- Zhou, M.; Li, J.; Dong, G.; Gao, S.; Feng, J.; Liu, R. Enhancement of Thermoelectric Performance for InTe by Selective Substitution and Grain Size Modulation. Crystals 2023, 13, 601. [Google Scholar] [CrossRef]
- Parashchuk, T.; Sidorenko, N.; Ivantsov, L.; Sorokin, A.; Maksymuk, M.; Dzundza, B.; Dashevsky, Z. Development of a solid-state multi-stage thermoelectric cooler. J. Power Sources 2021, 496, 229821. [Google Scholar] [CrossRef]
- Zhang, Y.; Xu, G.; Nozariasbmarz, A.; Li, W.; Raman, L.; Xing, C.; Sharma, S.; Liu, N.; Ghosh, S.; Joshi, G.; et al. Thermoelectric Cooling Performance Enhancement in BiSeTe Alloy by Microstructure Modulation via Hot Extrusion. Small Sci. 2024, 4, 2300245. [Google Scholar] [CrossRef]
- Yang, J.Y.; Chen, R.G.; Fan, X.A.; Zhu, W.; Bao, S.Q.; Duan, X.K. Microstructure control and thermoelectric properties improvement to n-type bismuth telluride based materials by hot extrusion. J. Alloy Compd. 2007, 429, 156–162. [Google Scholar] [CrossRef]
- Im, J.T.; Hartwig, K.T.; Sharp, J. Microstructural refinement of cast p-type Bi2Te3-Sb2Te3 by equal channel angular extrusion. Acta Mater. 2004, 52, 49–55. [Google Scholar] [CrossRef]
- Min, B.; Lim, S.S.; Jung, S.J.; Kim, G.; Lee, B.H.; Won, S.O.; Kim, S.K.; Rhyee, J.S.; Kim, J.S.; Baek, S.H. Texture-induced reduction in electrical resistivity of p-type (Bi.Sb)Te by a hot extrusion. J. Alloy Compd. 2018, 764, 261–266. [Google Scholar] [CrossRef]
- Lichnowski, A.J.; Saunders, G.A. The elastic constants of bismuth-antimony alloy single crystals. J. Phys. C Solid State Phys. 1976, 9, 927. [Google Scholar] [CrossRef]
- Lotgering, F.K. Topotactical Reactions with Ferrimagnetic Oxides Having Hexagonal Crystal Structures—1. J. Inorg. Nucl. Chem. 1959, 9, 113–123. [Google Scholar] [CrossRef]
- Jin, H.; Heremans, J.P. Optimization of the figure of merit in Bi100−xSbx/Al2O3 nanocomposites. Phys. Rev. Mater. 2018, 2, 115401. [Google Scholar] [CrossRef]
- Zebarjadi, M. Electronic cooling using thermoelectric devices. Appl. Phys. Lett. 2015, 106, 203506. [Google Scholar] [CrossRef]
- Chen, Z.; Han, Y.M.; Zhou, M.; Song, C.M.; Huang, R.J.; Zhou, Y.; Li, L.F. Thermoelectric properties of Ge-doped Bi85Sb15 alloys at low temperatures. J. Phys. Chem. Solids 2014, 75, 523–527. [Google Scholar] [CrossRef]
- Lukas, K.C.; Joshi, G.; Modic, K.; Ren, Z.F.; Opeil, C.P. Thermoelectric properties of Ho-doped Bi0.88Sb0.12. J. Mater. Sci. 2012, 47, 5729–5734. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, L.; Zhang, H.; Zhao, D.; Wang, D.; Liu, R.; Feng, J. High Mechanical Property and Texture Degree of Hot-Extruded Bi0.905Sb0.095. Crystals 2024, 14, 557. https://doi.org/10.3390/cryst14060557
Zhao L, Zhang H, Zhao D, Wang D, Liu R, Feng J. High Mechanical Property and Texture Degree of Hot-Extruded Bi0.905Sb0.095. Crystals. 2024; 14(6):557. https://doi.org/10.3390/cryst14060557
Chicago/Turabian StyleZhao, Linghao, Hongcheng Zhang, Degang Zhao, Dawei Wang, Ruiheng Liu, and Jianghe Feng. 2024. "High Mechanical Property and Texture Degree of Hot-Extruded Bi0.905Sb0.095" Crystals 14, no. 6: 557. https://doi.org/10.3390/cryst14060557
APA StyleZhao, L., Zhang, H., Zhao, D., Wang, D., Liu, R., & Feng, J. (2024). High Mechanical Property and Texture Degree of Hot-Extruded Bi0.905Sb0.095. Crystals, 14(6), 557. https://doi.org/10.3390/cryst14060557






