Thermally Induced Ion Magnetic Moment in H4O Superionic State
Abstract
1. Introduction
2. Calculation Method
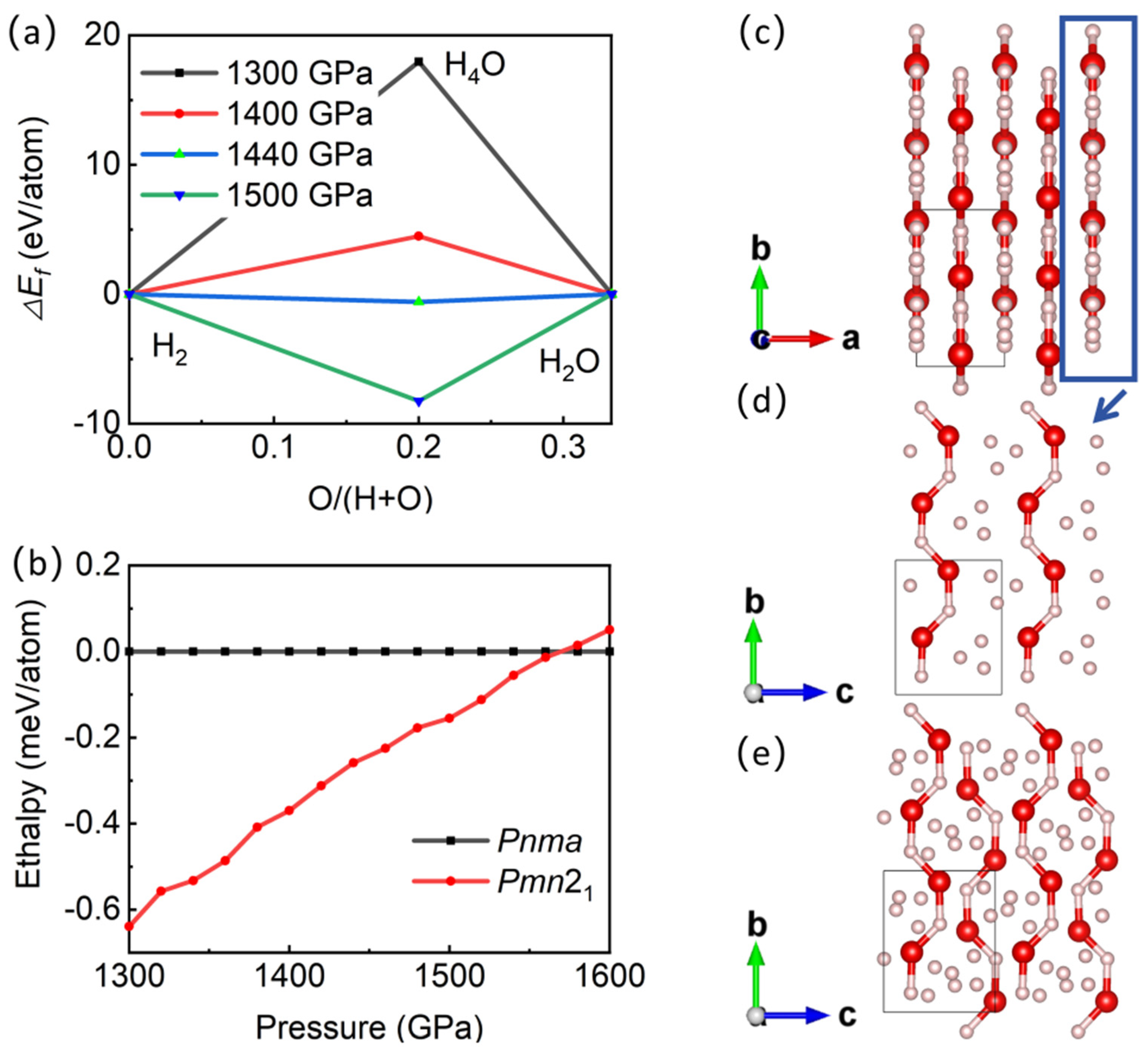
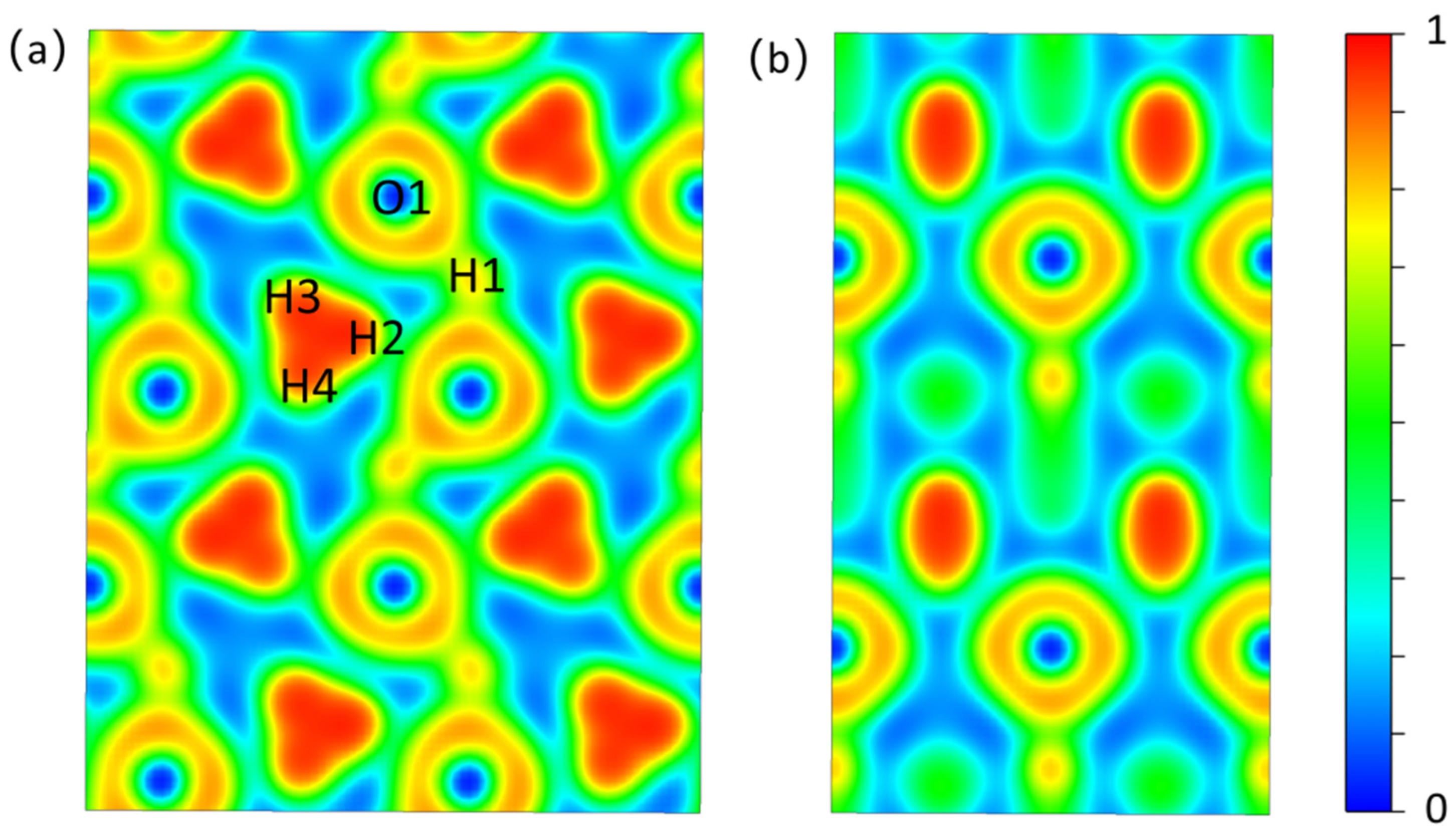
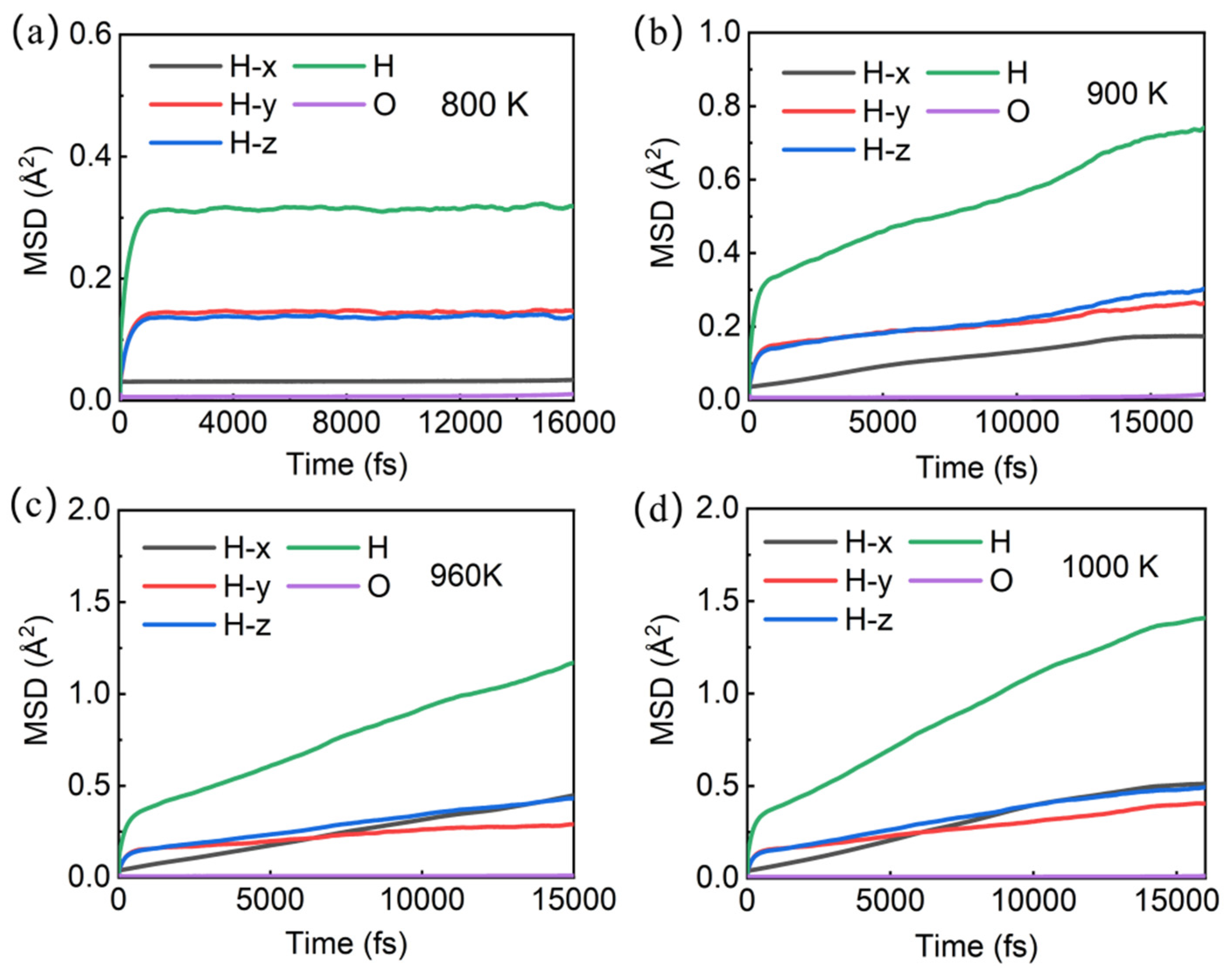
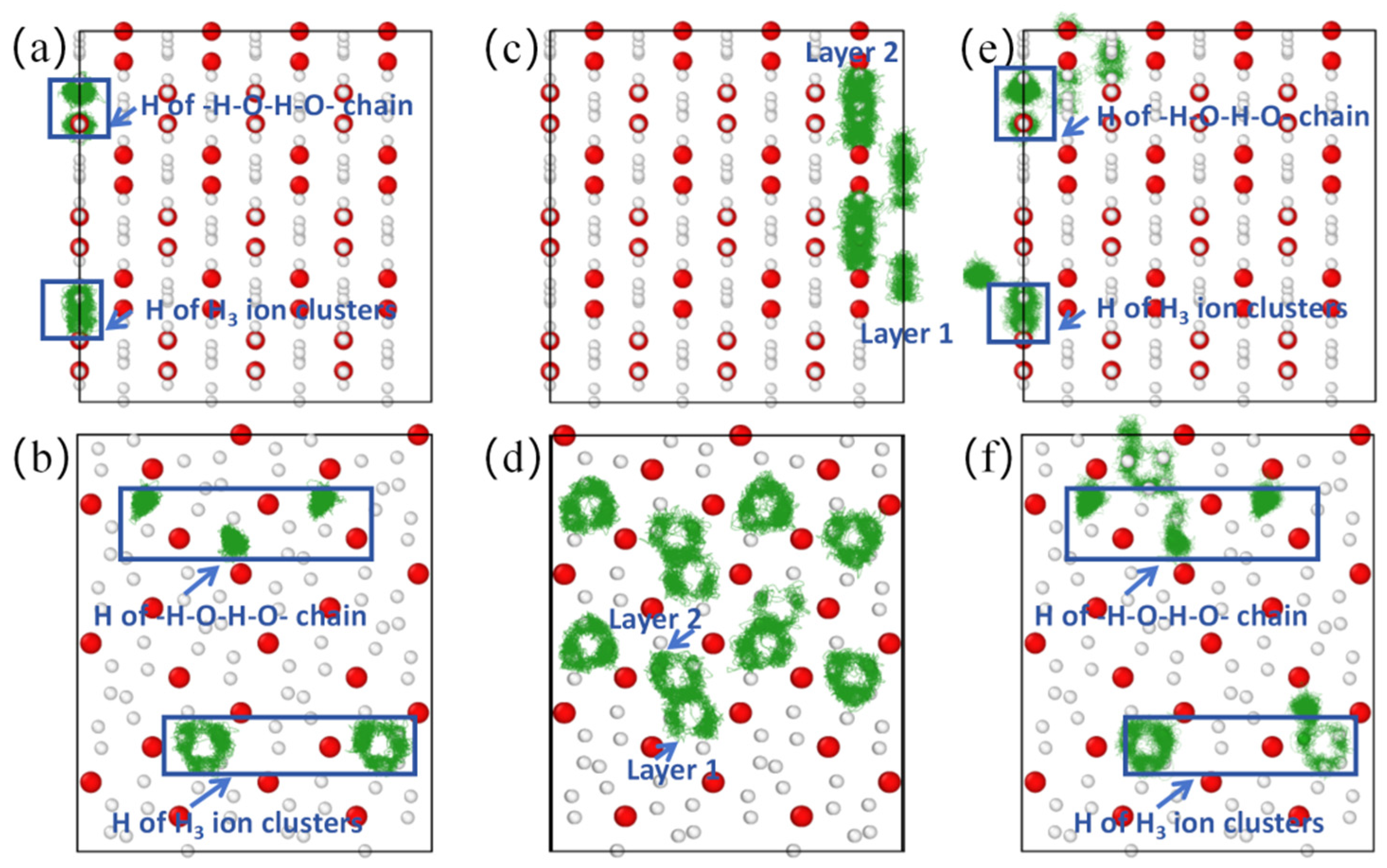
3. Results and Discussion
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ness, N.F.; Acuna, M.H.; Behannon, K.W.; Burlaga, L.F.; Connerney, J.E.; Lepping, R.P.; Neubauer, F.M. Magnetic fields at uranus. Science 1986, 233, 85–89. [Google Scholar] [CrossRef] [PubMed]
- Ness, N.F.; Acuna, M.H.; Burlaga, L.F.; Connerney, J.E.; Lepping, R.P.; Neubauer, F.M. Magnetic fields at neptune. Science 1989, 246, 1473–1478. [Google Scholar] [CrossRef] [PubMed]
- Helled, R.; Anderson, J.D.; Podolak, M.; Schubert, G. Interior Models of Uranus and Neptune. Astrophys. J. 2011, 726, 15. [Google Scholar] [CrossRef]
- Wang, Y.; Liu, H.; Lv, J.; Zhu, L.; Wang, H.; Ma, Y. High pressure partially ionic phase of water ice. Nat. Commun. 2011, 2, 563. [Google Scholar] [CrossRef]
- Wilson, H.F.; Wong, M.L.; Militzer, B. Superionic to Superionic Phase Change in Water: Consequences for the Interiors of Uranus and Neptune. Phys. Rev. Lett. 2013, 110, 151102. [Google Scholar] [CrossRef]
- Sun, J.; Clark, B.K.; Torquato, S.; Car, R. The phase diagram of high-pressure superionic ice. Nat. Commun. 2015, 6, 8156. [Google Scholar] [CrossRef]
- French, M.; Desjarlais, M.P.; Redmer, R. Ab initio calculation of thermodynamic potentials and entropies for superionic water. Phys. Rev. E 2016, 93, 022140. [Google Scholar] [CrossRef]
- Hernandez, J.-A.; Caracas, R. Superionic-Superionic Phase Transitions in Body-Centered Cubic H2O Ice. Phys. Rev. Lett. 2016, 117, 135503. [Google Scholar] [CrossRef]
- Millot, M.; Hamel, S.; Rygg, J.R.; Celliers, P.M.; Collins, G.W.; Coppari, F.; Fratanduono, D.E.; Jeanloz, R.; Swift, D.C.; Eggert, J.H. Experimental evidence for superionic water ice using shock compression. Nat. Phys. 2018, 14, 297–302. [Google Scholar] [CrossRef]
- Millot, M.; Coppari, F.; Rygg, J.R.; Barrios, A.C.; Hamel, S.; Swift, D.C.; Eggert, J.H. Nanosecond X-ray diffraction of shock-compressed superionic water ice. Nature 2019, 569, 251–255. [Google Scholar] [CrossRef]
- Yamane, R.; Komatsu, K.; Gouchi, J.; Uwatoko, Y.; Machida, S.; Hattori, T.; Ito, H.; Kagi, H. Experimental evidence for the existence of a second partially-ordered phase of ice VI. Nat. Commun. 2021, 12, 1129. [Google Scholar] [CrossRef] [PubMed]
- Cavazzoni, C.; Chiarotti, G.L.; Scandolo, S.; Tosatti, E.; Bernasconi, M.; Parrinello, M. Superionic and metallic states of water and ammonia at giant planet conditions. Science 1999, 283, 44–46. [Google Scholar] [CrossRef] [PubMed]
- Hubbard, W.B. Interiors of the giant planets. Science 1981, 214, 145–149. [Google Scholar] [CrossRef] [PubMed]
- Huang, P.; Liu, H.; Lv, J.; Li, Q.; Long, C.; Wang, Y.; Chen, C.; Hemley, R.J.; Ma, Y. Stability of H3O at extreme conditions and implications for the magnetic fields of Uranus and Neptune. Proc. Natl. Acad. Sci. USA 2020, 117, 5638–5643. [Google Scholar] [CrossRef]
- Vos, W.L.; Finger, L.W.; Hemley, R.J.; Mao, H.-K. Novel H2-H2O clathrates at high pressures. Phys. Rev. Lett. 1993, 71, 3150–3153. [Google Scholar] [CrossRef]
- Soubiran, F.; Militzer, B. Miscibility calculations for water and hydrogen in giant planets. Astrophys. J. 2015, 806, 228. [Google Scholar] [CrossRef]
- Oganov, A.R.; Glass, C.W. Crystal structure prediction using ab initio evolutionary techniques: Principles and applications. J. Chem. Phys. 2006, 124, 244704. [Google Scholar] [CrossRef]
- Kresse, G.; Furthmüller, J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B 1996, 54, 11169–11186. [Google Scholar] [CrossRef]
- Nosé, S. A unified formulation of the constant temperature molecular dynamics methods. J. Chem. Phys. 1984, 81, 511–519. [Google Scholar] [CrossRef]
- Hoover, W.G. Canonical dynamics: Equilibrium phase-space distributions. Phys. Rev. A 1985, 31, 1695–1697. [Google Scholar] [CrossRef]
- Shuichi, N. Constant Temperature Molecular Dynamics Methods. Prog. Theor. Phys. Suppl. 1991, 103, 1–46. [Google Scholar] [CrossRef]
- Zhang, S.; Wilson, H.F.; Driver, K.P.; Militzer, B. H4O and other hydrogen-oxygen compounds at giant-planet core pressures. Phys. Rev. B 2013, 87, 024112. [Google Scholar] [CrossRef]
- Burdett, J.K.; McCormick, T.A. Electron localization in molecules and solids: The meaning of ELF. J. Phys. Chem. A 1998, 102, 6366–6372. [Google Scholar] [CrossRef]
- Ayers, P.W. Electron localization functions and local measures of the covariance. J. Chem. Sci. 2005, 117, 441–454. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liang, X.; Peng, J.; Wu, F.; Wang, R.; Yang, Y.; Li, X.; Dong, H. Thermally Induced Ion Magnetic Moment in H4O Superionic State. Crystals 2025, 15, 304. https://doi.org/10.3390/cryst15040304
Liang X, Peng J, Wu F, Wang R, Yang Y, Li X, Dong H. Thermally Induced Ion Magnetic Moment in H4O Superionic State. Crystals. 2025; 15(4):304. https://doi.org/10.3390/cryst15040304
Chicago/Turabian StyleLiang, Xiao, Junhao Peng, Fugen Wu, Renhai Wang, Yujue Yang, Xingyun Li, and Huafeng Dong. 2025. "Thermally Induced Ion Magnetic Moment in H4O Superionic State" Crystals 15, no. 4: 304. https://doi.org/10.3390/cryst15040304
APA StyleLiang, X., Peng, J., Wu, F., Wang, R., Yang, Y., Li, X., & Dong, H. (2025). Thermally Induced Ion Magnetic Moment in H4O Superionic State. Crystals, 15(4), 304. https://doi.org/10.3390/cryst15040304





