Dimensional Stability and Process Capability of an Industrial Component Injected with Recycled Polypropylene
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
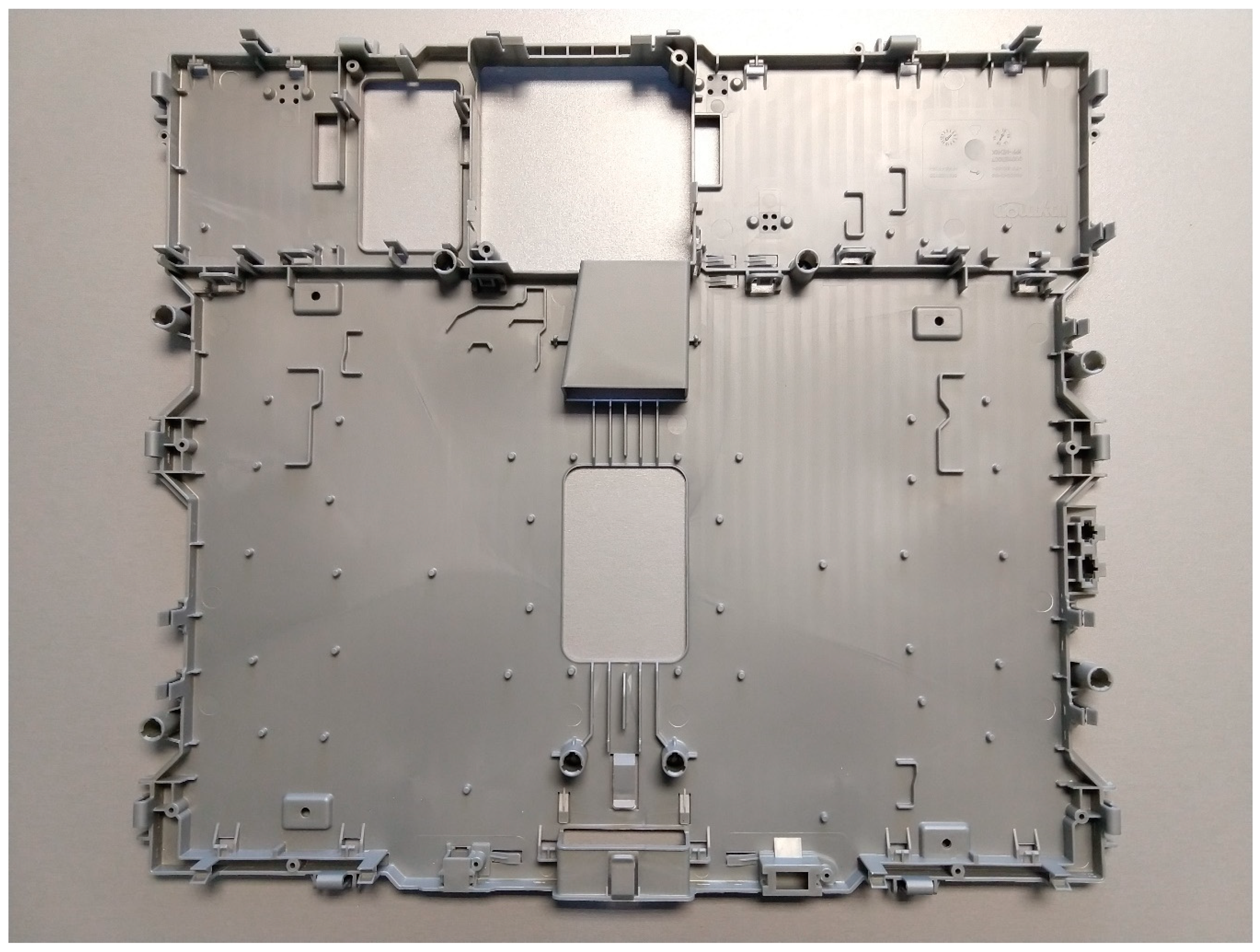
2.2. Dimensional Tests Methodology
- M1 = dimension measured after being heated at 50 °C
- Mnom = nominal dimension
- M0 = dimension measured at room temperature
- ∆M1 = dimension variation between samples heated at 50 °C and samples at room temperature.
- M2 = dimension measured after being heated at 80 °C
- Mnom = nominal dimension
- M0 = dimension measured at room temperature
- ∆M2 = dimension variation between samples heated at 80 °C and at room temperature.
- ∆M50-80 is the difference in measures between samples heated at 50 and 80 °C.
- ∆Mi is the difference in measures between the dimensions at Mi conditions and those at room temperature; both referred to the nominal dimension.
- M1 = dimension measured after being heated at 50 °C
- M2 = dimension measured after being heated at 80 °C
- -
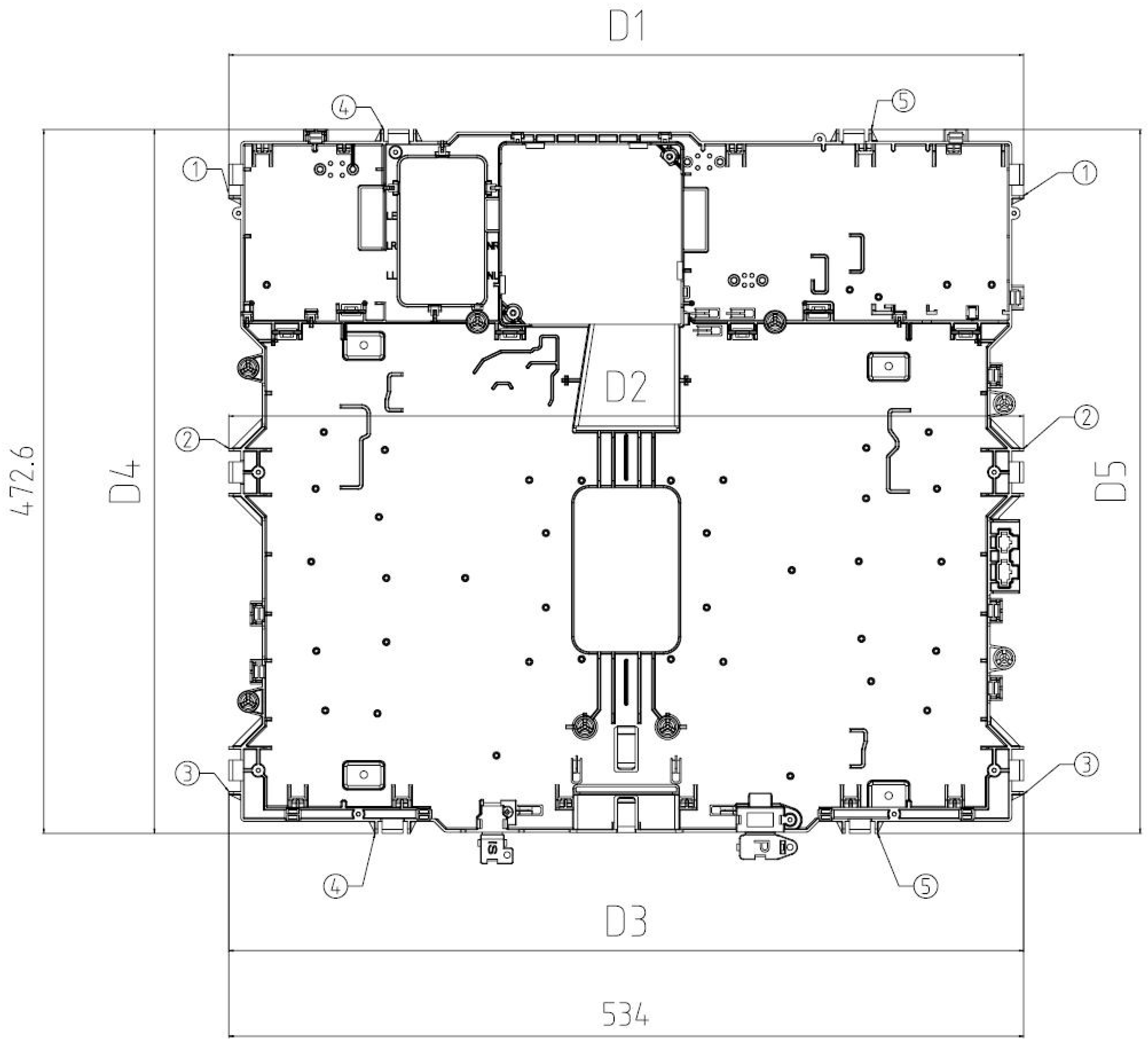
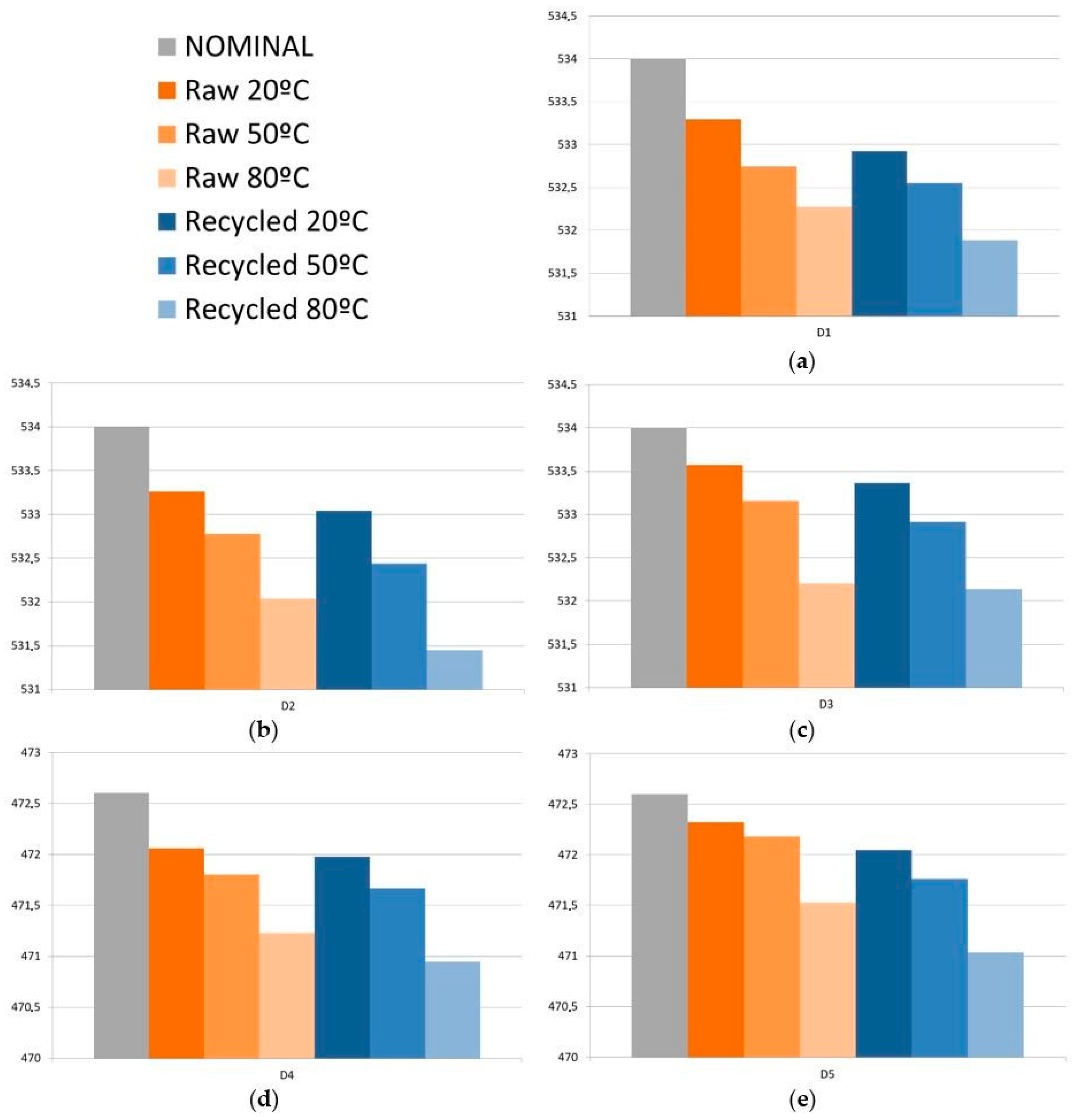
- D1 is the cartesian distance from fixing point 1 on the left edge of the part to fixing point 1 on the right edge. To determine the measurement of this distance, the line joining fixing points 1 and 3 is taken as a reference in such a way that D1 is normal to it. D1 nominal value is 534 ± 0.6 mm.
- -
- D2 is the cartesian distance from fixing point 2 on the left edge of the part to fixing point 2 on the right edge. To determine the measurement of this distance, the line joining fixing points 1 and 3 is taken as a reference in such a way that D2 is normal to it. D2 nominal value is 534 ± 0.6 mm.
- -
- D3 is the cartesian distance from fixing point 3 on the left edge of the part to fixing point 3 on the right edge. To determine the measurement of this distance, the line joining fixing points 1 and 3 is taken as a reference in such a way that D3 is normal to it. D3 nominal value is 534 ± 0.6 mm.
- -
- D4 is the cartesian distance from fixing point 4 on the upper edge of the part to fixing point 4 on the lower edge. To determine the measurement of this distance, the line joining fixing points 4 and 5 is taken as a reference in such a way that D4 is normal to it. D4 nominal value is 472.6 ± 0.6 mm.
- -
- D5 is the cartesian distance from fixing point 5 on the upper edge of the part to fixing point 5 on the lower edge. To determine the measurement of this distance, the line joining fixing points 4 and 5 is taken as a reference in such a way that D5 is normal to it. D5 nominal value is 472.6 ± 0.6 mm.
2.3. Process Capability Tests Methodology
- Cp stands for process capability to produce parts within the tolerance specification limits,
- Cpk stands for process capability to produce parts within the tolerance specification limits and near its nominal value,
- Cpu stands for the value between the process mean and the upper specification limit,
- Cpl stands for the value between the process mean and the lower specification limit,
- USL is the upper specification limit,
- LSL is the lower specification limit.
- Provided that measurements follow a normal distribution,
- σ is the standard deviation of the measures
- μ is the average value of n measured samples xi calculated as:where n = 25;
- xi is the value of each dimension for sample i.
3. Results and Discussion
3.1. Dimensions after Stabilization at Room Temperature
3.2. Dimensions after Storage, and Extreme Performance Conditions
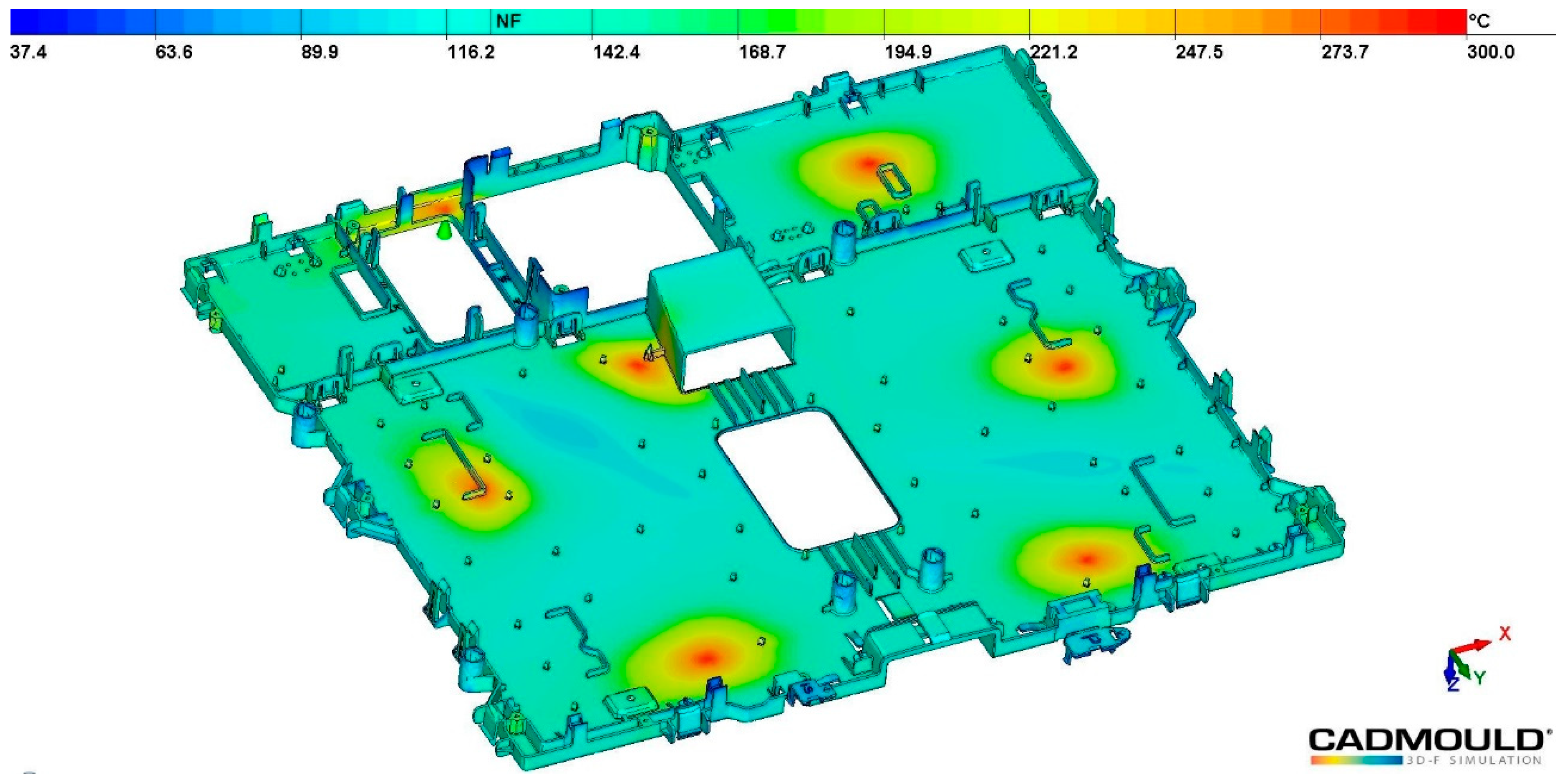
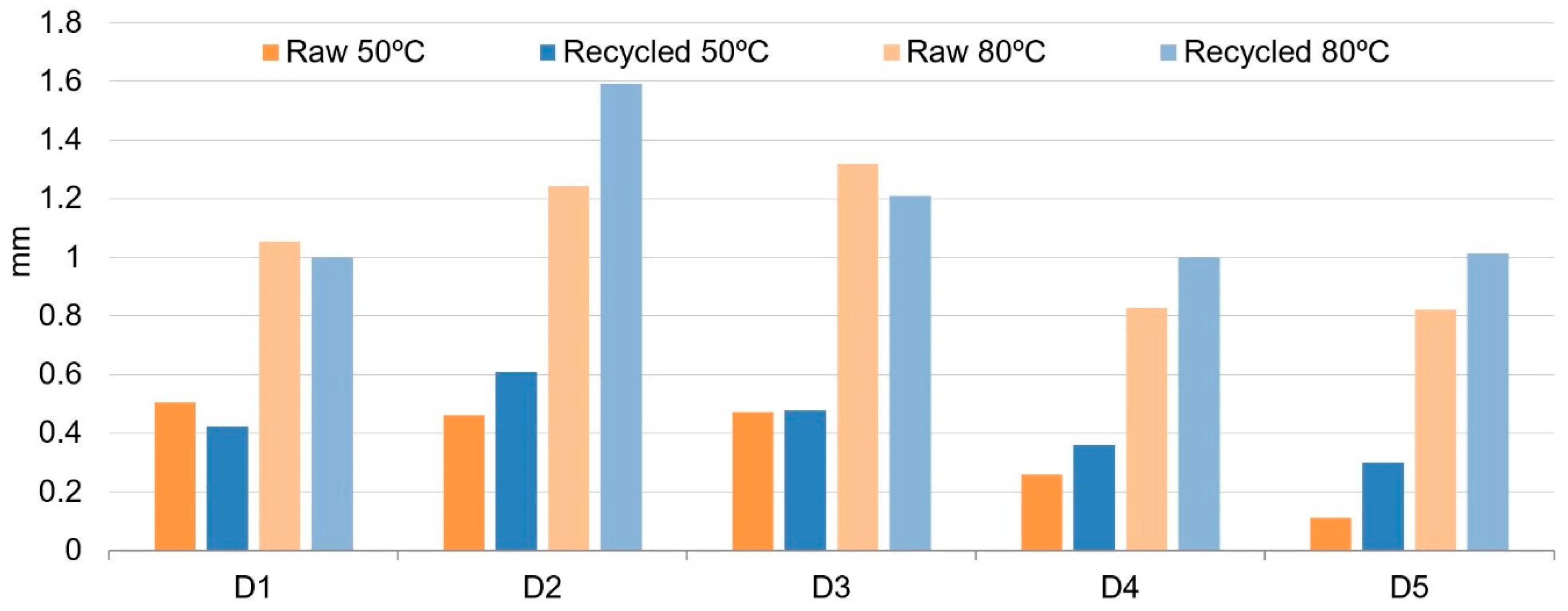
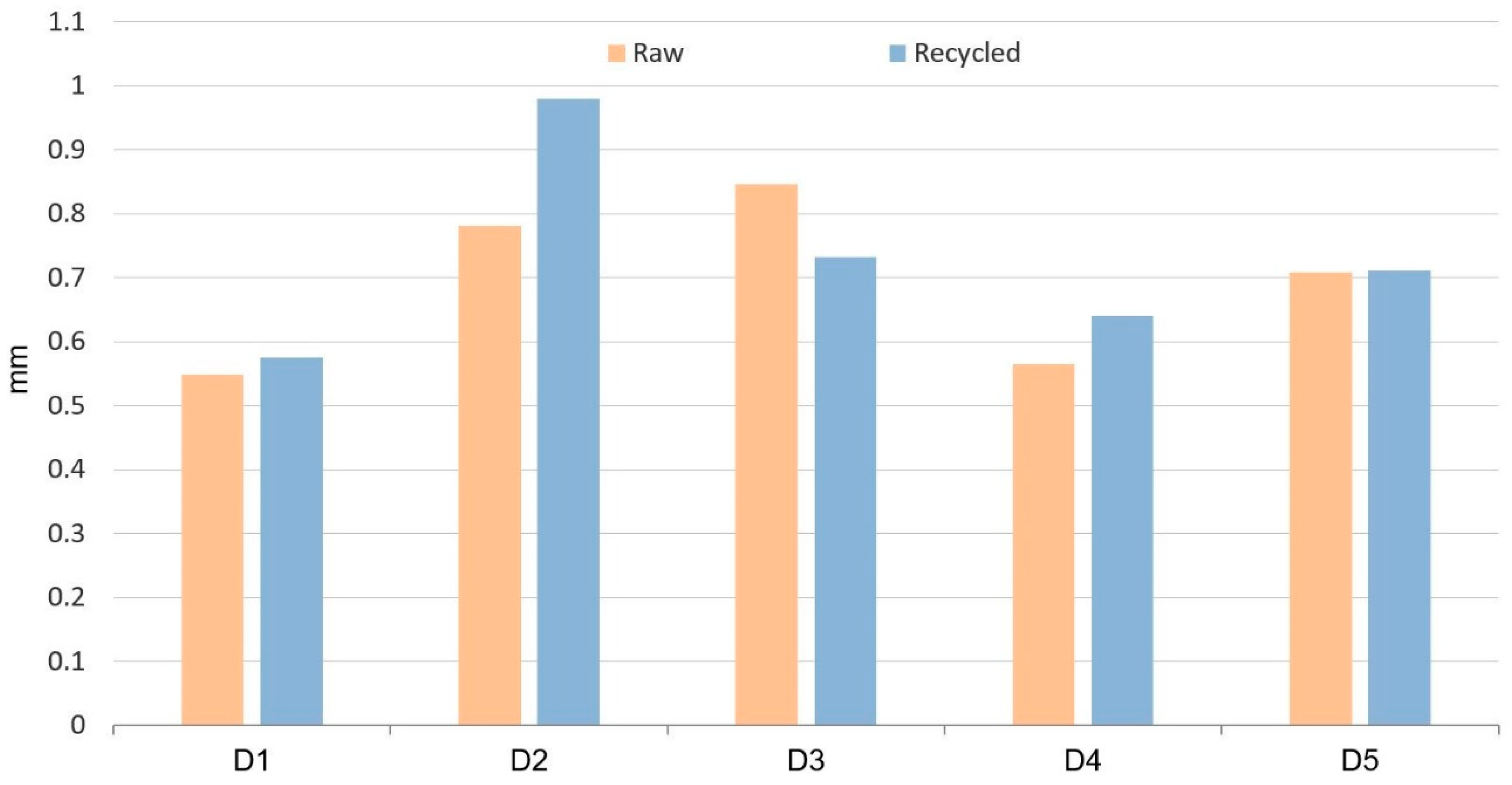
3.3. Dimensional Behavior after Heating
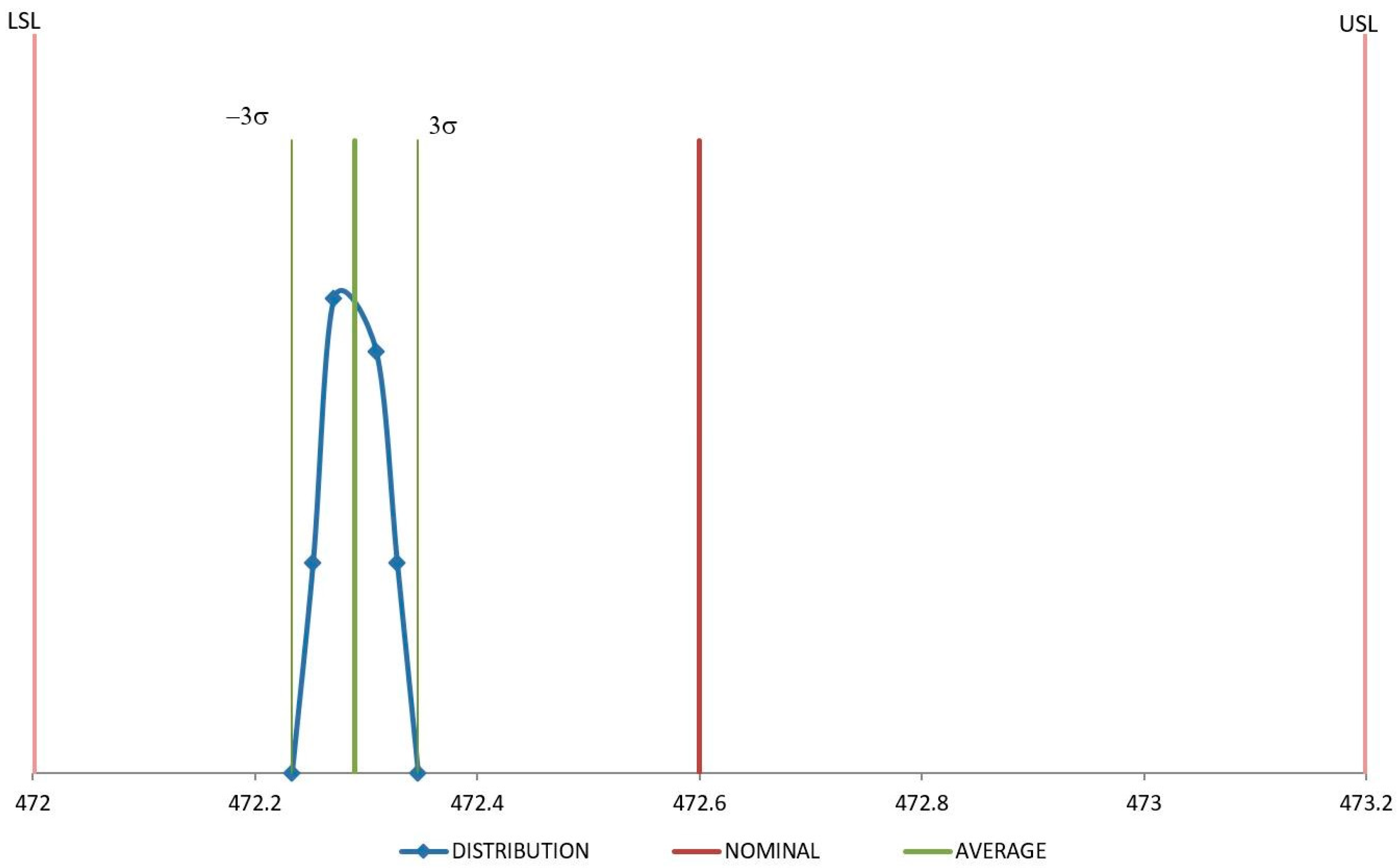
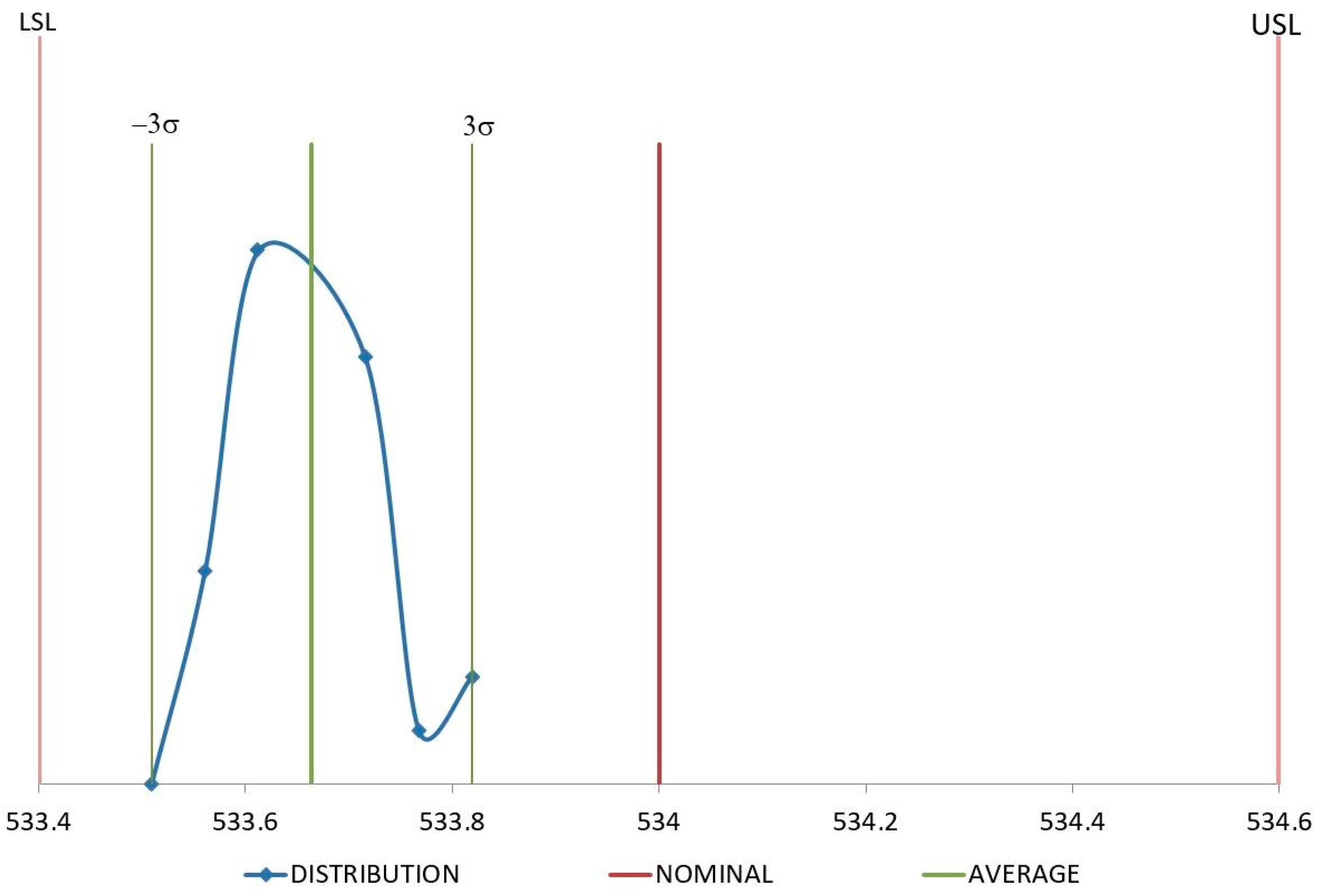
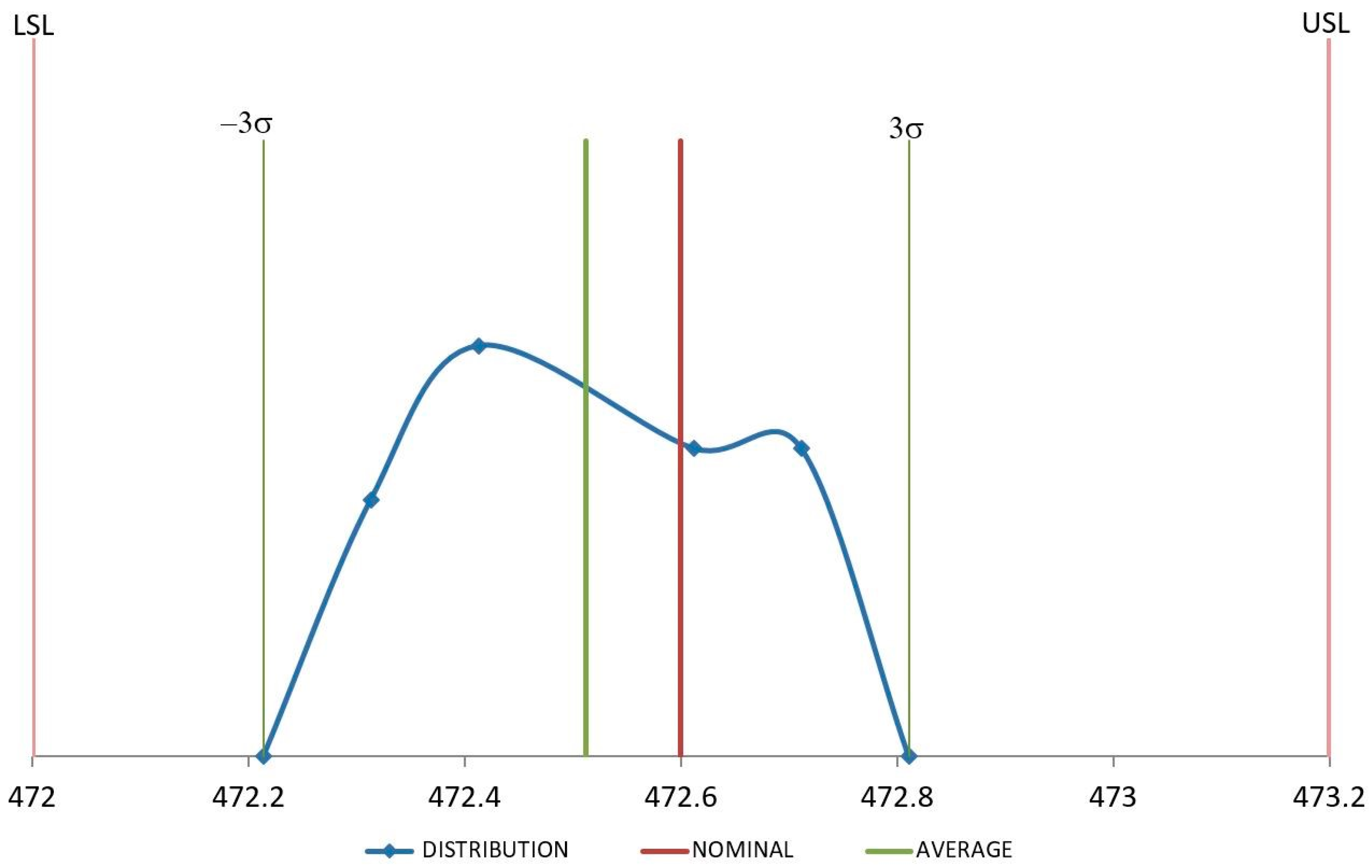
3.4. Process Capability Parameters (Cp and Cpk)
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Genis, A.V. Analysis of the Global and Russian Markets of Polypropylene and of Its Main Consumption Areas. Russ. J. Gen. Chem. 2017, 87, 2137–2150. [Google Scholar] [CrossRef]
- Hottle, T.A.; Bilec, M.M.; Landis, A.E. Biopolymer production and end of life comparisons using life cycle assessment. Resour. Conserv. Recycl. 2017, 122, 295–306. [Google Scholar] [CrossRef] [Green Version]
- A European Strategy for Plastics in a Circular Economy. European Commission Environment. Available online: https://eur-lex.europa.eu/resource.html?uri=cellar:2df5d1d2-fac7-11e7-b8f5-01aa75ed71a1.0001.02/DOC_1&format=PDF (accessed on 29 November 2018).
- A European Strategy for Plastics in a Circular Economy (Annexes). European Commission Environment. Available online: https://eur-lex.europa.eu/resource.html?uri=cellar:2df5d1d2-fac7-11e7-b8f5-01aa75ed71a1.0001.02/DOC_2&format=PDF (accessed on 29 November 2018).
- Trica, C.L.; Banacu, C.S.; Busu, M. Environmental Factors and Sustainability of the Circular Economy Model at the European Union Level. Sustainability 2019, 11, 1114. [Google Scholar] [CrossRef]
- De Mattos, C.A.; De Albuquerque, T.L.M. Enabling Factors and Strategies for the Transition Toward a Circular Economy (CE). Sustainability 2018, 10, 4628. [Google Scholar] [CrossRef]
- Cordova-Pizarro, D.; Aguilar-Barajas, I.; Romero, D.; Rodriguez, C.A. Circular Economy in the Electronic Products Sector: Material Flow Analysis and Economic Impact of Cellphone E-Waste in Mexico. Sustainability 2019, 11, 1361. [Google Scholar] [CrossRef]
- Poponi, S.; Colantoni, A.; Cividino, S.R.; Mosconi, E.M. The Stakeholders’ Perspective within the B Corp Certification for a Circular Approach. Sustainability 2019, 11, 1584. [Google Scholar] [CrossRef]
- Brown, P.; Bocken, N.; Balkenende, R. Why Do Companies Pursue Collaborative Circular Oriented Innovation? Sustainability 2019, 11, 635. [Google Scholar] [CrossRef]
- Woern, A.L.; Byard, D.J.; Oakley, R.B.; Fiedler, M.J.; Snabes, S.L.; Pearce, J.M. Fused Particle Fabrication 3-D Printing: Recycled Materials’ Optimization and Mechanical Properties. Materials 2018, 11, 1413. [Google Scholar] [CrossRef] [PubMed]
- Simões, C.; Costa Pinto, L.; Bernardo, C. Environmental and economic assessment of a road-safety product made with virgin and recycled HDPE: A comparative study. J. Environ. Manag. 2013, 114, 209–215. [Google Scholar] [CrossRef]
- Rajendran, S.; Scelsi, L.; Hodzic, A.; Soutis, C.; Al-Maadeed, M. Environmental impact assessment of composites containing recycled plastics. Resour. Conserv. Recycl. 2012, 60, 131–139. [Google Scholar] [CrossRef]
- Noda, R.; Komatsu, M.; Sumi, E.; Kasakura, T. Evaluation of material recycling for plastics: Environmental aspects. J. Mater. Cycles Waste Manag. 2001, 3, 118–125. [Google Scholar] [CrossRef]
- Vo, P.P.; Doan, H.N.; Kinashi, K.; Sakai, W.; Tsutsumi, N.; Huynh, D.P. Centrifugally Spun Recycled PET: Processing and Characterization. Polymers 2018, 10, 680. [Google Scholar] [CrossRef]
- Mai, V.-D.; Shin, S.-R.; Lee, D.-S.; Kang, I. Thermal Healing, Reshaping and Eco-friendly Recycling of Epoxy Resin Crosslinked with Schiff Base of Vanillin and Hexane-1,6-Diamine. Polymers 2019, 11, 293. [Google Scholar] [CrossRef] [PubMed]
- Galve, J.E.; Elduque, D.; Pina, C.; Javierre, C. Sustainable Supply Chain Management: The Influence of Disposal Scenarios on the Environmental Impact of a 2400 L Waste Container. Sustainability 2016, 8, 564. [Google Scholar] [CrossRef]
- Yazdanbakhsh, A.; Bank, L.C. A Critical Review of Research on Reuse of Mechanically Recycled FRP Production and End-of-Life Waste for Construction. Polymers 2014, 6, 1810–1826. [Google Scholar] [CrossRef] [Green Version]
- Devasahayam, S.; Raman, R.K.S.; Chennakesavulu, K.; Bhattacharya, S. Plastics—Villain or Hero? Polymers and Recycled Polymers in Mineral and Metallurgical Processing—A Review. Materials 2019, 12, 655. [Google Scholar] [CrossRef]
- Achilias, D.S.; Roupakias, C.; Megalokomos, P.; Lappas, A.A.; Antonakou, E.V. Chemical recycling of plastic wastes made from polyethylene (LDPE and HDPE) and polypropylene (PP). J. Hazard. Mater. 2007, 149, 536–542. [Google Scholar] [CrossRef]
- Aurrekoetxea, J.; Sarrionandia, M.A.; Urrutibeascoa, I.; Maspoch, M.L. Effects of recycling on the microstructure and the mechanical properties of isotactic polypropylene. J. Mater. Sci. 2001, 36, 2607–2613. [Google Scholar] [CrossRef]
- Kwon, D.E.; Park, B.K.; Lee, Y.-W. Solid-State Foaming of Acrylonitrile-Butadiene-Styrene/Recycled Polyethylene Terephthalate Using Carbon Dioxide as a Blowing Agent. Polymers 2019, 11, 291. [Google Scholar] [CrossRef]
- Dal Lago, E.; Boaretti, C.; Piovesan, F.; Roso, M.; Lorenzetti, A.; Modesti, M. The Effect of Different Compatibilizers on the Properties of a Post-Industrial PC/PET Blend. Materials 2019, 12, 49. [Google Scholar] [CrossRef]
- Samper, M.D.; Bertomeu, D.; Arrieta, M.P.; Ferri, J.M.; López-Martínez, J. Interference of Biodegradable Plastics in the Polypropylene Recycling Process. Materials 2018, 11, 1886. [Google Scholar] [CrossRef] [PubMed]
- Javierre, C.; Clavería, I.; Ponz, L.; Aísa, J.; Fernández, A. Influence of the recycled material percentage on the rheological behaviour of HDPE for injection moulding process. Waste Manag. 2007, 27, 656–663. [Google Scholar] [CrossRef]
- Fernandez, A.; Muniesa, M.; Javierre, C. In-line rheological testing of thermoplastics and monitored device for an injection moulding machine: Application to a raw and recycled polypropylene. Polym. Test. 2014, 33, 107–115. [Google Scholar] [CrossRef]
- Zheng, R.; Tanner, I.R.; Fan, X. Shrinkage and warpage. In Injection Molding: Integration of Theory and Modeling Methods; Springer: Berlin/Heidelberg, Germany, 2011; pp. 87–104. [Google Scholar]
- Wang, X.; Li, H.; Gu, J.; Li, Z.; Ruan, S.; Shen, C.; Wang, M. Pressure Analysis of Dynamic Injection Molding and Process Parameter Optimization for Reducing Warpage of Injection Molded Products. Polymers 2017, 9, 85. [Google Scholar] [CrossRef] [PubMed]
- Liao, S.J.; Chang, D.Y.; Chen, H.J.; Tsou, L.S.; Ho, J.R.; Yau, H.T.; Hsieh, W.H.; Wang, J.T.; Su, Y.C. Optimal process conditions of shrinkage and warpage of thin-wall parts. Polym. Eng. Sci. 2004, 44, 917–928. [Google Scholar] [CrossRef]
- Gandhi, U.; Song, Y.Y.; Mandapati, R. Semiempirical approach to predict shrinkage and warpage of fiber-reinforced polymers using measured material properties in finite element model. J. Thermoplast. Compos. Mater. 2017, 30, 1303–1319. [Google Scholar] [CrossRef]
- Jiang, Q.; Liu, H.; Xiao, Q.; Chou, S.; Xiong, A.; Nie, N. Three-dimensional numerical simulation of total warpage deformation for short-glass-fiber-reinforced polypropylene composite injection-molded parts using coupled FEM. J. Polym. Eng. 2017, 38, 493–502. [Google Scholar] [CrossRef]
- Barghash, M.A.; Alkaabneh, F.A. Shrinkage and Warpage Detailed Analysis and Optimization for the Injection Molding Process Using Multistage Experimental Design. Qual. Eng. 2014, 26, 319–334. [Google Scholar] [CrossRef]
- Khanjanzadeh, H.; Tabarsa, T.; Shakeri, A. Morphology, dimensional stability and mechanical properties of polypropylene–wood flour composites with and without nanoclay. J. Reinf. Plast. Compos. 2017, 31, 341–350. [Google Scholar] [CrossRef]
- De Carvalho Fernandes, A.; Fagali De Souza, A.; Leite Howarth, J.L. Mechanical and dimensional characterisation of polypropylene injection moulded parts in epoxy resin/aluminium inserts for rapid tooling. Int. J. Mater. Prod. Technol. 2016, 52, 37–52. [Google Scholar] [CrossRef]
- Kusumoto, N.; Takata, K.; Kurimoto, Y. Mechanical Properties and Dimensional Stabilities of Wood-Polypropylene Composites Prepared Using Mechanochemically Acetylated Japanese Cedar (Cryptomeria japonica) Wood Meal. BioResources 2016, 11, 3825–3839. [Google Scholar] [CrossRef]
- Li, S.; Zhao, G.; Wang, J. A method to improve dimensional accuracy and mechanical properties of injection molded polypropylene parts. J. Polym. Eng. 2017, 37, 323–334. [Google Scholar] [CrossRef]
- Castany, F.J.; Serraller, F.; Clavería, I.; Javierre, C. Methodology in gas assisted injection Molding. J. Mater. Process. Technol. 2003, 143–144, 214–218. [Google Scholar] [CrossRef]
- Nian, S.C.; Wu, C.Y.; Huang, M.S. Warpage control of thin-walled injection molding using local mold temperatures. Int. Commun. Heat Mass Transf. 2015, 61, 102–110. [Google Scholar] [CrossRef]
- Bergeret, A.; Pires, I.; Foulc, M.P.; Abadie, B.; Ferry, L.; Crespy, A. The hygrothermal behavior of glass-fibre-reinforced thermoplastic composites: A prediction of the composite lifetime. Polym. Test. 2001, 20, 753–763. [Google Scholar] [CrossRef]
- Ozcelik, B.; Erzurumlu, T. Comparison of the warpage optimization in the plastic injection moulding using ANOVA, neural network model and genetic algorithm. J. Mater. Process. Technol. 2006, 171, 437–445. [Google Scholar] [CrossRef]
- Mehat, N.M.; Kammarudin, S. Optimization of mechanical properties of recycled plastic products via optimal processing parameters using the Taguchi method. J. Mater. Process. Technol. 2011, 211, 1989–1994. [Google Scholar] [CrossRef]
- Kotz, S.; Johnson, N.L. Process Capability Indices, 1st ed.; Chapman and Hall/CRC: London, UK, 1993; pp. 43–74. [Google Scholar]
- Patel, S. The Tactical Guide to Six Sigma Implementation, 1st ed.; CRC Press: Boca Raton, FL, USA, 2016. [Google Scholar]
- Negri Bossi Products. Available online: https://www.negribossi.com/wp-content/uploads/VECTOR-depliant_Negri-Bossi_2016-10-3-1.pdf (accessed on 15 January 2019).
- Claveria, I.; Elduque, D.; Santolaria, J.; Pina, C.; Javierre, C.; Fernández, A. The influence of environmental conditions on the dimensional stability of components injected with PA6 and PA66. Polym. Test. 2016, 50, 15. [Google Scholar] [CrossRef]
- Zeiss Coordinate Measuring Machines. Available online: https://www.zeiss.com/metrology/products/systems/coordinate-measuring-machines.html (accessed on 15 January 2019).
- Zeiss Tactile Scanning Probes. Available online: https://www.zeiss.com/metrology/products/sensors/on-cmm/tactile-scanning-probe.html (accessed on 15 January 2019).
- Zeiss Calypso—Measuring Software for Geometry. Available online: https://www.zeiss.com/metrology/products/software/calypso-overview/calypso.html (accessed on 15 January 2019).
- Rogueda-Berriet, C.; Bahlouli, N.; Pessey, D.; Rémond, Y. Mechanical Behavior of Recycled Polypropylene Composites Under Tensile, Bending, and Creep Loading: Experimental and Modeling. J. Eng. Mater. Technol. 2011, 133, 030907. [Google Scholar] [CrossRef]
- Incarnato, L.; Scarfato, P.; Acierno, D. Rheological and mechanical properties of recycled polypropylene. Pol. Eng. Sci. 1999, 39, 749–755. [Google Scholar] [CrossRef]
- Katmer, S.; Esen, H.; Kataras, C. Residual stresses in injection molded shape memory polymer parts. In Proceedings of the 31st International Conference of the Polymer Processing Society, Jeju Island, Korea, 7–11 June 2015; AIP Publishing LLC: Melville, NY, USA, 2016. [Google Scholar]
- Kallel, A.; Lamraoui, M.; Fitoussi, J.; Tcharkhtchi, A. The Residual Stress Effect on the Shape Memory Polymers. Mater. Res. Proc. 2016, 2, 151–156. [Google Scholar] [CrossRef] [Green Version]
- Altan, M.; Yurci, M.E.; Nugay, M. Residual stresses determination in injection molded virgin and recycled HDPE blends: Mechanical properties and morphology. e-Polymers 2008, 8, 170. [Google Scholar] [CrossRef]
- Valenza, A.; La Mantia, F.P. Recycling of polymer waste: Part II—Stress degraded polypropylene. Polym. Degrad. Stab. 1988, 20, 63–73. [Google Scholar] [CrossRef]
- González-González, V.A.; Neira-Velázquez, G.; Angulo-Sánchez, J.L. Polypropylene chain scissions and molecular weight changes in multiple extrusion. Polym. Degrad. Stab. 1998, 60, 33–42. [Google Scholar] [CrossRef]
- Sun, X.; Su, X.; Tibberham, P.; Mao, J.; Tao, J. The application of modified PVT data on the warpage prediction of injection molded part. J. Pol. Res. 2016, 23, 86. [Google Scholar] [CrossRef]

| Raw | Recycled | |
|---|---|---|
| Density (g/cm3) | 1.22 | 1.25 |
| Vicat B °C 10 N (50 °C/h) | 90 | 94 |
| HDT °C 1.82 MN/m² ISO 75 242 °C | 70 | 74 |
| Molding shrinkage % ISO 294-4 | 1.35 | 1.15 |
| Ejection temperature (°C) | 110 | 118 |
| Raw Material | |||||
| D1 | D2 | D3 | D4 | D5 | |
| Avg M0 | 533.29 | 533.25 | 533.57 | 472.05 | 472.32 |
| Avg ∆M0 | 0.72 | 0.74 | 0.42 | 0.54 | 0.27 |
| σ | 0.25 | 0.05 | 0.10 | 0.07 | 0.26 |
| Recycled Material | |||||
| D1 | D2 | D3 | D4 | D5 | |
| Avg M0 | 532.92 | 533.03 | 533.36 | 471.97 | 472.04 |
| Avg ∆M0 | 1.07 | 0.96 | 0.63 | 0.62 | 0.55 |
| σ | 0.06 | 0.06 | 0.05 | 0.09 | 0.07 |
| Raw Material | |||||
| ∆T | D1 | D2 | D3 | D4 | D5 |
| 50–80 °C | 0.64 | 0.78 | 1.41 | 0.56 | 0.7 |
| 20–50 °C | 0.4 | 0.46 | 0.47 | 0.24 | 0.11 |
| Recycled Material | |||||
| 50–80 °C | 0.57 | 0.98 | 0.73 | 0.64 | 0.71 |
| 20–50 °C | 0.42 | 0.60 | 0.47 | 0.35 | 0.29 |
| Raw Material | Recycled Material | |||
|---|---|---|---|---|
| D1 | D4 | D1 | D4 | |
| Cp | 5.83 | 10.55 | 3.873 | 2.008 |
| Cpk | 2.35 | 5.09 | 1.704 | 1.715 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Galve, J.E.; Elduque, D.; Pina, C.; Clavería, I.; Acero, R.; Fernández, Á.; Javierre, C. Dimensional Stability and Process Capability of an Industrial Component Injected with Recycled Polypropylene. Polymers 2019, 11, 1063. https://doi.org/10.3390/polym11061063
Galve JE, Elduque D, Pina C, Clavería I, Acero R, Fernández Á, Javierre C. Dimensional Stability and Process Capability of an Industrial Component Injected with Recycled Polypropylene. Polymers. 2019; 11(6):1063. https://doi.org/10.3390/polym11061063
Chicago/Turabian StyleGalve, José Eduardo, Daniel Elduque, Carmelo Pina, Isabel Clavería, Raquel Acero, Ángel Fernández, and Carlos Javierre. 2019. "Dimensional Stability and Process Capability of an Industrial Component Injected with Recycled Polypropylene" Polymers 11, no. 6: 1063. https://doi.org/10.3390/polym11061063





