Hyperelastic Properties of Platinum Cured Silicones and its Applications in Active Compression
Abstract
1. Introduction
2. Materials and Methods
2.1. Material Properties and Characterisation
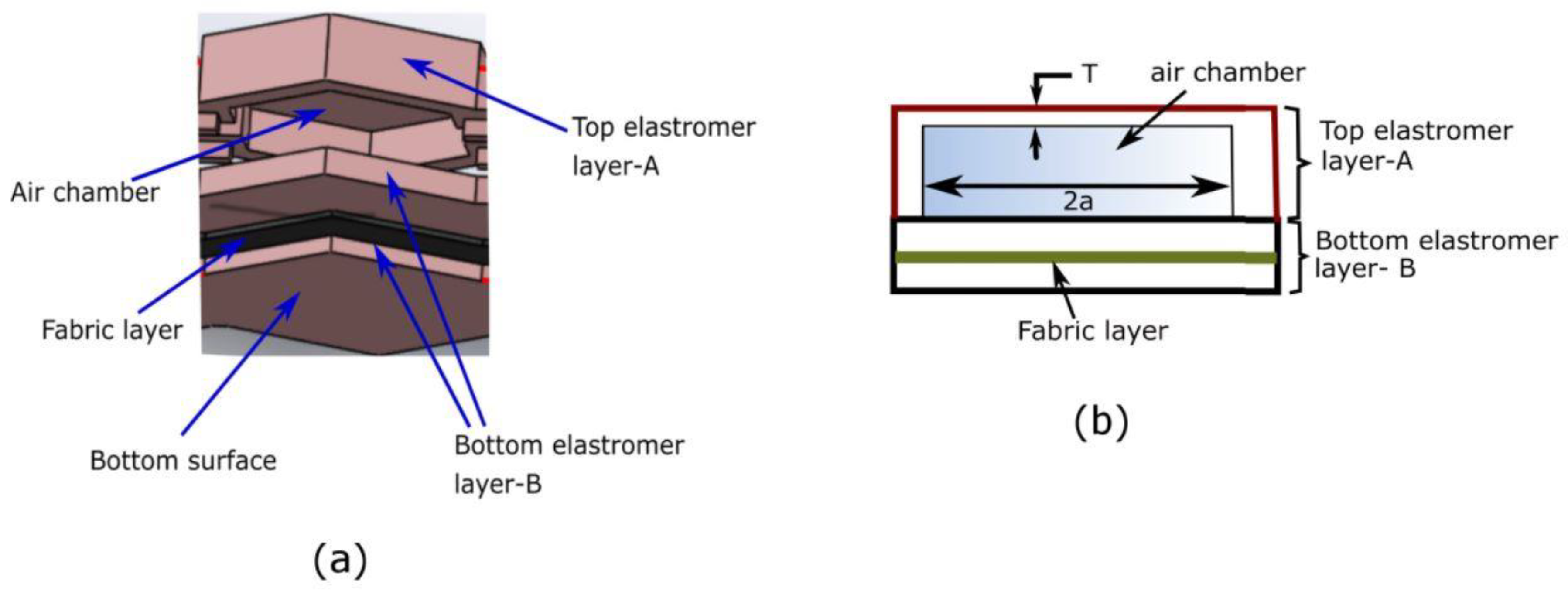
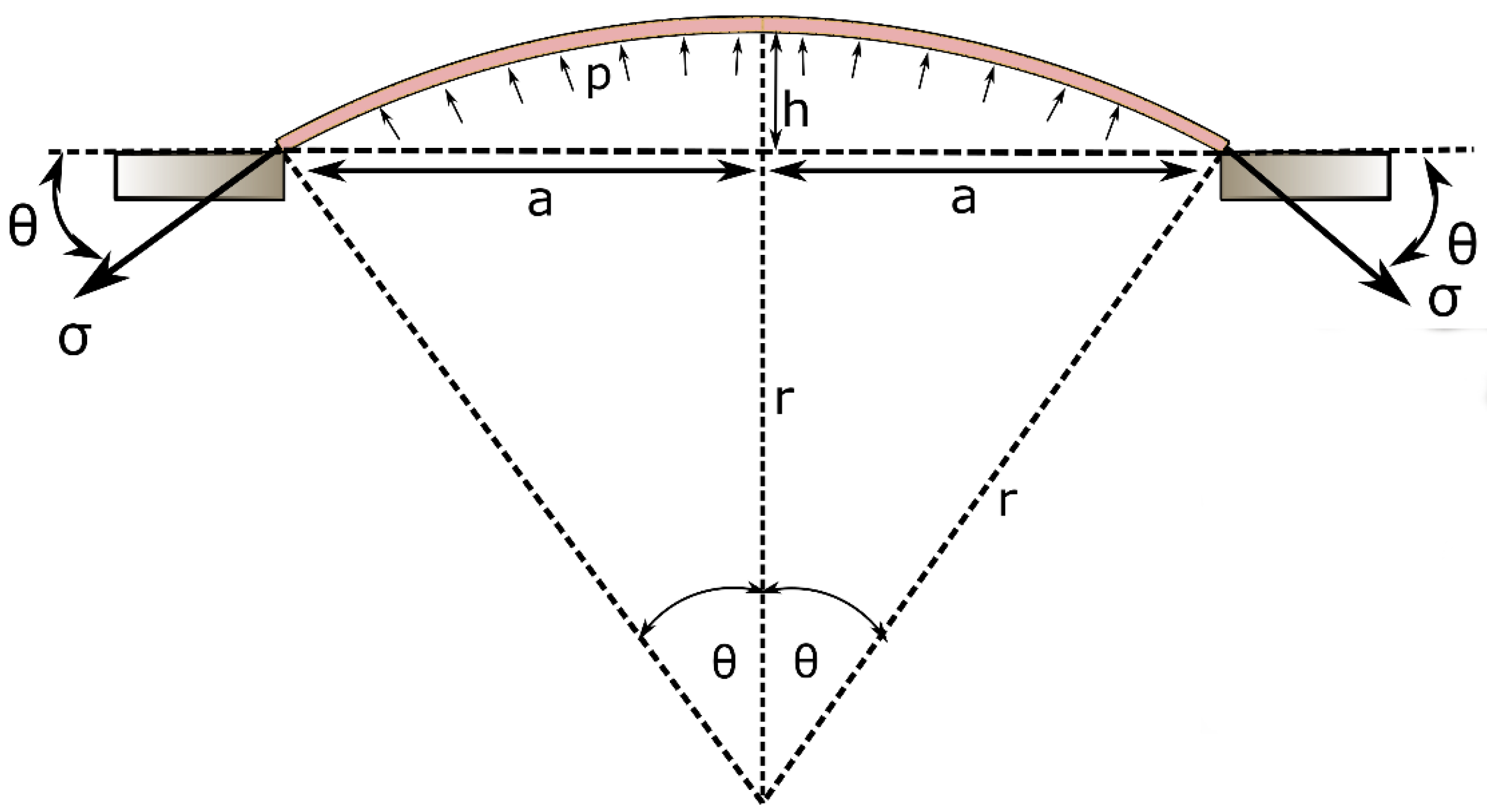
2.2. Mini-Bladder Design and Geometrical Analysis
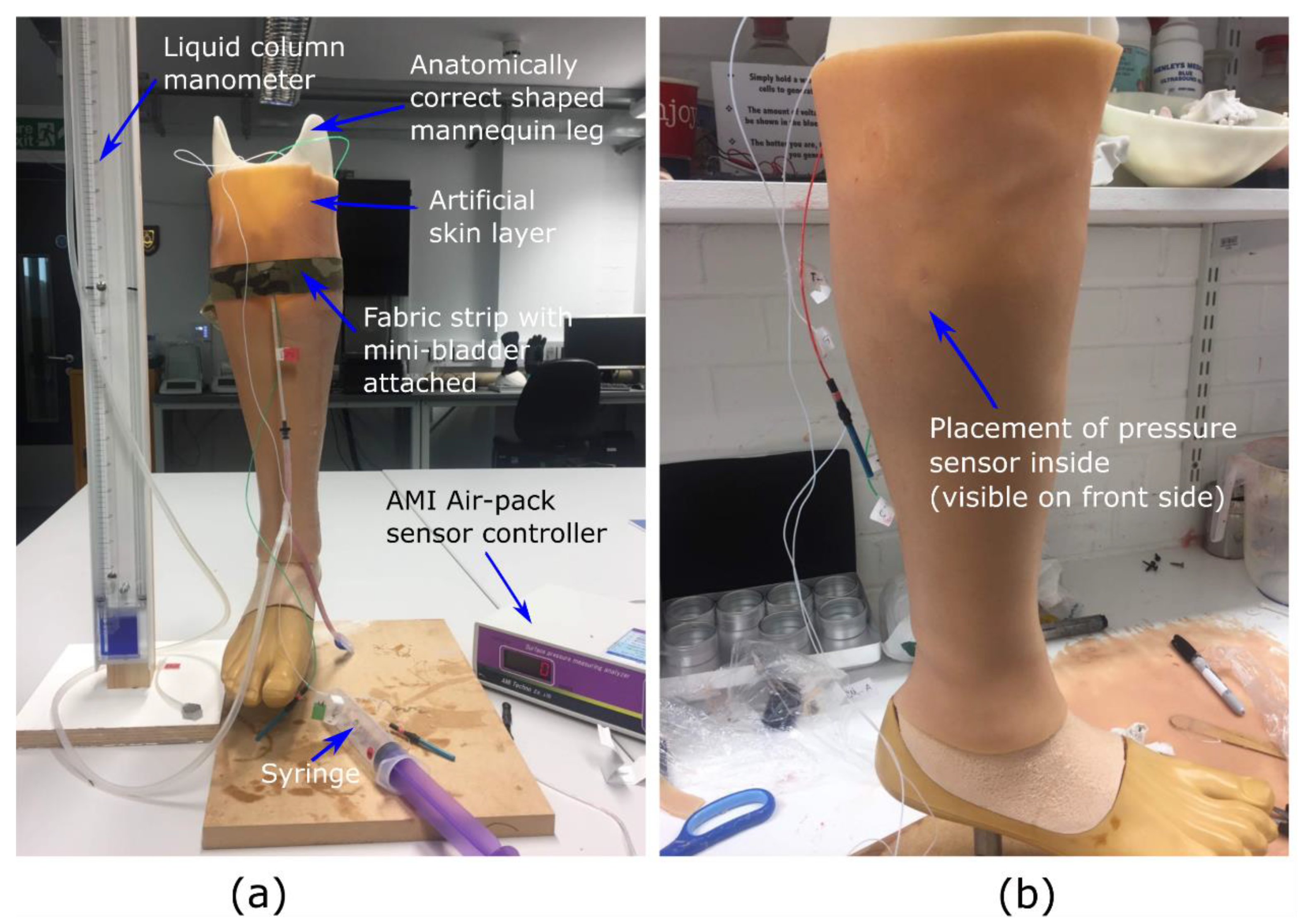
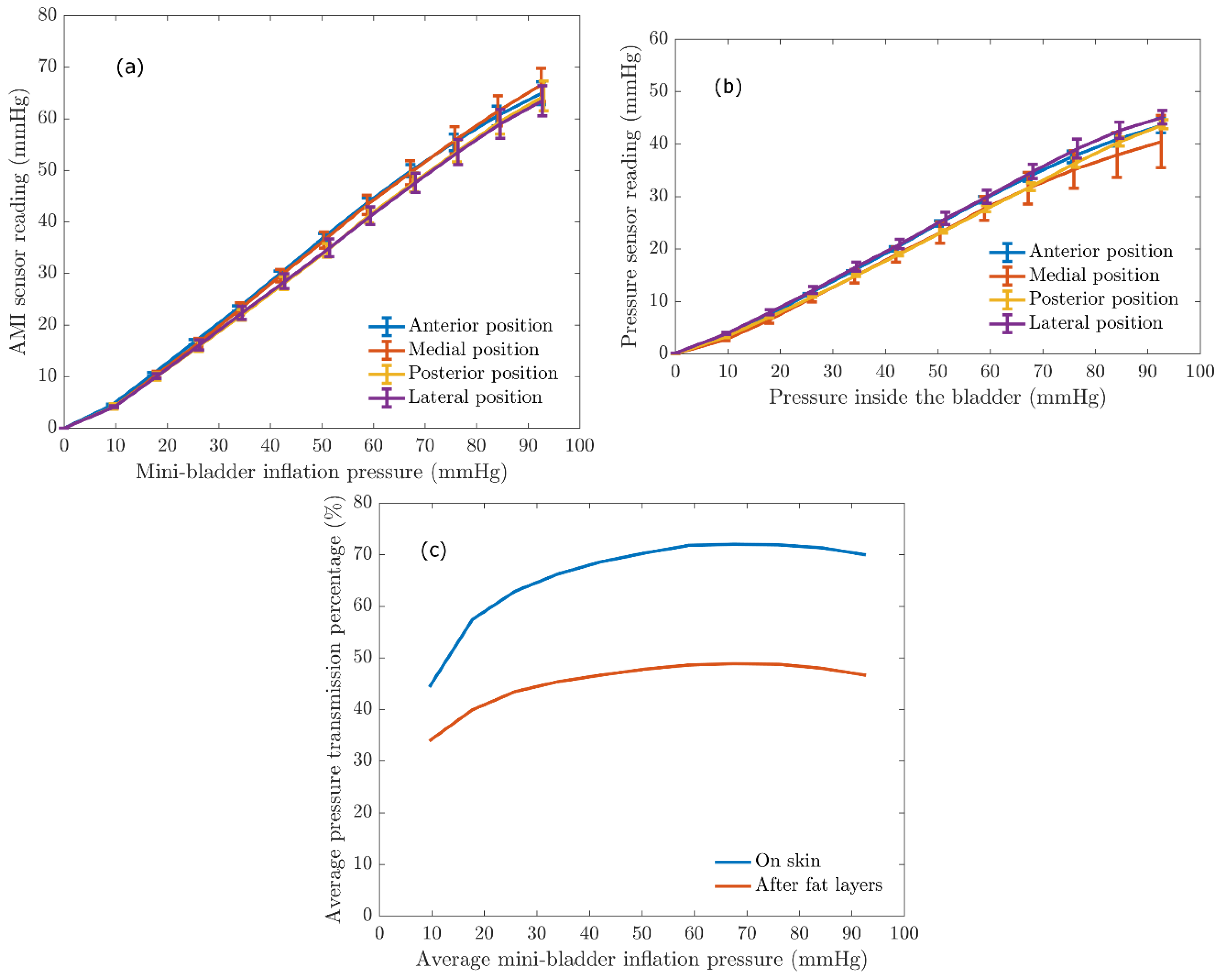
2.3. Pressure Transmission of Mini-Bladders on a Biofidelic Leg Surrogate
2.4. Characterisation of 3D Deformation Profile of Mini-Bladders
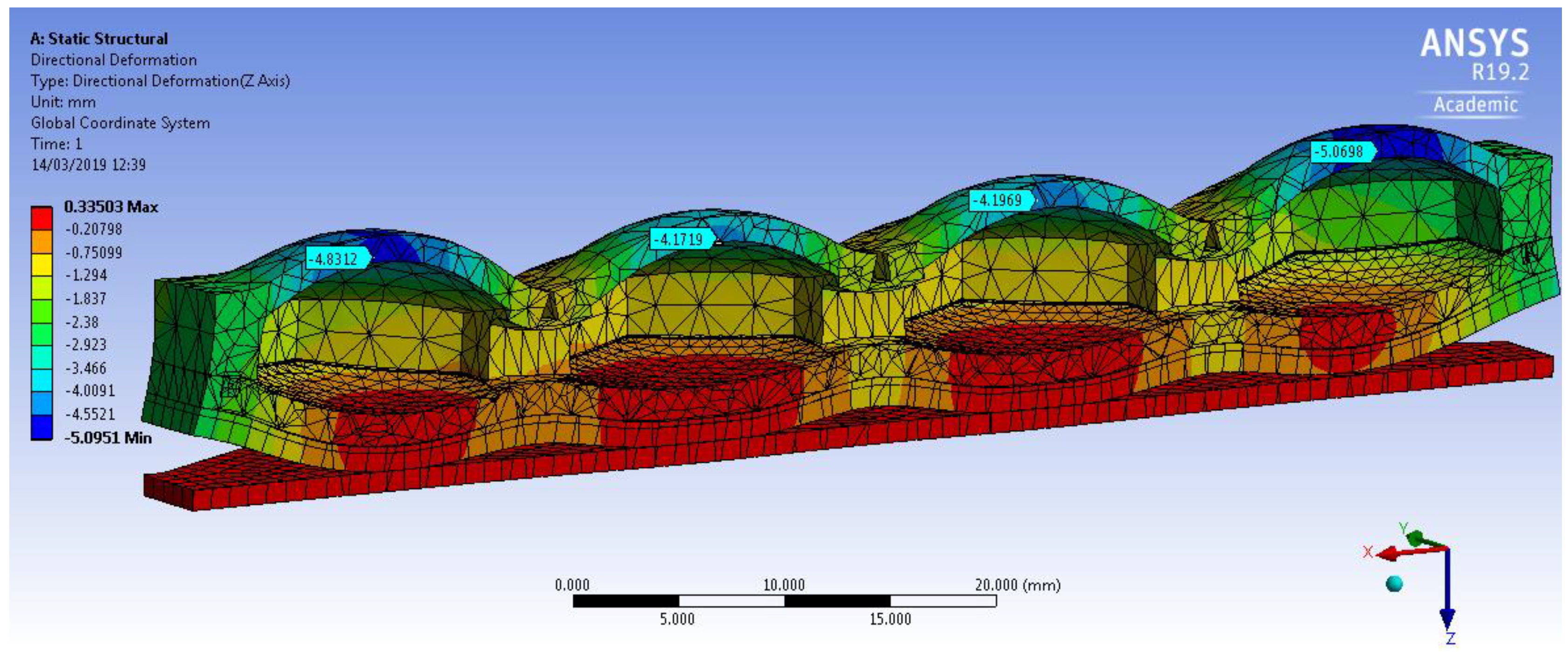
2.5. FEA Analysis of 3D Deformation Profile of Mini-Bladders
3. Results and Discussion
3.1. Model Parameters for Hyperelastic Equations
3.2. 3-D Deformation Profile Results of the Mini-Bladders
3.3. Interface Pressure Transmission Results
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Eklof, B.; Perrin, M.; Delis, K.T.; Rutherford, R.B.; Gloviczki, P. Updated terminology of chronic venous disorders: The VEIN-TERM transatlantic interdisciplinary consensus document. J. Vasc. Surg. 2009, 49, 498–501. [Google Scholar] [CrossRef]
- Langer, R.D.; Ho, E.; Denenberg, J.O.; Fronek, A.; Allison, M.; Criqui, M.H. Relationships between symptoms and venous disease: The San Diego population study. Arch. Intern. Med. 2005, 165, 1420–1424. [Google Scholar] [CrossRef] [PubMed]
- Eberhardt, R.T.; Raffetto, J.D. Chronic venous insufficiency. Circulation 2005, 111, 2398–2409. [Google Scholar] [CrossRef] [PubMed]
- Bergan, J.J.; Schmid-Schönbein, J.W.; Smith, P.D.; Nicolaides, A.N.; Boisseau, M.R.; Eklof, B. Chronic Venous Disease. N. Engl. J. Med. 2006, 355, 488–498. [Google Scholar] [CrossRef] [PubMed]
- Eklof, B. Classifying venous dissorder. In The Vein Book, 1st ed.; Bergan, J.J., Ed.; Elsevier Academic Press: San Diego, CA, USA, 2007; pp. 111–117. [Google Scholar]
- Fan, C. Venous Pathophysiology. Semin. Intervent. Radiol. 2005, 22, 157–161. [Google Scholar] [CrossRef] [PubMed]
- Fletcher, J.; Moffatt, C.; Partsch, H.; Vowden, K.; Vowden, P. Principles of compression in venous disease: A practitioner’s guide to treatment and prevention of venous leg ulcers. Wounds Int. 2013, 1–21. [Google Scholar]
- Xiong, Y.; Xiaoming, T. Compression garments for medical therapy and sports. Polymers 2018, 10, 663. [Google Scholar] [CrossRef]
- Callam, M.J. Epidemiology of varicose veins. Br. J. Surg. 1994, 81, 167–173. [Google Scholar] [CrossRef]
- Kaplan, R.; Criqui, M.; Denenberg, J.; Bergan, J.; Fronek, A. Quality of life in patients with chronic venous disease: San Diego population study. J. Vasc. Surg. 2003, 37, 1047–1053. [Google Scholar] [CrossRef]
- Kurz, X.; Lamping, D.; Kahn, S.; Baccaglini, U.; Zuccarelli, F.; Spreafico, G. Do varicose veins affect quality of life? Results of an international population-based study. J. Vasc. Surg. 2001, 34, 641–648. [Google Scholar] [CrossRef]
- Evans, C.; Fowkes, F.; Hajivassiliou, C.; Harper, D.; Ruckley, C. Epidemiology of varicose veins. A review. Int. Angiol. 1994, 13, 263–270. [Google Scholar] [PubMed]
- Beebe-Dimmer, J.L.; Pfeifer, J.R.; Engle, J.S.; Schottenfeld, D. The epidemiology of chronic venous insufficiency and varicose veins. Ann. Epidemiol. 2005, 15, 175–184. [Google Scholar] [CrossRef] [PubMed]
- Ruckley, C.V.; Evans, C.J.; Allan, P.L.; Lee, A.J.; Fowkes, F.G. Chronic venous insufficiency: Clinical and duplex correlations. The Edinburgh Vein Study of venous disorders in the general population. J. Vasc. Surg. 2002, 36, 520–525. [Google Scholar] [CrossRef] [PubMed]
- Piazza, G. Varicose veins. Circulation 2014, 130, 582–587. [Google Scholar] [CrossRef] [PubMed]
- Borges, E.L.; Ferraz, A.F.; Carvalho, D.V.; Matos, S.S.D.; Lima, V.L.D.A.N. Prevention of varicose ulcer relapse: A cohort study. Acta Paul. Enferm. 2016, 29, 9–16. [Google Scholar] [CrossRef]
- O’Meara, S.; Tierney, J.; Cullum, N.; Bland, M.J.; Franks, P.J.; Mole, T.; Scriven, M. Four layer bandage compared with short stretch bandage for venous leg ulcers: Systematic review and meta-analysis of randomised controlled trials with data from individual patients. Br. Med. J. 2009, 338, b1344. [Google Scholar] [CrossRef] [PubMed]
- Felty, C.L.; Rooke, T.W. Compression therapy for chronic venous insufficiency. Semin. Vasc. Surg. 2005, 18, 36–40. [Google Scholar] [CrossRef]
- O’Meara, S.; Cullum, N.A.; Nelson, E.A. Compression for venous leg ulcers. Cochrane Database Syst. Rev. 2009, 1. [Google Scholar] [CrossRef]
- Mosti, G.; Iabichella, M.L.; Partsch, H. Compression therapy in mixed ulcers increases venous output and arterial perfusion. J. Vasc. Surg. 2012, 55, 122–128. [Google Scholar] [CrossRef]
- Flour, M.; Clark, M.; Partsch, H. Dogmas and controversies in compression therapy: Report of an International Compression Club (ICC) meeting, Brussels, May 2011. Int. Wound J. 2013, 10, 516–526. [Google Scholar] [CrossRef]
- Mosti, G. Compression in leg ulcer treatment: Inelastic compression. Phlebology 2014, 29 (Suppl. 1), 146–152. [Google Scholar] [CrossRef]
- Lim, C.S.; Davies, A.H. Graduated compression stockings. Can. Med. Assoc. J. 2014, 186, 391–398. [Google Scholar] [CrossRef]
- Partsch, B.; Partsch, H. Calf compression pressure required to achieve venous closure from supine to standing positions. J. Vasc. Surg. 2005, 42, 734–738. [Google Scholar] [CrossRef]
- Stemmer, R.; Marescaux, J.; Furderer, C. The compression therapy of the lower-extrimities especially with compression stockings. Dermatologist 1980, 31, 355–365. [Google Scholar]
- Narayana, H.; Hu, J.; Kumar, B.; Shang, S.; Ying, M.; Young, R.J. Designing of advanced medical stocking using stress-memory polymeric filaments for pressure control and massaging. Mater. Sci. Eng. C 2018, 91, 263–273. [Google Scholar] [CrossRef]
- Calabrese, L.; Frediani, G.; Gei, M.; de Rossi, D.; Carpi, F. Active compression bandage made of electroactive elastomers. IEEE/ASME Trans. Mechatron. 2018, 23, 2328–2337. [Google Scholar] [CrossRef]
- Yang, S.T.; Ryu, J.W.; Park, S.H.; Lee, Y.B.; Koo, S.H.; Park, Y.L.; Lee, G. An active compression sleeve with variable pressure levels using a wire fabric mechanism and a soft sensor. Smart Mater. Struct. 2019, 28, 114002. [Google Scholar] [CrossRef]
- Fernando, A. Manufacture of Engineered Compression Systems Using Latest Flat-Bed Knitting Technology. Ph.D. Thesis, The University of Manchester (Alma), Manchester, UK, 2005. [Google Scholar]
- Thomas, S. The use of Laplace equation in the calculation of sub-bandage pressure. World Wide Wounds 2003, 3, 21–23. [Google Scholar]
- Thomas, S.; Fram, P. Laboratory-based evaluation of a compression-bandaging system. Nurs. Times 2003, 99, 24–28. [Google Scholar]
- Thomas, S. Bandages and bandaging: The science behind the art. Care Sci. Pr. 1990, 8, 50–60. [Google Scholar]
- Comerota, A.J.; Arbor, A. Intermittent pneumatic compression : Physiologic and clinical basis to improve management of venous leg ulcers. J. Vasc. Surg. 2011, 53, 1121–1129. [Google Scholar] [CrossRef] [PubMed]
- McGeown, J.G.; McHale, N.G.; Thornbury, K.D. Effects of varying patterns of external compression on lymph flow in the hindimb of the anaesthetized sheep. J. Physiol. 1988, 397, 449–457. [Google Scholar] [CrossRef] [PubMed]
- Delis, K.T.; Slimani, G.; Hafez, H.M.; Nicolaides, A.N. Enhancing venous outflow in the lower limb with intermittent pneumatic compression. A comparative haemodynamic analysis on the effect of foot vs. calf vs. foot and calf compression. Eur. J. Vasc. Endovasc. Surg. 2000, 19, 250–260. [Google Scholar] [CrossRef] [PubMed]
- Raines, J.K.; O’Donnell, T.F., Jr.; Kalisher, L.; Darling, R.C. Selection of patients with lymphedema for compression therapy. Am. J. Surg. 1977, 133, 430–437. [Google Scholar] [CrossRef]
- Zelikovski, A.; Manoach, M.; Giler, C.; Urca, I. Lymphapress: A new pneumatic device for the treatment of lymphedema of the limbs. Lymphology 1980, 13, 68–73. [Google Scholar] [PubMed]
- Sands, W.A.; McNeal, J.R.; Murray, S.R.; Stone, M.H. Dynamic Compression Enhances Pressure-to-Pain Threshold in Elite Athlete Recovery: Exploratory Study. J. Strength Cond. Res. 2015, 29, 1263–1272. [Google Scholar] [CrossRef]
- Sharmin, E.; Zafar, F. Polyurethane: An introduction. In Polyurethane; InTech: Rijeka, Croatia, 2012. [Google Scholar]
- Turjanmaa, K.; Alenius, H.; Mäkinen-Kiljunen, S.; Reunala, T.; Palosuo, T. Natural-rubber-latex allergy. In Handbook of Occupational Dermatology; Springer: Berlin/Heidelberg, Germany, 2000; pp. 719–729. [Google Scholar]
- Sussman, G.L.; Beezhold, D.H. Allergy to latex rubber. Ann. Intern. Med. 1995, 122, 43–46. [Google Scholar] [CrossRef]
- Shah, T.M. Dip molding of Polyurethane and Silicone for Latex-free, nonallergenic products. Med. Device Diagn. Ind. 2001, 23, 75–79. [Google Scholar]
- Penava, Ž.; Šimić-Penava, D.; Knezic, Ž. Determination of the elastic constants of plain woven fabrics by a tensile test in various directions. Fibres Text. East. Eur. 2014, 22, 57–63. [Google Scholar]
- Kalra, A.; Lowe, A.; Al-Jumaily, A. Mechanical behaviour of skin: A review. J. Mater. Sci. Eng. 2016, 5, 254–260. [Google Scholar]
- Ali, A.; Hosseini, M.; Sahari, B.B. A Review of Constitutive Models for Rubber-Like Materials. Am. J. Eng. Appl. Sci. 2010, 3, 232–239. [Google Scholar] [CrossRef]



© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nandasiri, G.K.; Ianakiev, A.; Dias, T. Hyperelastic Properties of Platinum Cured Silicones and its Applications in Active Compression. Polymers 2020, 12, 148. https://doi.org/10.3390/polym12010148
Nandasiri GK, Ianakiev A, Dias T. Hyperelastic Properties of Platinum Cured Silicones and its Applications in Active Compression. Polymers. 2020; 12(1):148. https://doi.org/10.3390/polym12010148
Chicago/Turabian StyleNandasiri, Gayani K., Anton Ianakiev, and Tilak Dias. 2020. "Hyperelastic Properties of Platinum Cured Silicones and its Applications in Active Compression" Polymers 12, no. 1: 148. https://doi.org/10.3390/polym12010148
APA StyleNandasiri, G. K., Ianakiev, A., & Dias, T. (2020). Hyperelastic Properties of Platinum Cured Silicones and its Applications in Active Compression. Polymers, 12(1), 148. https://doi.org/10.3390/polym12010148





